Studies of Scattering, Reflectivity, and Transmitivity in WBAN Channel: Feasibility of Using UWB

Abstract

The Wireless Personal Area Network (WPAN) is one of the fledging paradigms that the next generation of wireless systems is sprouting towards. Among them, a more specific category is the Wireless Body Area Network (WBAN) used for health monitoring. On the other hand, Ultra-Wideband (UWB) comes with a number of desirable features at the physical layer for wireless communications. One big challenge in adoption of UWB in WBAN is the fact that signals get attenuated exponentially. Due to the intrinsic structural complexity in human body, electromagnetic waves show a profound variation during propagation through it. The reflection and transmission coefficients of human body are highly dependent upon the dielectric constants as well as upon the frequency. The difference in structural materials such as fat, muscles and blood essentially makes electromagnetic wave attenuation to be different along the way. Thus, a complete characterization of body channel is a challenging task. The connection between attenuation and frequency of the signal makes the investigation of UWB in WBAN an interesting proposition. In this paper, we study analytically the impact of body channels on electromagnetic signal propagation with reference to UWB. In the process, scattering, reflectivity and transmitivity have been addressed with analysis of approximate layer-wise modeling, and with numerical depictions. Pulses with Gaussian profile have been employed in our analysis. It shows that, under reasonable practical approximations, the human body channel can be modeled in layers so as to have the effects of total reflections or total transmissions in certain frequency bands. This could help decide such design issues as antenna characteristics of implant devices for WBAN employing UWB.

1. Introduction

Wireless Body Area Networks (WBANs) have attracted interest in recent years because of a number of promising applications—specifically, in the field of health monitoring. Like everyday attire, in a WBAN, several small nodes are placed directly in, on or around the human body. Since WBAN nodes acquire their power from rechargeable batteries or by energy harvesting, it is essential that they be extremely energy-efficient [1]. Above and beyond the energy efficiency, the nodes are meant to be of low complexity to keep costs down, among other things. On the other hand, Ultra-Wideband (UWB) communication is a transmission technology that comes with such promises as low-power consumption [2], interference robustness [3], high local capacity [4], and less complex hardware, most of which are highly desirable for WBANs [5]. One key concern in this regard is about signal attenuation which occurs exponentially with frequency. This leads to the need to study electromagnetic propagation across human body as medium, with consideration for UWB signal as it relates to communication system parameters for implant devices. Particularly, Impulse-Radio (IR) [6] transmission appears to be well suited to reduce complexity, since major parts of narrowband communication systems such as mixers, RF (Radio Frequency) oscillators, or Phase-Locked Loops (PLLs) can be omitted in IR systems [7]. In order to accomplish the requirements mentioned above as they relate to energy efficiency and complexity reduction, the distinct behavior of the propagation channel has to be taken into account. For WBANs, this has to do with identification of the effects of propagation on or around the body. Unlike conventional wireless channels, a human body is rather complex in structure. Electromagnetic waves show a profound variation during propagation through human body as the reflection and transmission coefficients are highly dependent upon the dielectric constants [10], in addition to the frequency. Therefore, it is a challenging task to make a complete characterization of the human body channel. Due to the differences in structural materials such as fat, muscles, blood etc., electromagnetic wave attenuation is different across the different parts of the body. The higher the frequency, the more attenuation takes place, which limits the use of high frequency or UWB in WBAN. In this manuscript, we analyze the impact of the body channel on the signals in different frequency bands. Scattering, reflectivity, and transmitivity have been studied with analysis of approximate layer-wise modeling, and with relevant numerical rendering. Pulses, having Gaussian profile, have been used in our analysis. We illustrate that the body channel can be mathematically modeled as composed of layers, with total reflection and total transmission of the signal in certain frequency bands, so as to approximate the propagation effect. The rest of this paper is organized as follows: the whole mathematical model for wave propagation in biological media, scattering, reflection, and anti-reflection by single layer is given in Section 2; Section 3 describes numerical results and concluding remarks are given in Section 4.

2. Mathematical Model

2.1. Wave Propagation through Biological Media

From the appendix A, if the incident wave is a linearly polarized uniform plane wave travelling along the z-direction, then, for E and H, Equation (A.9) is of the form:

$$\overrightarrow{E}=E_i{e}^{-\alpha z}{e}^{j(\omega t-\beta z)}{i}_x$$

(1)

$$\overrightarrow{H}=H_i{e}^{-\alpha z}{e}^{j(\omega t-\beta z)}{i}_y$$

(2)

where E_i = ηH_i

The intrinsic impedance of biological material η is given by:

$$\eta =\sqrt{\frac{\mu }{\epsilon }}\left[1-0.378{\left(\frac{{\epsilon }^{″}}{{\epsilon }^{\prime }}\right)}^2+j0.5\left(\frac{{\epsilon }^{″}}{{\epsilon }^{\prime }}\right)\right]$$

(3)

The Pointing Vector, that is, the power flowing per unit area of cross section (W/m²), gives the power density associated with an EM wave:

$${\overrightarrow{P}}_i={\overrightarrow{E}}_i\times {\overrightarrow{H}}_i$$

(4)

For a uniform plane wave, time-average power flow is given by:

$$P_i=\frac{{|E_i|}^2}{2\eta }=\frac{1}{2}\eta {|H_i|}^2$$

(5)

The permittivity and frequency may also determine how far the EM wave penetrates into the body. The term depth of penetration (D_p) usually quantifies this. For objects with homogeneous properties and with RFR incident at right angles to the surface, depth of penetration is defined as the distance at which the power density is decreased by absorption to about 0.13534 of the body’s surface value. However, the magnitude of the electric and the magnetic field reduces by a factor of 0.36788. Depth of penetration is defined as:

$$D_p=\frac{1}{\alpha }$$

(6)

where α is the attenuation constant of the material in nepers per meter.

2.2. Scattering, Reflection, and Anti-Reflection

Sensor nodes find a human body, when they are placed, to be layered media. Fat, muscles etc. are such independent layers. Signals from wireless sensors in human body experience scattering, reflection, and diffraction by those layers. In Appendix B, we started with acoustic wave for mathematical formulation and then used these formulae for electromagnetic wave. We have established the basic equations for the wave propagation through the layered media. Now we will use those mathematical equations for calculating some wave properties such as scattering, reflection, and transmission while passing through layered media.

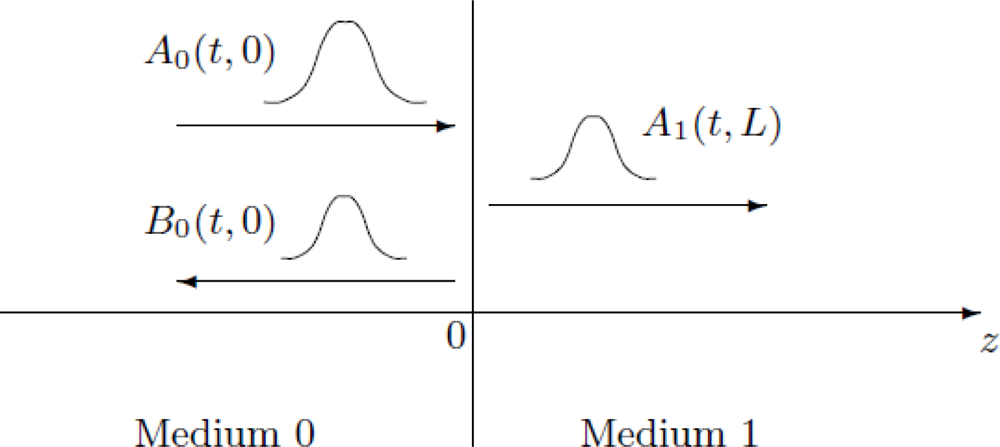
2.2.1. Scattering by a Single Interface

In this section we consider the case in which two homogeneous half-spaces are separated by an interface at z = 0 (Figure 3):

$$\rho (z)=\{\begin{array}{c}{\rho }_0 \mathit{if} z<0\\ {\rho }_1 \mathit{if} z>0\end{array} K(z)=\{\begin{array}{c}{K}_0 \mathit{if} z<0\\ K_1 \mathit{if} z>0\end{array}$$

The goal of this section is to analyze the scattering problem in terms of right- and left-going modes (Appendix B).

We introduce the local velocities $c_j=\sqrt{K_j/{\rho }_j}$ and impedances ${\zeta }_j=\sqrt{K_j {\rho }_j}$ and the right- and left-going modes defined by:

$$z<0:\{\begin{array}{c}{A}_0 (t,z)={\zeta }_0^{-1/2}p(t,z)+{\zeta }_0^{1/2}u(t,z)\\ B_0 (t,z)=-{\zeta }_0^{-1/2}p(t,z)+{\zeta }_0^{1/2}u(t,z)\end{array}$$

(7)

$$z>0:\{\begin{array}{c}{A}_1 (t,z)={\zeta }_1^{-1/2}p(t,z)+{\zeta }_1^{1/2}u(t,z)\\ B_1 (t,z)=-{\zeta }_1^{-1/2}p(t,z)+{\zeta }_1^{1/2}u(t,z)\end{array}$$

(8)

For j = 0, 1, the pairs (A_j, B_j) satisfy the following system in their respective half-spaces:

$$\frac{\partial }{\partial z}\left[\begin{array}{c}{A}_j\\ B_j\end{array}\right]=\frac{1}{c_j}\left[\begin{array}{cc}-1& 0\\ 0& 1\end{array}\right]\frac{\partial }{\partial t}\left[\begin{array}{c}{A}_j\\ B_j\end{array}\right]$$

(9)

which means that A_j (t, z) is a function of t − z/c_j only, and B_j (t, z) is a function of t + z/c_j only.

We assume that a right-going wave with the time profile f is incoming from the left and is partly reflected by the interface. We also assume a radiation condition in the right half-space so that no wave is coming from the right. Assume that f is completely supported in (0, ∞). We next introduce two ways to define proper boundary conditions:

(I) We can consider an initial value problem with initial conditions given at some time t₀ < 0 by:

$$u(t=t_0,z)=\frac{1}{2{\zeta }_0^{1/2}}f(t_0-z/c_0), p(t=t_0,z)=\frac{{\zeta }_0^{1/2}}{2}f(t_0-z/c_0)$$

(10)

As shown in the Appendix B, these initial conditions generate a pure right-going wave whose support at t = t₀ is in the interval z ∈ (−∞, c₀t₀), which lies in the left half-space.

(II) We can consider a point source located at some point z₀ < 0 and generating a forcing term of the from:

$$F(t,z)={\zeta }_0^{1/2}f(t-z_0/c_0)\delta (z-z_0)$$

(11)

As seen in the Appendix B, this point source generates two waves. The left-going wave is propagating into the negative z-direction and will never interact with the interface, so we will ignore it. The right-going wave first propagates in the homogeneous left half-space and it eventually interacts with the interface z = 0.

In terms of the right- and left-going waves, these two formulations give the same descriptions. We have A₀ (t, z) = f(t − z/c₀) for z < 0, and B₁(t, z) = 0 for z > 0, and consequently, at the interface z = 0:

$$A_0(t,0)=f(t), B_1(t,0)=0$$

(12)

Note that the delays introduced in the initial conditions (10) and in the forcing term (11) have been chosen so that the boundary conditions (12) have a very simple form.

The pairs (A₀, B₀) and (A₁, B₁) are coupled by the jump conditions at z = 0 corresponding to the continuity of the velocity and pressure fields:

$$\begin{array}{l}u(t,0)={\zeta }_0^{-1/2}\left(\frac{A_0(t,0)+B_0(t,0)}{2}\right)={\zeta }_1^{-1/2}\left(\frac{A_1(t,0)+B_1(t,0)}{2}\right)\\ p(t,0)={\zeta }_0^{1/2}\left(\frac{A_0(t,0)-B_0(t,0)}{2}\right)={\zeta }_1^{1/2}\left(\frac{A_1(t,0)-B_1(t,0)}{2}\right)\end{array}$$

which gives:

$$\left[\begin{array}{c}{A}_1(t,0)\\ B_1(t,0)\end{array}\right]=\mathit{J}\left[\begin{array}{c}{A}_0(t,0)\\ B_0(t,0)\end{array}\right], \mathit{J}=\left[\begin{array}{cc}{r}^{(+)}& r^{(-)}\\ r^{(-)}& r^{(+)}\end{array}\right]$$

(13)

with $r^{(\pm )}=\frac{1}{2}(\sqrt{{\zeta }_1/{\zeta }_0}\pm \sqrt{{\zeta }_0/{\zeta }_1})$. Note that (r⁽⁺⁾)² – (r⁽⁻⁾)² = 1. The matrix J can be interpreted as a propagator, since it “propagates” the right- and left-going modes from the left side of the interface to the right side. Such a propagator matrix will be called interface propagator in the following.

Taking into account the boundary conditions (12) yields:

$$\left[\begin{array}{c}{A}_1(t,0)\\ 0\end{array}\right]=\mathit{J}\left[\begin{array}{c}f(t)\\ B_0(t,0)\end{array}\right]$$

and solving this equation gives:

$$B_0(t,0)=𝒭 f(t), A_1(t,0)=𝒯 f(t)$$

where 𝒭 and $𝒯$ are the reflection and transmission coefficients of the interface:

$$𝒭=-\frac{r^{(-)}}{r^{(+)}}=\frac{{\zeta }_0-{\zeta }_1}{{\zeta }_0+{\zeta }_1}, 𝒯=\frac{1}{r^{(+)}}=\frac{2\sqrt{{\zeta }_0{\zeta }_1}}{{\zeta }_0+{\zeta }_1}$$

These coefficients satisfy the energy-conservation relation:

$${𝒭}^2+{𝒯}^2=1$$

meaning that the sum of the energies of the reflected and transmitted waves is equal to the energy of the incoming waves. Finally, the complete solution for z < 0 in terms of the right- and left-going modes is:

$$A_0(t,z)=f(t-z/c_0), B_0(t,z)=𝒭f(t+z/c_0)$$

and for z > 0:

$$A_1(t,z)=𝒯 f(t-z/c_1), B_1(t,z)=0$$

Using (7–8) we can obtain the pressure and velocity fields (Figure 4).

2.2.2. Single-Layer Case: Scattering

In this section, we consider the case of a homogeneous slab with thickness L embedded between two homogeneous half-spaces (Figure 5). Three regions can be described as follows:

$$\rho (z)=\{\begin{array}{c}{\rho }_0 \mathit{if} z<0,\\ {\rho }_1 \mathit{if} z\in [0,L],\\ {\rho }_2 \mathit{if} z<0,\end{array} K(z)=\{\begin{array}{c}{K}_0 \mathit{if} z<0\\ K_1 \mathit{if} z\in [0,L]\\ K_2 \mathit{if} z<0\end{array}$$

We introduce the local velocities $c_j=\sqrt{K_j/{\rho }_j}$ and impedances ${\zeta }_j=\sqrt{K_j {\rho }_j}$ and the local right- and left-going modes defined by:

$$A_j (t,z)={\zeta }_j^{-1/2}p (t,z)+{\zeta }_j^{1/2}u (t,z),B_j (t,z)=-{\zeta }_j^{-1/2}p (t,z)+{\zeta }_j^{1/2}u (t,z)$$

with j = 0 for z < 0, j = 1 for z ∈ [0, L], and j = 2 for z = L. The boundary conditions correspond to an impinging pulse at the interface z = 0 and a radiation condition at z = L₂:

$$A_0(t, 0)=f(t),B_2(t,L)=0$$

The propagation equations (9) in each homogeneous region show that A_j is a function of t − z / c_j only and B_j is a function of t + z / c_j only. The waves inside the slab [0, L] are therefore of the form:

$$A_1(t,z)=a_1(t-z/c_1),B_1(t,z)=b_1(t+z/c_1)$$

while the reflected wave for z <0 is of the form:

$$B_0(t,z)=b_0(t+z/c_0)$$

and the transmitted wave for z > L is of the form:

$$A_2(t,z)=a_2\left(t-\frac{z-L}{c_2}\right)$$

We want to indentify the functions b₀ and a₂, which give the shapes of the reflected and transmitted waves.

2.2.3. Single-Layer Case: Reflection and Transmission Coefficients

The unknown functions b₀ and a₂ can be obtained from the continuity conditions for the velocity and pressure at the two interfaces. At z = 0, we have:

$$\left[\begin{array}{c}{A}_1(t,0)\\ B_1(t,0)\end{array}\right]={\mathit{J}}_{\mathbf{0}} \left[\begin{array}{c}{A}_0(t,0)\\ B_0(t,0)\end{array}\right], {\mathit{J}}_{\mathbf{0}}=\left[\begin{array}{cc}{r}_0^{(+)}& r_0^{(-)}\\ r_0^{(-)}& r_0^{(+)}\end{array}\right]$$

with $r_0^{(\pm )}=\frac{1}{2}(\sqrt{{\zeta }_1/{\zeta }_0}\pm \sqrt{{\zeta }_0/{\zeta }_1})$. Similarly, at z = L:

$$\left[\begin{array}{c}{A}_2(t,L)\\ B_2(t,L)\end{array}\right]={\mathit{J}}_{\mathbf{1}} \left[\begin{array}{c}{A}_1(t,L)\\ B_1(t,L)\end{array}\right], {\mathit{J}}_{\mathbf{1}}=\left[\begin{array}{cc}{r}_1^{(+)}& r_1^{(-)}\\ r_1^{(-)}& r_1^{(+)}\end{array}\right]$$

with $r_1^{(\pm )}=\frac{1}{2}(\sqrt{{\zeta }_2/{\zeta }_1}\pm \sqrt{{\zeta }_1/{\zeta }_2})$. We can write these relations in terms of the functions a_j, b_j as:

$$\left[\begin{array}{c}{a}_1(t)\\ b_1(t)\end{array}\right]={\mathit{J}}_{\mathbf{0}} \left[\begin{array}{c}f(t)\\ b_0(t)\end{array}\right], \left[\begin{array}{c}{a}_2(t)\\ 0\end{array}\right]={\mathit{J}}_{\mathbf{1}} \left[\begin{array}{c}{a}_1(t-L /c_1)\\ b_1(t+L /c_1)\end{array}\right]$$

which can be solved to get the reflected and transmitted waves. The situation is more complicated than in the case of a single interface, because of the time delays ±L/c₁. A convenient and general way to handle these delays is by going to the frequency domain, so that the time shifts are replaced by phase factors. The Fourier transforms of the modes are defined by:

$${\widehat{a}}_j (\omega )=\int a_j (t)e^{i\omega t}\mathit{dt}, {\widehat{b}}_j (\omega )=\int a_j (t)e^{i\omega t}\mathit{dt}$$

They satisfy the interface conditions:

$$\left[\begin{array}{c}{\widehat{a}}_1(\omega )\\ {\widehat{b}}_1(\omega )\end{array}\right]={\mathit{J}}_{\mathbf{0}} \left[\begin{array}{c}\widehat{f}(\omega )\\ {\widehat{b}}_0(\omega )\end{array}\right], \left[\begin{array}{c}{\widehat{a}}_2(\omega )\\ 0\end{array}\right]={\mathit{J}}_{\mathbf{1}} \left[\begin{array}{c}{\widehat{a}}_1(\omega )e^{i\frac{\omega L}{c_1}}\\ {\widehat{b}}_1(\omega )e^{-i\frac{\omega L}{c_1}}\end{array}\right]$$

(14)

where we have used the identity:

$$\int a_1(t-L / c_1) e^{i\omega t}\mathit{dt}=\int a_1(s) e^{i\omega \left(s+\frac{L}{c_1}\right)}\mathit{ds}={\widehat{a}}_1(\omega ) e^{i\frac{\omega L}{c_1}}$$

Introducing the frequency-dependent matrix:

$${\widehat{\mathit{J}}}_1(\omega )=\left[\begin{array}{cc}{r}_1^{(+) }{e}^{i\frac{\omega L}{c_1}}& r_1^{(-) }{e}^{-i\frac{\omega L}{c_1}}\\ r_1^{(-) }{e}^{i\frac{\omega L}{c_1}}& r_1^{(+) }{e}^{-i\frac{\omega L}{c_1}}\end{array}\right]$$

The second equation of (14) can be written as:

$$\left[\begin{array}{c}{\widehat{a}}_2(\omega )\\ 0\end{array}\right]={\widehat{\mathit{J}}}_1(\omega )\left[\begin{array}{c}{\widehat{a}}_1(\omega )\\ {\widehat{b}}_1(\omega )\end{array}\right]$$

(15)

The syplectic matrix Ĵ₁(ω) is a propagator in the frequency domain. It propagates the right- and left-going modes from the right side of the interface 0 to the right side of the interface 1, and it depends on the layer thickness L. Finally, combining the first equation of (14) and (15), we obtain the relation:

$$\left[\begin{array}{c}{\widehat{a}}_2(\omega )\\ 0\end{array}\right]={\widehat{\mathrm{K}}}_0(\omega )\left[\begin{array}{c}\widehat{f}(\omega )\\ {\widehat{b}}_0(\omega )\end{array}\right]$$

(16)

where the frequency-dependent syplectic matrix:

$${\widehat{\mathrm{K}}}_0(\omega )={\widehat{\mathit{J}}}_1(\omega ){\mathit{J}}_{\mathbf{0}}=\left[\begin{array}{cc}\widehat{U}(\omega )& \overline{\widehat{V}(\omega )}\\ \widehat{V}(\omega )& \overline{\widehat{U}(\omega )}\end{array}\right]$$

is the overall propagator of the slab. Equation (16) shows that K̂₀(ω) propagates the right- and left-going modes from the left side of the interface 0 to the right side of the interface 1. We find explicitly:

$$\begin{array}{l}\widehat{U}(\omega )=r_0^{(+)}{r}_1^{(+)}{e}^{i\frac{\omega L}{c_1}}+r_0^{(-)}{r}_1^{(-)}{e}^{-i\frac{\omega L}{c_1}}\\ \widehat{V}(\omega )=r_0^{(+)}{r}_1^{(-)}{e}^{i\frac{\omega L}{c_1}}+r_0^{(-)}{r}_1^{(+)}{e}^{-i\frac{\omega L}{c_1}}\end{array}$$

By solving equation (16), whose unknowns are â₂ (ω) and b̂₀ (ω) and using the expressions of $r_j^{(\pm )}$, we obtain:

$${\widehat{b}}_0(\omega )=\widehat{𝒭}(\omega )\widehat{f}(\omega ), {\widehat{a}}_2(\omega )=\widehat{𝒯}(\omega )\widehat{f}(\omega )$$

where the frequency-dependent reflection and transmission coefficients are:

$$\widehat{𝒭}(\omega )=-\frac{\widehat{V}(\omega )}{\overline{\widehat{U}(\omega )}}=\frac{R_1{e}^{2i\frac{\omega \mathrm{L}}{{\mathrm{c}}_1}}+R_0}{1+R_0{R}_1{e}^{2i\frac{\omega \mathrm{L}}{{\mathrm{c}}_1}}}$$

(17)

$$\widehat{𝒯}(\omega )=\frac{1}{\overline{\widehat{U}(\omega )}}=\frac{T_0{T}_1{e}^{i\frac{\omega \mathrm{L}}{{\mathrm{c}}_1}}}{1+R_0{R}_1{e}^{2i\frac{\omega \mathrm{L}}{{\mathrm{c}}_1}}}$$

(18)

using that |Û(ω)|² − |V̂(ω)|² = 1. Here $R_0=\frac{{\zeta }_0-{\zeta }_1}{{\zeta }_0+{\zeta }_1}$, $R_1=\frac{{\zeta }_1-{\zeta }_2}{{\zeta }_1+{\zeta }_2}$, $T_0=\frac{2\sqrt{{\zeta }_0{\zeta }_1}}{{\zeta }_0+{\zeta }_1}$, and $T_1=\frac{2\sqrt{{\zeta }_1{\zeta }_2}}{{\zeta }_1+{\zeta }_2}$ are the reflection and transmission coefficients of the two interfaces. The reflection and transmission coefficients of the layer satisfy the energy conservation relation | 𝒭̂ (ω)|² + | $𝒯$̂(ω)|² = 1 for all ω, which means that the individual energies of the frequency components of the incoming pulse are preserved by the scattering process. The main qualitative difference between the scattering by a single interface and the scattering by single layer is that the reflection and transmission coefficients in the layer case are frequency-dependent. This frequency dependence originates from interference effects between the waves that are scattered back and forth by the two interfaces of the layer.

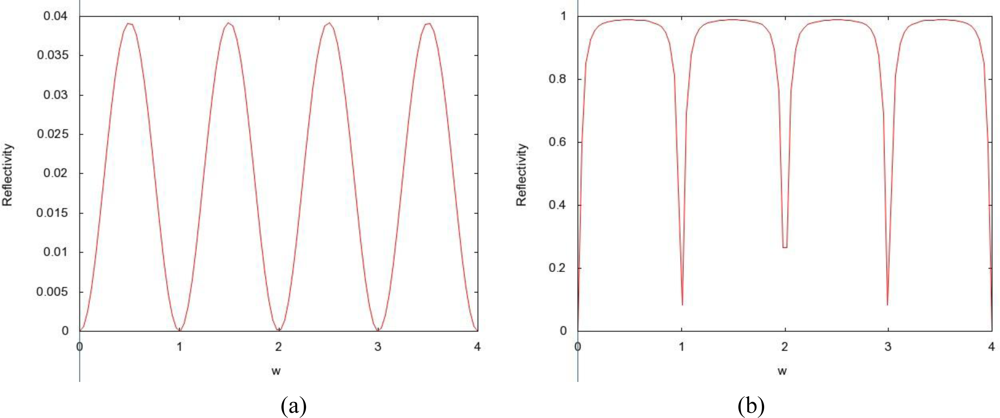
2.3. Filtering Property of the Layer

2.3.1. Reflection

Let us consider a layer embedded between two homogeneous half-spaces that have the same material properties, i.e., the situation in which ρ₂ = ρ₀ and K₂ = K₀. We then have R₁ = −R₀ and T₁ = T₀, which implies that the global reflectivity of the layer can be written as:

$${|\widehat{𝒭}(\omega )|}^2=1-\frac{1+R_0^4-2R_0^2}{1+R_0^4-2R_0^2 \mathit{cos}\left(\frac{2\omega L}{c_1}\right)}$$

(19)

The reflectivity is periodic with respect to the angular frequency ω with the period ω_c = πc₁/L. As a function of the angular frequency the reflectivity goes from the minimal value:

$${|\widehat{𝒭}|}_{\mathit{min}}^2=0 \mathit{for} \omega ={k\omega }_c,k\in \mathbb{Z}$$

to the maximal value:

$${|\widehat{𝒭}|}_{\mathit{max}}^2=1-{\left(\frac{1-R_0^2}{1+R_0^2}\right)}^2 \mathit{for} \omega =\left(k+\frac{1}{2}\right){\omega }_c,k\in \mathbb{Z}$$

This shows that for any value of the reflection coefficient R₀ of a single interface, there exist frequencies that are fully transmitted or fully reflected by the layer. If we consider the case of strong scattering $T_0^2\ll 1$, then the transmitted frequency bands have a width of the order of ${\omega }_c{T}_0^2$ around the fully transmitted frequencies kω_c. Outside of these bands, where total reflection occurs, the typical reflectivity is large, of order $1-T_0^4/4$.

2.3.2. Anti-Reflection

The total transmission phenomenon is also encountered in situations in which the two half-spaces are different. Indeed, consideration of human body part as an ideal, fully-transmitting layer is certainly beyond perfection. In a microscopic or constituent-wise sense, a human body-part, striated muscle for example, iscomposed of water (70.09%), ether-soluble extract (6.60%), crude protein (21.94%), etc. [35]. When one is faced with the task of modeling portions of human body as a medium for electromagnetic (signal) propagation, there are inevitable practical assumptions and approximations to be made. Thinner layers can be considered with more homogeneous characteristics, while for thicker setting with internal variability, the aggregate behavior sums up by and large. Thus a certain part of a human body like muscle or fat, when considered in macroscopic perspective, can be regarded as a continuum, and hence the idea of a planner-layered-medium assumption of human body tissues contextually holds for all practical modeling considerations. Such model could greatly affect related system design pertaining to crucial parameters (antenna and others); for instance, in addition to the frequency and bandwidth employed for the signal, the measurement of the depth of the body at which the transceiver of an implant device would function with acceptable accuracy has a lot to do with such parameters as permittivity, permeability, and impedance of the intermittent layers of body tissues.

Figure 7 shows a grossly approximated propagation system consisting of three layers: air, human body channel, and a transceiver. Admittedly, finding fully-transmitting or fully-reflecting layers in body-parts is unrealistic, but it is widely adopted practice to model systems using phantoms comprised of components having somewhat homogeneous characteristics, yet closely resembles body-parts in aggregate behavior. In some recent works ([33] and [34]) UWB antenna impedance matching has been studied in the context of biomedical implants. We assume that the two homogeneous half spaces (Figure 7) have different impedances ζ₀ ≠ ζ₂, then it is possible to choose the thickness L and the impedance ζ₁ of the layer so that a given frequency ω will be fully transmitted from one half-space to the other one, which would not be the case in absence of such a layer. From the analysis of the reflectivity function:

$${|\widehat{R}(\omega )|}^2=1-\frac{1-R_0^2-R_1^2+R_0^2{R}_1^2}{1+{2R}_0{R}_1 \mathit{cos}\left(\frac{2\omega L}{c_1}\right)+R_0^2{R}_1^2}$$

(20)

One can show that a necessary and sufficient condition for | 𝒭̂(ω)|² to be zero is that ${\mathrm{R}}_0^2+{\mathrm{R}}_1^2=-2{\mathrm{R}}_0{\mathrm{R}}_1\text{cos}\left(\frac{2\omega \mathrm{L}}{{\mathrm{c}}_1}\right)$. In the case ζ₀ ≠ ζ₂ this in turn enforces one to choose the impedance of the layer to be ${\zeta }_1=\sqrt{{\zeta }_0{\zeta }_2}$ (so that R₀ = R₁) and the thickness L to be chosen so that ωL/(πc₁) is half an integer (so that cos(2 ωL/c₁) = −1). Usually the thickness is chosen to be equal to a quarter of the wavelength, meaning ωL/(πc₁) = ½.

2.4. Path Loss in the Human Body (Near Field Far Field Consideration)

When EM RF waves propagate in freespace, the power received decreases at a rate of (1/d)ⁿ, n being the coefficient of pathloss. Other kinds of losses would be fading of signals due to multipath propagation. However, for propagation of EM waves in a lossy medium like human tissue, the losses would be mainly due to absorption of power in the tissue, where it is dissipated as heat. As the tissue medium is lossy and mostly consists of water, the EM waves are attenuated considerably before they reach the receiver. The Specific Absorption Rate (SAR) is useful in determining the amount of power lost due to heat dissipation. SAR is defined as power absorbed per unit mass of the tissue [29]. SAR is a standard measure of how much power is absorbed in the tissue and depends upon E- and H-field strengths. By determining the average SAR over the entire mass of the tissue between the transmitter and the receiver, we are able to compute the total power lost. SAR in the near field of the transmitting antenna depends mainly on the H-field, whereas the SAR in the far field of the transmitting antenna depends mainly on the E-field. We use Maxwell’s E- and H-fields equations for lossy medium to obtain the average SAR of the medium between the transmitting and the receiving antenna in the far field and near field, respectively. WBAN applications involve wireless communications between implanted biosensor nodes inside human body.

These nodes exchange data among themselves and also with the base-station. In general, the system model consists of numerous biosensor nodes placed inside the various parts of the human body surrounded by tissues. In particular, for the development of this model, we consider only one transmitting and one receiving antenna separated by a distance d. An elemental short dipole (dipole length_wavelength) in a lossy human tissue medium is considered for this purpose [28] and is shown in Figure 9. A small area of tissue surrounding the antenna is considered for our analysis. Thus we can safely assume the human tissue under consideration to be a homogeneous medium with no sharp edges, no rough surfaces and having uniform electric and magnetic properties. The received power is assumed to be due only to the power from the transmitter and not from any other source. The space around the radiating antenna is divided into near field and far field regions as shown in Figure 10.

The region of space immediately surrounding the antenna is known as the near field region. The extent of the near field in the case of short dipoles is given by d₀ = λ/2, where λ is the wavelength [30]. In the near field, the E- and H-field strengths vary rapidly with the distance from the antenna. The far field is the entire region beyond the near field. In the far field region, the E- and H-field exhibit a plane wave behavior. Power absorbed between the transmitting and receiving antennas can be considered as the sum of power absorbed in near field (P_NF) and far field (P_FF) regions. The total power absorbed between the two antennas is computed by numerical integration.

Consider an elemental oscillating electric dipole in a lossy medium of conductivity σ (S/m), permittivity ɛ (F/m), permeability μ (H/m), complex propagation constant γ, complex intrinsic impedance $\eta =\frac{\gamma }{\alpha +j\omega \varepsilon }$ [28] at frequency ω, as shown in Figure 8. The dipole consists of a short conducting wire of length dl, terminated in two small conductive spheres or disks. Assume that the current I is uniform and varies sinusoidally with time [28]. The electromagnetic field at a distance ‘R’ for an Hertzian dipole is derived from the vector potential A, given by [28]:

$$A=a_z\frac{\mu \mathit{Idl}}{4\pi }\frac{e^{-\gamma R}}{R}=a_z{A}_z$$

where a_z is the unit vector in the z-direction, γ is the propagation constant, given by γ = α + jβ; attenuation constant α and phase constant β is given by as [28]:

$$\begin{array}{c}\alpha =\omega \sqrt{\frac{\mu \epsilon }{2}}{\left[\sqrt{1+{\left(\frac{\sigma }{\omega \epsilon }\right)}^2}-1\right]}^{1/2} (\text{Neper}/\mathrm{m})\\ \beta =\omega \sqrt{\frac{\mu \epsilon }{2}}{\left[\sqrt{1+{\left(\frac{\sigma }{\omega \epsilon }\right)}^2}+1\right]}^{1/2} (\text{rad}/\mathrm{m})\end{array}$$

Spherical components of A (i.e., a_RA_R + a_θA_θ + a_φA_φ) are given by A_R = A_zcosθ, A_θ = −A_zsingθ and A_φ = 0. The magnetic field intensity H and the electric field intensity E is given by [28]:

$$\begin{array}{c}H=\frac{1}{\mu }(\nabla \times A)=a_{\phi }\frac{1}{\mu R}\left[\frac{\partial }{\partial R}({\mathit{RA}}_{\theta })-\frac{\partial }{\partial \theta }{A}_R\right]\\ E=\frac{1}{\alpha +j\omega \varepsilon }(\nabla \times H)=\frac{1}{\alpha +j\omega \varepsilon }\left[a_R\frac{1}{\mathit{Rsin}\theta }\frac{\partial }{\partial \theta }(H_{\phi }\mathit{sin}\theta )-a_{\theta }\frac{1}{R}\frac{\partial }{\partial R}({\mathit{RH}}_{\phi })\right]\end{array}$$

Solving the above magnetic and electric field equations for lossy medium and expressing in terms of complex impedance η we get:

$$E_R=\eta \frac{2\mathit{Idlcos}\theta }{4\pi }{e}^{-\gamma R} \left(\frac{1}{\gamma R^3}+\frac{1}{R^2}\right)$$

(21)

$$E_{\theta }=\eta \frac{\mathit{Idlsin}\theta }{4\pi }{e}^{-\gamma R} \left(\frac{1}{\gamma R^3}+\frac{1}{R^2}+\frac{\gamma }{R}\right)$$

(22)

$$H_{\phi }=\frac{\mathit{Idlsin}\theta }{4\pi }{e}^{-\gamma R} \left(\frac{1}{R^2}+\frac{\gamma }{R}\right)$$

(23)

2.4.1. Power Absorbed in the Near Field

The SAR in the near field is given by [31]:

$$\mathit{SAR}=\frac{\sigma }{\rho }\frac{\mu \omega }{\rho \sqrt{{\sigma }^2+{\varepsilon }^2{\omega }^2}}{(1+c_{\mathit{corr}} \tau )}^2{H}_{\mathit{rms}}^2 \mathit{watts}/\mathit{Kg}$$

where ρ is the density of the medium and c_corr is the correction factor to take into account the changed reflection properties for small distances R of the antenna from the scatterer. Since we assume both the transmitting and receiving antennae are in a same homogeneous medium, the plane wave reflection coefficient τ is zero. By substituting τ = 0 and RMS value of the H-field, the above equation reduces to:

$$\mathit{SAR}=\frac{\sigma }{\rho }\frac{\mu \omega }{\rho \sqrt{{\sigma }^2+{\varepsilon }^2{\omega }^2}}{\left(\frac{\mathit{Idlsin}\theta }{4\pi }{e}^{-\alpha R} \left(\frac{1}{R^2}+\frac{|\gamma |}{R}\right)\right)}^2$$

which gives the value of SAR at a point at distance ‘R’ and angle ‘θ’ from the dipole. Power at infinitely small volume (dV = R² sinθ dR dθ dφ) is:

$$\mathrm{\Delta }P=\mathit{SAR}\times \mathrm{\Delta }\mathit{mass}=\mathit{SAR}\times \rho \times \mathit{dV}$$

(24)

The power absorbed in the near field of the lossy tissue can be obtained by computing the average SAR over the entire tissue mass in the near field, which is obtained by integrating ΔP over the entire mass in the near field region, i.e., from the surface of the antenna (R = r) to the end of the near-field region (R = d₀):

$$\begin{array}{c}{P}_{\mathit{NF}}={\int }_{R=r}^{d_0}{\int }_{\theta =0}^{\pi }{\int }_{\phi =0}^{2\pi }\mathrm{\Delta }P\\ =\sigma \frac{\mu \omega }{\sqrt{{\sigma }^2+{\varepsilon }^2{\omega }^2}}{\left(\frac{\mathit{Idl}}{4\pi }\right)}^2{\int }_{R=r}^{d_0}{\int }_{\theta =0}^{\pi }{\int }_{\phi =0}^{2\pi }{R}^2{\mathit{sin}}^3\theta \times e^{-2\alpha R}\left(\frac{1}{R^4}+\frac{{\mathit{\left|}\gamma \mathit{\right|}}^2}{R^2}+\frac{2\mathit{\left|}\gamma \mathit{\right|}}{R^3}\right)\mathit{dRd}\theta d\phi \end{array}$$

Solving by numerical integration and writing $\frac{1}{\sqrt{{\sigma }^2+{\varepsilon }^2{\omega }^2}}$ as |η|/|γ|:

$$P_{\mathit{NF}}=\sigma \mu \omega \frac{|\eta |}{|\gamma |}\frac{I^2{\mathit{dl}}^2}{6\pi }[A+B+C]$$

(25)

where:

$$\begin{array}{l}A=e^{-2\alpha r}\left(\frac{{|\gamma |}^2}{2\alpha }+\frac{d_0-r}{{4r}^2}+\frac{|\gamma |(d_0-r)}{2r}\right)\\ B=e^{-2\alpha d_0}\left(\frac{-{|\gamma |}^2}{2\alpha }+\frac{d_0-r}{{4d}_0^2}+\frac{|\gamma |(d_0-r)}{{2d}_0}\right)\\ C=e^{-\alpha (d_0+r)}\left(\frac{2(d_0-r)}{{(d_0+r)}^2}+\frac{2|\gamma |(d_0-r)}{(d_0+r)}\right)\end{array}$$

The antenna dimensions depend on the wavelength of the wave in the medium given by ${\lambda }_m=\frac{2\pi }{\beta }$ [28].

2.4.2. Power Absorbed in the Far Field

Neglecting $\frac{1}{R^2},\frac{1}{R^3}\dots ..$ terms from field Equations (21)–(23) for the far field, we have:

$$\begin{array}{c}{E}_R=0,E_{\theta }=\eta \frac{\mathit{Idlsin}\theta }{4\pi }{e}^{-\gamma R} \left(\frac{\gamma }{R}\right)\\ H_{\phi }=\frac{\mathit{Idlsin}\theta }{4\pi }{e}^{-\gamma R}\left(\frac{\gamma }{R}\right)\end{array}$$

In the far field the specific absorption rate depends only on the E_rms value which is given by [29]:

$$\begin{array}{c}\mathit{SAR}=\frac{\sigma }{\rho }{E}_{\mathit{rms}}^2 \mathit{watts}/\mathit{Kg}\\ =\frac{\sigma }{\rho }{\left(|\eta ||\gamma |\frac{\mathit{Idlsin}\theta }{4\pi R}{e}^{-\alpha R}\right)}^2\end{array}$$

The power absorbed in the infinitely small volume (dV = R² sinθ dR dθ dφ) in the far field, at a distance R and angle θ from the dipole can again be obtained from (24):

$$\mathrm{\Delta }P=\sigma {\left(|\eta ||\gamma |\frac{\mathit{Idl}}{4\pi }\right)}^2 {\mathit{sin}}^3{\theta e}^{-2\alpha R}\mathrm{\hspace{0.17em} }\mathit{dR} d\theta d\phi$$

The total power absorbed in the far field of the lossy tissue between the source and destination antennas can be obtained by computing the average SAR over the entire tissue mass in the far field from distance d₀ to d (d₀ is the point where the far field starts). This is obtained by integrating ΔP over the mass in the far field between the two antennas:

$$P_{\mathit{FF}}={\int }_{R=d_0}^d{\int }_{\theta =0}^{\pi }{\int }_{\phi =0}^{2\pi }\mathrm{\Delta }P=\sigma {|\eta |}^2{|\gamma |}^2\frac{I^2{\mathit{dl}}^2\mathit{dl}}{12\pi \alpha }(e^{-2\alpha d_0}-e^{-2\alpha d})$$

(26)

Power received

The effective radiated power (ERP) is obtained by subtracting the loss in the near field (P_NF) and far field (P_FF between the transmitting and receiving antennas) from the transmitted power P_T (i.e., (P_T −P_Loss)G_t), where P_Loss = P_NF +P_FF is obtained from (25) and (26). The power density (P_e, Power per unit area) at a distance ’d’ is different in near field and far field regions:

• P_R in the Near Field: There is no general formula for the estimation of field strength in the near field zone [30]. Only measurements can provide a simple means of field evaluation. However, reasonable calculations can be made for antennas like dipole or monopole. When the receiving antenna is in the near field region of the transmitting antenna, the power density does not necessarily depend on the distance from the antenna, but varies rapidly with distance, and may exhibit oscillatory behavior. The magnitude of on-axis (main beam) power density varies according to the location in the near field and its maximum value is approximated by [32] P_e = 16 δP / πL², where L is the largest dimension of the antenna, P is P_T − P_NF, and δ is the aperture efficiency (typically 0.5–0.75) [32]. It can be approximated as δ = A_e/A (A_e is the effective aperture and A is the physical area of the antenna). The power received by the receiving antenna in the near field can be approximated by:

  $$P_R=P_e{A}_e=\frac{16\delta (P_T-P_{\mathit{NF}})}{\pi L^2}{A}_e$$
• P_R in the Far Field: On the other hand when the receiving antenna is in the far field region of the transmitting antenna, the power density is dependent on the distance d and is given by:

  $$P_e=\frac{(P_T-P_{\mathit{Loss}})}{4\pi d^2}{G}_t$$

The power received by the receiving antenna in the far field is P_R = P_eA_e, where the receiving antenna aperture A_e is given by $A_e=\frac{{\lambda }^2}{4\pi }{G}_r$ Here, G_t and G_r are the gain of the transmitting and receiving antenna, respectively. Thus the received power is:

$$P_R=\frac{(P_T-P_{\mathit{NF}}-P_{\mathit{FF}}){\lambda }^2}{{(4\pi d)}^2}{G}_t{G}_r$$

and a total phase change of e^−jβ is involved during the propagation of the wave. Thus, PMBA can be used for calculating the propagation loss using the two Equations (25) and (26).

3. Numerical Results

The permittivity of biological tissues depends on the type of tissues (e.g. skin, fat, or muscle), water content, temperature, and frequency. However, the permittivity and frequency may also determine how far the EM wave penetrates into the body. The term depth of penetration (D_p) usually quantifies this. It is observed from Equations (1) and (2) that the wave gets attenuated as it propagates in the biological material along the z-axis. As shown in Figure 1, variation of radiation power density has been compiled for four different frequencies (27 MHz, 100 MHz, 433 MHz, and 1,500 MHz) with respect to the depth in muscle. At a given depth, usage of lower frequency results in a higher power density as illustrated in Figure 1. We discovered a distinct feature (demonstrated by Figure 2) which states that attenuation is more in fat than that in muscle with respect to frequency. Expressions for left- and right-going modes of a pulse have been derived in appendix B. Equations (19) and (20) express the reflectivity and transmitivity of a layer respectively. Equations (7) and (8) are the expressions of the left- and right-going modes respectively of a pulse when scattered by a single interface between two layers. Figure 4. shows the scattering of a Gaussian pulse by an interface separating two homogeneous half-spaces (c₀, ζ₀, z < 0) and (c₁, ζ₁, z > 0). The spatial profiles of the velocity field (a) and of the pressure field (b) are plotted at times t = −4, t = −3,..., t = 6. Reflectivity | 𝒭̂(ω)|² versus frequency has been depicted by Figure 6. We can see here that, at a certain period ω_c = πc₁/L, the reflectivity maximum which is almost 0.04 for a layer with R₀ = −R₁ = 0.1 (a) and almost 1.0 for a layer with R₀ = −R₁ = 0.9 (b). In Figure 8, Transmitivity | $𝒯$̂ (ω)|² versus frequency curves have been drawn. Transmitivity has been found to vary periodically with a certain frequency. The period of this frequency band depends upon the choice of the layer thickness L. For a layer with R₀ = −R₁ = 0.1, transmitivity is about 1.0 (a) and for a layer with R₀ = −R₁ = 0.9, transmitivity is about 0.9 (b). Here the period is ω_c = πc₁/2L. From Equation (20), we can see that by the proper choice of the impedance of the layer to be ${\zeta }_1=\sqrt{{\zeta }_0{\zeta }_2}$ (so that R₀ = R₁) and the thickness L to be chosen so that ωL/(πc₁) is half an integer (so that cos(2 ωL/c₁) = −1), we can form a fully transmitting layer. Usually the thickness is chosen to be equal to a quarter of the wavelength, meaning ωL/(πc₁) = ½. Therefore, from the results shown above, we can infer that a layer can either fully reflect or fully transmit any incoming wave at a certain frequency or frequency band. We can use these results to UWB by proper choice of impedance and the thickness L. Power loss in near filed and far field due to absorption has also been analyzed. A propagation loss model (PMBA) for homogeneous tissue bodies has been presented, which compares PMBA with the freespace propagation model ( $P_R=P_T{G}_t{G}_r{\left(\frac{\lambda }{4\pi d}\right)}^n$; with loss coefficient n = 3). A frequency range of (900 MHz to 3 GHz) has been considered here. We have been able to make a conclusion that, compared to freespace, there is an additional 30–35 dB of attenuation at small distances the far field (Figure 11). This loss increases further with the distance and frequency. It is argued that the human body cannot be considered as layered a media. However, we have been able to homogenize the human body channel, which is shown in Appendix C.

4. Conclusions

Employing UWB in WBAN involves a lot of promise, just as there are a number of relevant challenges. We studied the technical feasibility in this regard with a concentration in electromagnetic propagation of the signal across human body. Unlike conventional wireless channels, human body comes with a great deal of structural complexity requiring significantly different design considerations. The reflection and transmission coefficients of human body are heavily dependent upon the dielectric constants as well as upon the frequency. In this work, we investigated a layer-wise model for electromagnetic propagation across the components of the body in regard to such key aspects as scattering, reflectivity, and transmitivity. Naturally, the segmentation in precise layers are not what we come across in a body. But, the approximate model employing homogenization could help assess the aggregate behavior of the wireless communication involving implant devices, thus guiding the potential design issues for antenna characteristics, for instance. We also presented numerical depictions of some of the pertinent signal characteristics. From here on, one could expect to further improve the model in terms of suitable layering and other parameters of approximations.