Determination of Long Horizontal Borehole Height in Roofs and Its Application to Gas Drainage
Abstract
:1. Introduction
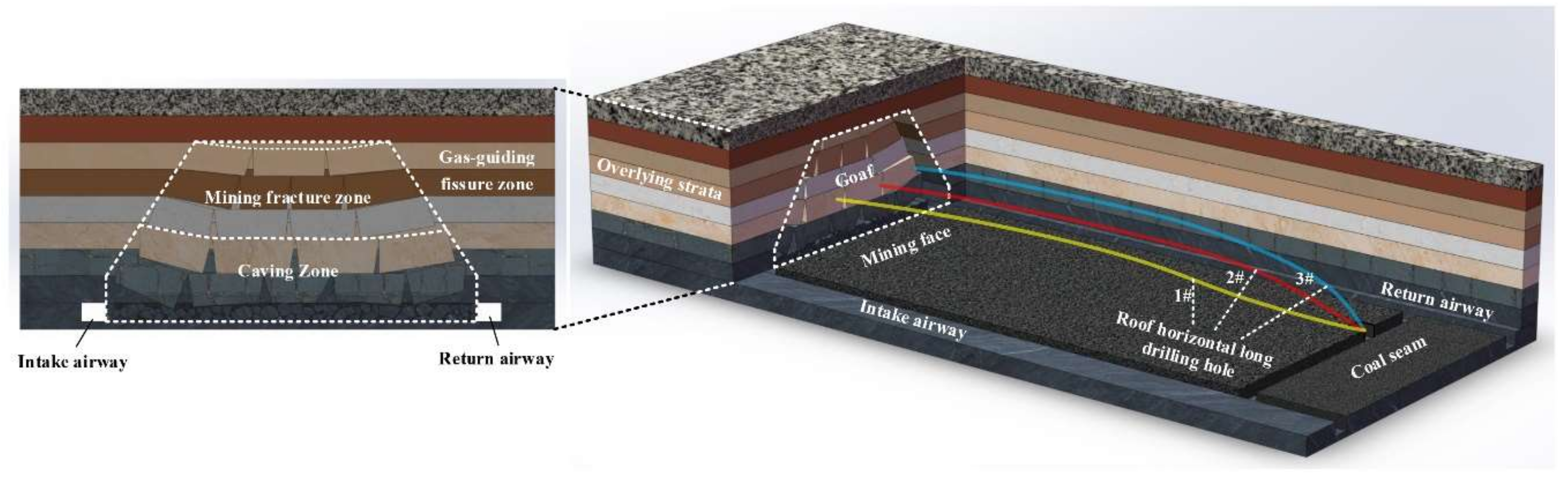
2. Geological and Mining Conditions of Tangkou Coal Mine
3. Analysis of Gas-Guiding Fracture Zone Height
3.1. Theoretical Analysis of Gas-Guiding Fracture Zone Height
3.2. Numerical Analysis of Gas-Guiding Fracture Zone Height
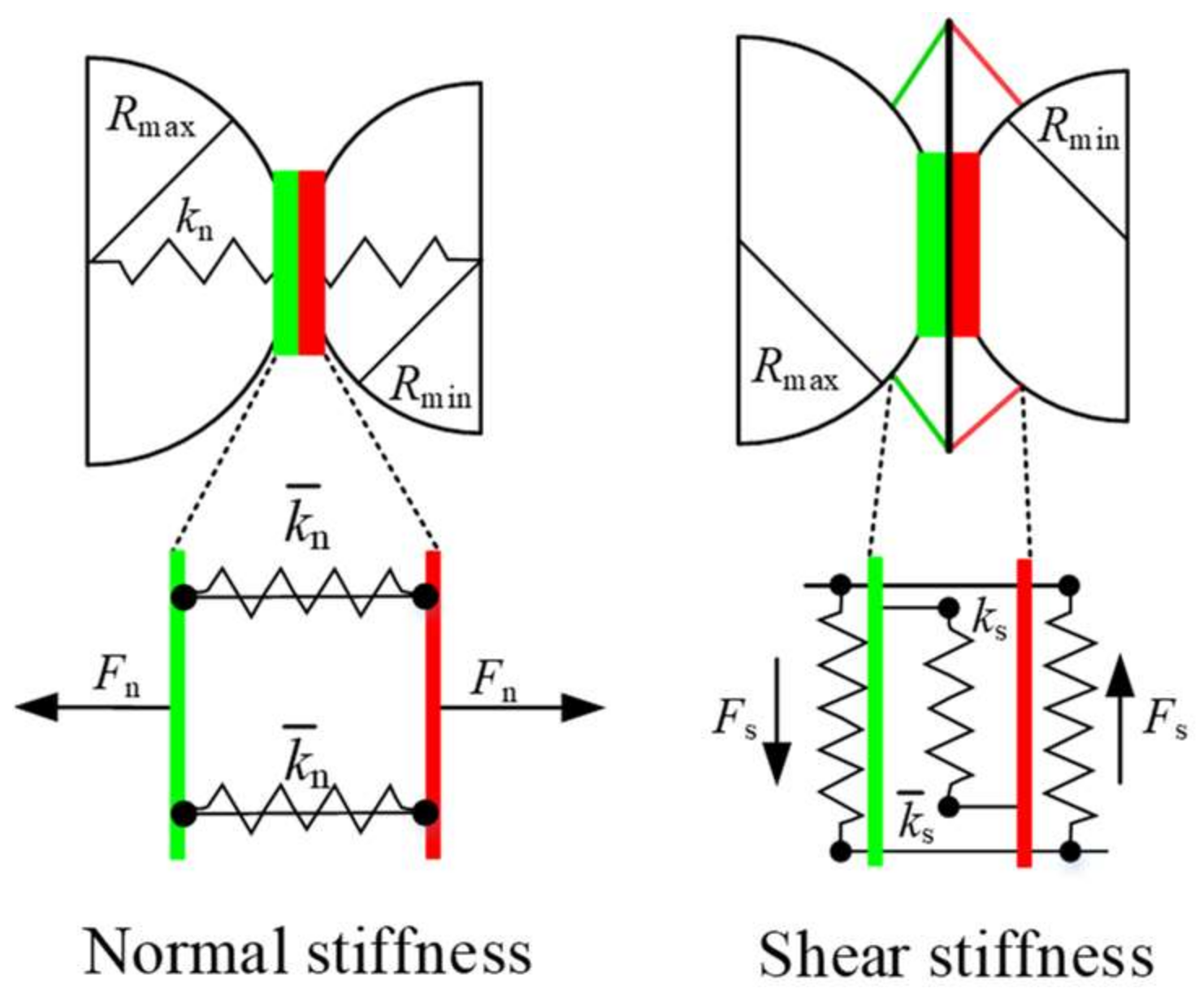
3.2.1. Particle Discrete Element Software PFC2D Principle
3.2.2. Macroscopic and Fine Parameter Selection and Inversion
3.2.3. Working Face Numerical Model and Measure Arrangement
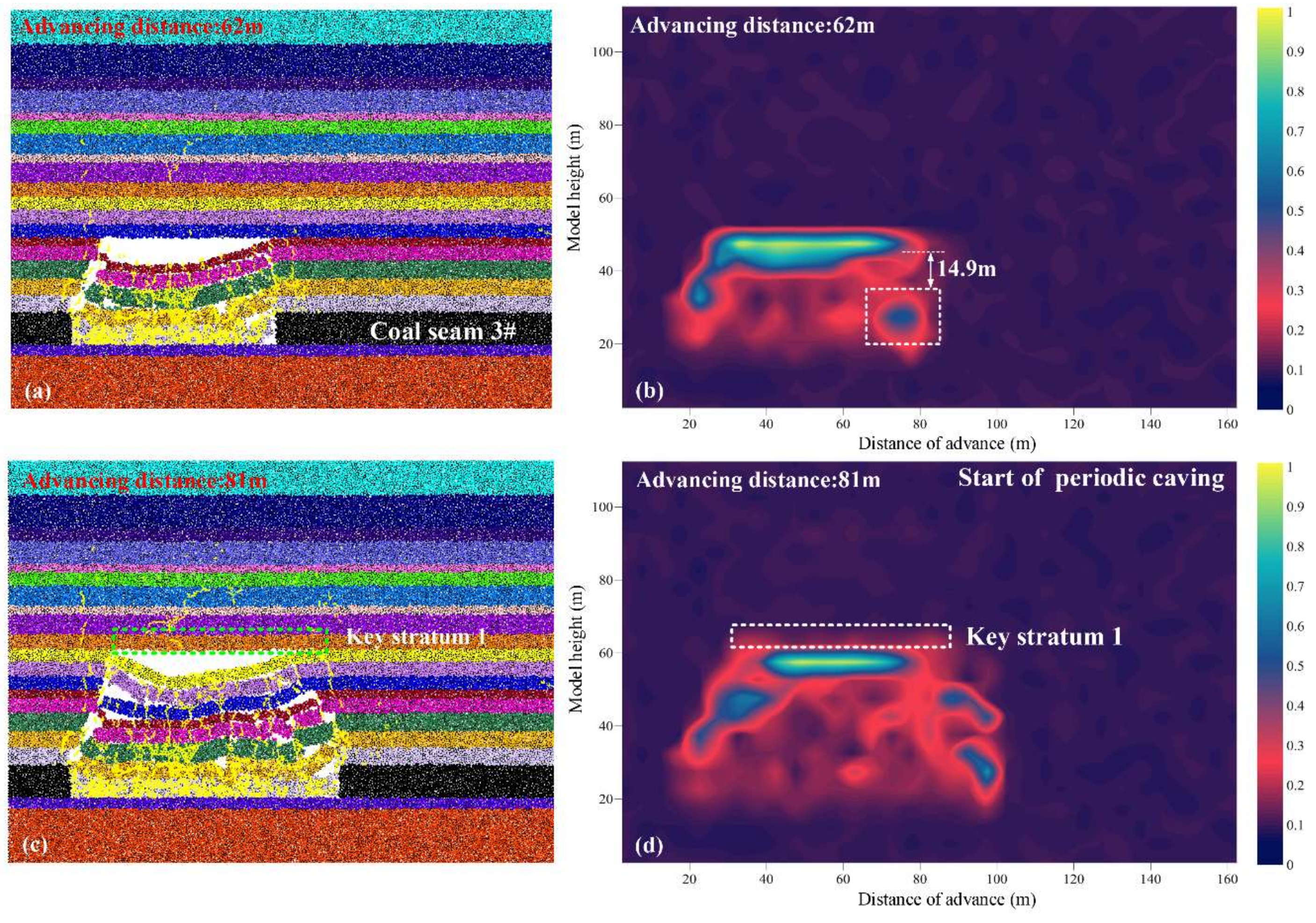
3.2.4. Analysis of Fissure and Porosity Changes
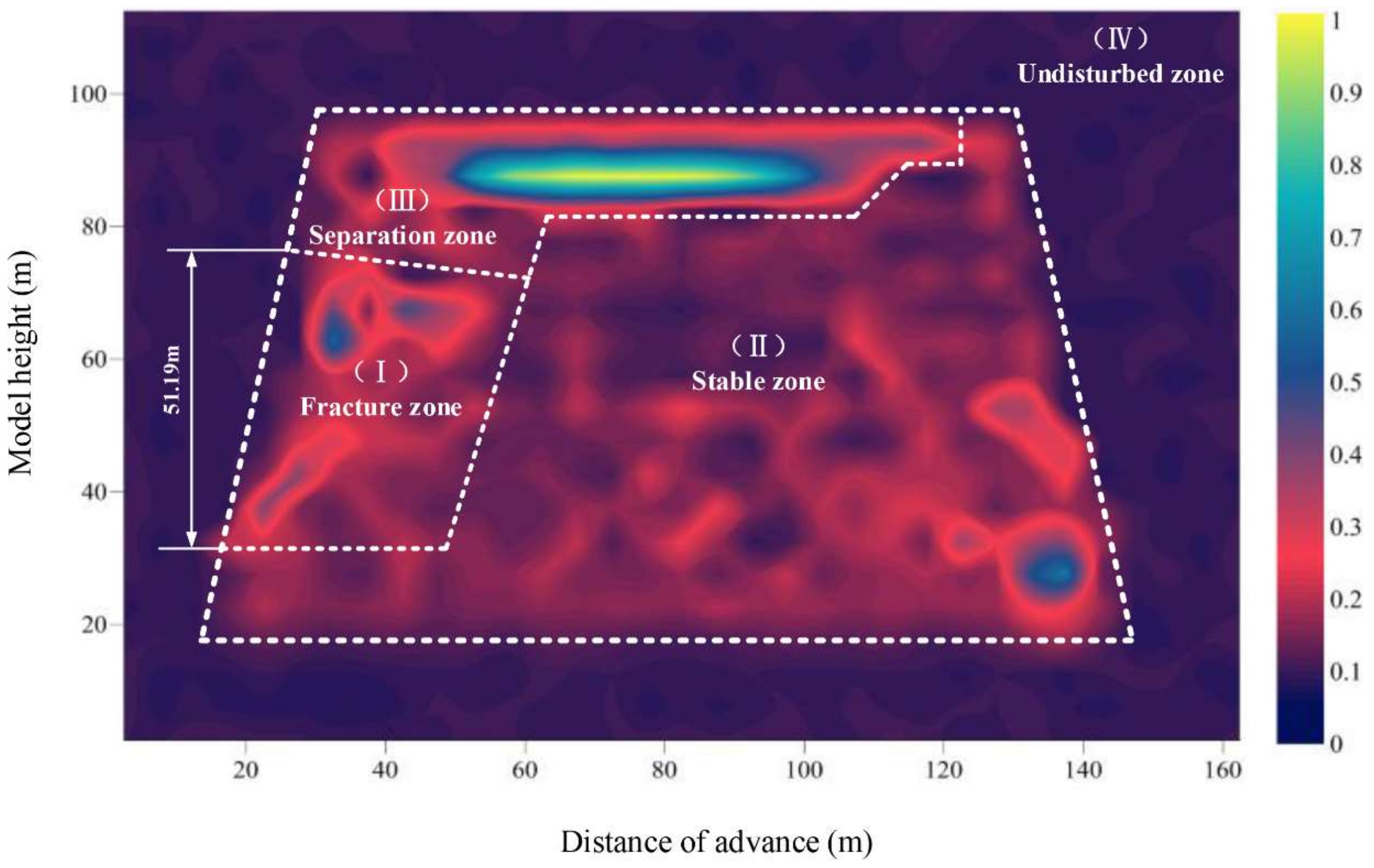
3.2.5. Gas-Guiding Fracture Zone Division
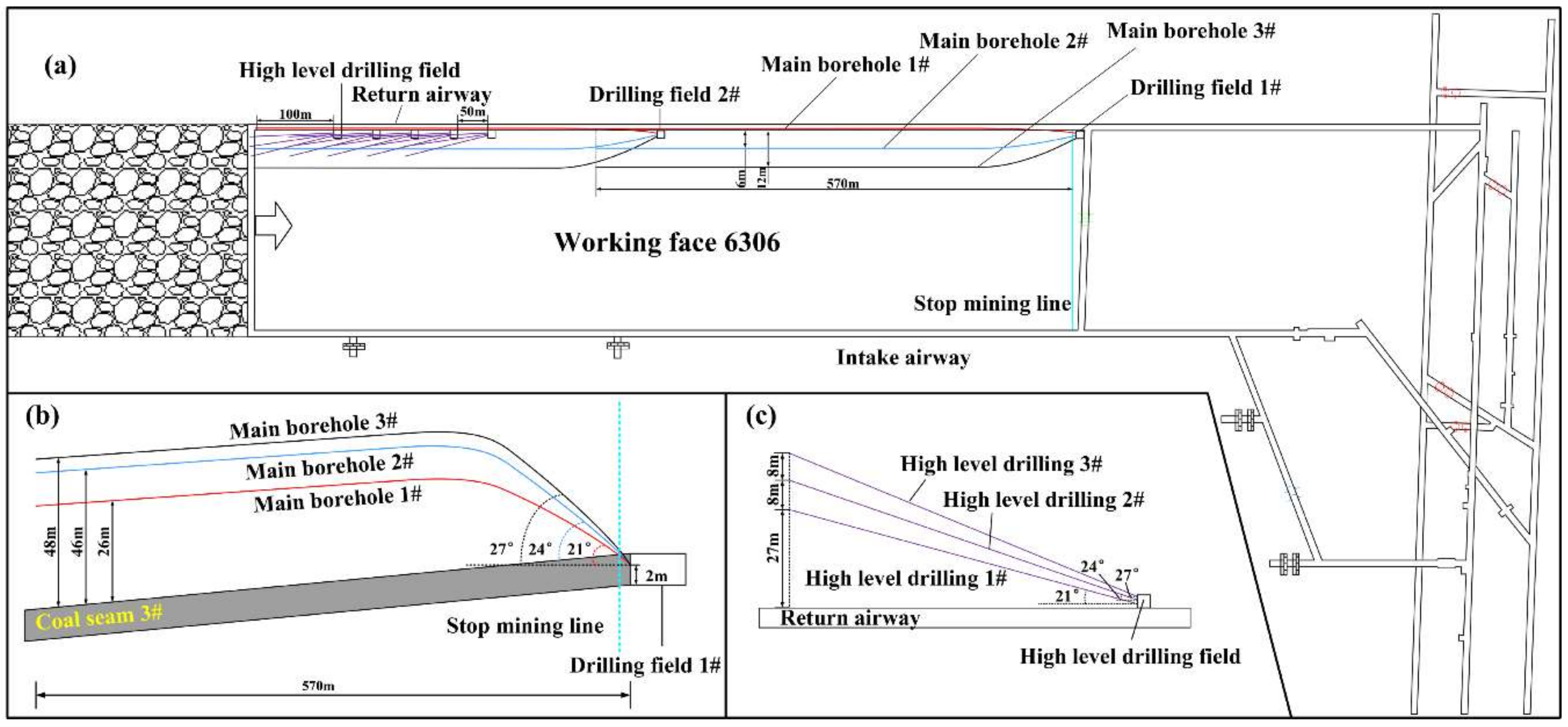
4. Field Application
4.1. Design of Methane Drainage Measures
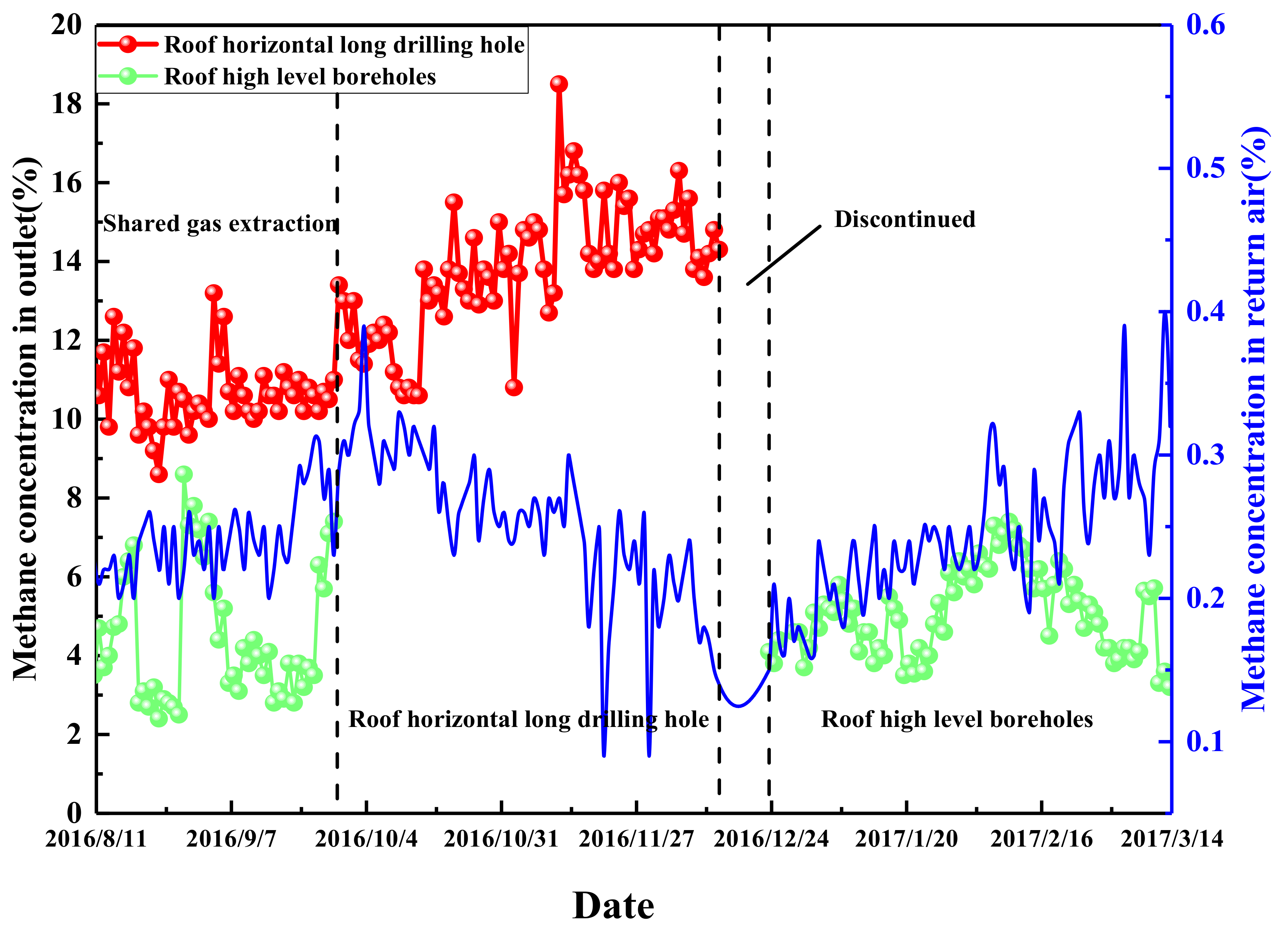
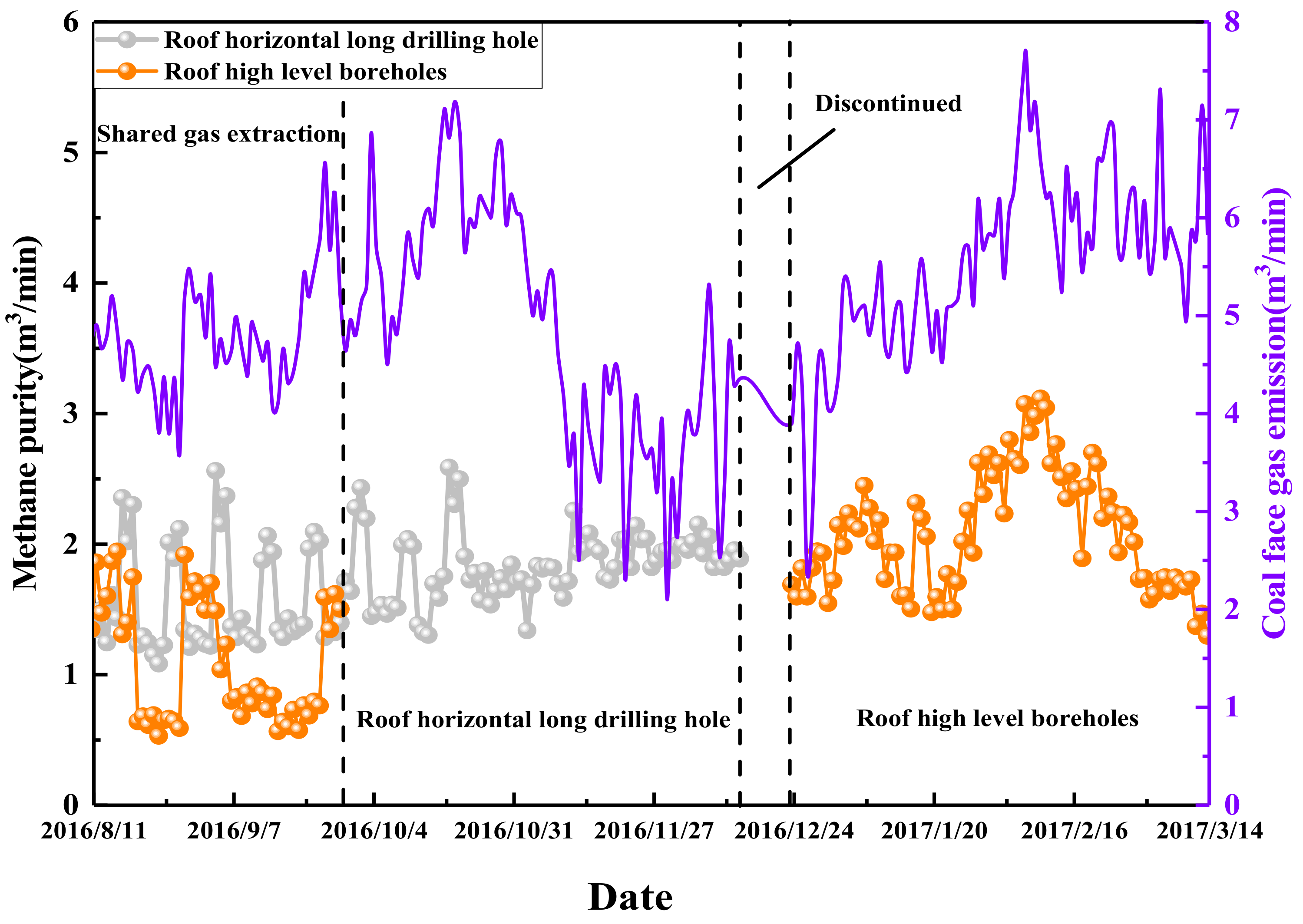
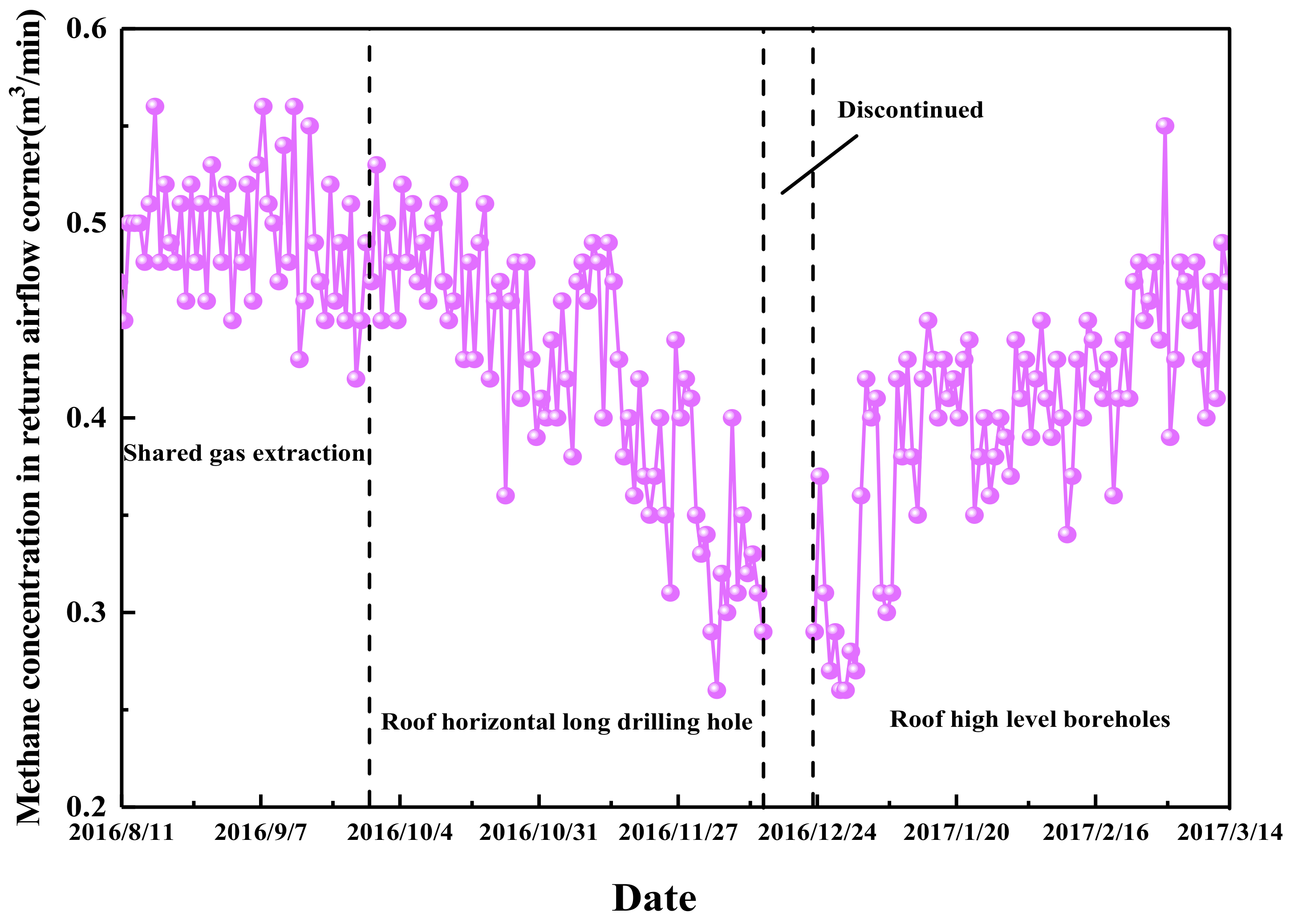
4.2. Comparative Analysis for Two Measure Gas Drainage Effects
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wu, Z.; Sun, R.; Han, S. Experiments on In-Seam Long-Borehole Directional Drilling Technology. China Coalbed Methane 2005, 2, 38–40. [Google Scholar]
- Karacan, C.Ö.; Ruiz, F.A.; Cotè, M.; Phipps, S. Coal mine methane: A review of capture and utilization practices with benefits to mining safety and to greenhouse gas reduction. Int. J. Coal Geol. 2011, 86, 121–156. [Google Scholar] [CrossRef]
- Karacan, C.Ö. Evaluation of the relative importance of coalbed reservoir parameters for prediction of methane inflow rates during mining of longwall development entries. Comput. Geosci. 2008, 34, 1093–1114. [Google Scholar] [CrossRef]
- Palmer, I. Coalbed methane completions: A world view. Int. J. Coal Geol. 2010, 82, 184–195. [Google Scholar] [CrossRef]
- Su, S.; Beath, A.; Guo, H.; Mallett, C. An assessment of mine methane mitigation and utilisation technologies. Prog. Energy Combust. Sci. 2005, 31, 123–170. [Google Scholar] [CrossRef]
- Wang, F.; Ren, T.; Tu, S.; Hungerford, F.; Aziz, N. Implementation of underground longhole directional drilling technology for greenhouse gas mitigation in Chinese coal mines. Int. J. Greenh. Gas Control 2012, 11, 290–303. [Google Scholar] [CrossRef]
- Zhou, F.; Xia, T.; Wang, X.; Zhang, Y.; Sun, Y.; Liu, J. Recent developments in coal mine methane extraction and utilization in China: A review. J. Nat. Gas Sci. Eng. 2016, 31, 437–458. [Google Scholar] [CrossRef]
- Cheng, W.; Liu, Z.; Yang, H.; Wang, W. Non-linear seepage characteristics and influential factors of water injection in gassy seams. Exp. Therm. Fluid Sci. 2018, 91, 41–53. [Google Scholar] [CrossRef]
- Ni, G.; Cheng, W.; Lin, B.; Zhai, C. Experimental study on removing water blocking effect (WBE) from two aspects of the pore negative pressure and surfactants. J. Nat. Gas Sci. Eng. 2016, 31, 596–602. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, S. Estimation of Pressure-Dependent Diffusive Permeability of Coal Using Methane Diffusion Coefficient: Laboratory Measurements and Modeling. Energy Fuels 2016, 30, 8968–8976. [Google Scholar] [CrossRef]
- Wang, G.; Wu, M.; Wang, R.; Xu, H.; Song, X. Height of the mining-induced fractured zone above a coal face. Eng. Geol. 2017, 216, 140–152. [Google Scholar] [CrossRef]
- Chu, T.; Yu, M.; Yang, S.; Xu, Q.; Meng, D.; Jia, H. Coupling between gas drainage and spontaneous combustion about close distance coal seams in U+II type workface. J. China Coal Soc. 2010, 35, 2–7. [Google Scholar]
- Szlazak, N.; Obracaj, D.; Swolkień, J. Methane drainage from roof strata using an overlying drainage gallery. Int. J. Coal Geol. 2014, 136, 99–115. [Google Scholar] [CrossRef]
- Huiming, Y.; Hu, L. Experimental research on coalbed gas drainage effect and economy of long directional borehole in roof. IOP Conf. Ser. Earth Environ. Sci. 2017, 64, 012012. [Google Scholar] [Green Version]
- Cheng, Z.; Bai, L.; Hai, W. Gas drainage with high level borehole in roof applied to gas control. Coal Eng. 2005, 9, 4–6. [Google Scholar]
- Sun, R.; Gang, W.; Zhang, W.; Liu, L. Experimental research on using approximate horizontal long—Hole instead of closed high extracted roadway to drain gas. Coal Geol. Explor. 2005, 33, 2–5. [Google Scholar]
- Lin, Z.; Peng, Y.; Zhou, Z.; Yun-long, Z. Application of directional drilling technology a long seam to Ningxia Mining Area. Coal Sci. Technol. 2008, 11, 47–51. [Google Scholar]
- Hungerford, F. An Assessment of the Status of Long-Hole in- Seam Drilling in Australia; Underground Coal Mining Exploration Techniques: Brisbane, Australia, 1991. [Google Scholar]
- Kroepsch, A.C. Horizontal drilling, changing patterns of extraction, and piecemeal participation: Urban hydrocarbon governance in Colorado. Energy Policy 2018. [Google Scholar] [CrossRef]
- Li, Y. Upper corner gas control based on high level directional long borehole. Coal Sci. Technol. 2018, 46, 215–213. [Google Scholar]
- An, F.; Wang, Z.; Yang, H.; Yang, S.; Pan, F.; Chen, T.; Xie, C. Application of directional boreholes for gas drainage of adjacent seams. Int. J. Rock Mech. Min. Sci. 2016, 90, 35–42. [Google Scholar] [CrossRef]
- Palchik, V. Bulking factors and extents of caved zones in weathered overburden of shallow abandoned underground workings. Int. J. Rock Mech. Min. Sci. 2015, 79, 227–240. [Google Scholar] [CrossRef]
- Xia, X.; Huang, Q. Study on the dynamic height of caved zone based on porosity. J. Min. Saf. Eng. 2014, 31, 102–107. [Google Scholar] [CrossRef]
- China Coal Research Institute Beijing Institute of Exploitation. Surface Movement and Overburden Failure of Coal Mines and Their Application; China Coal Industry Publishing House: Beijing, China, 1981. [Google Scholar]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Itasca Consulting Group Inc. PFC2D: Particle Flow Code in 2 Dimensions, version 5.0; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2017. [Google Scholar]
- Zou, Z.; Tang, H.; Xiong, C.; Su, A.; Criss, R.E. Kinetic characteristics of debris flows as exemplified by field investigations and discrete element simulation of the catastrophic Jiweishan rockslide, China. Geomorphology 2017, 295, 1–15. [Google Scholar] [CrossRef]
- Wang, T.; Zhou, W.; Chen, J.; Xiao, X.; Li, Y.; Zhao, X. Simulation of hydraulic fracturing using particle flow method and application in a coal mine. Int. J. Coal Geol. 2014, 121, 1–13. [Google Scholar] [CrossRef]







| Stratum | Lithology | Macroscopic Parameters | Mesoscopic Parameters | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Poisson’s Ratio | Elastic Modulus | Tensile Strength (MPa) | Cohesion (MPa) | Internal Friction Angle (°) | Krat | Emod (GPa) | Kn (GPa) | Ks (GPa) | Pb-kn (GPa) | Pb-ks (GPa) | ||
| J21 | Fine-grained sandstone | 0.15 | 7.09 | 4.66 | 11.05 | 39.4 | 1.2 | 4.5 | 9 | 7.4 | 5.6 | 4.7 |
| J20 | Siltstone | 0.23 | 18.1 | 1.36 | 6.32 | 36 | 1.76 | 12.7 | 25.4 | 14.3 | 15.8 | 9 |
| J19 | Mudstone | 0.24 | 8.91 | 4.66 | 11.05 | 36 | 1.76 | 6.3 | 13.3 | 7.3 | 8.3 | 4.3 |
| J18 | Siltstone | 0.24 | 8.91 | 3.06 | 5.77 | 36 | 1.76 | 6.3 | 13.3 | 7.3 | 8.3 | 4.3 |
| J17 | Fine-grained sandstone | 0.14 | 17.25 | 2.58 | 4.35 | 38.8 | 1.39 | 10.8 | 21.6 | 18.8 | 13.5 | 11.8 |
| J16 | Medium-grained sandstone | 0.18 | 15.36 | 1.36 | 6.32 | 35.3 | 1.39 | 10.1 | 20.2 | 14.5 | 12.6 | 9.1 |
| J15 | Mudstone | 0.15 | 7.1 | 1.6 | 7.6 | 39.4 | 1.2 | 4.5 | 9 | 7.46 | 5.6 | 4.6 |
| J14 | Fine-grained sandstone | 0.17 | 7.38 | 1.36 | 6.32 | 38.8 | 1.32 | 4.8 | 9.6 | 7.2 | 6 | 4.5 |
| J13 | Siltstone | 0.15 | 7.09 | 3.06 | 5.77 | 35.4 | 1.2 | 4.5 | 9 | 7.4 | 5.6 | 4.6 |
| J12 | Medium-grained sandstone | 0.14 | 17.25 | 1.36 | 6.32 | 38.8 | 1.14 | 10.8 | 21.6 | 18.8 | 13.5 | 11.8 |
| J11 | Fine-grained sandstone | 0.15 | 7.09 | 2.58 | 4.35 | 39.4 | 1.2 | 4.5 | 9 | 7.4 | 5.6 | 4.6 |
| J10 | Mudstone | 0.18 | 15.36 | 1.42 | 7.6 | 34.3 | 1.39 | 10.1 | 20.2 | 14.5 | 12.6 | 9 |
| J9 | Siltstone | 0.15 | 7.25 | 2.58 | 4.35 | 36.4 | 1.2 | 4.6 | 9.2 | 7.6 | 5.7 | 4.7 |
| J8 | Fine-grained sandstone | 0.18 | 15.36 | 1.6 | 7.6 | 35.3 | 1.39 | 10.1 | 20.2 | 14.5 | 12.6 | 9 |
| J7 | Siltstone | 0.15 | 7.25 | 1.33 | 7.6 | 35.4 | 1.2 | 4.6 | 9.2 | 7.6 | 5.7 | 4.7 |
| J6 | Fine-grained sandstone | 0.27 | 6.86 | 3.06 | 5.77 | 42.6 | 2.13 | 5.1 | 10.2 | 4.7 | 6.3 | 2.9 |
| J5 | Medium-grained sandstone | 0.15 | 7.25 | 1.33 | 6.32 | 35.4 | 1.2 | 4.6 | 9.2 | 7.6 | 5.7 | 4.7 |
| J4 | Mudstone | 0.27 | 6.86 | 2.58 | 4.35 | 42.6 | 2.13 | 5.1 | 10.2 | 4.7 | 6.3 | 2.9 |
| J3 | Coal seam 3 | 0.14 | 16.26 | 1.33 | 2.42 | 38 | 1.14 | 10.18 | 21.6 | 18.8 | 13.5 | 11.8 |
| J2 | Siltstone | 0.27 | 6.86 | 3.06 | 6.32 | 42.6 | 2.13 | 5.1 | 10.2 | 4.7 | 6.3 | 2.9 |
| J1 | Fine-grained sandstone | 0.18 | 15.36 | 4.66 | 11.58 | 35 | 1.39 | 10.1 | 20.2 | 14.5 | 12.6 | 9.1 |
| Quantity | Average Length (m) | Diameter (mm) | Distance of the Hole from the Drill Floor (m) | Spacing of Drilling Field in the Direction of Working Face (m) | Normal Suction Pressure (kPa) | |
|---|---|---|---|---|---|---|
| Roof high level boreholes | 5 | 90 | 96 | 2 | 50 | 45 |
| Roof long horizontal borehole | 2 | 570 | 96 | 2.5 | 550 | 45 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Fan, C.; Xu, H.; Liu, X.; Wang, R. Determination of Long Horizontal Borehole Height in Roofs and Its Application to Gas Drainage. Energies 2018, 11, 2647. https://doi.org/10.3390/en11102647
Wang G, Fan C, Xu H, Liu X, Wang R. Determination of Long Horizontal Borehole Height in Roofs and Its Application to Gas Drainage. Energies. 2018; 11(10):2647. https://doi.org/10.3390/en11102647
Chicago/Turabian StyleWang, Gang, Cheng Fan, Hao Xu, Xuelin Liu, and Rui Wang. 2018. "Determination of Long Horizontal Borehole Height in Roofs and Its Application to Gas Drainage" Energies 11, no. 10: 2647. https://doi.org/10.3390/en11102647
APA StyleWang, G., Fan, C., Xu, H., Liu, X., & Wang, R. (2018). Determination of Long Horizontal Borehole Height in Roofs and Its Application to Gas Drainage. Energies, 11(10), 2647. https://doi.org/10.3390/en11102647






