Numerical Simulation of Fluid Flow through Fractal-Based Discrete Fractured Network
Abstract
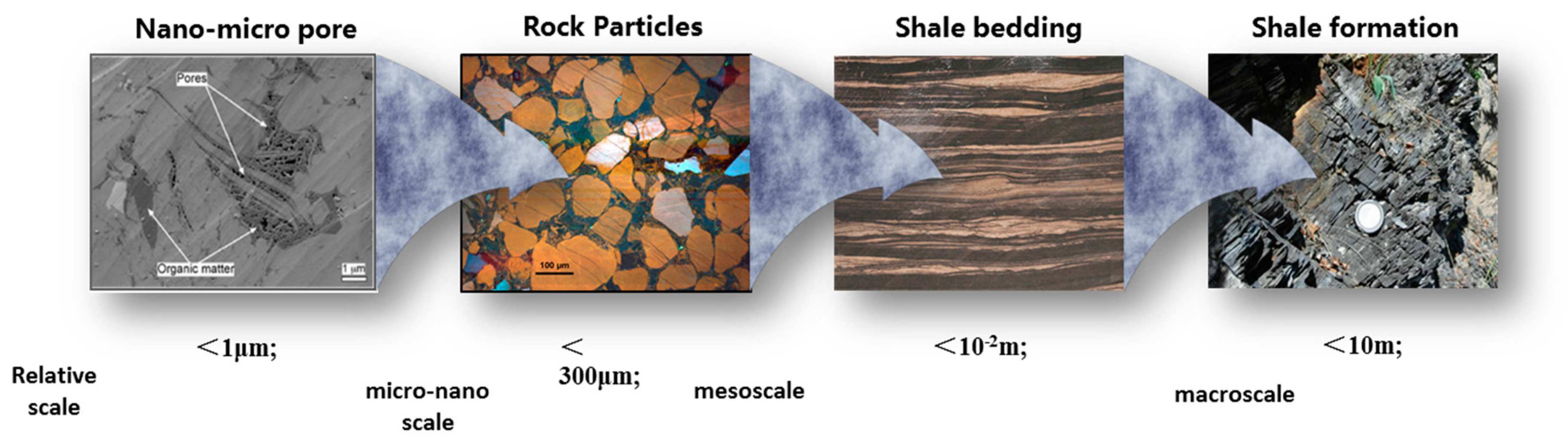
:1. Introduction
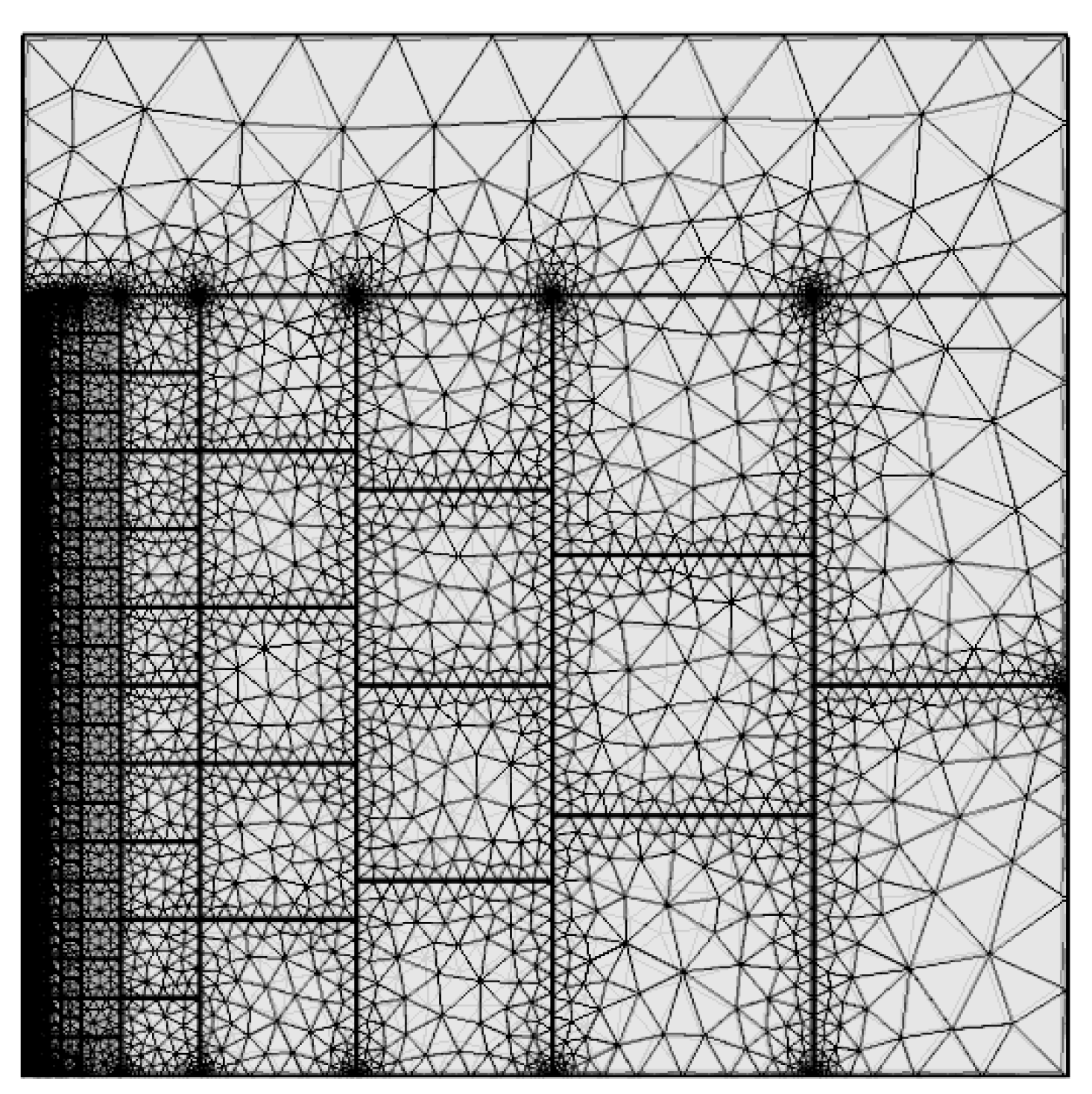
2. Discrete Fractal-Fracture Network (DFFN)
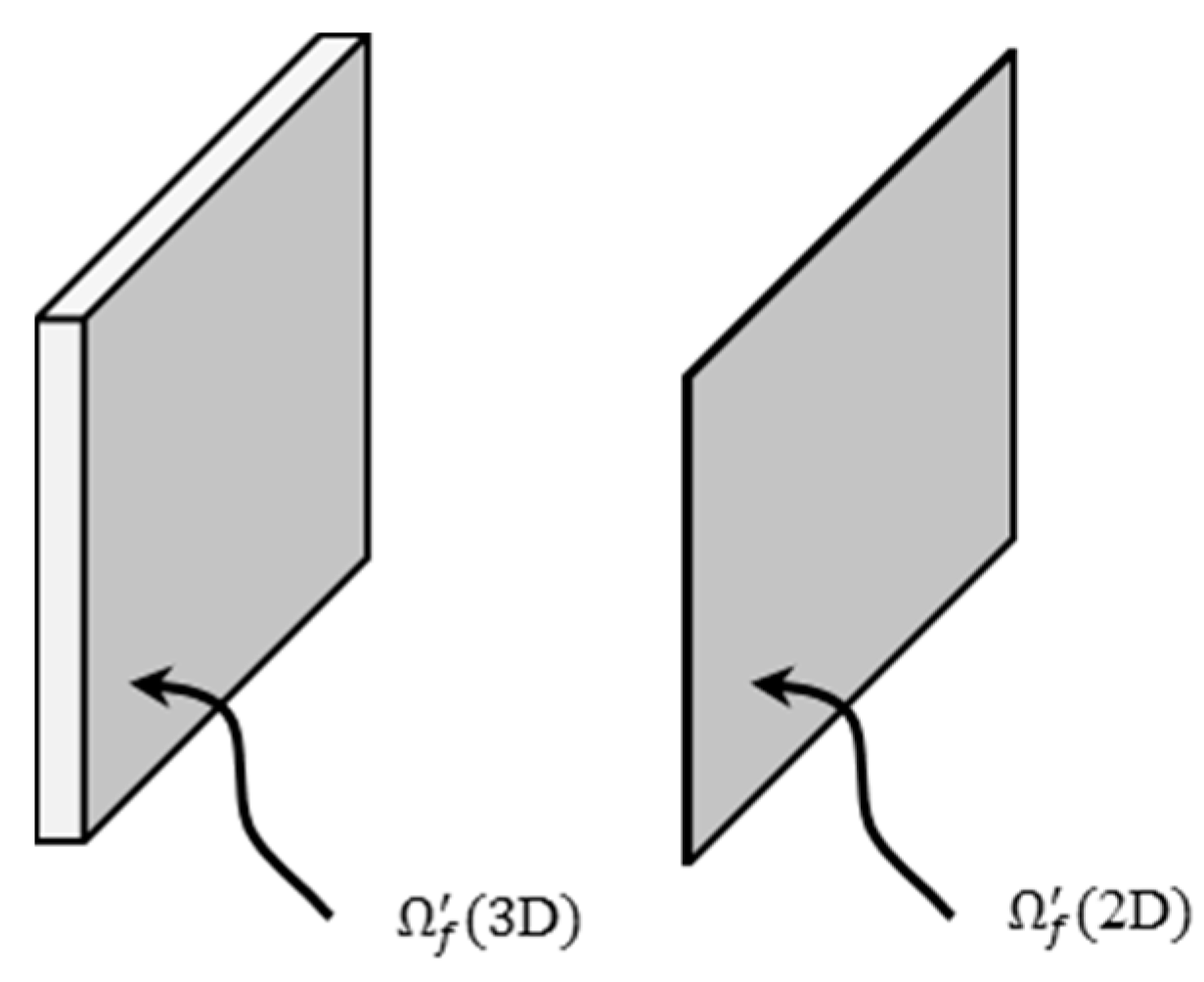
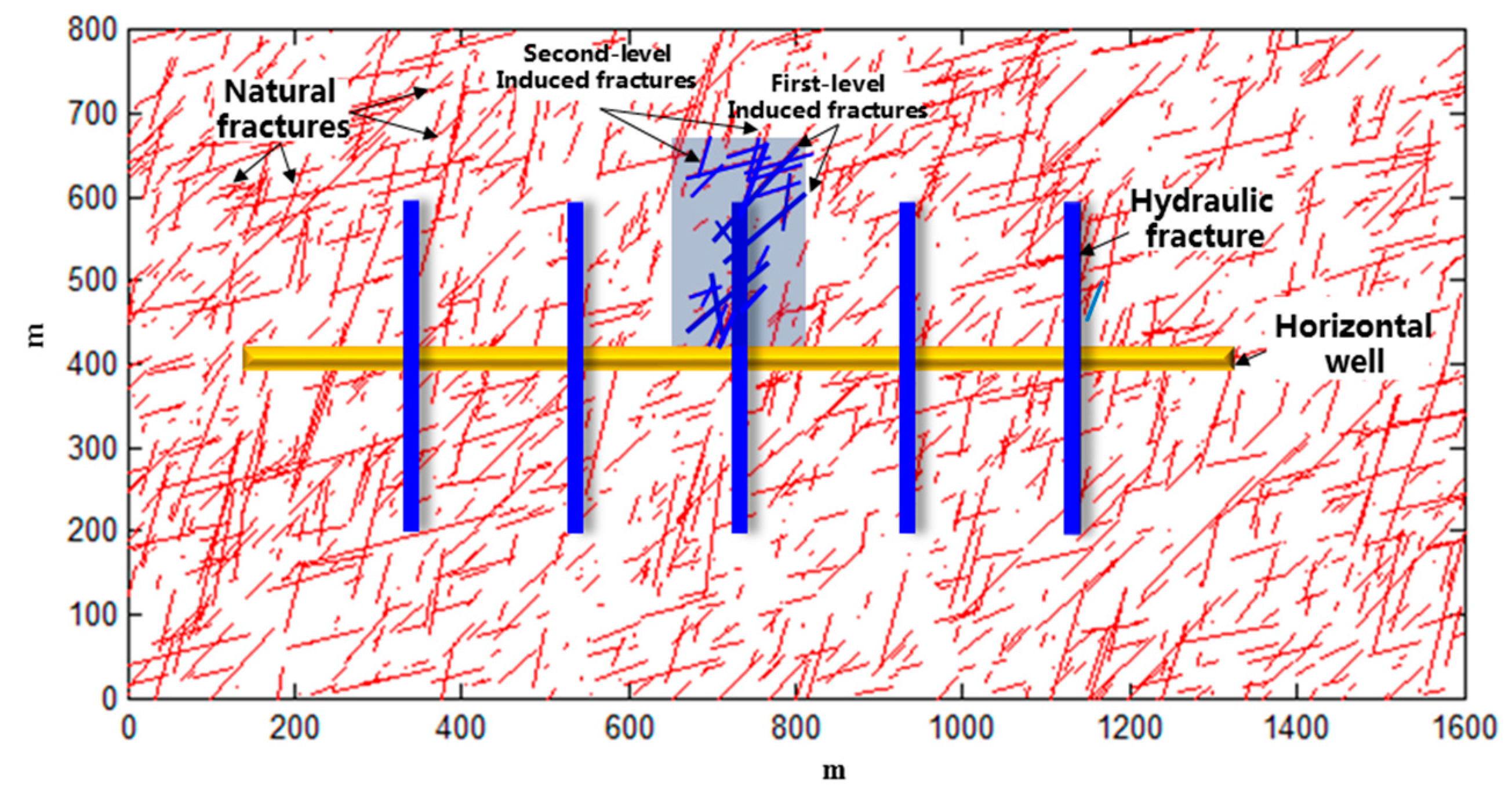
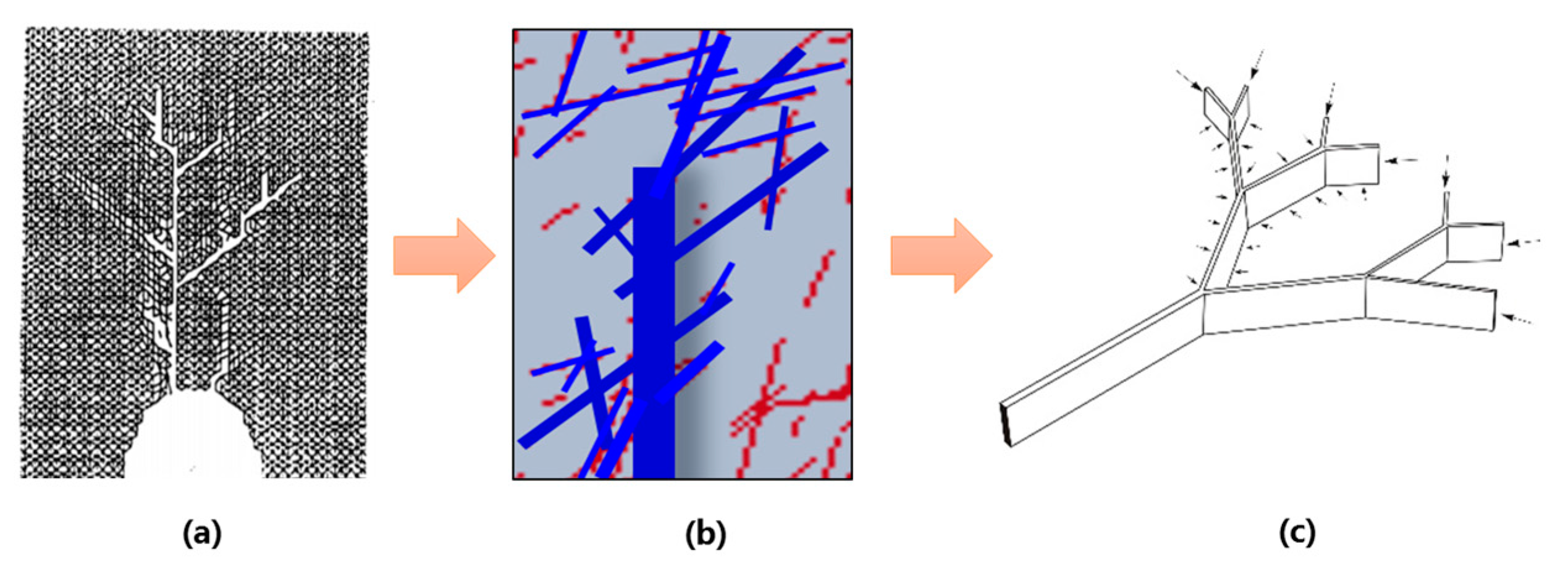
2.1. Physical Model
2.2. Mathematical Model of Discrete Fractal-Fracture Network
2.2.1. Flow in the Matrix
2.2.2. Flow in Complex Fracture Network
2.2.3. Flow in the Hydraulic Fracture
3. Results and Discussion
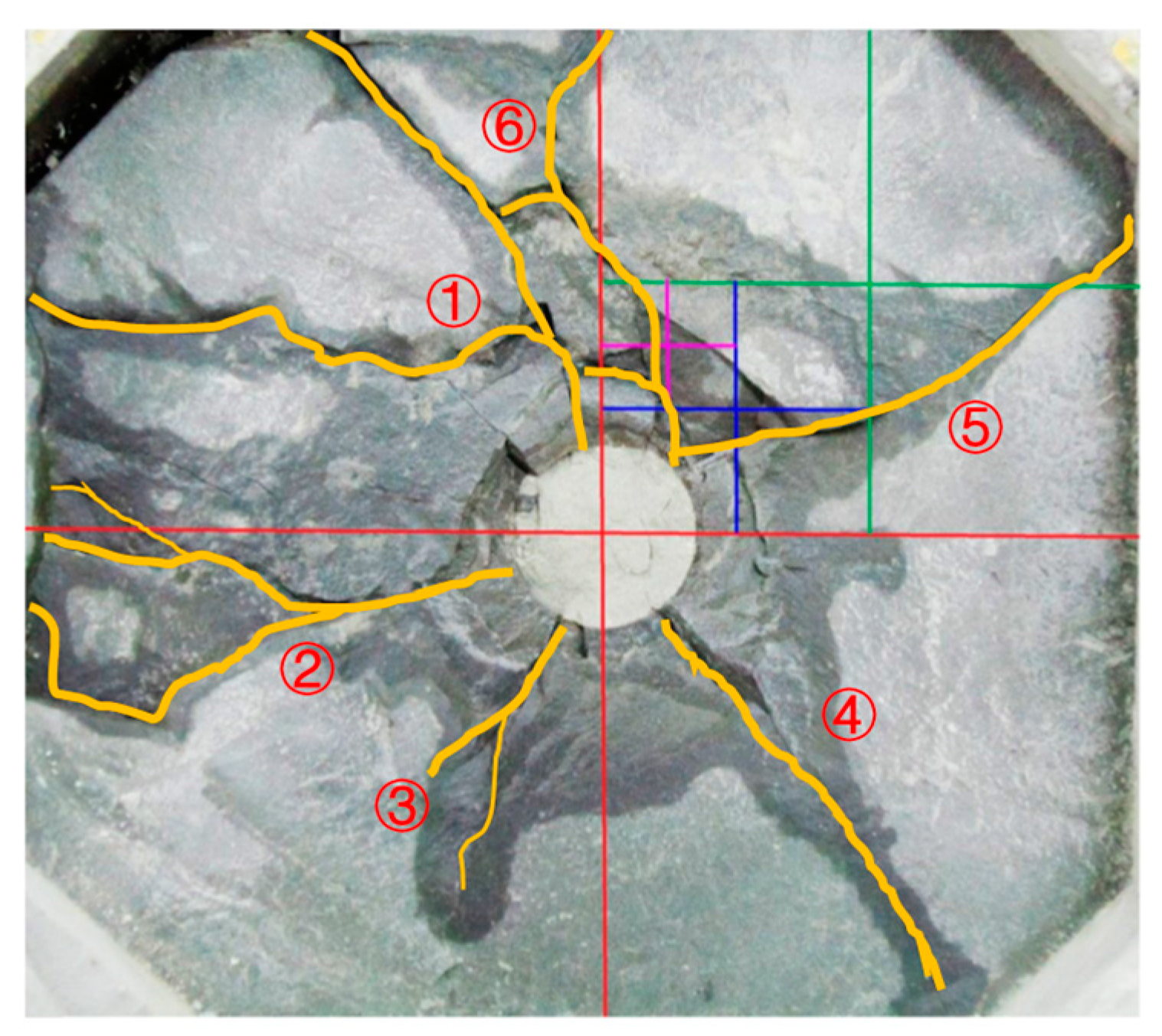
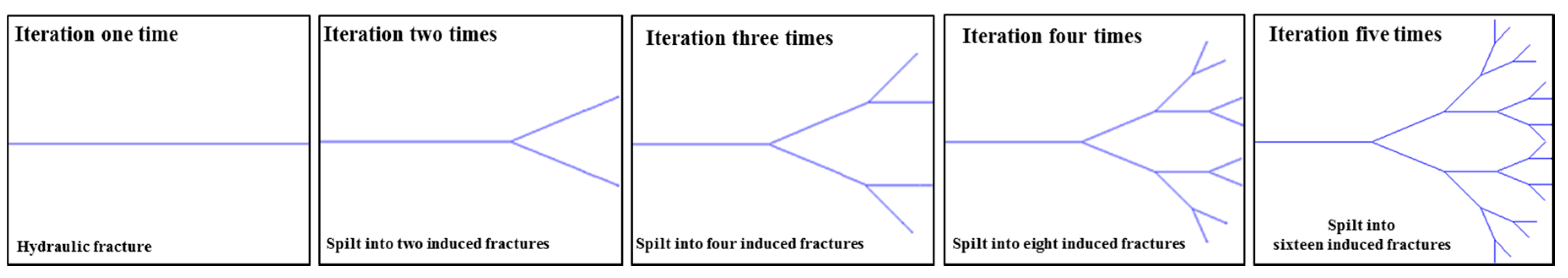
3.1. Fractal Fracture Network Pattern
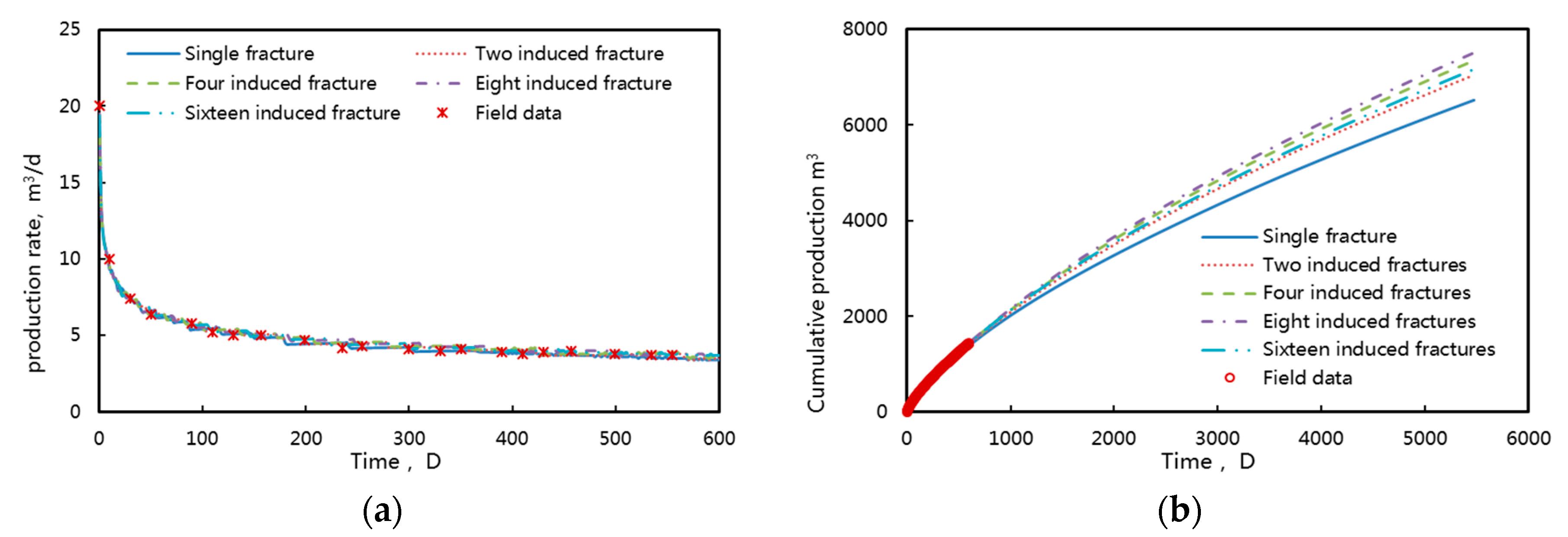
3.2. Multi-Scale Fractal Fracture Network Conductivity
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| L | Lateral length of horizontal well, m |
| N | Directions, x, y, and z |
| Distance to boundary in x-direction, m | |
| Distance to boundary in y-direction, m | |
| Dimensionless distance to x boundary | |
| Dimensionless distance to y boundary | |
| Permeability of reservoir matrix, m | |
| Permeability of fracture networks in SRV, mD | |
| Permeability of hydraulic fractures, mD | |
| Dimensionless permeability of reservoir matrix | |
| Dimensionless permeability of fracture networks in SRV | |
| Dimensionless permeability of hydraulic fractures | |
| Time, s | |
| Dimensionless time | |
| Dimensionless pressure | |
| Flow capacity coefficient | |
| Storability ratio | |
| Fluid flow velocity tensor in reservoir matrix, 10−3 m/s | |
| Original reservoir pressure, MPa | |
| Pore pressure of reservoir matrix, MPa | |
| Pressure in hydraulic fractures, MPa | |
| Pressure in complex fracture network system, MPa | |
| Fluid viscosity, mPa·s | |
| Reservoir porosity | |
| Original reservoir porosity | |
| Natural fractures porosity | |
| Fluid density, kg/m3 | |
| Original fluid density, kg/m3 | |
| Pore compressibility coefficient, MPa−1 | |
| Natural fracture compressibility coefficient, MPa−1 | |
| Hydraulic fracture compressibility coefficient, MPa−1 | |
| Fluid compressibility coefficient, MPa−1 | |
| volume flow rate in unit volume, s−1 | |
| Sink/source term in complex fracture system, s−1 | |
| Sink/source flow term in hydraulic fracture, s−1 | |
| Delta function. It equals to 1 when M = M’, otherwise, it equals to zero. |
Appendix A. Numerical Solution of Mathematical Model
References
- Bin, Y.; Moghanloo, R.G.; Da, Z. A novel integrated production analysis workflow for evaluation, optimization and predication in shale plays. Int. J. Coal Geol. 2017, 180, 18–28. [Google Scholar]
- Zhiyuan, W.; Baojiang, S.; Xiaohui, S. Calculation of temperature in fracture for carbon dioxide fracturing. SPE J. 2016, 12, 1491–1500. [Google Scholar]
- Warren, J.E.; Root, P.J. The behavior of naturally fractured reservoirs. SPE J. 1963, 3, 245–255. [Google Scholar] [CrossRef]
- Du, C.M.; Zhang, X.; Zhan, L.; Gu, H.; Hay, B.; Tushingham, K.; Ma, Y.Z. Modeling hydraulic fracturing induced fracture networks in shale gas reservoirs as a dual porosity system. In Proceedings of the Society of Petroleum Engineers International Oil and Gas Conference and Exhibition in China, Beijing, China, 8–10 June 2010. [Google Scholar]
- Li, J.; Du, C.; Zhang, X. Critical evaluation of shale gas reservoir simulation approaches: Single-porosity and dual-porosity modeling. In Proceedings of the Society of Petroleum Engineers SPE Middle East Unconventional Gas Conference and Exhibition, Muscat, Oman, 31 January–2 February 2011. [Google Scholar]
- Zimmerman, R.W.; Chen, G.; Hadgu, T.; Bodvarsson, G.S. Numerical dual-porosity model with a semi-analytical treatment of fracture/matric flow. Water Resour. Res. 1993, 29, 2127–2135. [Google Scholar] [CrossRef]
- Chong, Z.; Li, X.; Chen, X.; Zhang, J.; Lu, J. Numerical investigation into the effect of natural fracture density on hydraulic fracture network propagation. Energies 2017, 10, 914. [Google Scholar] [CrossRef]
- Chaudhary, A.S.; Ehlig-Economides, C.A.; Wattenbarger, R.A. Shale oil production performance from a stimulated reservoir volume. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 30 October–2 November 2011. [Google Scholar]
- Agboada, D.; Ahmadi, M. Production decline and numerical simulation model analysis of the Eagle Ford shale play. In Proceedings of the SPE Western Regional & AAPG Pacific Section Meeting Joint Technical Conference, Monterey, CA, USA, 19–25 April 2013. [Google Scholar]
- Odunuga, A.B. Investigating Drainage Patterns of Shale Production as a Function of Type Fracture System in the Reservoir; University of Oklahoma: Norman, OK, USA, 2012. [Google Scholar]
- Zhang, X.; Du, C.; Deimbacher, F.; Crick, M.; Harikesavanallur, A. Sensitivity studies of horizontal wells with hydraulic fractures in shale gas reservoirs. In Proceedings of the International Petroleum Technology Conference, Doha, Qatar, 7–9 December 2009. [Google Scholar]
- Cipolla, C.L.; Lolon, E.; Mayerhofer, M.J. Reservoir modeling and production evaluation in shale-gas reservoirs. In Proceedings of the International Petroleum Technology Conference, Doha, Qatar, 7–9 December 2009. [Google Scholar]
- Daobing, W.; Hongkui, G.; Xiaoqiong, W.; Jianbo, W.; Fanbao, M.; Yu, S.; Peng, H. A novel experimental approach for fracability evaluation in tight volcanic rock gas reservoirs. J. Nat. Gas Sci. Eng. 2014, 23, 239–249. [Google Scholar]
- Carlson, E.S.; Latham, G.V. Discrete Network Modeling for Tight Gas Fractured Reservoirs; Society of Petroleum Engineers: Richardson, TX, USA, 1993. [Google Scholar]
- Karimi-Fard, M.; Durlofsky, L.J.; Aziz, K. An efficient discrete-fracture model applicable for general-purpose reservoir simulators. SPE J. 2004, 9, 227–236. [Google Scholar] [CrossRef]
- Xu, W.; Thierceiin, M.J.; Ganguly, U. Wiremesh: A novel shale fracturing simulator. In Proceedings of the International Oil and Gas Conference and Exhibition in China, Beijing, China, 8–10 June 2010. [Google Scholar]
- Weng, X.; Kresse, O.; Cohen, C.E.; Wu, R.; Gu, H. Modeling of hydraulic fracture network propagation in a naturally fractured formation. SPE Prod. Oper. 2011, 26, 368–380. [Google Scholar] [CrossRef]
- Guo, T.; Zhang, S.; Ge, H. A new method for evaluating ability of forming fracture network in shale reservoir. Rock Soil Mech. 2013, 34, 947–954. (In Chinese) [Google Scholar]
- Al-Obaidy, R.T.I.; Gringarten, A.C.; Sovetkin, V. Modeling of Induced Hydraulically Fractured Wells in Shale Reservoirs Using “Branched” Fractals. In Proceedings of the SPE Annual Technical Conference and Exhibition, Amsterdam, The Netherlands, 27–29 October 2014. [Google Scholar]
- Mhiri, A.; Blasingame, T.A.; Moridis, G.J. Stochastic Modeling of a Fracture Network in a Hydraulically Fractured Shale-Gas Reservoir. In Proceedings of the SPE Annual Technical Conference and Exhibition, Houston, TX, USA, 28–30 September 2015. [Google Scholar]
- Wang, F.; Liu, Z.; Jiao, L.; Wang, C.; Guo, H. A Fractal Permeability Model Coupling Boundary-layer Effect for Tight Oil Reservoirs. Fractals 2017, 25, 1750042. [Google Scholar] [CrossRef]
- Xia, Y.; Cai, J.; Wei, W.; Hu, X.; Wang, X.; Ge, X. A New Method For Calculating Fractal Dimensions of Porous Media Based On Pore Size Distribution. Fractals 2017. [Google Scholar] [CrossRef]
- Huang, J.-I.; Kim, K. Fracture process zone development during hydraulic fracturing. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 1295–1298. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; He, J.; Zhao, Z.; Zheng, B. Investigation of fracturing network propagation in random naturally fractured and laminated block experiments. Energies 2016, 9, 588. [Google Scholar] [CrossRef]
- Bin, Y. Nanofluid pre-treatment, an effective strategy to improve the performance of low-salinity waterflooding. J. Pet. Sci. Eng. 2017. [Google Scholar] [CrossRef]
- Zhao, Y.; Shan, B.C.; Zhang, L.H. Seepage flow behaviors of multi-stage fractured horizontal wells in arbitrary shaped shale gas reservoirs. J. Pet. Sci. Eng. 2016, 13, 1–10. [Google Scholar] [CrossRef]
- Xu, P.; Yu, B.; Feng, Y.; Liu, Y. Analysis of permeability for the fractal-like tree network by parallel and series models. Phys. A Stat. Mech. Appl. 2006, 369, 884–894. [Google Scholar] [CrossRef]
- Wang, W.; Su, Y.; Zhang, X.; Sheng, G. Analysis of the complex fracture flow in multiple fractured horizontal wells with the fractal tree-like network models. Fractals Complex Geom. Patterns Scaling Nat. Soc. 2015, 23, 1550014. [Google Scholar] [CrossRef]
- Yuan, B.; Moghanloo, R.G.; Wang, W. Using nanofluids to control fines migration for oil recovery: Nanofluids co-injection or nanofluids pre-flush? A comprehensive answer. Fuel 2018, 215, 474–483. [Google Scholar] [CrossRef]
- Mayerhofer, M.J.; Lolon, E.; Warpinski, N.R.; Cipolla, C.L.; Walser, D.W.; Rightmire, C.M. What is stimulated reservoir volume? SPE Prod. Oper. 2010, 25, 89–98. [Google Scholar] [CrossRef]
- Zhang, T.; Li, Z.; Adenutsi, C.D.; Caspar, D.A.; Li, F. A new model for calculating permeability of natural fractures in dual-porosity reservoir. Adv. Geo-Energy Res. 2017, 1, 86–92. [Google Scholar] [CrossRef]
- Nezhad, M.M.; Javadi, A.A. Stochastic finite element approach to quantify and reduce uncertainty in pollutant transport modeling. ASCE J. Hazard. Toxic Radioact. Waste Manag. 2011, 15, 208–215. [Google Scholar] [CrossRef]
- Nezhad, M.M.; Javadi, A.A.; Rezania, M. Finite element modelling of contaminant transport considering effects of micro and macro heterogeneity of soil. J. Hydrol. 2011, 404, 332–338. [Google Scholar] [CrossRef]
- Niessner, J.; Helmig, R. Multi-physics modeling of flow and transport in porous media using a downscaling approach. Adv. Water Resour. 2009, 32, 845–850. [Google Scholar] [CrossRef]

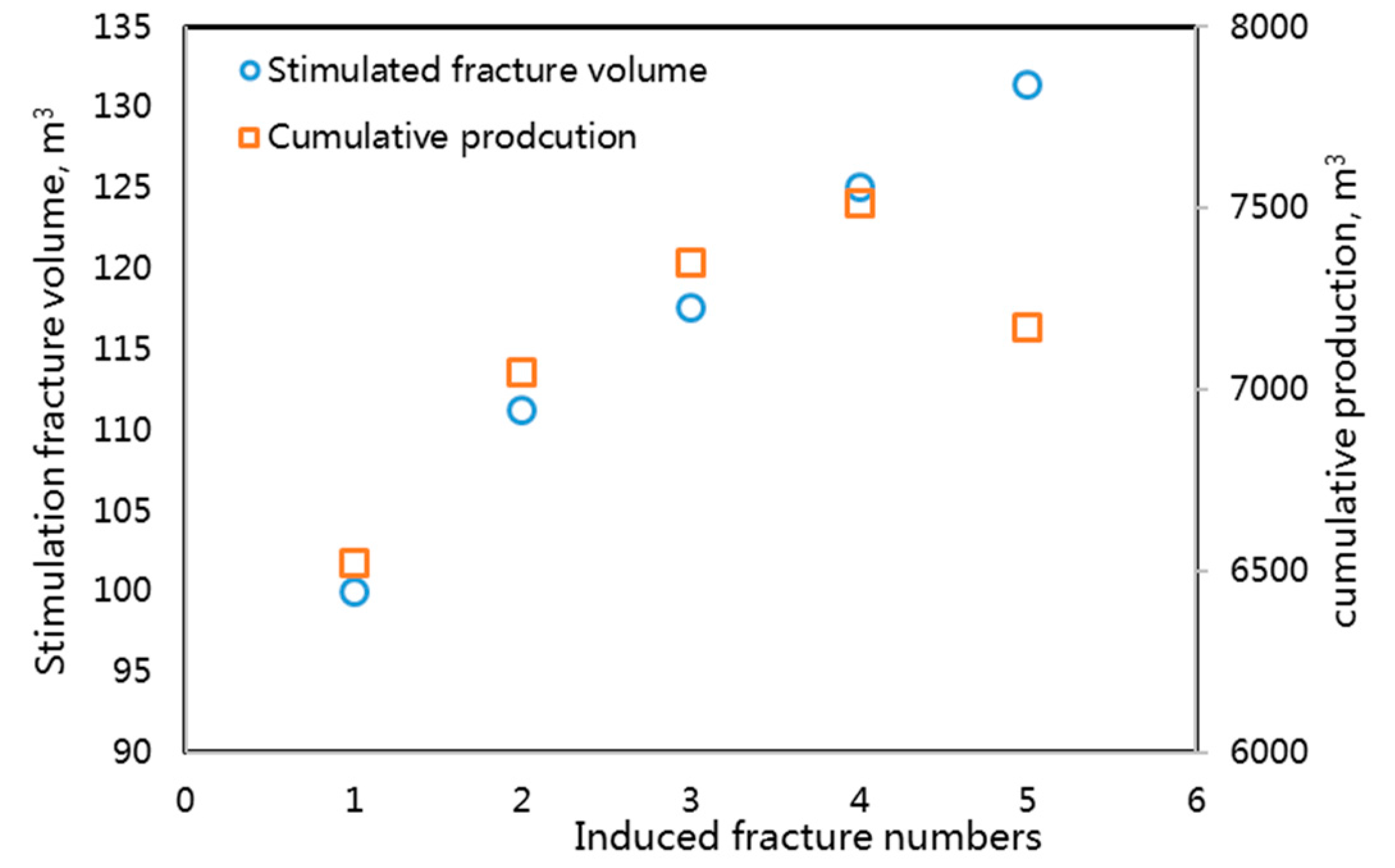

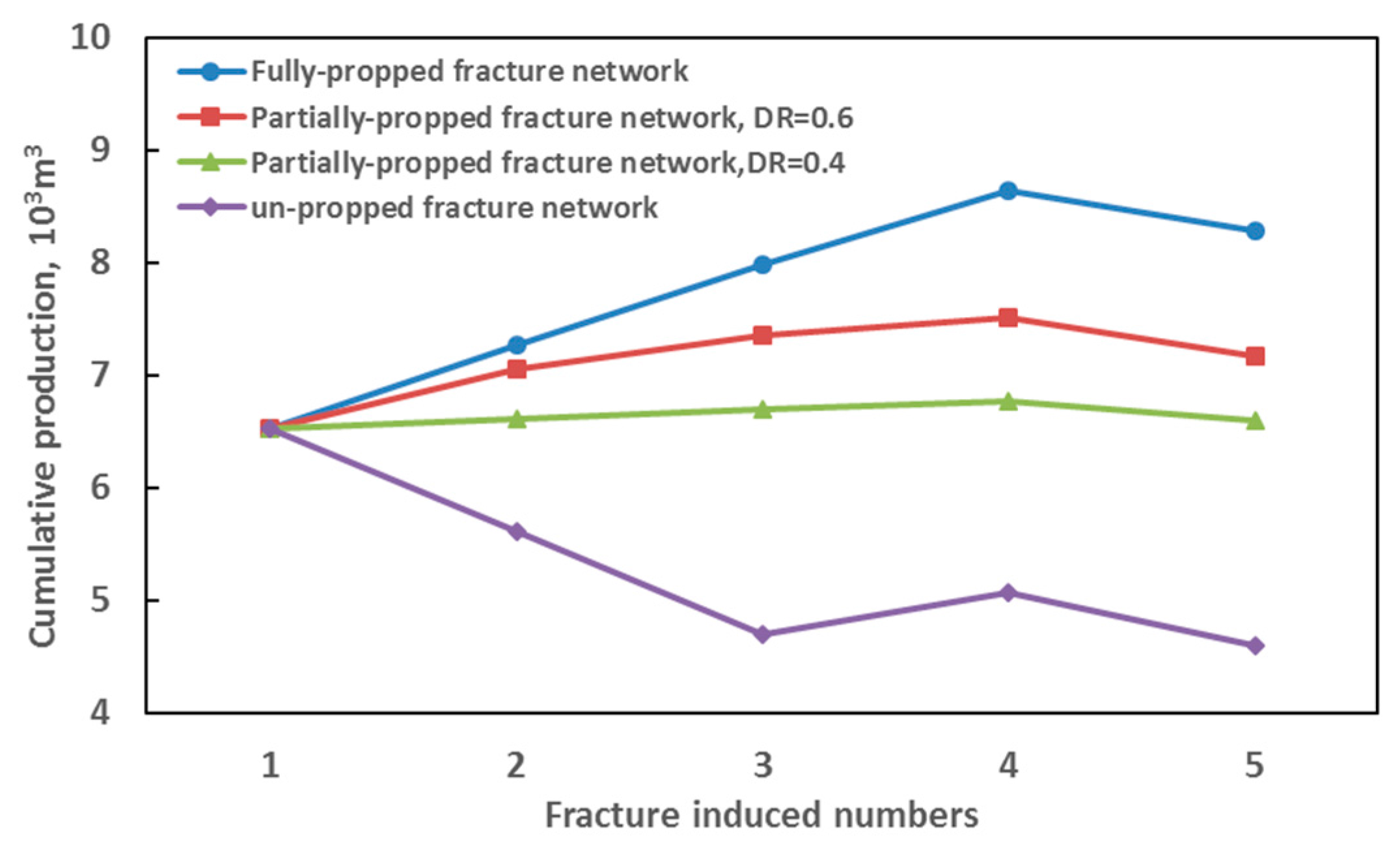

| Parameter, Symbol, Unit | Value |
|---|---|
| Fracture unit in the x, m | 300 |
| Fracture unit in the y, m | 200 |
| Reservoir thickness, m | 19 m |
| Matrix permeability, D | 2.3 × 10−4 |
| Matrix porosity | 0.108 |
| Matrix compressibility, pa−1 | 3.75 × 10−10 |
| Main fracture conductivity, D·cm | 200 |
| Main fracture porosity | 0.000015 |
| Fracture compressibility, pa−1 | 3.75 × 10−8 pa−1 |
| Scenario | Induced Fracture Permeability, D (Fracture Width) | Schematic Plot | |||||
|---|---|---|---|---|---|---|---|
| Main (5 cm) | Second (3 cm) | Third (1.8 cm) | Fourth (1.08 cm) | Fifth (0.648 cm) | |||
| Fully-propped fracture network | 2 | 2 | 2 | 2 | 2 |  | |
| Partially-propped fracture network | DR * = 0.6 | 2 | 1.2 | 0.72 | 0.432 | 0.259 |  |
| DR = 0.4 | 2 | 0.8 | 0.32 | 0.128 | 0.0512 |  | |
| Un-propped fracture network | 2 | 0.0512 | 0.0512 | 0.0512 | 0.0512 |  | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Su, Y.; Yuan, B.; Wang, K.; Cao, X. Numerical Simulation of Fluid Flow through Fractal-Based Discrete Fractured Network. Energies 2018, 11, 286. https://doi.org/10.3390/en11020286
Wang W, Su Y, Yuan B, Wang K, Cao X. Numerical Simulation of Fluid Flow through Fractal-Based Discrete Fractured Network. Energies. 2018; 11(2):286. https://doi.org/10.3390/en11020286
Chicago/Turabian StyleWang, Wendong, Yuliang Su, Bin Yuan, Kai Wang, and Xiaopeng Cao. 2018. "Numerical Simulation of Fluid Flow through Fractal-Based Discrete Fractured Network" Energies 11, no. 2: 286. https://doi.org/10.3390/en11020286
APA StyleWang, W., Su, Y., Yuan, B., Wang, K., & Cao, X. (2018). Numerical Simulation of Fluid Flow through Fractal-Based Discrete Fractured Network. Energies, 11(2), 286. https://doi.org/10.3390/en11020286





