Study of the Dynamics of a Single Bubble
Abstract
:1. Introduction
- -
- approximate analytical solutions. This approach involves simplifying assumptions, often with questionable physical justifications, to derive approximate analytical estimates.
- -
- numerical solutions. This approach involves the numerical solution of the original equations. The advancement of computer technology in recent years has undoubtedly contributed to a more detailed study of steam bubble dynamics, as it enables the numerical solution of even highly complex systems of equations with high accuracy in a relatively short time [68,69,70].
2. Factors Affecting the Behaviour of Cavitation Bubbles
2.1. Bubble Surface Motion and Rayleigh Equation
2.2. Pressure and Density in the Bubble
2.3. Heat and Mass Transfer across a Phase Interface
2.4. Heat Transfer in the Liquid Phase
2.5. Impact of External Pressure Changes on Bubble Dynamics
3. Discussion
- Thermophysical property-based approach. This method emphasises the temperature-dependent thermophysical properties of the bubble and surrounding liquid. The energy equation is employed to capture the temperature distribution and its influence on bubble behaviour. This approach provides a detailed representation of heat transfer and temperature effects within the bubble.
- Vapour pressure and temperature approach. In this approach, the focus is on determining the vapour pressure and corresponding temperature within the bubble. The energy equation is not explicitly used, simplifying the model’s equations but potentially affecting the accuracy of numerical predictions. This approach offers computational efficiency, but may compromise the accuracy of heat transfer simulations.
- Rayleigh equation approach. This method utilises the Rayleigh equation to calculate the current bubble radius based on the velocity of the phase boundary interface. It is primarily applied to the inertial stage of bubble dynamics, where inertia and pressure forces dominate the bubble’s motion. This approach is well-suited to capturing the initial growth and collapse of bubbles.
- Modified Rayleigh–Plesset equation approach. This approach employs a modified form of the Rayleigh–Plesset equation to determine the bubble radius during the dynamic stage of bubble dynamics. It accounts for the influence of heat and mass transfer on bubble behaviour. This approach provides a more comprehensive representation of bubble dynamics, including heat and mass transfer effects during the collapse and rebound stages.
- Bubble cluster dynamics approach. This method focusses on modelling the dynamics of bubble clusters, considering the interactions and collective behaviour of multiple bubbles within a cluster. The aim of this is to capture the influence of neighbouring bubbles on the overall dynamics of the cluster. This approach is particularly relevant for applications involving cavitation, where bubble clusters play a significant role.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| R | radius |
| τ | time |
| c | specific heat |
| λ | surface tension |
| j | mass flow |
| L | latent heat of vaporisation (condensation) |
| m | mass |
| p | pressure |
| q | specific heat flux |
| r | radial coordinate |
| T | temperature |
| wr | radial velocity |
| ρ | density |
| σ | interfacial tension coefficient |
| Indexes | |
| l | liquid |
| 0 | initial value |
| b | boiling |
| c | continuous phase |
| R | value of the parameter at the boundary with the bubble |
| sat | parameter value in saturation condition |
| s | vapour |
References
- Stringer, M.; Zeng, Z.; Zhang, X.; Chai, Y.; Li, W.; Zhang, J.; Ong, H.; Liang, D.; Dong, J.; Li, Y.; et al. Methodologies, technologies, and strategies for acoustic streaming-based acoustofluidics. Appl. Phys. Rev. 2023, 10, 011315. [Google Scholar] [CrossRef]
- Kumar, C.; Hejazian, M.; From, C.; Saha, S.C.; Sauret, E.; Gu, Y.; Nguyen, N.-T. Modeling of mass transfer enhancement in a magnetofluidic micromixer. Phys. Fluids 2019, 31, 063603. [Google Scholar] [CrossRef]
- Alcántara Avila, J.R.; Kong, Z.Y.; Lee, H.-Y.; Sunarso, J. Advancements in Optimization and Control Techniques for Intensifying Processes. Processes 2021, 9, 2150. [Google Scholar] [CrossRef]
- Gawande, G.; Mali, P.; Dhavane, P.; Nahata, Y. Hydrodynamic Cavitation—A Promising Technology for Water Treatment. Mater. Today Proc. 2024, 98. [Google Scholar] [CrossRef]
- Wang, B.; Su, H.; Zhang, B. Hydrodynamic cavitation as a promising route for wastewater treatment—A review. Chem. Eng. J. 2021, 412, 128685. [Google Scholar] [CrossRef]
- Zhang, K.; Zheng, J.; Xu, Y.; Liao, Z.; Huang, Y.; Lu, L. Enhanced fabrication of size-controllable chitosan-genipin nanoparticles using orifice-induced hydrodynamic cavitation: Process optimization and performance evaluation. Ultrason. Sonochemistry 2024, 106, 106899. [Google Scholar] [CrossRef]
- Tian, Z.; Shang, Q.; Pan, X.; Zhang, R.; Hua, M.; Zhao, Y.; Jiang, J. Experimental study on explosive boiling mechanism of superheated liquid containing ethanol impurities under rapid depressurization. Process Saf. Environ. Prot. 2022, 168, 443–453. [Google Scholar] [CrossRef]
- Pan, X.; He, L.; Tian, Z.; Zhang, R.; Hua, M.; Fang, Y.; Jiang, J. Experimental research on the explosion boiling mechanism of superheated liquids containing short chain alcohol impurities with different boiling points. Appl. Therm. Eng. 2024, 238, 121998. [Google Scholar] [CrossRef]
- Gao, W.; Qi, J.; Zhang, J.; Chen, G.; Wu, D. An experimental study on explosive boiling of superheated droplets in vacuum spray flash evaporation. Int. J. Heat Mass Transf. 2019, 144, 118552. [Google Scholar] [CrossRef]
- Pavlenko, A.M.; Koshlak, H. Application of Thermal and Cavitation Effects for Heat and Mass Transfer Process Intensification in Multicomponent Liquid Media. Energies 2021, 14, 7996. [Google Scholar] [CrossRef]
- Pavlenko, A. Energy conversion in heat and mass transfer processes in boiling emulsions. Therm. Sci. Eng. Prog. 2020, 15, 100439. [Google Scholar] [CrossRef]
- Pavlenko, A.M. Dispersed phase breakup in boiling of emulsion. Heat Transf. Res. 2018, 49, 633–641. [Google Scholar] [CrossRef]
- Pavlenko, A.M. Change of emulsion structure during heating and boiling. Int. J. Energy A Clean Environ. 2019, 20, 291–302. [Google Scholar] [CrossRef]
- Plesset, M.S.; Prosperetti, A. Bubble dynamics and cavitation. Annu. Rev. Fluid Mech. 1977, 9, 145–185. [Google Scholar] [CrossRef]
- Singhal, A.K.; Athavale, M.M.; Li, H.; Jiang, Y. Mathematical Basis and Validation of the Full Cavitation Model. ASME J. Fluids Eng. 2002, 124, 617–624. [Google Scholar] [CrossRef]
- Zwart, P.; Gerber, A.; Belamr, T. A Two-Phase Flow Model for Predicting Cavitation Dynamics. In Multiphase flow, Proceedings of the ICMF 2004 International Conference on Multiphase Flow, Yokohama, Japan, 30 May–3 June 2004; Yokohama Pacifico Conference Center: Yokohama, Japan; p. 2, Paper No. 152.
- Abu-Nab, A.K.; Hakami, A.M.; Abu-Bakr, A.F. Charged Cavitation Multibubbles Dynamics Model: Growth Process. Mathematics 2024, 12, 569. [Google Scholar] [CrossRef]
- Cogné, C.; Labouret, S.; Peczalski, R.; Louisnard, O.; Baillon, F.; Espitalier, F. Theoretical model of ice nucleation induced by acoustic cavitation. Part 1: Pressure and temperature profiles around a single bubble. Ultrason. Sonochemistry 2016, 29, 447–454. [Google Scholar] [CrossRef]
- Podbevšek, D.; Lokar, Ž.; Podobnikar, J.; Petkovšek, R.; Dular, M. Experimental evaluation of methodologies for single transient cavitation bubble generation in liquids. Exp. Fluids 2021, 62, 167. [Google Scholar] [CrossRef]
- Dular, M.; Coutier-Delgosha, O. Thermodynamic effects during growth and collapse of a single cavitation bubble. J. Fluid Mech. 2013, 736, 44–66. [Google Scholar] [CrossRef]
- Lam, J.; Lombard, J.; Dujardin, C.; Ledoux, G.; Merabia, S.; Amans, D. Dynamical study of bubble expansion following laser ablation in liquids. Appl. Phys. Lett. 2016, 108, 074104. [Google Scholar] [CrossRef]
- Mancas, S.C.; Rosu, H.C. Evolution of spherical cavitation bubbles: Parametric and closed-form solutions. Phys. Fluids 2016, 28, 022009. [Google Scholar] [CrossRef]
- Plesset, M.; Zwick, A. The growth of vapor bubbles in superheated liquids. J. Appl. Phys. 1954, 25, 493–500. [Google Scholar] [CrossRef]
- Prosperetti, A.; Plesset, M.S. Vapour-bubble growth in a superheated liquid. J. Fluid Mech. 1978, 85, 349–368. [Google Scholar] [CrossRef]
- Li, J.; Carrica, P.M. A population balance cavitation model. Int. J. Multiph. Flow 2021, 138, 103617. [Google Scholar] [CrossRef]
- Ghahramani, E.; Ström, H.; Bensow, R. Numerical simulation and analysis of multi-scale cavitating flows. J. Fluid Mech. 2021, 922, A22. [Google Scholar] [CrossRef]
- Dumond, J.J.; Magagnato, F.; Class, A. Stochastic-field cavitation model. Phys. Fluids 2013, 25, 073302. [Google Scholar] [CrossRef]
- Denner, F.; Evrard, F.; van Wachem, B. Modeling Acoustic Cavitation Using a Pressure-Based Algorithm for Polytropic Fluids. Fluids 2020, 5, 69. [Google Scholar] [CrossRef]
- Wang, Z.; Li, L.; Li, X.; Zhu, Z.; Yang, S.; Yang, G. Investigation on multiscale features of cavitating flow in convergent-divergent test section using Eulerian–Lagrangian method. Int. J. Mech. Sci. 2023, 238, 107853. [Google Scholar] [CrossRef]
- Budich, B.; Schmidt, S.J.; Adams, N.A. Numerical simulation and analysis of condensation shocks in cavitating flow. J. Fluid Mech. 2018, 838, 759–813. [Google Scholar] [CrossRef]
- Asnaghi, A.; Svennberg, U.; Bensow, R.E. Numerical and experimental analysis of cavitation inception behaviour for high-skewed low-noise propellers. Appl. Ocean. Res. 2018, 79, 197–214. [Google Scholar] [CrossRef]
- Kinzel, M.P.; Lindau, J.W.; Kunz, R.F. An Evaluation of CFD Cavitation Models using Streamline Data. In Proceedings of the 10th International Symposium on Cavitation (CAV2018); Katz, J., Ed.; ASME Press: New York, NY, USA, 2018. [Google Scholar] [CrossRef]
- Sun, L.; Guo, P.; Luo, X. Numerical investigation on inter-blade cavitation vortex in a Franics turbine. Renew. Energy 2020, 158, 64–74. [Google Scholar] [CrossRef]
- Yilmaz, N.; Aktas, B.; Atlar, M.; Fitzsimmons, P.A.; Felli, M. An experimental and numerical investigation of propeller-rudder-hull interaction in the presence of tip vortex cavitation (TVC). Ocean Eng. 2020, 216, 108024. [Google Scholar] [CrossRef]
- Grandjean, H.; Jacques, N.; Zaleski, S. Shock propagation in liquids containing bubbly clusters: A continuum approach. J. Fluid Mech. 2012, 701, 304–332. [Google Scholar] [CrossRef]
- Sikirica, A.; Čarija, Z.; Lučin, I.; Grbčić, L.; Kranjčević, L. Cavitation Model Calibration Using Machine Learning Assisted Workflow. Mathematics 2020, 8, 2107. [Google Scholar] [CrossRef]
- Folden, T.S.; Aschmoneit, F.J. A classification and review of cavitation models with an emphasis on physical aspects of cavitation. Phys. Fluids 2023, 35, 081301. [Google Scholar] [CrossRef]
- Mikic, B.B.; Rohsenow, W.M.; Griffith, P. On Bubble Growth Rates. Int. J. Heat Mass Transf. 1970, 13, 657–666. [Google Scholar] [CrossRef]
- Li, W.; Yu, Z. Cavitation models with thermodynamic effect for organic fluid cavitating flows in organic Rankine cycle systems: A review. Therm. Sci. Eng. Prog. 2021, 26, 101079. [Google Scholar] [CrossRef]
- Li, L.; Wang, Z.; Li, X.; Zhu, Z. Multiscale modeling of tip-leakage cavitating flows by a combined volume of fluid and discrete bubble model. Phys. Fluids 2021, 33, 062104. [Google Scholar] [CrossRef]
- Li, L.; Huo, Y.; Wang, Z.; Li, X.; Zhu, Z. Large eddy simulation of tip-leakage cavitating flow using a multiscale cavitation model and investigation on model parameters. Phys. Fluids 2021, 33, 092104. [Google Scholar] [CrossRef]
- Adamkowski, A.; Henke, A.; Lewandowski, M. Resonance of torsional vibrations of centrifugal pump shafts due to cavitation erosion of pump impellers. Eng. Fail. Anal. 2016, 70, 56–72. [Google Scholar] [CrossRef]
- Ghahramani, E.; Jahangir, S.; Neuhauser, M.; Bourgeois, S.; Poelma, C.; Bensow, R.E. Experimental and numerical study of cavitating flow around a surface mounted semi-circular cylinder. Int. J. Multiph. Flow 2020, 124, 103191. [Google Scholar] [CrossRef]
- Reuter, F.; Mettin, R. Mechanisms of Single Bubble Cleaning. Ultrason. Sonochemistry 2016, 29, 550–562. [Google Scholar] [CrossRef] [PubMed]
- Han, W.; Gu, Z.; Li, R.; Mi, J.; Bai, L.; Deng, W. Study on the Dynamic Characteristics of Single Cavitation Bubble Motion near the Wall Based on the Keller–Miksis Model. Processes 2024, 12, 826. [Google Scholar] [CrossRef]
- Li, W.; Li, Z.; Han, W.; Yan, S.; Zhao, Q.; Chen, F. Measured viscosity characteristics of Fe3O4 ferrofluid in magnetic and thermal fields. Phys. Fluids 2023, 35, 012002. [Google Scholar] [CrossRef]
- Li, W.; Li, Z.; Qin, Z.; Yan, S.; Wang, Z.; Peng, S. Influence of the solution pH on the design of a hydro-mechanical magneto-hydraulic sealing device. Eng. Fail. Anal. 2022, 135, 106091. [Google Scholar] [CrossRef]
- Ding, Q.; Li, X.; Cui, Y.; Lv, J.; Shan, Y.; Liu, Y. Study on Non-Spherical Deformation Velocity of a Single Cavitation Bubble. Processes 2024, 12, 553. [Google Scholar] [CrossRef]
- He, X.; Peng, H.; Zhang, J.; Yuan, H. Multiple vapor cavitation bubble interactions with a thermal lattice Boltzmann method. Ocean. Eng. 2022, 266 Pt 4, 113058. [Google Scholar] [CrossRef]
- Gevari, M.T.; Abbasiasl, T.; Niazi, S.; Ghorbani, M.; Koşar, A. Direct and indirect thermal applications of hydrodynamic and acoustic cavitation: A review. Appl. Therm. Eng. 2020, 171, 115065. [Google Scholar] [CrossRef]
- Mulbah, C.; Kang, C.; Mao, N.; Zhang, W.; Shaikh, A.R.; Teng, S. A review of VOF methods for simulating bubble dynamics. Prog. Nucl. Energy 2022, 154, 104478. [Google Scholar] [CrossRef]
- Pavlenko, A. Numerical Modeling of the Behavior of Bubble Clusters in Cavitation Processes. Energies 2024, 17, 1741. [Google Scholar] [CrossRef]
- Zhang, A.-M.; Li, S.-M.; Cui, P.; Li, S.; Liu, Y.-L. A unified theory for bubble dynamics. Phys. Fluids 2023, 35, 033323. [Google Scholar] [CrossRef]
- Van Gorder, R.A. Dynamics of the Rayleigh–Plesset equation modelling a gas-filled bubble immersed in an incompressible fluid. J. Fluid Mech. 2016, 807, 478–508. [Google Scholar] [CrossRef]
- Ghazivini, M.; Hafez, M.; Ratanpara, A.; Kim, M. A review on correlations of bubble growth mechanisms and bubble dynamics parameters in nucleate boiling. J. Therm. Anal. Calorim. 2022, 147, 6035–6071. [Google Scholar] [CrossRef]
- Bhati, J.; Paruya, S. Numerical simulation of bubble dynamics in pool boiling at heated surface. Int. J. Heat Mass Transf. 2020, 152, 119465. [Google Scholar] [CrossRef]
- Chakraborty, B.; Gallo, M.; Marengo, M.; De Coninck, J.; Casciola, C.M.; Miche, N.; Georgoulas, A. Multi-scale modelling of boiling heat transfer: Exploring the applicability of an enhanced volume of fluid method in sub-micron scales. Int. J. Thermofluids 2024, 22, 100683. [Google Scholar] [CrossRef]
- Sullivan, P.; Dockar, D.; Borg, M.K.; Enright, R.; Pillai, R. Inertio-thermal vapour bubble growth. J. Fluid Mech. 2022, 948, A55. [Google Scholar] [CrossRef]
- Saritha, G.; Banerjee, R. Bubble dynamics of a pressure-driven cavitating flow in a micro-scale channel using a high density pseudo-potential Lattice Boltzmann method. Heat Transfer Eng. 2020, 41, 622–636. [Google Scholar] [CrossRef]
- Bardia, R.; Trujillo, M.F. Assessing the physical validity of highly-resolved simulation benchmark tests for flows undergoing phase change. Int. J. Multiph. Flow 2019, 112, 52–62. [Google Scholar] [CrossRef]
- Theofanous, T.G.; Patel, P.D. Universal relations for bubble growth. Int. J. Heat Mass Transf. 1976, 19, 425–429. [Google Scholar] [CrossRef]
- Miyatake, O.; Tanaka, I.; Lior, N. A simple universal equation for bubble growth in pure liquids and binary solutions with a nonvolatile solute. Int. J. Heat Mass Transf. 1997, 40, 1577–1584. [Google Scholar] [CrossRef]
- Li, W.; Yu, Z.; Kadam, S. An improved cavitation model with thermodynamic effect and multiple cavitation regimes. Int. J. Heat Mass Transf. 2023, 205, 123854. [Google Scholar] [CrossRef]
- Wang, Q.; Gu, J.; Li, Z.; Yao, W. Dynamic modeling of bubble growth in vapor-liquid phase change covering a wide range of superheats and pressures. Chem. Eng. Sci. 2017, 172, 169–181. [Google Scholar] [CrossRef]
- Forster, H.K.; Zuber, N. Growth of a Vapor Bubble in a Superheated Liquid. J. Appl. Phys. 1954, 25, 474–478. [Google Scholar] [CrossRef]
- Morlat, T. Bubble growth rate in superheated droplets. Therm. Sci. Eng. Prog. 2022, 36, 101505. [Google Scholar] [CrossRef]
- Lesage, F.J.; Siedel, S.; Cotton, J.S.; Robinson, A.J. A mathematical model for predicting bubble growth for low Bond and Jakob number nucleate boiling. Chem. Eng. Sci. 2014, 112, 35–46. [Google Scholar] [CrossRef]
- Li, J.; Liao, Y.; Bolotnov, I.A.; Zhou, P.; Lucas, D.; Li, Q.; Gong, L. Direct numerical simulation of heat transfer on a deformable vapor bubble rising in superheated liquid. Phys. Fluids 2023, 35, 023319. [Google Scholar] [CrossRef]
- Deike, L. Mass transfer at the ocean-atmosphere interface: The role of wave breaking, droplets, and bubbles. Annu. Rev. Fluid Mech. 2022, 54, 191–224. [Google Scholar] [CrossRef]
- Yi, Q.; Guo, P.; Zuo, Z.; Liu, S. Modeling of vapor bubble dynamics considering the metastable fluid state. Phys. Fluids 2024, 36, 023326. [Google Scholar] [CrossRef]
- Suslick, K.S.; Eddingsaas, N.C.; Flannigan, D.J.; Hopkins, S.D.; Xu, H. The Chemical History of a Bubble. Acc. Chem. Res. 2018, 51, 2169–2178. [Google Scholar] [CrossRef]
- Denner, F.; Schenke, S. Modeling acoustic emissions and shock formation of cavitation bubbles. Phys. Fluids 2023, 35, 012114. [Google Scholar] [CrossRef]
- Ando, K.; Sugawara, M.; Sakota, R. Particle removal in ultrasonic water flow cleaning role of cavitation bubbles as cleaning agents. Solid State Phenom. 2021, 314, 218–221. [Google Scholar] [CrossRef]
- Ando, K.; Sakota, R.; Usui, H.; Ishibashi, T.; Matsuo, H.; Watanabe, K. Optimal injection distance in ultrasonic water flow cleaning. Solid State Phenom. 2023, 346, 258–262. [Google Scholar] [CrossRef]
- Mnich, D.; Reuter, F.; Denner, F.; Ohl, C.-D. Single cavitation bubble dynamics in a stagnation flow. J. Fluid Mech. 2024, 979, A18. [Google Scholar] [CrossRef]
- Shi, H.; Zhang, H.; Geng, L.; Qu, S.; Wang, X.; Nikrityuk, P.A. Dynamic behaviors of cavitation bubbles near biomimetic surfaces: A numerical study. Ocean. Eng. 2024, 292, 116628. [Google Scholar] [CrossRef]
- Pandit, A.V.; Sarvothaman, V.P.; Ranade, V.V. Estimation of chemical and physical effects of cavitation by analysis of cavitating single bubble dynamics. Ultrason. Sonochemistry 2021, 77, 105677. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.-N.; Ding, Z.-L.; Hu, J.-R.; Zheng, X.-X.; Yu, J.-X.; Hu, J.-S. Theoretical investigation on the cavitation bubble dynamics near three spherical particles based on Weiss theorem. J. Hydrodyn. 2023, 35, 1119–1130. [Google Scholar] [CrossRef]
- Chen, J.; Chen, T.; Geng, H.; Huang, B.; Cao, Z. Investigation on dynamic characteristics and thermal effects of single cavitation bubble in liquid nitrogen. Phys. Fluids 2024, 36, 023325. [Google Scholar] [CrossRef]
- Altay, R.; Sadaghiani, A.K.; Sevgen, M.I.; Şişman, A.; Koşar, A. Numerical and Experimental Studies on the Effect of Surface Roughness and Ultrasonic Frequency on Bubble Dynamics in Acoustic Cavitation. Energies 2020, 13, 1126. [Google Scholar] [CrossRef]
- Pavlenko, A.M.; Koshlak, H. Intensification of Gas Hydrate Formation Processes by Renewal of Interfacial Area between Phases. Energies 2021, 14, 5912. [Google Scholar] [CrossRef]
- Qin, Z.; Alehossein, H. Heat transfer during cavitation bubble collapse. Appl. Therm. Eng. 2016, 105, 1067–1075. [Google Scholar] [CrossRef]
- Yang, Y.; Shan, M.; Kan, X.; Duan, K.; Han, Q.; Juan, Y. Thermodynamic effects of gas adiabatic index on cavitation bubble collapse. Heliyon 2023, 9, e20532. [Google Scholar] [CrossRef] [PubMed]
- Choi, K.; Kim, S.; Kim, H.; Kim, C. Computational investigation on the non-isothermal phase change during cavitation bubble pulsations. Ocean. Eng. 2023, 285 Pt 2, 115414. [Google Scholar] [CrossRef]
- Tian, L.; Zhang, Y.; Yin, J.; Lv, L.; Zhang, J.; Zhu, J. Investigation on heat and mass transfer characteristics of a near-wall multi-cycle cavitation bubble and its thermal effects on the wall using an improved compressible multiphase model. Ocean. Eng. 2024, 298, 117118. [Google Scholar] [CrossRef]
- Bermudez-Graterol, J.M.; Nickaeen, M.; Skoda, R. Numerical simulation of spherical bubble collapse by a uniform bubble pressure approximation and detailed description of heat and mass transfer with phase transition. Appl. Math. Model. 2021, 96, 80–110. [Google Scholar] [CrossRef]
- Aganin, A.A.; Khismatullina, N.A. Influence of the phase interface mass transfer characteristics on the cavitation bubble collapse in water. Ocean. Eng. 2023, 283, 115013. [Google Scholar] [CrossRef]
- Wang, J.; Li, H.; Guo, W.; Wang, Z.; Du, T.; Wang, Y.; Abe, A.; Huang, C. Rayleigh-Taylor instability of cylindrical water droplet induced by laser-produced cavitation bubble. J. Fluid Mech. 2021, 919, A42. [Google Scholar] [CrossRef]
- Kim, C.; Choi, W.J.; Kang, W. Cavitation nucleation and its ductile-to-brittle shape transition in soft gels under translational mechanical impact. Acta Biomater. 2022, 142, 160–173. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Liao, Y.; Zhou, P.; Lucas, D.; Li, Q. Numerical study of flashing pipe flow using a TFM-PBM coupled method: Effect of interfacial heat transfer and bubble coalescence and breakup. Int. J. Therm. Sci. 2023, 193, 108504. [Google Scholar] [CrossRef]
- Yu, Z.; Li, J.; Zhang, X. A new hypothesis for cavitation nucleation in gas saturated solutions: Clustering of gas molecules lowers significantly the surface tension. Chin. J. Chem. Eng. 2022, 50, 347–351. [Google Scholar] [CrossRef]
- Khoo, M.T.; Venning, J.A.; Pearce, B.W.; Brandner, P.A. Nucleation and cavitation number effects on tip vortex cavitation dynamics and noise. Exp. Fluids 2021, 62, 216. [Google Scholar] [CrossRef]
- Pavlenko, A.; Koshlak, H.; Usenko, B. Heat and mass transfer in fluidized layer. Metall. Min. Ind. 2014, 6, 96–100. [Google Scholar]
- Dergarabedian, P. The rate of growth of vapor bubbles in superheated water. J Appl. Mech. 2021, 20, 537–545. [Google Scholar] [CrossRef]
- Zhou, G.; Prosperetti, A. Modelling the thermal behaviour of gas bubbles. J. Fluid Mech. 2020, 901, R3. [Google Scholar] [CrossRef]
- Cheng, F.; Ji, W. Numerical and experimental study on dynamic characteristics of cavitation bubbles. Ind. Lubr. Tribol. 2018, 70, 1119–1126. [Google Scholar] [CrossRef]
- Wu, P.; Bai, L.; Lin, W.; Wang, X. Mechanism and dynamics of hydrodynamic-acoustic cavitation (HAC). Ultrason. Sonochemistry 2018, 49, 89–96. [Google Scholar] [CrossRef] [PubMed]
- Peng, Y.; He, X.; Peng, H.; Lin, Y.; Zhang, J. Mesoscopic modeling of vapor cavitation bubbles collapse and interaction in near-wall region with a pseudopotential lattice Boltzmann method. Phys. Fluids 2022, 34, 092012. [Google Scholar] [CrossRef]
- Orthaber, U.; Zevnik, J.; Petkovšek, R.; Dular, M. Cavitation bubble collapse in a vicinity of a liquid-liquid interface—Basic research into emulsification process. Ultrason. Sonochemistry 2020, 68, 105224. [Google Scholar] [CrossRef]
- Peng, C.; Tian, S.; Li, G.; Sukop, M.C. Single-component multiphase lattice Boltzmann simulation of free bubble and crevice heterogeneous cavitation nucleation. Phys. Rev. E 2018, 98, 023305. [Google Scholar] [CrossRef]
- Liang, X.-X.; Linz, N.; Freidank, S.; Paltauf, G.; Vogel, A. Comprehensive analysis of spherical bubble oscillations and shock wave emission in laser-induced cavitation. J. Fluid Mech. 2022, 940, A5. [Google Scholar] [CrossRef]
- Geng, S.; Yao, Z.; Zhong, Q.; Du, Y.; Xiao, R.; Wang, F. Propagation of Shock Wave at the Cavitation Bubble Expansion Stage Induced by a Nanosecond Laser Pulse. J. Fluids Eng. 2021, 143, 051209. [Google Scholar] [CrossRef]
- Peng, K.; Tian, S.; Zhang, Y.; Li, J.; Qu, W.; Li, C. The violent collapse of vapor bubbles in cryogenic liquids. Ultrason. Sonochemistry 2024, 104, 106845. [Google Scholar] [CrossRef] [PubMed]
- Shi, Y.; Luo, K.; Chen, X.; Li, D.; Jia, L. A new cavitation model considering inter-bubble action. Int. J. Nav. Archit. Ocean. Eng. 2021, 13, 566–574. [Google Scholar] [CrossRef]
- Liang, L.; Xu, L.; Zhang, H.; Bing, C.; Lihai, C. The numerical investigationon bubble interaction dynamics in hydrodynamic cavitation. Mechanics 2021, 27, 115. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, G.; Zhang, J.; Tian, M.; Li, S. The bubble merger in rectangular microchannels during boiling processes based on conjugate heat transfer. Appl. Therm. Eng. 2024, 247, 123093. [Google Scholar] [CrossRef]
- Pavlenko, A.M.; Basok, B.I. Kinetics of water evaporation from emulsions. Heat Transf. Res. 2005, 36, 425–430. [Google Scholar] [CrossRef]
- Pavlenko, A.M.; Basok, B.I.; Avramenko, A.A. Heat conduction of a multi-layer disperse particle of emulsion. Heat Transf. Res. 2005, 36, 55–61. [Google Scholar] [CrossRef]
- Pavlenko, A.M. Thermodynamic Features of the Intensive Formation of Hydrocarbon Hydrates. Energies 2020, 13, 3396. [Google Scholar] [CrossRef]
- Wang, Q. Multi-oscillations of a bubble in a compressible liquid near a rigid boundary. J. Fluid Mech. 2014, 745, 509–536. [Google Scholar] [CrossRef]
- Wang, Q.X.; Blake, J.R. Non-spherical bubble dynamics in a compressible liquid. Part 1. Travelling acoustic wave. J. Fluid Mech. 2010, 659, 191–224. [Google Scholar] [CrossRef]
- Zhang, A.M.; Wang, S.P.; Wu, G.X. Simulation of bubble motion in a compressible liquid based on the three dimensional wave equation. Eng. Anal. Bound. Elem. 2013, 37, 1179–1188. [Google Scholar] [CrossRef]
- Tian, Z.-L.; Liu, Y.-L.; Zhang, A.-M.; Tao, L. Energy dissipation of pulsating bubbles in compressible fluids using the Eulerian finite-element method. Ocean. Eng. 2020, 196, 106714. [Google Scholar] [CrossRef]
- Xiao, W.; Zhang, A.M.; Wang, S.P. Investigation of bubble dynamics of underwater explosion based on improved compressible numerical model. Appl. Ocean. Res. 2016, 59, 472–482. [Google Scholar] [CrossRef]
- Liu, J.; Xiao, W.; Yao, X. Pressure characteristics of a nonspherical underwater explosion bubble in a compressible fluid. Phys. Fluids 2024, 36, 057145. [Google Scholar] [CrossRef]
- Prosperetti, A.; Lezzi, A. Bubble dynamics in a compressible liquid. Part 1. First-order theory. J. Fluid Mech. 1986, 168, 457–478. [Google Scholar] [CrossRef]
- Zheng, X.; Wang, X.; Zhang, Y. A single oscillating bubble in liquids with high Mach number. Ultrason. Sonochemistry 2022, 85, 105985. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Li, Z.; Han, W.; Tan, S.; Yan, S.; Wang, D.; Yang, S. Time-mean equation and multi-field coupling numerical method for low-Reynolds-number turbulent flow in ferrofluid. Phys. Fluids 2023, 35, 125145. [Google Scholar] [CrossRef]
- Fourest, T.; Deletombe, E.; Faucher, V.; Arrigoni, M.; Dupas, J.; Laurens, J.-M. Comparison of Keller–Miksis model and finite element bubble dynamics simulations in a confined medium. Application to the Hydrodynamic Ram. Eur. J. Mech. B/Fluids 2018, 68, 66–75. [Google Scholar] [CrossRef]
- Wang, Q.; Sandberg, M.; Lin, Y.; Yin, S.; Hang, J. Impacts of Urban Layouts and Open Space on Urban Ventilation Evaluated by Concentration Decay Method. Atmosphere 2017, 8, 169. [Google Scholar] [CrossRef]
- Zanje, S.; Iyer, K.; Murallidharan, J.S.; Punekar, H.; Gupta, V.K. Development of generalized bubble growth model for cavitation and flash boiling. Phys. Fluids 2021, 33, 077116. [Google Scholar] [CrossRef]
- Bardia, R.; Trujillo, M.F. An improved categorization of vapor bubble collapse: Explaining the coupled nature of hydrodynamic and thermal mechanisms. Int. J. Heat Mass Transf. 2019, 145, 118754. [Google Scholar] [CrossRef]
- Colombet, D.; Da Silva, E.G.; Fortes-Patella, R. On numerical simulation of cavitating flows under thermal regime. Int. J. Heat Mass Transf. 2017, 105, 411–428. [Google Scholar] [CrossRef]
- Chen, T.; Huang, B.; Wang, G.; Wang, K. Effects of fluid thermophysical properties on cavitating flows. J. Mech. Sci. Technol. 2015, 29, 4239–4246. [Google Scholar] [CrossRef]
- Chen, T.; Huang, B.; Wang, G.; Zhao, X. Numerical study of cavitating flows in a wide range of water temperatures with special emphasis on two typical cavitation dynamics. Int. J. Heat Mass Transf. 2016, 101, 886–900. [Google Scholar] [CrossRef]
- Zhu, J.; Zhao, D.; Xu, L.; Zhang, X. Interactions of vortices, thermal effects and cavitation in liquid hydrogen cavitating flows. Int. J. Hydrog. Energy 2016, 41, 614–631. [Google Scholar] [CrossRef]
- Kinzel, M.P.; Lindau, J.W.; Kunz, R.F. A Unified Homogenous Multiphase CFD Model for Cavitation. In Proceedings of the ASME 2017 Fluids Engineering Division Summer Meeting, Waikoloa, HI, USA, 30 July–3 August 2017; Volume 1B. [Google Scholar] [CrossRef]
- Pavlenko, A.; Koshlak, Y. Heat and Mass Transfer During Phase Transitions in Liquid Mixtures. Rocz. Ochr. Śr. 2019, 21, 234–249. [Google Scholar]
- Cattaneo, M.; Supponen, O. Shell viscosity estimation of lipid-coated microbubbles. Soft Matter 2023, 19, 5925–5941. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.; Habchi, C. Real-fluid phase transition in cavitation modeling considering dissolved non-condensable gas. Phys. Fluids 2020, 32, 032102. [Google Scholar] [CrossRef]
- Kähler, G.; Bonelli, F.; Gonnella, G.; Lamura, A. Cavitation inception of a van der Waals fluid at a sack-wall obstacle. Phys. Fluids 2015, 27, 123307. [Google Scholar] [CrossRef]
- Bidi, S.; Koukouvinis, P.; Papoutsakis, A.; Shams, A.; Gavaises, M. Numerical study of real gas effects during bubble collapse using a disequilibrium multiphase model. Ultrason. Sonochemistry 2022, 90, 106175. [Google Scholar] [CrossRef]
- Delale, C.F.; Pasinlioğlu, Ş. On the gas pressure inside cavitation bubbles. Phys. Fluids 2023, 35, 023330. [Google Scholar] [CrossRef]
- Peng, K.; Qin, F.G.F.; Jiang, R.; Qu, W.; Wang, Q. Production and dispersion of free radicals from transient cavitation Bubbles: An integrated numerical scheme and applications. Ultrason. Sonochemistry 2022, 88, 106067. [Google Scholar] [CrossRef]
- Nigmatulin, R.I.; Khabeev, N.S.; Nagiev, F.B. Dynamics, heat and mass transfer of vapour-gas bubbles in a liquid. Int. J. Heat Mass Transf. 1981, 24, 1033–1044. [Google Scholar] [CrossRef]
- Li, J.; Carrica, P.M. Numerical study of the cavitating flow over backward facing step with a polydisperse two-phase flow model. Phys. Fluids 2023, 35, 063313. [Google Scholar] [CrossRef]
- Denner, F. The Gilmore-NASG model to predict single-bubble cavitation in compressible liquids. Ultrason. Sonochemistry 2021, 70, 105307. [Google Scholar] [CrossRef]
- Bermudez-Graterol, J.M.; Skoda, R. Numerical simulation of bubble dynamics and segregation in binary heptane/dodecane mixtures. J. Fluid Mech. 2022, 947, A9. [Google Scholar] [CrossRef]
- Joshi, S.; Franc, J.P.; Ghigliotti, G.; Fivel, M. Bubble collapse induced cavitation erosion: Plastic strain and energy dissipation investigations. J. Mech. Phys. Solids 2020, 134, 103749. [Google Scholar] [CrossRef]
- Schmidt, D.; Gopalakrishnan, S.; Jasak, H. Multi-dimensional simulation of thermal non-equilibrium channel flow. Int. J. Multiph. Flow 2010, 36, 284–292. [Google Scholar] [CrossRef]
- Stasheuski, S.; Keskinen, K.; Schmidt, D.P.; Vuorinen, V.; Hyvönen, J.; Kaario, O. Impact of modelling assumptions in cavitating flow of simplified injector. Int. J. Multiph. Flow 2024, 177, 104847. [Google Scholar] [CrossRef]
- Mottyll, S.; Skoda, R. Numerical 3D flow simulation of ultrasonic horns with attached cavitation structures and assessment of flow aggressiveness and cavitation erosion sensitive wall zones. Ultrason. Sonochem 2016, 31, 570–589. [Google Scholar] [CrossRef] [PubMed]
- Magnotti, G.M.; Battistoni, M.; Saha, K.; Som, S. Development and validation of the cavitation-induced erosion risk assessment tool. Transp. Eng. 2020, 2, 100034. [Google Scholar] [CrossRef]
- Trummler, T.; Schmidt, S.J.; Adams, N.A. Effect of stand-off distance and spatial resolution on the pressure impact of near-wall vapor bubble collapses. Int. J. Multiph. Flow 2021, 141, 103618. [Google Scholar] [CrossRef]
- Ahmed, A.; Duret, B.; Reveillon, J.; Demoulin, F.X. Numerical simulation of cavitation for liquid injection in non-condensable gas. Int. J. Multiph. Flow 2020, 127, 103269. [Google Scholar] [CrossRef]
- Santos, E.G.; Shi, J.; Venkatasubramanian, R.; Hoffmann, G.; Gavaises, M.; Bauer, W. Modelling and prediction of cavitation erosion in GDi injectors operated with E100 fuel. Fuel 2021, 289, 119923. [Google Scholar] [CrossRef]
- Trummler, T.; Schmidt, S.J.; Adams, N.A. Numerical investigation of non-condensable gas effect on vapor bubble collapse. Phys. Fluids 2021, 33, 096107. [Google Scholar] [CrossRef]
- Brandao, F.L.; Bhatt, M.; Mahesh, K. Numerical study of cavitation regimes in flow over a circular cylinder. J. Fluid Mech. 2020, 885, A19. [Google Scholar] [CrossRef]
- Pavlenko, A.; Koshlak, H.; Usenko, B. The processes of heat and mass exchange in the vortex devices. Metall. Min. Ind. 2014, 6, 55–59. [Google Scholar]
- Aganin, A.A.; Mustafin, I.N. Cavitation bubble collapse and rebound in water: Influence of phase transitions. Int. J. Multiph. Flow 2022, 157, 104256. [Google Scholar] [CrossRef]
- Ohashi, K.; Kobayashi, K.; Fujii, H.; Watanabe, M. Vapor condensation induced by fast-moving liquid film in the presence of noncondensable gas molecules. Int. Commun. Heat Mass Transf. 2023, 142, 106622. [Google Scholar] [CrossRef]
- Naranmandula, Q. Influence of large bubbles on cavitation effect of small bubbles in cavitation multi-bubbles. Acta Phys. Sin. 2019, 68, 167–175. [Google Scholar]
- Joshi, S.; Franc, J.P.; Ghigliotti, G.; Fivel, M. SPH modelling of a cavitation bubble collapse near an elasto-visco-plastic material. J. Mech. Phys. Solids 2019, 125, 420–439. [Google Scholar] [CrossRef]
- Aliabadi, A.; Taklifi, A. The effect of magnetic field on dynamics of gas bubbles in visco-elastic fluids. Appl. Math. Model. 2012, 36, 2567–2577. [Google Scholar] [CrossRef]
- Veshchunov, M. On the Theory of Two-Dimensional Homogeneous Nucleation on Close-Packed Faces of Crystals Growing from Vapour. J. Exp. Theor. Phys. 2021, 133, 449–454. [Google Scholar] [CrossRef]
- Ljung, A.L.; Lundström, T. Heat and mass transfer boundary conditions at the surface of a heated sessile droplet. Heat Mass Transf. 2017, 53, 3581–3591. [Google Scholar] [CrossRef]
- Hao, Y.; Zhang, Y.; Prosperetti, A. Mechanics of gas-vapor bubbles. Phys. Rev. Fluids 2017, 2, 034303. [Google Scholar] [CrossRef]
- Chen, T.; Wang, G.; Huang, B.; Wang, K. Numerical study of thermodynamic effects on liquid nitrogen cavitating flows. Cryogenics 2015, 70, 21–27. [Google Scholar] [CrossRef]
- Bhatt, M.; Mahesh, K. Numerical investigation of partial cavitation regimes over a wedge using large eddy simulation. Int. J. Multiph. Flow 2020, 122, 103155. [Google Scholar] [CrossRef]
- Yu, A.; Tang, Q.; Zhou, D.; Liu, J. Entropy production analysis in two-phase cavitation flows with thermodynamic cavitation model. Appl. Therm. Eng. 2020, 171, 115099. [Google Scholar] [CrossRef]
- Yu, A.; Tang, Q.; Zhou, D. Daqing Entropy production analysis in thermodynamic cavitating flow with the consideration of local compressibility. Int. J. Heat Mass Transf. 2020, 153, 119604. [Google Scholar] [CrossRef]
- Hong, F.; Yuan, J.; Zhou, B. Application of a new cavitation model for computations of unsteady turbulent cavitating flows around a hydrofoil. J. Mech. Sci. Technol. 2017, 31, 249–260. [Google Scholar] [CrossRef]
- Feng, H.; Wan, Y.; Fan, Z. Numerical investigation of turbulent cavitating flow in an axial flow pump using a new transport-based model. J. Mech. Sci. Technol. 2020, 34, 745–756. [Google Scholar] [CrossRef]
- Yuan, W.; Sauer, J.; Schnerr, G.H. Modeling and Computation of Unsteady Cavitation Flows in Injection Nozzles. Mécanique Ind. 2001, 2, 383–394. Available online: https://www.sciencedirect.com/science/article/pii/S1296213901011204 (accessed on 30 October 2001). [CrossRef]
- Priy, A.; Ahmad, I.; Khan, M.K.; Pathak, M. Bubble Interaction and Heat Transfer Characteristics of Microchannel Flow Boiling With Single and Multiple Cavities. ASME J. Thermal Sci. Eng. Appl. 2024, 16, 061010. [Google Scholar] [CrossRef]
- Vaca-Revelo, D.; Gnanaskandan, A. Numerical assessment of the condensation shock mechanism in sheet to cloud cavitation transition. Int. J. Multiph. Flow 2023, 169, 104616. [Google Scholar] [CrossRef]
- Long, X.; Liu, Q.; Ji, B.; Lu, Y. Numerical investigation of two typical cavitation shedding dynamics flow in liquid hydrogen with thermodynamic effects. Int. J. Heat Mass Transf. 2017, 109, 879–893. [Google Scholar] [CrossRef]
- Li, W.; Yu, Z. Cavitating flows of organic fluid with thermodynamic effect in a diaphragm pump for organic Rankine cycle systems. Energy 2021, 237, 121495. [Google Scholar] [CrossRef]
- Xue, R.; Ruan, Y.; Liu, X.; Cao, F.; Hou, Y. The influence of cavitation on the flow characteristics of liquid nitrogen through spray nozzles: A CFD study. Cryogenics 2017, 86, 42–56. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, Y.; Hou, H.; Zhang, J.; Xu, C. Entropy production diagnostic analysis of energy consumption for cavitation flow in a two-stage LNG cryogenic submerged pump. Int. J. Heat Mass Transf. 2019, 129, 342–356. [Google Scholar] [CrossRef]
- Song, P.; Sun, J. Cryogenic cavitation mitigation in a liquid turbine expander of an air-separation unit through collaborative fine-tuned optimization of impeller and fairing cone geometries. Energies 2020, 13, 50. [Google Scholar] [CrossRef]
- Xu, B.; Feng, J.; Wan, F.; Zhang, D.; Shen, X.; Zhang, W. Numerical investigation of modified cavitation model with thermodynamic effect in water and liquid nitrogen. Cryogenics 2020, 106, 103049. [Google Scholar] [CrossRef]
- Li, W.; Yang, Y.; Shi, W.; Zhao, X.; Li, W. The correction and evaluation of cavitation model considering the thermodynamic effect. Math. Probl. Eng. 2018, 2018, 7217513. [Google Scholar] [CrossRef]
- Kim, H.; Kim, C. A physics-based cavitation model ranging from inertial to thermal regimes. Int. J. Heat Mass Transf. 2021, 181, 121991. [Google Scholar] [CrossRef]
- Shepherd, J.E.; Sturtevant, B. Rapid evaporation at the superheat limit. J. Fluid Mech. 1982, 121, 379–402. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Furzeland, R.M.; Ijpelaar, M.J.M. Rapid evaporation at the superheat limit. Int. J. Heat Mass Transf. 1988, 31, 1687–1700. [Google Scholar] [CrossRef]

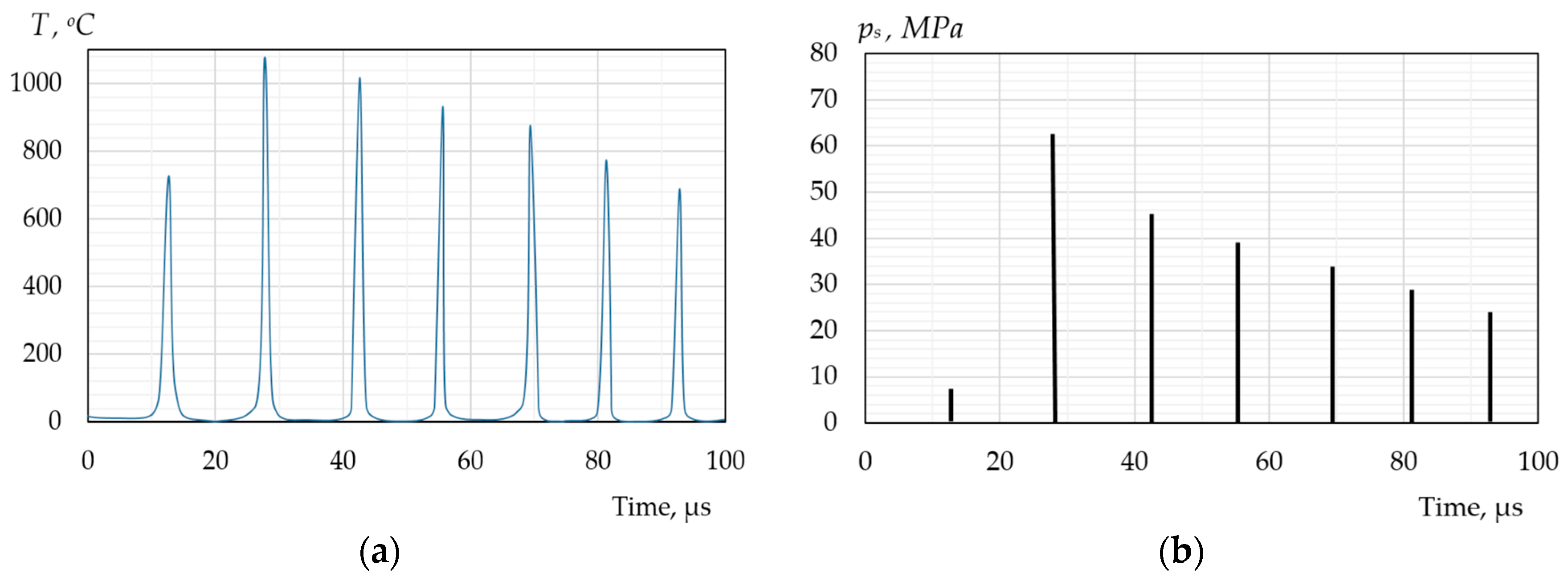
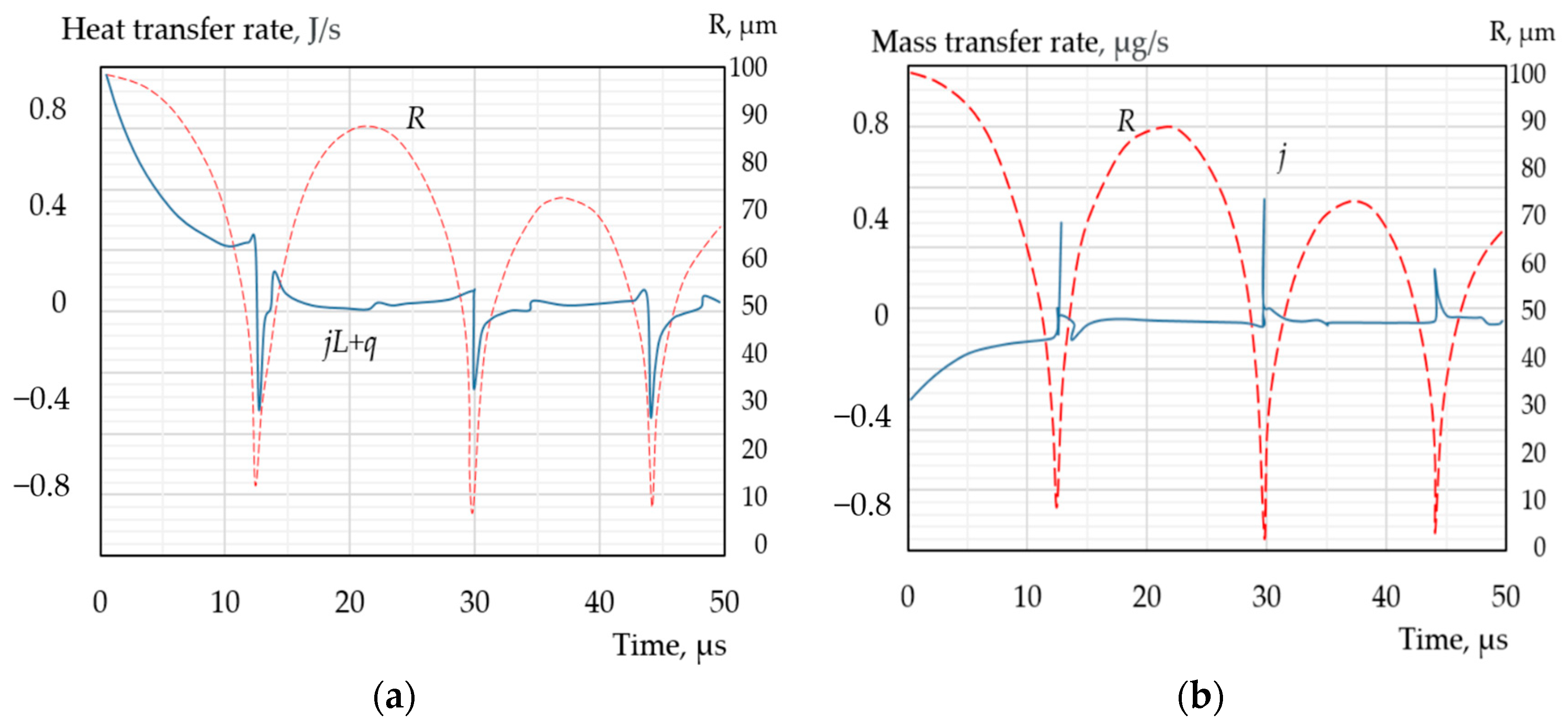

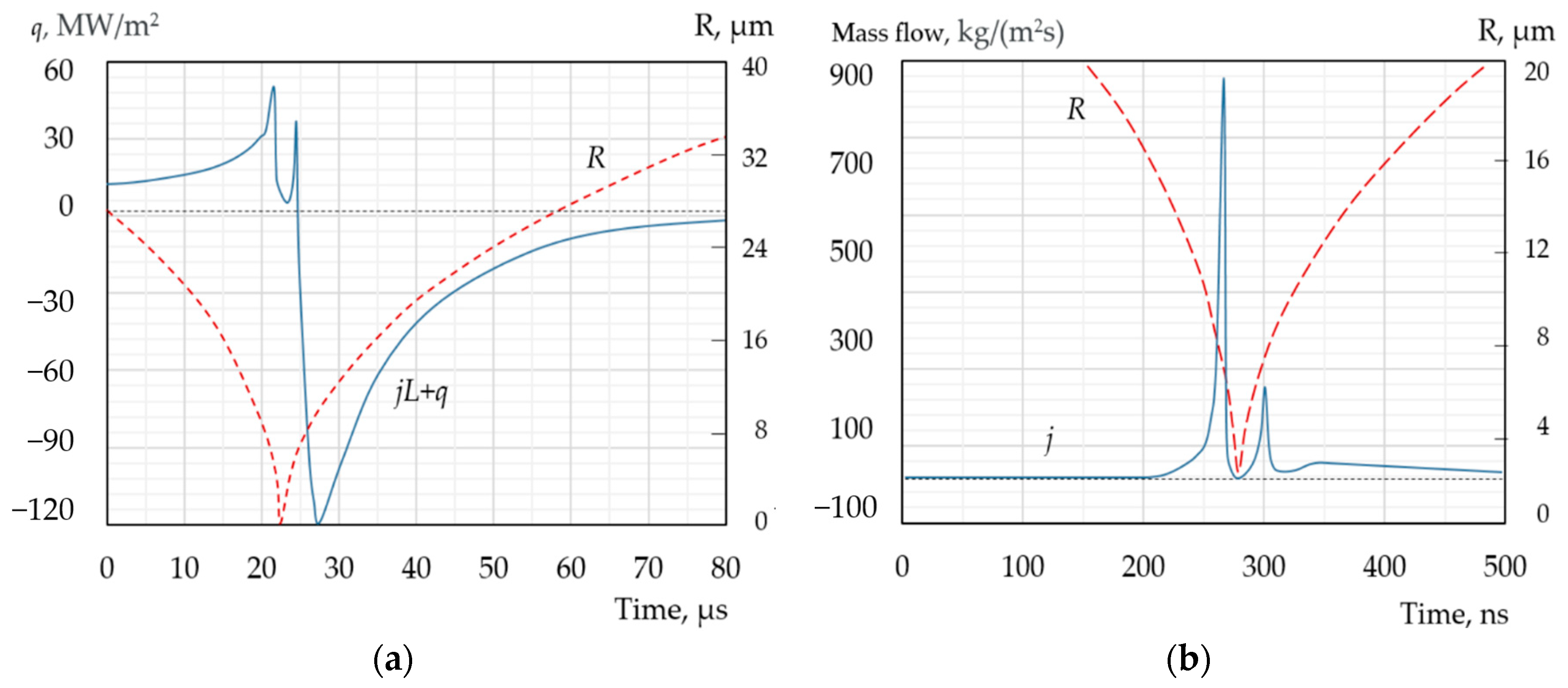
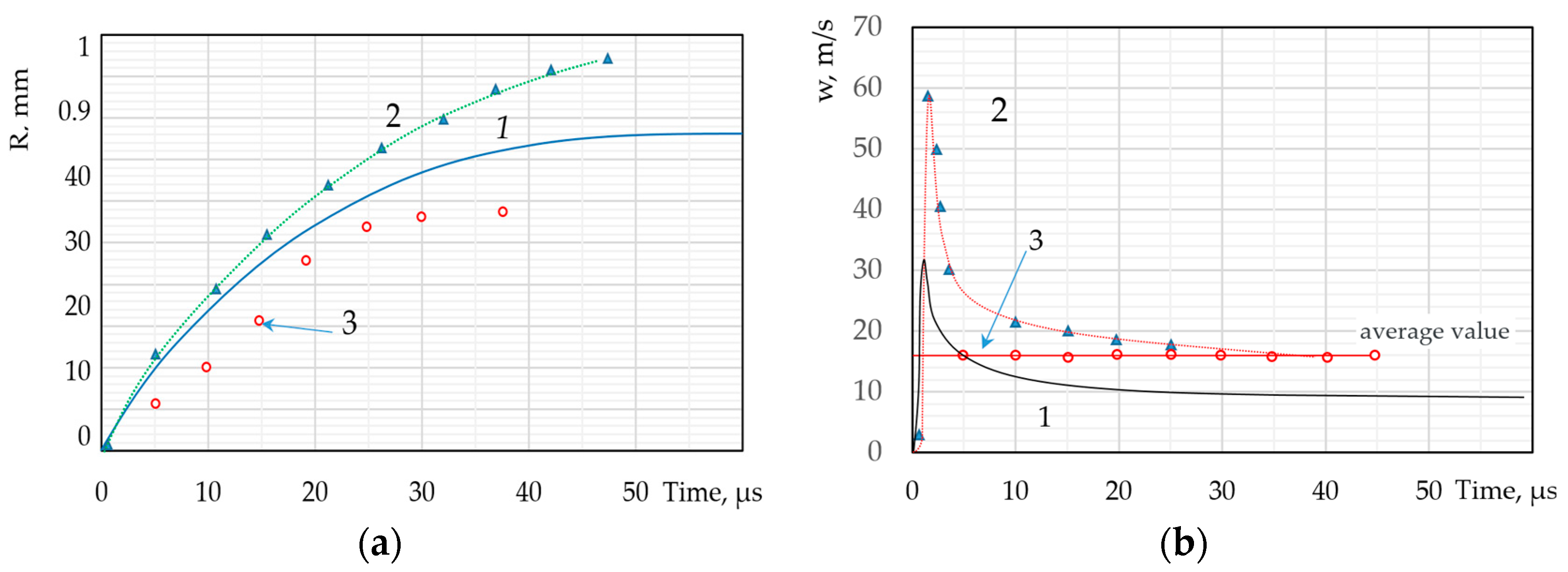
| Types of Models | The Modelling Approach Used | Publications |
|---|---|---|
| Modelling bubbles with separate schemes | These models employ distinct equations for the inertial and thermal stages of bubble growth | [39,40,41,42,43] |
| Models using equations of state | These models incorporate equations of state to describe the relationship between fluid properties and thermodynamic parameters | [44,45,46,47,48] |
| Modelling the thermodynamic state of liquid–vapour phase interfaces | These models focus on the thermodynamic behaviour of the phase boundaries between the liquid and vapour phases | [49,50] |
| Models with transport equations | These models utilise transport equations to account for heat and mass transfer processes during bubble dynamics | [30,31,51] |
| Bubble dynamics models based on the Rayleigh–Plesset equation | These models employ the Rayleigh-Plesset equation to describe the radial motion of bubbles | [52,53,54,55] |
| Machine learning models | These models leverage machine learning techniques to predict bubble dynamics based on experimental data or simulations | [35,36] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pavlenko, A.; Koshlak, H. Study of the Dynamics of a Single Bubble. Energies 2024, 17, 4236. https://doi.org/10.3390/en17174236
Pavlenko A, Koshlak H. Study of the Dynamics of a Single Bubble. Energies. 2024; 17(17):4236. https://doi.org/10.3390/en17174236
Chicago/Turabian StylePavlenko, Anatoliy, and Hanna Koshlak. 2024. "Study of the Dynamics of a Single Bubble" Energies 17, no. 17: 4236. https://doi.org/10.3390/en17174236
APA StylePavlenko, A., & Koshlak, H. (2024). Study of the Dynamics of a Single Bubble. Energies, 17(17), 4236. https://doi.org/10.3390/en17174236