Correlation of Macroscopic Fracture Behavior with Microscopic Fracture Mechanism for AHSS Sheet
Abstract
:1. Introduction
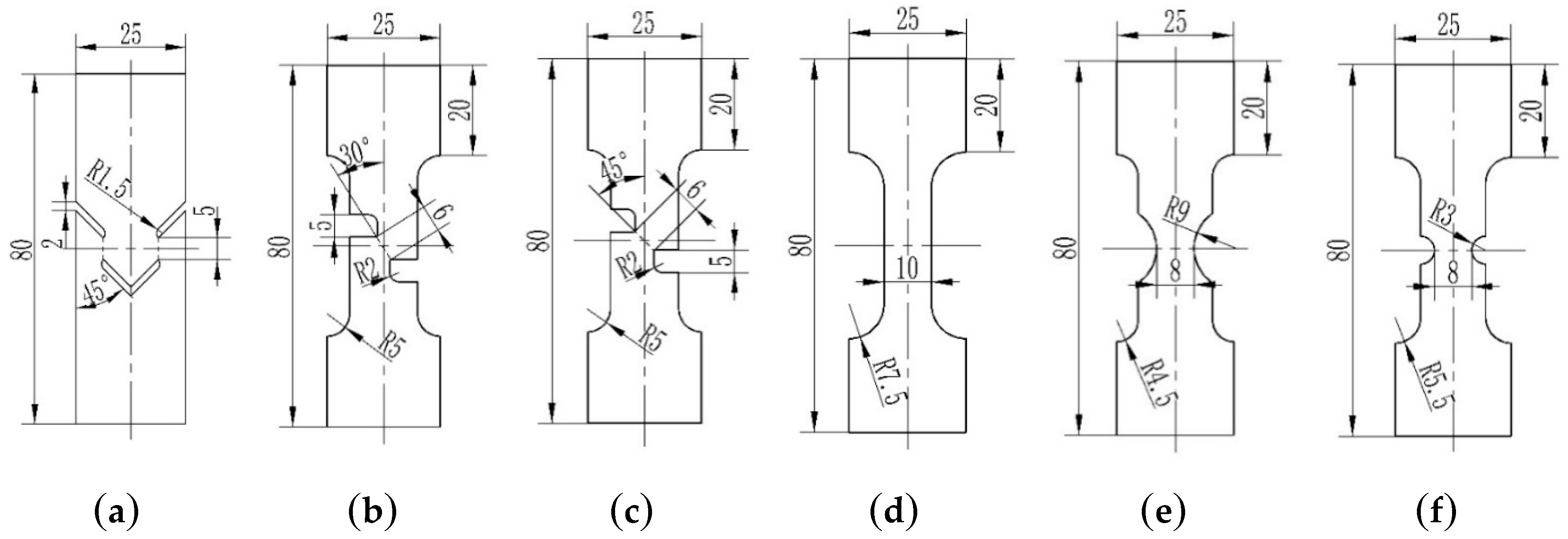
2. Experimental and Simulation Conditions
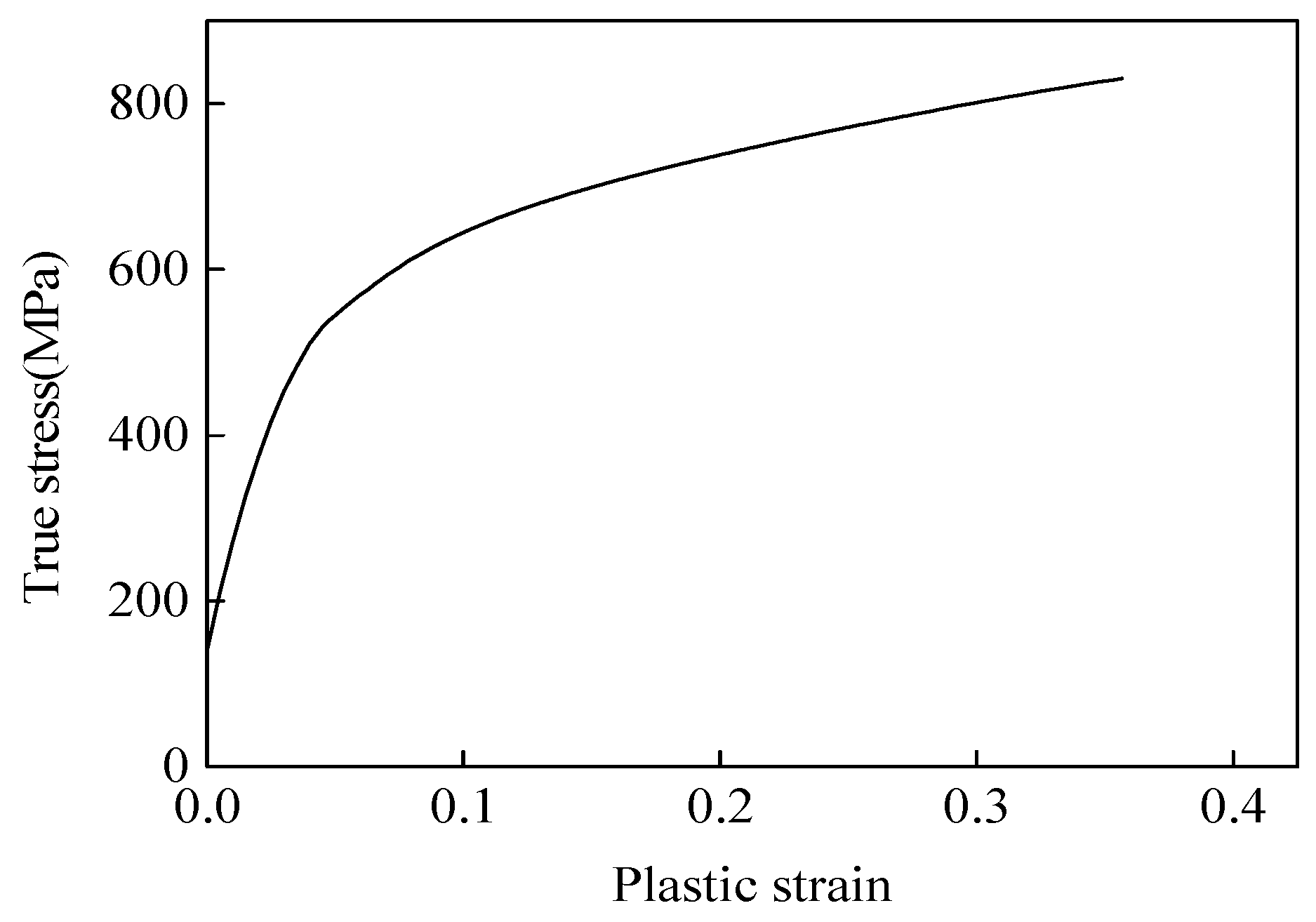
2.1. Experimental Procedure
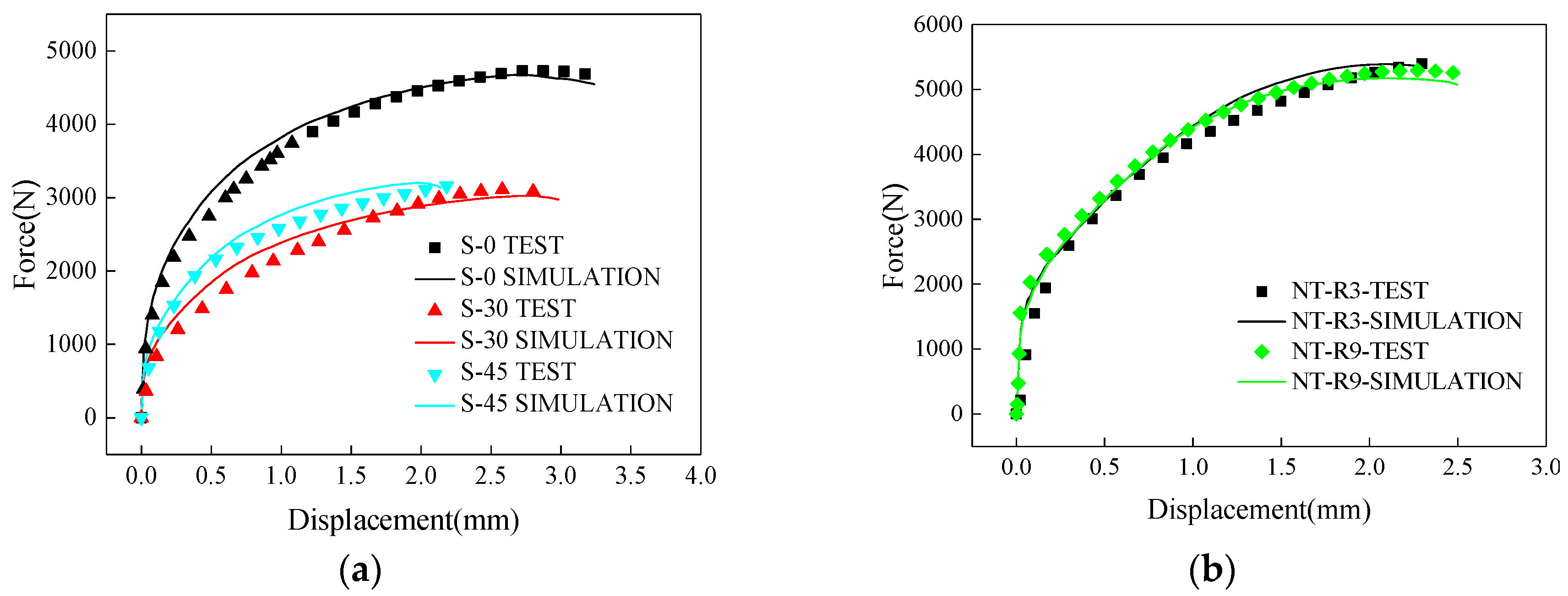
2.2. Finite Element (FE) Modeling
3. Results and Discussions
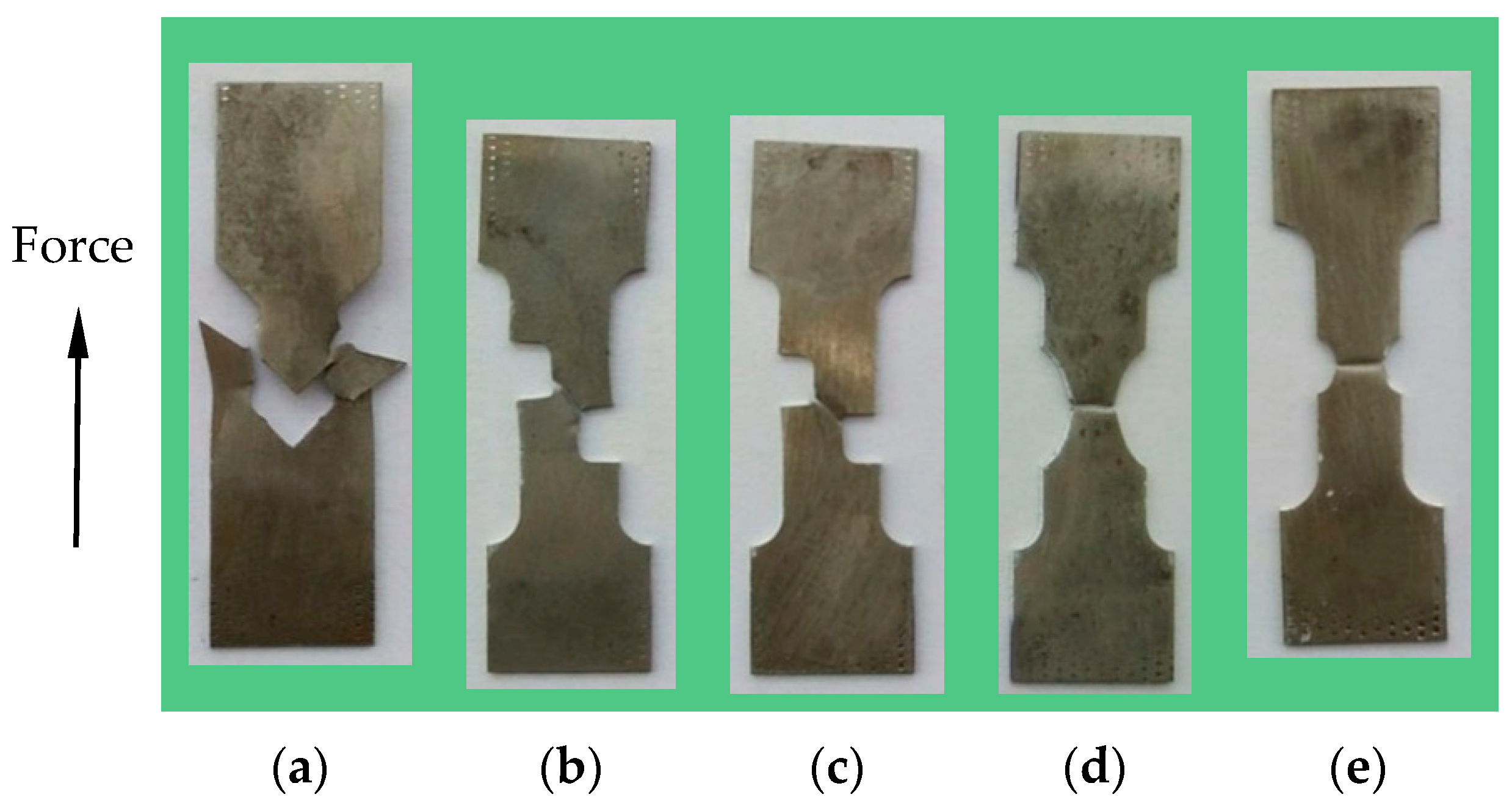
3.1. Macroscopic Fracture Behavior
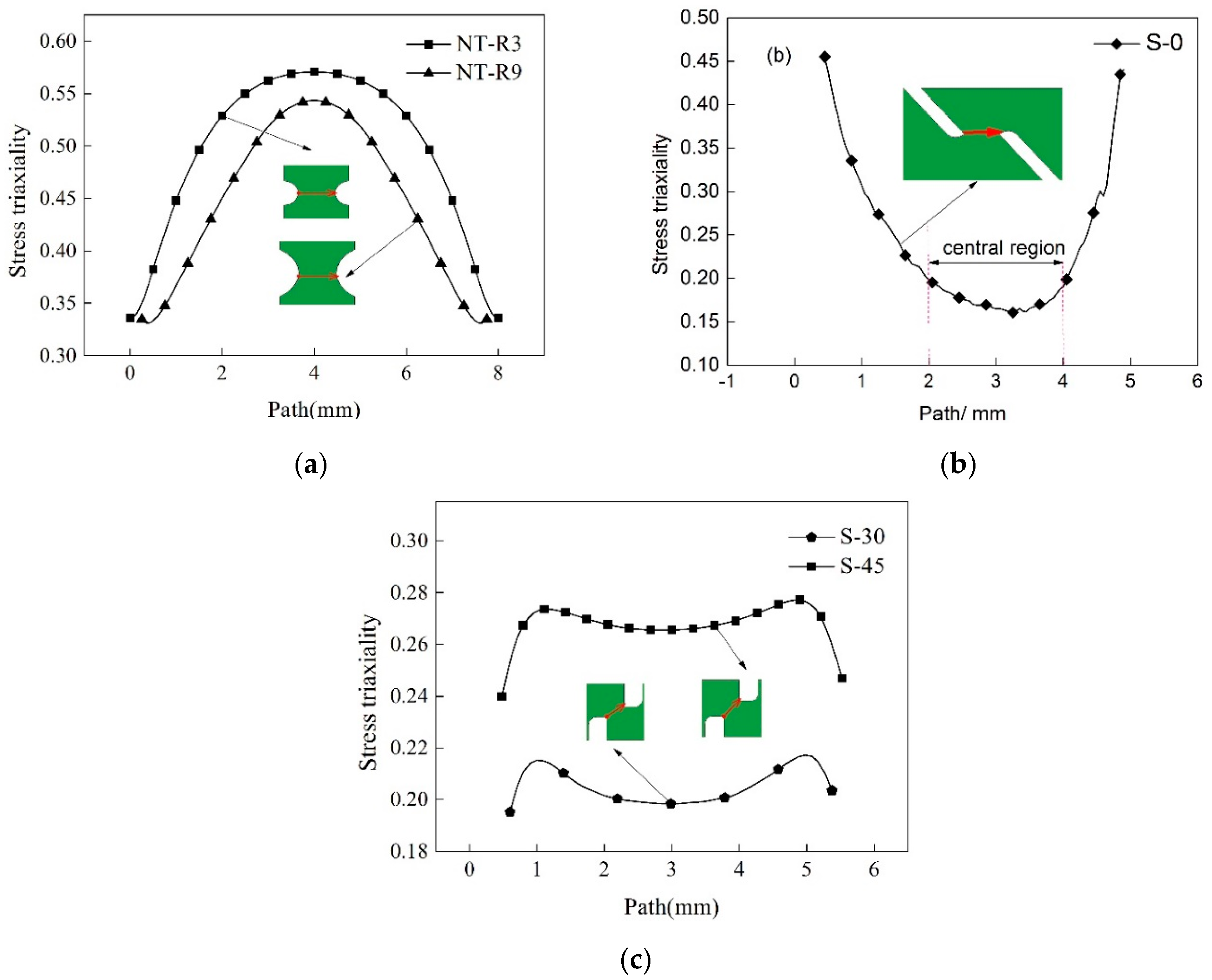
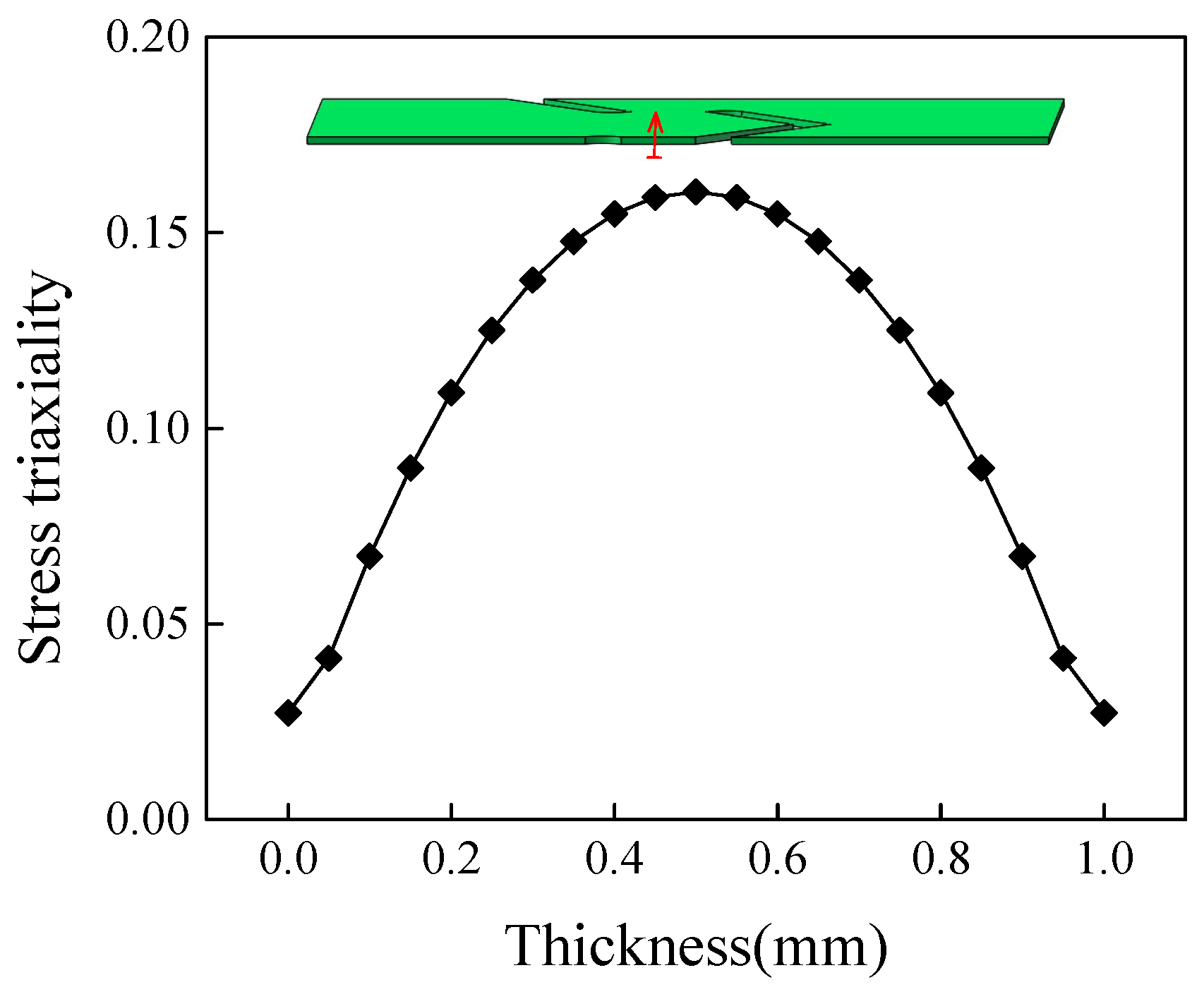
3.2. Stress Triaxiality Evolution
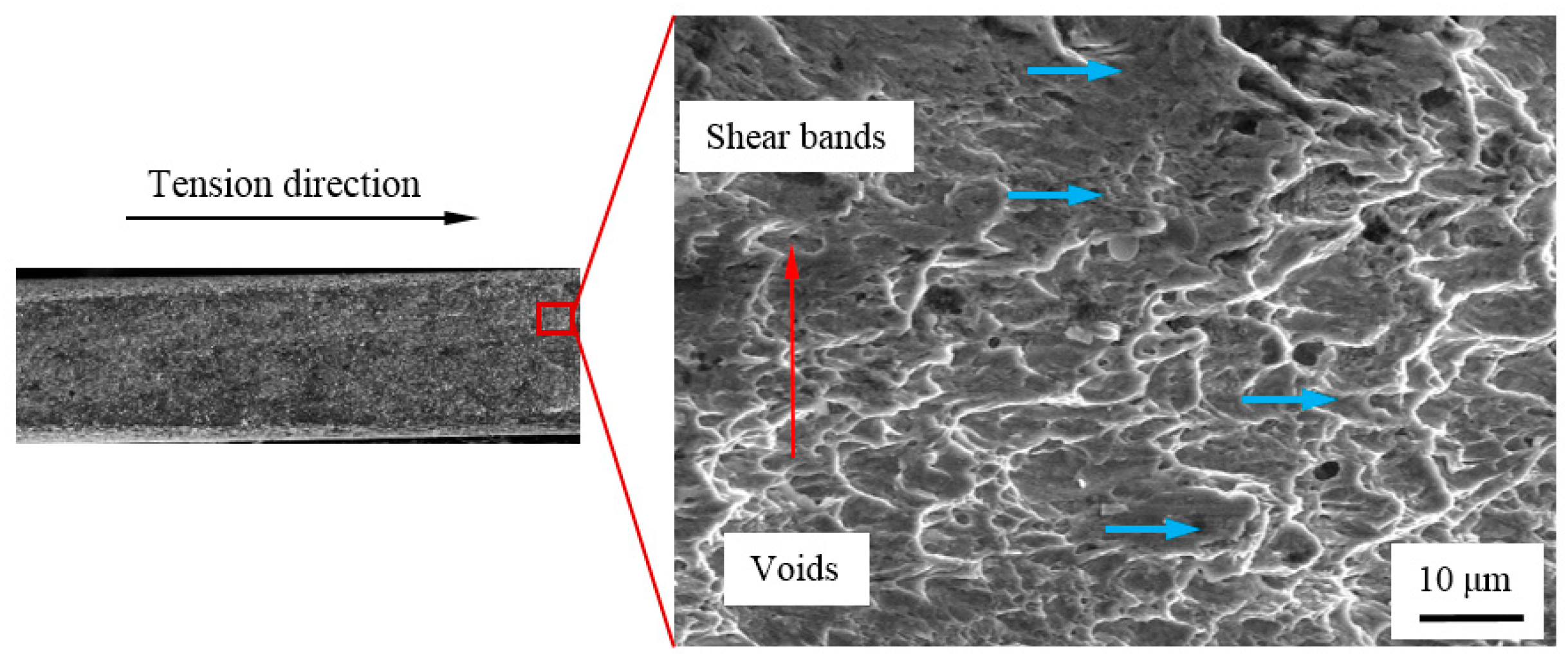
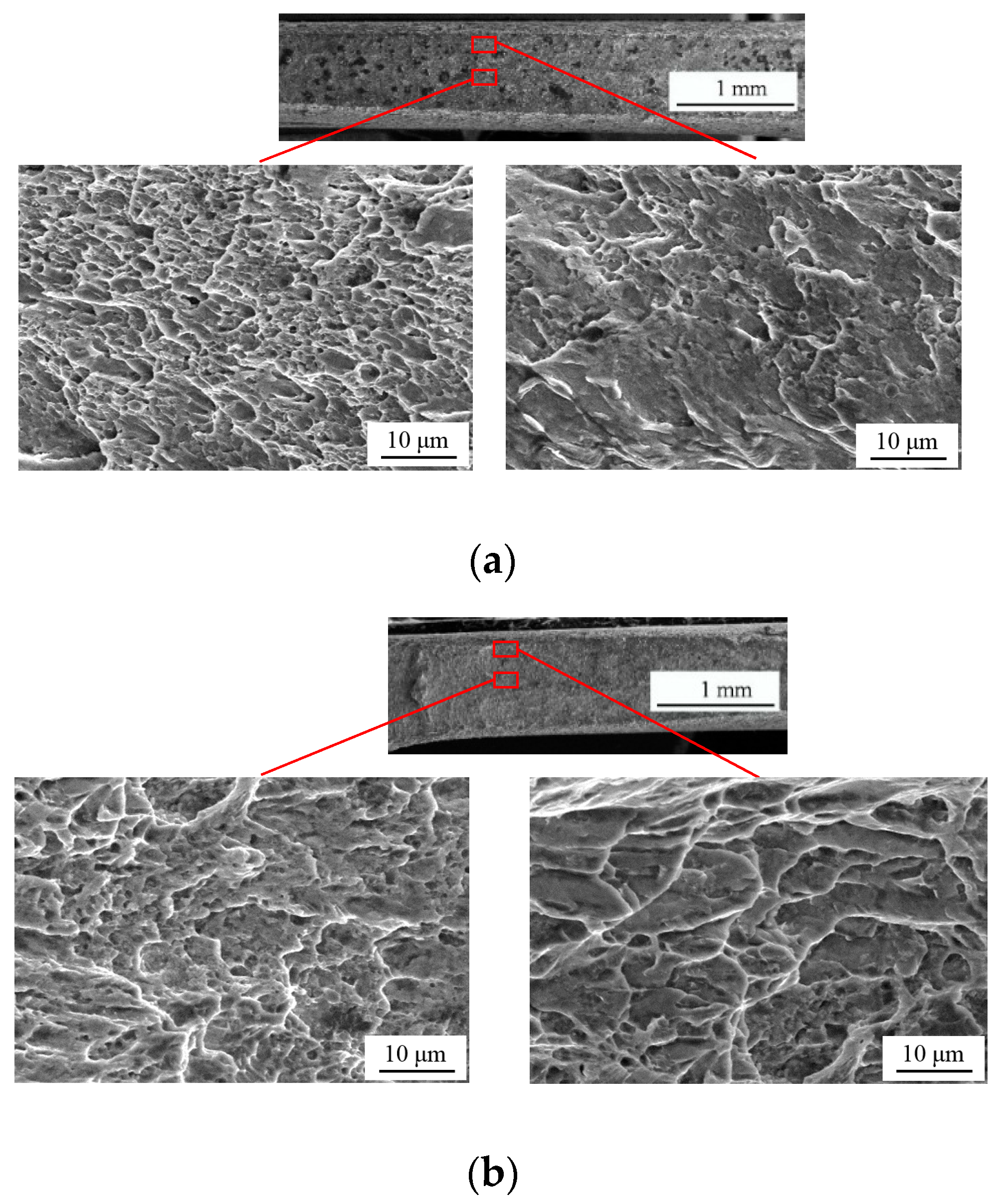
3.3. Microscopic Fracture Mechanism
3.4. Analytical Relationship between Void Size and Stress Triaxiality
4. Conclusions
- Obvious necking localization occurred in two notched specimens of NT-R3 and NT-R9. The corresponding void-dominated fracture has a macroscopic appearance with numerical voids in the central area of the fracture area and an oblique appearance at the limited edge area, whereas in the shear specimen S-0 very minor voids appeared on the smooth fracture surface and therefore shear fracture occurred. As designed intermediate shear-type specimens of S-30 and S-45, the corresponding fracture phenomena changed with a feature characterized by an increasing number of voids with growing size as well as decreasing shear-slip appearance.
- The stress triaxiality influenced deformation mode (tension-dominated deformation, shear deformation, shear-dominated deformation) and thus induced various fracture mechanisms (void-dominated fracture, shear-slip dominated fracture). An increase in stress triaxiality (especially higher than 1/3) enhanced voids growth effect and made voids-dominated fracture with significant necking occur easily. With the decrease of stress triaxiality from 1/3 to 0, the micro-fractography of shear-slip increased and the fracture mode gradually shifted from void-dominated fracture to shear fracture.
- Increasing stress triaxiality promoted voids growth for the tension-dominated range with stress triaxiality larger than 0.33. For the stress triaxiality range 0.11(specimen S-0) to 0.33 (specimen DB), the interaction of shear and tension effect initially increased void sizes from 1 μm to 1.9 μm but the trend of growth weakened again. This variation tendency of void size with stress triaxiality coincided well with that of fracture strain. The void radius of different stress states can be well predicted by the Rice–Tracey model with all relative errors lower than 13%.
Author Contributions
Funding
Conflicts of Interest
References
- Li, Y.; Luo, M.; Gerlach, J.; Wierzbicki, T. Prediction of shear-induced fracture in sheet metal forming. J. Mater. Process. Technol. 2010, 210, 1858–1869. [Google Scholar] [CrossRef]
- Rice, J.R.; Tracey, D.M. On the ductile enlargement of voids in triaxial stress fields. J. Mech. Phys. Solids 1969, 17, 201–217. [Google Scholar] [CrossRef]
- Brozzo, P.; Deluca, B.; Rendina, R. A new method for the prediction of formability limits in metal sheets. In Proceedings of the 7th Biennial Conference of the International Deep Drawing Research Group, Amsterdam, The Netherlands, 9–13 October 1972. [Google Scholar]
- Bai, Y.; Wierzbicki, T. Application of extended Mohr-Coulomb criterion to ductile fracture. Int. J. Fract. 2010, 161, 1–20. [Google Scholar] [CrossRef]
- Malcher, L.; Andrade Pires, F.M.; César De Sá, J.M.A. An extended GTN model for ductile fracture under high and low stress triaxiality. Int. J. Plast. 2014, 54, 193–228. [Google Scholar] [CrossRef]
- Brünig, M.; Chyra, O.; Albrecht, D.; Driemeier, L.; Alves, M. A ductile damage criterion at various stress triaxialities. Int. J. Plast. 2008, 24, 1731–1755. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. On fracture locus in the equivalent strain and stress triaxiality space. Int. J. Mech. Sci. 2004, 46, 81–98. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. A Comparative Study on Various Ductile Crack Formation Criteria. J. Eng. Mater. Technol. 2004, 126, 314. [Google Scholar] [CrossRef]
- Li, H.; Fu, M.W.; Lu, J.; Yang, H. Ductile fracture: Experiments and computations. Int. J. Plast. 2011, 27, 147–180. [Google Scholar] [CrossRef]
- Xue, L. Constitutive modeling of void shearing effect in ductile fracture of porous materials. Eng. Fract. Mech. 2008, 75, 3343–3366. [Google Scholar] [CrossRef]
- Gao, X.; Zhang, T.; Zhou, J.; Graham, S.M.; Hayden, M.; Roe, C. On stress-state dependent plasticity modeling: Significance of the hydrostatic stress, the third invariant of stress deviator and the non-associated flow rule. Int. J. Plast. 2011, 27, 217–231. [Google Scholar] [CrossRef]
- Brünig, M.; Albrecht, D.; Gerke, S. Modeling of ductile damage and fracture behavior based on different micromechanisms. Int. J. Damage Mech. 2011, 20, 558–577. [Google Scholar] [CrossRef]
- Qian, L.Y.; Fang, G.; Zeng, P.; Wang, Q. Experimental and numerical investigations into the ductile fracture during the forming of flat-rolled 5083-O aluminum alloy sheet. J. Mater. Process. Technol. 2015, 220, 264–275. [Google Scholar] [CrossRef]
- Qian, L.; Paredes, M.; Wierzbicki, T.; Sparrer, Y.; Feuerstein, M.; Zeng, P.; Fang, G. Experimental and numerical study on shear-punch test of 6060 T6 extruded aluminum profile. Int. J. Mech. Sci. 2016, 118, 205–218. [Google Scholar] [CrossRef]
- Luo, M.; Wierzbicki, T. Numerical failure analysis of a stretch-bending test on dual-phase steel sheets using a phenomenological fracture model. Int. J. Solids Struct. 2010, 47, 3084–3102. [Google Scholar] [CrossRef] [Green Version]
- Lou, Y.; Huh, H.; Lim, S.; Pack, K. New ductile fracture criterion for prediction of fracture forming limit diagrams of sheet metals. Int. J. Solids Struct. 2012, 49, 3605–3615. [Google Scholar] [CrossRef] [Green Version]
- Lou, Y.; Huh, H. Extension of a shear-controlled ductile fracture model considering the stress triaxiality and the Lode parameter. Int. J. Solids Struct. 2013, 50, 447–455. [Google Scholar] [CrossRef] [Green Version]
- Kubík, P.; Šebek, F.; Hůlka, J.; Petruška, J. Calibration of ductile fracture criteria at negative stress triaxiality. Int. J. Mech. Sci. 2016, 108–109, 90–103. [Google Scholar] [CrossRef]
- Lou, Y.; Yoon, J.W.; Huh, H. Modeling of shear ductile fracture considering a changeable cut-off value for stress triaxiality. Int. J. Plast. 2014, 54, 56–80. [Google Scholar] [CrossRef]
- Brünig, M.; Gerke, S.; Schmidt, M. Damage and failure at negative stress triaxialities: Experiments, modeling and numerical simulations. Int. J. Plast. 2018, 102, 70–82. [Google Scholar] [CrossRef]
- Khan, A.S.; Liu, H. Strain rate and temperature dependent fracture criteria for isotropic and anisotropic metals. Int. J. Plast. 2012, 37, 1–15. [Google Scholar] [CrossRef]
- Zhu, H.; Zhu, L.; Chen, J.H.; Lv, X.F. Investigation of fracture mechanism of 6063 aluminum alloy under different stress states. Int. J. Fract. 2007, 146, 159–172. [Google Scholar] [CrossRef]
- Lou, Y.; Yoon, J.W.; Huh, H.; Chao, Q.; Song, J.H. Correlation of the maximum shear stress with micro-mechanisms of ductile fracture for metals with high strength-to-weight ratio. Int. J. Mech. Sci. 2018, 146–147, 583–601. [Google Scholar] [CrossRef]
- Ghajar, R.; Mirone, G.; Keshavarz, A. Ductile failure of X100 pipeline steel–Experiments and fractography. Mater. Des. 2013, 43, 513–525. [Google Scholar] [CrossRef]
- Rousselier, G.; Luo, M. A fully coupled void damage and Mohr-Coulomb based ductile fracture model in the framework of a Reduced Texture Methodology. Int. J. Plast. 2014, 55, 1–24. [Google Scholar] [CrossRef]
- Weck, A.; Wilkinson, D.S. Experimental investigation of void coalescence in metallic sheets containing laser drilled holes. Acta Mater. 2008, 56, 1774–1784. [Google Scholar] [CrossRef]
- Morgeneyer, T.F.; Starink, M.J.; Sinclair, I. Evolution of voids during ductile crack propagation in an aluminium alloy sheet toughness test studied by synchrotron radiation computed tomography. Acta Mater. 2008, 56, 1671–1679. [Google Scholar] [CrossRef] [Green Version]
- Barsoum, I.; Faleskog, J. Micromechanical analysis on the influence of the Lode parameter on void growth and coalescence. Int. J. Solids Struct. 2011, 48, 925–938. [Google Scholar] [CrossRef] [Green Version]
- Brünig, M.; Gerke, S.; Hagenbrock, V. Stress-state-dependence of damage strain rate tensors caused by growth and coalescence of micro-defects. Int. J. Plast. 2014, 63, 49–63. [Google Scholar] [CrossRef]
- Hu, X.; Wilkinson, D.S.; Jain, M.; Mishra, R.K. The influence of particle shape, volume fraction and distribution on post-necking deformation and fracture in uniaxial tension of AA5754 sheet materials. Int. J. Solids Struct. 2009, 46, 2650–2658. [Google Scholar] [CrossRef] [Green Version]
- Brünig, M.; Gerke, S.; Hagenbrock, V. Micro-mechanical studies on the effect of the stress triaxiality and the Lode parameter on ductile damage. Int. J. Plast. 2013, 50, 49–65. [Google Scholar] [CrossRef]
- Mohr, D.; Marcadet, S.J. Micromechanically-motivated phenomenological Hosford-Coulomb model for predicting ductile fracture initiation at low stress triaxialities. Int. J. Solids Struct. 2015, 67–68, 40–55. [Google Scholar] [CrossRef]
- Rakin, M.; Sedmak, A.; Matejic, P.; Zrilic, M.; Sedmak, S. Numerical simulation of ductile fracture initiation by application of Rice-Tracey void growth model. In Proceedings of the ECF13, San Sebastian, Spain, 6–9 September 2000. [Google Scholar]
- Qian, L.; Fang, G.; Zeng, P. Modeling of the ductile fracture during the sheet forming of aluminum alloy considering non-associated constitutive characteristic. Int. J. Mech. Sci. 2017, 126, 55–66. [Google Scholar] [CrossRef]


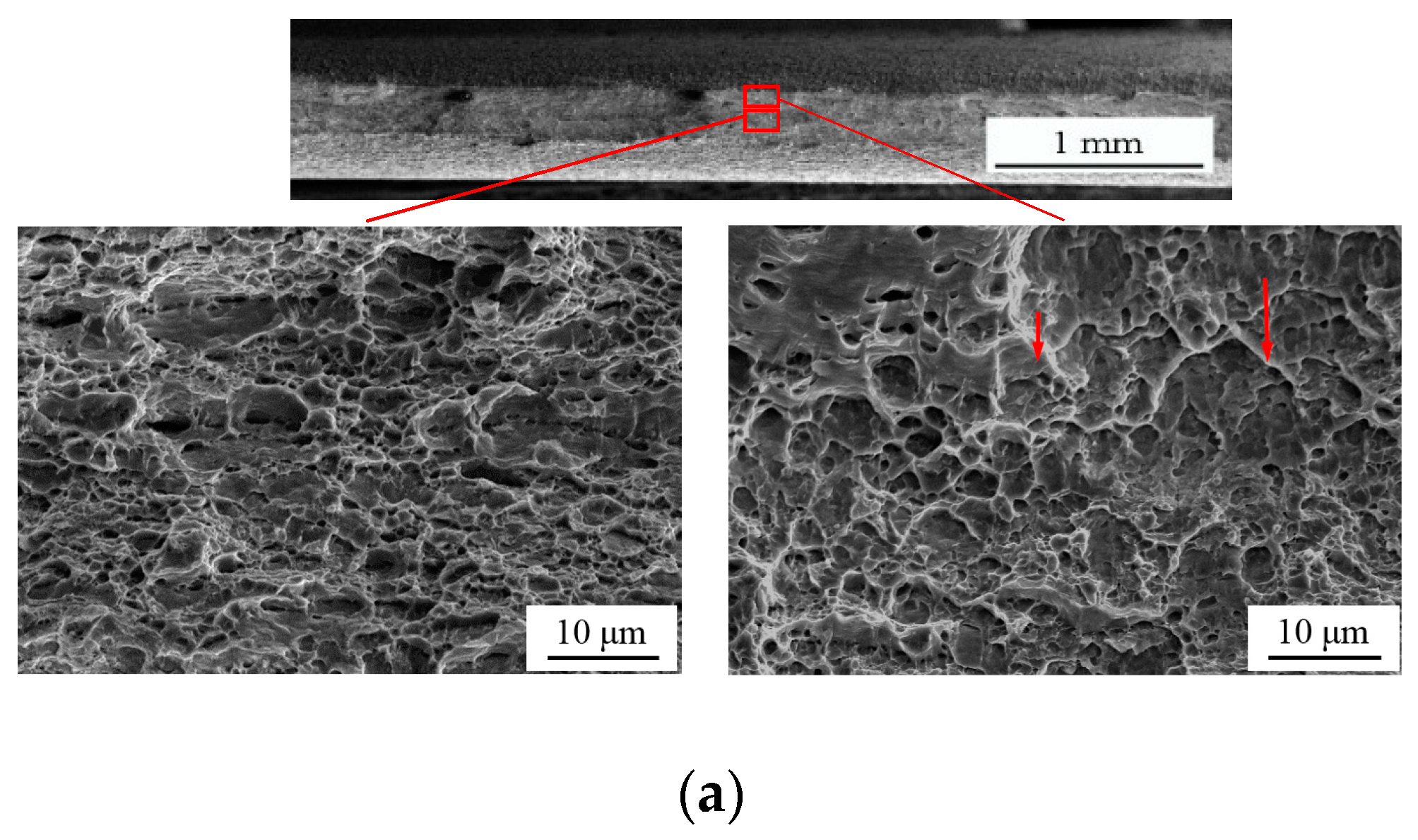
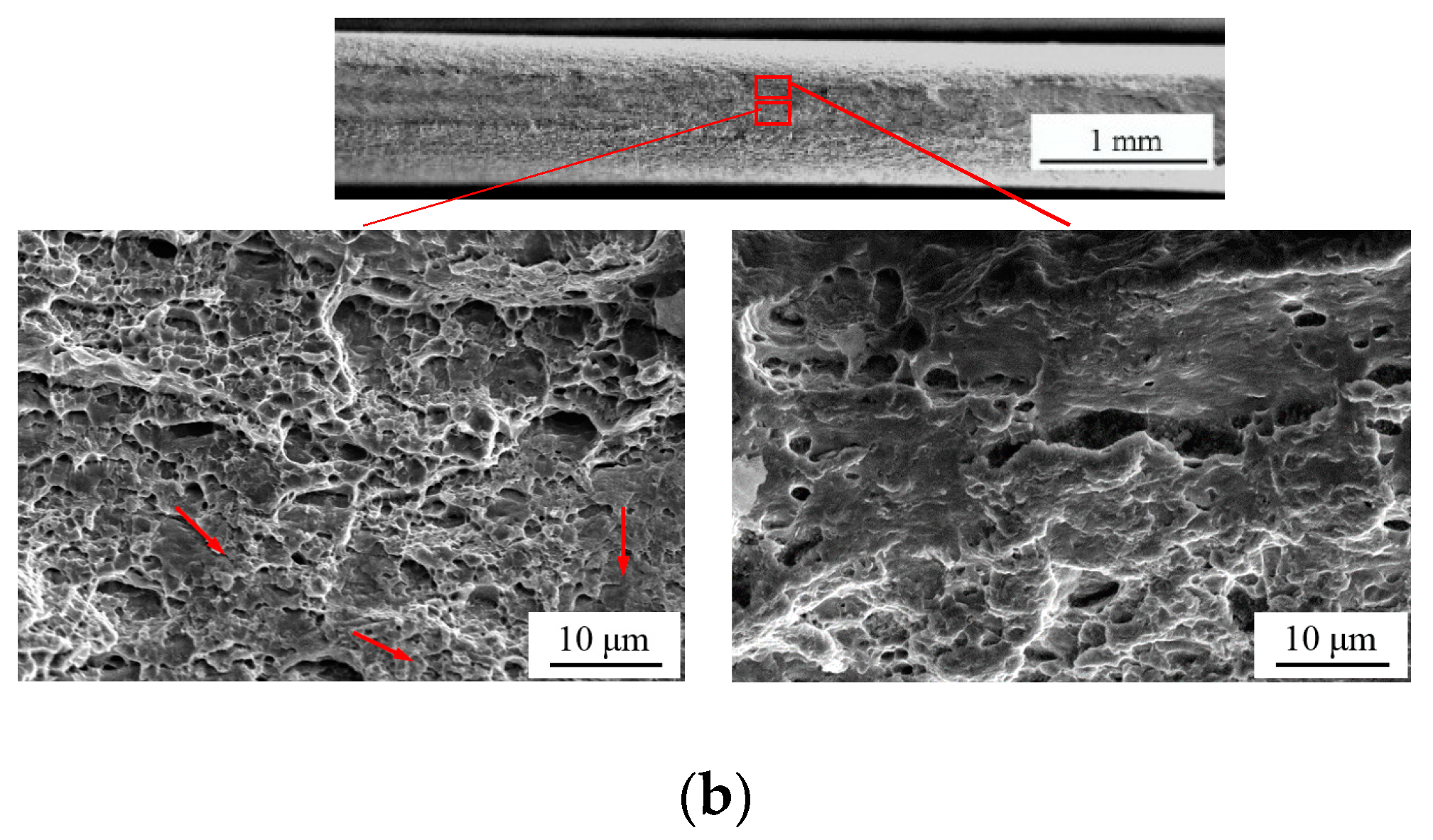


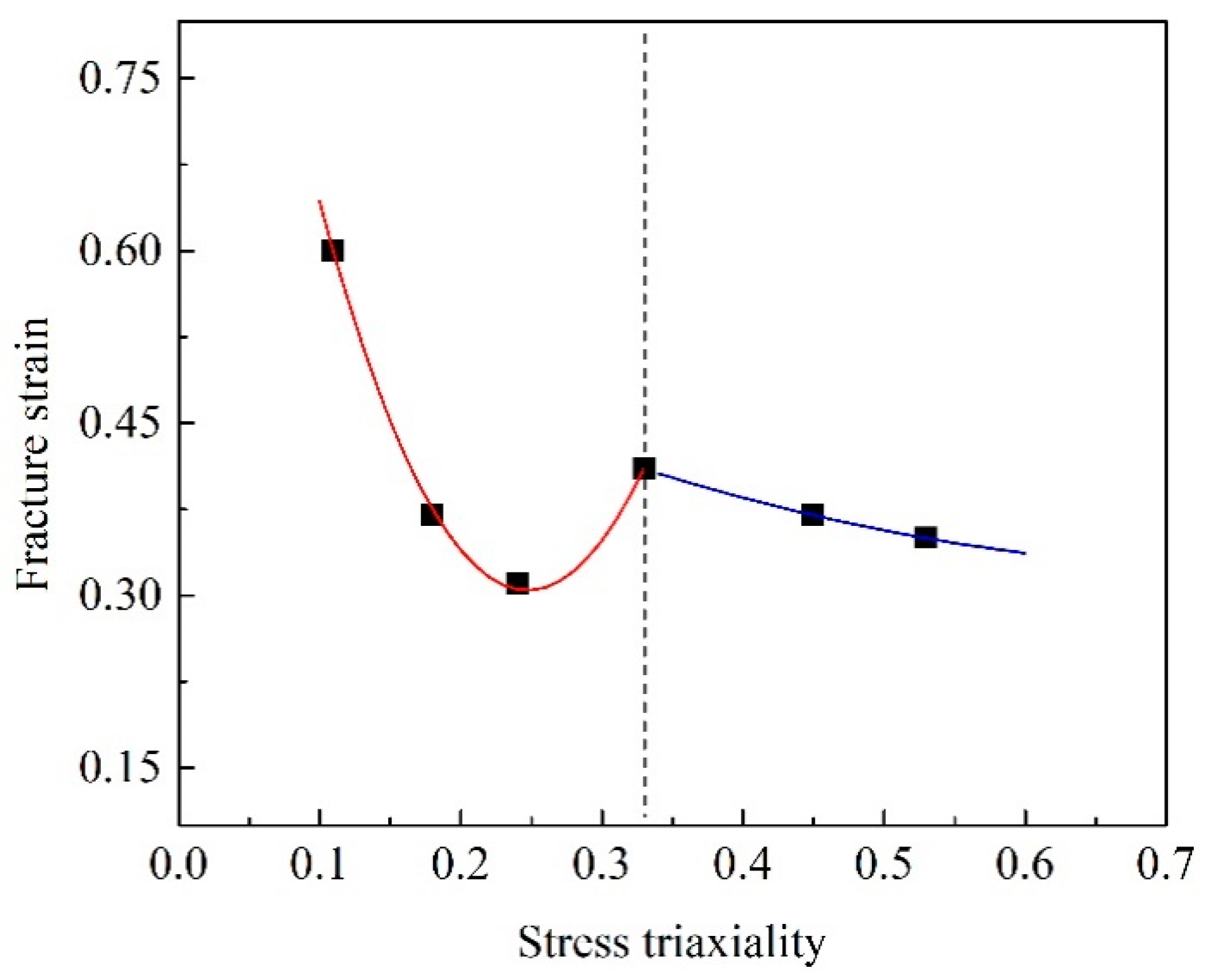
| Item | S-0 | S-30 | S-45 | DB | NT-R9 | NT-R3 |
|---|---|---|---|---|---|---|
| Stress triaxiality | 0.11 | 0.18 | 0.24 | 0.33 | 0.45 | 0.53 |
| Measured void radius Rexp (µm) | 1.00 | 1.71 | 1.90 | 1.55 | 1.71 | 2.13 |
| Calculated void radius Rsim (µm) | 0.87 | 1.58 | 1.79 | 1.50 | 1.78 | 1.99 |
| Relative error ∆ (%) | 13.00 | 7.60 | 5.62 | 3.23 | −4.09 | 6.57 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qian, L.; Wang, X.; Sun, C.; Dai, A. Correlation of Macroscopic Fracture Behavior with Microscopic Fracture Mechanism for AHSS Sheet. Materials 2019, 12, 900. https://doi.org/10.3390/ma12060900
Qian L, Wang X, Sun C, Dai A. Correlation of Macroscopic Fracture Behavior with Microscopic Fracture Mechanism for AHSS Sheet. Materials. 2019; 12(6):900. https://doi.org/10.3390/ma12060900
Chicago/Turabian StyleQian, Lingyun, Xiaocan Wang, Chaoyang Sun, and Anyi Dai. 2019. "Correlation of Macroscopic Fracture Behavior with Microscopic Fracture Mechanism for AHSS Sheet" Materials 12, no. 6: 900. https://doi.org/10.3390/ma12060900
APA StyleQian, L., Wang, X., Sun, C., & Dai, A. (2019). Correlation of Macroscopic Fracture Behavior with Microscopic Fracture Mechanism for AHSS Sheet. Materials, 12(6), 900. https://doi.org/10.3390/ma12060900






