First-Principle Calculation on Inelastic Electron Scattering in Diamond and Graphite
Abstract
:1. Introduction
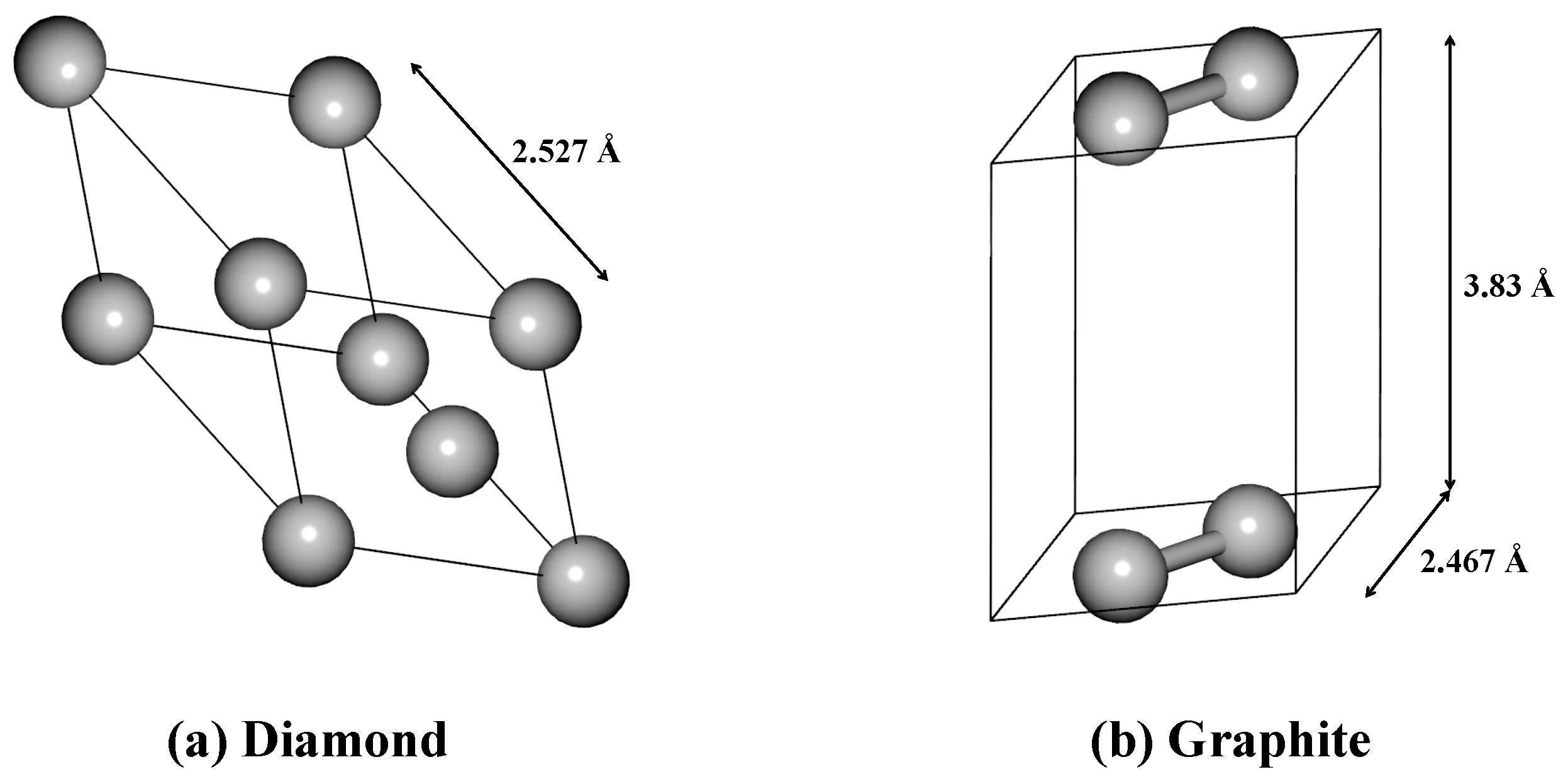
2. Methods and Settings
3. Results and Discussion
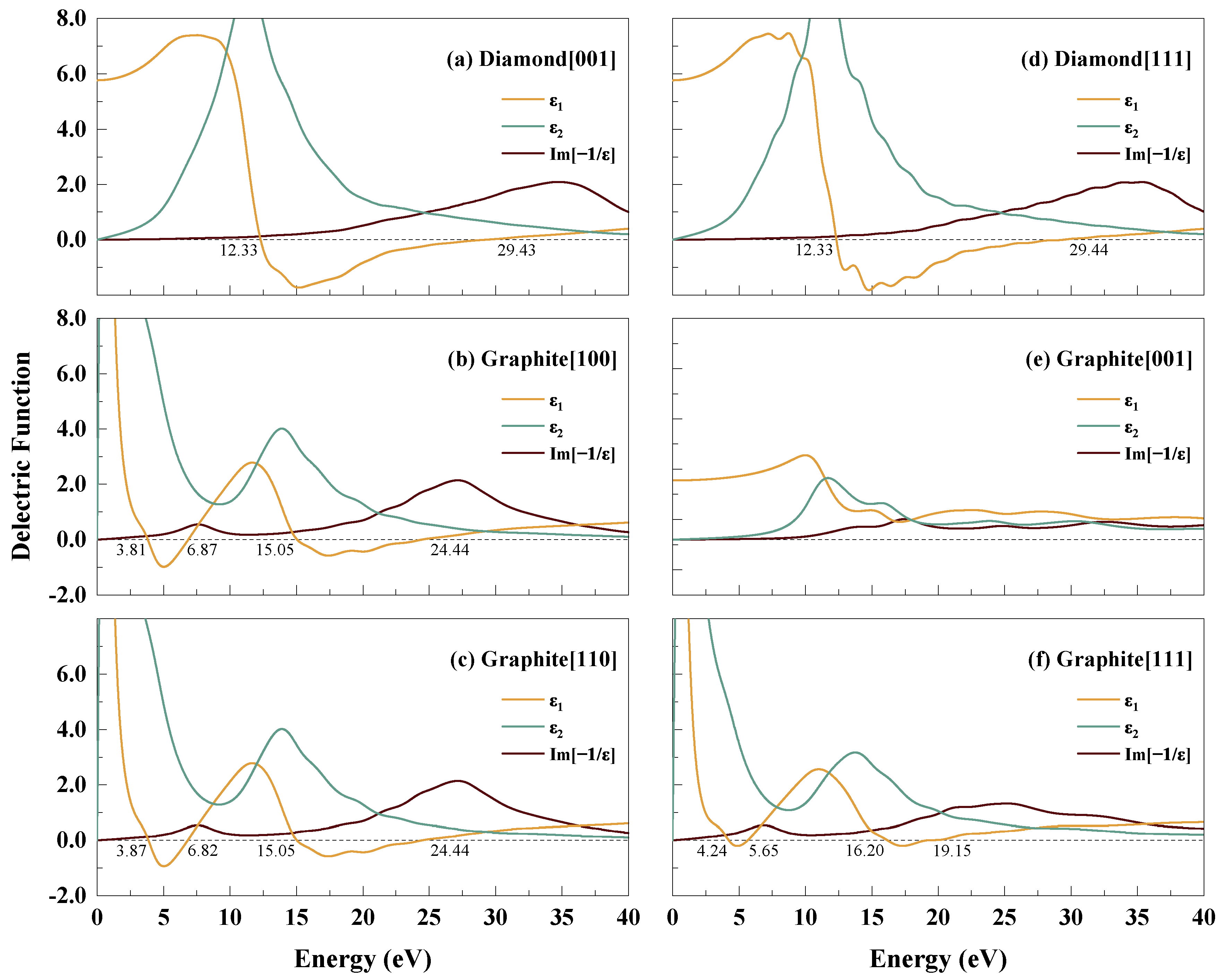
3.1. Dielectric Functions
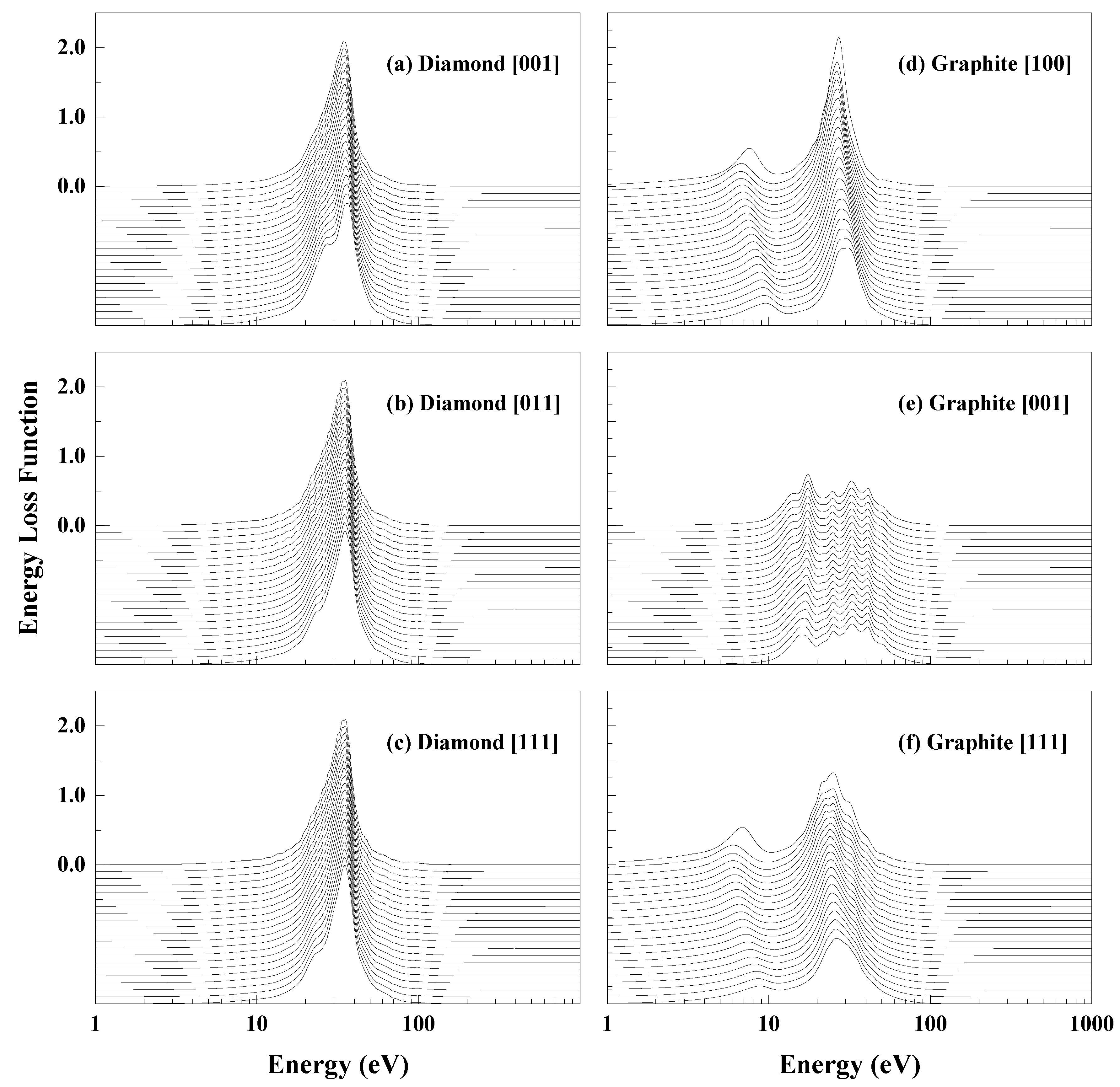
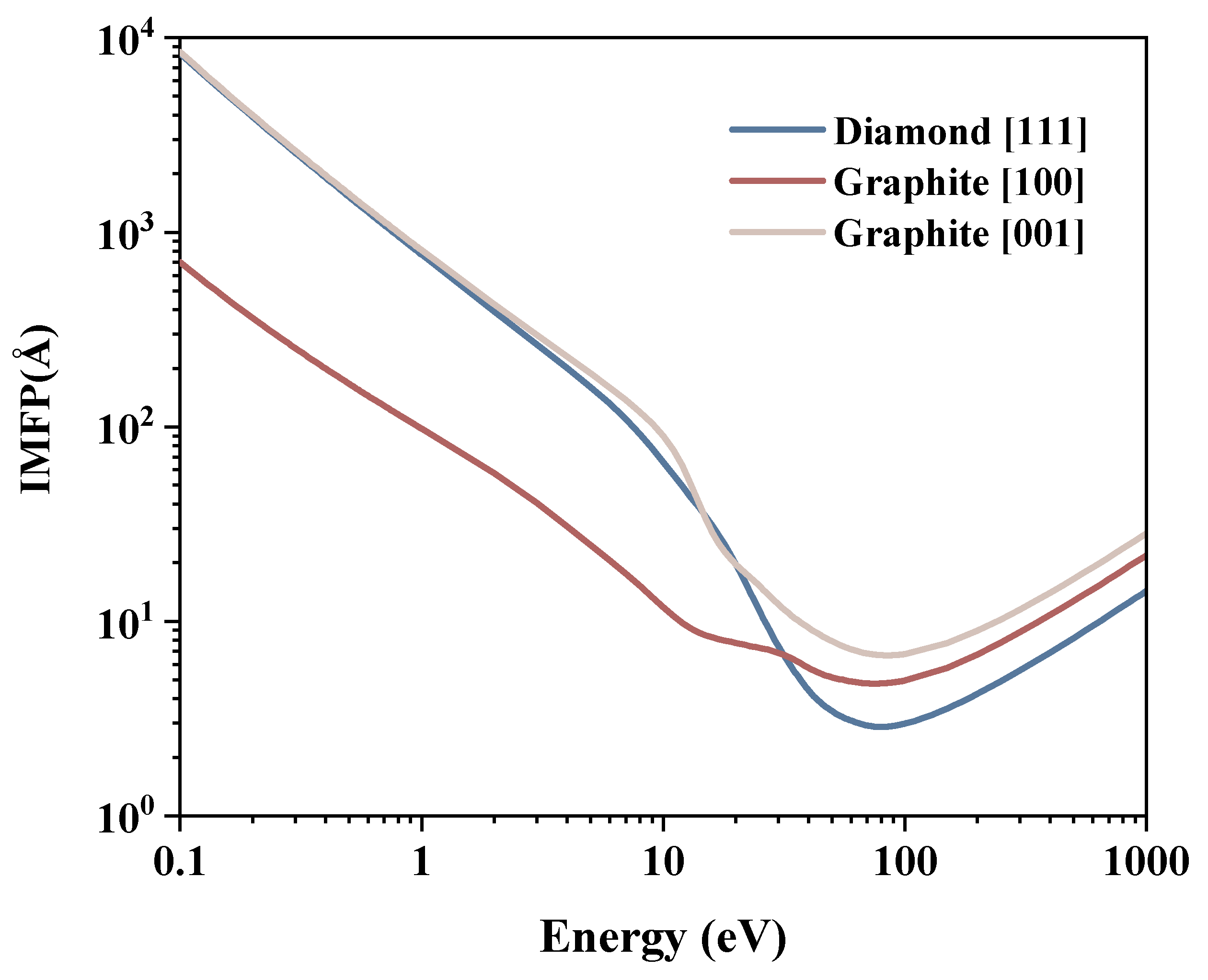
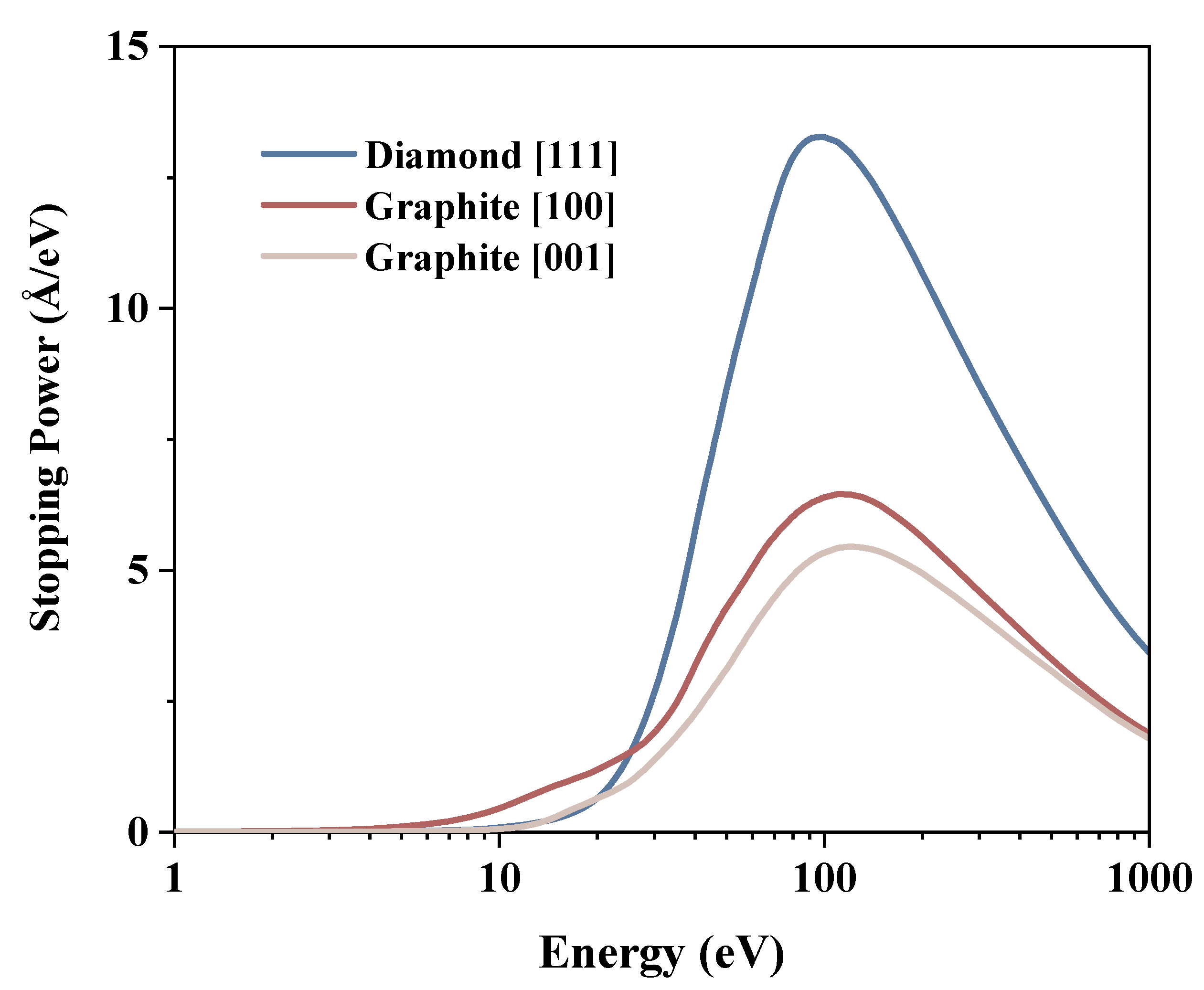
3.2. Transport of Energetic Electrons
3.3. Secondary Electron Excitation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mendes, J.C.; Liehr, M.; Li, C. Diamond/GaN HEMTs: Where from and Where to? Materials 2022, 15, 415. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wang, Y.; Wang, S.; Wei, W.; Wang, Y. Comparison of Carbon Thin Films with Low Secondary Electron Yield Deposited in Neon and Argon. Coatings 2020, 10, 884. [Google Scholar] [CrossRef]
- Cao, M.; Zhang, X.S.; Liu, W.H.; Wang, H.G.; Li, Y.D. Secondary electron emission of graphene-coated copper. Diam. Relat. Mater. 2016, 73, 199–203. [Google Scholar] [CrossRef]
- Salemme, R.; Baglin, V.; Bregliozzi, G.; Chiggiato, P.; Kersevan, R. Amorphous carbon coatings at cryogenic temperatures with LHC type beams: First results with the COLDEX experiment. In Proceedings of the 6th International Particle Accelerator Conference (IPAC’ 15), Richmond, VA, USA, 3–8 May 2015. [Google Scholar]
- Wei, K.; Wang, R.; Li, J.; Liu, B.; Wang, H. Secondary electron emission properties of double-layer B-doped diamond films. Diam. Relat. Mater. 2020, 106, 107826. [Google Scholar] [CrossRef]
- Wei, K.; Li, J.; Liu, B.; Wu, R.; Wang, H. Effect of Hydrogen Plasma Treatment on Secondary Electron Emission Properties of Polycrystalline Diamond Films. Vacuum 2019, 172, 109046. [Google Scholar] [CrossRef]
- Cimino, R.; Commisso, M.; Grosso, D.R.; Demma, T.; Baglin, V.; Flammini, R.; Larciprete, R. Nature of the Decrease of the Secondary-Electron Yield by Electron Bombardment and its Energy Dependence. Phys. Rev. Lett. 2012, 109, 64801. [Google Scholar] [CrossRef]
- Penn, D.R. Electron mean-free-path calculations using a model dielectric function. Phys. Rev. B Condens. Matter 1987, 35, 482. [Google Scholar] [CrossRef]
- Ding, Z.J.; Li, H.M.; Tang, X.D.; Shimizu, R. Monte Carlo simulation of absolute secondary electron yield of Cu. Appl. Phys. A 2004, 78, 585–587. [Google Scholar] [CrossRef]
- Ding, Z.J.; Tang, X.D. Monte Carlo study of secondary electron emission. J. Appl. Phys. 2001, 89, 718. [Google Scholar] [CrossRef]
- Ding, Z.J.; Shimizu, R. A Monte Carlo Modeling of Electron Interaction with Solids Including Cascade Secondary Electron Production. Scanning 1996, 18, 92–113. [Google Scholar] [CrossRef]
- Mao, S.F.; Li, Y.G.; Zeng, R.G.; Ding, Z.J. Electron inelastic scattering and secondary electron emission calculated without the single pole approximation. J. Appl. Phys. 2008, 104, 251. [Google Scholar] [CrossRef]
- Da, B.; Shinotsuka, H.; Yoshikawa, H.; Ding, Z.J.; Tanuma, S. Extended Mermin Method for Calculating the Electron Inelastic Mean Free Path. Phys. Rev. Lett. 2014, 113, 063201. [Google Scholar] [CrossRef] [PubMed]
- Polak, M.P.; Morgan, D. MAST-SEY: MAterial Simulation Toolkit for Secondary Electron Yield. A monte carlo approach to secondary electron emission based on complex dielectric functions. Comput. Mater. Sci. 2021, 193, 110281. [Google Scholar] [CrossRef]
- Gutierrez, R.E.; Matanovic, I.; Polak, M.P.; Johnson, R.S.; Morgan, D.; Schamiloglu, E. First principles inelastic mean free paths coupled with Monte Carlo simulation of secondary electron yield of Cu-Ni, Cu-Zn, and Mo-Li. J. Appl. Phys. 2021, 129, 175105. [Google Scholar] [CrossRef]
- Materials Project. Available online: https://materialsproject.org/ (accessed on 11 January 2022).
- Giannozzi, P.; Baroni, S.; Bonini, N.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Chiarotti, G.L.; Cococcioni, M.; Dabo, I.; et al. QUANTUM ESPRESSO: A modular and open-source software project for quantum simulations of materials. J. Phys. Condens. Matter 2009, 21, 395502. [Google Scholar] [CrossRef]
- Giannozzi, P.; Andreussi, O.; Brumme, T.; Bunau, O.; Nardelli, M.B.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Cococcioni, M.; et al. Advanced capabilities for materials modelling with Quantum ESPRESSO. J. Phys. Condens. Matter 2017, 29, 465901. [Google Scholar] [CrossRef] [Green Version]
- QUANTUMESPRESSO—QUANTUMESPRESSO. Available online: https://www.quantum-espresso.org/. (accessed on 8 March 2022).
- Perdew, J.P.; Chevary, J.A.; Vosko, S.H.; Jackson, K.A.; Pederson, M.R.; Singh, D.J.; Fiolhais, C. Erratum: Atoms, molecules, solids, and surfaces: Applications of the generalized gradient approximation for exchange and correlation. Phys. Rev. B 1993, 48, 4978. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [Green Version]
- Rappe, A.M.; Rabe, K.M.; Kaxiras, E.; Joannopoulos, J. Optimized pseudopotentials. Phys. Rev. B 1990, 41, 1227. [Google Scholar] [CrossRef]
- Vanderbilt, D. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B 1990, 41, 7892. [Google Scholar] [CrossRef]
- Corso, A.D. Pseudopotentials periodic table: From H to Pu. Comput. Mater. Sci. 2014, 95, 337–350. [Google Scholar] [CrossRef]
- Timrov, I.; Vast, N.; Gebauer, R.; Baroni, S. Electron energy-loss and inelastic X-ray scattering cross sections from time-dependent density-functional perturbation theory. Phys. Rev. B Condens. Matter 2013, 88, 064301. [Google Scholar] [CrossRef] [Green Version]
- Timrov, I.; Vast, N.; Gebauer, R.; Baroni, S. turboEELS—A code for the simulation of the electron energy loss and inelastic X-ray scattering spectra using the Liouville–Lanczos approach to time-dependent density-functional perturbation theory. Comput. Phys. Commun. 2015, 196, 460–469. [Google Scholar] [CrossRef]
- Motornyi, O.; Vast, N.; Timrov, I.; Baseggio, O.; Baroni, S.; Dal Corso, A. Electron energy loss spectroscopy of bulk gold with ultrasoft pseudopotentials and the Liouville-Lanczos method. Phys. Rev. B 2020, 102, 035156. [Google Scholar] [CrossRef]
- Alkauskas, A.; Schneider, S.D.; Hebert, C.; Sagmeister, S.; Draxl, C. Dynamic structure factors of Cu, Ag, and Au: Comparative study from first principles. Phys. Rev. B 2013, 88, 195124. [Google Scholar] [CrossRef] [Green Version]
- Alkauskas, A.; Schneider, S.D.; Sagmeister, S.; Ambrosch-Draxl, C.; Hébert, C. Theoretical analysis of the momentum-dependent loss function of bulk Ag. Ultramicroscopy 2010, 110, 1081–1086. [Google Scholar] [CrossRef]
- Timrov, I.; Markov, M.; Gorni, T.; Raynaud, M.; Motornyi, O.; Gebauer, R.; Baroni, S.; Vast, N. Ab initio study of electron energy loss spectra of bulk bismuth up to 100 eV. Phys. Rev. B 2017, 95, 094301. [Google Scholar] [CrossRef]
- Waidmann, S.; Knupfer, M.; Arnold, B.; Fink, J.; Fleszar, A.; Hanke, W. Local-field effects and anisotropic plasmon dispersion in diamond. Phys. Rev. B Condens. Matter 2000, 61, 10149. [Google Scholar] [CrossRef]
- Bourke, J.D.; Chantler, C.T. Momentum-Dependent Lifetime Broadening of Electron Energy Loss Spectra: A Self-Consistent Coupled-Plasmon Model. J. Phys. Chem. Lett. 2015, 6, 314–319. [Google Scholar] [CrossRef]
- Tanuma, S.; Powell, C.J.; Penn, D.R. Calculations of electron inelastic mean free paths. Surf. Interface Anal. 2005, 37, 1–14. [Google Scholar] [CrossRef]
- Tanuma, S.; Powell, C.J.; Penn, D.R. Calculations of stopping powers of 100 eV–30 keV electrons in 31 elemental solids. J. Appl. Phys. 2008, 103, 063707. [Google Scholar] [CrossRef]
- Szajman, J.; Liesegang, J.; Jenkin, J.G.; Leckey, R. Is there a universal mean-free-path curve for electron inelastic scattering in solids? J. Electron Spectrosc. Relat. Phenom. 1981, 23, 97–102. [Google Scholar] [CrossRef]
- Bellissimo, A.; Pierantozzi, G.M.; Ruocco, A.; Stefani, G.; Taborelli, M. Secondary Electron Generation Mechanisms in Carbon Allotropes at Low Impact Electron Energies. J. Electron Spectrosc. Relat. Phenom. 2019, 241, 146883. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, R.-Q.; Cao, M.; Li, Y.-D. First-Principle Calculation on Inelastic Electron Scattering in Diamond and Graphite. Materials 2022, 15, 3315. https://doi.org/10.3390/ma15093315
Yan R-Q, Cao M, Li Y-D. First-Principle Calculation on Inelastic Electron Scattering in Diamond and Graphite. Materials. 2022; 15(9):3315. https://doi.org/10.3390/ma15093315
Chicago/Turabian StyleYan, Run-Qi, Meng Cao, and Yong-Dong Li. 2022. "First-Principle Calculation on Inelastic Electron Scattering in Diamond and Graphite" Materials 15, no. 9: 3315. https://doi.org/10.3390/ma15093315





