Research on the Yellow River Basin Energy Structure Transformation Path under the “Double Carbon” Goal
Abstract
:1. Introduction
- (1)
- Considering the energy resource endowment characteristics of the Yellow River Basin, this paper proposes an energy structure transformation path for the inclusive development of traditional and renewable, clean energy in the Yellow River Basin;
- (2)
- Unlike most studies, this paper reflects the impact of energy system resilience on local governments and energy companies in the payoff matrix. It explores the impact of increased energy resilience on the strategy choice of participating subjects;
- (3)
- This study provides a new perspective to explore the impact of the introduction of penalty parameters on energy structure transformation in the Yellow River Basin, while emphasizing the “back-up guaranteed” by coal in the Yellow River Basin.
2. Literature Review
2.1. Energy Transition Studies
2.2. Evolutionary Game
3. Methodology
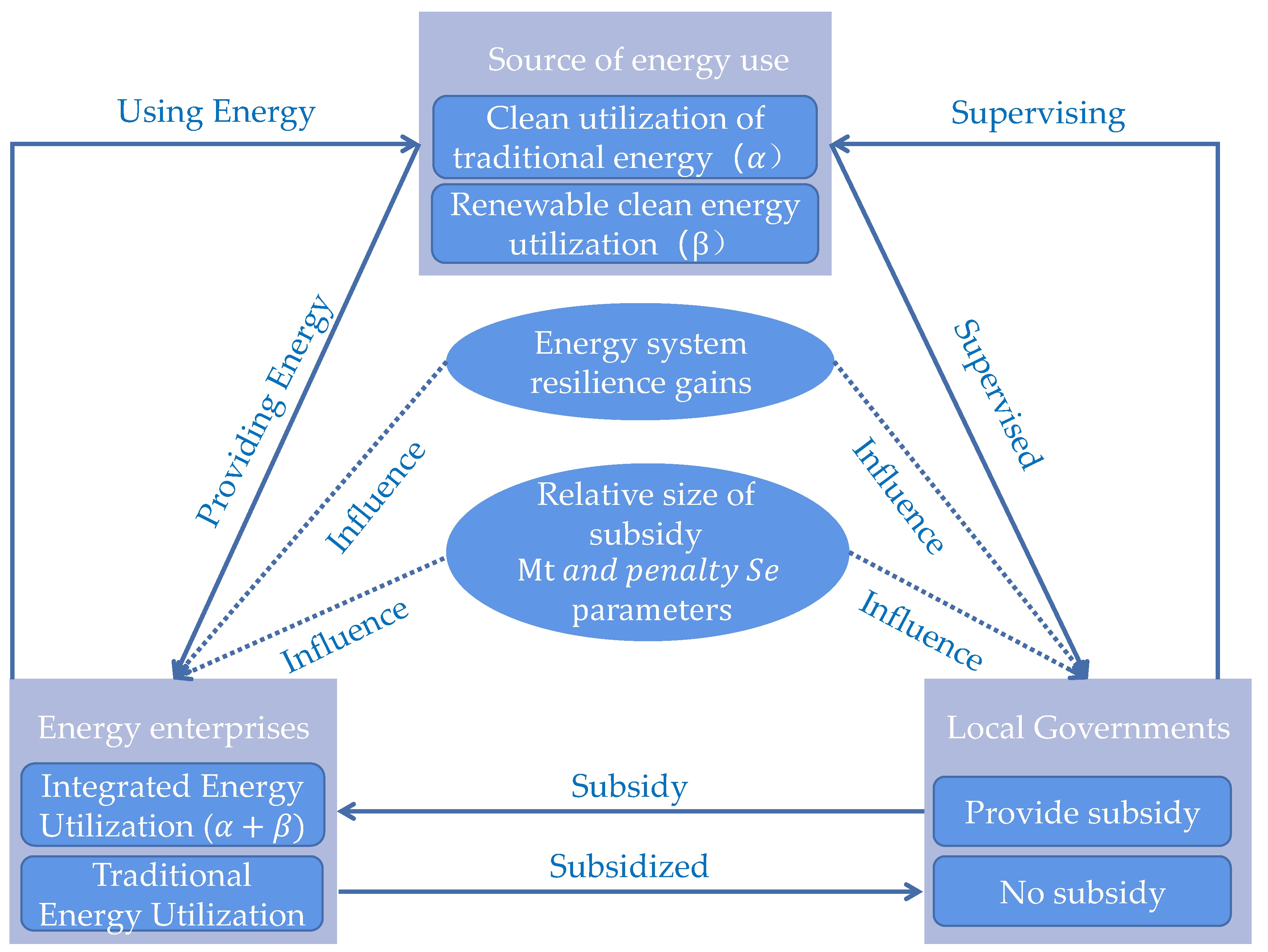
3.1. Evolutionary Game Model Construction
3.1.1. Model Hypothesis
3.1.2. Game Matrix
3.2. Evolutionary Equilibrium Analysis
3.2.1. Stability Conditions for the Evolution of Energy Companies
3.2.2. Stability Conditions for the Evolution of Local Governments
3.2.3. Stability Analysis of Replication Dynamics System
4. Results and Discussion
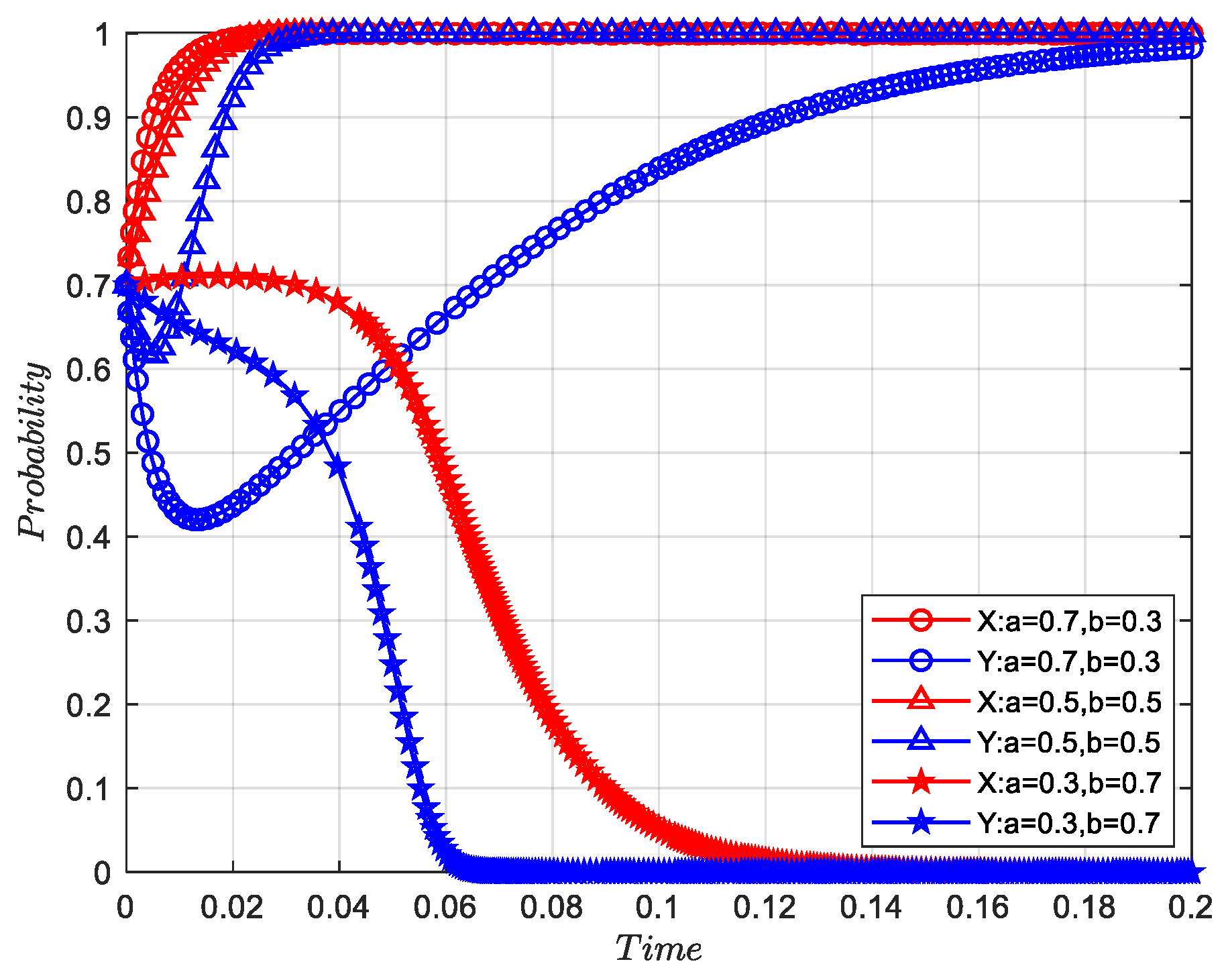
4.1. Impact of the Initial Probability on Replicated Dynamical Systems
4.2. Impacts of Different Ratios of Clean and Renewable Energy Use of Traditional Energy on Replicating Dynamic Systems
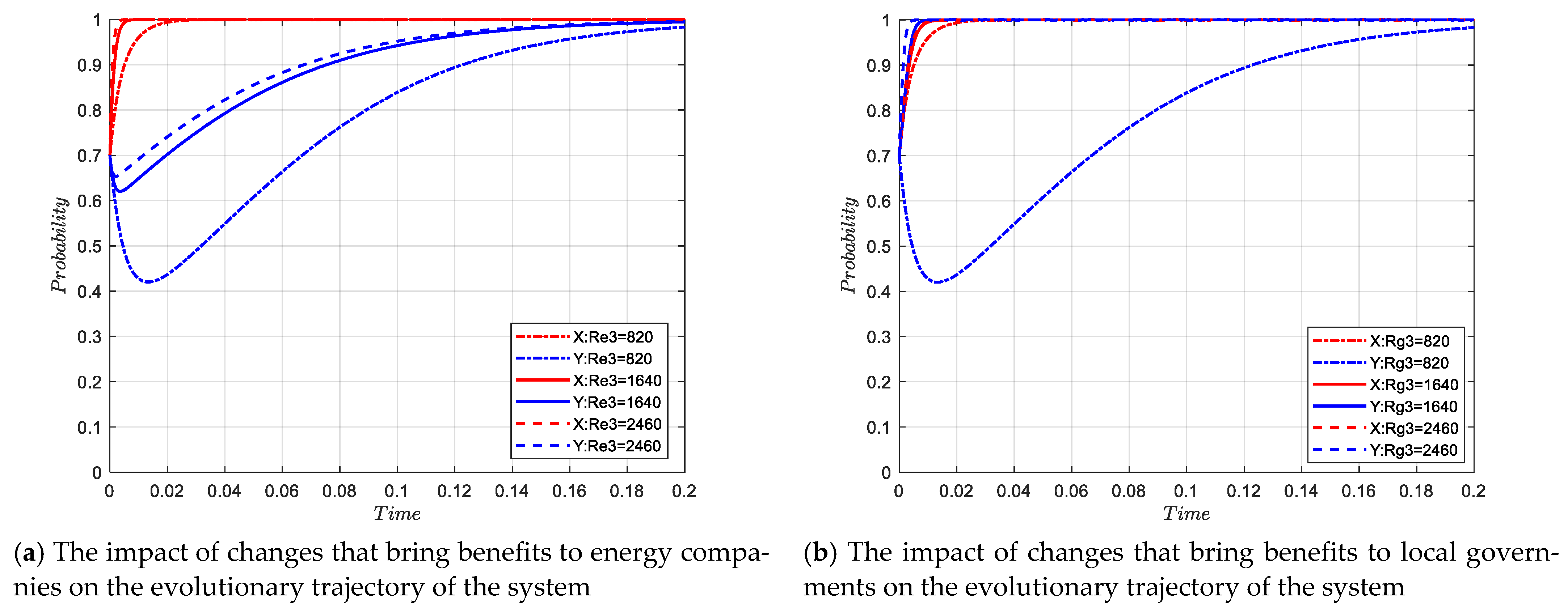
4.3. Impact of Changes in Energy System Resilience Gains Re3 and Rg3 on Replicated Dynamic Systems
4.4. Impact of Changes in Local Government Subsidy Parameters Mt and Mr on Replicated Dynamic Systems
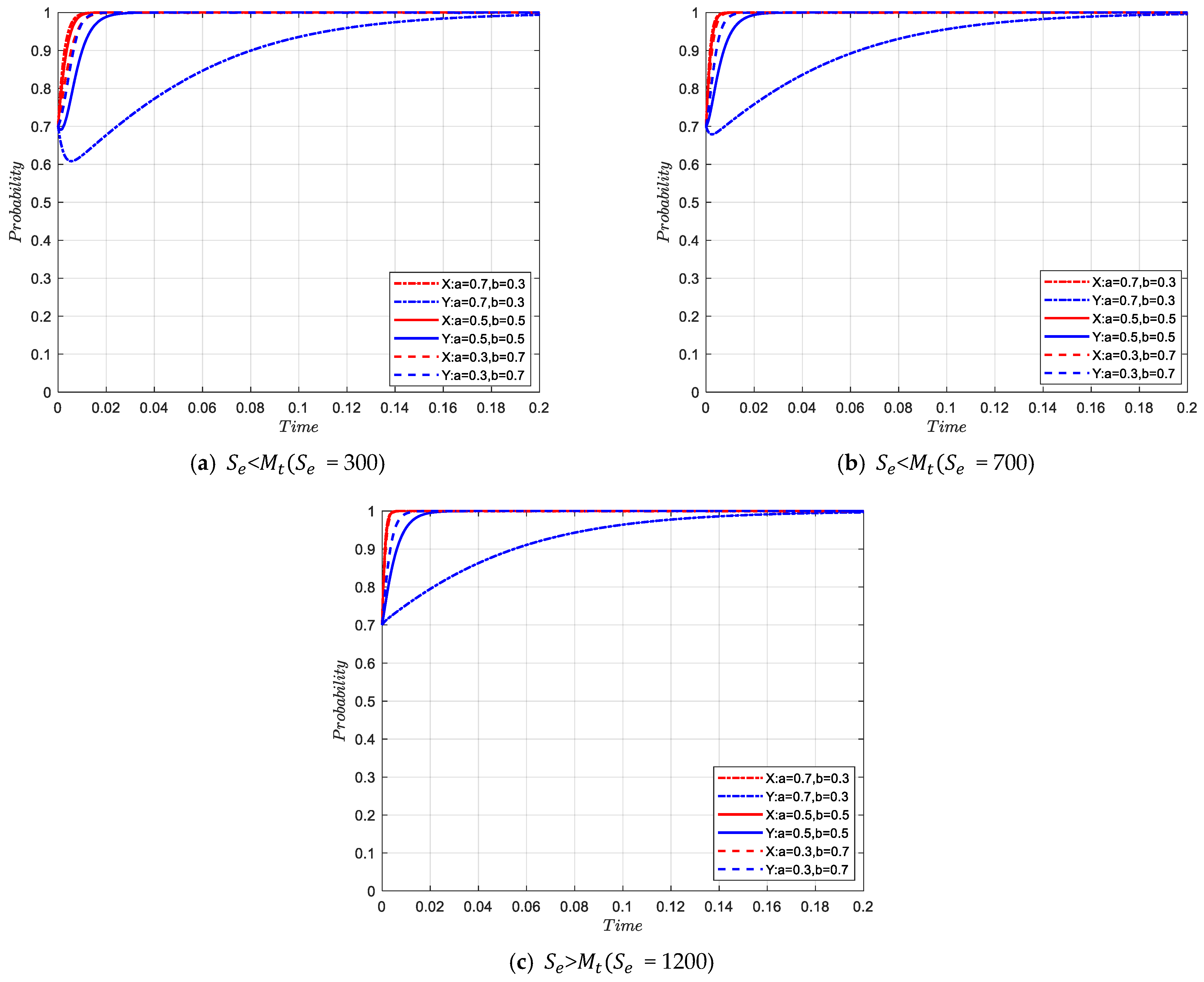
4.5. Impact of The Introduction of Penalty Parameters on Replicated Dynamic Systems
4.6. Summary
5. Conclusions and Policy Implications
5.1. Conclusions and Recommendations
- (1)
- The high ratio of clean utilization of traditional energy and the low ratio of renewable, clean energy can promote the transformation of the energy structure in the Yellow River Basin. Therefore, based on the resource endowment of the Yellow River Basin and the utilization of renewable and clean energy, while ensuring a reasonable utilization ratio for the two energy sources, the pace of clean utilization of traditional energy sources in the Yellow River Basin should be accelerated. Moreover, while increasing the ability to utilize renewable clean energy technologies, clean technologies, such as CCUS [47,48], should be vigorously developed, and renewable clean energy sources, such as solar, wind, and hydro energy, should be cultivated actively;
- (2)
- The strategic choices of energy companies are influenced by the capacity for both types of energy utilization, while the strategic choices of local governments are primarily influenced by the capacity for renewable, clean energy utilization. Therefore, for energy companies, it is a top priority to improve the technology level for the clean use of traditional energy and carry out special technical research on the use of renewable energy to improve the ability to use both types of energy. For local governments, improving the sensitivity of energy system resilience according to energy companies’ revenue generation and increasing subsidies for renewable, clean energy utilization play a crucial role in the energy structure transformation by energy companies;
- (3)
- The introduction of penalty parameters not only ensures a reasonable range of subsidies for the clean utilization of traditional energy, but also provides a guarantee for the maturation of renewable, clean energy utilization technologies. Therefore, a better combination of subsidy and penalty parameters can motivate local governments and energy companies to move toward integrated energy use. When energy companies are driven by innovation to improve the clean utilization of traditional energy sources and master the core technology of renewable, clean energy utilization, this move is of great value to the transformation of the energy structure in the Yellow River Basin;
- (4)
- In the short term, the clean use of traditional energy is the most economically feasible option, and the impact of one strategy on the other between energy companies and local governments is not significant at this stage. In the medium and long term, renewable, clean energy in the Yellow River Basin can enter a whole new stage of development, and the impact of the interaction between the two strategies is very significant at this stage. Therefore, combined with China’s energy transition trend, in the short term, to balance economic and environmental sustainability, it is necessary to improve the level of technology for the clean and efficient utilization of traditional energy, i.e., the energy transition should take moderate means. In the medium and long term, scientific development planning for renewable, clean energy and the active promotion of a high percentage of renewable, clean energy utilization should be implemented to meet the growing energy demand.
5.2. Limitations and Future Research
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xu, M.; Zhang, X.; Shen, S.; Wei, S.; Fan, J.L. Assessment of potential, cost, and environmental benefits of CCS-EWR technology for coal-fired power plants in Yellow River Basin of China. J. Environ. Manag. 2021, 292, 112717. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Ming, B.; Huang, Q.; Wang, Y.; Zheng, X.; Zhang, W. Risk-averse day-ahead generation scheduling of hydro–wind–photovoltaic complementary systems considering the steady requirement of power delivery. Appl. Energy 2022, 309, 118467. [Google Scholar] [CrossRef]
- Zhang, F.; Gallagher, K.S.; Myslikova, Z.; Narassimhan, E.; Bhandary, R.R.; Huang, P. From fossil to low carbon: The evolution of global public energy innovation. WIREs Clim. Change 2021, 12, e734. [Google Scholar] [CrossRef]
- Lin, B.; Li, Z. Towards world’s low carbon development: The role of clean energy. Appl. Energy 2022, 307, 118160. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, B.; Wang, B.; Wang, Z. Evolutionary trend of the coal industry chain in China: Evidence from the analysis of I-O and APL model. Resour. Conserv. Recycl. 2019, 145, 399–410. [Google Scholar] [CrossRef]
- Tian, J.; Yu, L.; Xue, R.; Zhuang, S.; Shan, Y. Global low-carbon energy transition in the post-COVID-19 era. Appl. Energy 2022, 307, 118205. [Google Scholar] [CrossRef]
- Chen, W.; Hu, Z.-H. Using evolutionary game theory to study governments and manufacturers’ behavioral strategies under various carbon taxes and subsidies. J. Clean. Prod. 2018, 201, 123–141. [Google Scholar] [CrossRef]
- Fan, R.; Dong, L. The dynamic analysis and simulation of government subsidy strategies in low-carbon diffusion considering the behavior of heterogeneous agents. Energy Policy 2018, 117, 252–262. [Google Scholar] [CrossRef]
- Zheng, S.; Yu, L. The government’s subsidy strategy of carbon-sink fishery based on evolutionary game. Energy 2022, 254, 124282. [Google Scholar] [CrossRef]
- Guo, P.; Kong, J.; Guo, Y.; Liu, X. Identifying the influencing factors of the sustainable energy transitions in China. J. Clean. Prod. 2019, 215, 757–766. [Google Scholar] [CrossRef]
- Maswabi, M.G.; Chun, J.; Chung, S.-Y. Barriers to energy transition: A case of Botswana. Energy Policy 2021, 158, 112514. [Google Scholar] [CrossRef]
- Zhang, S.; Shinwari, R.; Zhao, S.; Dagestani, A.A. Energy transition, geopolitical risk, and natural resources extraction: A novel perspective of energy transition and resources extraction. Resour. Policy 2023, 83, 103608. [Google Scholar] [CrossRef]
- Khan, I.; Hou, F.; Zakari, A.; Tawiah, V.K. The dynamic links among energy transitions, energy consumption, and sustainable economic growth: A novel framework for IEA countries. Energy 2021, 222, 119935. [Google Scholar] [CrossRef]
- Liu, H.; Khan, I.; Zakari, A.; Alharthi, M. Roles of trilemma in the world energy sector and transition towards sustainable energy: A study of economic growth and the environment. Energy Policy 2022, 170, 113238. [Google Scholar] [CrossRef]
- Kern, F.; Smith, A. Restructuring energy systems for sustainability? Energy transition policy in the Netherlands. Energy Policy 2008, 36, 4093–4103. [Google Scholar] [CrossRef]
- Haas, T. Comparing energy transitions in Germany and Spain using a political economy perspective. Environ. Innov. Soc. Transit. 2019, 31, 200–210. [Google Scholar] [CrossRef]
- Gulagi, A.; Ram, M.; Solomon, A.A.; Khan, M.; Breyer, C. Current energy policies and possible transition scenarios adopting renewable energy: A case study for Bangladesh. Renew. Energy 2020, 155, 899–920. [Google Scholar] [CrossRef]
- Adewuyi, O.B.; Kiptoo, M.K.; Afolayan, A.F.; Amara, T.; Alawode, O.I.; Senjyu, T. Challenges and prospects of Nigeria’s sustainable energy transition with lessons from other countries’ experiences. Energy Rep. 2020, 6, 993–1009. [Google Scholar] [CrossRef]
- Chen, B.; Xiong, R.; Li, H.; Sun, Q.; Yang, J. Pathways for sustainable energy transition. J. Clean. Prod. 2019, 228, 1564–1571. [Google Scholar] [CrossRef]
- Li, T.; Liu, P.; Li, Z. Quantitative relationship between low-carbon pathways and system transition costs based on a multi-period and multi-regional energy infrastructure planning approach: A case study of China. Renew. Sustain. Energy Rev. 2020, 134, 110159. [Google Scholar] [CrossRef]
- Gardumi, F.; Keppo, I.; Howells, M.; Pye, S.; Avgerinopoulos, G.; Lekavičius, V.; Galinis, A.; Martišauskas, L.; Fahl, U.; Korkmaz, P.; et al. Carrying out a multi-model integrated assessment of European energy transition pathways: Challenges and benefits. Energy 2022, 258, 124329. [Google Scholar] [CrossRef]
- Su, X.; Tan, J. Regional energy transition path and the role of government support and resource endowment in China. Renew. Sustain. Energy Rev. 2023, 174, 113150. [Google Scholar] [CrossRef]
- Kong, L.-S.; Tan, X.-C.; Gu, B.-H.; Yan, H.-S. Significance of achieving carbon neutrality by 2060 on China’s energy transition pathway: A multi-model comparison analysis. Adv. Clim. Change Res. 2023, 14, 32–42. [Google Scholar] [CrossRef]
- Tian, T.; Sun, S. Low-carbon transition pathways in the context of carbon-neutral: A quadrilateral evolutionary game analysis. J. Environ. Manag. 2022, 322, 116105. [Google Scholar] [CrossRef]
- Li, J.; Li, M. Research of Carbon Emission Reduction Potentials in the Yellow River Basin, Based on Cluster Analysis and the Logarithmic Mean Divisia Index (LMDI) Method. Sustainability 2022, 14, 5284. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, H.; Ahmad, M.; Xue, C. Analysis of influencing factors of carbon emissions in resource-based cities in the Yellow River basin under carbon neutrality target. Environ. Sci. Pollut. Res. Int. 2022, 29, 23847–23860. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, S.; Wang, J. Impact of environmental regulation intensity on green innovation efficiency in the Yellow River Basin, China. J. Clean. Prod. 2022, 373, 133789. [Google Scholar] [CrossRef]
- Zhao, K.; Zhang, R.; Liu, H.; Wang, G.; Sun, X. Resource Endowment, Industrial Structure, and Green Development of the Yellow River Basin. Sustainability 2021, 13, 4530. [Google Scholar] [CrossRef]
- Mao, J.; Wu, Q.; Zhu, M.; Lu, C. Effects of Environmental Regulation on Green Total Factor Productivity: An Evidence from the Yellow River Basin, China. Sustainability 2022, 14, 2015. [Google Scholar] [CrossRef]
- Zhou, Y.; Li, D.; Li, W.; Mei, D.; Zhong, J. Drag Effect of Economic Growth and Its Spatial Differences under the Constraints of Resources and Environment: Empirical Findings from China’s Yellow River Basin. Int. J. Environ. Res. Public Health 2022, 19, 3027. [Google Scholar] [CrossRef]
- Wang, H.; Tao, J.; Xu, J.; Li, Z. Positive or negative coordination? Spatiotemporal coupling analysis between economic growth and carbon neutrality in the Yellow River Basin. Energy Rep. 2023, 9, 140–153. [Google Scholar] [CrossRef]
- Song, M.; Zhang, L.; Gao, Y.; Li, E. Spatiotemporal evolution and influence mechanism of the carbon footprint of energy consumption at county level in the Yellow River Basin. Sci. Total Environ. 2023, 883, 163710. [Google Scholar] [CrossRef] [PubMed]
- Xia, Y.; Shi, J.; Zhao, Z.; Liu, Z.; Liu, X.; Zhang, C. Mapping the coal-water nexus in the Yellow River Basin. J. Clean. Prod. 2023, 411, 137249. [Google Scholar] [CrossRef]
- Wang, Y.; Song, J.; Sun, H. Coupling interactions and spatial equilibrium analysis of water-energy-food in the Yellow River Basin, China. Sustain. Cities Soc. 2023, 88, 104293. [Google Scholar] [CrossRef]
- Wang, S.; Yang, J.; Wang, A.; Liu, T.; Du, S.; Liang, S. Coordinated analysis and evaluation of water–energy–food coupling: A case study of the Yellow River basin in Shandong Province, China. Ecol. Indic. 2023, 148, 110138. [Google Scholar] [CrossRef]
- Luo, L.; Wang, Y.; Liu, Y.; Zhang, X.; Fang, X. Where is the pathway to sustainable urban development? Coupling coordination evaluation and configuration analysis between low-carbon development and eco-environment: A case study of the Yellow River Basin, China. Ecol. Indic. 2022, 144, 109473. [Google Scholar] [CrossRef]
- de Amorim, W.S.; Valduga, I.B.; Ribeiro, J.M.P.; Williamson, V.G.; Krauser, G.E.; Magtoto, M.K.; de Andrade Guerra, J.B.S.O. The nexus between water, energy, and food in the context of the global risks: An analysis of the interactions between food, water, and energy security. Environ. Impact Assess. Rev. 2018, 72, 1–11. [Google Scholar] [CrossRef]
- Zhang, T.Y.; Dong, L.Y.; Feng, X.M.; Tan, Q. Optimization model for enhancing water-use efficiency from the perspective of water-energy-food nexus. IOP Conf. Ser. Earth Environ. Sci. 2019, 344, 012130. [Google Scholar] [CrossRef]
- Maynard Smith, J. The theory of games and the evolution of animal conflicts. J. Theor. Biol. 1974, 47, 209–221. [Google Scholar] [CrossRef] [Green Version]
- Selten, R. Evolutionary stability in extensive two-person games—Correction and further development. Math. Soc. Sci. 1988, 16, 223–266. [Google Scholar] [CrossRef]
- Friedman, D. On economic applications of evolutionary game theory. J. Evol. Econ. 1998, 8, 15–43. [Google Scholar] [CrossRef] [Green Version]
- Wu, B.; Liu, P.; Xu, X. An evolutionary analysis of low-carbon strategies based on the government–enterprise game in the complex network context. J. Clean. Prod. 2017, 141, 168–179. [Google Scholar] [CrossRef]
- Jiao, J.; Chen, J.; Li, l.; LI, f. A Study of Local Governments’ and Enterprises’ Actions in the Carbon Emission Mechanism of Subsidy or Punishment Based on the Evolutionary Game. Chin. J. Manag. Sci. 2017, 25, 140–150. (In Chinese) [Google Scholar]
- Ahmadi, S.; Saboohi, Y.; Vakili, A. Frameworks, quantitative indicators, characters, and modeling approaches to analysis of energy system resilience: A review. Renew. Sustain. Energy Rev. 2021, 144, 110988. [Google Scholar] [CrossRef]
- Chai, R.; Li, G. Renewable clean energy and clean utilization of traditional energy:An evolutionary game model of energy structure transformation of power enterprises. Syst. Eng. Theory Pract. 2022, 42, 184–197. (In Chinese) [Google Scholar]
- Wang, X.-P.; Zhang, Z.-M.; Guo, Z.-H.; Su, C.; Sun, L.-H. Energy structure transformation in the context of carbon neutralization: Evolutionary game analysis based on inclusive development of coal and clean energy. J. Clean. Prod. 2023, 398, 136626. [Google Scholar] [CrossRef]
- Jiang, K.; Ashworth, P. The development of Carbon Capture Utilization and Storage (CCUS) research in China: A bibliometric perspective. Renew. Sustain. Energy Rev. 2021, 138, 110521. [Google Scholar] [CrossRef]
- Yan, Y.; Borhani, T.N.; Subraveti, S.G.; Pai, K.N.; Prasad, V.; Rajendran, A.; Nkulikiyinka, P.; Asibor, J.O.; Zhang, Z.; Shao, D.; et al. Harnessing the power of machine learning for carbon capture, utilisation, and storage (CCUS)—A state-of-the-art review. Energy Environ. Sci. 2021, 14, 6122–6157. [Google Scholar] [CrossRef]




| Symbol | Description | Symbol | Description |
|---|---|---|---|
| The cost of using traditional energy, such as coal | The cost of clean utilization of traditional energy | ||
| The cost of utilization of renewable, clean energy | Base revenue earned by energy companies using traditional energy, such as coal | ||
| Benefits of clean use of traditional energy by energy companies | Benefits for energy companies using renewable, clean energy | ||
| Benefits to energy companies from the resulting increase in energy system resilience, with local government subsidies for integrated energy use | Benefits to local governments from the resulting increase in energy system resilience, with local government subsidies for integrated energy use | ||
| Environmental benefits to government from energy companies’ clean use of traditional energy | Environmental benefits to government from energy companies’ use of renewable, clean energy | ||
| Environmental losses caused by energy companies to local governments when using traditional energy | Penalties for integrated energy use by energy companies that do not use the subsidies while ensuring the energy supply | ||
| Government subsidies for renewable, clean energy use by energy companies | Government subsidies for clean use of traditional energy by energy companies | ||
| The proportion of traditional clean energy use (renewable, clean energy use) within the integrated energy use | Probability of strategy choice by energy companies and local governments in the Yellow River Basin |
| Local Governments | |||||
|---|---|---|---|---|---|
| Provide Subsidies (y) | No Subsidies (1 − y) | ||||
| Companies Payoff | Governments Payoff | Companies Payoff | Governments Payoff | ||
| Energy companies | Use of integrated energy | ||||
| Use of traditional energy | |||||
| Equilibrium Points | λ1 | λ2 |
|---|---|---|
| Saddle point | ||
| Stage I (I′) | Stage II (II′) | |||||
|---|---|---|---|---|---|---|
| Equilibrium points | State | State | ||||
| (0,0) | −(+) | − | ESS (Instability point) | −(+) | − | ESS (Instability point) |
| (0,1) | +(−) | − | Instability point (ESS) | +(−) | + | Saddle point (Instability point) |
| (1,0) | + | + | Saddle point | + | + | Saddle point |
| (1,1) | − | + | Instability point | − | − | ESS |
| (x*,y*) | Saddle point | Saddle point | ||||
| Stage III (III′) | Stage IV (IV′) | |||||
| Equilibrium points | State | State | ||||
| (0,0) | −(+) | + | Instability point (Saddle point) | −(+) | + | Instability point (Saddle point) |
| (0,1) | +(−) | − | Instability point (ESS) | +(−) | + | Saddle point (Instability point) |
| (1,0) | + | − | Instability point | + | − | Instability point |
| (1,1) | − | + | Instability point | − | − | ESS |
| (x*,y*) | Saddle point | Saddle point | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, X.; Shi, Y.; Li, Y. Research on the Yellow River Basin Energy Structure Transformation Path under the “Double Carbon” Goal. Sustainability 2023, 15, 9695. https://doi.org/10.3390/su15129695
Liang X, Shi Y, Li Y. Research on the Yellow River Basin Energy Structure Transformation Path under the “Double Carbon” Goal. Sustainability. 2023; 15(12):9695. https://doi.org/10.3390/su15129695
Chicago/Turabian StyleLiang, Xiaoxia, Yi Shi, and Yan Li. 2023. "Research on the Yellow River Basin Energy Structure Transformation Path under the “Double Carbon” Goal" Sustainability 15, no. 12: 9695. https://doi.org/10.3390/su15129695
APA StyleLiang, X., Shi, Y., & Li, Y. (2023). Research on the Yellow River Basin Energy Structure Transformation Path under the “Double Carbon” Goal. Sustainability, 15(12), 9695. https://doi.org/10.3390/su15129695







