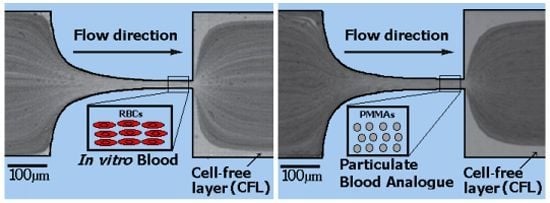
Particulate Blood Analogues Reproducing the Erythrocytes Cell-Free Layer in a Microfluidic Device Containing a Hyperbolic Contraction
Abstract
:1. Introduction
2. Experimental Section
2.1. Working Fluids and Microchannel Geometry
- Dextran 40 (Dx40, = 1.05 g/cm), containing 5% by volume of ovine RBCs, i.e., hematocrit of 5% (v/v);
- A two-phase viscoelastic solution made of xanthan gum (115 ppm) diluted in the solvent dextran 40, carrying 5% by weight (4.4% v/v) of PMMA spherical particles of 6 µm diameter ( = 1.20 g/cm) and 0.05% by weight of sodium dodecyl sulfate (SDS);
- Dextran 40 carrying 5% by weight (4.4% v/v) of PMMA spherical particles of 6 µm diameter and 0.05% by weight of sodium dodecyl sulfate (SDS);
- Additionally, a solution of only Dx40 and another solution of xanthan gum (115 ppm) diluted in Dx40 were also analyzed.
| Acronym | PMMA | XG | Dx40 | RBC’s |
|---|---|---|---|---|
| X1 | - | 115 ppm | ✓ | - |
| X2 | 5% (w/w) | 115 ppm | ✓ | - |
| D1 | - | - | ✓ | - |
| D2 | 5% (w/w) | - | ✓ | - |
| B1 | - | - | ✓ | 5% (w/w) |


2.2. Fluid Rheology
2.3. Image Analysis

3. Results and Discussion
3.1. Results


3.2. Discussion

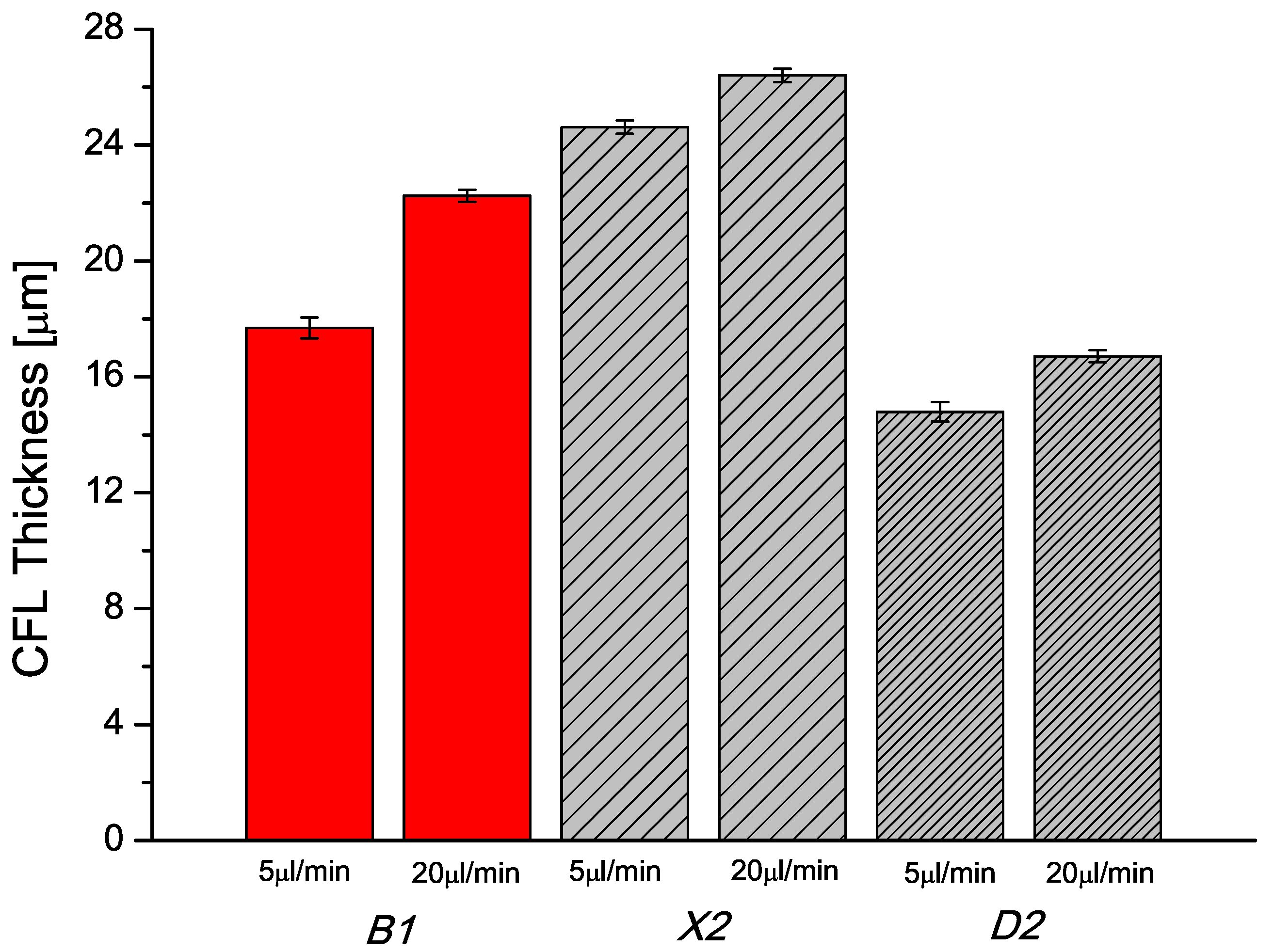
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Pries, A.R.; Secomb, T.W.; Gaehtgens, P. Biophysical aspects of blood flow in the microvasculature. Cardiovasc. Res. 1996, 32, 654–667. [Google Scholar] [CrossRef]
- Pincombe, B.; Mazumdar, J.; Hamilton-Craig, I. Effects of multiple stenos and post-stenotic dilatation on non-Newonian blood flow in small arteries. Med. Biol. Eng. Comput. 1999, 37, 595–599. [Google Scholar] [CrossRef] [PubMed]
- Wong, K.; Mazumdar, J.; Pincombe, B.; Worthley, S.; Prashanthan, S.; Abbott, D. Theoretical modeling of micro-scale biological phenomena in human coronary arteries. Med. Biol. Eng. Comput. 2006, 44, 971–982. [Google Scholar] [CrossRef] [PubMed]
- Marcinkowska-Gapinska, A.; Gapinski, J.; Elikowski, W.; Jaroszyk, F.; Kubisz, L. Comparison of three rheological models of shear flow behavior studied on blood samples from post-infarction patients. Med. Biol. Eng. Comput. 2007, 45, 837–844. [Google Scholar] [CrossRef] [PubMed]
- Dintenfass, L. Blood Rheology in Cardio-vascular Diseases. Nature 1963, 199, 813–815. [Google Scholar] [CrossRef] [PubMed]
- Lima, R.; Ishikawa, T.; Imai, Y.; Yamaguchi, T. Blood Flow Behavior in Microchannels: Past, Current and Future Trends. In Single and Two-Phase Flows on Chemical and Biomedical Engineering; Dias, R., Martins, A.A., Lima, R., Mata, T.M., Eds.; Bentham Science Publishers: Sharjah, UAE, 2012; pp. 513–547. [Google Scholar]
- Garcia, V.; Dias, R.; Lima, R. In Vitro Blood Flow Behaviour in Microchannels with Simple and Complex Geometries. In Applied Biological Engineering - Principles and Practice; Naik, G.R., Ed.; InTech: Rijeka, Croatia, 2012; pp. 393–416. [Google Scholar]
- Campo-Deaño, L.; Dullens, R.P.A.; Aarts, D.G.A.L.; Pinho, F.T.; Oliveira, M.S.N. Viscoelasticity of blood and viscoelastic blood analogues for use in polydymethylsiloxane in vitro models of the circulatory system. Biomicrofluidics 2013, 7, 034102. [Google Scholar] [CrossRef] [PubMed]
- Cebral, J.R.; Castro, M.A.; Burgess, J.E.; Pergolizzi, R.S.; Sheridan, M.J.; Putman, C.M. Characterization of cerebral aneurysms for assessing risk of rupture by using patient-specific computational hemodynamics models. Am. J. Neuroradiol. 2005, 26, 2550–2559. [Google Scholar] [PubMed]
- Pries, A.R.; Secomb, T.W.; Gaehtgens, P.; Gross, J.F. Blood flow in microvascular networks: Experiments and simulation. Circ. Res. 1990, 67, 826–834. [Google Scholar] [CrossRef] [PubMed]
- Toksvang, L.N.; Berg, R.M.G. Using a classic paper by Robin Fåhraeus and Torsten Lindqvist to teach basic hemorheology. Adv. Physiol. Educ. 2013, 37, 129–133. [Google Scholar] [CrossRef] [PubMed]
- Anastasiou, A.D.; Spyrogianni, A.S.; Koskinas, K.C.; Giannoglou, G.D.; Paras, S.V. Experimental investigation of the flow of a blood analogue fluid in a replica of a bifurcated small artery. Med. Eng. Phys. 2012, 34, 211–218. [Google Scholar] [CrossRef] [PubMed]
- Bui, A.; Sutalo, I.; Manasseh, R.; Liffman, K. Dynamics of pulsatile flow in fractal models of vascular branching networks. Med. Biol. Eng. Comput. 2009, 47, 763–772. [Google Scholar] [CrossRef] [PubMed]
- Fåhraeus, R.; Lindqvist, T. The viscosity of the blood in narrow capillary tubes. Am. J. Physiol. 1931, 96, 562–568. [Google Scholar]
- Goldsmith, H.L.; Turitto, V.T. Rheological aspects of thrombosis and haemostasis: Basic principles and applications. Thromb. Haemost. 1986, 55, 415–435. [Google Scholar] [PubMed]
- Chien, S.; Usami, S.; Skalak, R. Handbook of Physiology-The Cardiovascular System IV; Renkin, E.M., Michel, C.C., Eds.; American Physiological Society: Bethesda, MD, USA, 1984. [Google Scholar]
- Lima, R.; Wada, S.; Tsubota, K.; Yamaguchi, T. Confocal micro-PIV measurements of three-dimensional profiles of cell suspension flow in a square microchannel. Meas. Sci. Technol. 2006, 17, 797–808. [Google Scholar] [CrossRef]
- Lima, R.; Ishikawa, T.; Imai, Y.; Takeda, M.; Wada, S.; Yamaguchi, T. Measurement of individual red blood cell motions under high hematocrit conditions using a confocal micro-PTV system. Ann. Biomed. Eng. 2009, 37, 1546–1559. [Google Scholar] [CrossRef] [PubMed]
- Abkarian, M.; Faivre, M.; Horton, R.; Smistrup, K.; Best-Popescu, C.A.; Stone, H.A. Cellular-scale hydrodynamics. Biomed. Matter. 2008, 3, 034011. [Google Scholar] [CrossRef] [PubMed]
- Maeda, N.; Suzuki, Y.; Tanaka, J.; Tateishi, N. Erythrocyte flow and elasticity of microvessels evaluated by marginal cell-free layer and flow resistance. Am. J. Physiol. Heart Circ. Physiol. 1996, 271, 2454–2461. [Google Scholar]
- Vennemann, P.; Kiger, K.T.; Lindken, R.; Groenendijk, B.C.; Stekelenburg-de Vos, S.; ten Hagen, T.L.; Ursem, N.; Poelmann, R.E.; Westerweel, J.; Hierck, B.P. In vivo micro particle image velocimetry measurements of blood-plasma in the embryonic avian heart. J. Biomech. 2006, 39, 1191–1200. [Google Scholar] [CrossRef] [PubMed]
- Cho, S.; Ye, S.S.; Leo, H.L.; Kim, S. Computational Simulation of NO/O2 Transport in Arterioles: Role of Cell-Free Layer. In Visualization and Simulation of Complex Flows in Biomedical Engineering; Lecture Notes in Computational Vision and Biomechanics Volume 12; Lima, R., Imai, Y., Ishikawa, T., Oliveira, M.S.N., Eds.; Springer: Berlin, Germany, 2014; pp. 89–100. [Google Scholar]
- Ishikawa, T.; Fujiwara, H.; Matsuki, N.; Yoshimoto, T.; Imai, Y.; Ueno, H.; Yamaguchi, T. Asymmetry of blood flow and cancer cell adhesion in a microchannel with symmetric bifurcation and confluence. Biomed. Microdevices 2011, 13, 159–167. [Google Scholar] [CrossRef] [PubMed]
- Leble, V.; Lima, R.; Dias, R.; Fernandes, C.; Ishikawa, T.; Imai, Y.; Yamaguchi, T. Asymmetry of red blood cell motions in a microchannel with diverging and converging bifurcation. Biomicrofluidics 2011, 5, 044120. [Google Scholar] [CrossRef] [PubMed]
- Faivre, M.; Abkarian, M.; Bickraj, K.; Stone, H.A. High-speed microfluidic differential manometer for cellular-scale hydrodynamics. Biorheology 2006, 43, 147–159. [Google Scholar] [PubMed]
- Zhao, R.; Antaki, J.F.; Naik, T.; Bachman, T.N.; Kameneva, M.V.; Wu, Z.J. Microscopic investigation of erythrocyte deformation dynamics. Biorheology 2006, 43, 747–765. [Google Scholar] [PubMed]
- Lee, S.S.; Yim, Y.; Ahn, K.H.; Lee, S.J. Extensional flow-based assessment of red blood cell deformability using hyperbolic converging microchannel. Biomed. Microdevices 2009, 11, 1021–1027. [Google Scholar] [CrossRef] [PubMed]
- Yaginuma, T.; Oliveira, M.S.N.; Lima, R.; Ishikawa, T.; Yamaguchi, T. Human red blood cell behaviour under homogeneous extensional flow in a hyperbolic-shaped microchannel. Biomicrofluidics 2013, 7, 054110. [Google Scholar] [CrossRef] [PubMed]
- Sollier, E.; Cubizolles, M.; Fouillet, Y.; Achard, J.L. Fast and continuous plasma extraction from whole human blood based on expanding cell-free layer devices. Biomed. Microdevices 2010, 12, 485–497. [Google Scholar] [CrossRef] [PubMed]
- Pinho, D.; Rodrigues, R.O.; Yaginuma, T.; Faustino, V.; Bento, D.; Fernandes, C.S.; Garcia, V.; Pereira, A.I.; Lima, R. Motion of rigid particles flowing in a microfluidic device with a pronounced stenosis: trajectories and deformation index. In Proceedings of the 11th World Congress on Computational Mechanics (WCCM XI), Barcelona, Spain, 20–25 July 2014; pp. 6234–6240.
- Rodrigues, R.O.; Pinho, D.; Faustino, V.; Lima, R. A simple microfluidic device for the deformability assessment of blood cells in a continuous flow. Biomed. Microdevices 2015, 17, 108. [Google Scholar] [CrossRef] [PubMed]
- Pinho, D.; Rodrigues, R.O.; Faustino, V.; Yaginuma, T.; Exposto, J.; Lima, R. Red blood cells radial dispersion in blood flowing through microchannels: The role of temperature. J. Biomech. 2015. [Google Scholar] [CrossRef] [PubMed]
- Pinto, E.; Faustino, V.; Rodrigues, R.O.; Pinho, D.; Garcia, V.; Miranda, J.M.; Lima, R. A rapid and low-cost nonlithographic method to fabricate biomedical microdevices for blood flow analysis. Micromachines 2015, 6, 121–135. [Google Scholar] [CrossRef]
- Rodrigues, R.; Faustino, V.; Pinto, E.; Pinho, D.; Lima, R. Red Blood Cells deformability index assessment in a hyperbolic microchannel: The diamide and glutaraldehyde effect. WebmedCentral Biomed. Eng. 2013, 4, WMC004375. [Google Scholar]
- Nguyen, T.T.; Biadillah, Y.; Mongrain, R.; Brunette, J.; Tardif, J.C.; Bertrand, O.F. A method for matching the refractive index and kinematic viscosity of a blood analog for flow visualization in hydraulic cardiovascular models. J. Biomech. Eng. 2004, 126, 529–535. [Google Scholar] [CrossRef] [PubMed]
- van den Broek, C.N.; Pullens, R.A.; Frobert, O.; Rutten, M.C.; Hartog, W.F.; van de Vosse, F.N. Medium with blood-analog mechanical properties for cardiovascular tissue culturing. Biorheology 2008, 45, 651–661. [Google Scholar] [PubMed]
- Wickramasinghe, S.R.; Kahr, C.M.; Han, B. Mass transfer in blood oxygenators using blood analogue fluids. Biotechnol. Prog. 2002, 18, 867–873. [Google Scholar] [CrossRef] [PubMed]
- Sousa, P.C.; Pinho, F.T.; Oliveira, M.S.N.; Alves, M.A. Extensional flow of blood analogue solutions in microfluidic devices. Biomicrofluidics 2011, 5, 014108. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Maruyama, O.; Yamane, T.; Tsunemoto, N.; Nishida, M.; Tsutsui, T.; Jikuya, T. A preliminary study of micropasule suspension for hemolysis evaluation of artificial organs. Artif. Organs 1999, 23, 274–279. [Google Scholar] [CrossRef] [PubMed]
- Maruyama, O.; Yamane, T.; Nishida, M.; Aouidef, A.; Tsutsui, T.; Jikuya, T.; Masuzawa, T. Fractural characteristic evaluation of a microcapsule suspension using a rotational shear stressor. ASAIO J. 2002, 48, 365–373. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, T.T.; Mongrain, R.; Prakash, S.; Tardif, J.C. Development of a Blood Analog for the Hernodynamic Efficiency Evaluation of Cardiovascular Devices. In Proceedings of the Canadian Design Engineering Network Conference, Montreal, QC, Canada, 29–30 July 2004.
- Gregory, T.R. Nucleotypic effects without nuclei: Genome size and erythrocyte size in mammals. NRC Res. Press 2000, 43, 895–901. [Google Scholar] [CrossRef]
- Campo-Deaño, L.; Galindo-Rosales, F.J.; Pinho, F.T.; Alves, M.A.; Oliveira, M.S.N. Flow of low viscosity Boger fluids through a microfluidic hyperbolic contraction. J. Non-Newt. Fluid Mech. 2011, 166, 1286–1296. [Google Scholar] [CrossRef] [Green Version]
- Waite, L.; Fine, J. Review of Basic Fluid Mechanics Concepts. In Applied Biofluid Mechanics; The McGraw-Hill Companies: New York, NY, USA, 2007; pp. 1–33. [Google Scholar]
- Pinho, D.; Gayubo, F.; Pereira, A.; Lima, R. A comparison between a manual and automatic method to characterize red blood cell trajectories. Int. J. Numer. Methods Biomed. Eng. 2013, 29, 977–987. [Google Scholar] [CrossRef] [PubMed]
- Einstein, A. Eine neue bestimmung der molekuldimensionen. Ann. Phys. 1906, 19, 289–306. [Google Scholar] [CrossRef]
- Guth, E.; Simha, R. Untersuchungen uber die Viskositat von Suspensionen und Losungen. 3. Uber die Viskositat von Kugelsuspensionen. Kolloid Z. 1936, 74, 266. (In German) [Google Scholar] [CrossRef]
- Thurston, G.B. Rheological parameters for the viscosity, viscoelasticity and thixotropy of blood. Biorheology 1979, 16, 149–162. [Google Scholar] [PubMed]
- Yeleswarapu, K.K.; Kameneva, M.V.; Rajagopal, K.R.; Antaki, J.F. The flow of blood in tubes: Theory and experiment. Mech. Res. Commun. 1998, 25, 257–262. [Google Scholar] [CrossRef]
- Segre, G.; Silberberg, A. Radial particle displacements in Poiseuille flow of suspensions. Nature 1961, 189, 136–157. [Google Scholar] [CrossRef]
- Di Carlo, D. Continuous inertial focusing, ordering, and separation of particles in microchannels. Proc. Natl. Acad. Sci. USA 2007, 104, 18892–18897. [Google Scholar] [CrossRef] [PubMed]
- Karimi, A.; Yazdi, S.; Ardekani, A.M. Hydrodynamic mechanisms of cell and particle trapping in microfluidics. Biomicrofluidics 2013, 7, 021501. [Google Scholar] [CrossRef] [PubMed]
- Chhabra, R.P. Bubbles, Drops, and Particles in Non-Newtonian Fluids, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Tehrani, M.A. An experimental study of particle migration in pipe flow of viscoelastic fluids. Rheology 1996, 40, 1057–1077. [Google Scholar] [CrossRef]
- Loon, S.V.; Fransaer, J.; Clasen, C.; Vermant, J. String formation in sheared suspensions in rheologically complex media: The essential role of shear thinning. J. Rheol. 2014, 58, 237. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Calejo, J.; Pinho, D.; Galindo-Rosales, F.J.; Lima, R.; Campo-Deaño, L. Particulate Blood Analogues Reproducing the Erythrocytes Cell-Free Layer in a Microfluidic Device Containing a Hyperbolic Contraction. Micromachines 2016, 7, 4. https://doi.org/10.3390/mi7010004
Calejo J, Pinho D, Galindo-Rosales FJ, Lima R, Campo-Deaño L. Particulate Blood Analogues Reproducing the Erythrocytes Cell-Free Layer in a Microfluidic Device Containing a Hyperbolic Contraction. Micromachines. 2016; 7(1):4. https://doi.org/10.3390/mi7010004
Chicago/Turabian StyleCalejo, Joana, Diana Pinho, Francisco J. Galindo-Rosales, Rui Lima, and Laura Campo-Deaño. 2016. "Particulate Blood Analogues Reproducing the Erythrocytes Cell-Free Layer in a Microfluidic Device Containing a Hyperbolic Contraction" Micromachines 7, no. 1: 4. https://doi.org/10.3390/mi7010004
APA StyleCalejo, J., Pinho, D., Galindo-Rosales, F. J., Lima, R., & Campo-Deaño, L. (2016). Particulate Blood Analogues Reproducing the Erythrocytes Cell-Free Layer in a Microfluidic Device Containing a Hyperbolic Contraction. Micromachines, 7(1), 4. https://doi.org/10.3390/mi7010004