Nanostructured Composites Based on Liquid-Crystalline Elastomers
Abstract
1. Introduction
2. LCE-Based Composites with Carbon Micro- and Nanostructured Materials
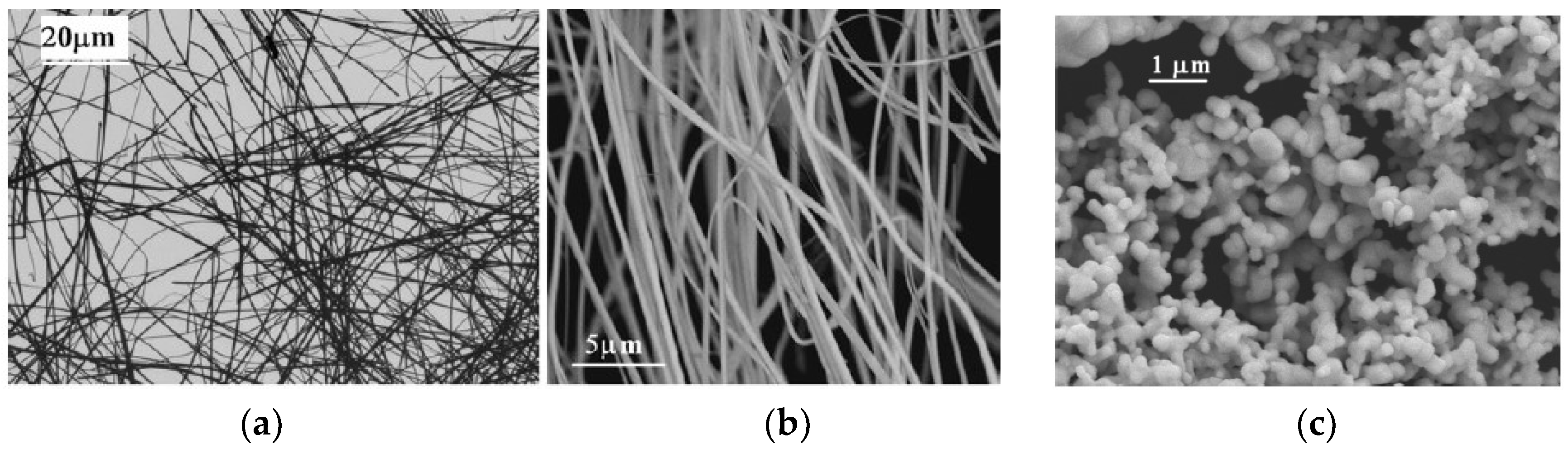
2.1. Sample Preparation and Characterization of Carbon-Based LCE Composites
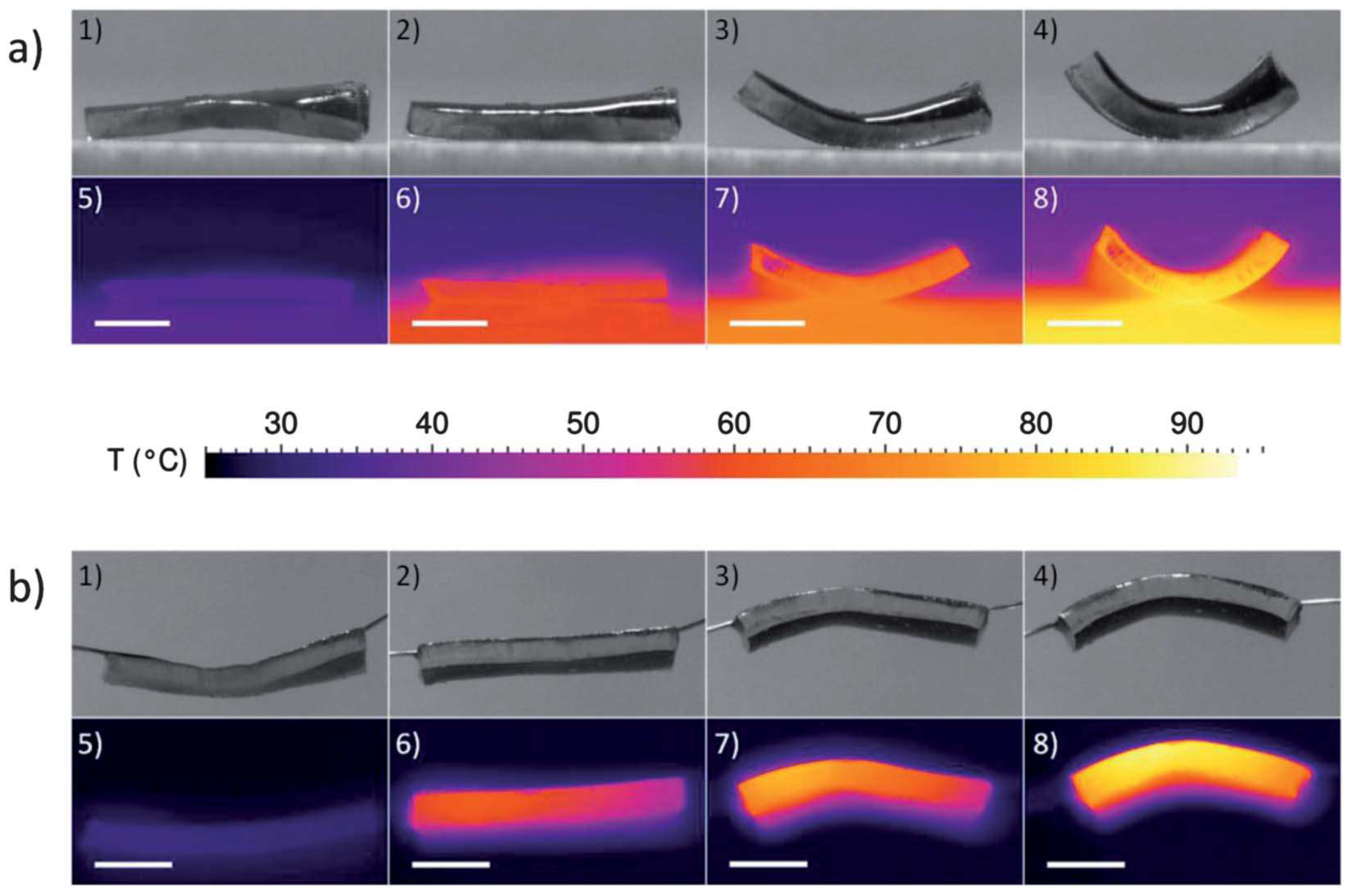
2.2. Applications of the Carbon-Based LCE Composites
3. LCE-Based Composites with Other Nanomaterials
3.1. Preparation of Nanomaterial-Based LCE Composites
3.2. Alignment of Nanomaterials in the LCE-Based Composites and Orientational-Ordering Properties
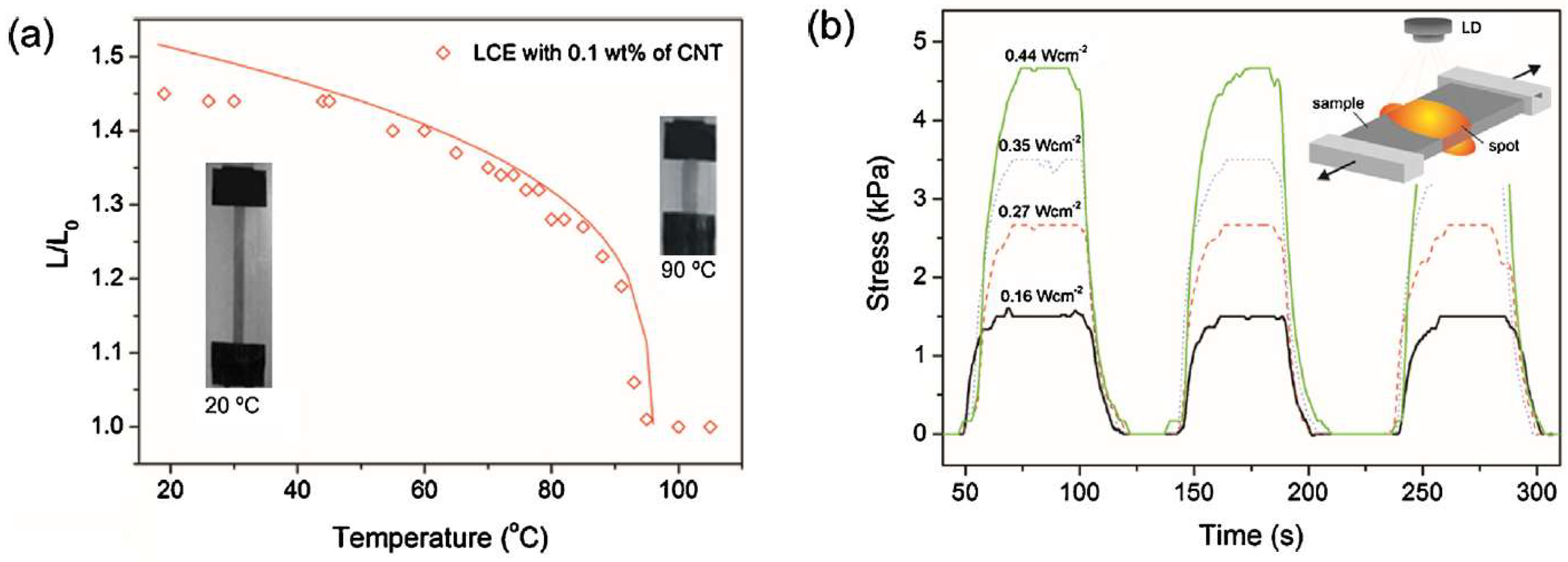
3.3. Actuation Properties and Applications of Nanomaterial-Based LCE Composites
4. LCE-Based Bilayer Composites
4.1. Preparation of Bilayered (or Multilayered) Systems
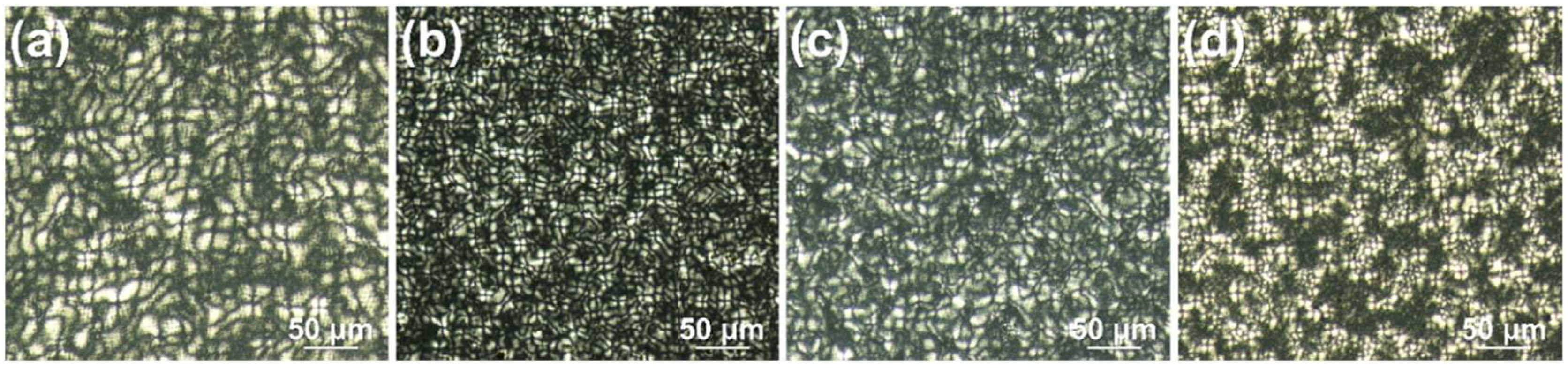
4.2. Bending Actuation and Surface Micro-Wrinkling Phenomena
5. General Conclusions and Perspectives
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| AFM | Atomic force microscopy |
| C60 | Fullerene |
| CNT | Carbon nanotube |
| GNP | Gold nanoparticle |
| DLS | Dynamic light scattering |
| LC | Liquid crystal |
| LCE | Liquid-crystal elastomer |
| LCE@GNP | Liquid-crystal elastomer composite including gold nanoparticles |
| LCP | Liquid-crystal polymer |
| LMMLC | Low-molecular-mass liquid crystal |
| LSCE | Liquid single-crystal elastomer |
| MWCNT | Multi-walled carbon nanotube |
| MEMS | Micro-electromechanical systems |
| N | Nematic phase |
| NEMS | Nano-electromechanical systems |
| NMR | Nuclear magnetic resonance |
| PEDOT | Poly(3,4-ethylenedioxythiophene) |
| PEDOT:PSS | Poly(3,4-ethylenedioxythiophene):poly(styrenesulfonate) |
| PCNT | Polymer composite made of CNT |
| PC60 | Polymer composite made of C60 |
| PG | Polymer composite made of graphene |
| PN | Paranematic phase |
| PS | Poly(styrene) |
| S | Orientational-order parameter |
| SAXS | Small-angle X-ray scattering |
| SANS | Small-angle neutron scattering |
| SEM | Scanning electron microscopy |
| SmA | Smectic A phase |
| SWCNT TEM | Single-walled carbon nanotube Trasmission electron microscopy |
| TN-PN | Temperature of the transition from the nematic to the paranematic phases |
| WAXS | Wide-angle X-ray scattering |
| XRD | X-ray diffraction |
References
- Chung, T.-S. Thermotropic Liquid Crystal Polymers: Thin-Film, Polymerization, Characterization, Blends and Applications; CRC Press: London, UK, 2001; ISBN 978-1566769433. [Google Scholar]
- Finkelmann, H. Synthesis, structure, and properties of liquid crystalline side-chain polymers. In Polymer Liquid Crystals, 1st ed.; Ciferri, A., Krigbaum, W.R., Meyer, R.B., Eds.; Academic Press: New York, NY, USA, 1982; pp. 35–61. ISBN 0-12-174680-1. [Google Scholar]
- Encinar, M.; Martínez-Gómez, A.; Rubio, R.G.; Pérez, E.; Bello, A.; Prolongo, M.G. X-ray Diffraction, Calorimetric, and Dielectric Relaxation Study of the Amorphous and Smectic States of a Main Chain Liquid Crystalline Polymer. J. Phys. Chem. B 2012, 116, 9846–9859. [Google Scholar] [CrossRef] [PubMed]
- Warner, M.; Terentjev, E.M. Liquid Crystal Elastomers; Oxford University Press: Cambridge, MA, USA, 2007; ISBN 978-0815100072. [Google Scholar]
- Ohm, C.; Brehmer, M.; Zentel, R. Liquid crystalline elastomers as actuators and sensors. Adv. Mater. 2010, 22, 3366–3387. [Google Scholar] [CrossRef] [PubMed]
- Kularatne, R.S.; Kim, H.; Boothby, J.M.; Ware, T.H. Liquid Crystal Elastomer Actuators: Synthesis, Alignment, and Applications. J. Polym. Sci. Part B Polym. Phys. 2017, 55, 395–411. [Google Scholar] [CrossRef]
- Terentjev, E.M. Physical properties of Liquid Crystalline Elastomers. In Cross-Linked Liquid Crystalline Systems: From Rigid Polymer Networks to Elastomers, 1st ed.; Broer, D.J., Crawford, G.P., Zumer, S., Eds.; CRC Press: Boca Raton, FL, USA, 2011; pp. 349–379. ISBN 978-1-4200-4622-9. [Google Scholar]
- Li, M.-H.; Keller, P. Side-on Nematic Liquid-Crystalline Elastomers for Artificial Muscle Applications. In Cross-Linked Liquid Crystalline Systems: From Rigid Polymer Networks to Elastomers, 1st ed.; Broer, D.J., Crawford, G.P., Zumer, S., Eds.; CRC Press: Boca Raton, FL, USA, 2011; pp. 565–586. ISBN 978-1-4200-4622-9. [Google Scholar]
- Kato, T.; Uchida, J.; Ichikawa, T.; Sakamoto, T. Functional liquid crystals towards the next generation of materials. Angew. Chem. Int. Ed. 2018, 57, 2–19. [Google Scholar] [CrossRef] [PubMed]
- White, T.W. Photomechanical effects in liquid crystalline polymer networks and elastomers. J. Polym. Sci. Part B Polym. Phys. 2018, 56, 695–705. [Google Scholar] [CrossRef]
- Shahsavan, H.; Yu, L.; Jakli, A.; Zhao, B.X. Smart biomimetic micro/nanostructures based on liquid crystal elastomers and networks. Soft Matter 2017, 13, 8006–8022. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.M.; Serpe, M.J. Stimuli-Responsive Polymers for Actuation. ChemPhysChem 2017, 18, 1451–1465. [Google Scholar] [CrossRef] [PubMed]
- Toshchevikov, V.; Petrova, T.; Saphiannikova, M. Kinetics of Ordering and Deformation in Photosensitive Azobenzene LC Networks. Polymers 2018, 10, 531. [Google Scholar] [CrossRef]
- De Gennes, P.-G. Réflexions sur un type de polymères nématiques. C. R. Acad. Sci. Paris Ser. B. 1975, 281, 101–103. [Google Scholar]
- Finkelmann, H.; Kock, H.J.; Rehage, G. Investigations on liquid-crystalline polysiloxanes. 3. Liquid crystalline elastomers: A new type of liquid crystalline materials. Makromol. Chem. Rapid Commun. 1981, 2, 317–322. [Google Scholar] [CrossRef]
- Greve, A.; Finkelmann, H. Nematic elastomers: The dependence of phase transformation and orientation processes on crosslinking topology. Macromol. Chem. Phys. 2001, 202, 2926–2946. [Google Scholar] [CrossRef]
- Li, M.H.; Keller, P. Artificial muscles based on liquid crystal elastomers. Philos. Trans. R. Soc. Lond. Ser. A 2006, 364, 2763–2777. [Google Scholar] [CrossRef] [PubMed]
- Pei, Z.; Yang, Y.; Chen, Q.; Terentjev, E.M.; Wei, Y.; Ji, Y. Mouldable liquid-crystalline elastomer actuators with exchangeable covalent bonds. Nat. Mater. 2014, 13, 36–41. [Google Scholar] [CrossRef] [PubMed]
- Hessberger, T.; Braun, L.; Zentel, R. Microfluidic Synthesis of Actuating Microparticles from a Thiol-Ene Based Main-Chain Liquid Crystalline Elastomer. Polymers 2016, 8, 410. [Google Scholar] [CrossRef]
- Resetic, A.; Milavec, J.; Zupancic, B.; Domenici, V.; Zalar, B. Polymer-dispersed liquid crystal elastomers. Nat. Commun. 2016, 7, 13140. [Google Scholar] [CrossRef] [PubMed]
- Domenici, V. H-2 NMR studies of liquid crystal elastomers: Macroscopic vs. molecular properties. Prog. Nucl. Magn. Reson. Spectrosc. 2012, 63, 1–32. [Google Scholar] [CrossRef] [PubMed]
- Bar-Cohen, Y. (Ed.) Electroactive Polymer (EAP) Actuators as Artificial Muscles: Reality, Potential, and Challenges, 2nd ed.; SPIE Press Monograph: Los Angeles, CA, USA, 2004; Volume PM136, ISBN 9780819452979. [Google Scholar]
- Wang, T.; Farajollahi, M.; Choi, Y.S.; Lin, I.-T.; Marshall, J.E.; Thompson, N.M.; Kar-Narayan, S.; Madden, J.D.W.; Smoukov, S.K. Electroactive polymers for sensing. Interface Focus 2016, 6, 20160026. [Google Scholar] [CrossRef] [PubMed]
- Meng, H.; Li, G. A review of stimuli-responsive shape memory polymer composites. Polymer 2013, 54, 2199–2221. [Google Scholar] [CrossRef]
- Van Oosten, C.L.; Bastiaansen, C.M.W.; Broer, D.J. Printed artificial cilia from liquid-crystal network actuators modularly driven by light. Nat. Mater. 2009, 8, 677–682. [Google Scholar] [CrossRef] [PubMed]
- Beyer, P.; Terentjev, E.M.; Zentel, R. Monodomain liquid crystal main chain elastomers by photocrosslinking. Macromol. Rapid Commun. 2007, 28, 1485–1490. [Google Scholar] [CrossRef]
- Ikeda, T.; Mamiya, J.; Yu, Y.L. Photomechanics of liquid-crystalline elastomers and other polymers. Angew. Chem.-Int. Ed. 2007, 46, 506–528. [Google Scholar] [CrossRef] [PubMed]
- Devetak, M.; Zupancic, B.; Lebar, A.; Umek, P.; Zalar, B.; Domenici, V.; Ambrozic, G.; Zigon, M.; Copic, M.; Drevensek-Olenik, I. Micropatterning of light-sensitive liquid-crystal elastomers. Phys. Rev. E 2009, 80, 050701. [Google Scholar] [CrossRef] [PubMed]
- Marshall, J.E.; Terentjev, E.M. Photo-sensitivity of dye-doped liquid crystal elastomers. Soft Matter 2013, 9, 8547–8551. [Google Scholar] [CrossRef]
- Ji, Y.; Marshall, J.E.; Terentjev, E.M. Nanoparticle-Liquid Crystalline Elastomer Composites. Polymers 2012, 4, 316–340. [Google Scholar] [CrossRef]
- Jiang, H.; Li, C.; Huang, X. Actuators based on liquid crystalline elastomer materials. Nanoscale 2013, 5, 5225–5240. [Google Scholar] [CrossRef] [PubMed]
- Ware, T.H.; McConney, M.E.; Wie, J.J.; Tondiglia, V.P.; White, T.J. Actuating materials. Voxelated liquid crystal elastomers. Science 2015, 347, 982–984. [Google Scholar] [CrossRef] [PubMed]
- Ware, T.H.; Biggins, J.S.; Shick, A.F.; Warner, M.; White, T.J. Localized soft elasticity in liquid crystal elastomers. Nat. Commun. 2016, 7, 10781. [Google Scholar] [CrossRef] [PubMed]
- Camacho-Lopez, M.; Finkelmann, H.; Palffy-Muhoray, P.; Shelley, M. Fast liquid-crystal elastomer swims into the dark. Nat. Mater. 2004, 3, 307–310. [Google Scholar] [CrossRef] [PubMed]
- Kupfer, J.; Finkelmann, H. Nematic liquid single-crystal elastomers. Makromol. Chem. Rapid Commun. 1991, 12, 717–726. [Google Scholar] [CrossRef]
- Chambers, M.; Zalar, B.; Remskar, M.; Zumer, S.; Finkelmann, H. Actuation of liquid crystal elastomers reprocessed with carbon nanoparticles. Appl. Phys. Lett. 2006, 89, 243116. [Google Scholar] [CrossRef]
- Chambers, M.; Zalar, B.; Remskar, M.; Kovac, J.; Finkelmann, H.; Zumer, S. Investigations on an integrated conducting nanoparticle-liquid crystal elastomer layer. Nanotechnology 2007, 18, 415706. [Google Scholar] [CrossRef]
- Chambers, M.; Zalar, B.; Remskar, M.; Finkelmann, H.; Zumer, S. Piezoresistivity and electro-thermomechanical degradation of a conducting layer of nanoparticles integrated at the liquid crystal elastomer surface. Nanotechnology 2008, 19, 155501. [Google Scholar] [CrossRef] [PubMed]
- Chambers, M.; Finkelmann, H.; Remskar, M.; Sanchez-Ferrer, A.; Zalar, B.; Zumer, S. Liquid crystal elastomer-nanoparticle systems for actuation. J. Mater. Chem. 2009, 19, 1524–1531. [Google Scholar] [CrossRef]
- Marshall, J.E.; Ji, Y.; Torras, N.; Zinoviev, K.; Terentjev, E.M. Carbon-nanotube sensitized nematic elastomers for IR-visible photo-actuation. Soft Matter 2012, 8, 1570–1574. [Google Scholar] [CrossRef]
- Li, C.; Liu, Y.; Lo, C.; Jiang, H. Reversible white-light actuation of carbon nano-tube incorporated liquid crystalline elastomer nanocomposites. Soft Matter 2011, 7, 7511–7516. [Google Scholar] [CrossRef]
- Ahir, S.V.; Huang, Y.Y.; Terentjev, E.M. Polymers with aligned carbon nanotubes: Active composite materials. Polymer 2008, 49, 3841–3854. [Google Scholar] [CrossRef]
- Torras, N.; Zinoviev, K.E.; Camargo, C.J.; Campo, E.M.; Campanella, H.; Esteve, F.; Marshall, J.E.; Terentjev, E.M.; Omastová, M.; Krupa, I.; et al. Tactile device based on opto-mechanical actuation of liquid crystal elastomers. Sens. Actuators A 2014, 208, 104–112. [Google Scholar] [CrossRef]
- Lama, G.C.; Cerruti, P.; Lavorgna, M.; Carfagna, C.; Ambrogi, V.; Gentile, G. Controlled Actuation of a Carbon Nanotube/Epoxy Shape-Memory Liquid Crystalline Elastomer. J. Phys. Chem. C 2016, 120, 24417–24426. [Google Scholar] [CrossRef]
- Meng, Z.-Y.; Chen, L.; Zhong, H.-Y.; Yang, R.; Liu, X.-F.; Wang, Y.-Z. Effect of different dimensional carbon nanoparticles on the shape memory behavior of thermotropic liquid crystalline polymer. Compos. Sci. Technol. 2017, 138, 8–14. [Google Scholar] [CrossRef]
- Lee, S.K.; Kim, H.; Shim, B.S. Graphene: An emerging material for biological tissue engineering. Carbon Lett. 2013, 14, 63–75. [Google Scholar] [CrossRef]
- Yang, Y.; Zhan, W.; Peng, R.; He, C.; Pang, X.; Shi, D.; Jiang, T.; Lin, Z. Graphene-Enabled Superior and Tunable Photomechanical Actuation in Liquid Crystalline Elastomer Nanocomposites. Adv. Mater. 2015, 27, 6376–6381. [Google Scholar] [CrossRef] [PubMed]
- Dong, L.; Tong, X.; Zhang, H.; Chen, M.; Zhao, Y. Near-infrared light-driven locomotion of a liquid crystal polymer trilayer actuator. Mater. Chem. Front. 2018, 2, 1383–1388. [Google Scholar] [CrossRef]
- Hess, W.M.; Herd, C.R. Microstructure, morphology and general physical properties. In Carbon Black: Science and Technology, 2nd ed.; Donnet, J.P., Bansal, R., Wang, M.-J., Eds.; Marcel Dekker Inc.: New York, NY, USA, 1993; ISBN 0-8247-8975-X. [Google Scholar]
- Laszlo, K.; Podkoscilny, P.; Dabrowski, A. Heterogeneity of polymer-based active carbons in adsorption of aqueous solutions of phenol and 2,3,4-trichlorophenol. Langmuir 2003, 19, 5287–5294. [Google Scholar] [CrossRef]
- Urayama, K.; Takigawa, T. Electro-mechanical effects in swollen Nematic Elastomers. In Cross-Linked Liquid Crystalline Systems: From Rigid Polymer Networks to Elastomers, 1st ed.; Broer, D.J., Crawford, G.P., Zumer, S., Eds.; CRC Press: Boca Raton, FL, USA, 2011; pp. 473–486. ISBN 978-1-4200-4622-9. [Google Scholar]
- Boothby, J.M.; Kim, H.; Ware, T.H. Shape changes in chemoresponsive liquid crystal elastomers. Sens. Actuators B Chem. 2017, 240, 511–518. [Google Scholar] [CrossRef]
- Cmok, L.; Petelin, A.; Copic, M. Nematic fluctuations and semisoft elasticity in swollen liquid-crystal elastomers. Phys. Rev. E 2015, 91, 042502. [Google Scholar] [CrossRef] [PubMed]
- Skacej, G. Sample preparation affects the nematic-isotropic transition in liquid crystal elastomers: Insights from molecular simulation. Soft Matter 2018, 14, 1408–1416. [Google Scholar] [CrossRef] [PubMed]
- Lucantonio, A.; DeSimone, A. Coupled swelling and nematic reordering in liquid crystal gels. Soft Matter 2017, 13, 7907–7915. [Google Scholar] [CrossRef] [PubMed]
- Camargo, C.J.; Campanella, H.; Marshall, J.E.; Torras, N.; Zinoviev, K.; Terentjev, E.M.; Esteve, J. Batch fabrication of optical actuators using nanotube–elastomer composites towards refreshable Braille displays. J. Micromech. Microeng. 2012, 22, 075009. [Google Scholar] [CrossRef]
- Li, C.; Liu, Y.; Huang, X.; Jiang, H. Direct Sun-Driven Artificial Heliotropism for Solar Energy Harvesting Based on a Photo-Thermomechanical Liquid Crystal Elastomer Nanocomposite. Adv. Funct. Mater. 2012, 22, 5166–5174. [Google Scholar] [CrossRef]
- Torras, N.; Zinoviev, K.E.; Camargo, C.J.; Campo, E.M.; Campanella, H.; Esteve, J.; Marshal, J.E.; Terentjev, E.M.; Omastova, M.; Krupa, I.; et al. Nematic Opto-Mechanical Actuators for the Fabrication of Refreshable Tactile Systems. Transducers 2013, 13, 1691–1694. [Google Scholar]
- Domenici, V.; Zupancic, B.; Remskar, M.; Laguta, V.V.; Veracini, C.A.; Zalar, B. New composites based on liquid crystalline elastomers and electroactive nanomaterials. In Artificial Muscle Actuators Using Electro-active Polymers; Advances in Science and Technology; Vincenzini, P., BarCohen, Y., Carpi, F., Eds.; Trans Tech Publications LTD: Durnten-Zurich, Switzerland, 2009; Volume 61, pp. 34–40. ISBN 978-3-908158-27-1. [Google Scholar]
- Domenici, V.; Zupancic, B.; Laguta, V.V.; Belous, A.G.; V’yunov, O.I.; Remskar, M.; Zalar, B. PbTiO3 Nanoparticles Embedded in a Liquid Crystalline Elastomer Matrix: Structural and Ordering Properties. J. Phys. Chem. C 2010, 114, 10782–10789. [Google Scholar] [CrossRef]
- Domenici, V.; Conradi, M.; Remskar, M.; Virsek, M.; Zupancic, B.; Mrzel, A.; Chambers, M.; Zalar, B. New composite films based on MoO3-x nanowires aligned in a liquid single crystal elastomer matrix. J. Mater. Sci. 2011, 46, 3639–3645. [Google Scholar] [CrossRef]
- Kaiser, A.; Winkler, M.; Krause, S.; Finkelmann, H.; Schmidt, A.M. Magnetoactive liquid crystal elastomer nanocomposites. J. Mater. Chem. 2009, 19, 538–543. [Google Scholar] [CrossRef]
- Haberl, J.M.; Sanchez-Ferrer, A.; Mihut, A.M.; Dietsch, H.; Hirt, A.M.; Mezzenga, R. Liquid-Crystalline Elastomer-Nanoparticle Hybrids with Reversible Switch of Magnetic Memory. Adv. Mater. 2013, 25, 1787–1791. [Google Scholar] [CrossRef] [PubMed]
- Haberl, J.M.; Sanchez-Ferrer, A.; Mihut, A.M.; Dietsch, H.; Hirt, A.M.; Mezzenga, R. Strain-induced macroscopic magnetic anisotropy from smectic liquid-crystalline elastomer-maghemite nanoparticle hybrid nanocomposites. Nanoscale 2013, 5, 5539–5548. [Google Scholar] [CrossRef] [PubMed]
- Herrera-Posada, S.; Mora-Navarro, C.; Ortiz-Bermudez, P.; Torres-Lugo, M.; McElhinny, K.M.; Evans, P.G.; Calcagno, B.O.; Acevedo, A. Magneto-responsive liquid crystalline elastomer nanocomposites as potential candidates for dynamic cell culture substrates. Mater. Sci. Eng. C 2016, 65, 369–378. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Evans, J.S.; Lee, T.; Senyuk, B.; Keller, P.; He, S.; Smalyukh, I.I. Optical manipulation of shape-morphing elastomeric liquid crystal microparticles doped with gold nanocrystals. Appl. Phys. Lett. 2012, 100, 241901. [Google Scholar] [CrossRef]
- Montazami, R.; Spillmann, C.M.; Naciri, J.; Ratna, B.R. Enhanced thermomechanical properties of a nematic liquid crystal elastomer doped with gold nanoparticles. Sens. Actuators A 2012, 178, 175–178. [Google Scholar] [CrossRef]
- Wjjcik, M.M.; Wrjbel, J.; Janczuk, Z.Z.; Mieczkowski, J.; Gorecka, E.; Choi, J.; Cho, M.; Pociecha, D. Liquid-Crystalline Elastomers with Gold Nanoparticle Cross-Linkers. Chem. Eur. J. 2017, 23, 8912–8920. [Google Scholar] [CrossRef] [PubMed]
- Lebar, A.; Kutnjak, Z.; Zumer, S.; Finkelmann, H.; Sanchez-Ferrer, A.; Zalar, B. Evidence of supercritical behavior in liquid single crystal elastomers. Phys. Rev. Lett. 2005, 94, 197801. [Google Scholar] [CrossRef] [PubMed]
- Lebar, A.; Cordoyiannis, G.; Kutnjak, Z.; Zalar, B. The Isotropic-to-Nematic Conversion in Liquid Crystalline Elastomers. In Liquid Crystal Elastomers: Materials and Applications; Advances in Polymer Science; De Jeu, W.H., Ed.; Springer-Verlag: Berlin, Germany, 2012; ISBN 978-3-642-31581-7. [Google Scholar]
- Domenici, V.; Zalar, B. Paranematic-nematic phase transition in liquid crystalline elastomers: A 2H-NMR study. Phase Trans. 2010, 83, 1014–1025. [Google Scholar] [CrossRef]
- Domenici, V.; Ambrozic, G.; Copic, M.; Lebar, A.; Drevensek-Olenik, I.; Umek, P.; Zalar, B.; Zupancic, B.; Zigon, M. Interplay between nematic ordering and thermomechanical response in a side-chain liquid single crystal elastomer containing pendant azomesogen units. Polymer 2009, 50, 4837–4844. [Google Scholar] [CrossRef]
- Milavec, J.; Domenici, V.; Zupancic, B.; Resetic, A.; Bubnov, A.; Zalar, B. Deuteron NMR resolved mesogen vs. crosslinker molecular order and reorientational exchange in liquid single crystal elastomers. Phys. Chem. Chem. Phys. 2016, 18, 4071–4077. [Google Scholar] [CrossRef] [PubMed]
- Domenici, V.; Milavec, J.; Zupančič, B.; Bubnov, A.; Hamplova, V.; Zalar, B. Brief overview on 2H NMR studies of polysiloxane-based side-chain nematic elastomers. Magn. Reson. Chem. 2014, 52, 649–655. [Google Scholar] [CrossRef] [PubMed]
- Degert, C.; Davidson, P.; Megtert, S.; Petermann, D.; Mauzac, M. X-ray diffraction by liquid-crystalline elastomers. Liq. Cryst. 1992, 12, 779–798. [Google Scholar] [CrossRef]
- Davison, P. X-ray diffraction by liquid crystalline side-chain polymers. Prog. Polym. Sci. 1996, 21, 893–950. [Google Scholar] [CrossRef]
- Noirez, L.; Keller, P.; Davidson, P.; Hardouin, F.; Cotton, J.P. Backbone conformation study of a side chain polyacrylate through a re-entrant polymorphism. J. Phys. France 1988, 49, 1993–1999. [Google Scholar] [CrossRef]
- Fikelmann, H.; Kaufhold, W.; Noirez, L.; Tenbosch, A.; Sixou, P. Chain Conformation in Nematic Elastomers. J. Phys. II 1994, 4, 1363–1373. [Google Scholar]
- De Jeu, W.H.; Ostrovskii, B.I. Order and Disorder in Liquid-Crystalline Elastomers. In Liquid Crystal Elastomers: Materials and Applications; Advances in Polymer Science; De Jeu, W.H., Ed.; Springer: Berlin/Heidelberg, Germany, 2012; Volume 250, pp. 187–234. [Google Scholar]
- Liu, X.Y.; Wang, X.G.; Liu, T.; Keller, P. Gold Nanoparticles Incorporated Nematic Gel Micropillars Capable of Laser Actuation at Room Temperature. Macromolecules 2016, 49, 8322–8331. [Google Scholar] [CrossRef]
- Lu, X.; Zhang, H.; Fei, G.; Yu, B.; Tong, X.; Xia, S.; Zhao, Y. Liquid-Crystalline Dynamic Networks Doped with Gold Nanorods Showing Enhanced Photocontrol of Actuation. Adv. Mater. 2018, 30, 1706597. [Google Scholar] [CrossRef] [PubMed]
- Hernandez, J.C.; Reyes, J.A. Optical band gap in a cholesteric elastomer doped by metallic nanospheres. Phys. Rev. E 2017, 96, 062701. [Google Scholar] [CrossRef] [PubMed]
- Ge, S.J.; Shan, Z.N.; Deng, L.L.; Lin, B.P.; Yang, H. Near-infrared-responsive Copper Sulfide Nanoparticle/Liquid Crystal Elastomer Composites. Acta Polym. Sin. 2017, 10, 1633–1640. [Google Scholar] [CrossRef]
- Zhang, J.D.; Wang, J.; Zhao, L.N.; Yang, W.L.; Bi, M.; Wang, Y.C.; Mu, H.Y.; Li, Y.X.; Wang, B.S.; Gao, Y.C.; et al. Photo Responsive Silver Nanoparticles Incorporated Liquid Crystalline Elastomer Nanocomposites Based on Surface Plasmon Resonance. Chem. Res. Chin. Univ. Chin. 2017, 33, 839–846. [Google Scholar] [CrossRef]
- Romiti, S. New Actuators Based on Liquid Crystal Elastomers and Conductive Polymers. Master’s Thesis, University of Pisa, Pisa, Italy, 2012. [Google Scholar]
- Domenici, V.; Greco, F.; Mattoli, V.; Zalar, B.; Zupancic, B.; Assaf, T.; Romiti, S. Reversible heat-induced microwrinking of PEDOT:PSS nanofilm surface over a monodomain Liquid Crystal Elastomer. In Proceedings of the 6th International Liquid Crystal Elastomer Conference, ILCEC2011, Lisbon, Portugal, 6 September 2011. [Google Scholar]
- Greco, F.; Domenici, V.; Assaf, T.; Romiti, S.; Mattoli, V. Bending Actuation of a Composite Liquid Crystal Elastomer via Direct Joule Heating. In Proceedings of the IEEE RAS-EMBS International Conference on Biomedical Robotics and Biomechatronics, Rome, Italy, 24–27 June 2014; Desai, J.P., Jay, L.P.S., Zollo, L., Eds.; IEEE Publisher: New York, NY, USA, 2012; ISBN 978-1-4577-1200-5. [Google Scholar]
- Greco, F.; Domenici, V.; Romiti, S.; Assaf, T.; Zupancic, B.; Milavec, J.; Zalar, B.; Mazzolai, B.; Mattoli, V. Reversible Heat-Induced Microwrinkling of PEDOT:PSS Nanofilm Surface Over a Monodomain Liquid Crystal Elastomer. Mol. Cryst. Liq. Cryst. 2013, 572, 40–49. [Google Scholar] [CrossRef]
- Greco, F.; Domenici, V.; Desii, A.; Sinibaldi, E.; Zupancic, B.; Zalar, B.; Mazzolai, B.; Mattoli, V. Liquid single crystal elastomer/conducting polymer bilayer composite actuator: Modelling and experiments. Soft Matter 2013, 9, 11405–11416. [Google Scholar] [CrossRef]
- Agrawal, A.; Luchette, P.; Palffy-Muhoray, P.; Biswal, S.L.; Chapman, W.G.; Verduzco, R. Surface wrinkling in liquid crystal elastomers. Soft Matter 2012, 8, 7138–7142. [Google Scholar] [CrossRef]
- Agrawal, A.; Yun, T.H.; Pesek, S.L.; Chapman, W.G.; Verduzco, R. Shape-responsive liquid crystal elastomer bilayers. Soft Matter 2014, 10, 1411–1415. [Google Scholar] [CrossRef] [PubMed]
- Zupancic, B.; Zalar, B.; Remskar, M.; Domenici, V. Actuation of Gold-Coated Liquid Crystal Elastomers. App. Phys. Express 2013, 6, 021701. [Google Scholar] [CrossRef]
- Kolpak, A.; Domenici, V. Energy Storage Actuators Based On Liquid Crystal Elastomers; Scientific Report of the UNIPI-MIT 2014 PROJECT; MISTI: Boston, MA, USA, 26 July 2016. [Google Scholar]
- Domenici, V.; Milavec, J.; Cresta, V.; Resetic, A.; Zupancic, B.; Zalar, B.; Wagih, M.; Romano, G.; Kolpak, A. Bilayered composite materials based on liquid crystalline elastomers: Experiments and simulations. In Proceedings of the Materials Research Society 2016, Spring Meeting and Exhibit, SESSION SM1.1: Liquid Crystals in Displays and Beyond—Shape Control and Actuation, Phoenix, AZ, USA, 30 March–2 April 2016. [Google Scholar]
- Cui, Y.; Wang, C.; Sim, K.; Chen, J.; Li, Y.; Xing, Y.; Yu, C.; Song, J. A simple analytical thermo-mechanical model for liquid crystal elastomer bilayer structures. AIP Adv. 2018, 8, 025215. [Google Scholar] [CrossRef]
- Greco, F.; Mattoli, V. Introduction to Active Smart Materials for Biomedical Applications. In Piezoelectric Nanomaterials for Biomedical Applications; Ciofani, G., Menciassi, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2012; pp. 1–27. ISBN 978-3-642-28043-6. [Google Scholar]
- Greco, F.; Zucca, A.; Taccola, S.; Menciassi, A.; Fujie, T.; Haniuda, H.; Takeoka, S.; Dario, P.; Mattoli, V. Ultra-thin conductive free-standing PEDOT/PSS nanofilms. Soft Matter 2011, 7, 10642–10650. [Google Scholar] [CrossRef]
- Xu, F.; Lu, T.J.; Seffen, K.A. Biothermomechanics of skin tissues. J. Mech. Phys. Solids 2008, 56, 1852–1884. [Google Scholar] [CrossRef]
- Hsueh, C.H. Modeling of elastic deformation of multilayers due to residual stresses and external bending. J. Appl. Phys. 2002, 91, 9652–9656. [Google Scholar] [CrossRef]
- Nikishkov, G.P. Curvature estimation for multilayer hinged structures with initial strains. J. Appl. Phys. 2003, 94, 5333–5336. [Google Scholar] [CrossRef]
- Christophersen, M.; Shapiro, B.; Smela, E. Characterization and modelling of PPy bilayer microactuators. Part 1. Curvature. Sens. Actuators B Chem. 2006, 115, 596–609. [Google Scholar] [CrossRef]
- Chung, J.Y.; Nolte, A.J.; Stafford, C.M. Surface wrinkling: A versatile platform for measuring thin-film properties. Adv. Mater. 2011, 23, 349–368. [Google Scholar] [CrossRef] [PubMed]
- Cerda, E.; Mahadevan, L. Geometry and Physics of Wrinkling. Phys. Rev. Lett 2003, 90, 074302. [Google Scholar] [CrossRef] [PubMed]
- Harris, A.K.; Wild, P.; Stopak, D. Silicone rubber substrata: A new wrinkle in the study of cell locomotion. Science 1980, 208, 177–179. [Google Scholar] [CrossRef] [PubMed]
- Boothby, J.M.; Ware, T.H. Dual-responsive, shape-switching bilayers enabled by liquid crystal elastomers. Soft Matter 2017, 13, 4349–4356. [Google Scholar] [CrossRef] [PubMed]
- Tian, H.M.; Wang, Z.J.; Chen, Y.L.; Shao, J.Y.; Gao, T.; Cai, S.Q. Polydopamine-Coated Main-Chain Liquid Crystal Elastomer as Optically Driven Artificial Muscle. ACS Appl. Mater. Interfaces 2018, 10, 8307–8316. [Google Scholar] [CrossRef] [PubMed]
- Yuan, J.K.; Luna, A.; Neri, R.; Zakri, C.; Colin, A.; Poulin, P. Giant Electrostriction of Soft Nanocomposites Based on Liquid Crystalline Graphene. ACS Nano 2018, 12, 1688–1695. [Google Scholar] [CrossRef] [PubMed]
- Guin, T.; Kowalski, B.A.; Rao, R.; Auguste, A.D.; Grabowski, C.A.; Lloyd, P.F.; Tondiglia, V.P.; Maruyama, B.; Vaia, R.A.; White, T.J. Electrical Control of Shape in Voxelated Liquid Crystalline Polymer Nanocomposites. ACS Appl. Mater. Interfaces 2018, 10, 1187–1194. [Google Scholar] [CrossRef] [PubMed]
- Osicka, J.; Ilcikova, M.; Mrlik, M.; Minarik, A.; Pavlinek, V.; Mosnacek, J. The Impact of Polymer Grafting from a Graphene Oxide Surface on Its Compatibility with a PDMS Matrix and the Light-Induced Actuation of the Composites. Polymers 2017, 9, 264. [Google Scholar] [CrossRef]
- Chen, L.X.; Liu, J.Q.; Wang, X.L.; Ji, B.; Chen, X.A.; Yang, B. Flexible Capacitive Hydrogel Tactile Sensor With Adjustable Measurement Range Using Liquid Crystal and Carbon Nanotubes Composites. IEEE Trans. Electron Devices 2017, 64, 1968–1972. [Google Scholar] [CrossRef]
- Li, Z.; Yang, Y.; Wang, Z.H.; Zhang, X.Y.; Chen, Q.M.; Qian, X.J.; Liu, N.; Wei, Y.; Ji, Y. Polydopamine nanoparticles doped in liquid crystal elastomers for producing dynamic 3D structures. J. Mater. Chem. A 2017, 5, 6740–6746. [Google Scholar] [CrossRef]
- Ko, H.; Javey, A. Smart Actuators and Adhesives for Reconfigurable Matter. Acc. Chem. Res. 2017, 50, 691–702. [Google Scholar] [CrossRef] [PubMed]
- Echeverria, C.; Almeida, P.L.; Feio, G.; Figueirinhas, J.L.; Godinho, M.H. A cellulosic liquid crystal pool for cellulose nanocrystals: Structure and molecular dynamics at high shear rates. Eur. Polym. J. 2015, 72, 72–81. [Google Scholar] [CrossRef]
- Echeverria, C.; Aguirre, L.E.; Merino, E.G.; Almeida, P.L.; Godinho, M.H. Carbon Nanotubes as Reinforcement of Cellulose Liquid Crystalline Responsive Networks. ACS Appl. Mater. Interfaces 2015, 7, 21005–21009. [Google Scholar] [CrossRef] [PubMed]
- Bai, J.; Shi, Z.; Yin, J.; Tian, M.; Qu, R. Shape Reconfiguration of a Biomimetic Elastic Membrane with a Switchable Janus Structure. Adv. Funct. Mater. 2018, in press. [Google Scholar] [CrossRef]
- Rey, A.D.; Servio, P.; Valenci, E.E.H. Stress-Sensor Device Based on Flexoelectric Liquid Crystalline Membranes. ChemPhysChem 2014, 15, 1405–1412. [Google Scholar] [CrossRef] [PubMed]
- Gregorc, M.; Li, H.; Domenici, V.; Ambrozic, G.; Copic, M.; Drevensek-Olenik, I. Kinetics of Holographic Recording and Spontaneous Erasure Processes in Light-Sensitive Liquid Crystal Elastomers. Materials 2012, 5, 741–753. [Google Scholar] [CrossRef] [PubMed]
- Prijatelj, M.; Ellabban, M.A.; Fally, M.; Domenici, V.; Copic, M.; Drevensek-Olenik, I. Peculiar behaviour of optical polarization gratings in light-sensitive liquid crystalline elastomers. Opt. Mater. Express 2016, 6, 961–970. [Google Scholar] [CrossRef]
- Bošnjaković, D.; Gregorc, M.; Li, H.; Čopič, M.; Domenici, V.; Drevenšek-Olenik, I. Mechanical Manipulation of Diffractive Properties of Optical Holographic Gratings from Liquid-Crystalline Elastomers. Appl. Sci. 2018. submitted. [Google Scholar]
- Trcek, M.; Lavric, M.; Cordoyiannis, G.; Zalar, B.; Rozic, B.; Kralj, S.; Tzitzios, V.; Nounesis, G.; Kutnjak, Z. Electrocaloric and elastocaloric effects in soft materials. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 20150301. [Google Scholar] [CrossRef] [PubMed]
- Wei, R.B.; Wang, Z.C.; Zhang, H.X.; Liu, X.B. Photo-responsive liquid crystalline elastomer with reduced chemically modified graphene oxide. Liq. Cryst. 2016, 43, 1009–1016. [Google Scholar] [CrossRef]
- Jampani, V.S.R.; Mulder, D.J.; De Sousa, K.R.; Gélébart, A.; Lagerwall, J.P.F.; Schenning, A.P.H.J. Micrometer-Scale Porous Buckling Shell Actuators Based on Liquid Crystal Networks. Adv. Opt. Mater. 2018, 1801209. [Google Scholar] [CrossRef]
- Cheng, Q.; Song, Z.; Ma, T.; Smith, B.B.; Tang, T.; Yu, H.; Jiang, H.; Chan, C.K. Folding Paper-Based Lithium-Ion Batteries for Higher Areal Energy Densities. Nano Lett. 2013, 13, 4969–4974. [Google Scholar] [CrossRef] [PubMed]
- Hwang, T.; Kwon, H.-Y.; Oh, J.-Y.; Hong, J.-P.; Hong, S.-C.; Lee, Y.; Choi, H.R.; Kim, K.J.; Bhuiya, M.H.; Nam, J.D. Transparent actuator made with few layer graphene electrode and dielectric elastomer, for variable focus lens. Appl. Phys. Lett. 2013, 103, 023106. [Google Scholar] [CrossRef]















© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cresta, V.; Romano, G.; Kolpak, A.; Zalar, B.; Domenici, V. Nanostructured Composites Based on Liquid-Crystalline Elastomers. Polymers 2018, 10, 773. https://doi.org/10.3390/polym10070773
Cresta V, Romano G, Kolpak A, Zalar B, Domenici V. Nanostructured Composites Based on Liquid-Crystalline Elastomers. Polymers. 2018; 10(7):773. https://doi.org/10.3390/polym10070773
Chicago/Turabian StyleCresta, Vanessa, Giuseppe Romano, Alexej Kolpak, Boštjan Zalar, and Valentina Domenici. 2018. "Nanostructured Composites Based on Liquid-Crystalline Elastomers" Polymers 10, no. 7: 773. https://doi.org/10.3390/polym10070773
APA StyleCresta, V., Romano, G., Kolpak, A., Zalar, B., & Domenici, V. (2018). Nanostructured Composites Based on Liquid-Crystalline Elastomers. Polymers, 10(7), 773. https://doi.org/10.3390/polym10070773