Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials
Abstract
:1. Introduction
2. Materials and Methods
3. Results
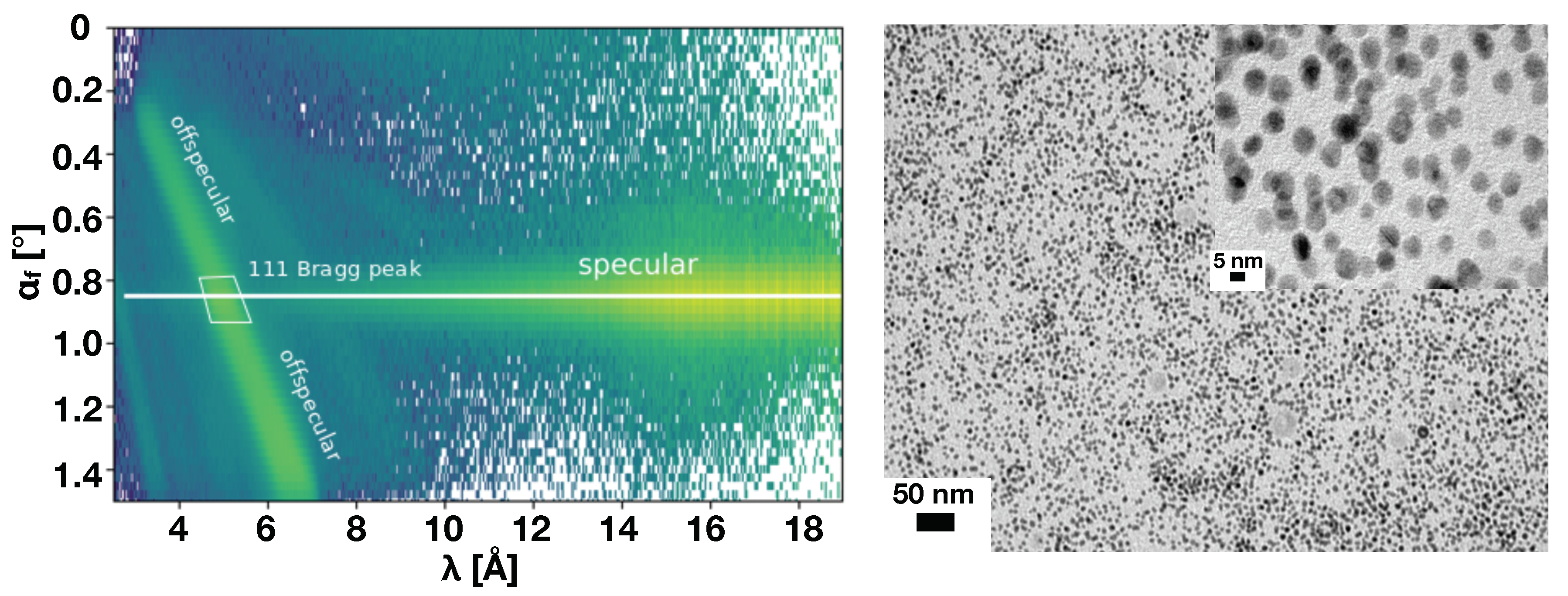
3.1. GISANS
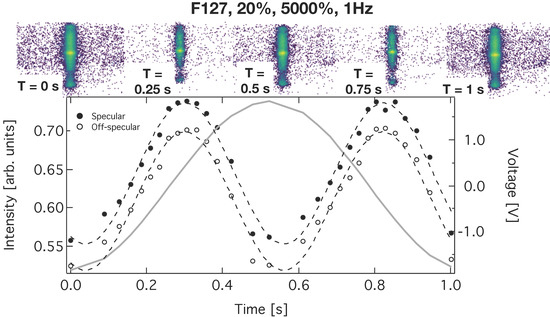
3.2. Time Resolved Measurements
3.3. Polarised Time Resolved Measurements
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| GISANS | Grazing incidence small angle neutron scattering. |
| NR | Neutron reflectivity |
| TEM | Transmission electron microscopy |
| SLD | Scattering length density |
| FCC | Face centered cubic |
References
- Müller-Buschbaum, P.; Metwalli, E.; Moulin, J.-F.; Kudryashov, V.; Haese-Seiller, M.; Kampmann, R. Time of flight grazing incidence small angle neutron scattering. Eur. Phys. J. Spec. Top. 2009, 167, 107–112. [Google Scholar] [CrossRef]
- Wolff, M.; Herbel, J.; Adlmann, F.; Dennison, A.J.C.; Liesche, G.; Gutfreund, P.; Rogers, S. Depth-resolved gracing-incidence time-of-flight neutron scattering from solid-liquid surfaces. J. Appl. Cryst. 2014, 47, 130. [Google Scholar] [CrossRef]
- Dalgliesh, R.M.; Lau, Y.G.J.; Richardson, R.M.; Riley, D.J. Millisecond time resolution neutron reflection from a nematic liquid crystal. Rev. Sci. Instrum. 2004, 75, 2955. [Google Scholar] [CrossRef]
- Adlmann, F.A.; Gutfreund, P.; Ankner, J.F.; Browning, J.F.; Parizzi, A.; Vacaliuc, B.; Halbert, C.E.; Rich, J.P.; Dennisona, A.J.C.; Wolff, M. Towards neutron scattering experiments with sub- millisecond time resolution. J. Appl. Cryst. 2015, 48, 220–226. [Google Scholar] [CrossRef]
- Lopez-Barron, C.R.; Porcar, L.; Eberle, A.P.R.; Wagner, N.J. Dynamics of melting and recrystallization in a polymeric micellar crystal subjected to large amplitude oscillatory shear flow. Phys. Rev. Lett. 2012, 108, 258301. [Google Scholar] [CrossRef] [PubMed]
- Lopez-Barron, C.R.; Gurnon, K.; Eberle, A.P.R.; Porcar, L.; Wagner, N.J. Microstructural evolution of a model and shear-banding micellar solution during shear and startup and cessation. Phys. Rev. E 2014, 89, 042301. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.M.; Eberle, A.P.R.; Gurnon, A.K.; Porcar, L.; Wagner, N.J. The microstructure and rheology of a model, thixotropic nanoparticle gel under steady shear and large amplitude oscillatory shear (LAOS). J. Rheol. 2014, 58, 1301. [Google Scholar] [CrossRef]
- Gurnon, A.K.; Lopez-Barron, C.R.; Eberle, A.P.R.; Porcar, L.; Wagner, N.J. Spatiotemporal stress and structure evolution in dynamically sheared polymer-like micellar solutions. Soft Matter 2014, 10, 2889. [Google Scholar] [CrossRef] [PubMed]
- Muetze, A.; Heunemann, P.; Fischer, P. On the appearance of vorticity and gradient shear bands in wormlike micellar solutions of different CPCl/salt systems. J. Rheol. 2014, 58, 1647. [Google Scholar] [CrossRef]
- Calabrese, M.A.; Wagner, N.J.; Rogers, S.A. An optimized protocol for the analysis of time-resolved elastic scattering experiments. Soft Matter 2016, 12, 2301–2308. [Google Scholar] [CrossRef] [PubMed]
- Wiedenmann, A.; Keiderling, U.; Habicht, K.; Russina, M.; Gähler, R. Dynamics of field-induced ordering in magnetic colloids studied by new time-resolved small-angle neutron-scattering techniques. Phys. Rev. Lett. 2006, 97, 057202. [Google Scholar] [CrossRef] [PubMed]
- Kipping, D.; Gähler, R.; Habicht, K. Small angle neutron scattering at very high time resolution: Principle and simulations of ‘TISANE’. Phys. Lett. A 2008, 372, 1541–1546. [Google Scholar] [CrossRef]
- James, M.; Nelson, A.; Brule, A.; Schulz, J.C. Platypus: A time-of-flight neutron reflectometer at Australia’s new research reactor. J. Neutron Res. 2006, 14, 91–108. [Google Scholar] [CrossRef]
- James, M.; Nelson, A.; Holt, S.A.; Saerbeck, T.; Hamilton, W.A.; Klose, F. The multipurpose time-of-flight neutron reflectometer “Platypus” at Australia’s OPAL reactor. Nucl. Inst. Methods Phys. Res. A 2011, 632, 112–123. [Google Scholar] [CrossRef]
- Müller-Buschbaum, P. Grazing incidence small-angle neutron scattering: challenges and possibilities. Polym. J. 2013, 45, 34. [Google Scholar] [CrossRef]
- Wolff, M. Grazing incidence scattering in JDN 23 - French-Swedish Winterschool on Neutron Scattering: Applications to Soft Matter. EPJ Web Conf. 2018, 188, 04002. [Google Scholar] [CrossRef]
- Saerbeck, T.; Klose, F.; le Brun, A.P.; Fazi, J.; Brule, A.; Nelson, A.; Holt, S.A.; James, M. Polarization “down under”: The polarized time-of-flight neutron reflectometer PLATYPUS. Rev. Sci. Instrum. 2012, 83, 081301. [Google Scholar] [CrossRef] [PubMed]
- Wolff, M.; Kuhns, P.; Liesche, G.; Ankner, J.F.; Browning, J.; Gutfreund, P. Combined neutron reflectometry and rheology. J. Appl. Cryst. 2013, 46, 1729. [Google Scholar] [CrossRef]
- Mortensen, K.; Batsberg, W.; Hvidt, S. Effects of PEO-PPO Diblock Impurities on the Cubic Structure of Aqueous PEO-PPO-PEO Pluronics Micelles: Fcc and bcc Ordered Structures in F127. Macromolecules 2008, 41, 1720–1727. [Google Scholar] [CrossRef]
- Wolff, M.; Scholz, U.; Hock, R.; Magerl, A.; Zabel, H. Crystallisation of micelles at chemically terminated surfaces. Phys. Rev. Lett. 2004, 92, 255501. [Google Scholar] [CrossRef] [PubMed]
- Hu, F.; MacRenaris, K.W.; Waters, E.A.; Liang, T.; Schultz-Sikma, E.A.; Eckermann, A.L.; Meade, T.J. Ultrasmall, Water-Soluble Magnetite Nanoparticles with High Relaxivity for Magnetic Resonance Imaging. J. Phys. Chem. C 2009, 113, 20855. [Google Scholar] [CrossRef] [PubMed]
- ImageJ Userguide. Available online: http://rsbweb.nih.gov/ij/docs/user-guide.pdf (accessed on 6 March 2019).
- Saini, A.; Wolff, M. Macroscopic Alignment of Micellar Crystals with Magnetic Microshearing. Langmuir 2019. [Google Scholar] [CrossRef] [PubMed]
- Pozzo, D.C.; Walker, L.M. Shear Orientation of Nanoparticle Arrays Templated in a Thermoreversible Block Copolymer Micellar. Macromolecules 2007, 40, 5801–5811. [Google Scholar] [CrossRef]
- Guo, L.; Colby, R.H.; Lin, M.Y.; Dado, G.P. Micellar structure changes in aqueous mixtures of nonionic surfactants. J. Rheol. 2001, 45, 1223. [Google Scholar] [CrossRef]
- Wolff, M.; Magerl, A.; Zabel, H. Crystallization of soft crystals. Langmuir 2009, 25, 64. [Google Scholar] [CrossRef] [PubMed]
- Wolff, M.; Magerl, A.; Zabel, H. Structure of polymer micelles close to the solid interface. Eur. Phys. J. E 2005, 16, 141. [Google Scholar] [CrossRef]
- Wolff, M.; Steitz, R.; Gutfreund, P.; Voss, N.; Gerth, S.; Walz, M.; Magerl, A.; Zabel, H. Shear induced relaxation of polymer micelles at the solid-liquid interface. Langmuir 2008, 24, 11331. [Google Scholar] [CrossRef] [PubMed]
- Adlmann, F.A.; Busch, S.; Vacaliuc, B.; Nelson, A.; Ankner, J.F.; Browning, J.F.; Parizzi, A.; Bilheux, J.-K.; Halbert, C.E.; Korolkovas, A.; et al. Normalization of stroboscopic neutron scattering experiments. Nucl. Methods Phys. Res. B 2018, 434, 61–65. [Google Scholar] [CrossRef]
Sample Availability: From the authors. |





© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wolff, M.; Saini, A.; Simonne, D.; Adlmann, F.; Nelson, A. Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials. Polymers 2019, 11, 445. https://doi.org/10.3390/polym11030445
Wolff M, Saini A, Simonne D, Adlmann F, Nelson A. Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials. Polymers. 2019; 11(3):445. https://doi.org/10.3390/polym11030445
Chicago/Turabian StyleWolff, Maximilian, Apurve Saini, David Simonne, Franz Adlmann, and Andrew Nelson. 2019. "Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials" Polymers 11, no. 3: 445. https://doi.org/10.3390/polym11030445
APA StyleWolff, M., Saini, A., Simonne, D., Adlmann, F., & Nelson, A. (2019). Time Resolved Polarised Grazing Incidence Neutron Scattering from Composite Materials. Polymers, 11(3), 445. https://doi.org/10.3390/polym11030445