Application of MODFLOW with Boundary Conditions Analyses Based on Limited Available Observations: A Case Study of Birjand Plain in East Iran
Abstract
:1. Introduction
2. Materials and Methods
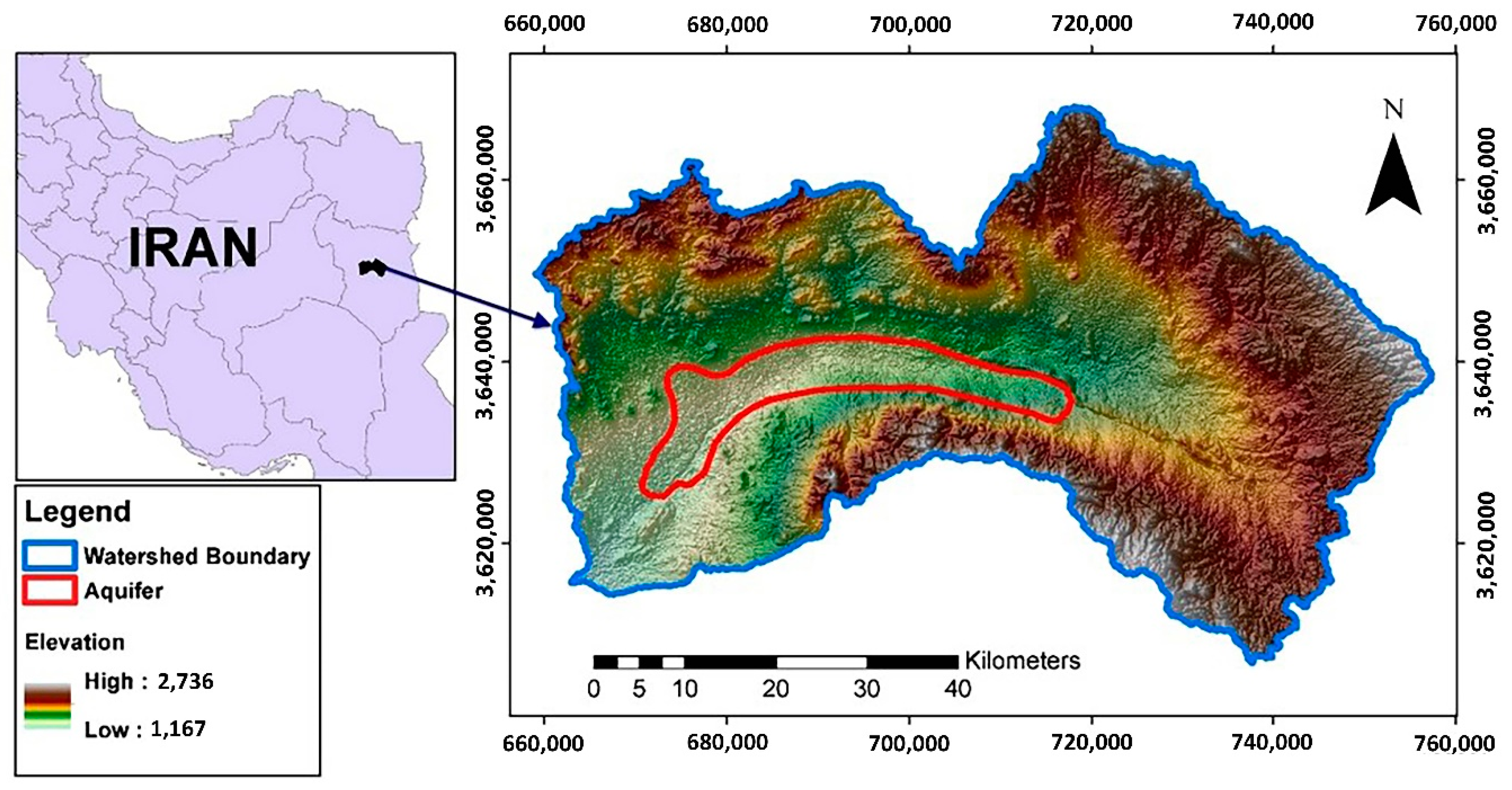
2.1. Study Area
2.2. Groundwater Modeling of Birjand Aquifer
Governing Equations
2.3. Groundwater Conceptual Model of Birjand Aquifer
- Lack of adequate knowledge and incomplete information about the physical properties of alluvial deposits of plain, which is the main reservoir of groundwater;
- Lack of adequate and accurate statistics and information on meteorological and climatic parameters and other parameters in the study area for estimating the water balance components;
- Lack of sufficient observation wells and other observations in the area;
- Lack of accurate and adequate statistics and criteria on the method and extent of utilization of Birjand groundwater resource;
- Lack of sufficient exploratory wells in the study plain to understand the physical and geometric characteristics of the aquifer;
- Error in piezometer recorded values;
- Lack of adequate pumping tests in the study area and therefore, lack of sufficient information on the hydrodynamic coefficients of the aquifer;
- Lack of sufficient understanding of the hydraulic behavior of an aquifer’s surrounding formations and their relationship with the aquifer, and the consequent lack of proper and precise definition of boundary conditions;
- Lack of sufficient information on the hydraulic connections between surface water (e.g., river or lake) and groundwater resources;
- Lack of sufficient information for calculating agricultural, urban, and industrial backwaters;
2.3.1. Boundary Conditions
- In the northeast part of the aquifer, there is an exchange of groundwater between Birjand and Marak aquifers (Figure 6). The groundwater flow direction in this area is from the Marak aquifer towards Birjand aquifer (the water levels are higher in the outlet of the Marak aquifer) with the flow rate of about 3.56 million cubic meters per year.
- The second input boundary area to the Birjand aquifer located in the south as shown in Figure 10. The southern parts of the Birjand aquifer have the highest elevations of the land structure in the adjacent areas of the Birjand aquifer. In addition, there are alluvial fans as unconsolidated sedimentary deposits in these parts which, due to having steep slopes, can recharge the aquifer during the precipitation.
- There is another lateral input boundary in the northwest of the aquifer, which looks like a camel hump. In this area, there is a large fan-shaped alluvial cone that has been washed out or eroded over the years from high altitudes and dispersed in a large area with a perimeter of about 17.5 km, as shown in Figure 11. Precipitation over this alluvial cone flows through specified paths and then penetrates into this vast area and joins the Birjand aquifer.
- Lithology, as well as soil type and soil texture investigations in the study region are identified the fourth input boundary. In Figure 6, the southern part of the aquifer and from the central side towards the west of the aquifer, geologically are formed from predominantly sandstone, siltstone, phyllite, slate, and minor limestone, which have very low permeability. As a result, due to the fact that water cannot penetrate rapidly, it becomes runoff and flows downstream, and penetrates as soon as it enters the aquifer’s alluvial zone, causing the aquifer recharge. The relatively high slope in this area, which speeds up the runoff from precipitation, as well as the presence of a large mountain above this rock that has average precipitation above the Birjand plain, are some factors that help to recharge the aquifer. The distance between the second and fourth major input areas (both of which are located in the southern part of the Birjand aquifer) mainly is not considered as an input boundary because there are relatively low elevations and slight/gentle slopes between these elevated areas in the southern part. In other words, in the area between these two inputs, the stone structure is far from the aquifer boundary. Due to aridity of this region (high evapotranspiration and small precipitation), the amount of water that penetrates in this area is not transported to the Birjand aquifer.
- There is another input boundary in the northern areas of the Birjand aquifer. There are two large alluvial fans in this area, with a tip distance of about 7.2 km (Figure 12) and at the base, these are located entirely within the aquifer boundary and their distance is reduced by about half. These alluvial fans build a place for penetrating the runoffs to the aquifer and recharging it. In the upstream part of the right (eastern) alluvial fan, there are some human activities, such as leveling the ground, constructing a small earth dam, and farming. Therefore, input flows from this side can be ignored and considered as a no-flow boundary. However, the upstream of the left alluvial fan (western) remains almost virtually undisturbed without any considerable human activities.
2.3.2. Model Parameters
2.3.3. Model Computational Grid
3. Results
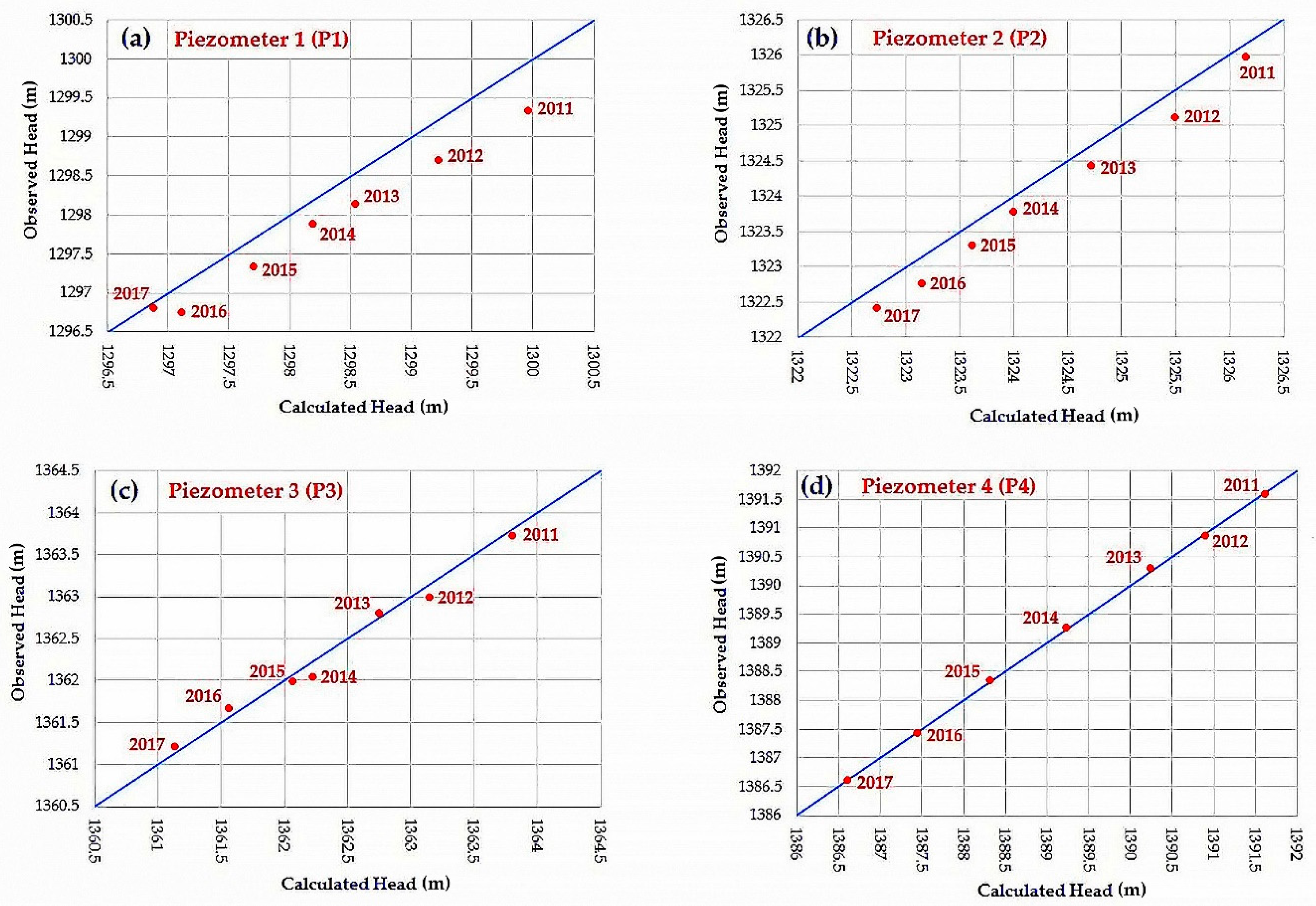
3.1. Model Calibration
3.2. Model Evaluation
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sattari, M.T.; Mirabbasi, R.; Sushab, R.S.; Abraham, J. Prediction of Level in Ardebil Plain Using Support Vector Regression and M5 Tree Model. Groundwater 2018, 56, 636–646. [Google Scholar] [CrossRef] [PubMed]
- Khalili, K.; Tahoudi, M.N.; Mirabbasi, R.; Ahmadi, F. Investigation of spatial and temporal variability of precipitation in Iran over the last half century. Stoch. Environ. Res. Risk Assess. 2016, 30, 1205–1221. [Google Scholar] [CrossRef]
- Rejani, R.; Jha, M.K.; Panda, S.N.; Mull, R. Simulation modeling for efficient groundwater management in balasore coastal basin, India. Water Resour. Manag. 2008, 22, 23–50. [Google Scholar] [CrossRef]
- van Engelenburg, J.; Hueting, R.; Rijpkema, S.; Teuling, A.J.; Uijlenhoet, R.; Ludwig, F. Impact of Changes in Groundwater Extractions and Climate Change on Groundwater-Dependent Ecosystems in a Complex Hydrogeological Setting. Water Resour. Manag. 2018, 32, 259–272. [Google Scholar] [CrossRef]
- Izady, A.; Abdalla, O.; Joodavi, A.; Chen, M. Groundwater Modeling and Sustainability of a Transboundary Hardrock–Alluvium Aquifer in North Oman Mountains. Water 2017, 9, 161. [Google Scholar] [CrossRef]
- Uddameri, V.; Singaraju, S.; Karim, A.; Gowda, P.; Bailey, R.; Schipanski, M. Understanding Climate-Hydrologic-Human Interactions to Guide Groundwater Model Development for Southern High Plains. J. Contemp. Water Res. Educ. 2017, 162, 79–99. [Google Scholar] [CrossRef] [Green Version]
- Khadri, S.F.R.; Pande, C. Ground water flow modeling for calibrating steady state using MODFLOW software: A case study of Mahesh River basin, India. Model. Earth Syst. Environ. 2016, 2, 39. [Google Scholar] [CrossRef]
- Reilly, T.E.; Harbaugh, A.W. Guidelines for Evaluating Ground-Water Flow Models; US Geological Survey, Scientific Investigations Report 2004–5038; USGS: Reston, VA, USA, 2004.
- Rapantova, N.; Tylcer, J.; Vojtek, D. Numerical modelling as a tool for optimisation of ground water exploitation in urban and industrial areas. Procedia Eng. 2017, 209, 92–99. [Google Scholar] [CrossRef]
- Hogeboom, R.H.J.; van Oel, P.R.; Krol, M.S.; Booij, M.J. Modelling the Influence of Groundwater Abstractions on the Water Level of Lake Naivasha, Kenya Under Data-Scarce Conditions. Water Resour. Manag. 2015, 29, 4447–4463. [Google Scholar] [CrossRef] [Green Version]
- Baalousha, H. Fundamentals of groundwater modelling. In Groundwater: Modelling, Management and Contamination; Konig, L.F., Weiss, J.L., Eds.; Nova Science Publishers, Inc.: New York, NY, USA, 2009; pp. 149–166. ISBN 9781604568325. [Google Scholar]
- McDonald, M.G.; Harbaugh, A.W. A Modular Three-Dimensional Finite-Difference Ground-Water Flow Model; U.S. Geological Survey, Open File Report 83–875; US Geological Survey: Reston, VA, USA, 1988.
- Panagopoulos, G. Application of MODFLOW for simulating groundwater flow in the Trifilia karst aquifer, Greece. Environ. Earth Sci. 2012, 67, 1877–1889. [Google Scholar] [CrossRef]
- Kushwaha, R.K.; Pandit, M.K.; Goyal, R. MODFLOW Based Groundwater Resource Evaluation and Prediction in Mendha Sub-Basin, NE Rajasthan. J. Geol. Soc. India 2009, 74, 449–458. [Google Scholar] [CrossRef]
- Wang, S.; Shao, J.; Song, X.; Zhang, Y.; Huo, Z.; Zhou, X. Application of MODFLOW and geographic information system to groundwater flow simulation in North China Plain, China. Environ. Geol. 2008, 55, 1449–1462. [Google Scholar] [CrossRef]
- Abdulla, F.A.; Al-Khatib, M.A.; Al-Ghazzawi, Z.D. Development of groundwater modeling for the Azraq Basin, Jordan. Environ. Geol. 2000, 40, 11–18. [Google Scholar] [CrossRef]
- Gurwin, J.; Lubczynski, M. Modeling of complex multi-aquifer systems for groundwater resources evaluation—Swidnica study case (Poland). Hydrogeol. J. 2005, 13, 627–639. [Google Scholar] [CrossRef]
- Lutz, A.; Thomas, J.M.; Pohll, G.; McKay, W.A. Groundwater resource sustainability in the Nabogo Basin of Ghana. J. Afr. Earth Sci. 2007, 49, 61–70. [Google Scholar] [CrossRef]
- Jingli, S.; Ling, L.; Yali, C.; Zhaoji, Z. Groundwater Flow Simulation and its Application in Groundwater Resource Evaluation in the North China Plain, China. Acta Geol. Sin. Engl. Ed. 2013, 87, 243–253. [Google Scholar] [CrossRef]
- Pisinaras, V.; Petalas, C.; Tsihrintzis, V.A.; Karatzas, G.P. Integrated modeling as a decision-aiding tool for groundwater management in a Mediterranean agricultural watershed. Hydrol. Process. 2013, 27, 1973–1987. [Google Scholar] [CrossRef]
- Katpatal, Y.B.; Pophare, A.M.; Lamsoge, B.R. A groundwater flow model for overexploited basaltic aquifer and Bazada formation in India. Environ. Earth Sci. 2014, 72, 4413–4425. [Google Scholar] [CrossRef]
- Chakraborty, S.; Maity, P.K.; Das, S. Investigation, simulation, identification and prediction of groundwater levels in coastal areas of Purba Midnapur, India, using MODFLOW. Environ. Dev. Sustain. 2019, 1–13. [Google Scholar] [CrossRef]
- Jang, C.S.; Chen, C.F.; Liang, C.P.; Chen, J.S. Combining groundwater quality analysis and a numerical flow simulation for spatially establishing utilization strategies for groundwater and surface water in the Pingtung Plain. J. Hydrol. 2016, 533, 541–556. [Google Scholar] [CrossRef]
- Bushira, K.M.; Hernandez, J.R.; Sheng, Z. Surface and groundwater flow modeling for calibrating steady state using MODFLOW in Colorado River Delta, Baja California, Mexico. Model. Earth Syst. Environ. 2017, 3, 815–824. [Google Scholar] [CrossRef]
- Habel, S.; Fletcher, C.H.; Rotzoll, K.; El-kadi, A.I. Development of a model to simulate groundwater inundation induced by sea-level rise and high tides in Honolulu, Hawaii. Water Res. 2017, 114, 122–134. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Huang, G.; Qu, Z.; Pereira, L.S. Using MODFLOW and GIS to Assess Changes in Groundwater Dynamics in Response to Water Yellow River Basin. Water Resour. Manag. 2011, 25, 2035–2059. [Google Scholar] [CrossRef]
- Lachaal, F.; Mlayah, A.; Bédir, M.; Tarhouni, J.; Leduc, C. Implementation of a 3-D groundwater flow model in a semi-arid region using MODFLOW and GIS tools: The Zéramdine–Béni Hassen Miocene aquifer system (east-central Tunisia). Comput. Geosci. 2012, 48, 187–198. [Google Scholar] [CrossRef]
- El-Zehairy, A.A.; Lubczynski, M.W.; Gurwin, J. Interactions of artificial lakes with groundwater applying an integrated MODFLOW solution. Hydrogeol. J. 2018, 26, 109–132. [Google Scholar] [CrossRef]
- Xue, S.; Liu, Y.; Liu, S.; Li, W.; Wu, Y.; Pei, Y. Numerical simulation for groundwater distribution after mining in Zhuanlongwan mining area based on visual MODFLOW. Environ. Earth Sci. 2018, 77, 400. [Google Scholar] [CrossRef]
- Chatterjee, R.; Jain, A.K.; Chandra, S.; Tomar, V.; Parchure, P.K.; Ahmed, S. Mapping and management of aquifers suffering from over-exploitation of groundwater resources in Baswa-Bandikui watershed, Rajasthan, India. Environ. Earth Sci. 2018, 77, 157. [Google Scholar] [CrossRef]
- United States Geological Survey (USGS). MODFLOW and Related Programs. Available online: https://www.usgs.gov/mission-areas/water-resources/science/modflow-and-related-programs?qt-science_center_objects=0#qt-science_center_objects (accessed on 14 March 2019).
- Iran Water Resources Management Company. Available online: https://www.wrm.ir (accessed on 23 April 2019).
- Regional Water Company of South Khorasan. The National Program on Controlling the Groundwater Exploitation; Regional Water Company of South Khorasan: Birjand, Iran, 2019. [Google Scholar]
- Noor, H. Analysis of Groundwater Resource Utilization and Their Current Condition in Iran. Iran. J. Rainwater Catchment Syst. 2017, 5, 29–38. (In Persian) [Google Scholar]
- Sadeghi-Tabas, S.; Akbarpour, A.; Pourreza-Bilondi, M.; Samadi, S. Toward reliable calibration of aquifer hydrodynamic parameters: Characterizing and optimization of arid groundwater system using swarm intelligence optimization algorithm. Arab. J. Geosci. 2016, 9, 719. [Google Scholar] [CrossRef]
- Javan, J.; Fal-Soleiman, M. Water Crisis and Importance of Water Productivity in Agriculture in Dry Area of Iran (Case Study: Birjand Plain). Geogr. Dev. Iran. J. 2008, 6, 115–138. (In Persian) [Google Scholar]
- Sherif, M.M.; Singh, V.P. Effect of climate change on sea water intrusion in coastal aquifers. Hydrol. Process. 1999, 13, 1277–1287. [Google Scholar] [CrossRef]
- Waterloo Hydrogeologic Company. Available online: https://www.waterloohydrogeologic.com/resources/visual-modflow-flex-resources/introduction-groundwater-modeling-with-visual-modflow (accessed on 2 March 2019).
- Diersch, H.-J.G. FEFLOW: Finite Element Modeling of Flow, Mass and Heat Transport in Porous and Fractured Media; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]
- Owen, S.J.; Jones, N.L.; Holland, J.P. A Comprehensive Modeling Environment for the Simulation of Groundwater Flow and Transport. Eng. Comput. 1996, 12, 235–242. [Google Scholar] [CrossRef]
- Karimi, L.; Motagh, M.; Entezam, I. Modeling groundwater level fluctuations in Tehran aquifer: Results from a 3D unconfined aquifer model. Groundw. Sustain. Dev. 2019, 8, 439–449. [Google Scholar] [CrossRef]
- Nan, T.; Li, K.; Wu, J.; Yin, L. Assessment of groundwater exploitation in an aquifer using the random walk on grid method: A case study at Ordos, China. Hydrogeol. J. 2018, 26, 1669–1681. [Google Scholar] [CrossRef]
- Su, X.; Yuan, W.; Du, S.; Cui, G.; Bai, J.; Du, S. Responses of groundwater vulnerability to groundwater extraction reduction in the Hun River Basin, northeastern China. Hum. Ecol. Risk Assess. 2017, 23, 1121–1139. [Google Scholar] [CrossRef]
- Meredith, E.; Blais, N. Quantifying irrigation recharge sources using groundwater modeling. Agric. Water Manag. 2019, 214, 9–16. [Google Scholar] [CrossRef]
- Qiu, S.; Liang, X.; Xiao, C.; Huang, H.; Fang, Z.; Lv, F. Numerical simulation of groundwater flow in a River Valley Basin in Jilin Urban Area, China. Water 2015, 7, 5768–5787. [Google Scholar] [CrossRef]
- Roy, P.K.; Roy, S.S.; Giri, A.; Banerjee, G.; Majumder, A.; Mazumdar, A. Study of impact on surface water and groundwater around flow fields due to changes in river stage using groundwater modeling system. Clean Technol. Environ. Policy 2015, 17, 145–154. [Google Scholar] [CrossRef]
- Sobeih, M.M.; El-Arabi, N.E.; Helal, E.E.D.Y.; Awad, B.S. Management of water resources to control groundwater levels in the southern area of the western Nile delta, Egypt. Water Sci. 2017, 31, 137–150. [Google Scholar] [CrossRef] [Green Version]
- Moridi, A.; MajdzadehTabatabaie, M.R.; Esmaeelzade, S. Holistic Approach to Sustainable Groundwater Management in Semi- arid Regions. Int. J. Environ. Res. 2018, 12, 347–355. [Google Scholar] [CrossRef]
- Hashemi, H.; Berndtsson, R.; Kompani-Zare, M. Steady-State Unconfined Aquifer Simulation of the Gareh-Bygone Plain, Iran. Open Hydrol. J. 2012, 6, 58–67. [Google Scholar] [CrossRef]
- Malekinezhad, H.; Banadkooki, F.B. Modeling impacts of climate change and human activities on groundwater resources using MODFLOW. J. Water Clim. Chang. 2017, 9, 156–177. [Google Scholar] [CrossRef]
- Jalut, Q.H.; Abbas, N.L.; Mohammad, A.T. Management of groundwater resources in the Al-Mansourieh zone in the Diyala River Basin in Eastern Iraq. Groundw. Sustain. Dev. 2018, 6, 79–86. [Google Scholar] [CrossRef]
- He, X.; Sonnenborg, T.O.; Jørgensen, F.; Høyer, A.-S.; Møller, R.R.; Jensen, K.H. Analyzing the effects of geological and parameter uncertainty on prediction of groundwater head and travel time. Hydrol. Earth Syst. Sci. 2013, 17, 3245–3260. [Google Scholar] [CrossRef] [Green Version]
- Dupuit, J. Études Théoriques et Pratiques sur le Mouvement des Eaux Dans les Canaux Découverts et à Travers les Terrains Perméabls, 2nd ed.; Dunod: Paris, France, 1863. [Google Scholar]
- Pholkern, K.; Saraphirom, P.; Cloutier, V.; Srisuk, K. Use of Alternative Hydrogeological Conceptual Models to Assess the Potential Impact of Climate Change on Groundwater Sustainable Yield in Central Huai Luang Basin, Northeast Thailand. Water 2019, 11, 241. [Google Scholar] [CrossRef]
- Poeter, E.; Anderson, D. Multimodel Ranking and Inference in Ground Water Modeling. Ground Water 2005, 43, 597–605. [Google Scholar] [CrossRef]
- Hamraz, B.S.; Akbarpour, A.; Bilondi, M.P.; Tabas, S.S. On the assessment of ground water parameter uncertainty over an arid aquifer. Arab. J. Geosci. 2015, 8, 10759–10773. [Google Scholar] [CrossRef]
- Sadeghi-Tabas, S.; Samadi, S.Z.; Akbarpour, A.; Pourreza-Bilondi, M. Sustainable groundwater modeling using single- and multi-objective optimization algorithms. J. Hydroinform. 2017, 19, 97–114. [Google Scholar] [CrossRef]
- Kalantari, M.; Akbarpour, A.; Khatibinia, M. Numerical Modeling of Groundwater Flow in Unconfined Aquifer in Steady State with Isogeometric Method. Modares Civ. Eng. J. 2018, 18, 195–206. (In Persian) [Google Scholar]
- Hassanpour, M.; Khozeymehnezhad, H. Placement of nutrient wells for artificial nutrition and improvement of aquifer quality in Birjand plain using treated wastewater. Health 2018, 4, 215–226. (In Persian) [Google Scholar]
- Mohtashami, A.; Akbarpour, A.; Mollazadeh, M. Development of two-dimensional groundwater flow simulation model using meshless method based on MLS approximation function in unconfined aquifer in transient state. J. Hydroinform. 2017, 19, 640–652. [Google Scholar] [CrossRef] [Green Version]
- Roozbahani, A.; Ebrahimi, E.; Banihabib, M.E. A Framework for Ground Water Management Based on Bayesian Network and MCDM Techniques. Water Resour. Manag. 2018, 32, 4985–5005. [Google Scholar] [CrossRef]
- Kardan Moghadam, H.; Banihabib, M.E.; Javadi, S. Quantitative Sustainability Analysis of Aquifer System (Case Study: South Khorasan—Birjand Aquifer). J. Water Soil 2018, 31, 1587–1601. (In Persian) [Google Scholar]
- Batu, V. Numerical Flow and Solute Transport Modeling in Aquifers. In Applied Flow and Solute Transport Modeling in Aquifers: Fundamental Principles and Analytical and Numerical Methods; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2006; pp. 215–344. ISBN 9780849335747. [Google Scholar]
- Regional Water Company of South Khorasan. Quantifying the Water Resources in Birjand Region, Report Version 3; Regional Water Company of South Khorasan: Birjand, Iran, 2017. [Google Scholar]
- Anderson, M.P.; Woessner, W.W.; Hunt, R.J. APPLIED GROUNDWATER MODELING: Simulation of Flow and Advective Transport, 2nd ed.; Academic Press, Elsevier: New York, NY, USA, 2015; ISBN 9780120581030. [Google Scholar]
- GMS 10.4 Tutorial: MODFLOW—Automated Parameter Estimation; Aquaveo: Provo, Utah, UT, USA, 2018.
- XMS Wiki: Online Help for GMS, SMS, and WMS. Available online: https://www.xmswiki.com/wiki/GMS:Parameters#Parameterizing_the_model (accessed on 25 April 2019).
- Thangarajan, M. Groundwater Flow and Mass Transport. Modeling: Theory and Application; Capital Publishing Company: New Delhi, India, 2007; ISBN 9788185589619. [Google Scholar]
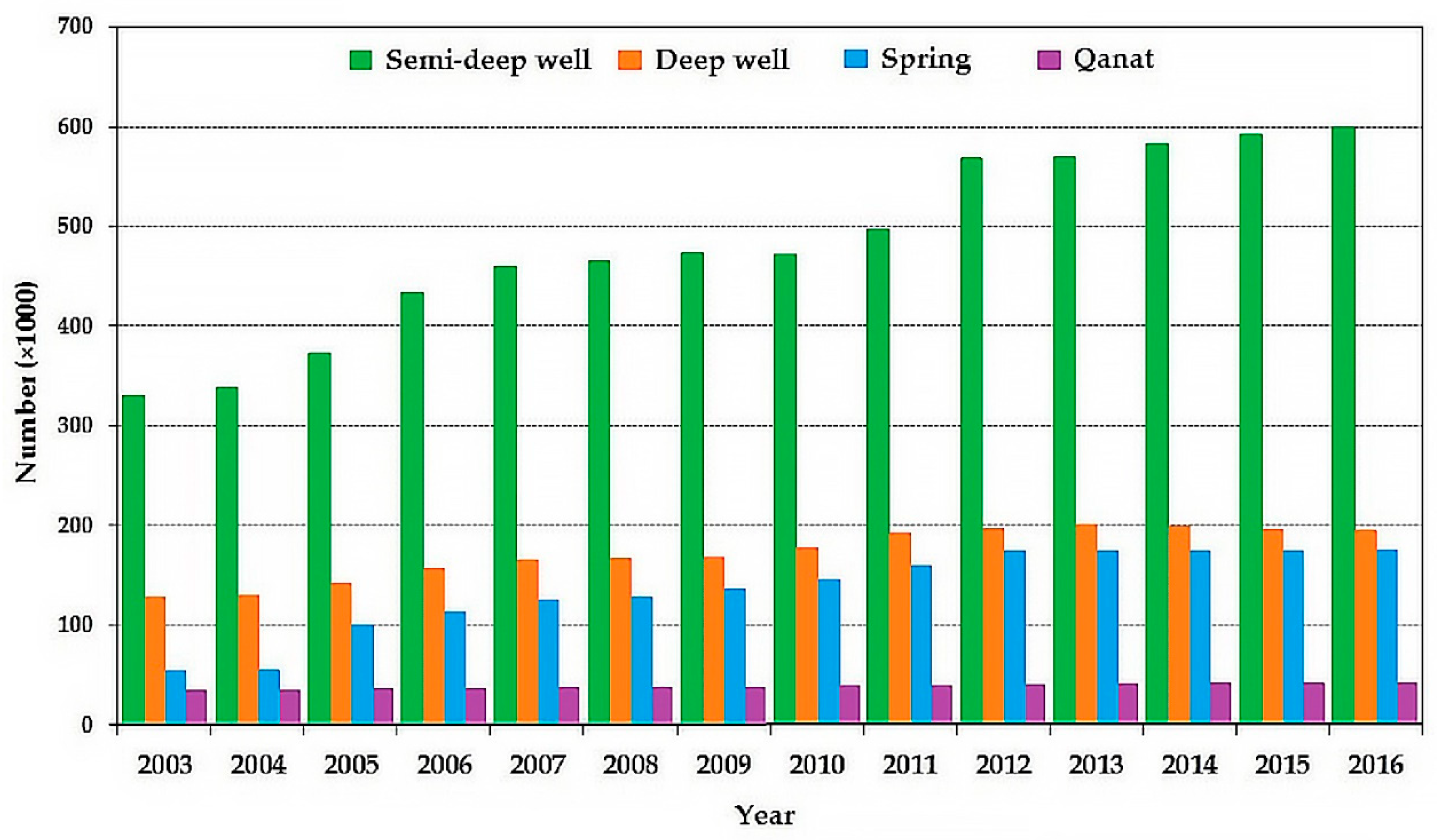
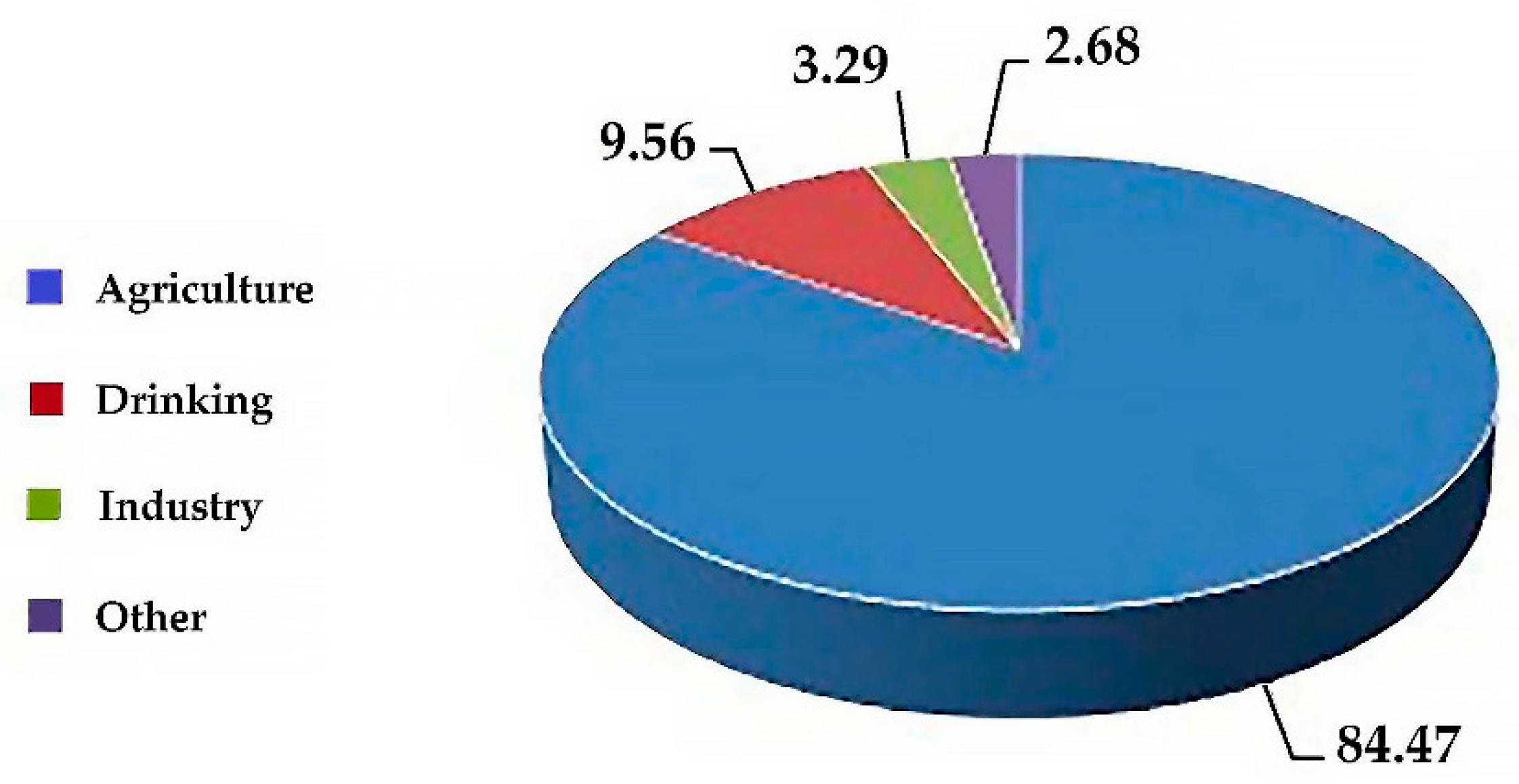
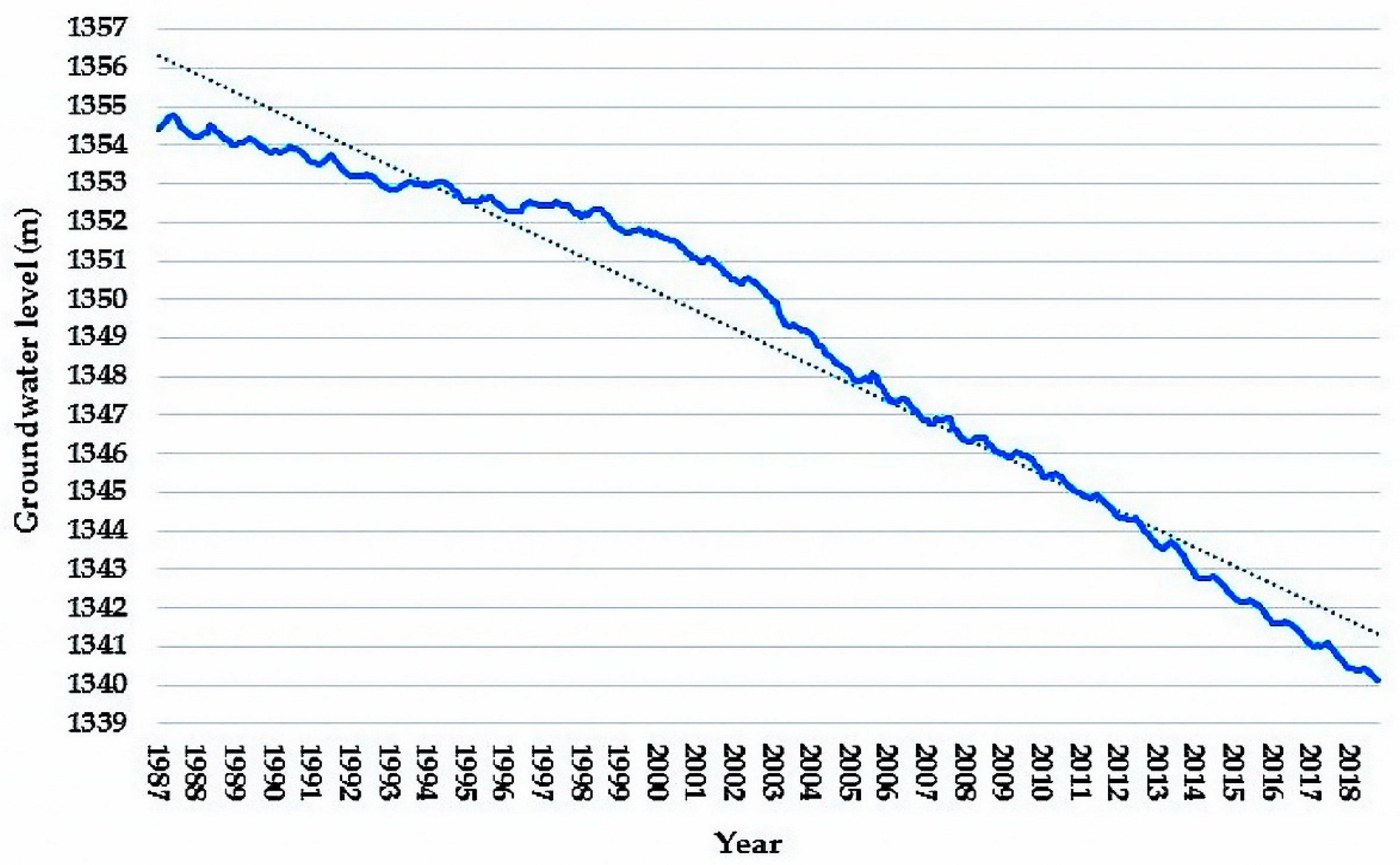




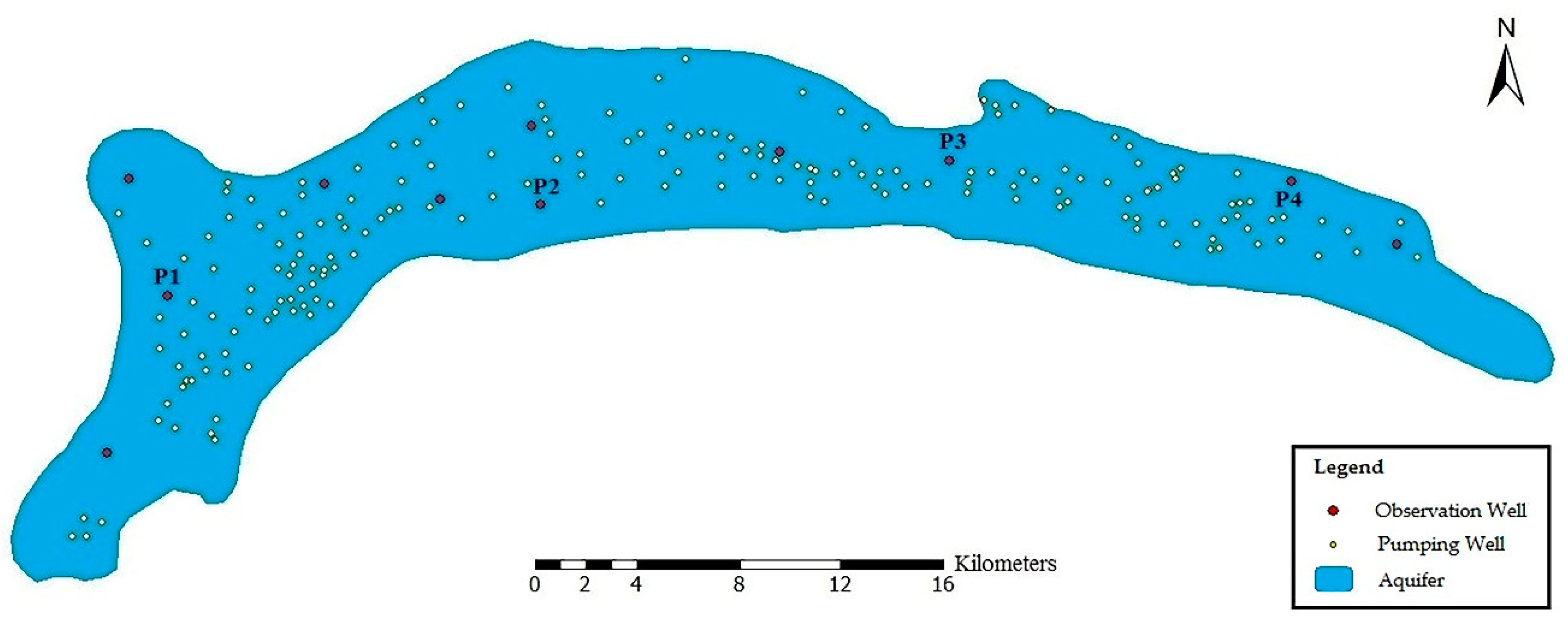




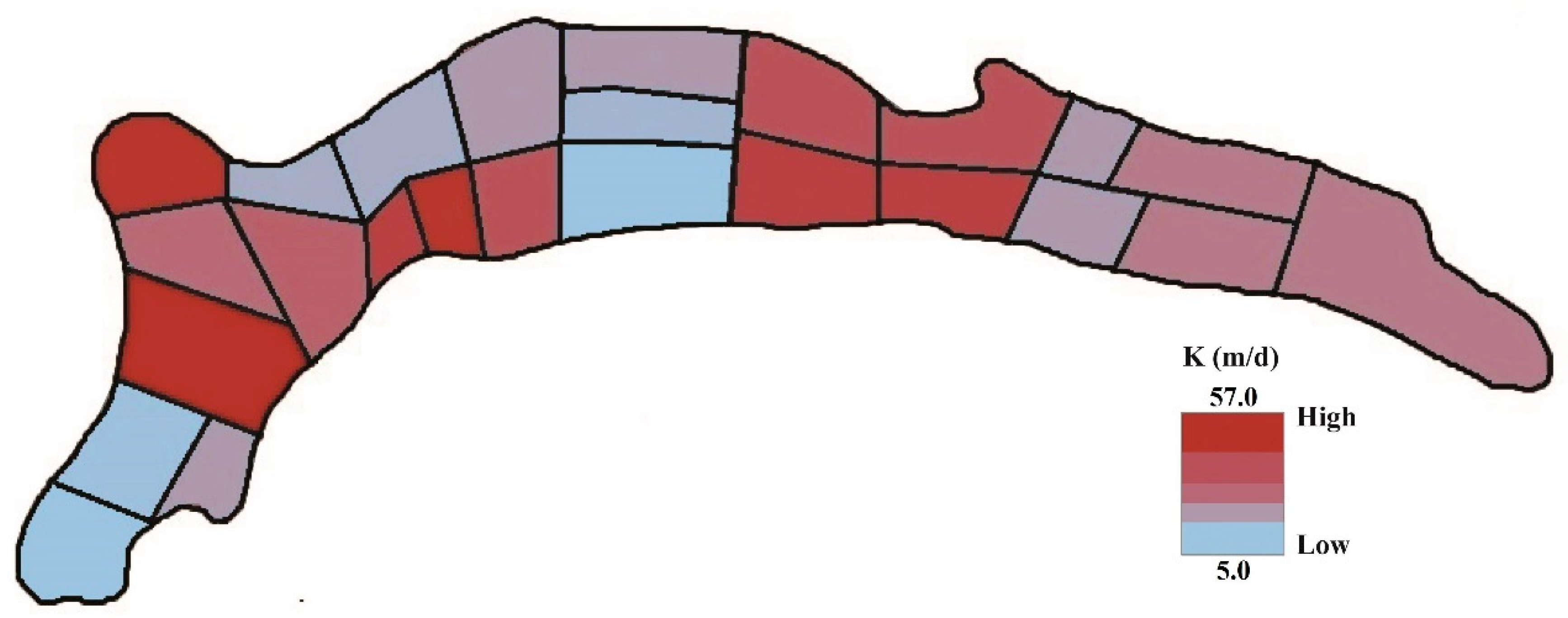
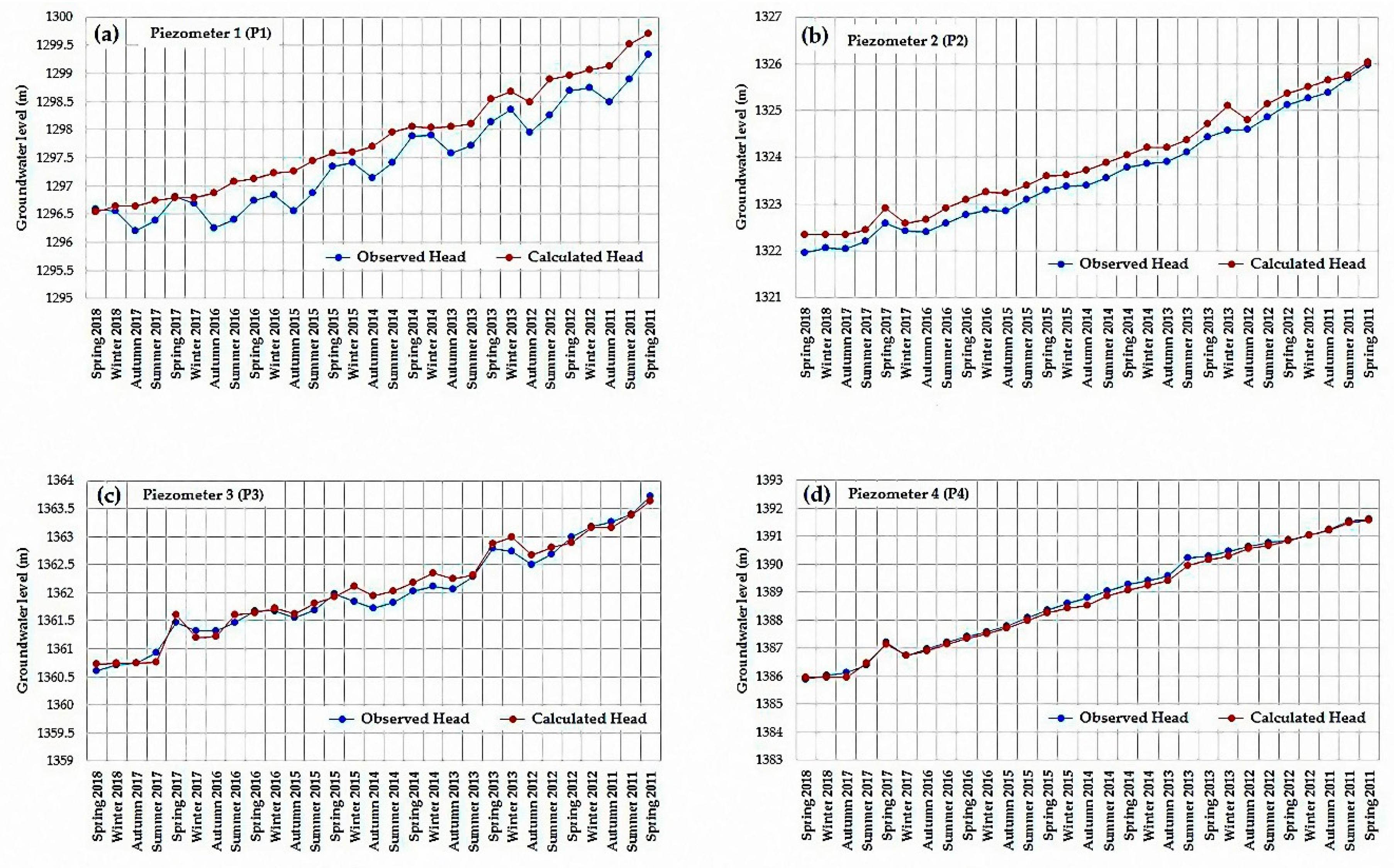
| Inputs (MCM/Year) | Outputs (MCM/Year) | ||
|---|---|---|---|
| Lateral underground inflow | 25.44 | Discharge and extraction (well, qanat, spring) | 73.56 |
| Infiltration of precipitation | 4.08 | Lateral underground outflow | 1.15 |
| Infiltration of runoff | 4.47 | Drainage | 0.00 |
| Infiltration of agricultural wastewater | 17.87 | evapotranspiration | 0.00 |
| Infiltration of drinking and industrial wastewaters | 14.32 | The total volume of discharge | 74.71 |
| The total volume of recharge | 66.18 | ||
| Season | Mean Error (m) | Mean Absolute Error (m) | Root Mean Square Error (m) |
|---|---|---|---|
| Spring 2018 | −0.04 | 0.14 | 0.18 |
| Winter 2018 | 0.03 | 0.14 | 0.19 |
| Autumn 2017 | 0.00 | 0.16 | 0.22 |
| Summer 2017 | 0.03 | 0.17 | 0.20 |
| Spring 2017 | 0.13 | 0.17 | 0.23 |
| Winter 2017 | 0.14 | 0.19 | 0.25 |
| Autumn 2016 | 0.05 | 0.22 | 0.28 |
| Summer 2016 | 0.02 | 0.26 | 0.30 |
| Spring 2016 | 0.09 | 0.22 | 0.27 |
| Winter 2016 | 0.09 | 0.23 | 0.27 |
| Autumn 2015 | 0.04 | 0.27 | 0.33 |
| Summer 2015 | 0.05 | 0.28 | 0.32 |
| Spring 2015 | 0.16 | 0.26 | 0.35 |
| Winter 2015 | 0.15 | 0.27 | 0.31 |
| Autumn 2014 | 0.05 | 0.27 | 0.30 |
| Summer 2014 | 0.03 | 0.27 | 0.30 |
| Spring 2014 | 0.14 | 0.26 | 0.28 |
| Winter 2014 | 0.12 | 0.25 | 0.27 |
| Autumn 2013 | 0.04 | 0.27 | 0.30 |
| Summer 2013 | 0.13 | 0.28 | 0.32 |
| Spring 2013 | 0.08 | 0.25 | 0.27 |
| Winter 2013 | 0.03 | 0.27 | 0.30 |
| Autumn 2012 | 0.01 | 0.24 | 0.31 |
| Summer 2012 | −0.01 | 0.25 | 0.31 |
| Spring 2012 | 0.14 | 0.23 | 0.30 |
| Winter 2012 | 0.14 | 0.24 | 0.31 |
| Autumn 2011 | 0.06 | 0.24 | 0.33 |
| Summer 2011 | 0.06 | 0.25 | 0.33 |
| Spring 2011 | 0.13 | 0.23 | 0.31 |
| Season | Mean Error (m) | Mean Absolute Error (m) | Root Mean Square Error (m) |
|---|---|---|---|
| Spring 2017 | 0.06 | 0.13 | 0.20 |
| Spring 2016 | 0.05 | 0.19 | 0.24 |
| Spring 2015 | 0.06 | 0.20 | 0.27 |
| Spring 2014 | 0.05 | 0.18 | 0.23 |
| Spring 2013 | 0.03 | 0.19 | 0.24 |
| Spring 2012 | −0.05 | 0.22 | 0.28 |
| Spring 2011 | −0.03 | 0.22 | 0.30 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aghlmand, R.; Abbasi, A. Application of MODFLOW with Boundary Conditions Analyses Based on Limited Available Observations: A Case Study of Birjand Plain in East Iran. Water 2019, 11, 1904. https://doi.org/10.3390/w11091904
Aghlmand R, Abbasi A. Application of MODFLOW with Boundary Conditions Analyses Based on Limited Available Observations: A Case Study of Birjand Plain in East Iran. Water. 2019; 11(9):1904. https://doi.org/10.3390/w11091904
Chicago/Turabian StyleAghlmand, Reza, and Ali Abbasi. 2019. "Application of MODFLOW with Boundary Conditions Analyses Based on Limited Available Observations: A Case Study of Birjand Plain in East Iran" Water 11, no. 9: 1904. https://doi.org/10.3390/w11091904
APA StyleAghlmand, R., & Abbasi, A. (2019). Application of MODFLOW with Boundary Conditions Analyses Based on Limited Available Observations: A Case Study of Birjand Plain in East Iran. Water, 11(9), 1904. https://doi.org/10.3390/w11091904





