The Fourth Fundamental Form IV of Dini-Type Helicoidal Hypersurface in the Four Dimensional Euclidean Space
Abstract
:1. Introduction
2. Preliminaries
3. Helicoidal Hypersurface
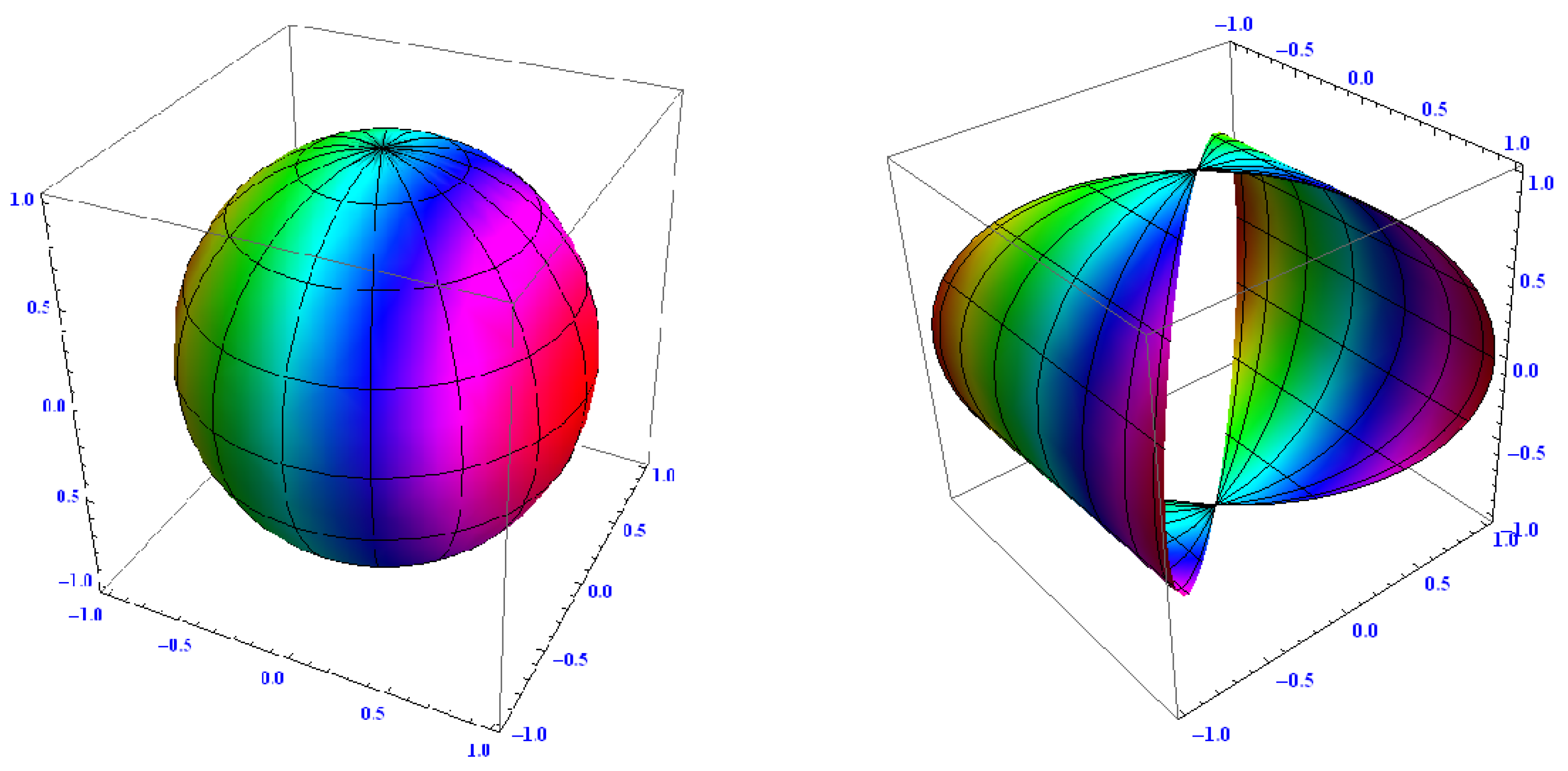
4. Dini-Type Helicoidal Hypersurface and the Fourth Fundamental Form
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Euler, L. Methodus Inveniendi Lineas Curvas Maximi Minimive Propietate Gaudeates Sive Solutio Problematis Isoperimetrici Latissimo Sensu Accepti; Opera Omnia Citation: Series 1 Original Source Citation; Apud Marcum-Michaelem Bousquet & Socios: Genevæ, Switzerland, 1744; Volume 24, pp. 1–322. [Google Scholar]
- Meusnier, J.B. Memoire sur la courbure des surfaces. Mem. Mathem. Phys. Acad. Sci. Paris, Pres. par Div. Savans 1785, 10, 477–510, Presented in 1776. [Google Scholar]
- Bour, E. Théorie de la déformation des surfaces. J. l.Êcole Imp. Polytech. 1862, 22, 1–148. [Google Scholar]
- Dini, U. Sopra le funzioni di una variabile complessa. Ann. Di Matematica Pura Appl. 1871, 4, 159–174. [Google Scholar] [CrossRef]
- Moore, C. Surfaces of rotation in a space of four dimensions. Ann. Math. 1919, 21, 81–93. [Google Scholar] [CrossRef]
- Moore, C. Rotation surfaces of constant curvature in space of four dimensions. Bull. Amer. Math. Soc. 1920, 26, 454–460. [Google Scholar] [CrossRef] [Green Version]
- Do Carmo, M.; Dajczer, M. Helicoidal surfaces with constant mean curvature. Tohoku Math. J. 1982, 34, 351–367. [Google Scholar] [CrossRef]
- Chen, B.Y. Total Mean Curvature and Submanifolds of Finite Type; World Scientific: Singapore, 1984. [Google Scholar]
- Hano, J.; Nomizu, K. Surfaces of revolution with constant mean curvature. Tohoku Math. J. 1984, 36, 427–437. [Google Scholar] [CrossRef]
- Roussos, I.M. The helicoidal surfaces as Bonnet surfaces. Tohoku Math. J. 1988, 40, 485–490. [Google Scholar] [CrossRef]
- Ripoll, J.B. Helicoidal minimal surfaces in hyperbolic space. Nagoya Math. J. 1989, 114, 65–75. [Google Scholar] [CrossRef] [Green Version]
- Dillen, F. Ruled submanifolds of finite type. Proc. Am. Math. Soc. 1992, 114, 795–798. [Google Scholar] [CrossRef]
- Baikoussis, C.; Verstraelen, L. On the Gauss map of helicoidal surfaces. Rend. Sem. Mat. Messina Ser. II 1993, 2, 31–42. [Google Scholar]
- Hoffman, D.; Wei, F.S.; Karcher, H. Adding handles to the helicoid. Bull. Amer. Math. Soc. 1993, 29, 77–84. [Google Scholar] [CrossRef] [Green Version]
- Gray, A. Modern Differential Geometry of Curves and Surfaces with Mathematica, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1997; pp. 493–495. [Google Scholar]
- Baikoussis, C.; Koufogiorgos, T. Helicoidal surfaces with prescribed mean or Gaussian curvature. J. Geom. 1998, 63, 25–29. [Google Scholar] [CrossRef]
- Dillen, F.; Kühnel, W. Ruled Weingarten surfaces in Minkowski 3-space. Manuscripta Math. 1999, 98, 307–320. [Google Scholar] [CrossRef]
- Ikawa, T. Bour’s theorem and Gauss map. Yokohama Math. J. 2000, 48, 173–180. [Google Scholar]
- Sasahara, N. Spacelike helicoidal surfaces with constant mean curvature in Minkowski 3-space. Tokyo J. Math. 2000, 23, 477–502. [Google Scholar] [CrossRef]
- Ikawa, T. Bour’s theorem in Minkowski geometry. Tokyo J. Math. 2001, 24, 377–394. [Google Scholar] [CrossRef]
- Choi, M.; Kim, Y.H. Characterization of the helicoid as ruled surfaces with pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2001, 38, 753–761. [Google Scholar]
- Yoon, D.W. Rotation surfaces with finite type Gauss map in E4. Indian J. Pure Appl. Math. 2001, 32, 1803–1808. [Google Scholar]
- Beneki, C.C.; Kaimakamis, G.; Papantoniou, B.J. Helicoidal surfaces in three-dimensional Minkowski space. J. Math. Anal. Appl. 2002, 275, 586–614. [Google Scholar] [CrossRef] [Green Version]
- Güler, E.; Vanlı, A. Bour’s theorem in Minkowski 3-space. J. Math. Kyoto 2006, 46, 47–63. [Google Scholar] [CrossRef]
- Güler, E.; Vanlı, A. On the mean, Gauss, the second Gaussian and the second mean curvature of the helicoidal surfaces with light-like axis in . Tsukuba J. Math. 2008, 32, 49–65. [Google Scholar] [CrossRef]
- Stamatakis, S.; Al-Zoubi, H. Surfaces of revolution satisfying ΔIIIx = Ax. J. Geom. Graph. 2010, 14, 181–186. [Google Scholar]
- Ji, F.; Kim, Y.H. Helicoidal CDPC-surfaces in Minkowski 3-space. Int. J. Geom. Methods Mod. Phys. 2010, 7, 979–988. [Google Scholar] [CrossRef]
- Ji, F.; Kim, Y.H. Mean curvatures and Gauss maps of a pair of isometric helicoidal and rotation surfaces in Minkowski 3-space. J. Math. Anal. Appl. 2010, 368, 623–635. [Google Scholar] [CrossRef] [Green Version]
- Güler, E.; Yaylı, Y.; Hacısalihoğlu, H.H. Bour’s theorem on the Gauss map in 3-Euclidean space. Hacet. J. Math. Stat. 2010, 39, 515–525. [Google Scholar]
- Arslan, K.; Kılıç Bayram, B.; Bulca, B.; Kim, Y.H.; Murathan, C.; Öztürk, G. Rotational embeddings in E4 with pointwise 1-type Gauss map. Turk. J. Math. 2011, 35, 493–499. [Google Scholar]
- Dursun, U.; Turgay, N.C. General rotational surfaces in Euclidean space E4 with pointwise 1-type Gauss map. Math. Commun. 2012, 17, 71–81. [Google Scholar]
- Arslan, K.; Bayram, B.; Bulca, B.; Öztürk, G. Generalized rotation surfaces in E4. Results Math. 2012, 61, 315–327. [Google Scholar] [CrossRef]
- Dursun, U.; Turgay, N.C. Minimal and pseudo-umbilical rotational surfaces in Euclidean space E4. Mediterr. J. Math. 2013, 10, 497–506. [Google Scholar] [CrossRef]
- Perdomo, O.M. Helicoidal minimal surfaces in R3. Illinois J. Math. 2013, 57, 87–104. [Google Scholar] [CrossRef]
- Ji, F.; Kim, Y.H. Isometries between minimal helicoidal surfaces and rotation surfaces in Minkowski space. Appl. Math. Comput. 2013, 220, 1–11. [Google Scholar] [CrossRef]
- Kim, Y.H.; Turgay, N.C. Surfaces in E4 with L1-pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2013, 50, 935–949. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.H.; Turgay, N.C. Classifications of helicoidal surfaces with L1-pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2013, 50, 1345–1356. [Google Scholar] [CrossRef] [Green Version]
- Güler, E. A new kind of helicoidal surface of value m. Int. Electron. J. Geom. 2014, 7, 154–162. [Google Scholar] [CrossRef]
- López, R.; Demir, E. Helicoidal surfaces in Minkowski space with constant mean curvature and constant Gauss curvature. Cent. Eur. J. Math. 2014, 12, 1349–1361. [Google Scholar] [CrossRef] [Green Version]
- Arslan, K.; Bulca, B.; Milousheva, V. Meridian surfaces in E4 with pointwise 1-type Gauss map. Bull. Korean Math. Soc. 2014, 51, 911–922. [Google Scholar] [CrossRef] [Green Version]
- Ganchev, G.; Milousheva, V. General rotational surfaces in the 4-dimensional Minkowski space. Turk. J. Math. 2014, 38, 883–895. [Google Scholar] [CrossRef] [Green Version]
- Babaarslan, M.; Yaylı, Y. Space-like loxodromes on rotational surfaces in Minkowski 3-space. J. Math. Anal. Appl. 2014, 409, 288–298. [Google Scholar] [CrossRef]
- Güler, E.; Yaylı, Y. Generalized Bour’s theorem. Kuwait J. Sci. 2015, 42, 79–90. [Google Scholar]
- Senoussi, B.; Bekkar, M. Helicoidal surfaces with ΔJr=Ar in 3-dimensional Euclidean space. Stud. Univ. Babeş-Bolyai Math. 2015, 60, 437–448. [Google Scholar]
- Hoffman, D.; Traizet, M.; White, B. Helicoidal minimal surfaces of prescribed genus. Acta Math. 2016, 216, 217–323. [Google Scholar] [CrossRef]
- Kim, D.S.; Kim, J.R.; Kim, Y.H. Cheng-Yau operator and Gauss map of surfaces of revolution. Bull. Malays. Math. Sci. Soc. 2016, 39, 1319–1327. [Google Scholar] [CrossRef] [Green Version]
- Güler, E.; Magid, M.; Yaylı, Y. Laplace Beltrami operator of a helicoidal hypersurface in four space. J. Geom. Symmetry Phys. 2016, 41, 77–95. [Google Scholar] [CrossRef] [Green Version]
- Hieu, D.T.; Thang, N.N. Bour’s theorem in 4-dimensional Euclidean space. Bull. Korean Math. Soc. 2017, 54, 2081–2089. [Google Scholar]
- Arslan, K.; Bulca, B.; Kosova, D. On generalized rotational surfaces in Euclidean spaces. J. Korean Math. Soc. 2017, 54, 999–1013. [Google Scholar] [CrossRef] [Green Version]
- Babaarslan, M.; Kayacık, M. Time-like loxodromes on helicoidal surfaces in Minkowski 3-space. Filomat 2017, 31, 4405–4414. [Google Scholar] [CrossRef]
- Güler, E.; Hacısalihoğlu, H.H.; Kim, Y.H. The Gauss map and the third Laplace-Beltrami operator of the rotational hypersurface in 4-space. Symmetry 2018, 10, 398. [Google Scholar] [CrossRef] [Green Version]
- Aleksieva, Y.; Milousheva, V.; Turgay, N.C. General rotational surfaces in pseudo-Euclidean 4-space with neutral metric. Bull. Malays. Math. Sci. Soc. 2018, 41, 1773–1793. [Google Scholar] [CrossRef] [Green Version]
- Goemans, W. Flat double rotational surfaces in Euclidean and Lorentz-Minkowski 4-space. Publ. Inst. Math. 2018, 103, 61–68. [Google Scholar] [CrossRef]
- Güler, E.; Turgay, N.C. Cheng-Yau operator and Gauss map of rotational hypersurfaces in 4-space. Mediterr. J. Math. 2019, 16, 1–16. [Google Scholar] [CrossRef]
- Güler, E.; Kişi, Ö. Dini-type helicoidal hypersurfaces with timelike axis in Minkowski 4-space . Mathematics 2019, 7, 205. [Google Scholar] [CrossRef] [Green Version]
- Yoon, D.W.; Lee, J.W.; Lee, C.W. Constructions of helicoidal surfaces by using curvature functions in isotropic space. Hacet. J. Math. Stat. 2019, 48, 959–965. [Google Scholar] [CrossRef]
- Güler, E. Helical hypersurfaces in Minkowski geometry . Symmetry 2020, 12, 1206. [Google Scholar] [CrossRef]
- Dursun, U. Rotational Weingarten surfaces in hyperbolic 3-space. J. Geom. 2020, 111, 1–12. [Google Scholar] [CrossRef]
- Güler, E. Fundamental form IV and curvature formulas of the hypersphere. Malaya J. Mat. 2020, 8, 2008–2011. [Google Scholar] [CrossRef]
- López, R.; Pámpano, Á. Classification of rotational surfaces with constant skew curvature in 3-space forms. J. Math. Anal. Appl. 2020, 489, 1–19. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Güler, E. The Fourth Fundamental Form IV of Dini-Type Helicoidal Hypersurface in the Four Dimensional Euclidean Space. Axioms 2021, 10, 186. https://doi.org/10.3390/axioms10030186
Güler E. The Fourth Fundamental Form IV of Dini-Type Helicoidal Hypersurface in the Four Dimensional Euclidean Space. Axioms. 2021; 10(3):186. https://doi.org/10.3390/axioms10030186
Chicago/Turabian StyleGüler, Erhan. 2021. "The Fourth Fundamental Form IV of Dini-Type Helicoidal Hypersurface in the Four Dimensional Euclidean Space" Axioms 10, no. 3: 186. https://doi.org/10.3390/axioms10030186





