The Effect of Nb/Ti Ratio on Hardness in High-Strength Ni-Based Superalloys
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Heat Treatment
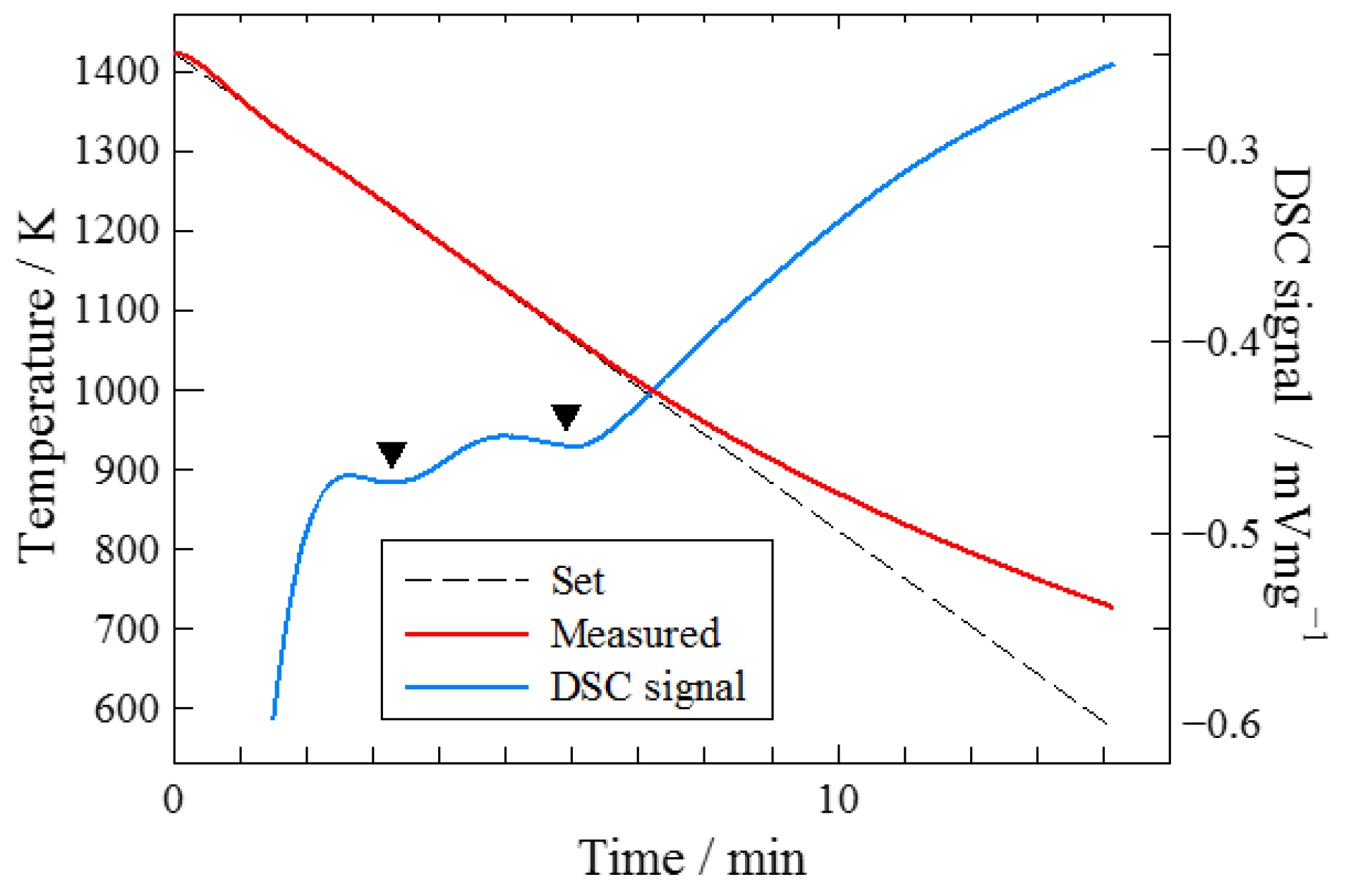
2.3. Microstructure Observation
2.4. Nanoindentation
3. Results
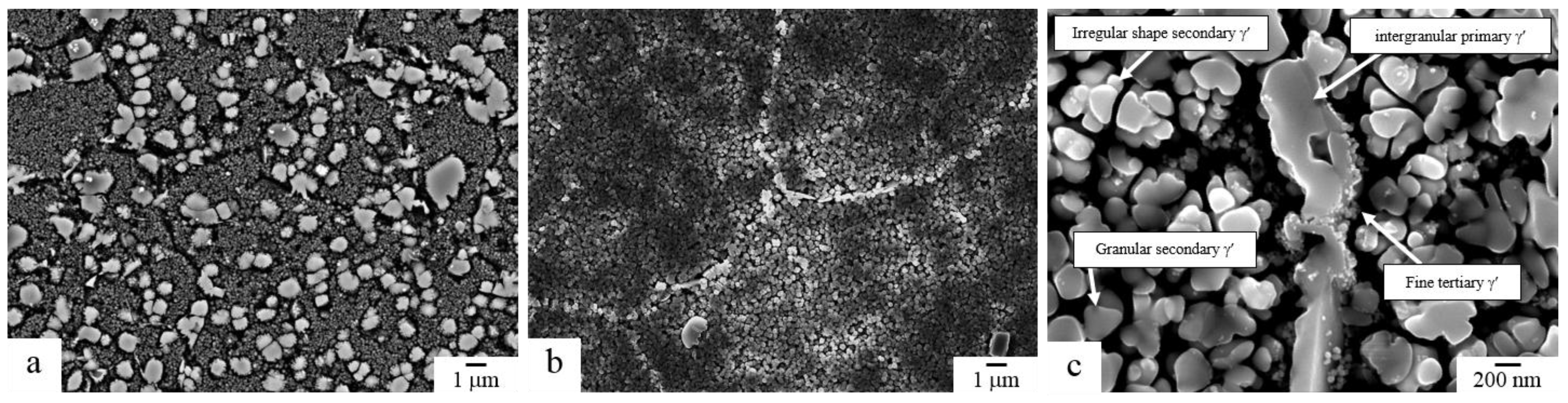
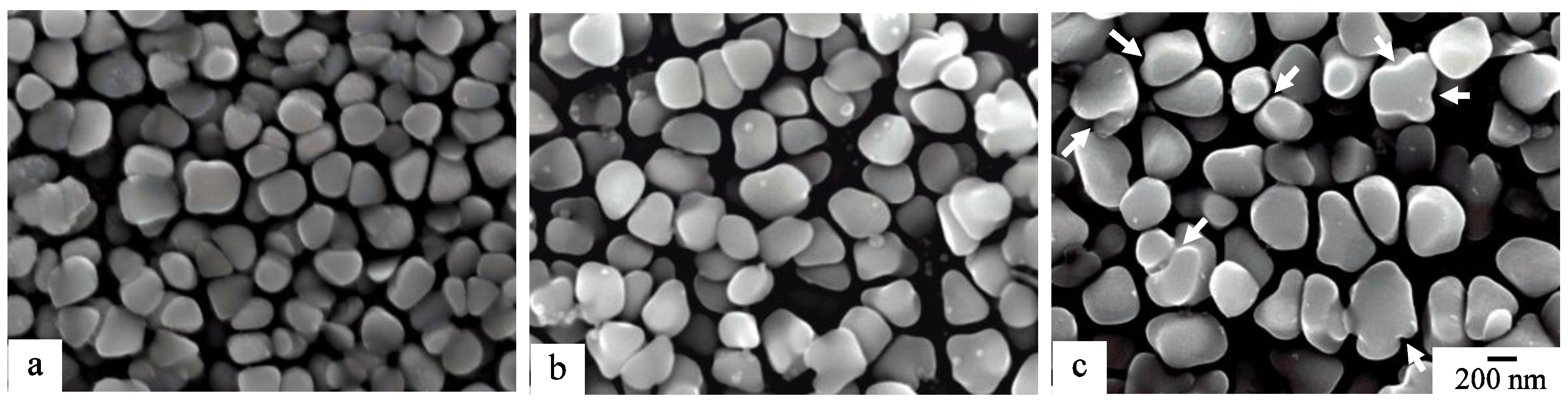
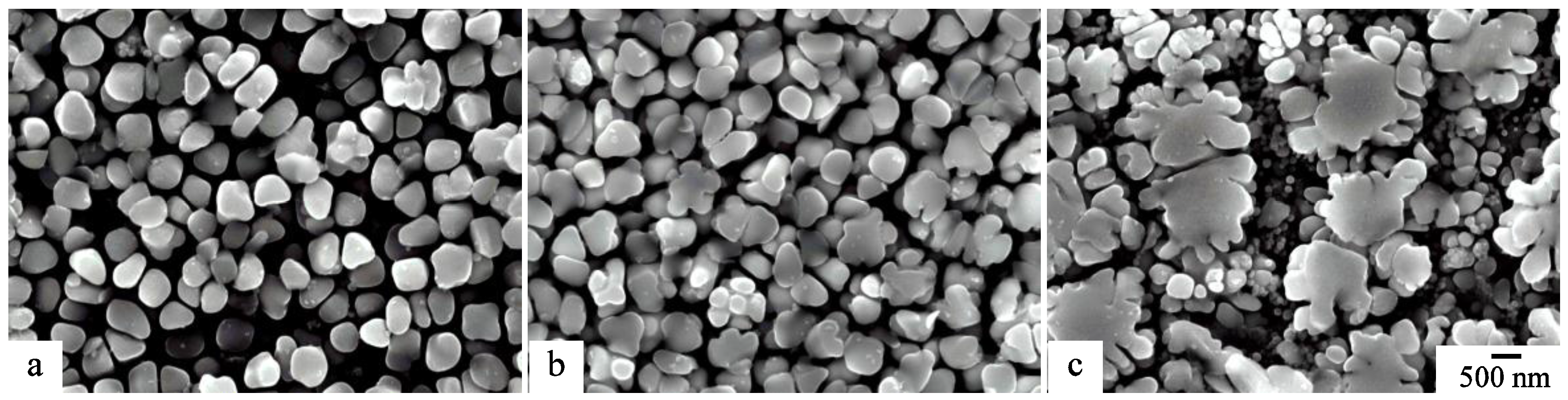
3.1. Initial Microstructure
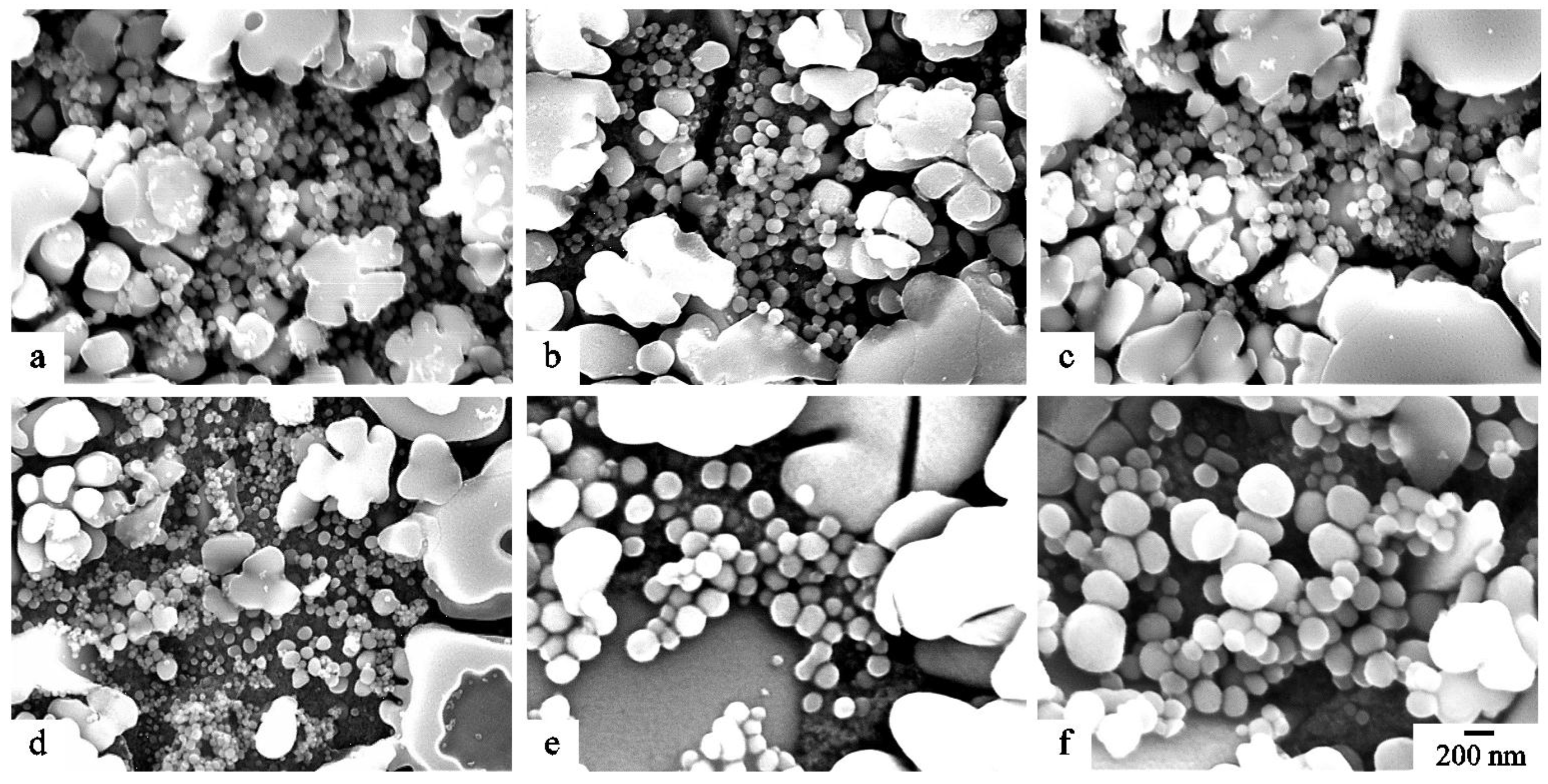
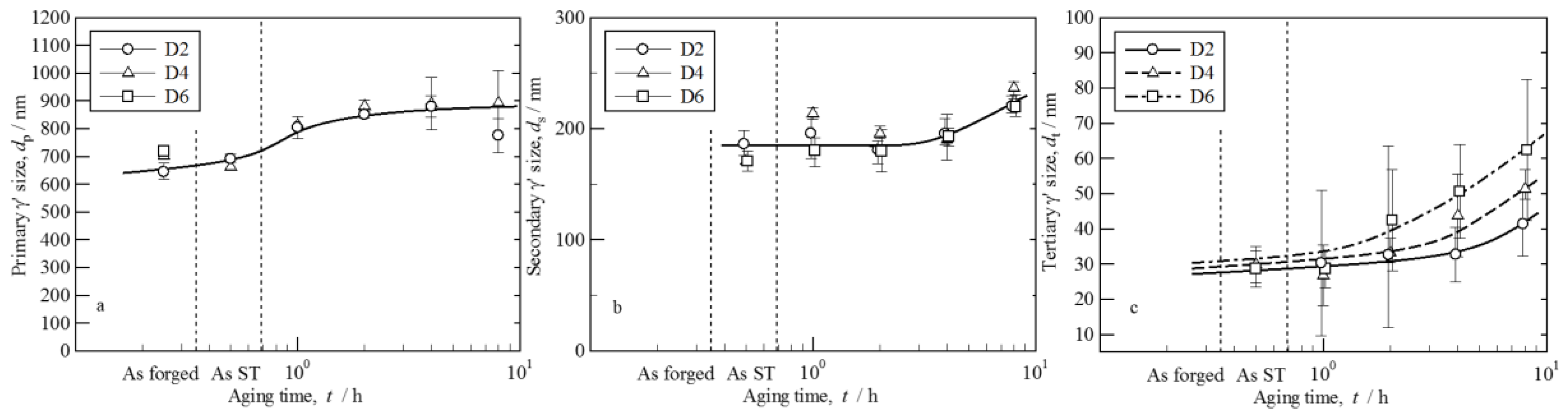
3.2. Microstructure Evolution with Aging
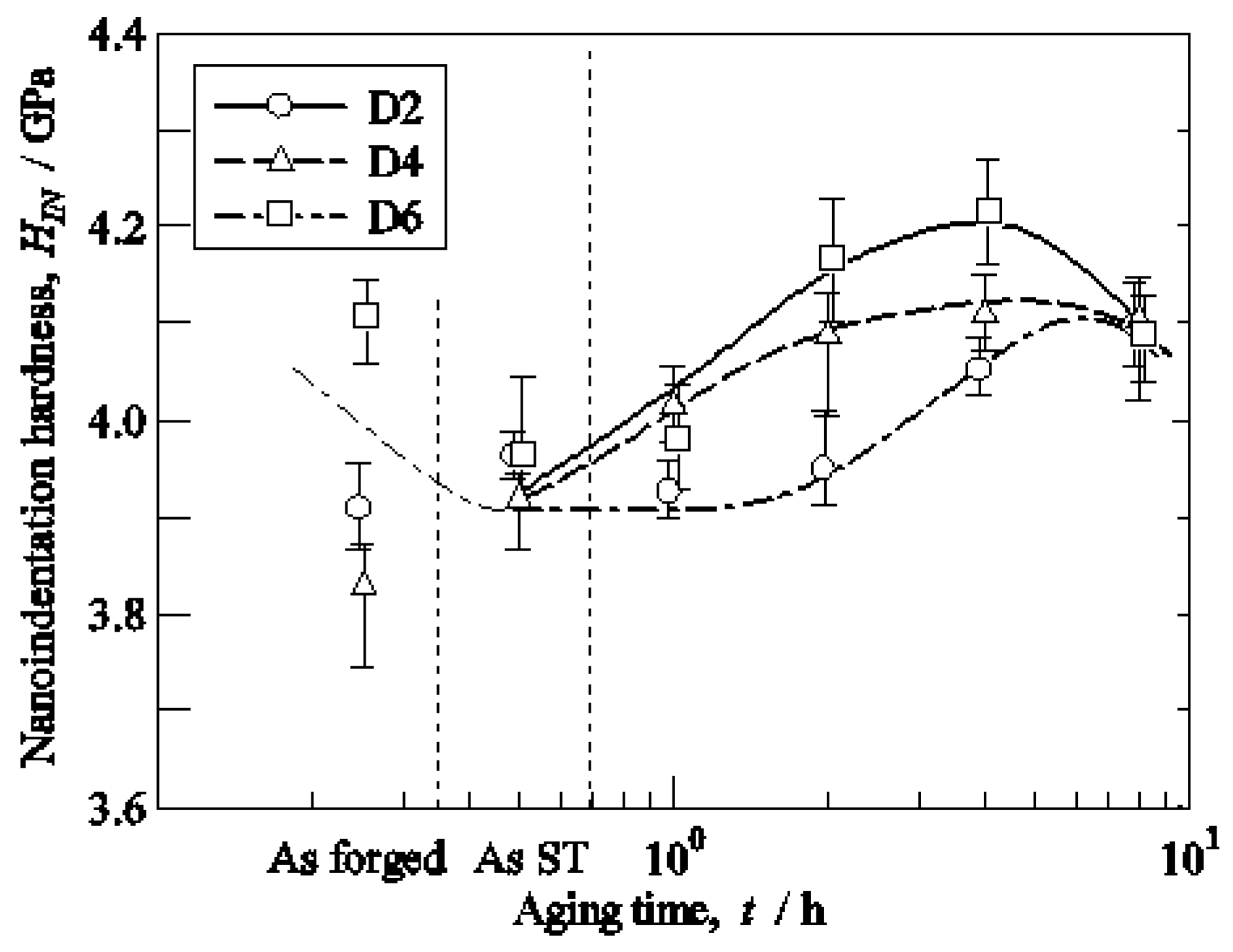
3.3. Nanoindentation Hardness
4. Discussion
4.1. The Effect of the Nb/Ti Ratio on the Multimodal Distribution of the γ′ Precipitates
4.2. Age Hardening Behaviour of the ABD Alloys
5. Conclusions
- Near-supersolvus solution-treated and cooled alloys show multimodal distributions of γ′ precipitates containing relatively coarse primary and secondary γ′ precipitates at the grain boundary and interior, respectively, and fine tertiary γ′ precipitates form a pool of them around the grain boundary or primary γ′ precipitates.
- All of the alloys studied show typical age-hardening behaviour at 1123 K, but higher peak hardness is obtained in the alloys that have higher Nb/Ti ratios.
- The microstructures of solution-treated alloys are similar and imply that they are independent of the Nb/Ti ratio; however, faster growth rates are observed for the tertiary γ′ precipitate in the alloy with higher Nb/Ti ratios. Larger tertiary γ′ precipitate are believed to be the reason why the higher hardness of the alloys studied.
- The Nb/Ti ratio does not influence the morphologies of primary and secondary precipitates, but it does influence tertiary γ′ precipitates; this might be due to Nb and Ti partitioning at high temperatures.
- Several pieces of the evidences are observed for cyclic coarsening or splitting in all of the alloys. The size of the secondary γ′ precipitates remained constant, which might be due to cyclic coarsening of the precipitates.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- IATA—Carbon-Natural Growth by 2020. Available online: http://www.iata.org/pressroom/pr/Pages/2009-06-08-03.aspx (accessed on 13 December 2016).
- Reed, R.C. The Superalloys: Fundamentals and Applications; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Miller, R.F.; Ansell, G.S. Low Temperature Mechanical Behavior of Ni–15Cr–Al–Ti–Mo Alloys. Metall. Trans. A 1977, 8, 1979–1991. [Google Scholar] [CrossRef]
- Maniar, G.N.; Bridge, J.E.; James, H.M.; Heydt, G.B. Correlation of Gamma-Gamma Prime Mismatch and Strengthening in Ni/Fe-Ni Base Alloys Containing Aluminum and Titanium as Hardeners. Metall. Trans. A 1970, 1, 31–42. [Google Scholar]
- Xu, Y.; Zhang, L.; Li, J.; Xiao, X.; Cao, X.; Jia, G.; Shen, Z. Relationship between Ti/Al Ratio and Stress-Rupture Properties in Nickel-Based Superalloy. Mater. Sci. Eng. A 2012, 544, 48–53. [Google Scholar] [CrossRef]
- Guo, E.C.; Ma, F.J. The Strengthening Effect of Niobium on Ni–Cr–Ti Type Wrought Superalloy. In Proceedings of the Fourth International Symposium on Superalloys, Champion, PA, USA, 21–25 September 1980; pp. 431–438.
- Mishima, Y.; Ochiai, S.; Hamao, N.; Yodogawa, M.; Suzuki, T. Solid Solution Hardening of Nickel—Role of Transition Metal and B-subgroup Solutes—. Trans. Jpn. Inst. Met. 1986, 27, 656–664. [Google Scholar] [CrossRef]
- Reed, R.C.; Tao, T.; Warnken, N. Alloys-By-Design: Application to Nickel-Based Single Crystal Superalloys. Acta Mater. 2009, 57, 5898–5913. [Google Scholar] [CrossRef]
- Zhu, Z.; Höglund, L.; Larsson, H.; Reed, R.C. Isolation of Optimal Compositions of Single Crystal Superalloys by Mapping of a Material’s Genome. Acta Mater. 2015, 90, 330–343. [Google Scholar] [CrossRef]
- Morinaga, M.; Yukawa, N.; Adachi, H.; Ezaki, H. New PHACOMP and Its Applications to Alloy Design. In Proceedings of the Fifth International Symposium on Superalloys, Champion, PA, USA, 7–11 October 1984; pp. 523–532.
- Shi, Z.; Liu, S.; Yue, X.; Wang, X.; Li, J. Effect of Nb content on Microstructure Stability and Stress Rupture Properties of Single Crystal Superalloy Containing Re and Ru. J. Cent. South Univ. 2016, 23, 1293–1300. [Google Scholar] [CrossRef]
- Rae, C.M.F.; Reed, R.C. The precipitation of Topologically Close-Packed Phases in Rhenium-Containing Superalloys. Acta Mater. 2001, 49, 4113–4125. [Google Scholar] [CrossRef]
- Singh, A.R.P.; Nag, S.; Chattopadhyay, S.; Ren, Y.; Tiley, J.; Viswanathan, G.B.; Fraser, H.L.; Banerjee, R. Mechanisms Related to Different Generations of γ′ Precipitation during Continuous Cooling of a Nickel Base Superalloy. Acta Mater. 2013, 61, 280–293. [Google Scholar] [CrossRef]
- Sarosi, P.M.; Wang, B.; Simmons, J.P.; Wang, Y.; Mills, M.J. Formation of Multimodal Size Distributions of γ′ in a Nickel-base Superalloy during Interrupted Continuous Cooling. Scr. Mater. 2007, 57, 767–770. [Google Scholar] [CrossRef]
- Radis, R.; Schaffer, M.; Albu, M.; Kothleitner, G.; Pölt, P.; Kozeschnik, E. Multimodal Size Distributions of γ′ Precipitates during Continuous Cooling of UDIMET 720Li. Acta Mater. 2009, 57, 5739–5747. [Google Scholar] [CrossRef]
- Raynor, D.; Silcock, J.M. Strengthening Mechanisms in γ′ Precipitating Alloys. Metal Sci. J. 1970, 4, 121–130. [Google Scholar] [CrossRef]
- Reppich, B. Some New Aspects Concerning Particle Hardening Mechanisms in γ′ Precipitating Ni-Base Alloys—I. Theoretical Concept. Acta Metall. 1982, 30, 87–94. [Google Scholar] [CrossRef]
- Jackson, M.; Reed, R.C. Heat Treatment of UDIMET 720Li: The Effect of Microstructure on Properties. Mater. Sci. Eng. A 1999, 259, 85–97. [Google Scholar] [CrossRef]
- Collins, D.; Stone, H. A Modelling Approach to Yield Strength Optimisation in a Nickel-Base Superalloy. Int. J. Plast. 2014, 54, 96–112. [Google Scholar] [CrossRef]
- Galindo-Nava, E.I.; Connor, L.D.; Rae, C.M.F. On the Prediction of the Yield Stress of Unimodal and Multimodal γ′ Nickel-Base Superalloys. Acta Mater. 2015, 98, 377–390. [Google Scholar] [CrossRef]
- Collins, D.M.; Crudden, D.J.; Alabort, E.; Connolley, T.; Reed, R.C. Time-Resolved Synchrotron Diffractometry of Phase Transformations in High Strength Nickel-Based Superalloys. Acta Mater. 2015, 94, 244–256. [Google Scholar] [CrossRef]
- Mitchell, R.J.; Preuss, M.; Hardy, M.C.; Tin, S. Influence of Composition and Cooling Rate on Constrained and Unconstrained Lattice Parameters in Advanced Polycrystalline Nickel-Base Superalloys. Mater. Sci. Eng. A 2006, 423, 282–291. [Google Scholar] [CrossRef]
- Baldan, A. Review Progress in Ostwald Ripening Theories and Their Applications to the γ′-Precipitates in Nickel-Based Superalloys. J. Mater. Sci. 2002, 37, 2379–2405. [Google Scholar] [CrossRef]
- Ricks, R.A.; Porter, A.J.; Ecob, R.C. The Growth of γ′ Precipitates in Nickel-base Superalloys. Acta Metall. 1983, 31, 43–53. [Google Scholar] [CrossRef]
- Qiu, C.L.; Andrews, P. On the Formation of Irregular-Shaped Gamma Prime and Serrated Grain Boundaries in a Nickel-Based Superalloy during Continuous Cooling. Mater. Charact. 2013, 76, 28–34. [Google Scholar] [CrossRef]
- Chen, Y.; Prasath Babu, R.; Slater, T.J.A.; Bai, M.; Mitchell, R.; Ciuca, O.; Preuss, M.; Haigh, S.J. An Investigation of Diffusion-Mediated Cyclic Coarsening and Reversal Coarsening in an Advanced Ni-Based Superalloy. Acta Mater. 2016, 110, 295–305. [Google Scholar] [CrossRef]
- Zhao, X.; Duddu, R.; Bordas, S.P.A.; Qu, J. Effects of Elastic Strain Energy and Interfacial Stress on the Equilibrium Morphology of Misfit Particles in Heterogeneous Solids. J. Mech. Phys. Solids 2013, 61, 1433–1445. [Google Scholar] [CrossRef]

| Parameters | Titanium | Niobium |
|---|---|---|
| Atomic number | 22 | 41 |
| Period | 4 | 5 |
| Melting point of pure metal | 1941 K | 2750 K |
| Atomic radius | 0.160 nm | 0.146 nm |
| Diffusion coefficient in A1–Ni at 1173 K [2] | 5 × 10−16 m2·s−1 | 3 × 10−16 m2·s−1 |
| Vegard coefficient for L12–Ni3Al [2] | 2.5 × 10−4 nm/atom % | 4.5 × 10−4 nm/atom % |
| Metal-d levels [10] | 2.271 eV | 2.117 eV |
| Alloy | Ni | Cr | Co | Mo | W | Al | Ti | Ta | Nb | C | B | Zr |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| D2 | Bal. | 18.7 | 18.2 | 0 | 0.9 | 8 | 4.1 | 0.6 | 0 | 0.127 | 0.078 | 0.037 |
| D4 | Bal. | 18.7 | 18.2 | 0 | 0.9 | 8 | 3.6 | 0.6 | 0.4 | 0.127 | 0.078 | 0.037 |
| D6 | Bal. | 18.7 | 18.2 | 0 | 0.9 | 8 | 2.8 | 0.6 | 1.2 | 0.127 | 0.078 | 0.037 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hisazawa, H.; Terada, Y.; Adziman, F.; Crudden, D.J.; Collins, D.M.; Armstrong, D.E.J.; Reed, R.C. The Effect of Nb/Ti Ratio on Hardness in High-Strength Ni-Based Superalloys. Metals 2017, 7, 71. https://doi.org/10.3390/met7030071
Hisazawa H, Terada Y, Adziman F, Crudden DJ, Collins DM, Armstrong DEJ, Reed RC. The Effect of Nb/Ti Ratio on Hardness in High-Strength Ni-Based Superalloys. Metals. 2017; 7(3):71. https://doi.org/10.3390/met7030071
Chicago/Turabian StyleHisazawa, Hiromu, Yoshihiro Terada, Fauzan Adziman, David J. Crudden, David M. Collins, David E.J. Armstrong, and Roger C. Reed. 2017. "The Effect of Nb/Ti Ratio on Hardness in High-Strength Ni-Based Superalloys" Metals 7, no. 3: 71. https://doi.org/10.3390/met7030071
APA StyleHisazawa, H., Terada, Y., Adziman, F., Crudden, D. J., Collins, D. M., Armstrong, D. E. J., & Reed, R. C. (2017). The Effect of Nb/Ti Ratio on Hardness in High-Strength Ni-Based Superalloys. Metals, 7(3), 71. https://doi.org/10.3390/met7030071






