Grouting for Tunnel Stability Control and Inadequate Grouting Section Recognition: A Case Study of Countermeasure of Giant Karst Cave
Abstract
:1. Introduction
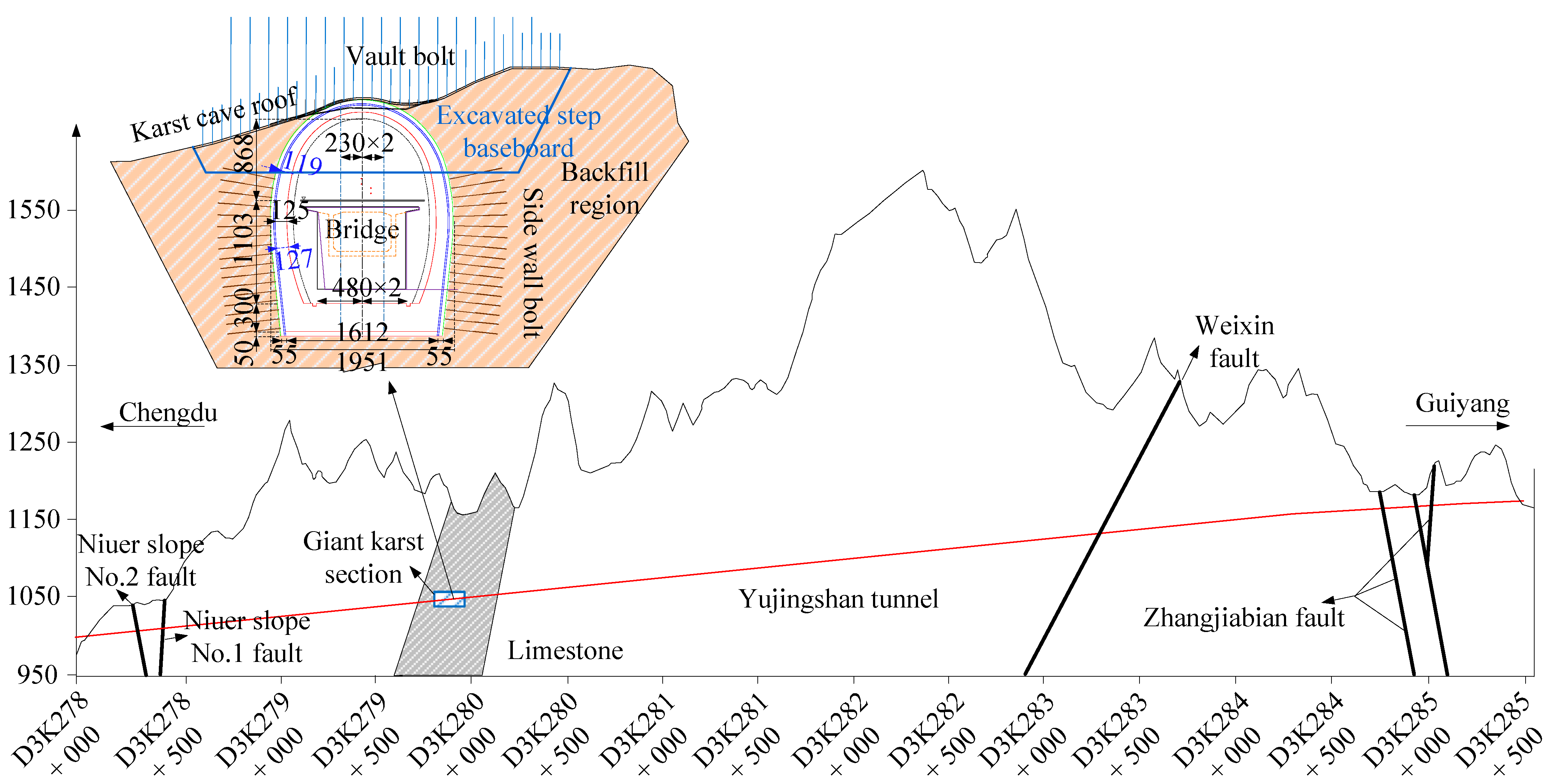
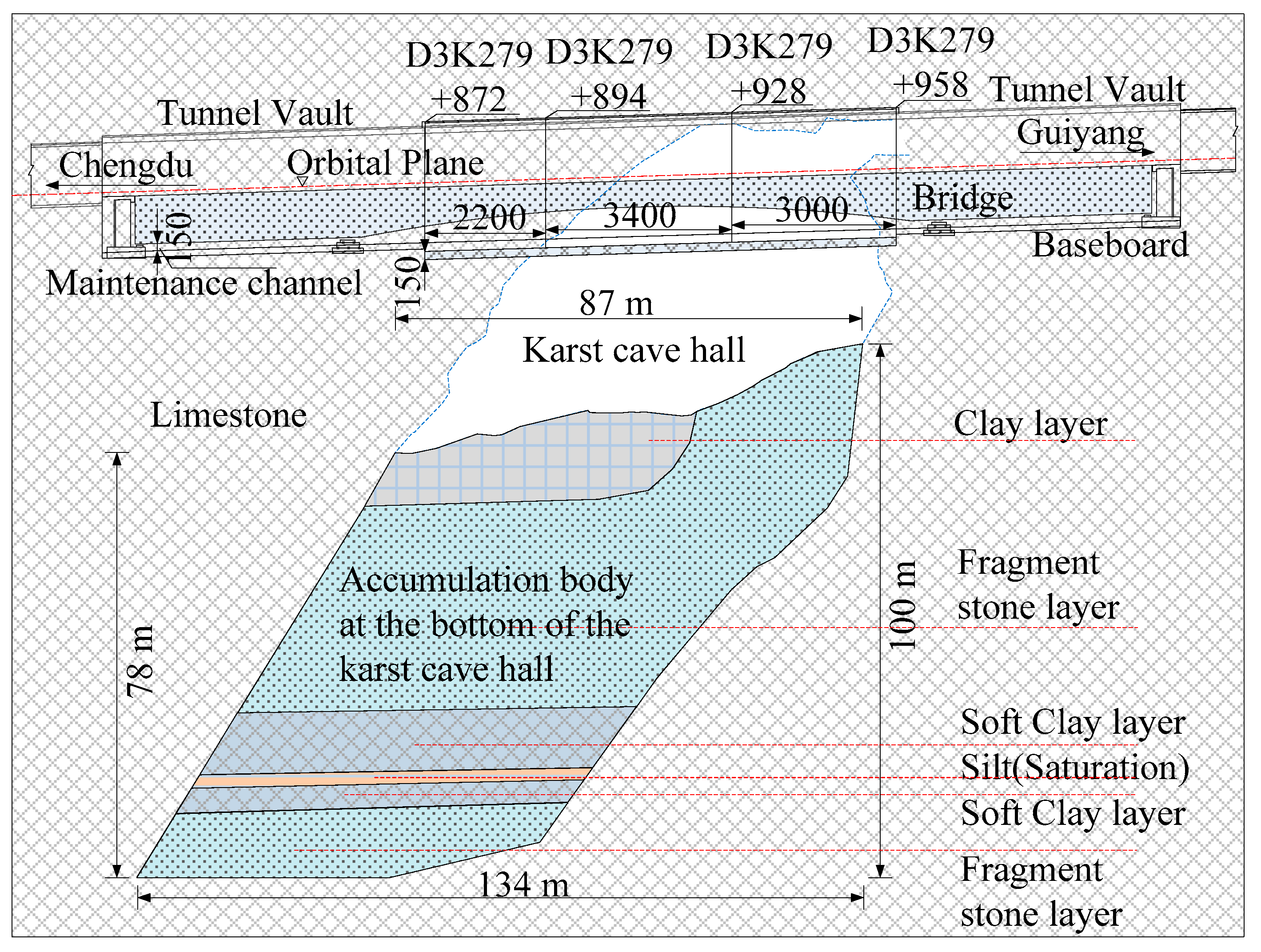
2. Background of the Yujingshan Tunnel
3. Numerical Simulation of Tunnel Excavation without Grouting
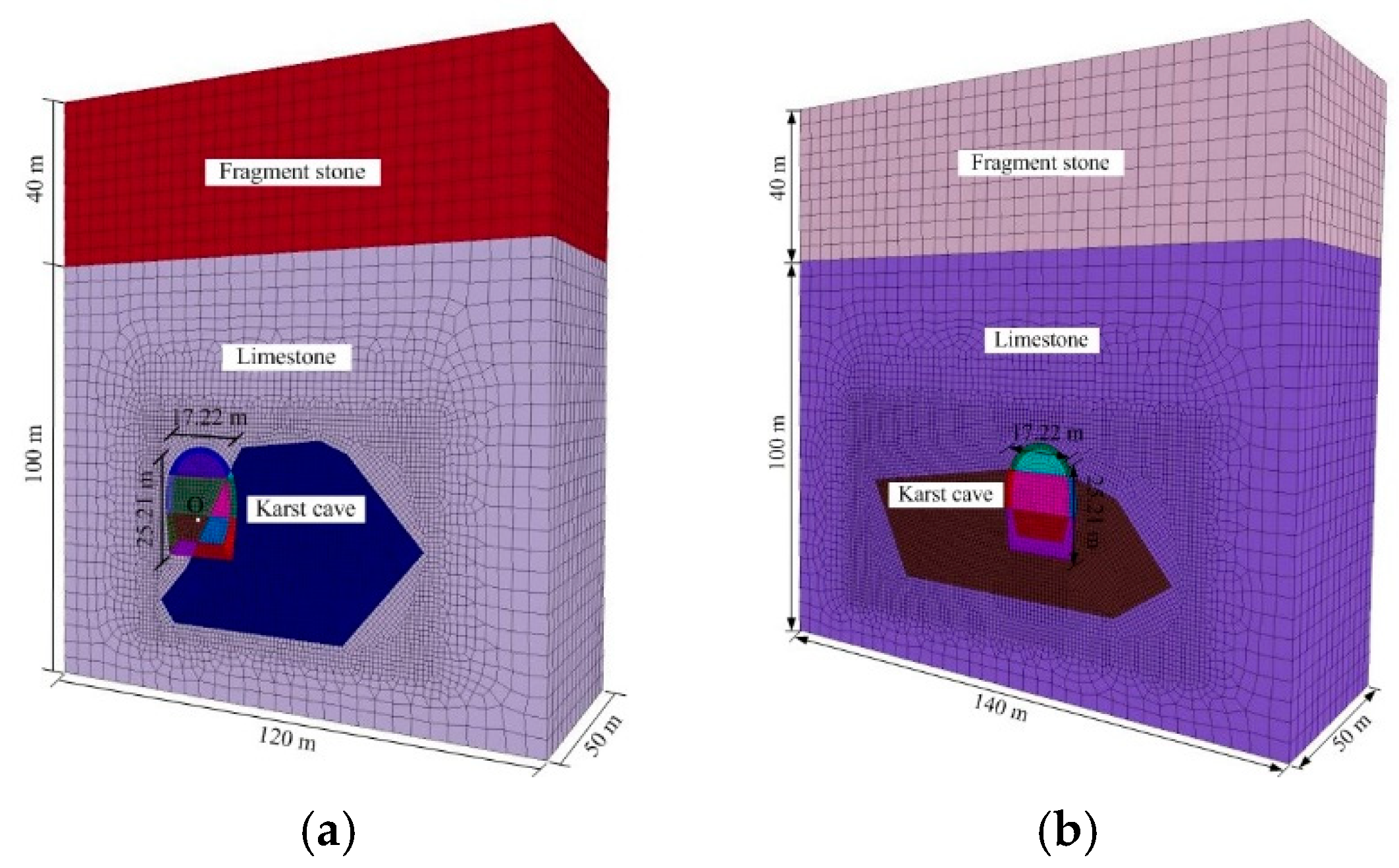
3.1. The Selection of the Tunnel Section and Establishment of Numerical Model
3.2. The Validation of Numerical Model
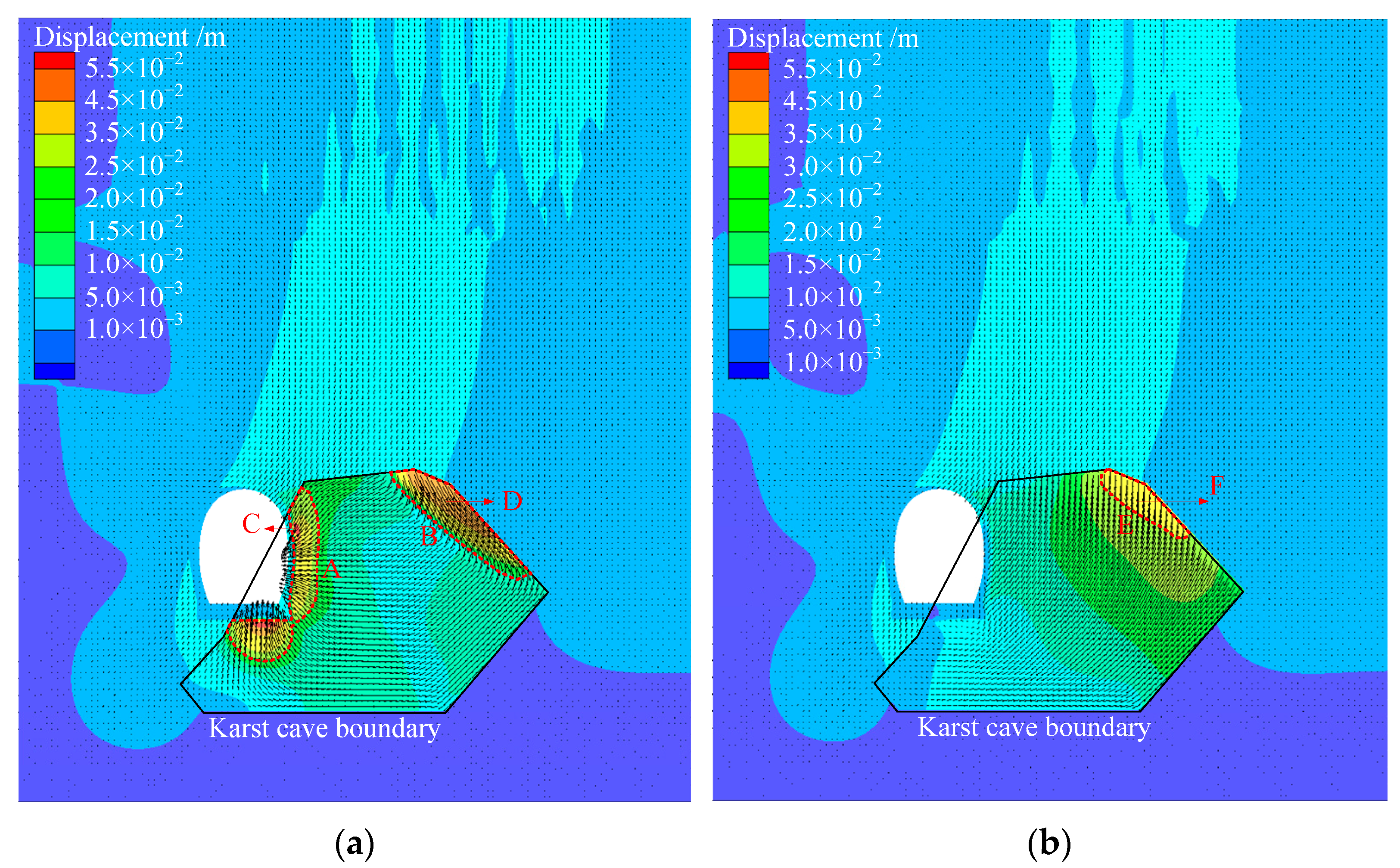
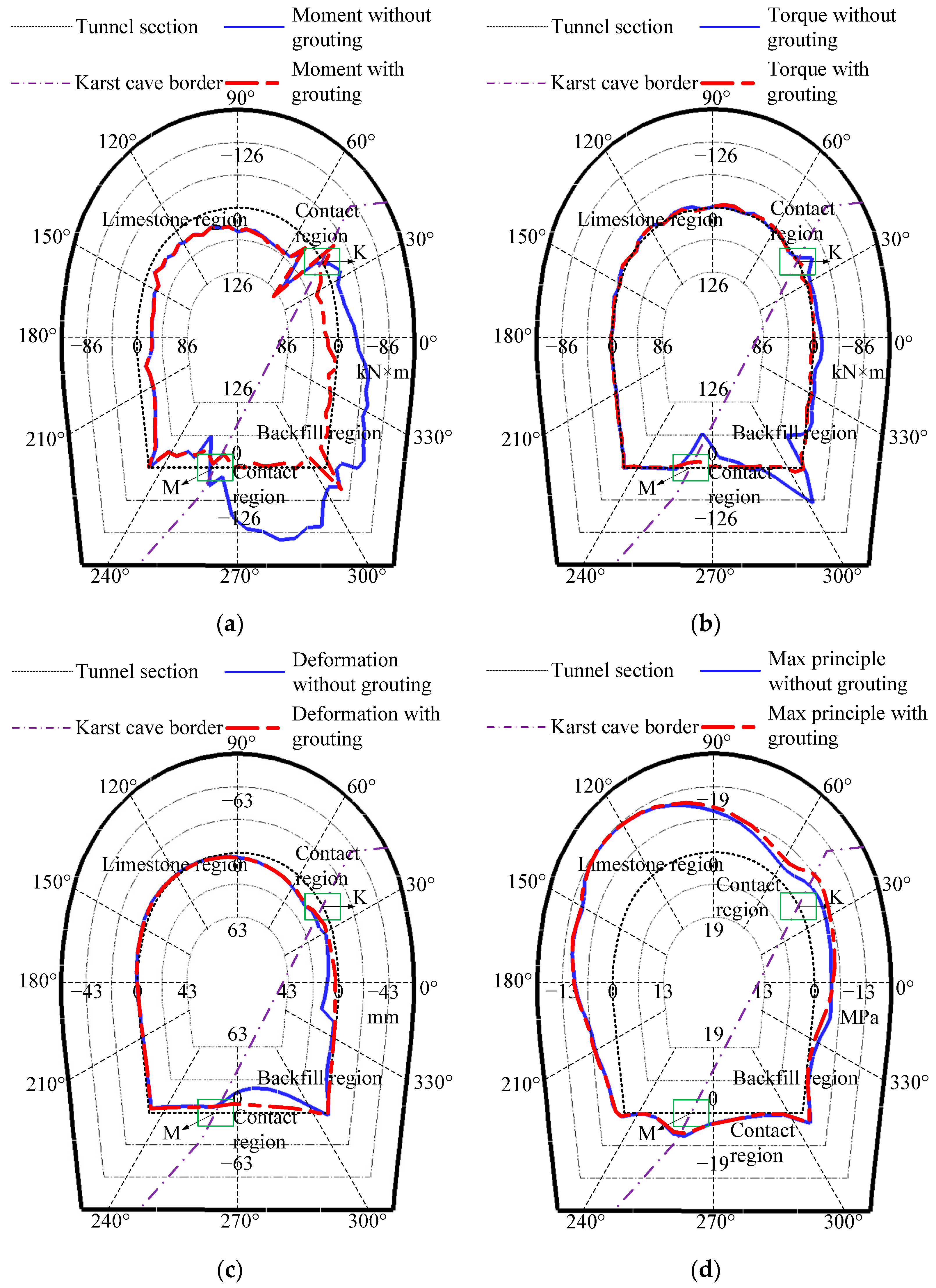
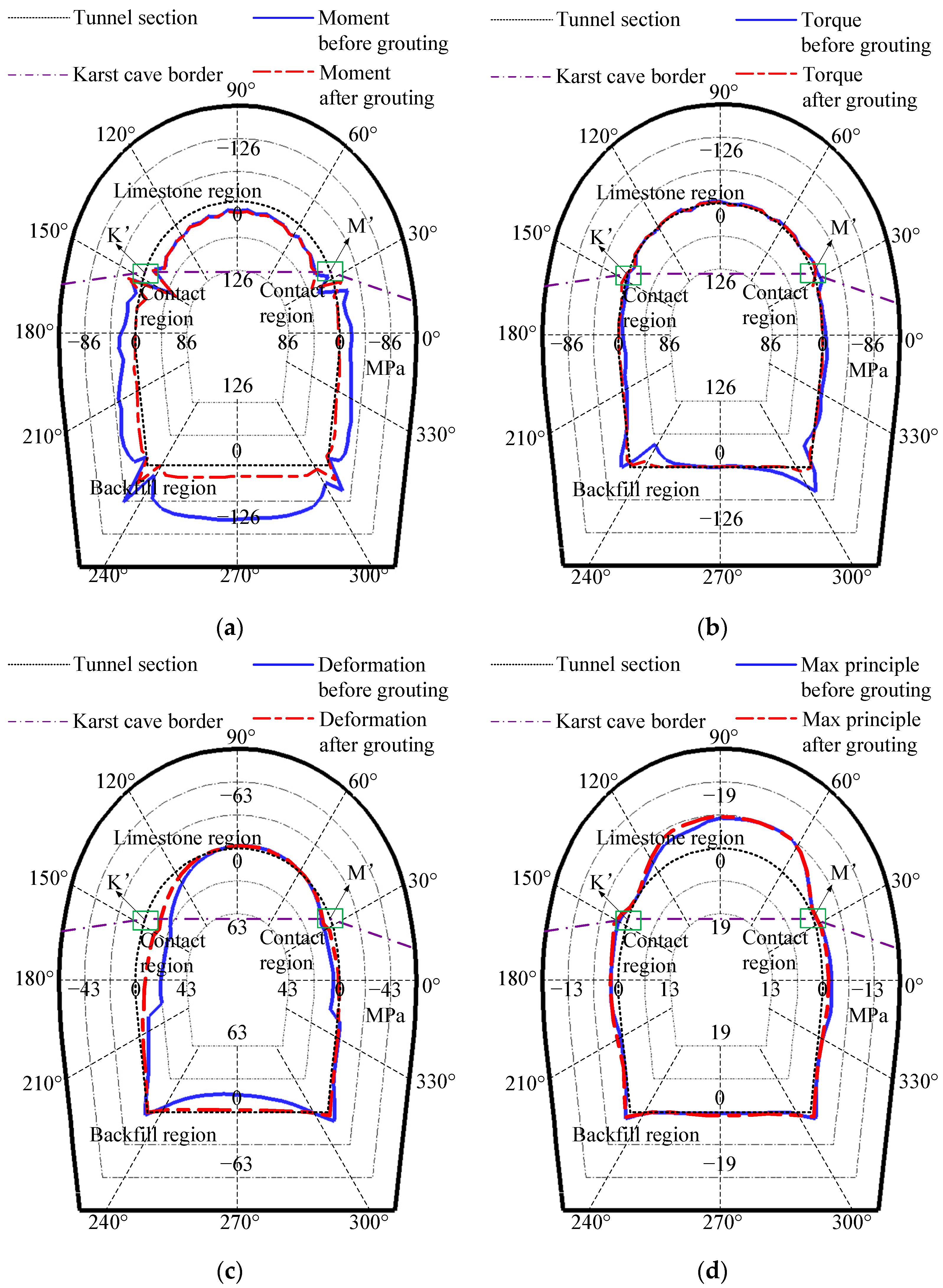
3.3. Mechanical Response Analysis of I-Type Section Tunnel
3.4. Mechanical Response Analysis of II-Type Section Tunnel
4. Grouting Design and Scheme
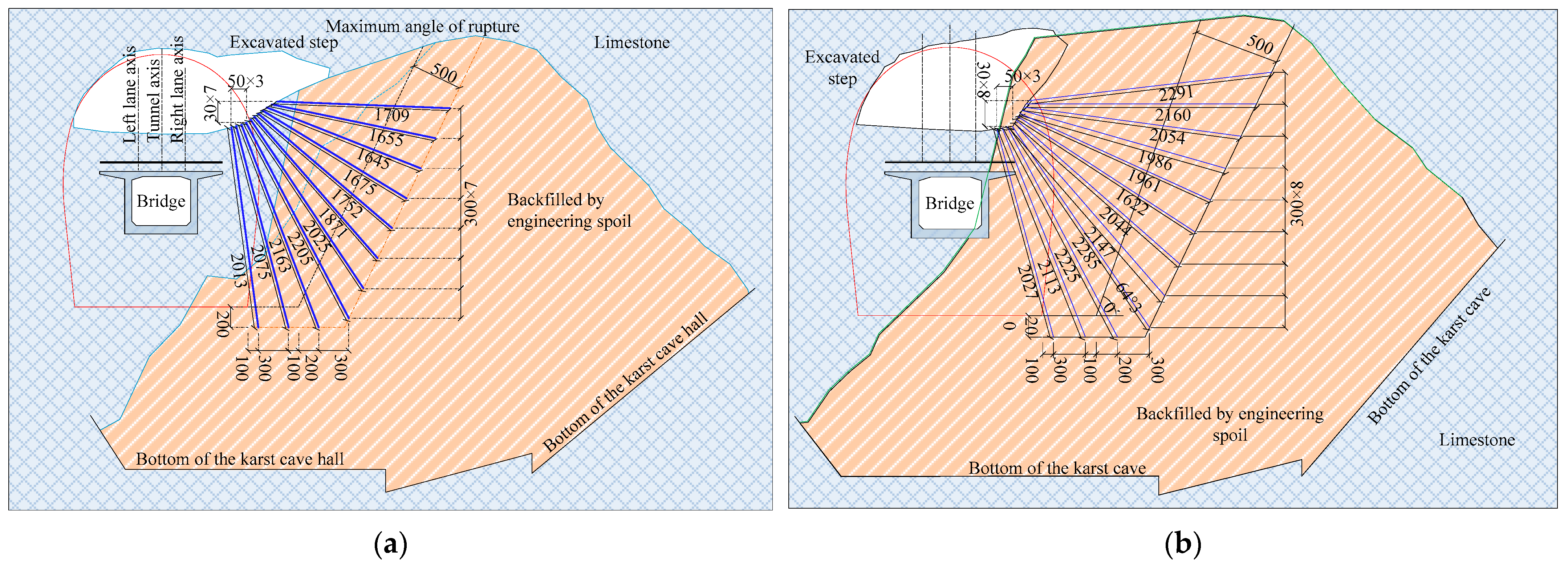
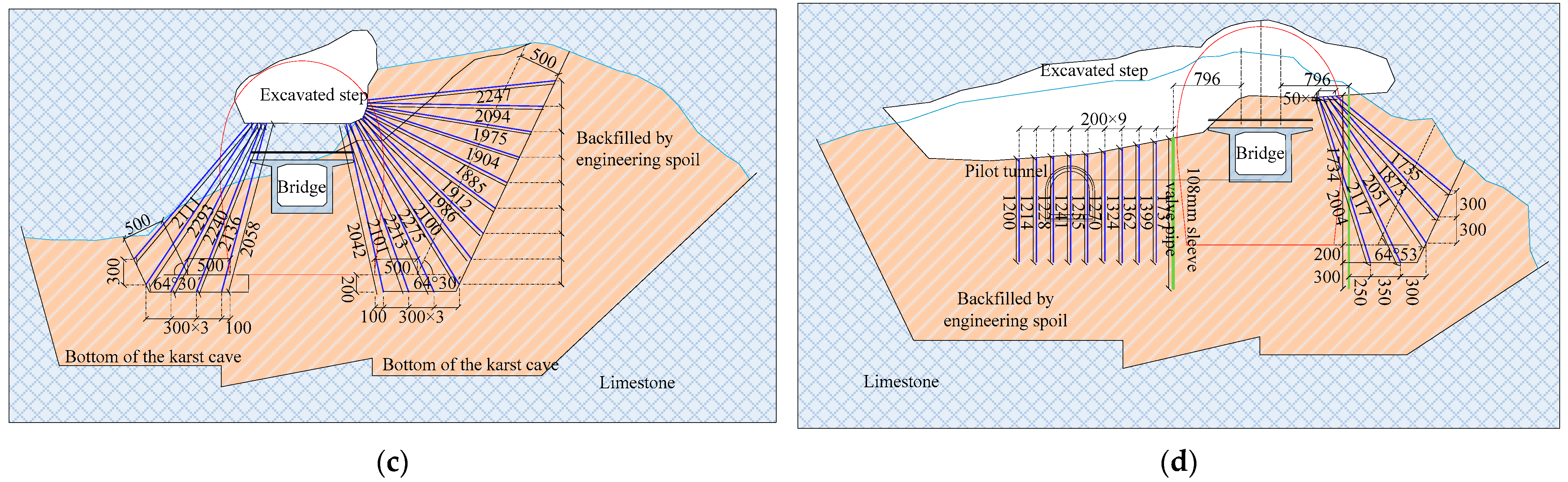
4.1. Grouting Penetration Distance
4.2. Yujinghsan Tunnel Side Wall Grouting Reinforcement Scheme
5. Recognition and Validation of Inadequate Grouting Section
5.1. Analysis of Grouting Record
5.2. Single Hole Grouting Quantity Analysis
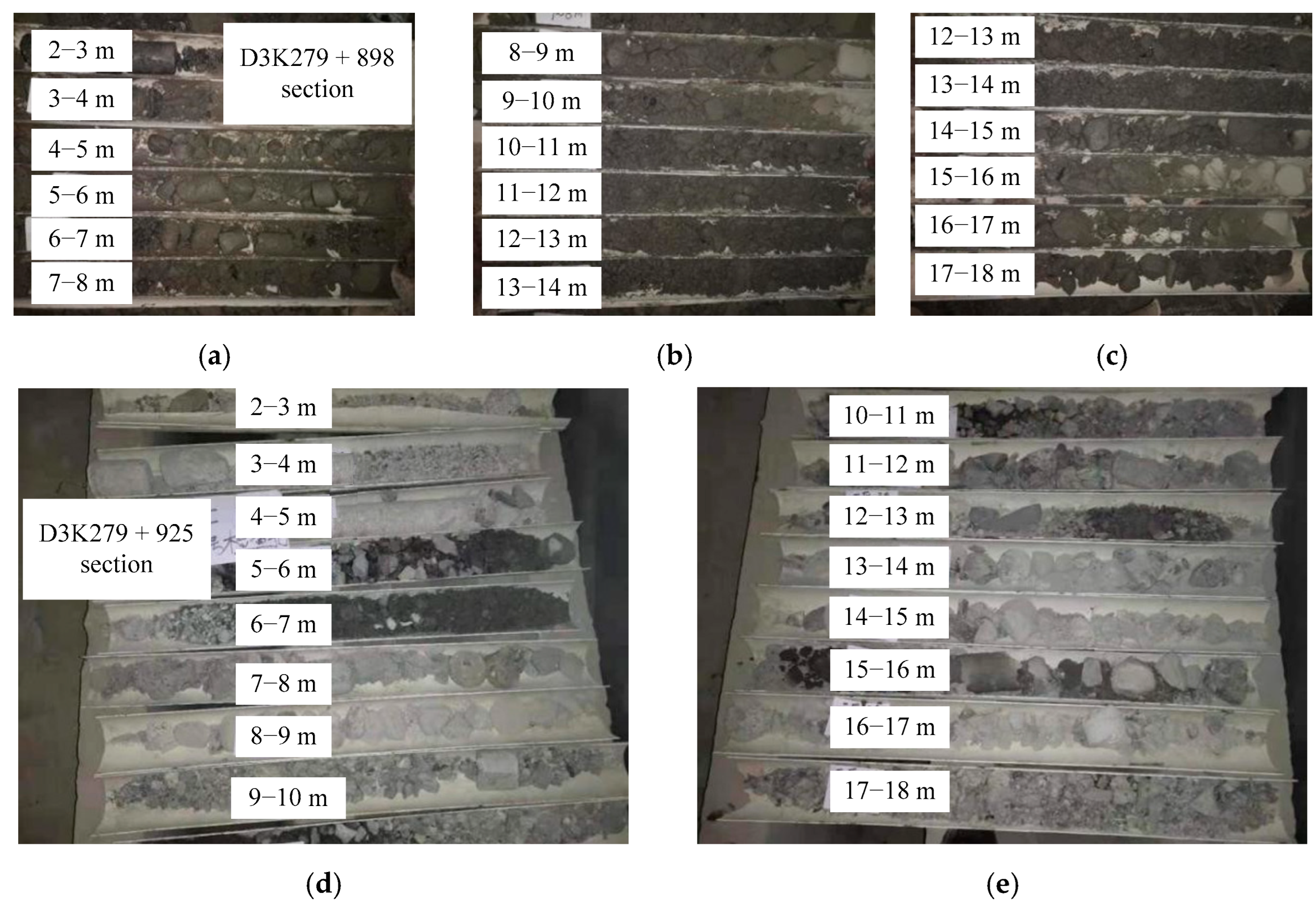
5.3. Validation of Recognition Result of Inadequate Reinforcement Section
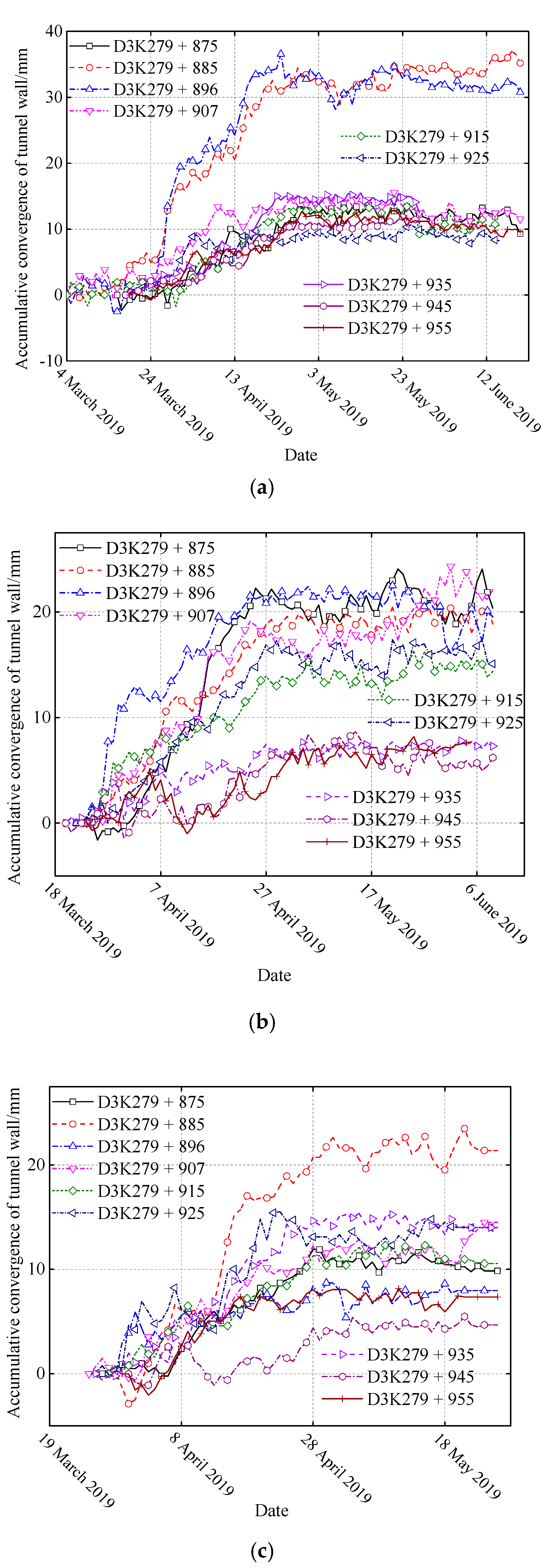
5.4. Application Effect Evaluation of Countermeasure by Field Monitor
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, N.; Pei, J.; Cao, C.; Liu, X.; Huang, Y.; Mei, G. Geological investigation and treatment measures against water inrush hazard in karst tunnels: A case study in Guiyang, southwest China. Tunn. Undergr. Space Technol. 2022, 124, 104491. [Google Scholar] [CrossRef]
- Wu, B.; Sun, W.; Cai, G.; Meng, G. Reliability analysis of shallow-buried tunnel construction adjacent to karst cave. Comput. Geotech. 2022, 145, 104673. [Google Scholar] [CrossRef]
- Zhang, N.; Zhou, A.; Pan, Y.; Shen, S.-L. Measurement and prediction of tunnelling-induced ground settlement in karst region by using expanding deep learning method. Measurement 2021, 183, 109700. [Google Scholar] [CrossRef]
- Lai, J.; Zhou, H.; Wang, K.; Qiu, J.; Wang, L.; Wang, J.; Feng, Z. Shield-driven induced ground surface and Ming Dynasty city wall settlement of Xi’an metro. Tunn. Undergr. Space Technol. 2019, 97, 103220. [Google Scholar] [CrossRef]
- Wang, F.; Zhao, Y.; Li, C.; Li, C.; Lan, T.; Ping, S.; Cao, Y. An experimental study on the corrosion characteristics of the karst tunnel engineering area in Southwest China. Bull. Eng. Geol. Environ. 2019, 78, 4047–4061. [Google Scholar] [CrossRef]
- Li, D.T.; Luo, Y. Measurement of carbonate rocks distribution area in China. Carsol. Sin. 1983, 2, 147–150. [Google Scholar]
- Li, S.C.; Zhou, Z.Q.; Ye, Z.H.; Li, L.P.; Zhang, Q.Q.; Xu, Z.H. Comprehensive geophysical prediction and treatment measures of karst caves in deep buried tunnel. J. Appl. Geophys. 2015, 116, 247–257. [Google Scholar] [CrossRef]
- Zheng, Y.; He, S.; Yu, Y.; Zheng, J.; Zhu, Y.; Liu, T. Characteristics, challenges and countermeasures of giant karst cave: A case study of Yujingshan tunnel in high-speed railway. Tunn. Undergr. Space Technol. 2021, 114, 103988. [Google Scholar] [CrossRef]
- Alija, S.; Torrijo, F.J.; Quinta-Ferreira, M. Geological engineering problems associated with tunnel construction in karst rock masses: The case of Gavarres tunnel (Spain). Eng. Geol. 2013, 157, 103–111. [Google Scholar] [CrossRef]
- Schneider, A.; Lavdas, N. Albula tunnel II: Concept for tunneling in karst-like cellular dolomite, Underground—The Way to the Future. In Proceedings of the World Tunnel Congress, WTC, Geneva, Switzerland, 31 May–1 June 2013; pp. 1027–1034. [Google Scholar]
- Song, K.I.; Cho, G.C.; Chang, S.B. Identification, remediation, and analysis of karst sinkholes in the longest railroad tunnel in South Korea. Eng. Geol. 2012, 135–136, 92–105. [Google Scholar] [CrossRef]
- Yang, J.; Zhang, C.; Fu, J.; Wang, S.; Ou, X.; Xie, Y. Pre-grouting reinforcement of underwater karst area for shield tunneling passing through Xiangjiang River in Changsha, China. Tunn. Undergr. Space Technol. 2020, 100, 103380. [Google Scholar] [CrossRef]
- Li, H.; Liu, J.; Wu, J.; Xu, Z.; Zhang, X.; Zhang, L.; Li, Z. Grouting sealing method of flow-control speed-down in karst pipelines and its engineering application. Tunn. Undergr. Space Technol. 2021, 108, 103695. [Google Scholar] [CrossRef]
- Shangxin, F.; Yufei, Z.; Yujie, W.; Shanyong, W.; Ruilang, C. A comprehensive approach to karst identification and groutability evaluation—A case study of the Dehou reservoir, SW China. Eng. Geol. 2020, 269, 105529. [Google Scholar] [CrossRef]
- Shen, S.-L.; Cui, Q.-L.; Ho, C.-E.; Xu, Y.-S. Ground Response to Multiple Parallel Microtunneling Operations in Cemented Silty Clay and Sand. J. Geotech. Geoenviron. Eng. 2016, 142, 04016001. [Google Scholar] [CrossRef]
- Zhang, C.; Fu, J.; Yang, J.; Ou, X.; Ye, X.; Zhang, Y. Formulation and performance of grouting materials for underwater shield tunnel construction in karst ground. Constr. Build. Mater. 2018, 187, 327–338. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, P.; Wang, G.; Li, S.; Zhang, X.; Zhang, Q.; Wang, Q.; Liu, J. Parameters Optimization of Curtain Grouting Reinforcement Cycle in Yonglian Tunnel and Its Application. Math. Probl. Eng. 2015, 2015, 615736. [Google Scholar] [CrossRef] [Green Version]
- Cui, Q.-L.; Shen, S.-L.; Xu, Y.-S.; Wu, H.-N.; Yin, Z.-Y. Mitigation of geohazards during deep excavations in karst regions with caverns: A case study. Eng. Geol. 2015, 195, 16–27. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Wu, J.; Zhang, X.; Zhang, L.; Li, Z. Grouting sealing mechanism of water gushing in karst pipelines and engineering application. Constr. Build. Mater. 2020, 254, 119250. [Google Scholar] [CrossRef]
- Li, S.; Qi, Y.; Li, Z.; Li, H.; Zhang, J. A novel treatment method and construction technology of the pipeline gushing water geohazards in karst region. Tunn. Undergr. Space Technol. 2021, 113, 103939. [Google Scholar] [CrossRef]
- Lin, S.-S.; Shen, S.-L.; Zhou, A.; Xu, Y.-S. Novel model for risk identification during karst excavation. Reliab. Eng. Syst. Saf. 2021, 209, 107435. [Google Scholar] [CrossRef]
- Yau, K.; Paraskevopoulou, C.; Konstantis, S. Spatial variability of karst and effect on tunnel lining and water inflow. A probabilistic approach. Tunn. Undergr. Space Technol. 2020, 97, 103248. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, S.; Li, L.; Han, J.; Zhang, B.; Hou, D.; Wang, J.; Lin, C. A preliminary study of the properties of potassium phosphate magnesium cement-based grouts admixed with metakaolin, sodium silicate and bentonite. Constr. Build. Mater. 2020, 262, 119893. [Google Scholar] [CrossRef]
- Lindquist, E.S. The Strength and Deformation Properties of Melange; University of California: Berkeley, CA, USA, 1994; p. 287. [Google Scholar]
- Medley, E.W. The Engineering Characterization of Melanges and Similar Block-in-Matrix Rocks (Bimrocks); University of California: Berkeley, CA, USA, 1994. [Google Scholar]
- Li, S.; Yang, Z.; Tian, X.; Xiao, Y.; Li, X.; Liu, X. Influencing factors of scale effects in large-scale direct shear tests of soil-rock mixtures based on particle breakage. Tunn. Undergr. Space Technol. 2021, 113, 103939. [Google Scholar] [CrossRef]
- Vallejo, L.E.; Mawby, R. Porosity influence on the shear strength of granular material–clay mixtures. Eng. Geol. 2000, 58, 125–136. [Google Scholar] [CrossRef]
- Wang, T.; Yan, C.; Zheng, Y.; Jiao, Y.-Y.; Zou, J. Numerical study on the effect of meso-structure on hydraulic conductivity of soil-rock mixtures. Comput. Geotech. 2022, 146, 104726. [Google Scholar] [CrossRef]
- Li, X.; Liao, Q.L.; He, J.M. In-situ tests and a stochastic structural model of rock and soil aggregate in the three gorges reservoir area, China. Int. J. Rock Mech. Min. Sci. 2004, 41, 702–707. [Google Scholar] [CrossRef]
- Li, S.; Zhao, M.; Wang, Y.; Rao, Y. A New Numerical Method for Dem–Block and Particle Model. Int. J. Rock Mech. Min. Sci. 2004, 41, 414–418. [Google Scholar] [CrossRef]
- Wang, S.; Zhu, Y.; Ma, W.; Wang, Z.; Li, G. Effects of rock block content and confining pressure on dynamic characteristics of soil-rock mixtures. Eng. Geol. 2020, 280, 105963. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; Zheng, B. Stress-strain behavior of soil-rock mixture at medium strain rates—Response to seismic dynamic loading. Soil Dyn. Earthq. Eng. 2017, 93, 7–17. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, D.; Fang, Q.; Dui, G.; Chu, Z. Analytical solution for deep tunnels in strain-softening rocks modeled by different elastic strain definitions with the unified strength theory. Sci. China Tech. Sci. 2022, 65, 2503–2519. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, D.; Li, A.; Lu, S.; Tai, Q.; Chu, Z. Model test and numerical analysis for the face failure mechanism of large cross-section tunnels under different ground conditions. Tunn. Undergr. Space Technol. 2022, 130, 104735. [Google Scholar] [CrossRef]
- Kun, Y. Study on the Comprehensive Treatment of Karst along the Tunnel of Chengdu-Guizhou Newly Built Railway Line. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2018. (In Chinese). [Google Scholar]
- Wang, Y. Study on the Groundwater Environment Impact Prediction and Protection Measures for Yujingshan Tunnel Project. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2015. (In Chinese). [Google Scholar]
- Xie, S.; Huang, F.; Wang, G.; Zhu, C.; Su, Y.; Qian, F. Mechanical response to different excavation methods of Qichongcun tunnel in Guiyang City, China. Adv. Mater. Sci. Eng. 2021, 2021, 7848874. [Google Scholar] [CrossRef]
- Huang, Y.; Liu, N.; Song, B.; Wu, B.; Cao, C. Construction of multiple intersections of tunnel structures in limestone and mudstone section: A case study. Arab. J. Sci. Eng. 2022, 47, 12817–12837. [Google Scholar]
- Ding, W.; Tan, S.; Zhu, R.; Jiang, H.; Zhang, Q. Study on the damage process and numerical simulation of tunnel excavation in water-rich soft rock. Appl. Sci. 2021, 11, 8906. [Google Scholar] [CrossRef]
- Xu, Z.; Lin, P.; Xing, H.; Pan, D.; Huang, X. Hydro-mechanical Coupling Response Behaviors in Tunnel Subjected to a Water-Filled Karst Cave. Rock Mech. Rock Eng. 2021, 54, 3737–3756. [Google Scholar] [CrossRef]
- Zhang, Q.-Y.; Ren, M.-Y.; Duan, K.; Wang, W.-S.; Gao, Q.; Lin, H.-X.; Xiang, W.; Jiao, Y.-Y. Geo-mechanical model test on the collaborative bearing effect of rock-support system for deep tunnel in complicated rock strata. Tunn. Undergr. Space Technol. 2019, 91, 103001. [Google Scholar] [CrossRef]
- Zhu, W.; Li, Y.; Li, S.; Shugang, W.; Qianbing, Z. Quasi-three-dimensional physical model tests on a cavern complex under high in-situ stresses. Int. J. Rock Mech. Min. Sci. 2011, 48, 199–209. [Google Scholar]






| Name | Bulk Modulus /MPa | Shear Modulus /MPa | Cohesion /kPa | Friction Angle /° | Density /kg/m3 |
|---|---|---|---|---|---|
| Fragment stone | 43.01 | 16.49 | 0 | 30 | 2300 |
| Limestone | 4340 | 2360 | 800 | 60 | 2650 |
| Engineering spoil (compaction state) | 21.52 | 10.63 | 20 | 12 | 2140 |
| Shotcrete | 29,500 | 12,300 | - | - | 2400 |
| Second lining | 35,000 | 13,500 | −900 s | - | 2400 |
| Grouting reinforced engineering spoil | 150 | 75 | 110 | 38- | 2800 |
| C30 Shotcrete Thickness /cm | Grouted Bolt (arch/3.0 m) | Grouted Bolt (Side Wall /5.0 m) | Steel Mesh | Steel Arch | ||||
|---|---|---|---|---|---|---|---|---|
| Model | Space /(m × m) | Model | Space /(m × m) | Model | Space /(m × m) | Model | Space /m | |
| 20 | Φ32 | 1.2 × 1.0 | Φ32 | 1.2 × 1.0 | Φ6 | 0.2 × 0.2 | I20b | 1.0 |
| Index | Value | Index | Value |
|---|---|---|---|
| Stop pressure | 2–3 MPa | Backfill material ratio | Water: Cement: Soil = 1.6:1:1 |
| Penetration distance | 1.5–2.1 m | Backfilled rubble | 5–10 mm |
| Grouting flow | 77–165 L/min | Back step distance | 1 m |
| Single slurry ratio (SAC) | W:C = (0.6–1):1 | Cement-sodium silicate slurry (C-S) | W:C:S = (0.6–1):1:1 |
| Mileage Number | Drill Length/m | Actual Grouted Hole Length/m | Grouting Volume/m3 | Grouting Volume Per Meter | |||
|---|---|---|---|---|---|---|---|
| SAC | C-S | Total | SAC | Total | |||
| D3K279 + 872 | 175 | 175 | 240.79 | 0 | 240.79 | 1.38 | 1.38 |
| D3K279 + 875.5 | 177 | 177 | 213.03 | 0 | 213.03 | 1.20 | 1.20 |
| D3K279 + 878 | 176.5 | 176.5 | 216.45 | 0 | 216.45 | 1.23 | 1.23 |
| D3K279 + 880.5 | 176.5 | 176.5 | 230.92 | 0 | 230.92 | 1.31 | 1.31 |
| D3K279 + 883 | 209.5 | 209.5 | 229.21 | 0 | 229.21 | 1.09 | 1.09 |
| D3K279 + 885.5 | 211 | 211 | 249.47 | 0 | 249.47 | 1.18 | 1.18 |
| D3K279 + 888 | 251.7 | 217.3 | 249.21 | 0 | 249.21 | 1.15 | 1.15 |
| D3K279 + 890.5 | 250.5 | 188.5 | 202.63 | 0 | 202.63 | 1.07 | 1.07 |
| D3K279 + 893 | 227.5 | 205 | 229.08 | 0 | 229.08 | 1.12 | 1.12 |
| D3K279 + 895.5 | 229 | 198 | 316.32 | 0 | 316.32 | 1.60 | 1.60 |
| D3K279 + 898 | 223 | 211 | 198.29 | 0 | 198.29 | 0.94 | 0.94 |
| D3K279 + 900.5 | 225.5 | 210.5 | 191.84 | 0 | 191.84 | 0.91 | 0.91 |
| D3K279 + 903 | 247 | 231 | 141.71 | 76.50 | 218.21 | 0.61 | 0.94 |
| D3K279 + 905.5 | 246.5 | 245.5 | 208.03 | 23.10 | 231.13 | 0.85 | 0.94 |
| D3K279 + 908 | 249.5 | 247.5 | 179.21 | 146.32 | 325.53 | 0.72 | 1.32 |
| D3K279 + 910.5 | 265 | 265 | 259.68 | 12.58 | 272.26 | 0.98 | 1.03 |
| D3K279 + 913 | 268 | 256 | 200.45 | 60.55 | 261.00 | 0.78 | 1.02 |
| D3K279 + 915.5 | 269.5 | 247 | 200.66 | 70.76 | 271.42 | 0.81 | 1.10 |
| D3K279 + 918 | 160.5 | 160.5 | 0 | 189.92 | 189.92 | 0 | 1.18 |
| D3K279 + 920.5 | 158 | 158 | 57.89 | 115.37 | 173.26 | 0.37 | 1.10 |
| D3K279 + 923 | 160.5 | 144.5 | 81.84 | 84.36 | 166.20 | 0.57 | 1.15 |
| D3K279 + 925.5 | 160.5 | 160.5 | 0 | 194.29 | 194.29 | 0 | 1.21 |
| D3K279 + 928 | 139 | 139 | 80.26 | 75.93 | 156.19 | 0.58 | 1.12 |
| D3K279 + 930.5 | 152 | 152 | 94.21 | 65.18 | 159.39 | 0.62 | 1.05 |
| D3K279 + 933 | 135 | 135 | 66.45 | 68.80 | 135.25 | 0.49 | 1.00 |
| D3K279 + 935.5 | 135 | 135 | 11.84 | 140.31 | 152.15 | 0.09 | 1.13 |
| Grouting Section | Drill Length/m | Actual Grouted Hole Length/m | Grouting Quantity/m3 | Grouting Quantity Per Meter/m2 | |||
|---|---|---|---|---|---|---|---|
| SAC | C-S | Total | SAC | Total | |||
| D3K279 + 903 | 131 | 131 | 85.53 | 48.73 | 134.26 | 0.65 | 1.02 |
| D3K279 + 905.5 | 109 | 100.5 | 87.76 | 12.53 | 100.29 | 0.87 | 1.00 |
| D3K279 + 908 | 130.5 | 124 | 92.89 | 31.11 | 124.00 | 0.75 | 1.00 |
| D3K279 + 910.5 | 131.5 | 129.5 | 78.03 | 56.84 | 134.87 | 0.60 | 1.04 |
| D3K279 + 913 | 316.8 | 306.8 | 204.61 | 296.90 | 501.51 | 0.67 | 1.63 |
| D3K279 + 915.5 | 94 | 83 | 95.39 | 49.85 | 145.24 | 1.15 | 1.75 |
| D3K279 + 918 | 88.8 | 102 | 167.11 | 60.05 | 227.16 | 1.64 | 2.23 |
| D3K279 + 920.5 | 87 | 76 | 111.18 | 49.11 | 160.29 | 1.46 | 2.11 |
| D3K279 + 923 | 87 | 75 | 142.11 | 50.10 | 192.21 | 1.89 | 2.56 |
| D3K279 + 925.5 | 134.8 | 73.3 | 105.26 | 67.5 | 172.76 | 1.44 | 2.36 |
| D3K279 + 928 | 132.7 | 82.6 | 78.62 | 144.12 | 222.74 | 0.95 | 2.70 |
| D3K279 + 930.5 | 90.7 | 79.6 | 157.89 | 23.43 | 181.32 | 1.98 | 2.28 |
| D3K279 + 933 | 76.7 | 81.1 | 186.18 | 51.21 | 237.39 | 2.30 | 2.93 |
| D3K279 + 935.5 | 137 | 82 | 121.38 | 49.03 | 170.41 | 1.48 | 2.08 |
| D3K279 + 938 | 134.2 | 78.5 | 216.84 | 65.08 | 283.92 | 2.76 | 3.62 |
| D3K279 + 940.5 | 153.3 | 65 | 108.55 | 29.42 | 137.97 | 1.67 | 2.12 |
| D3K279 + 943 | 140 | 70 | 160.00 | 44.24 | 204.24 | 2.29 | 2.92 |
| D3K279 + 945.5 | 126 | 40 | 38.16 | 67.76 | 105.92 | 0.95 | 2.65 |
| D3K279 + 948 | 127 | 44 | 63.55 | 21.79 | 85.34 | 1.44 | 1.94 |
| D3K279 + 950.5 | 135.5 | 39 | 36.84 | 40.69 | 77.53 | 0.94 | 1.99 |
| D3K279 + 953 | 135.5 | 54.5 | 85.53 | 0 | 85.53 | 1.57 | 1.57 |
| D3K279 + 955.5 | 391 | 136.5 | 198.30 | 90.63 | 288.93 | 1.45 | 2.12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, P.; Peng, F.; Sun, Z.; Zhang, D. Grouting for Tunnel Stability Control and Inadequate Grouting Section Recognition: A Case Study of Countermeasure of Giant Karst Cave. Appl. Sci. 2022, 12, 11895. https://doi.org/10.3390/app122311895
Peng P, Peng F, Sun Z, Zhang D. Grouting for Tunnel Stability Control and Inadequate Grouting Section Recognition: A Case Study of Countermeasure of Giant Karst Cave. Applied Sciences. 2022; 12(23):11895. https://doi.org/10.3390/app122311895
Chicago/Turabian StylePeng, Peng, Feng Peng, Zhenyu Sun, and Dingli Zhang. 2022. "Grouting for Tunnel Stability Control and Inadequate Grouting Section Recognition: A Case Study of Countermeasure of Giant Karst Cave" Applied Sciences 12, no. 23: 11895. https://doi.org/10.3390/app122311895
APA StylePeng, P., Peng, F., Sun, Z., & Zhang, D. (2022). Grouting for Tunnel Stability Control and Inadequate Grouting Section Recognition: A Case Study of Countermeasure of Giant Karst Cave. Applied Sciences, 12(23), 11895. https://doi.org/10.3390/app122311895







