Reflection Analysis of Impermeable Slopes under Bimodal Sea Conditions
Abstract
:1. Introduction
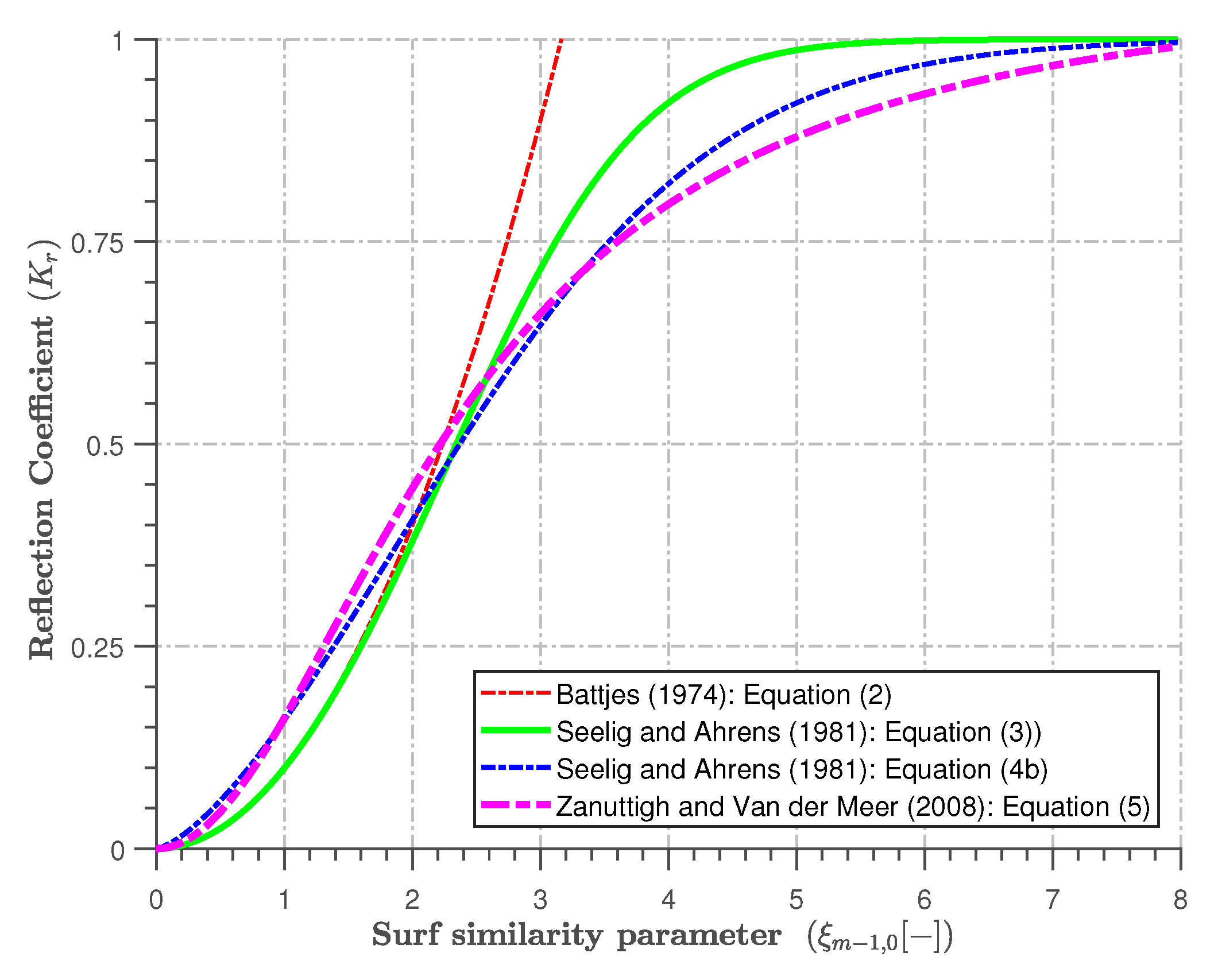
2. Reflection Characteristics of Smooth Impermeable Slopes
3. Material and Methods
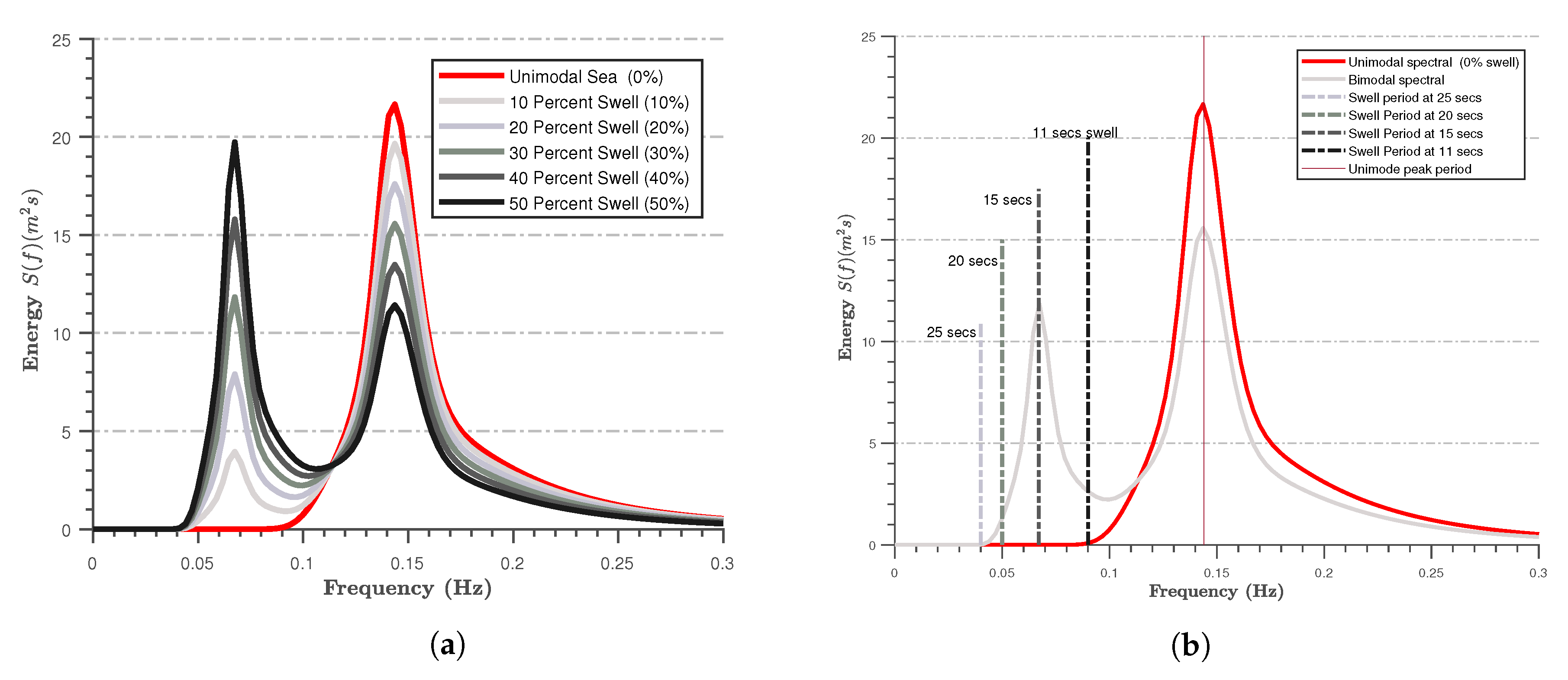
3.1. Design of Model Tests
3.2. Reflection Analysis
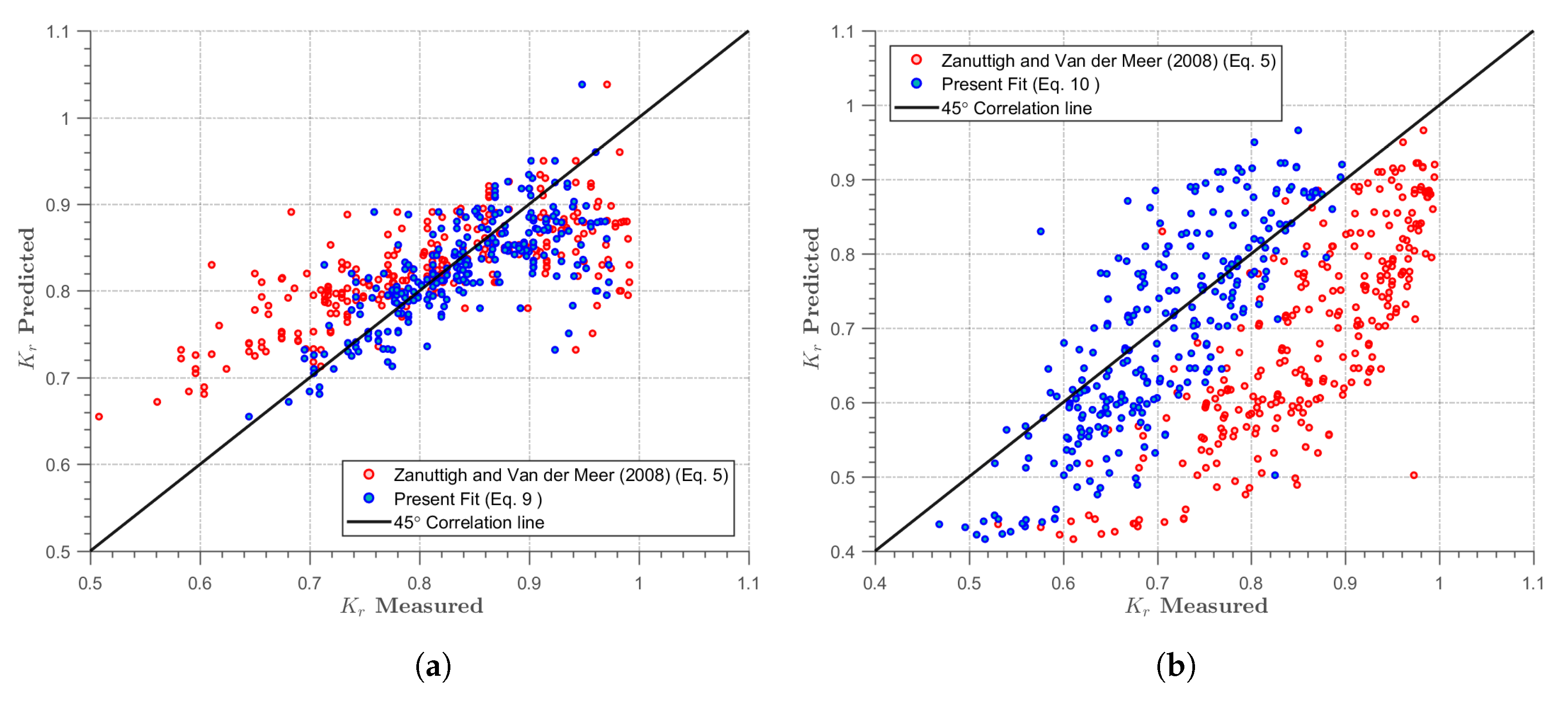
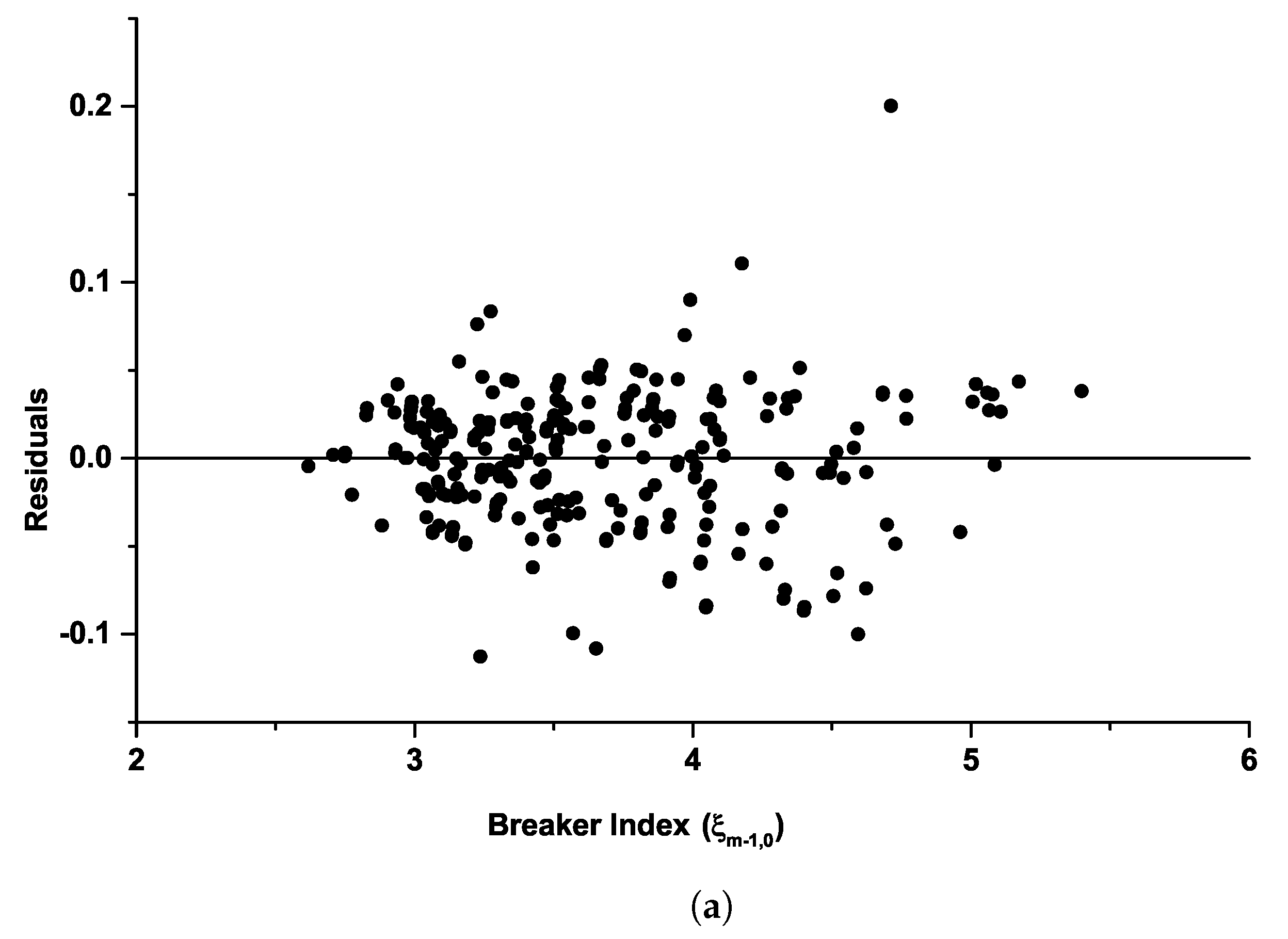
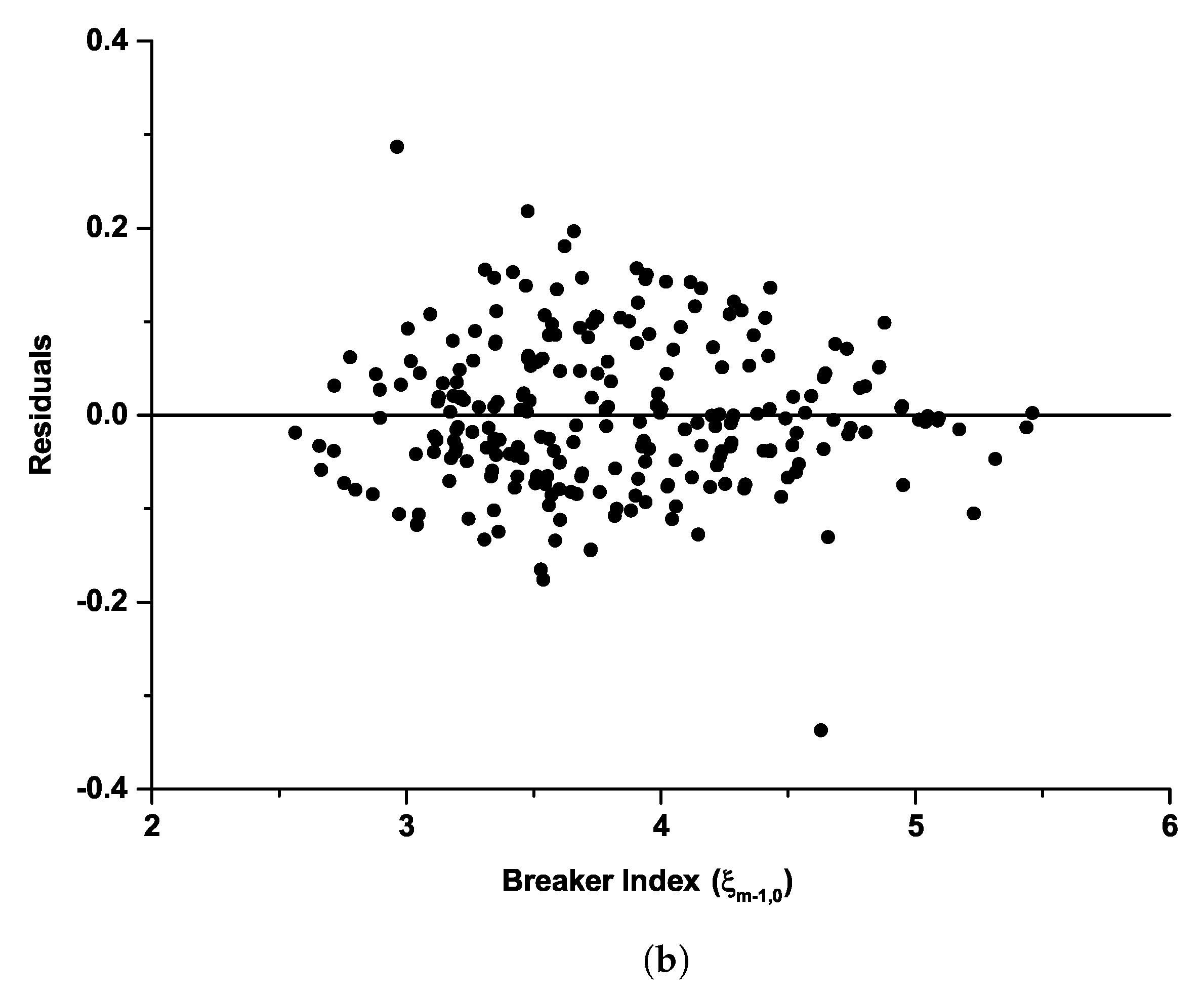
3.3. Estimation of Reflection Parameters
4. Results and Discussions
4.1. Influence of Wall Slope on Reflection Characteristics
4.2. Influence of Water Depth Variations
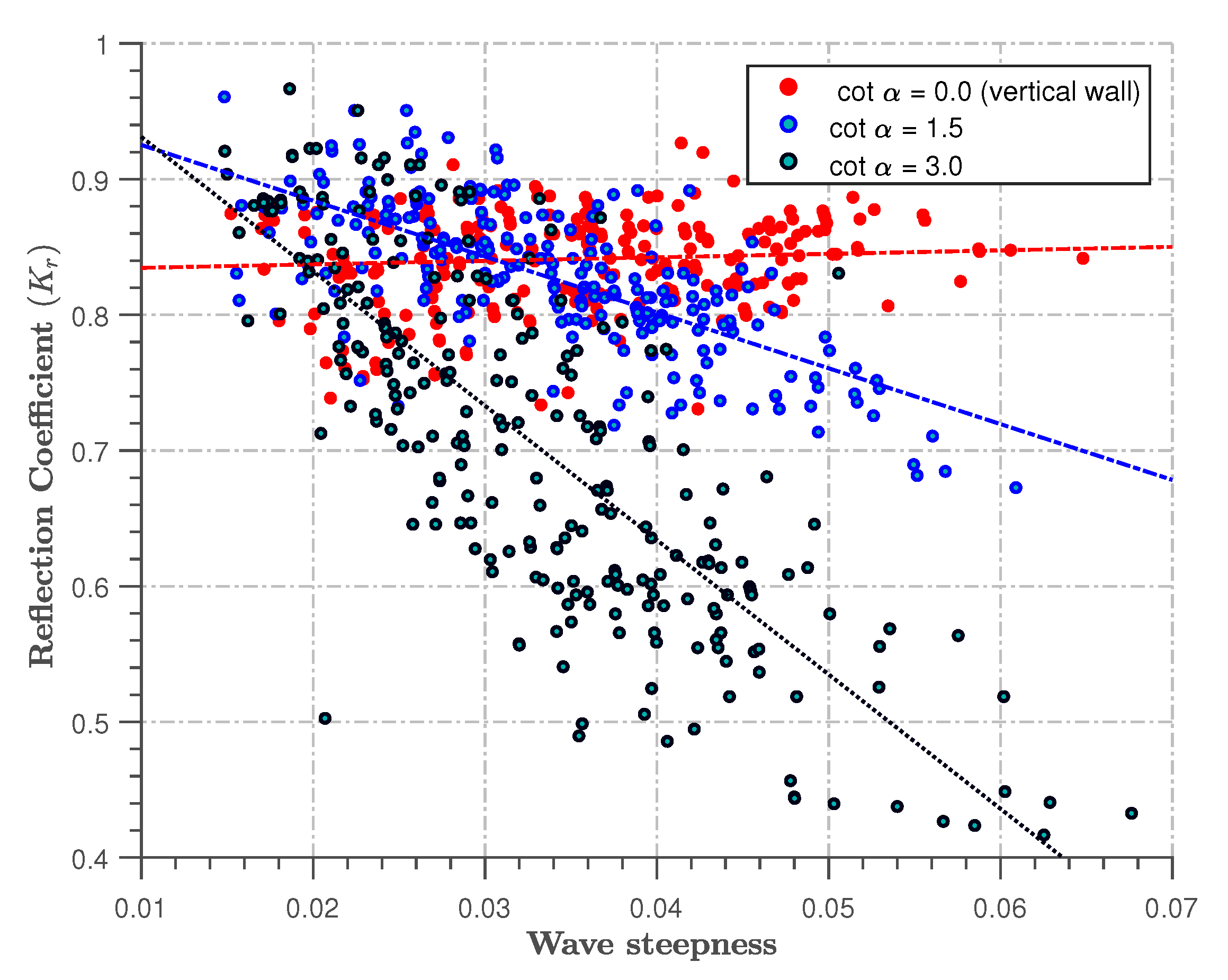
4.3. Influence of Wave Steepness
4.4. Effects of the Crest Freeboard
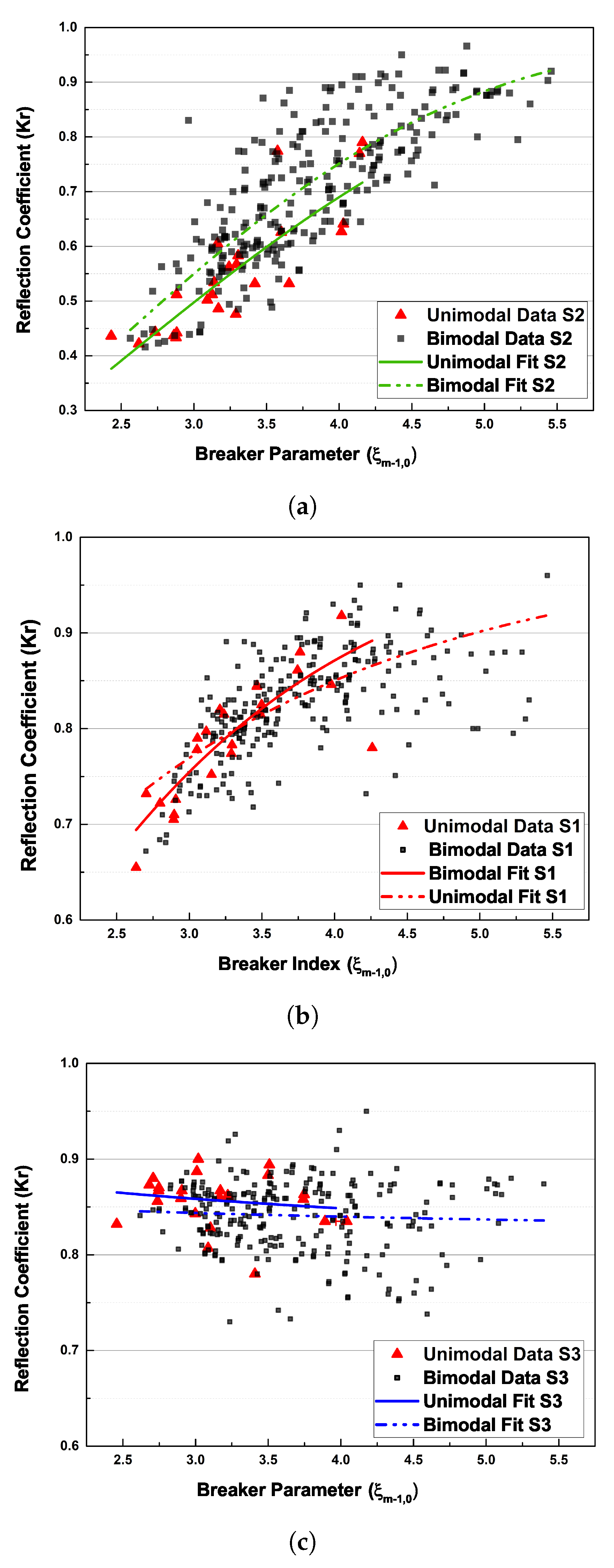
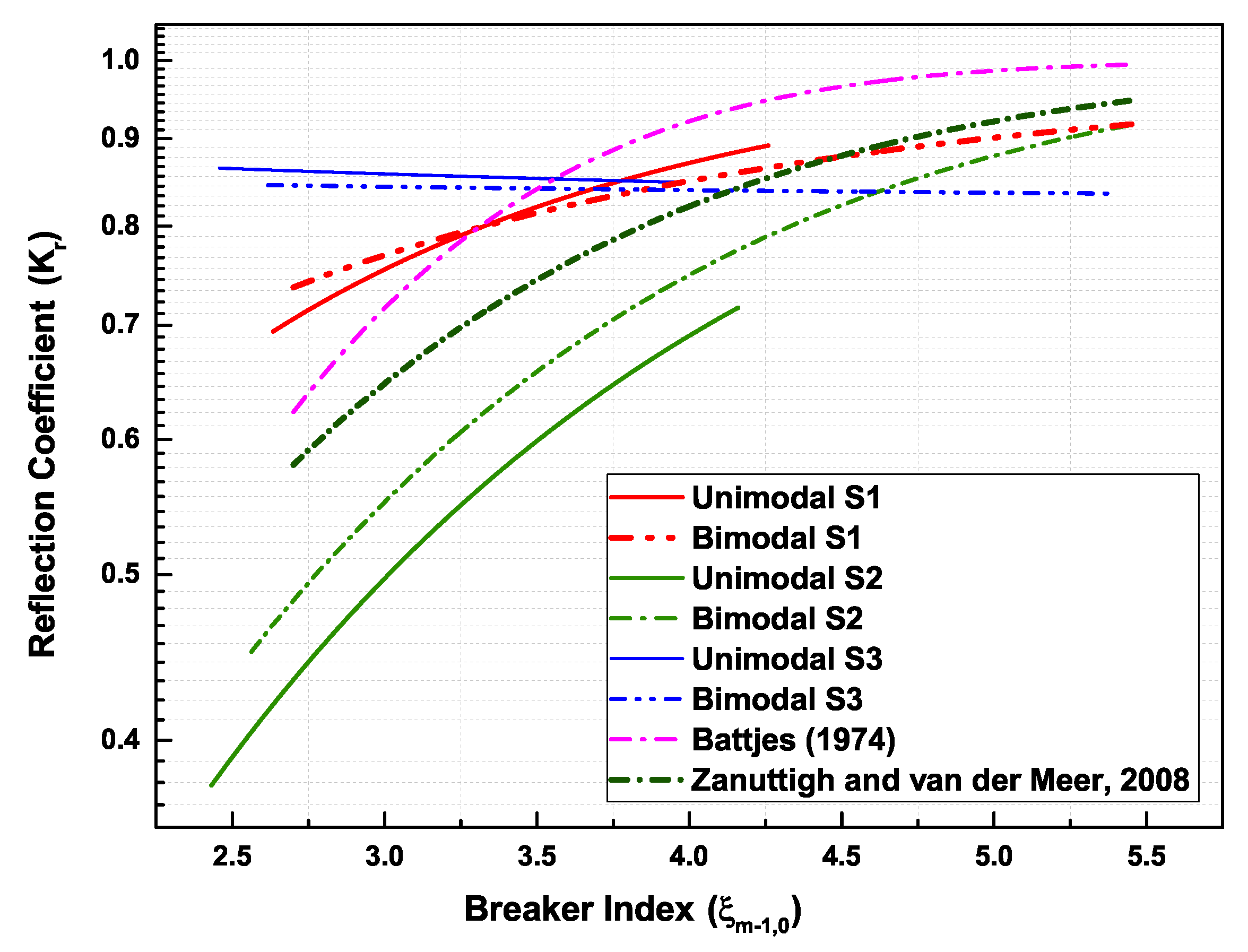
5. Reflection Coefficients of Steep Slopes Under Bimodal Waves
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| The structure slope | |
| Breaker index or surf similarity parameter | |
| Breaker Index with based on | |
| Breaker Index with based on | |
| Peak period | |
| FFT | Fast Fourier Transform |
| g | Acceleration due to gravity |
| h | Water depth |
| H | Wave height |
| Significant wave height | |
| IFFT | Inverse Fast Fourier Transform |
| H | Significant wave height |
| Jonswap | Joint North Sea Wave Project |
| Reflection coefficient | |
| The linear wave length | |
| Crest freeboard | |
| SSER | Sea-swell energy ratio |
| The spectra wave period | |
| Peak periods of swell wave at 11 s | |
| Peak periods of swell wave at 15 s | |
| Peak periods of swell wave at 20 s | |
| Peak periods of swell wave at 25 s | |
| Peak period of wind wave | |
| UK | United Kingdom |
| Wave steepness derived from | |
| Reflection coefficient |
References
- Andersen, T.L.; Frigaard, P.; Burcharth, H.F. Lecture Notes for the Course in Water Wave Mechanics; Department of Civil Engineering, Aalborg University: Aalborg, Denmark, 2014. [Google Scholar]
- Zanuttigh, B.; van Der Meer, J.W. Wave reflection from coastal structures in design conditions. Coast. Eng. 2008, 55, 771–779. [Google Scholar] [CrossRef]
- Hawkes, P.J.; Coates, T.; Jones, R.J. Impacts of Bimodal Seas on Beaches, Hydraulic Research Wallingford. Hydraul. Res. Wallingford 1998, 265, 80. [Google Scholar]
- Thompson, A.D.; Reeve, D.E.; Karunarathna, H. Modelling extreme wave overtopping at Aberystwyth Promenade. Water 2017, 9, 663. [Google Scholar] [CrossRef] [Green Version]
- Polidoro, A.; Pullen, T.; Eade, J. Gravel beach profile response allowing for bimodal sea states. Proc. Inst. Civ. Eng. Marit. Eng. 2018, 171, 145–146. [Google Scholar] [CrossRef]
- Miche, M. Le pouvoir reflêchissant des ouvrages maritimes exposés à l’action de la houl. Annales de Ponts et Chaussées 1951, 121, 285–319. [Google Scholar]
- Ursell, J.; Dean, R.G.; Yu, Y. Forced small amplitude water waves: a comparison of theory and experiment. J. Fluid Mech. 1960, 7, 33–52. [Google Scholar] [CrossRef]
- Battjes, J.A. Surf similarity. In Proceedings of the 14th International Conference on Coastal Engineering, ASCE, Copenhagen, Denmark, 24–28 June 1974; pp. 460–480. [Google Scholar]
- Iribarren, C.; Nogales, C. Protection des Ports. In Proceedings of the XVIIth International Naval Congress, Lisbon, Portugal, 1 January 1949; Volume 4, pp. 31–80. [Google Scholar]
- Seelig, W.N. Two-Dimensional Tests of Wave Transmission and Reflection Characteristics of Laboratory Breakwaters; Technical Report; Coastal Engineering Research Centre: Fort Belvoir, VA, USA, 1980. [Google Scholar]
- Seelig, W.N.; Ahrens, J.P. Estimation of Wave Reflection and Energy Dissipation Coefficients for Beaches, Revetments, and Breakwaters; Technical Paper No. 81-1; US Army Corps of Engineers: Washington, DC, USA, 1981; pp. 1–41. [Google Scholar]
- Davidson, M.; Bird, P.; Bullock, G.; Huntley, D. A new non-dimensional number for the analysis of wave reflection from rubble mound breakwaters. Coast. Eng. 1996, 28, 93–120. [Google Scholar] [CrossRef]
- Gunbak, A. The Stability of Rubble Mound Breakwaters in Relation to Wave Breaking and Run-Down Characteristics and to the Tan; Div. Port and Ocean Eng., The Norwegian Institute of Technology: Trondheim, Norway, 1976. [Google Scholar]
- DeBok, D.H. A Large Scale Model Study of Placed Stone Breakwaters. Master’s Thesis, Oregon State University, Corvallis, OR, USA, 1977. [Google Scholar]
- Manual, S.P. Department of the Army; US Army Corps of Engineers: Washington, DC, USA, 1984. [Google Scholar]
- Numata, A. Laboratory formulation for transmission and reflection at permeable breakwaters of artificial blocks. Coast. Eng. Jpn. 1976, 19, 47–58. [Google Scholar] [CrossRef]
- Gimenez-Curto, L. Behaviour of Rubble Mound Breakwaters Under Wave Action. Ph.D. Thesis, University of Santander, Cantabria, Spain, 1979. [Google Scholar]
- Losada, M.A.; Gimenez-Curto, L.A. An approximation to the failure probability of maritime structures under a sea state. Coast. Eng. 1981, 5, 147–157. [Google Scholar] [CrossRef]
- Postma, G. Wave Reflection From Rock Slopes under Random Wave Attack. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 1989. [Google Scholar]
- Allsop, N.; Channell, A. Wave Reflections in Harbours: The Reflection Performance of Wave Screens; Report OD; HR Wallingford: Wallingford, UK, 1988; Volume 102. [Google Scholar]
- Van der Meer, J.W. Conceptual design of rubble mound breakwaters. In Advances In Coastal And Ocean Engineering: (Volume 1); World Scientific: Singapore, 1995; pp. 221–315. [Google Scholar]
- Oumeraci, H.; Partenscky, H. Wave-induced pore pressure in rubble mound breakwaters. In Coastal Engineering; ASCE: Delft, The Netherlands, 1991; pp. 1334–1347. [Google Scholar]
- Muttray, M.; Oumeraci, H.; Zimmermann, C.; Partenscky, H. Wave energy dissipation on and in rubble mound structures. In Coastal Engineering; ASCE: Venice, Italy, 1993; pp. 1434–1447. [Google Scholar]
- Allsop, N.; Hettiarachchi, S. Reflections from Coastal Structures. Proceedings of the 21st International Conference on Coastal Engineering, Torremolinos, Spain, 20–25 June 1988; Edge, B.L., Ed.; American Society of Civil Engineers: New York, NY, USA, 1988; pp. 782–794. [Google Scholar]
- Shimada, A.; Fujimoto, T.; Saito, S.; Sakakiyama, T.; Hirakuchi, H. Scale effects on stability and wave reflection regarding armor units. In Coastal Engineering; ASCE: New York, NY, USA, 1987; pp. 2238–2252. [Google Scholar]
- Neelamani, S.; Sandhya, N. Wave reflection, run-up, run-down and pressures on plane, dentated and serrated seawalls. Coast. Eng. J. 2004, 46, 141–169. [Google Scholar] [CrossRef]
- Wang, B.; van der Meer, J.W.; Otta, A.K.; Chadwick, A.J.; Horrillo-Caraballo, J. Reflection of obliquely incident waves at low-crested structures. In Coastal Dynamics 2005: State of the Practice; Elsevier: Amsterdam, The Netherlands, 2006; pp. 1–12. [Google Scholar]
- Zanuttigh, B.; Andersen, T.L. Wave reflection in 3D conditions. Coast. Eng. 2010, 57, 531–538. [Google Scholar] [CrossRef]
- Li, Y.; Dong, G.; Liu, H.; Sun, D. The reflection of oblique incident waves by breakwaters with double-layered perforated wall. Coast. Eng. 2003, 50, 47–60. [Google Scholar] [CrossRef]
- Koraim, A.; Rageh, O. Hydrodynamic performance of vertical porous structures under regular waves. China Ocean. Eng. 2013, 27, 451–468. [Google Scholar] [CrossRef]
- Young, D.M.; Testik, F.Y. Wave reflection by submerged vertical and semicircular breakwaters. Ocean. Eng. 2011, 38, 1269–1276. [Google Scholar] [CrossRef]
- Koraim, A.S.; Heikal, E.M.; Zaid, A.A. Hydrodynamic characteristics of porous seawall protected by submerged breakwater. Applied Ocean Research 2014, 46, 1–14. [Google Scholar] [CrossRef]
- Lee, J.I.; Shin, S. Experimental study on the wave reflection of partially perforated wall caissons with single and double chambers. Ocean. Eng. 2014, 91, 1–10. [Google Scholar] [CrossRef]
- Esmaeili, M.; Rahbani, M.; Khaniki, A.K. Experimental investigating on the reflected waves from the caisson-type vertical porous seawalls. Acta Oceanol. Sin. 2019, 38, 117–123. [Google Scholar] [CrossRef]
- Orimoloye, S.; Karunarathna, H.; Reeve, D.E. Effects of Swell on Wave Height Distribution of Energy-Conserved Bimodal Seas. J. Mar. Sci. Eng. 2019, 7, 79. [Google Scholar] [CrossRef] [Green Version]
- Allsop, N.; Bruce, T.; Pearson, J.; Besley, P. Wave overtopping at vertical and steep seawalls. Mar. Eng. 2005, 158, 103–114. [Google Scholar] [CrossRef] [Green Version]
- Schäffer, H.A. Second-order wavemaker theory for irregular waves. Ocean. Eng. 1996, 23, 47–88. [Google Scholar] [CrossRef]
- Zelt, J.; Skjelbreia, J.E. Estimating incident and reflected wave fields using an arbitrary number of wave gauges. In Proceedings of the 23rd ICCE, Venice, Italy, 4–9 October 1992; pp. 777–788. [Google Scholar]
- Orimoloye, S.; Horrillo-Caraballo, J.; Karunarathna, H.; Reeve, D.E. Modelling wave overtopping of steep impermeable structures under bimodal sea conditions. In Proceedings of the Coastal Structures, Hannover, Germany, 30 September–2 October 2019. [Google Scholar]
- Mansard, E.P.; Funke, E. The measurement of incident and reflected spectra using a least squares method. In Proceedings of the 17th Coastal Engineering Conference, Sydney, Australia, 23–28 March 1980; pp. 154–172. [Google Scholar]
- Reeve, D.; Chadwick, A.; Fleming, C. Coastal Engineering: Processes, Theory and Design Practice; Spon: London, UK, 2015; 518p. [Google Scholar]
- Owen, M. Design of Seawalls Allowing for Wave Overtopping; HR Report number Ex 924; Hydraulic Station: Wallingford, UK, 1988. [Google Scholar]
- Van Gent, M.R. The modelling of wave action on and in coastal structures. Coast. Eng. 1994, 22, 311–339. [Google Scholar] [CrossRef] [Green Version]
- Nassar, K.; Negm, A. Determination of optimum wave reflection of seawalls via experimental modeling. In Scour and Erosion: Proceedings of the 8th International Conference on Scour and Erosion, Oxford, UK, 12–15 September 2016; CRC Press: Boca Raton, FL, USA, 2016; p. 255. [Google Scholar]
- Negm, A.; Nassar, K. Determination of Wave Reflection Formulae for Vertical and Sloped Seawalls Via Experimental Modelling. Procedia Eng. 2016, 154, 919–927. [Google Scholar] [CrossRef] [Green Version]
- Goda, Y. Random Seas and Design of Maritime Structures; World Scientific Publishing Co. Pte. Ltd.: Singapore, 2010; p. 708. [Google Scholar]





| Test No | (m) | (s) | (s) | (s) | (s) | (s) | h(m) | = 0.0 | = 1.5 | = 3.0 | No. of Tests |
|---|---|---|---|---|---|---|---|---|---|---|---|
| T001 | 0.125 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T002 | 0.125 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T003 | 0.125 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T004 | 0.125 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T005 | 0.1 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T006 | 0.125 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T007 | 0.1 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T008 | 0.125 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T009 | 0.1 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T010 | 0.1 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T011 | 0.125 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T012 | 0.125 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T013 | 0.075 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T014 | 0.075 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T015 | 0.075 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T016 | 0.075 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.65 | 0 | 1.5 | 3.0 | 13 |
| T017 | 0.1 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T018 | 0.1 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T019 | 0.1 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T020 | 0.1 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.7 | 0 | 1.5 | 3.0 | 13 |
| T021 | 0.075 | 1.11 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T022 | 0.075 | 1.26 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T023 | 0.075 | 1.42 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
| T024 | 0.075 | 1.58 | 1.74 | 2.37 | 3.16 | 3.95 | 0.6 | 0 | 1.5 | 3.0 | 13 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Orimoloye, S.; Karunarathna, H.; Reeve, D.E. Reflection Analysis of Impermeable Slopes under Bimodal Sea Conditions. J. Mar. Sci. Eng. 2020, 8, 133. https://doi.org/10.3390/jmse8020133
Orimoloye S, Karunarathna H, Reeve DE. Reflection Analysis of Impermeable Slopes under Bimodal Sea Conditions. Journal of Marine Science and Engineering. 2020; 8(2):133. https://doi.org/10.3390/jmse8020133
Chicago/Turabian StyleOrimoloye, Stephen, Harshinie Karunarathna, and Dominic E. Reeve. 2020. "Reflection Analysis of Impermeable Slopes under Bimodal Sea Conditions" Journal of Marine Science and Engineering 8, no. 2: 133. https://doi.org/10.3390/jmse8020133





