Novel Magnetic Field Modeling Method for a Low-Speed, High-Torque External-Rotor Permanent-Magnet Synchronous Motor
Abstract
:1. Introduction
2. Structure and Analytical Model
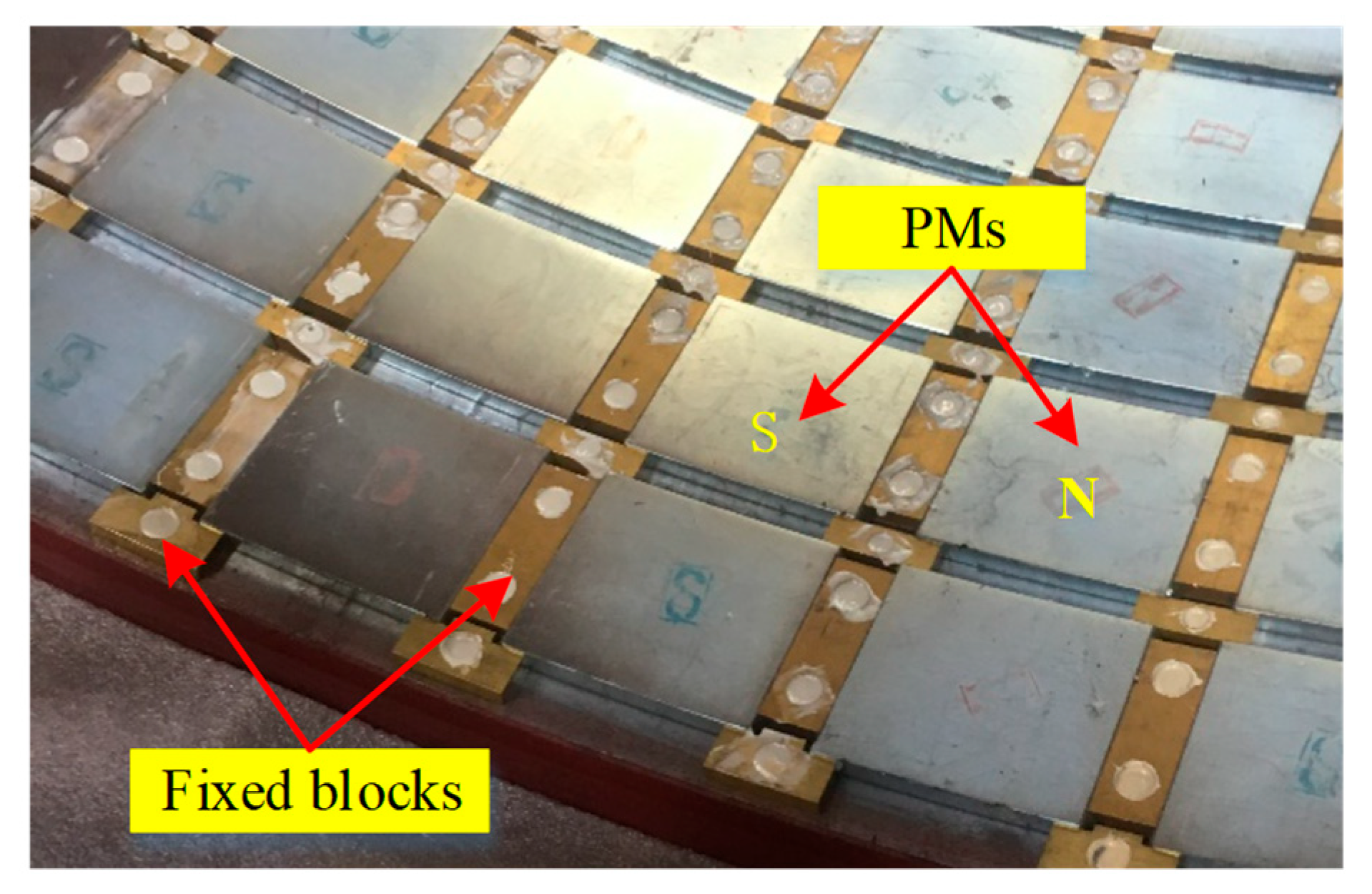
2.1. The Structural Composition of LHPMSM
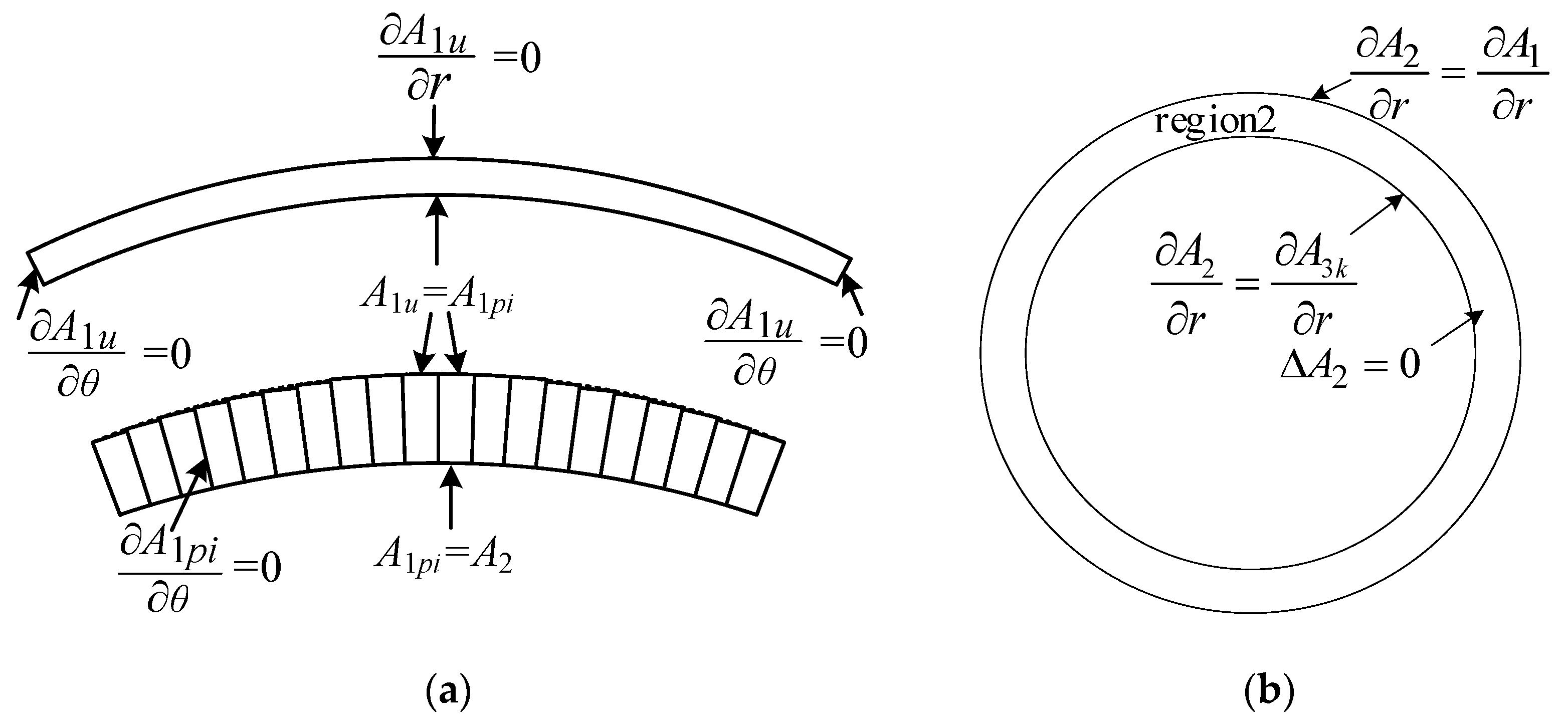
2.2. Analytical Model
3. Modeling and Analysis of Magnetic Fields
3.1. PM Region
3.2. The Other Regions
3.3. Cogging Torque
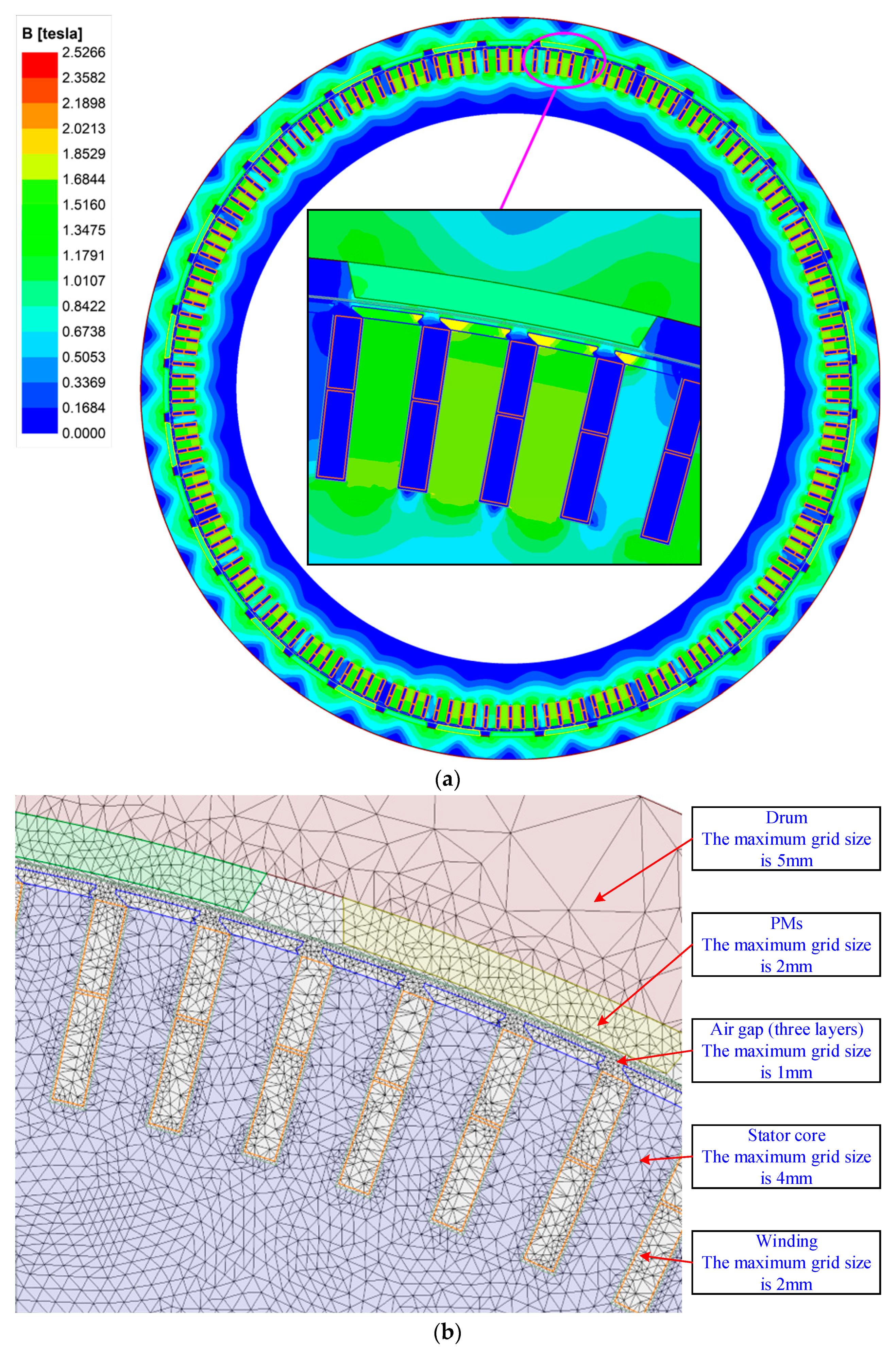
4. FEM Results and Comparative Analysis
- (1)
- Establish the FEM of the prototype based on the parameters shown in Table 1. Magnetostatic analysis is conducted to obtain the calculation results the air gap flux density under no-load conditions.
- (2)
- During the transient analysis process, set the prototype operating parameters shown in Table 2 and add ideal three-phase alternating current excitation. The radial and tangential air gap magnetic flux density and electromagnetic torque under load conditions can be obtained.
- (3)
- Still conducting the analysis in transient mode, removing the excitation source, modifying the speed to 1 r/s, and dividing the air gap domain into three layers with a maximum grid size of 1 mm, the distribution of the cogging torque can be obtained.
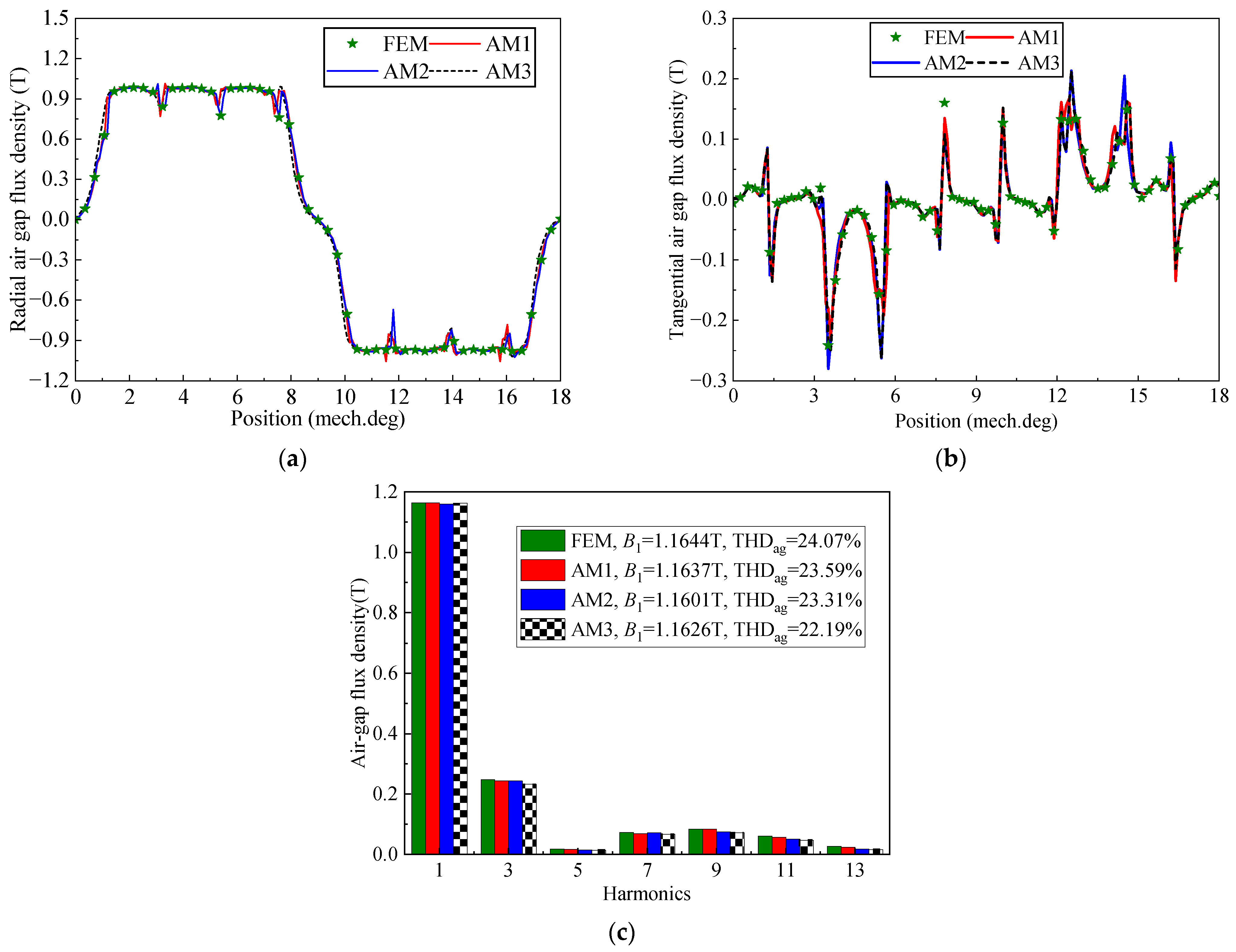
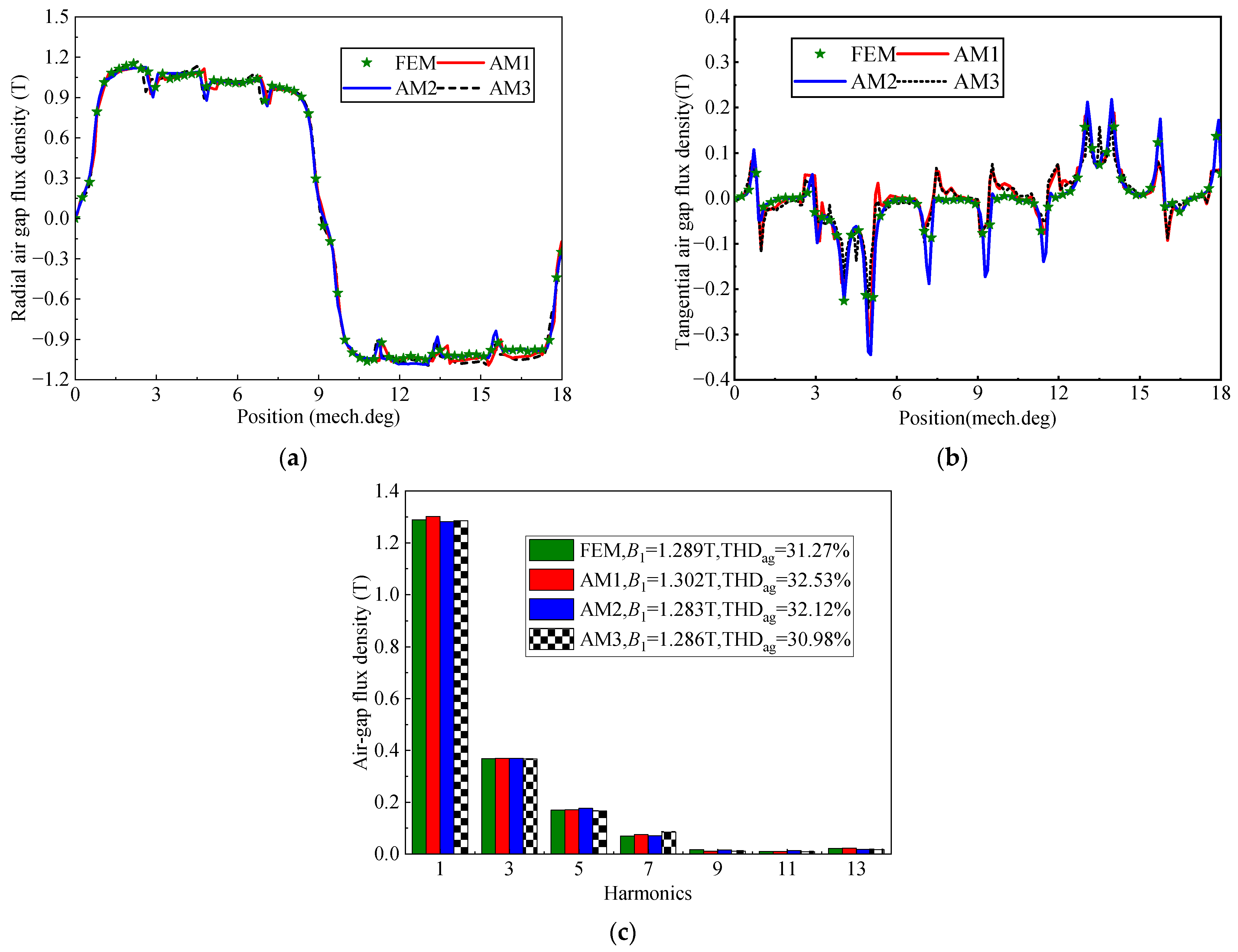
4.1. Air Gap Flux Density
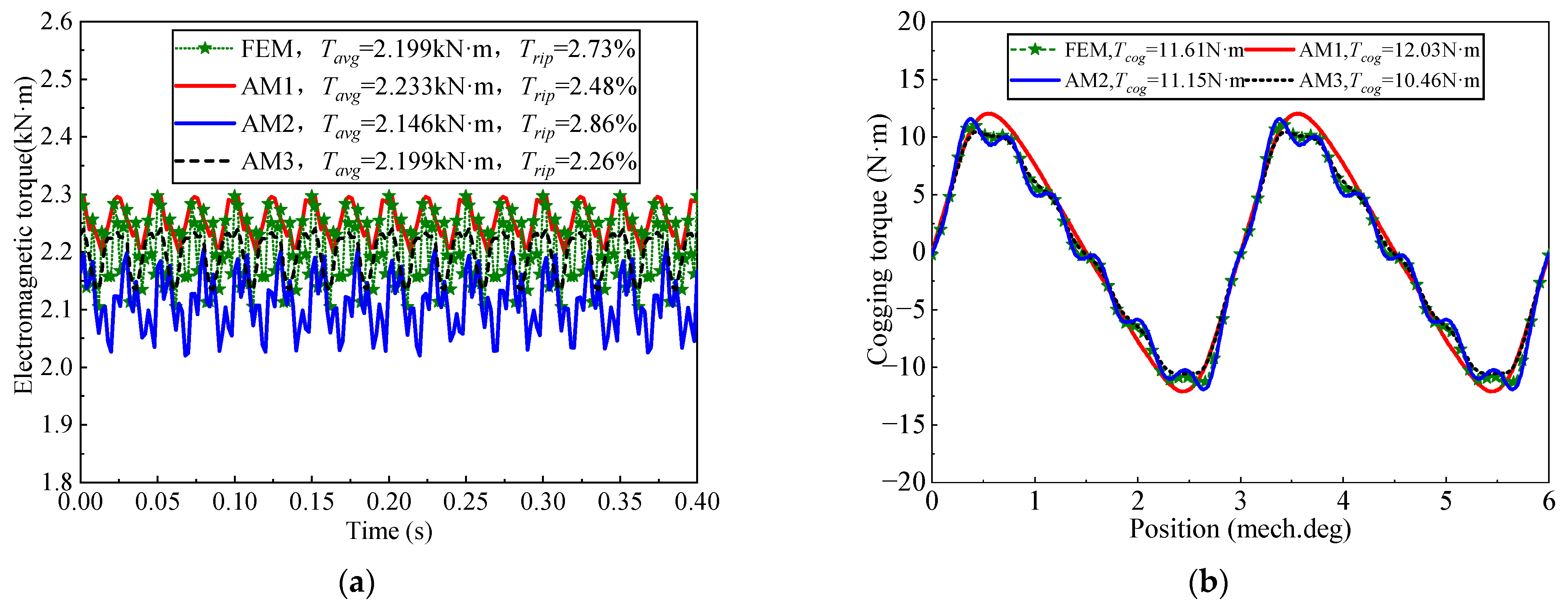
4.2. Torque Characteristics
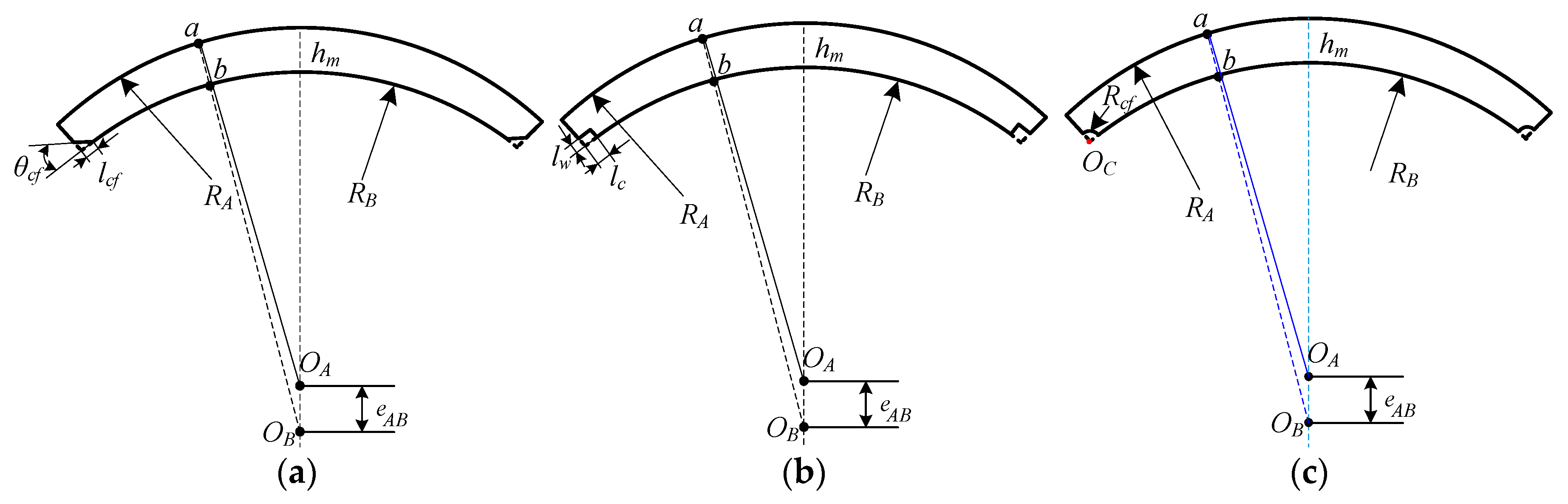
5. Edge Cutting Models for PM
6. Experimental Results
7. Conclusions
- (1)
- Compared to the ideal circular ring model (AM1) and segmented circular ring model (AM2), the dual-layer permanent magnet simplified model (AM3) shows a higher degree of agreement with the FEM. Its average electromagnetic torque is equal to the FEM calculation result, but the torque ripple is the lowest among the four models (the average electromagnetic torque values for AM1, AM2, AM3, and FEM are 2.233 kN·m, 2.146 kN·m, 2.199 kN·m, and 2.199 kN·m, respectively; the torque ripple values are 2.48%, 2.86%, 2.26%, and 2.73%, respectively). Moreover, the THD of the air gap flux density of AM3 is the lowest under both no-load and load conditions.
- (2)
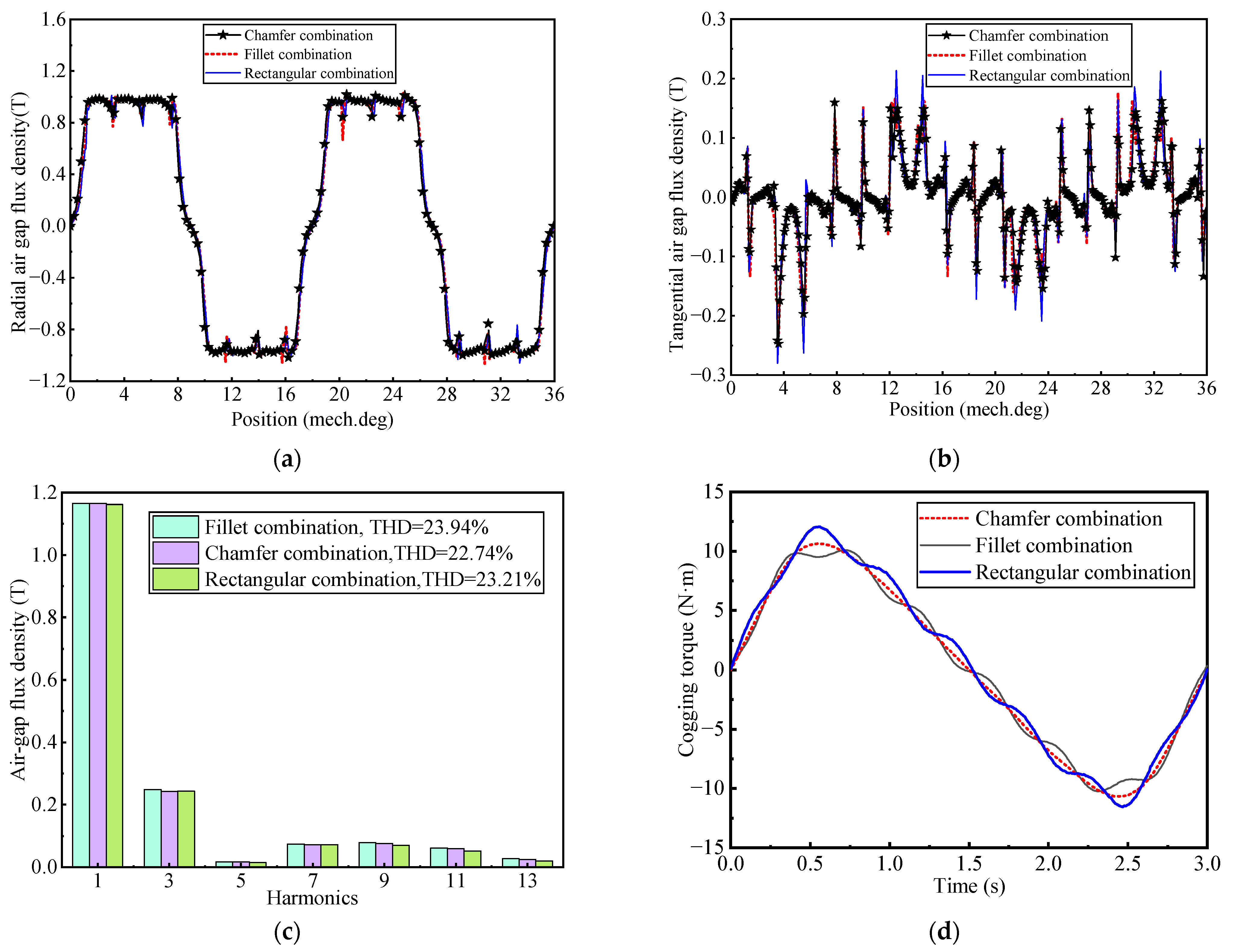
- Under the premise of the same amount of PM usage, the chamfered combination PM has superior electromagnetic characteristics. Specifically, the THD of the air gap flux density is 2% and 4% lower than the fillet and rectangular combinations, the average electromagnetic torque is higher by 1.9% and 4.8%, the torque ripple is lower by 11.7% and 2.9%, and the cogging torque is lower by 16.3% and 5.1%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Integration Constants
- (1)
- Region 1u and Region 1p
- (2)
- Region 1p and Region 2
References
- Lu, E.; Li, W.; Yang, X.F.; Xu, S.Y. Composite Sliding Mode Control of a Permanent Magnet Direct-Driven System for a Mining Scraper Conveyor. IEEE Access 2017, 5, 22399–22408. [Google Scholar] [CrossRef]
- Tlali, P.M.; Wang, R.J.; Gerber, S.; Botha, C.D.; Kamper, M.J. Design and Performance Comparison of Vernier and Conventional PM Synchronous Wind Generators. IEEE Trans. Ind. Appl. 2020, 56, 2570–2579. [Google Scholar] [CrossRef]
- Sheng, L.C.; Li, W.; Wang, Y.Q.; Fan, M.B.; Yang, X.F. Sensorless Control of a Shearer Short-Range Cutting Interior Permanent Magnet Synchronous Motor Based on a New Sliding Mode Observer. IEEE Access 2017, 5, 18439–18450. [Google Scholar] [CrossRef]
- Crider, J.M.; Sudhoff, S.D. An Inner Rotor Flux-Modulated Permanent Magnet Synchronous Machine for Low-Speed High-Torque Applications. IEEE Trans. Energy Convers. 2015, 30, 1247–1254. [Google Scholar] [CrossRef]
- Popescu, M.; Staton, D.A.; Jennings, S.; Schnüttgen, J.; Barucki, T. A Line-Fed Permanent-Magnet Motor Solution for Drum-Motor and Conveyor-Roller Applications. IEEE Trans. Ind. Appl. 2013, 49, 832–840. [Google Scholar] [CrossRef]
- Song, Y.D.; Zhang, Z.Y.; Yu, S.Y.; Zhang, F.G.; Zhang, Y. Analysis and Reduction of Cogging Torque in Direct-Drive External-Rotor Permanent Magnet Synchronous Motor for Belt Conveyor Application Cogging Torque Reduction of ERPMSM. IET Electr. Power Appl. 2021, 15, 668–680. [Google Scholar] [CrossRef]
- Kou, S.K.; Kou, Z.M.; Wu, J.; Wang, Y.D. Modeling and Simulation of a Novel Low-Speed High-Torque Permanent Magnet Synchronous Motor with Asymmetric Stator Slots. Machines 2022, 10, 1143. [Google Scholar] [CrossRef]
- Chu, W.Q.; Zhu, Z.Q. Reduction of On-Load Torque Ripples in Permanent Magnet Synchronous Machines by Improved Skewing. IEEE Trans. Magn. 2013, 49, 3822–3825. [Google Scholar] [CrossRef]
- Chu, W.Q.; Zhu, Z.Q. Investigation of Torque Ripples in Permanent Magnet Synchronous Machines with Skewing. IEEE Trans. Magn. 2013, 49, 1211–1220. [Google Scholar] [CrossRef]
- Wang, H.M.; Guan, P.J.; Liu, S.; Wu, S.; Shi, T.N.; Guo, L.Y. Modeling and Analyzing for Magnetic Field of Segmented Surface-Mounted PM Motors with Skewed Poles. J. Electr. Eng. Technol. 2022, 17, 1097–1109. [Google Scholar] [CrossRef]
- Li, Z.; Chen, J.H.; Zhang, C.; Liu, L.; Wang, X.Z. Cogging Torque Reduction in External-Rotor Permanent Magnet Torque Motor Based on Different Shape of Magnet. In Proceedings of the 2017 IEEE International Conference on Cybernetics and Intelligent Systems (CIS) and IEEE Conference on Robotics, Automation and Mechatronics (RAM), Ningbo, China, 19–21 November 2017; pp. 304–309. [Google Scholar]
- Chen, Z.F.; Xia, C.L.; Geng, Q.; Yan, Y. Modeling and Analyzing of Surface-Mounted Permanent-Magnet Synchronous Machines with Optimized Magnetic Pole Shape. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Xing, Z.Z.; Wang, X.H.; Zhao, W.L. Electromagnetic Vibration Reduction of Surface-Mounted Permanent Magnet Synchronous Motors Based on Eccentric Magnetic Poles. J. Electr. Eng. Technol. 2023, 18, 3603–3614. [Google Scholar] [CrossRef]
- Ding, Z.A.; Wu, X.Z.; Chen, C.T.; Yuan, X.B. Magnetic Field Analysis of Surface-Mounted Permanent Magnet Motors Based on an Improved Conformal Mapping Method. IEEE Trans. Ind. Appl. 2023, 59, 1689–1698. [Google Scholar] [CrossRef]
- Ni, Y.Y.; Chen, K.; Xiao, B.X.; Qian, W.; Wang, Q.J. Analytical Modeling of PM Electrical Machines with Eccentric Surface-Inset Halbach Magnets. IEEE Trans. Magn. 2021, 57, 1–9. [Google Scholar] [CrossRef]
- Kong, X.H.; Hua, Y.T.; Zhang, Z.R.; Wang, C.; Liu, Y. Analytical Modeling of High-Torque-Density Spoke-Type Permanent Magnet In-Wheel Motor Accounting for Rotor Slot and Eccentric Magnetic Pole. IEEE Trans. Transp. Electrif. 2021, 7, 2683–2693. [Google Scholar] [CrossRef]
- Wang, H.M.; Liu, S.; Wu, S.; Guo, L.Y.; Shi, T.N. Optimal Design of Permanent Magnet Structure to Reduce Unbalanced Magnetic Pull in Surface-Mounted Permanent-Magnet Motors. IEEE Access 2020, 8, 77811–77819. [Google Scholar] [CrossRef]
- Pang, Y.; Zhu, Z.Q.; Feng, Z.J. Cogging Torque in Cost-Effective Surface-Mounted Permanent-Magnet Machines. IEEE Trans. Magn. 2011, 47, 2269–2276. [Google Scholar] [CrossRef]
- Chai, F.; Liang, P.X.; Pei, Y.L.; Cheng, S.K. Magnet Shape Optimization of Surface-Mounted Permanent-Magnet Motors to Reduce Harmonic Iron Losses. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
- Wang, F.; Zhao, F.Q.; Ruan, Z.B.; Liu, Y.; Lai, H.K. The Modified Analytical Model of Sine Pole-Shaping Surface-Mounted PM Motor. IEEJ Trans. Electr. Electron. Eng. 2022, 17, 1346–1354. [Google Scholar] [CrossRef]
- Liu, C.; Xu, Y.; Zou, J.; Yu, G.; Zhuo, L. Systems, Permanent Magnet Shape Optimization Method for PMSM Air Gap Flux Density Harmonics Reduction. CES Trans. Electr. Mach. Syst. 2021, 5, 284–290. [Google Scholar] [CrossRef]
- Wang, K.; Zhu, Z.Q.; Ombach, G. Torque Enhancement of Surface-Mounted Permanent Magnet Machine Using Third-Order Harmonic. IEEE Trans. Magn. 2014, 50, 104–113. [Google Scholar] [CrossRef]
- Hu, P.F.; Wang, D. Analytical Average Torque Optimization Method of Parallel MagnetizedSurface-Mounted Permanent-MagnetMachine withThird-OrderHarmonic Shaping Method. IEEJ Trans. Electr. Electron. Eng. 2020, 15, 1377–1383. [Google Scholar] [CrossRef]
- Chabchoub, M.; Salah, I.B.; Krebs, G.; Neji, R.; Marchand, C. PMSM Cogging Torque Reduction: Comparison Between Different Shapes of Magnet. In Proceedings of the 2012 First International Conference on Renewable Energies and Vehicular Technology (REVET), Hammamet, Tunisia, 26–28 March 2012. [Google Scholar]
- Zhu, H.Q.; Xu, Y. Permanent Magnet Parameter Design and Performance Analysis of Bearingless Flux Switching Permanent Magnet Motor. IEEE Trans. Ind. Appl. 2021, 68, 4153–4163. [Google Scholar] [CrossRef]
- Li, J.; Wu, X.; Chen, C. Analytical Calculation of Air Gap Magnetic Field for Permanent Magnet Machines Based on Pole Partition Processing. Proc. CSEE 2021, 41, 6390–6398. [Google Scholar]
- Wang, K.; Sun, H.; Zhu, S.; Liu, C. Airgap Magnetic Field Reconstruction for Torque Ripple Mitigation of Permanent Magnet Machines. Proc. CSEE 2023, 43, 2563–2572. [Google Scholar]
- Yu, Z.Y.; Li, Y.; Jing, Y.T.; Du, J.M.; Wang, Z.C. Analytical model for magnetic field calculation of SPMSM with chamfered pole considering iron core saturation. IET Electr. Power Appl. 2020, 14, 1856–1864. [Google Scholar] [CrossRef]
- Hu, H.Z.; Zhao, J.; Liu, X.D.; Guo, Y.G. Magnetic Field and Force Calculation in Linear Permanent-Magnet Synchronous Machines Accounting for Longitudinal End Effect. IEEE Trans. Ind. Appl. 2016, 63, 7632–7643. [Google Scholar] [CrossRef]
- Hua, Y.; Liu, Y.; Pan, W.; Diao, X.; Zhu, H. Multi-objective Optimization Design of Bearingless Permanent Magnet Synchronous Motor Using Improved Particle Swarm Optimization Algorithm. Proc. CSEE 2023, 43, 4443–4451. [Google Scholar]





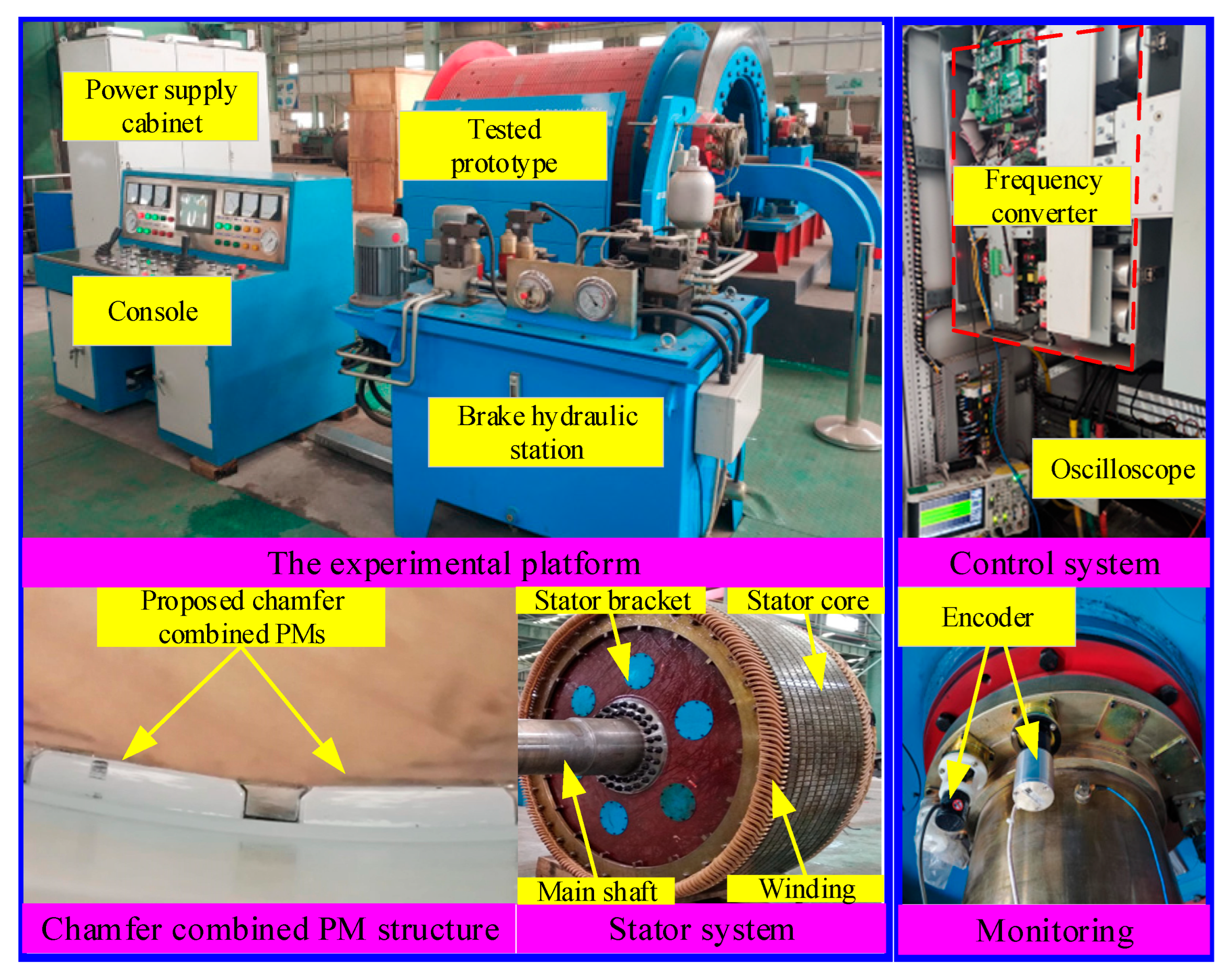
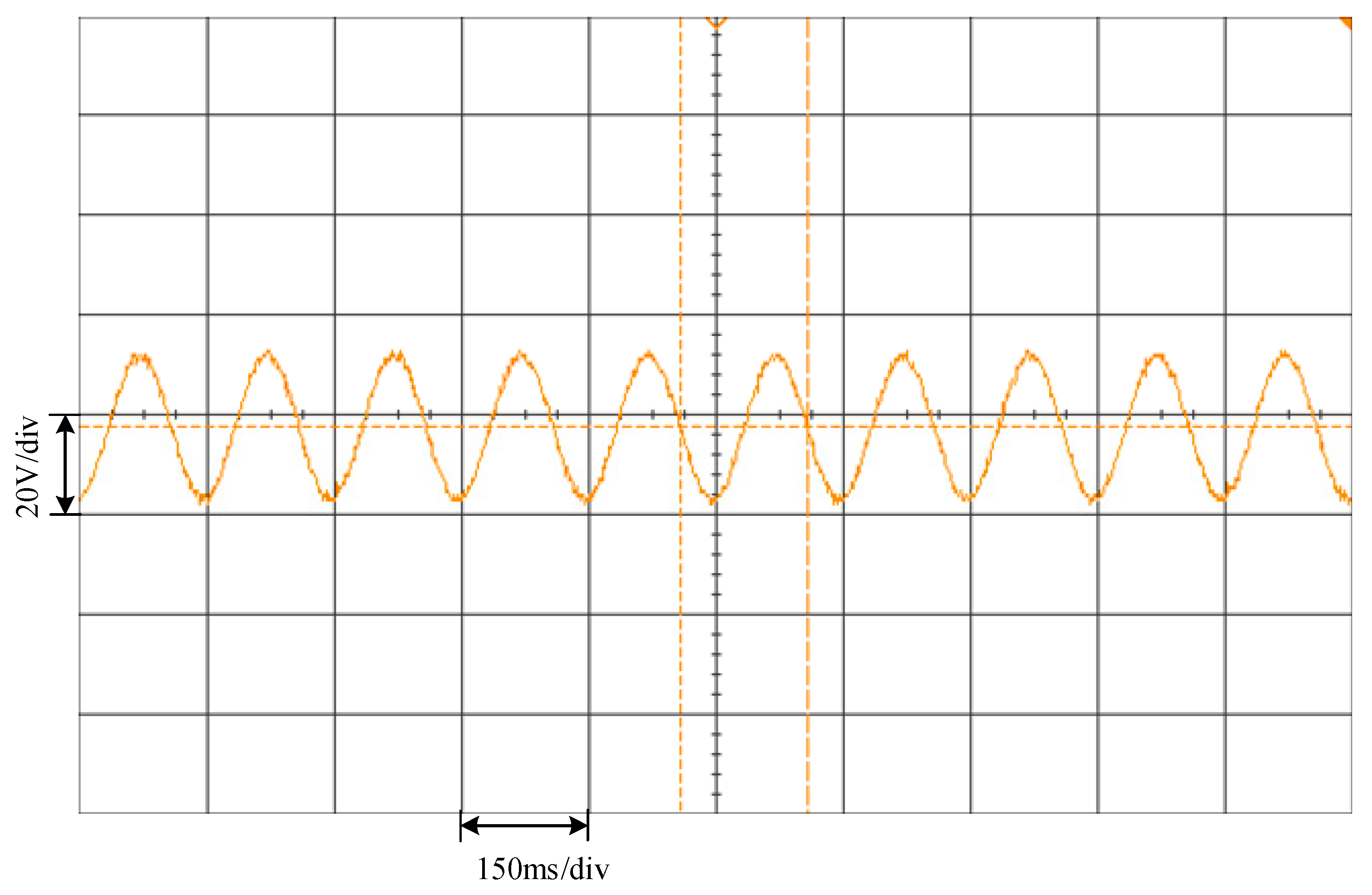

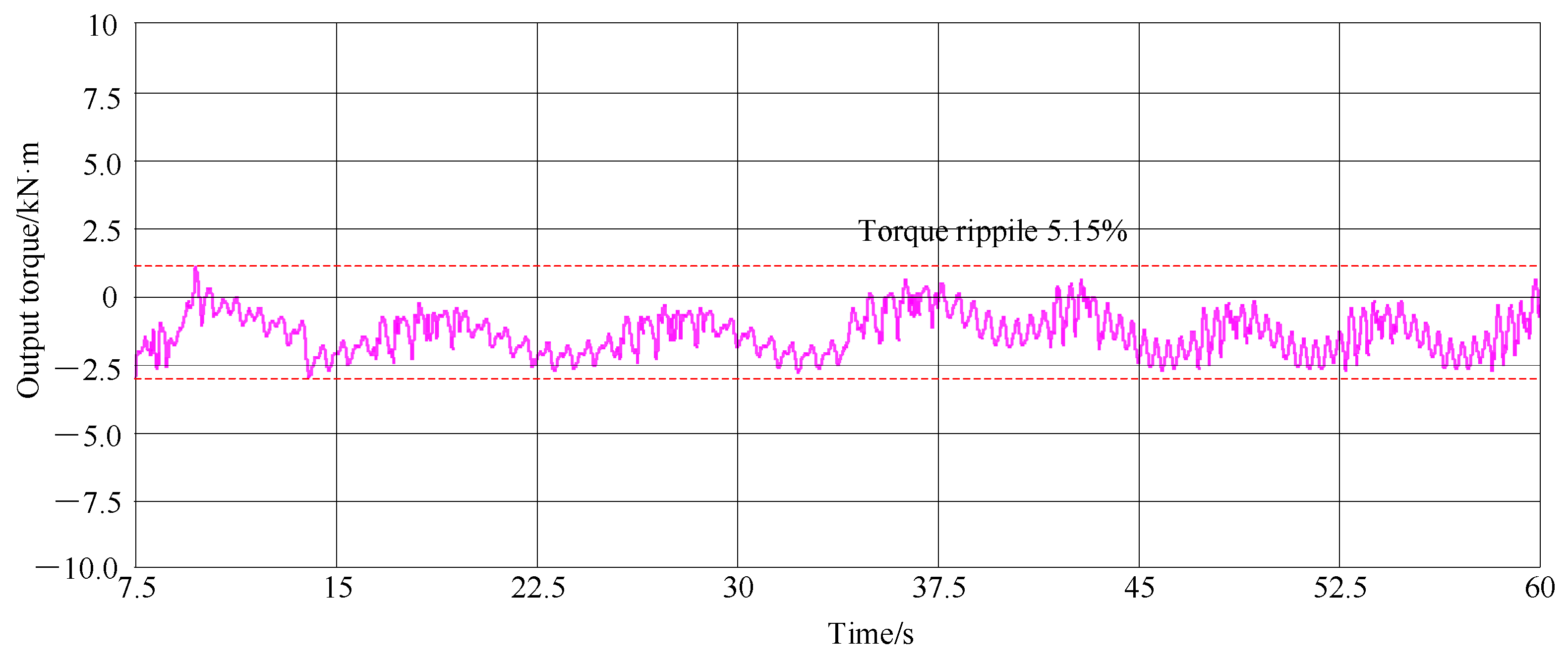
| Parameter | Item | Value |
|---|---|---|
| p | Number of pole pairs | 20 |
| Q | Number of slots | 168 |
| R1 | Semidiameter of slot bottom | 764 mm |
| R2 | Inside semidiameter of slot opening | 824 mm |
| R3 | Outside semidiameter of slot body | 821 mm |
| RB | Inside semidiameter of PMs | 840 mm |
| R5 | Outside semidiameter of slot opening | 825 mm |
| RA | Outside semidiameter of PMs | 840 mm |
| Rm | Inner arc radius of Region 1u | variable |
| φ2 | Center angles of Region 1p | variable |
| hm | Maximum thickness of PM | 12 mm |
| b | Tooth width of stator | 20 mm |
| h0 | Toothed boot height | 1 mm |
| l | Depth of U-shaped groove | 1.29 mm |
| hs1 | Width of U-shaped groove | 3 mm |
| hs2 | Stator tooth height | 57 mm |
| g | Air gap length | 3 mm |
| αp | Pole arc coefficient | 0.94 |
| λ | Asymmetry of slot opening | variable |
| ξ | Asymmetry of inside slot | variable |
| δ | Slot pitch angle of stator | 2.14° |
| Parameters | Items | Value |
|---|---|---|
| UN | Nominal voltage | 1140 V |
| IN | Nominal current | 171 A |
| PN | Nominal frequency | 9.16 Hz |
| nN | Nominal rotating speed | 26.5 r/min |
| RN | Resistance per phase | 1.05 Ω |
| / | Number of conductors | 28 |
| / | Number of parallel branches | 8 |
| Type | Symbol | Value |
|---|---|---|
| Chamfer combination | lcf | 5 mm |
| θcf | 30° | |
| Fillet combination | Rcf | 5.2 mm |
| Rectangular combination | lw | 1.4 mm |
| lc | 5 mm | |
| Axial length of PM | lpm | 98 mm |
| Comparison | Air Gap Flux Density (T) | Air Gap Flux Density THD THDag (%) | Average Value of Electromagnetic Torque Tavg (kN·m) | Torque Ripple Trip (%) | Cogging Torque Tcog (N·m) | No-Load Back EMF THD | |
|---|---|---|---|---|---|---|---|
| Br | Bt | THDemf (%) | |||||
| Chamfer cutting | 1.165 | 0.0482 | 23.4% | 2.06 | 4.96% | 10.09 | 9.85 |
| Rectangular cutting | 1.165 | 0.0512 | 23.9% | 2.02 | 5.62% | 12.06 | 10.19 |
| Fillet cutting | 1.162 | 0.0481 | 24.5% | 1.96 | 5.11% | 10.64 | 11.29 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kou, S.; Kou, Z.; Wu, J.; Wang, Y. Novel Magnetic Field Modeling Method for a Low-Speed, High-Torque External-Rotor Permanent-Magnet Synchronous Motor. Electronics 2023, 12, 5025. https://doi.org/10.3390/electronics12245025
Kou S, Kou Z, Wu J, Wang Y. Novel Magnetic Field Modeling Method for a Low-Speed, High-Torque External-Rotor Permanent-Magnet Synchronous Motor. Electronics. 2023; 12(24):5025. https://doi.org/10.3390/electronics12245025
Chicago/Turabian StyleKou, Shaokai, Ziming Kou, Juan Wu, and Yandong Wang. 2023. "Novel Magnetic Field Modeling Method for a Low-Speed, High-Torque External-Rotor Permanent-Magnet Synchronous Motor" Electronics 12, no. 24: 5025. https://doi.org/10.3390/electronics12245025
APA StyleKou, S., Kou, Z., Wu, J., & Wang, Y. (2023). Novel Magnetic Field Modeling Method for a Low-Speed, High-Torque External-Rotor Permanent-Magnet Synchronous Motor. Electronics, 12(24), 5025. https://doi.org/10.3390/electronics12245025






