Robust Nonsingular Terminal Sliding Mode Control of a Buck Converter Feeding a Constant Power Load
Abstract
:1. Introduction
- Eliminating the negative effect of the CPL caused by the interaction between the DC-DC buck converter and a voltage-controlled boost converter acting as a CPL.
- Avoiding the singularity problem caused by the conventional terminal sliding mode controller.
- Experimental validation of the suggested controller.
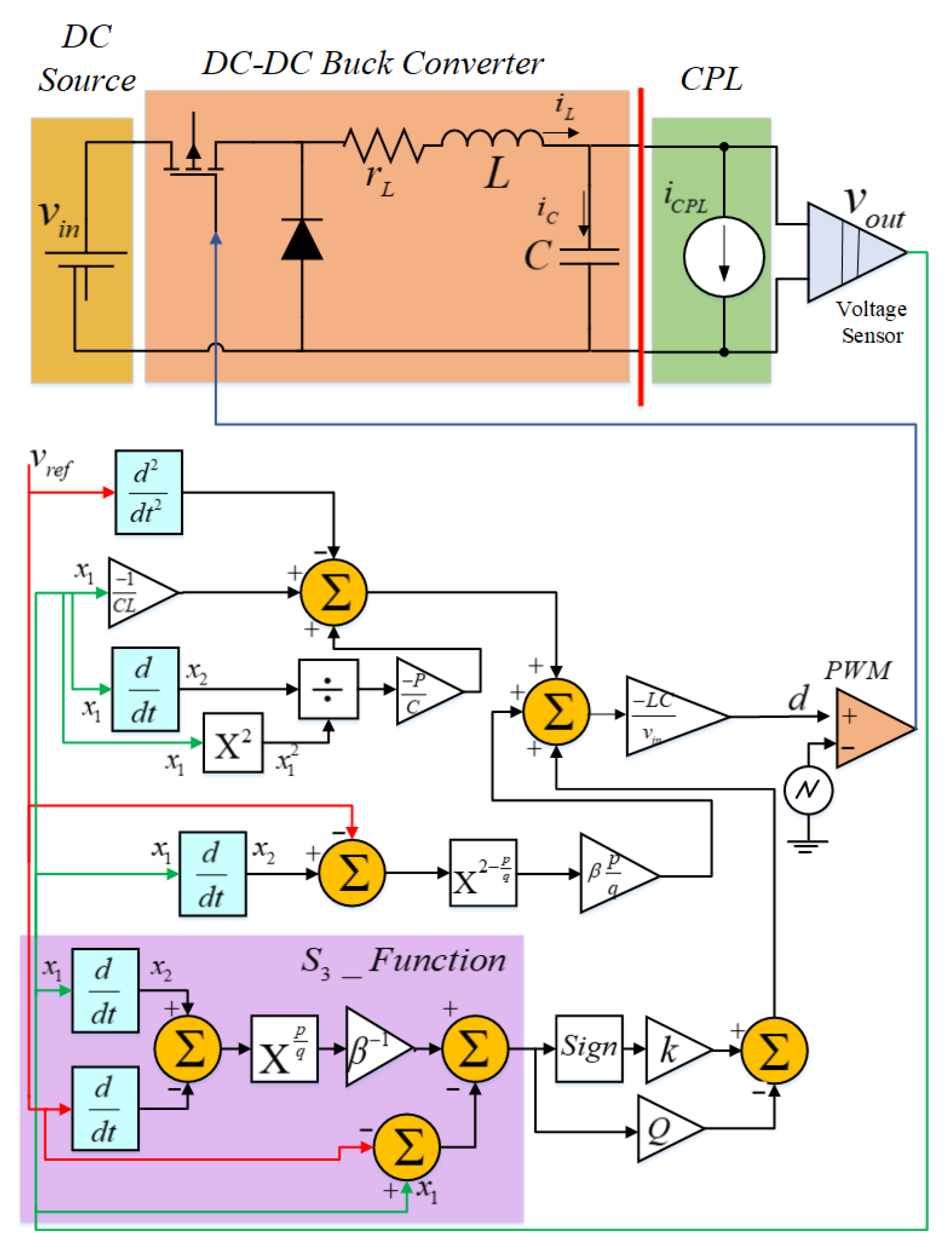
2. System Description and Problem Statement
3. Basics of Terminal Sliding Mode Controller
4. NTSM Controller Applied to a Buck Converter Feeding a CPL
4.1. Application of an NTSM Controller to a Buck Converter Supplying a CPL
- (1)
- If , must be smaller than 0, which yields:
- (2)
- If , must be greater than 0, which yields:
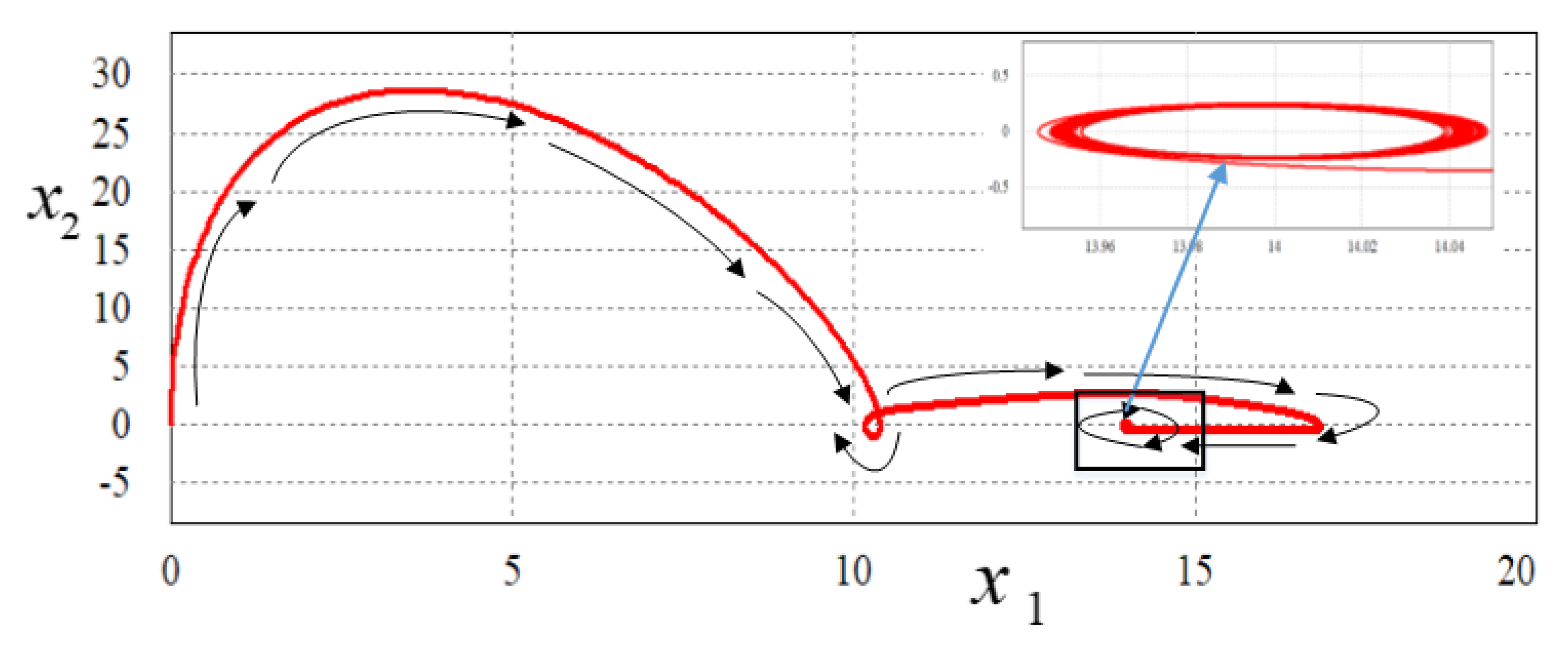
4.2. Control Design and Stability Analysis of NTSM Controller
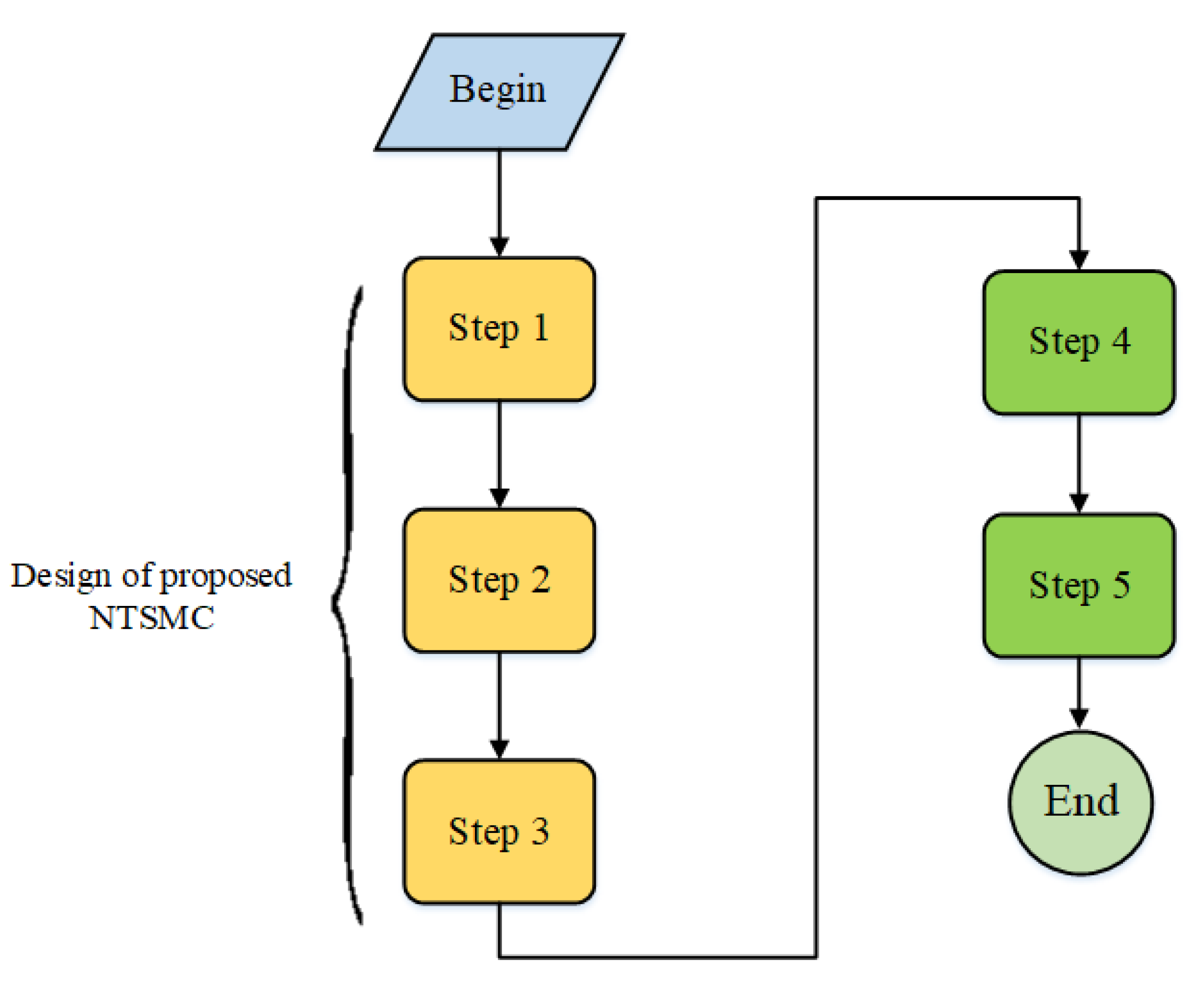
4.3. Implementation of the Proposed NTSM Controller
- Step 1: defining the studied state variables of the system;
- Step 2: Selecting new state variables ;
- Step 3: Performing the new model design by using ;
- Step 4: Constructing NTSM control with the new model;
- Step 5: Lyapunov condition test in order to ensure the stability.
5. Results and Discussion
- (Case 1)
- Response to sudden changes in the power consumed by the CPL.
- (Case 2)
- Response against input voltage fluctuations.
5.1. Simulation Results
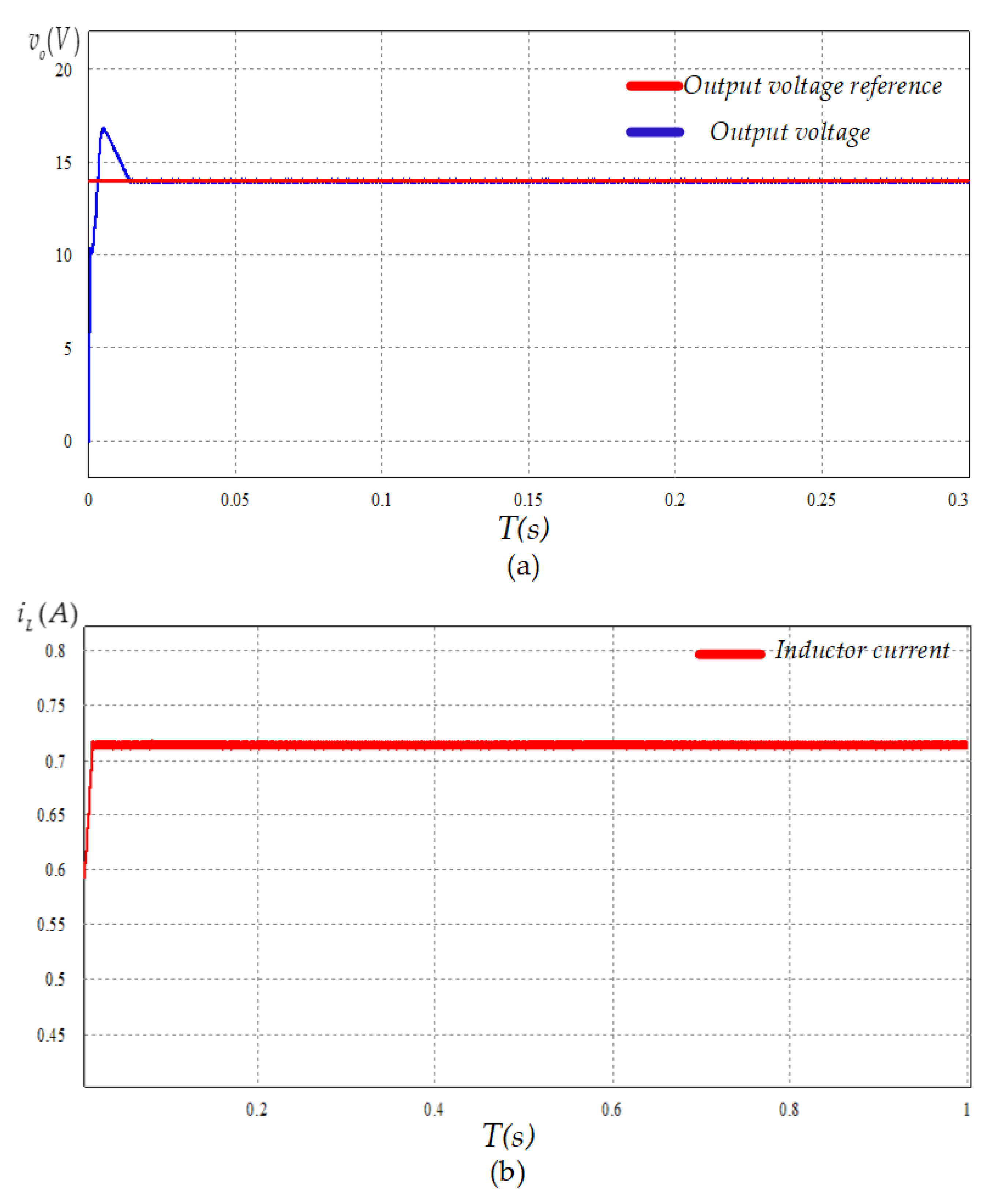
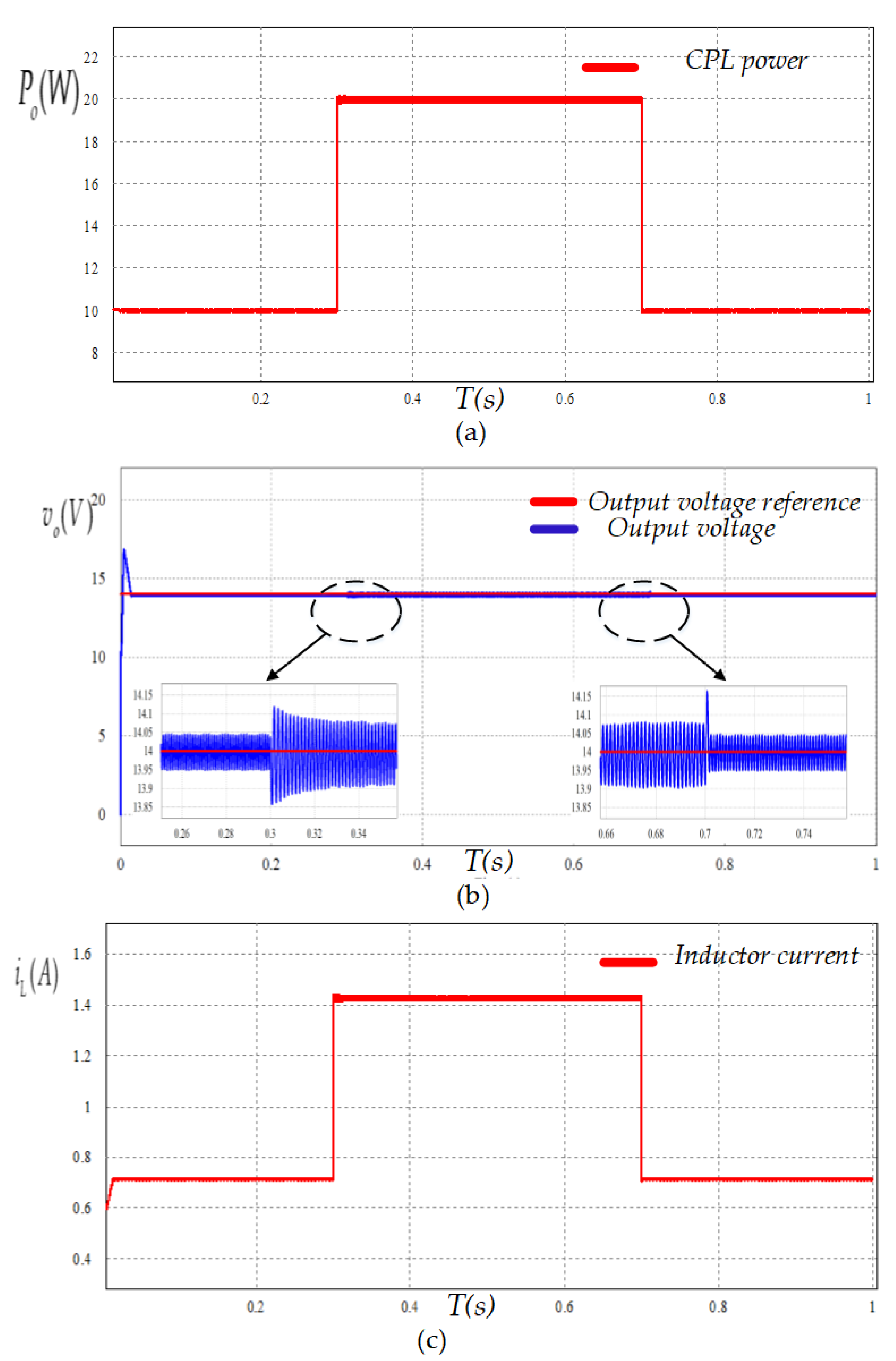
5.1.1. Power Demand Variation Test
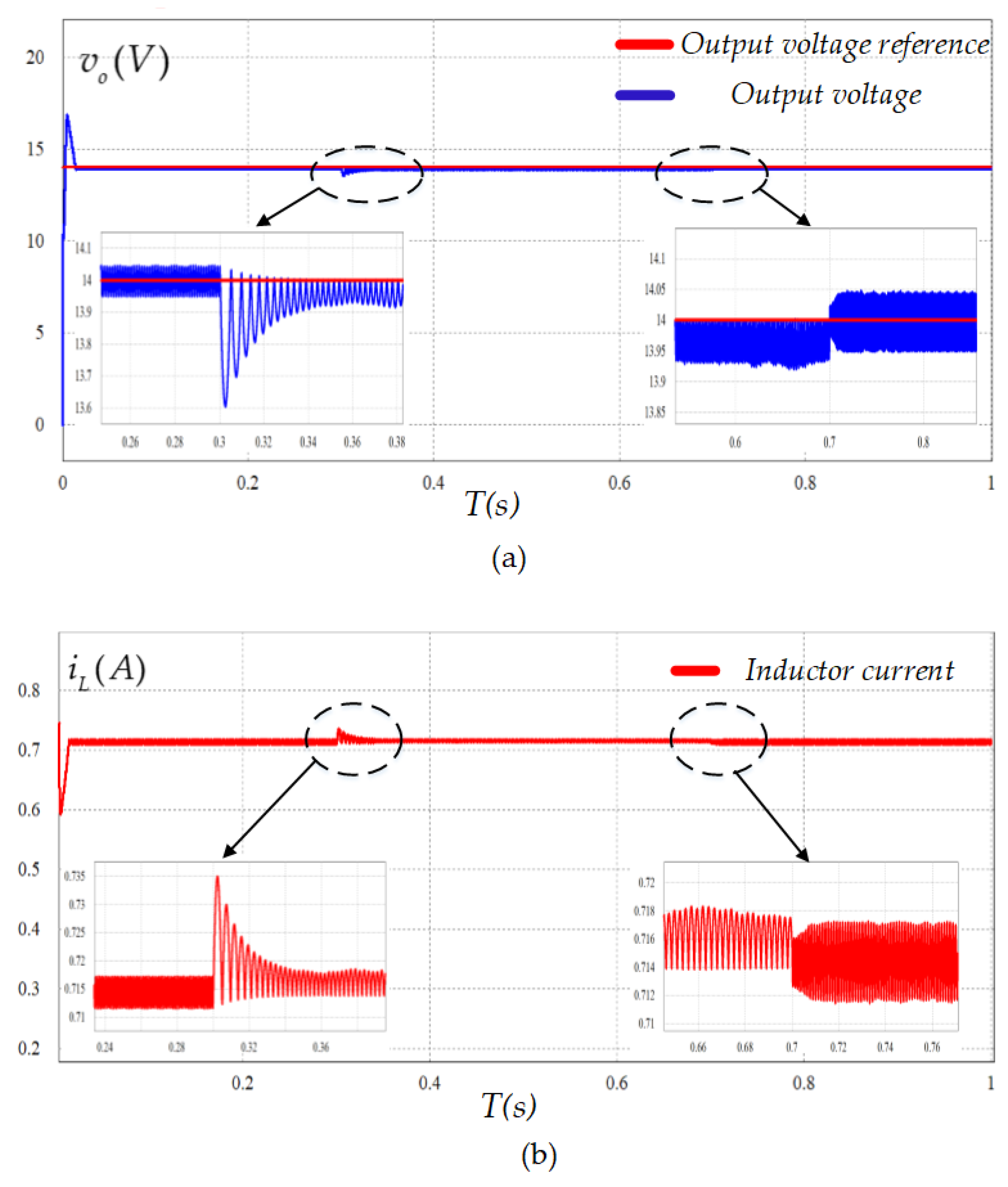
5.1.2. Input Voltage Fluctuation Test
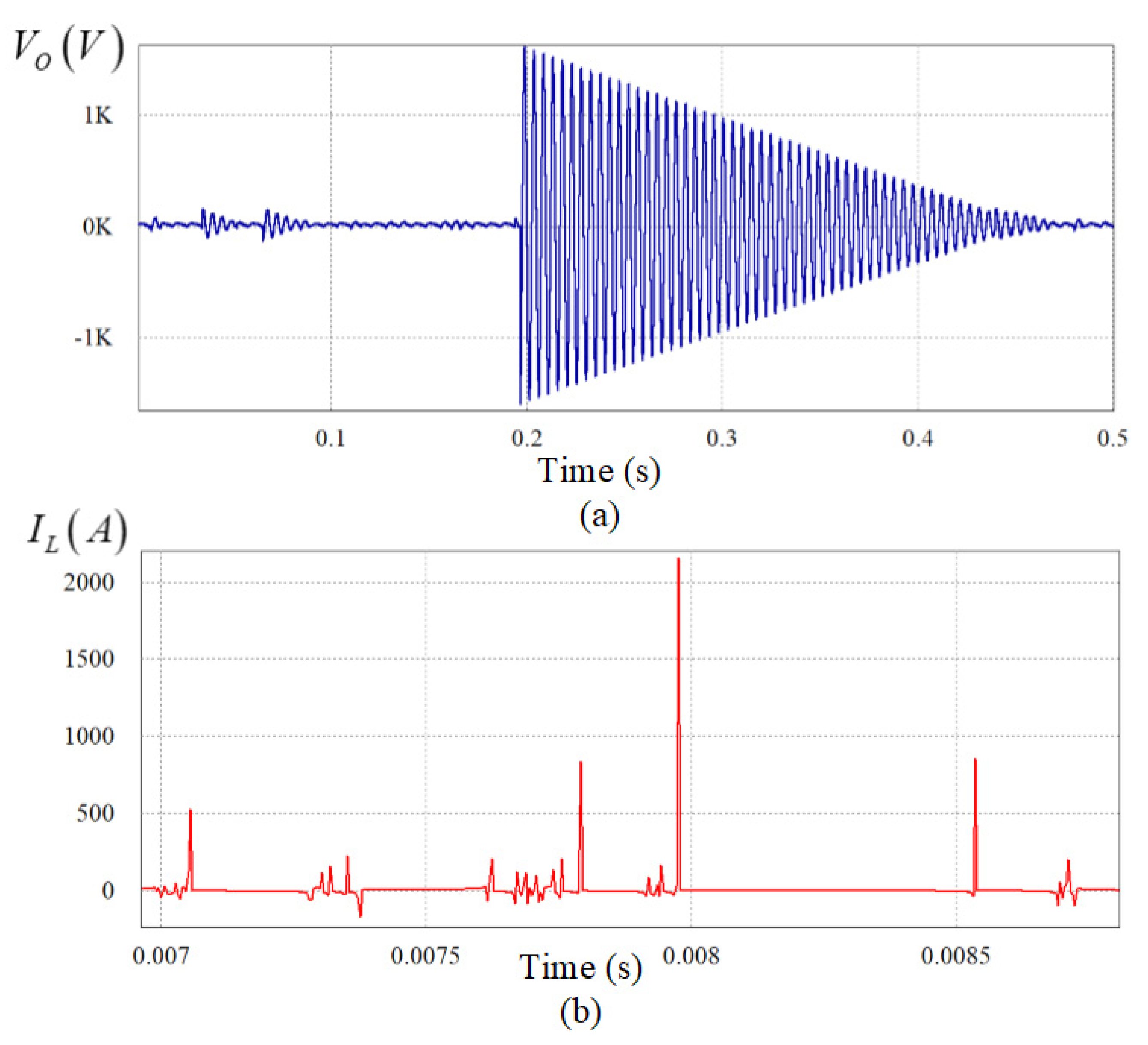
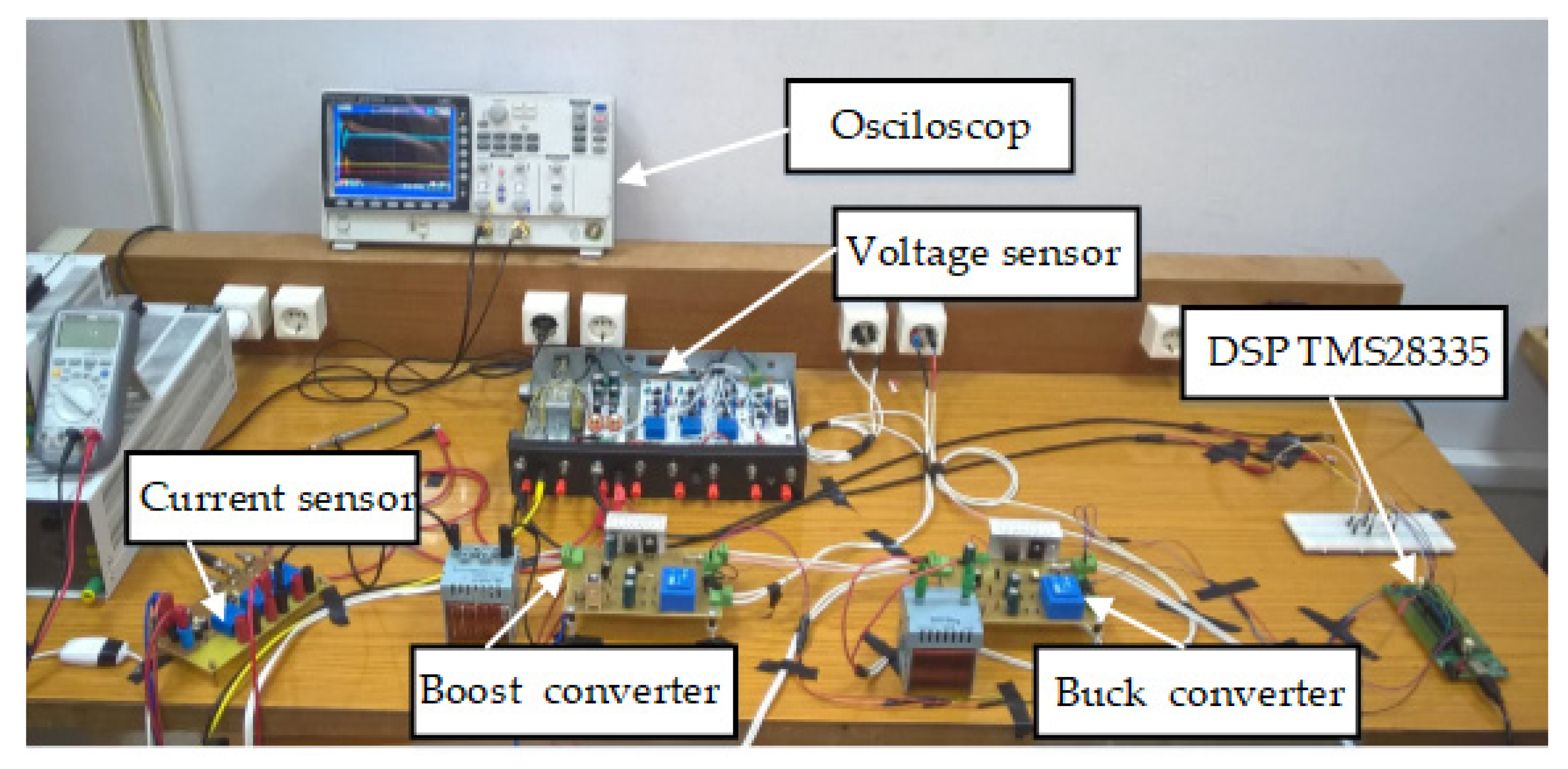
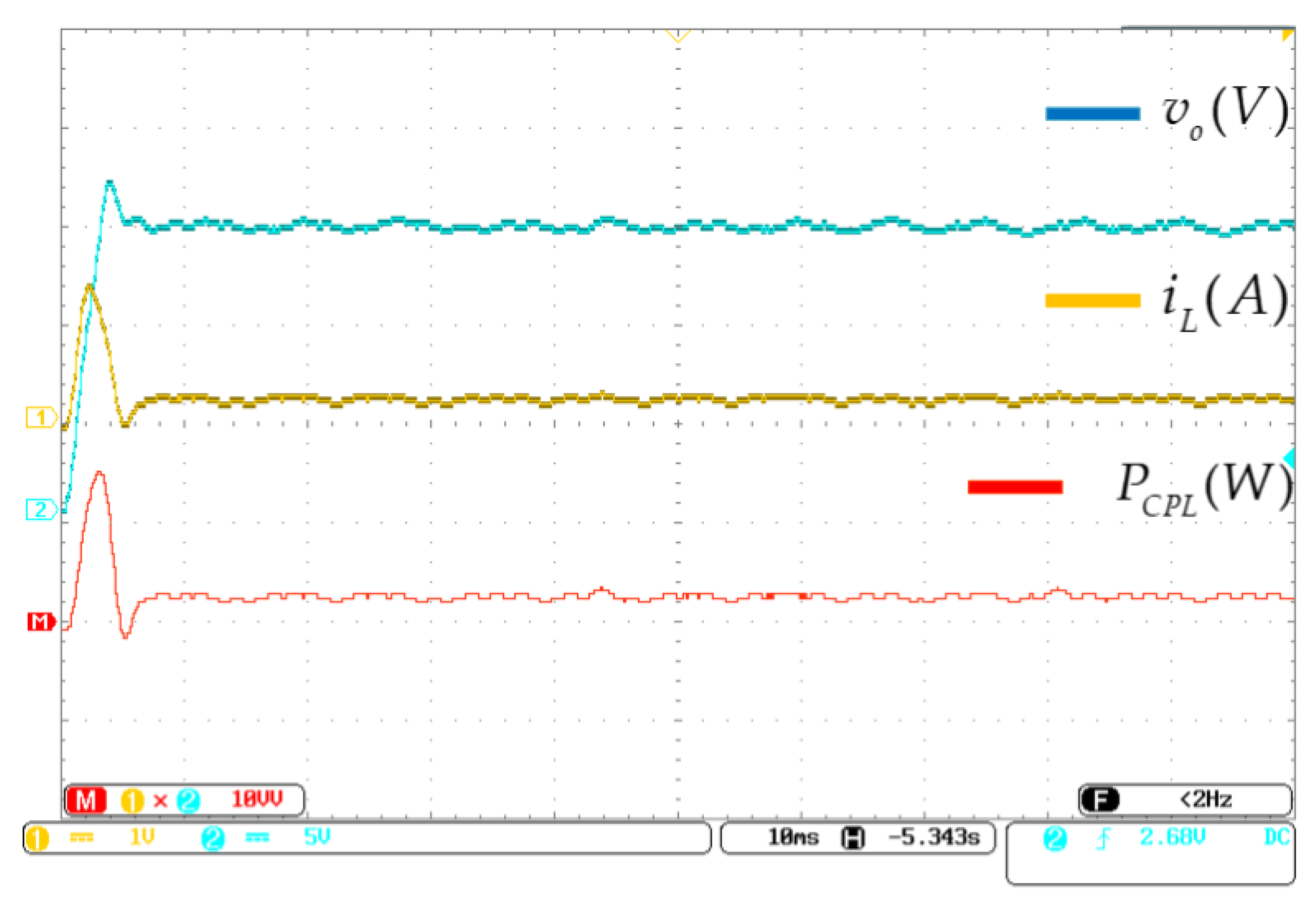
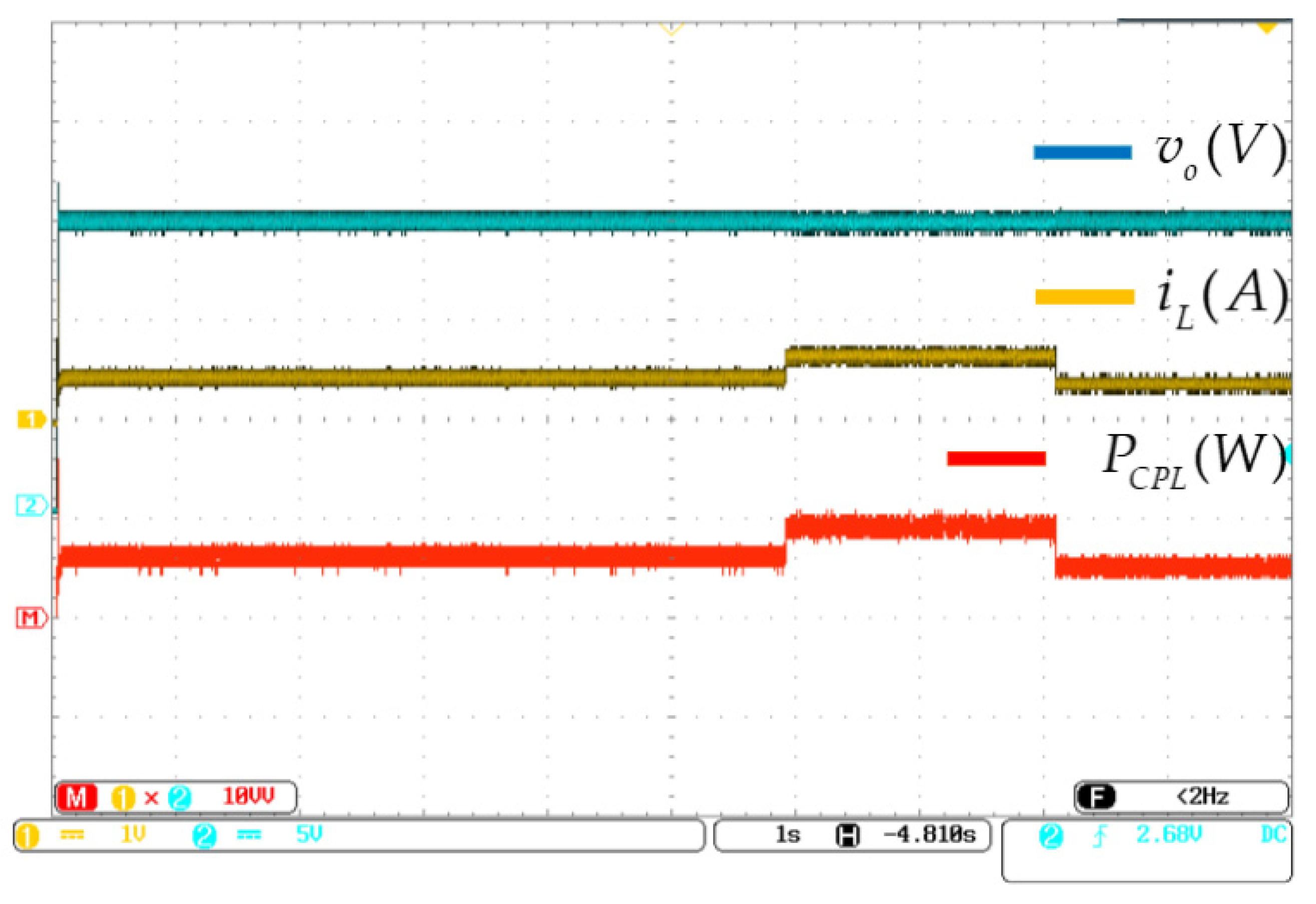
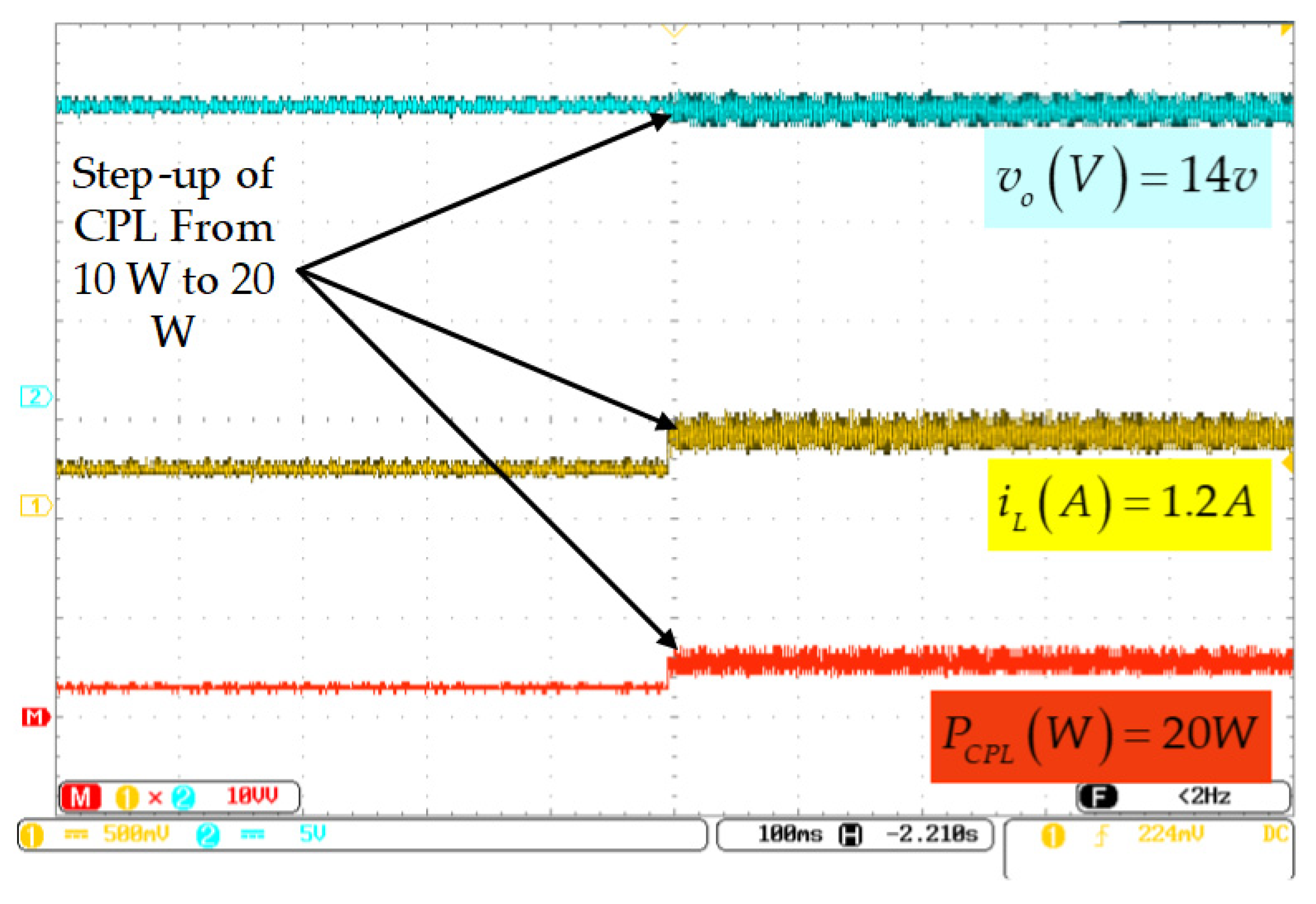
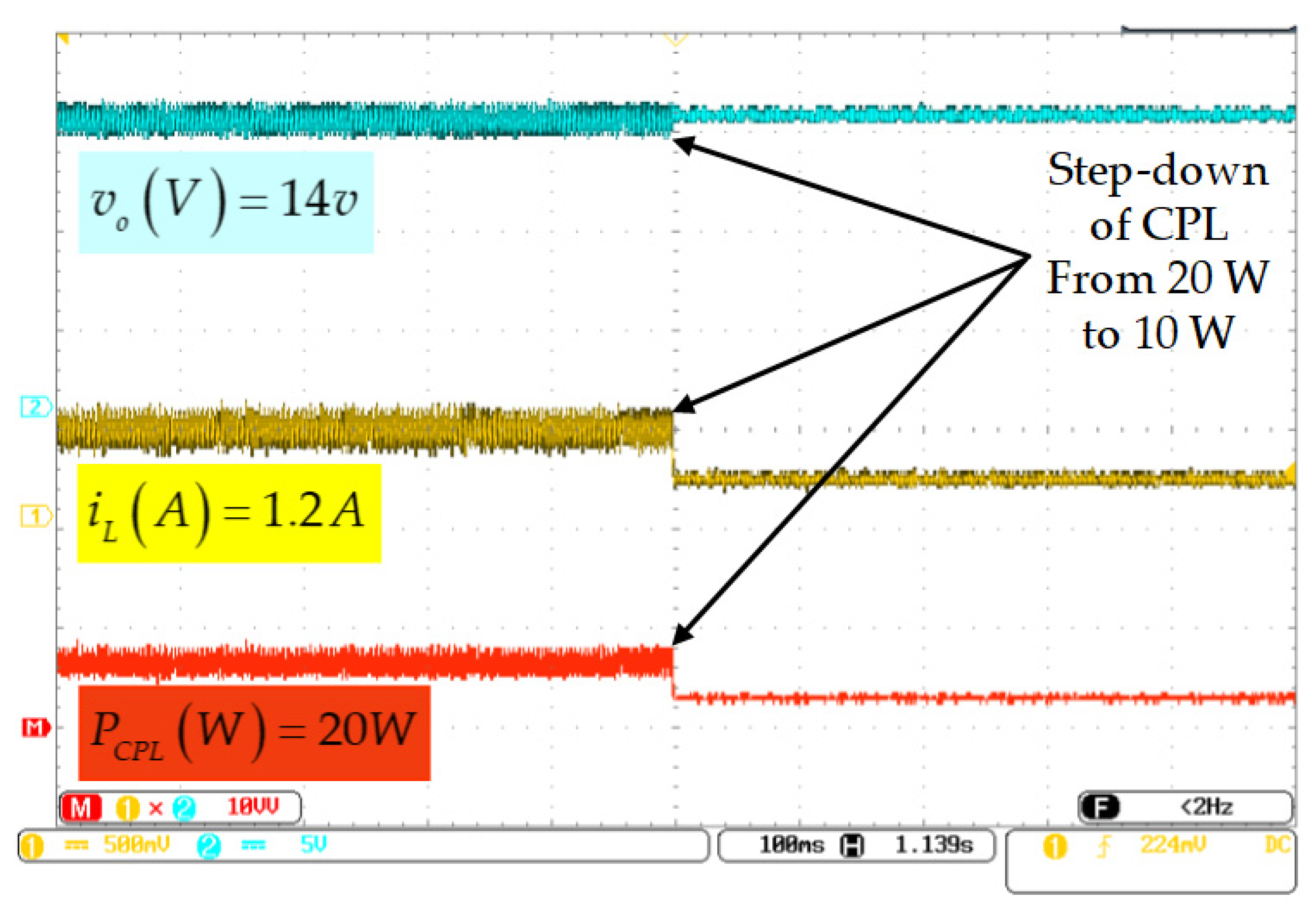
5.2. Experimental Results and Discussion
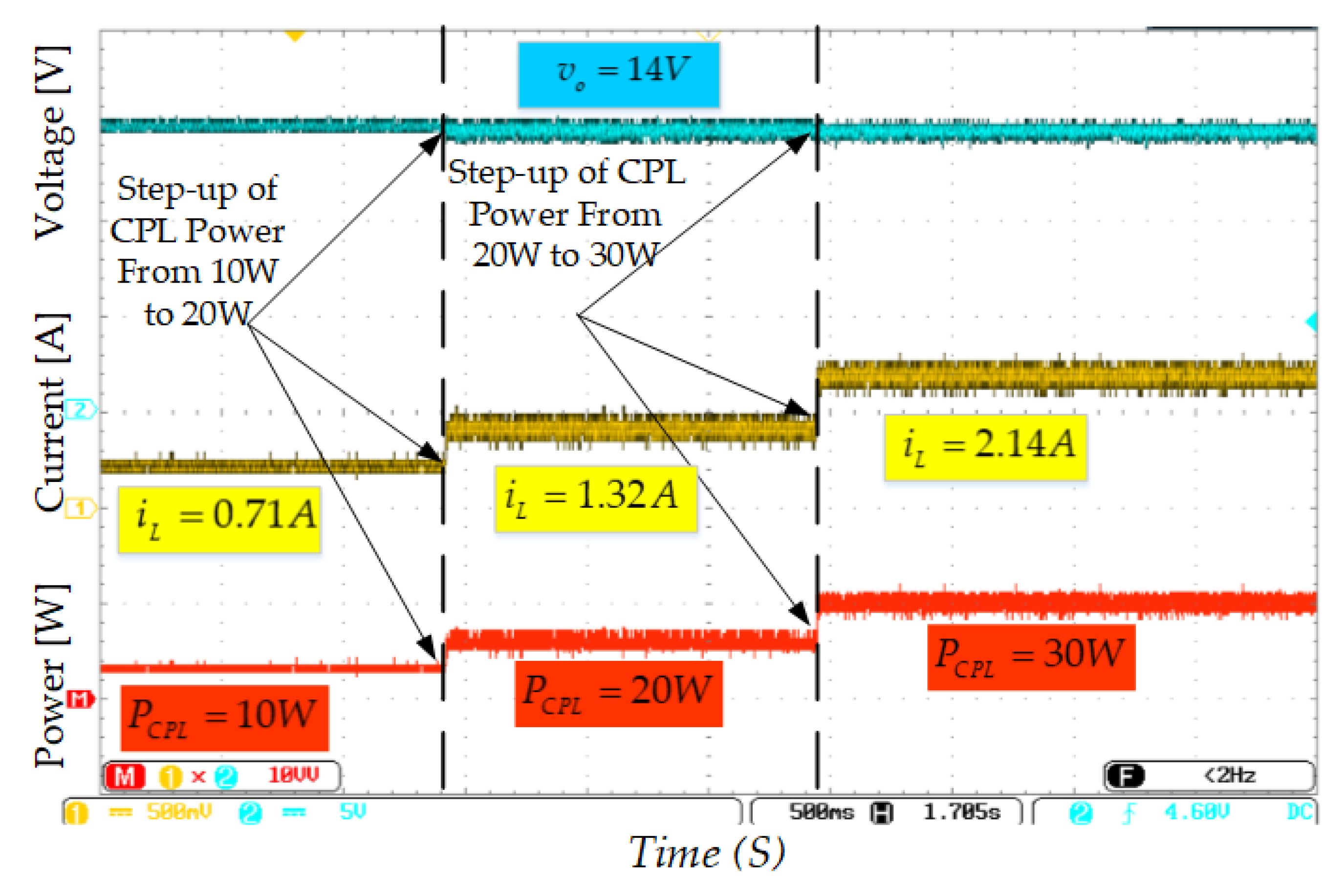
5.2.1. CPL Changing Experiment
5.2.2. Input Voltage Variation Test
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| vin | Input voltage |
| vo | Output voltage |
| d | Duty cycle |
| L | Inductance |
| C | Capacitance |
| ic | Capacitance current |
| iL | Inductor current |
| io | Output current |
| P | Power consumed by the CPL |
| RLoad | Resistive load |
| vBus | DC bus voltage |
| vref | Voltage reference |
| Pin | Input power |
| Po | Output power |
| rin | Incremental negative resistance |
| fsw | Switching frequency |
| x1 | Output voltage state variable |
| x2 | Time derivative of x1 |
| b(x), f(x) | Nonlinear functions in terms of x |
| g(x) | Disturbances and uncertainties function |
| IG | Constant greater than zero |
| u | Control law |
| β | Constant and positive coefficient |
| p, q | Odd integers greater than zero |
| η, λ | Constant coefficients greater than zero |
| e | Tracking error |
| usw | Switching control of the SMC |
| ueq | Equivalent control law |
| Q, k | Constant and positive coefficients |
| s(t) | Nonlinear surface function |
| V(s) | Lyapunov function |
| NTSM | Nonsingular terminal sliding mode |
| DSP | Digital signal processor |
| CCM | Continuous condition mode |
| CPL | Constant power load |
| CVL | Constant voltage load |
| CCL | Constant current load |
| AC | Alternative current |
| TSM | Terminal sliding mode |
| SMC | Sliding mode controller |
| MG | Microgrid |
| DC | Direct current |
| CCS | Code Composer Studio |
| ADC | Analog digital conveter |
| RES | Renewable energies sources |
| PWM | Pulse-width modulation |
Appendix A

| N | The Equipment |
|---|---|
| 1 | DC-DC buck converter |
| 2 | DC-DC boost converter |
| 3 | DC source giving a 28 V |
| 4 | DSP TMS28335 C2000 microcontroller |
| 5 | Voltage sensor LA25-NP (717087) |
| 6 | Current sensor LV25-P (714227) |
| 7 | Buck converter’s inductance L = 2.7 mH |
| 8 | Boost converter’s inductance Lb = 0.52 mH |
| 9 | Buck converter’s capacitance C = 470 uF |
| 10 | Boost converter’s capacitance Cb = 470 uF |
| 11 | Boost converter’s resistive load RLoad = 30 Ω |
| 12 | Code Composer Studio software (CCS) |
| 13 | PSIM software |
References
- Lasseter, R.H. Micro Grids; IEEE Power Engineering Society, Winter Meeting: New York, NY, USA, 2002; Volume 1, pp. 305–308. [Google Scholar]
- ragičević, T.; Lu, X.; Vasquez, J.C.; Guerrero, J.M. DC Microgrids–Part I: A Review of Control Strategies and Stabilization Techniques. IEEE Trans. Power Electron. 2016, 31, 4876–4891. [Google Scholar]
- Ochoa, D.; Martinez, S.; Arévalo, P. Extended Simplified Electro-Mechanical Model of a Variable-Speed Wind Turbine for Grid Integration Studies: Emulation and Validation on a Microgrid Lab. Electronics 2022, 11, 3945. [Google Scholar] [CrossRef]
- Guerrero, J.M.; Vasquez, J.C.; Teodorescu, R. Hierarchical control of droop-controlled DC and AC microgrids—A general approach towards standardization. In Proceedings of the 35th Annual Conference of IEEE Industrial Electronics, Porto, Portugal, 3–5 November 2009; pp. 4305–4310. [Google Scholar]
- Zhang, F.; Yan, Y. Start-Up Process and Step Response of a DC–DC Converter Loaded by Constant Power Loads. IEEE Trans. Ind. Electron. 2010, 58, 298–304. [Google Scholar] [CrossRef]
- Al-Nussairi, M.K.; Bayindir, R.; Padmanaban, S.; Mihet-Popa, L.; Siano, P. Constant Power Loads (CPL) with Microgrids: Problem Definition, Stability Analysis and Compensation Techniques. Energies 2017, 10, 1656. [Google Scholar] [CrossRef]
- Emadi, A.; Fahimi, B.; Ehsani, M. On the concept of negative impedance instability in the more electric aircraft power systems with constant power loads. SAE Trans. 1999, 108, 689–699. [Google Scholar]
- Emadi, A.; Khaligh, A.; Rivetta, C.H.; Williamson, G.A. Constant Power Loads and Negative Impedance Instability in Automotive Systems: Definition, Modeling, Stability, and Control of Power Electronic Converters and Motor Drives. IEEE Trans. Veh. Technol. 2006, 55, 1112–1125. [Google Scholar] [CrossRef]
- Céspedes, M.; Beechner, T.; Xing, L.; Sun, J. Stabilization of constant-power loads by passive impedance damping. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition (APEC), Palm Springs, CA, USA, 21–25 February 2010; pp. 2174–2180. [Google Scholar]
- Cespedes, M.; Xing, L.; Sun, J. Constant-Power Load System Stabilization by Passive Damping. IEEE Trans. Power Electron. 2011, 26, 1832–1836. [Google Scholar] [CrossRef]
- Liu, X.; Ma, S. Large signal stabilization method of constant power loads by adding R parallel damping filters. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 1314–1319. [Google Scholar]
- Lu, X.; Sun, K.; Huang, L.; Guerrero, J.M.; Vasquez, J.C.; Xing, Y. Virtual impedance-based stability improvement for DC microgrids with constant power loads. In Proceedings of the IEEE Energy Conversion Congress and Exposition (ECCE), Pittsburgh, PA, USA, 14–18 September 2014; pp. 2670–2675. [Google Scholar]
- Wu, M.; Lu, D.D. Adding virtual resistance in source side converters for stabilization of cascaded connected two stage converter systems with constant power loads in dc microgrids. In Proceedings of the International Power Electronics Conference, Hiroshima, Japan, 18–21 May 2014; pp. 3553–3556. [Google Scholar]
- Rahimi, A.M.; Emadi, A. Active damping in dc/dc power electronic converters: A novel method to overcome the problems of constant power loads. IEEE Trans. Ind. Electron. 2009, 56, 1428–1439. [Google Scholar] [CrossRef]
- Sulligoi, G.; Bosich, D.; Giadrossi, G.; Zhu, L.; Cupelli, M.; Monti, A. Multiconverter medium voltage dc power systems on ships: Constant-power loads instability solution using linearization via state feedback control. IEEE Trans. Smart Grid 2014, 5, 2543–2552. [Google Scholar] [CrossRef]
- Zhang, Q.; Xu, C.; Wen, C.; Wang, P.; Yeong, L.M. A novel adaptive backstepping controller for stabilization of DC/DC converter feeding constant power load. In Proceedings of the IEEE 26th International Symposium on Industrial Electronics (ISIE), Edinburgh, UK, 19–21 June 2017; pp. 570–575. [Google Scholar]
- Xu, Q.; Zhang, C.; Wen, C.; Wang, P. A Novel Composite Nonlinear Controller for Stabilization of Constant Power Load in DC Microgrid. IEEE Trans. Smart Grid 2019, 10, 752–761. [Google Scholar] [CrossRef]
- Zhao, Y.; Member, S.; Qiao, W.; Member, S.; Ha, D.; Member, S. A Sliding-Mode Duty-Ratio Controller for DC/DC Buck Converters with Constant Power Loads. IEEE Trans. Ind. Appl. 2014, 50, 1448–1458. [Google Scholar] [CrossRef]
- Singh, S.; Fulwani, D.; Kumar, V. Robust sliding-mode control of dc/dc boost converter feeding a constant power load. IET Power Electron. 2015, 8, 1230–1237. [Google Scholar] [CrossRef]
- Sami, I.; Abid, A.; Khan, N.; Zaid, M.M.; Ahmad, H.; Ali, H. Supertwisting Sliding Mode Control of Multi-converter MVDC power systems under constant power loads. In Proceedings of the IEEE 4th International Conference on Computing, Power and Communication Technologies (GUCON), Kuala Lumpur, Malaysia, 24–29 September 2021; pp. 1–5. [Google Scholar]
- Hassan, M.A.; Li, E.-P.; Li, X.; Li, T.; Duan, C.; Chi, S. Adaptive Passivity-Based Control of DC-DC Buck Power Converter with Constant Power Load in DC Microgrid Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 2029–2040. [Google Scholar] [CrossRef]
- Hassan, M.A.; Sadiq, M.; Hoang, L.Q.N.; Su, C.L. Adaptive Passivity-Based Control for Bidirectional DC-DC Power Converter Supplying Constant Power Loads in DC Shipboard Microgrids. In Proceedings of the IEEE International Future Energy Electronics Conference (IFEEC), Taipei, Taiwan, 16–19 November 2021; pp. 1–6. [Google Scholar]
- Khaligh, A.; Rahimi, A.M.; Emadi, A. Negative impedance stabilizing pulse adjustment control technique for dc/dc converters operating in discontinuous conduction mode and driving constant power loads. IEEE Trans. Veh. Technol. 2007, 56, 2005–2016. [Google Scholar] [CrossRef]
- Liutanakul, P.; Awan, A.B.; Pierfederici, S.; Nahid-Mobarakeh, B.; Meibody-Tabar, F. Linear stabilization of a dc bus supplying a constant power load: A general design approach. IEEE Trans. Power Electron. 2009, 25, 475–488. [Google Scholar] [CrossRef]
- Alidrissi, Y.; Ouladsine, R.; Elmouatamid, A.; Errouissi, R.; Bakhouya, M. Constant Power Load Stabilization in DC Microgrids Using Continuous-Time Model Predictive Control. Electronics 2022, 11, 1481. [Google Scholar] [CrossRef]
- Vafamand, A.; Vafamand, N.; Razavi-far, R.; Zarei, J.; Saif, M. Generalized Predictive Control of Power Converter in DC Microgrids with Constant Power Load. In Proceedings of the IEEE International Conference on Environment and Electrical Engineering and 2021 IEEE Industrial and Commercial Power Systems Europe (EEEIC/I&CPS Europe), Bari, Italy, 7–10 September 2021; pp. 1–6. [Google Scholar]
- Du, W.; Zhang, J.; Zhang, Y.; Qian, Z. Stability criterion for cascaded system with constant power load. IEEE Trans. Power Electron. 2012, 28, 1843–1851. [Google Scholar] [CrossRef]
- Liu, X.; Bian, Y.; Cao, M.; Zhang, Z.; Meng, Q. The Control Parameter Determination Method for Bidirectional DC-DC Power Converters Interfaced Storage Systems Based on Large Signal Stability Analysis. Electronics 2019, 8, 1018. [Google Scholar] [CrossRef]
- Zhang, X.; Ruan, X.; Zhong, Q. Improving the Stability of Cascaded DC/DC Converter Systems via Shaping the Input Impedance of the Load Converter with a Parallel or Series Virtual Impedance. IEEE Trans. Ind. Electron. 2015, 62, 7499–7512. [Google Scholar] [CrossRef]
- Yong, F.; Yu, X.; Man, Z. Non-singular terminal sliding mode control and its application for robot manipulators. In Proceedings of the 2001 IEEE International Symposium on Circuits and Systems, Sydney, Australia, 6–9 May 2001; pp. 545–548. [Google Scholar]
- Fan, L.; Yu, Y. Adaptive Non-singular Terminal Sliding Mode Control for DC-DC Converters. Adv. Electr. Comput. Eng. 2011, 11, 119–122. [Google Scholar] [CrossRef]
- Komurcugil, H. Adaptive terminal sliding-mode control strategy for DC–DC buck converters. ISA Trans. 2012, 51, 673–681. [Google Scholar] [CrossRef] [PubMed]
- Feng, Y.; Yu, X.; Han, F. On nonsingular terminal sliding-mode control of nonlinear systems. Automatica 2013, 49, 1715–1722. [Google Scholar] [CrossRef]
- Feng, Y.; Yu, X.; Man, Z. Non-singular terminal sliding mode control of rigid manipulators. Automatica 2002, 38, 2159–2167. [Google Scholar] [CrossRef]







| Methods | Advantages | Drawbacks |
|---|---|---|
| Passive damping [9,10,11] |
|
|
| Active damping [12,13,14] |
|
|
| Backstepping and passivity-based control [16,17,21,22] |
|
|
| Model predictive control (MPC) [25,26] |
|
|
| Sliding mode control (SMC) [6,18,19,20] |
|
|
| The feedback linearization technique [15] |
|
|
| Pulse width adjustment [23] |
|
|
| Variable | Measure | Unit |
|---|---|---|
| Voltage Reference | 14 | V |
| Inductance | 6 | mH |
| Input Voltage | 28 | V |
| Capacitance | 2 | mF |
| CPL Power | 10 | W |
| Switching Frequency | 25 | kHz |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Louassaa, K.; Chouder, A.; Rus-Casas, C. Robust Nonsingular Terminal Sliding Mode Control of a Buck Converter Feeding a Constant Power Load. Electronics 2023, 12, 728. https://doi.org/10.3390/electronics12030728
Louassaa K, Chouder A, Rus-Casas C. Robust Nonsingular Terminal Sliding Mode Control of a Buck Converter Feeding a Constant Power Load. Electronics. 2023; 12(3):728. https://doi.org/10.3390/electronics12030728
Chicago/Turabian StyleLouassaa, Khalil, Aissa Chouder, and Catalina Rus-Casas. 2023. "Robust Nonsingular Terminal Sliding Mode Control of a Buck Converter Feeding a Constant Power Load" Electronics 12, no. 3: 728. https://doi.org/10.3390/electronics12030728
APA StyleLouassaa, K., Chouder, A., & Rus-Casas, C. (2023). Robust Nonsingular Terminal Sliding Mode Control of a Buck Converter Feeding a Constant Power Load. Electronics, 12(3), 728. https://doi.org/10.3390/electronics12030728







