Quantum Field Theory of Neutrino Mixing in Spacetimes with Torsion
Abstract
:1. Introduction
2. Spacetime Torsion and Dirac Field Quantization
2.1. Spacetime Torsion
2.2. Dirac Field Quantization on Constant Torsional Background
2.3. Dirac Field Quantization with Time-Dependent Torsion
3. Flavor Mixing with Torsion
3.1. Bogoliubov Coefficients with Constant Torsion
3.2. Bogoliubov Coefficients with Time-Dependent Torsion
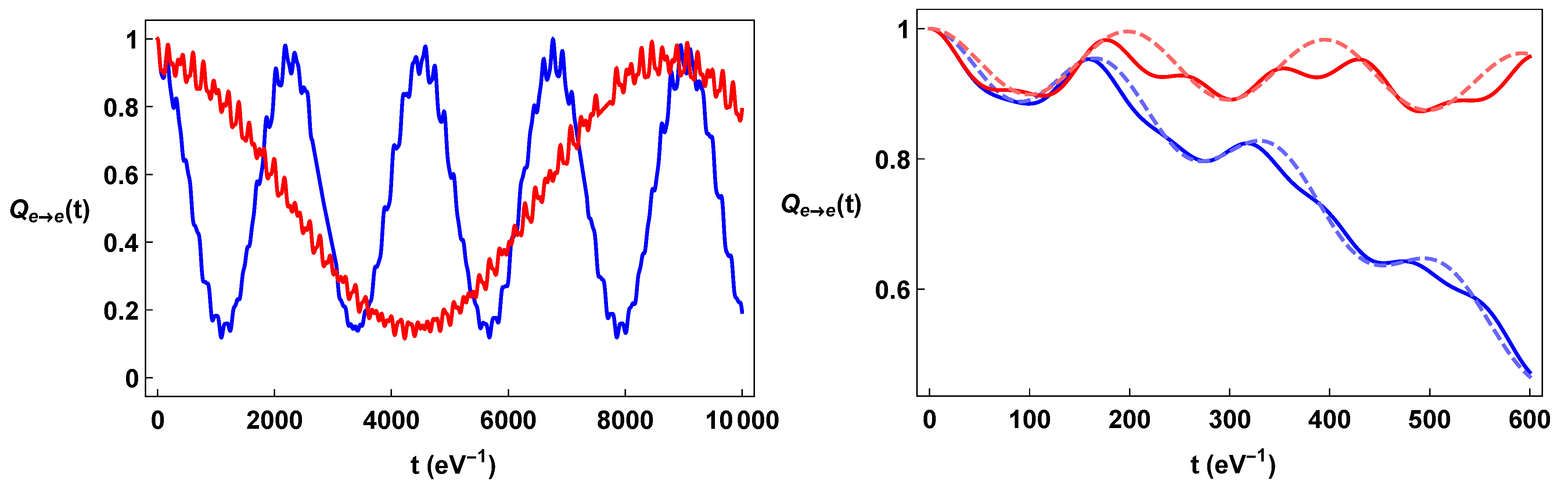
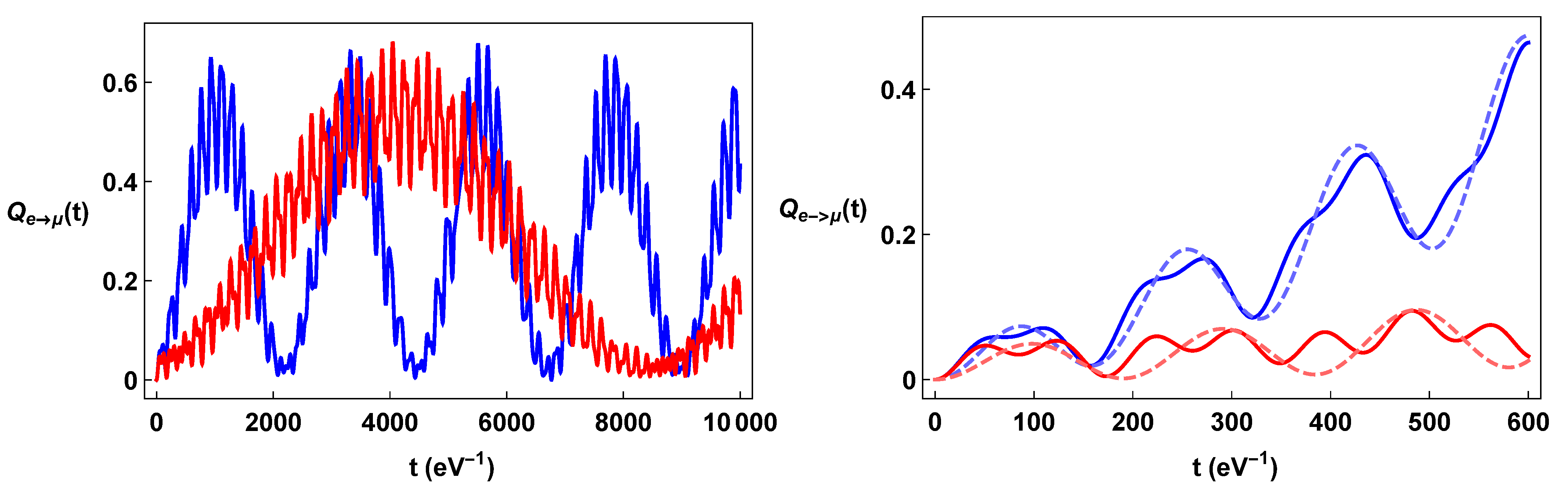
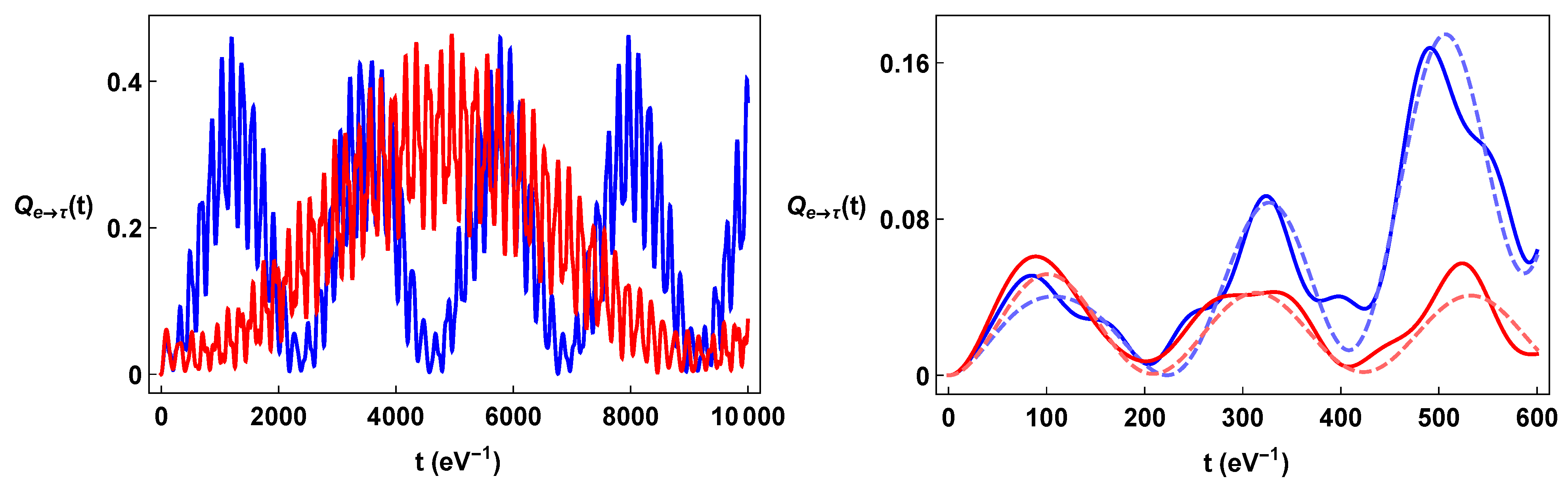
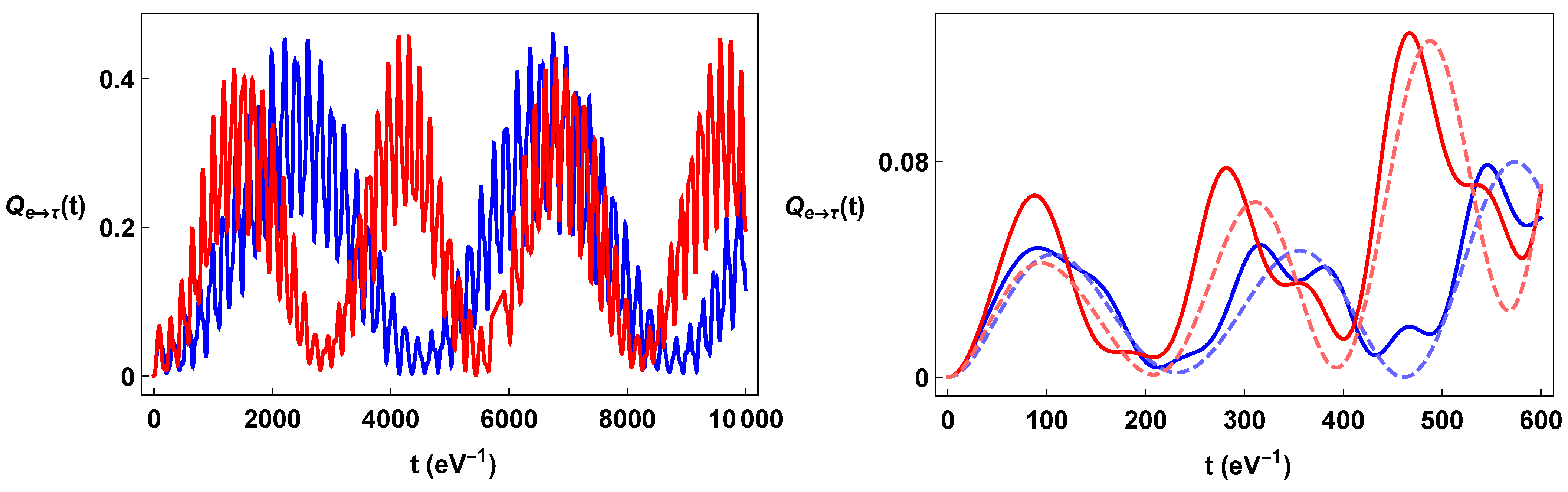
4. Neutrino Oscillations with Background Torsion
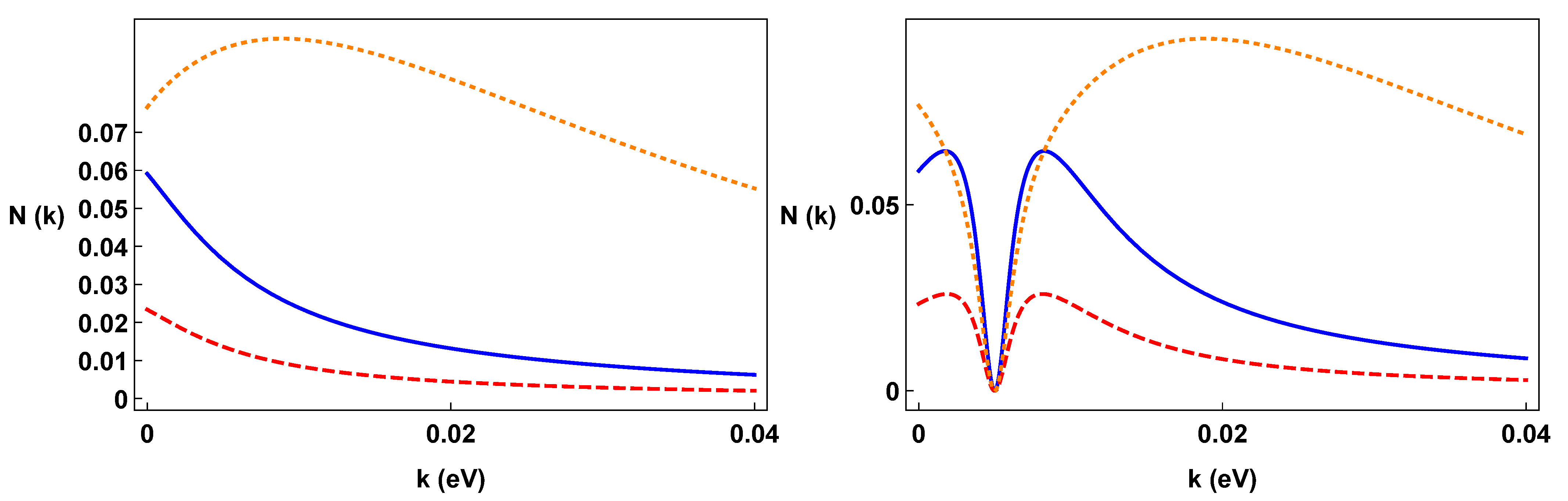
4.1. Neutrino Oscillation with Constant Torsion
4.2. Neutrino Oscillations with Time-Dependent Torsion
5. CP Violation and Flavor Vacuum
5.1. Violation and Flavor Vacuum Condensate with Constant Torsion
5.2. Violation and Flavor Vacuum Condensate for Time-Dependent Torsion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Useful Formulae
Appendix B. Charges for Three Flavor Mixing with Torsion
| 1 | In the ultrarelativistic case (), one has:
|
References
- Capozziello, S.; Laurentis, M.D. Extended theory of gravity. Phys. Rep. 2011, 509, 167–321. [Google Scholar] [CrossRef]
- Heisenberg, L. Review on f(Q) gravity. Phys. Rep. 2024, 1066, 1–78. [Google Scholar] [CrossRef]
- Anagnostopoulos, F.K.; Basilakos, S.; Saridakis, E.N. First evidence that non-metricity f(Q) gravity could challenge ΛCDM. Phys. Lett. B 2021, 822, 136634. [Google Scholar] [CrossRef]
- Capozziello, S.; D’Agostino, R. Model-independent reconstruction of f(Q), non-metric gravity. Phys. Lett. B 2022, 832, 137229. [Google Scholar] [CrossRef]
- Lin, R.H.; Zhai, X.H. Spherically symmetric configuration in f(Q) gravity. Phys. Rev. D 2021, 103, 124001. [Google Scholar] [CrossRef]
- Xu, Y.; Li, G.; Liang, S.D. f(Q,T) gravity. Eur. Phys. J. C 2019, 79, 708. [Google Scholar] [CrossRef]
- Shankaranarayanan, S.; Johnson, J.P. Modified theories of gravity: Why, how and what? Gen. Relativ. Gravit. 2022, 44, 54. [Google Scholar] [CrossRef]
- Bamba, K.; Capozziello, S.; Nojiri, S.I.; Odintsov, S.D. Dark energy cosmology: The equivalent description via different theoretical models and cosmography tests. Astrophys. Sace Sci. 2012, 342, 155–228. [Google Scholar] [CrossRef]
- Khyllep, W.; Dutta, J.; Saridakis, E.N.; Yesmakhanova, K. Cosmology in f(Q) gravity: A unified dynamical systems analysis of the background and perturbations. Phys. Rev. D 2023, 107, 044022. [Google Scholar] [CrossRef]
- Berti, E.; Barausse, E.; Cardoso, V.; Gualtieri, L.; Pani, P.; Sperhake, U.; Stein, L.C.; Wex, N.; Yagi, K.; Baker, T.; et al. Testing general relativity with present and future astrophysical observations. Class. Quantum Grav. 2015, 32, 243001. [Google Scholar] [CrossRef]
- Sebastiani, L.; Vagnozzi, S.; Myrzakulov, R. Mimetic Gravity: A review of recent developments and applications to cosmology and astrophysics. Adv. High Energy Phys. 2017, 2017, 3156915. [Google Scholar] [CrossRef]
- Rubin, V.C.; Ford, W.K.; Thonnard, N. Rotational properties of 21 SC galaxies with a large range of luminosities from NGC 4605(r = 4kpc) to UGC 2885(R = 122 kpc). Astrophys. J. 1980, 238, 471–487. [Google Scholar] [CrossRef]
- Salucci, P. The distribution of dark matter in galaxies. Astron. Astro. Phys. Rev. 2019, 27, 2. [Google Scholar] [CrossRef]
- Freese, K. Status of dark matter in the universe. Int. J. Mod. Phys. D 2017, 26, 1730012. [Google Scholar] [CrossRef]
- Valentino, E.D.; Melchiorri, A.; Mena, O.; Vignozzi, S. Nonminimal dark sector physics and cosmological tension. Phys. Rev D 2020, 101, 6. [Google Scholar] [CrossRef]
- Rubin, V. Dark matter in spiral galaxies. Sci. Am. 1983, 248, 6. [Google Scholar] [CrossRef]
- Rubin, V., W. K. Ford, Rotation of the Andromeda Nebula from a Spectroscopic survey of emission regions. Astrophys. J. 1970, 159, 379. [Google Scholar] [CrossRef]
- Garret, K.; Duda, G. Dark matter: A primer. Advandes Astron. 2010, 2011, 968283. [Google Scholar] [CrossRef]
- Smith, P.F.; Lewin, J.D. Dark matter detection. Phys. Rep. 1990, 187, 5. [Google Scholar] [CrossRef]
- Hutten, M.; Kerszberg, D. TeV Dark Matter Searches in the Extragalactic Gamma-ray. Galaxies 2022, 10, 5. [Google Scholar] [CrossRef]
- Perivolaropoulos, L.; Skara, F. Challenges for ΛCDM: An update. New Astron. Rev. 2022, 95, 101659. [Google Scholar]
- Fruscianti, N.; Perenon, L. Effective field theory of dark energy: A review. Phys. Rep. 2020, 857, 1–63. [Google Scholar] [CrossRef]
- Oks, E. Brief review of recent advances in undestanding dark matter and dark energy. New Astron. Rev. 2021, 93, 101632. [Google Scholar] [CrossRef]
- Lonappan, A.I.; Kumar, S.; Dinda, B.R.; Sen, A.A. Bayesian evidences for dark energy models in light of current observational data. Phys. Rev. 2018, 97, 4. [Google Scholar] [CrossRef]
- Steinhardt, P.J.; Turok, N. Why the cosmological constant is small and positive. Science 2006, 312, 1180–1183. [Google Scholar] [CrossRef]
- Mehrabi, A.; Basilakos, S. Dark energy reconstruction based on the Padé approximation: An expansion around the ΛCDM. Eur. Phys. J. C 2018, 78, 889. [Google Scholar] [CrossRef]
- Brans, C.; Dicke, R.H. Mach’s principle and a Relativistic Theory of Gravitation. Phys. Rev. 1961, 124, n925. [Google Scholar] [CrossRef]
- Quiros, I. Selected topics in scalar-tensor theories and beyond. Int. J. Mod. Phys. D 2019, 28, 1930012. [Google Scholar] [CrossRef]
- Kobayashi, T. Horndeski theory and beyond: A review. Rep. Prog. Phys. 2019, 82, 086901. [Google Scholar] [CrossRef] [PubMed]
- Capozziello, S.; Cardone, V.F.; Carloni, S.; Troisi, A. Can higher order curvature theories explain rotation curves of galaxies? Phys. Lett. A 2004, 326, 292–296. [Google Scholar] [CrossRef]
- Capozziello, S.; Cardone, V.F.; Carloni, S.; Troisi, A. Higher order curvature theories of gravity matchet with observations: A bridge between dark energy and dark matter problems. Aip Conf. Proc. 2005, 751, 54–63. [Google Scholar]
- Cherubini, C.; Bini, D.; Capozziello, S.; Ruffini, R. Second order scalar invariants of the Riemann tensor: Applications to black hole spacetimes. Int. J. Mod. Phys. D 2002, 11, 827–841. [Google Scholar] [CrossRef]
- Hehl, F.W.; Von der Heyde, P.; Kerlick, G.D. General relativity with spin and torsion: Foundations and prospects. Rev. Mod. Phys. 1976, 48, 393. [Google Scholar] [CrossRef]
- Shapiro, I.L. Physical aspects of the space-time torsion. Phys. Rep. 2002, 357, 113–213. [Google Scholar] [CrossRef]
- Mavromatos, N.E.; Pais, P.; Iorio, A. Torsion at different scale: From materials to the universe. Universe 2023, 9, 516. [Google Scholar] [CrossRef]
- Capozziello, S.; Cianci, R.; Stornaiolo, C.; Vignolo, S. f(R) gravity with torsion: The metric-affine approach. Class. Quantum Gravity 2007, 24, 6417. [Google Scholar] [CrossRef]
- Capozziello, S.; Cianci, R.; Stornaiolo, C.; Vignolo, S. f(R) cosmology with torsion. Phys. Scr. 2008, 78, 065010. [Google Scholar] [CrossRef]
- Vignolo, S.; Fabbri, L.; Cianci, R. Dirac spinors in Bianchi-I f(R)- cosmology with torsion. J. Math. Phys. 2011, 52, 112502. [Google Scholar] [CrossRef]
- Capozziello, S.; Vignolo, S. Metric-affine f(R)- gravity with torsion: An overview. Ann. Der Phys. 2010, 522, 238–248. [Google Scholar] [CrossRef]
- Fabbri, L.; Vignolo, S. A modified theroy of gravity with torsion and its applications to cosmology and particle physics. Int. J. Theor. Phys. 2010, 51, 3186. [Google Scholar] [CrossRef]
- Fabbri, L.; Vignolo, S. Dirac fields in f(R) gravity with torsion. Class. Quantum Gravity 2011, 28, 125002. [Google Scholar] [CrossRef]
- Fabbri, L.; Vignolo, S. ELKO and Dirac Spinors seen from Torsion. Int. J. Mod. Phys. D 2014, 23, 1444001. [Google Scholar] [CrossRef]
- Vignolo, S.; Fabbri, L.; Stornaiolo, C. A square-torsion modification of Einstein-Cartan theory. Ann. Der Phys. 2012, 524, 826–839. [Google Scholar] [CrossRef]
- Fabbri, L.; Vignolo, S.; Carloni, S. Renormalizability of the Dirac equation in torsion gravity with nonminimal coupling. Phys. Rev. D 2014, 90, 024012. [Google Scholar] [CrossRef]
- van de Venn, A.; Vasak, D.; Kirsch, J.; Struckmeier, J. Torsional dark energy in quadratic gauge gravity. Eur. Phys. J. C 2023, 83, 288. [Google Scholar] [CrossRef]
- Cabral, F.; Lobo, F.; Rubiera-Garcia, D. Imprints from a Riemann-Cartan space-time on the energy levels of Dirac spinors. Class. Quantum Grav. 2021, 38, 195008. [Google Scholar] [CrossRef]
- Cirilo-Lombardo, D.J. Fermion helicity flip and fermion oscillation induced by dynamical torsion field. EPL 2019, 127, 10002. [Google Scholar] [CrossRef]
- Aartsen, M. et al. [IceCube Collaboration] Neutrino emission from the direction of the Blazar TXS 0506+ 056 prior to the IceCube-170922A alert. Science 2018, 361, 6398. [Google Scholar]
- Aartsen, M. et al. [The IceCube et al.] Multimessenger observations of a flaring blazar coincident with high-energy neutrino IceCube-170922A. Science 2018, 361, eaat1378. [Google Scholar] [CrossRef] [PubMed]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for Continuous and Transient Neutrino Emission Associated with IceCube’s Highest-Energy Tracks: An 11-Year Analysis. Astrophys. J. 2024, 964, 40. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for 10-1000 GeV Neutrinos from Gamma Ray Bursts with IceCube. Astrophys. J. 2024, 964, 126. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for Neutrino Lines from Dark Matter Annihilation and Decay with IceCube. Phys. Rev. D 2023, 108, 102004. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for Extended Sources of Neutrino Emission in the Galactic Plane with IceCube. Astrophys. J. 2023, 956, 20. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Searches for Connections between Dark Matter and High-Energy Neutrinos with IceCube. J. Cosmol. Astrophys. Phys. 2023, 10, 3. [Google Scholar]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for Correlations of High-Energy Neutrinos Detected in IceCube with Radio-Bright AGN and Gamma-Ray Emission from Blazars. Astrophys. J. 2023, 954, 75. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Observation of Seasonal Variations of the Flux of High-Energy Atmospheric Neutrinos with IceCube. Eur. Phys. J. C 2023, 83, 777. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [ICECUBE Collaboration] Search for sub-TeV Neutrino Emission from Novae with IceCube-DeepCore. Astrophys. J. 2023, 953, 160. [Google Scholar] [CrossRef]
- Abud, A.A. et al. [DUNE Collaboration] Impact of cross-section uncertainties on supernova neutrino spectral parameter fitting in the deep underground neutrino experiment. Phys. Rev. D 2023, 107, 112012. [Google Scholar] [CrossRef]
- Abud, A. A. et al. [DUNE Collaboration] Low exposure long-baseline neutrno oscillation sensitivity of the DUNE experiment. Phys. Rev. D 2022, 105, 072006. [Google Scholar] [CrossRef]
- Abi, B. et al. [DUNE Collaboration] Prospects for beyond the prospects for beyond the standard model physics searches at the Deep Underground Neutrino Experiment. Eur. Phys. J. C 2021, 81, 322. [Google Scholar] [CrossRef] [PubMed]
- Sabelnikov, A.; Santin, G.; Skorokhvatov, M.; Sobel, H.; Steele, J.; Steinberg, R.; Sukhotin, S.; Tomshaw, S.; Veron, D.; Vyrodov, V.; et al. Search for neutrino oscillations on a long base-line at the CHOOZ nuclear power station. Eur. Phys. J. C 2003, 27, 331–374. [Google Scholar]
- Abdurashitov, J.N.; Gavrin, V.N.; Girin, S.V.; Gorbachev, V.V.; Ibragimova, T.V.; Kalikhov, A.V.; Khairnasov, N.G.; Knodel, T.V.; Mirmov, I.N.; Shikhin, A.A.; et al. Measurement of the solar neutrino capture rate with gallium metal. Phys. Rev. C 1999, 60, 055801. [Google Scholar] [CrossRef]
- Adamson, P.; Aliaga, L.; Ambrose, D.; Anfimov, N.; Antoshkin, A.; Arrieta-Diaz, E.; Augsten, K.; Aurisano, A.; Backhouse, C.; Baird, M.; et al. Search for active-sterile neutrino mixing using neutral-current interactions in NOvA. Phys. Rev. D 2017, 96, 072006. [Google Scholar] [CrossRef]
- Buchmüller, W. Neutrino, Grand Unification and Leptogenesis. arXiv, 2004; arXiv:hep-ph/0204288. [Google Scholar]
- Capolupo, A.; Carloni, S.; Quaranta, A. Quantum flavor vacuum in the expanding universe: A possible candidate for cosmological dark matter? Phys. Rev. D 2022, 105, 105013. [Google Scholar] [CrossRef]
- Capolupo, A.; Giampaolo, S.M.; Lambiase, G.; Quaranta, A. Probing quantum field theory particle mixing and dark-matter-like effects with Rydberg atoms. EPJ C 2020, 80, 423. [Google Scholar] [CrossRef]
- Kaplan, D.B.; Nelson, A.E.; Weiner, N. Neutrino Oscillation as a probe of Dark Energy. Phys. Rev. Lett. 2004, 93, 091801. [Google Scholar] [CrossRef] [PubMed]
- Bilenky, S.M.; Pontecorvo, B. Lepton mixing and neutrino oscillations. Phys. Rep. 1978, 41, 225. [Google Scholar] [CrossRef]
- Bilenky, S.M.; Petcov, S.T. Massive neutrinos and neutrino oscillations. Rev. Mod. Phys. 1987, 59, 671. [Google Scholar] [CrossRef]
- Aad, G. et al. [ATLAS Collaboration] Observation of a new particle in the search for the standard Model Higgs boson with the ATLAS detector at the LHC. Phys. Lett. B 2012, 716, 1–29. [Google Scholar] [CrossRef]
- Fukuda, Y.; Hayakawa, T.; Ichihara, E.; Inoue, K.; Ishihara, K.; Ishino, H.; Itow, Y.; Kajita, T.; Kameda, J.; Kasuga, S.; et al. Evidence for Oscillation of Atmospheric Neutrinos. Phys. Rev. Lett. 1998, 81, 1562–1567. [Google Scholar] [CrossRef]
- Blasone, M.; Vitiello, G. Quantum Field Theory of Fermion Mixing. Ann. Phys. 1995, 244, 283–311. [Google Scholar] [CrossRef]
- Blasone, M.; Capolupo, A.; Vitiello, G. Quantum field theory of three flavor neutrino mixing and oscillations with CP violation. Phys. Rev. D 2002, 66, 025033. [Google Scholar] [CrossRef]
- Capolupo, A.; Capozziello, S.; Vitiello, G. Neutrino mixing as a source of dark energy. Phys. Lett. A 2007, 363, 53. [Google Scholar] [CrossRef]
- Capolupo, A. Dark matter and dark energy induced by condensates. Adv. High Energy Phys. 2016, 2016, 8089142. [Google Scholar] [CrossRef]
- Capolupo, A. Quantum vacuum, dark matter, dark energy and spontaneous supersymmetry breaking. Adv. High Energy Phys. 2018, 2018, 9840351. [Google Scholar] [CrossRef]
- Fujii, K.; Habe, C.; Yabuki, T. Note on the field theory of neutrino mixing. Phys. Rev. D 1999, 59, 113003. [Google Scholar] [CrossRef]
- Hannabuss, K.C.; Latimer, D.C. The quantum field theory of fermion mixing. J. Phys. A 2000, 33, 1369. [Google Scholar] [CrossRef]
- Blasone, M.; Capolupo, A.; Romei, O.; Vitiello, G. Quantum field theory of boson mixing. Phys. Rev. D 2001, 63, 125015. [Google Scholar] [CrossRef]
- Alfinito, E.; Blasone, M.; Iorio, A.; Vitiello, G. Squeezed neutrino Oscillations in Quantum Field Theory. Phys. Lett. B 1995, 362, 91. [Google Scholar] [CrossRef]
- Grossman, Y.; Lipkin, H.J. Flavor oscillations from a spatially localized source: A simple general treatment. Phys. Rev. D 1997, 55, 2760. [Google Scholar] [CrossRef]
- Piriz, D.; Roy, M.; Wudka, J. Neutrino oscillations in strong gravitational fields. Phys. Rev. D 1996, 54, 1587. [Google Scholar] [CrossRef] [PubMed]
- Cardall, C.Y.; Fuller, G.M. Neutrino oscillations in curved spacetime: A heuristic treatment. Phys. Rev. D 1997, 55, 7960. [Google Scholar] [CrossRef]
- Buoninfante, L.; Luciano, G.G.; Petruzziello, L.; Smaldone, L. Neutrino oscillations in extended theories of gravity. Phys. Rev. D 2020, 101, 024016. [Google Scholar] [CrossRef]
- Capolupo, A.; Giampaolo, S.M.; Quaranta, A. Geometric phase of neutrinos: Differences between Dirac and Majorana neutrinos. Phys. Lett. B 2021, 820, 136489. [Google Scholar] [CrossRef]
- Luciano, G.G. On the flavor/mass dichotomy for mixed neutrinos: A phenomenologically motivated analysis based on lepton charge conservation in neutron decay. EPJ Plus 2023, 138, 83. [Google Scholar] [CrossRef]
- Capolupo, A.; Quaranta, A. Neutrinos, mixed bosons, quantum reference frames and entanglement. J. Phys. G 2023, 50, 055003. [Google Scholar] [CrossRef]
- Capolupo, A.; Quaranta, A. neutrino capture on tritium as a probe of flavor vacuum condensate and dark matter. Phys. Lett. B 2023, 839, 137776. [Google Scholar] [CrossRef]
- Bilenky, S.M.; Hošek, J.; Petcov, S.T. On the oscillations of neutrinos with Dirac and Majorana masses. Phys. Lett. B 1980, 94, 495–498. [Google Scholar] [CrossRef]
- Capolupo, A.; Giampaolo, S.M.; Hiesmayr, B.C.; Lambiase, G.; Quaranta, A. On the geometric phase for Majorana and Dirac neutrinos. J. Phys. G 2023, 50, 025001. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Montanino, D.; Palazzo, A. Global analysis of three-flavor neutrino masses and mixing. Prog. Part. Nucl. Phys. 2006, 57, 742–795. [Google Scholar] [CrossRef]
- Capozzi, F.; Valentino, E.D.; Lisi, E.; Marrone, A.; Melchiorri, A.; Palazzo, A. Global constraints on absolute neutrino masses and their ordering. Phys. Rev. D 2017, 95, 096014. [Google Scholar] [CrossRef]
- Capozzi, F.; Lisi, E.; Marrone, A.; Montanino, D.; Palazzo, A. Neutrino masses and mixings: Status of known and unknown 3v parameters. Nucl. Phys. B 2016, 908, 218–234. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Montanino, D.; Palazzo, A. Observables sensitive to absolute neutrino masses. II. Phys. Rev. D 2008, 79, 033010. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Melchiorri, A.; Palazzo, A.; Serra, P.; Silk, J. Observables sensitive to absolute neutrino masses: Constraints and correlations from world neutrino data. Phys. Rev. D 2004, 70, 113003. [Google Scholar] [CrossRef]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Melchiorri, A.; Palazzo, A.; Serra, P.; Silk, J.; Slosar, A. Observables sensitive to absolute neutrino masses: A reappraisal after WMAP 3-year and first MINOS results. Phys. Rev. D 2007, 75, 053001. [Google Scholar] [CrossRef]
- Adak, M.; Dereli, T.; Ryder, H. Neutrino Oscillations Induced by Space-Time Torsion. Class. Quantum Grav. 2001, 18, 1503–1512. [Google Scholar] [CrossRef]
- Fabbri, L.; Vignolo, S. A torsional completion of gravity for Dirac matter fields and its applications to neutrino oscillations. Mod. Phys. Lett. A 2016, 31, 1650014. [Google Scholar] [CrossRef]
- Betti, M.G. et al. [PTOLEMY Collaboration] Neutrino physics with the PTOLEMY project: Active neutrino properties and the light sterile case. JCAP 2019, 2019, 47. [Google Scholar] [CrossRef]
- Kapusta, J.I. Neutrino superfluidity. Phys. Rev. Lett. 2004, 93, 251801. [Google Scholar] [CrossRef]
- Capolupo, A.; Lambiase, G.; Quaranta, A. Neutrinos in curved spacetime: Particle mixing and flavor oscillations. Phys. Rev. D 2020, 101, 095022. [Google Scholar] [CrossRef]
- Capolupo, A.; Lambiase, G.; Quaranta, A. Fermion mixing in curved spacetime. J. Phys. Conf. Ser. 2023, 2533, 012050. [Google Scholar] [CrossRef]
- Capolupo, A.; Quaranta, A.; Serao, R. Field Mixing in Curved Spacetime and Dark Matter. Symmetry 2023, 15, 807. [Google Scholar] [CrossRef]
- Capolupo, A.; Quaranta, A.; Setaro, P.A. Boson mixing and flavor oscillations in curved spacetime. Phys. Rev. D 2022, 106, 043013. [Google Scholar] [CrossRef]
- Capolupo, A.; Quaranta, A. Boson mixing and flavor vacuum in the expanding Universe: A possible candidate for the dark energy. Phys. Lett. B 2023, 840, 137889. [Google Scholar] [CrossRef]
- Blasone, M.; Lambiase, G.; Luciano, G.G.; Petruzziello, L.; Smaldone, L. Time-energy uncertainty relation for neutrino oscillation curved spacetime. Quantum Grav. 2020, 37, 155004. [Google Scholar] [CrossRef]
- Abi, B.; Acciarri, R.; Acero, M.A.; Adamov, G.; Adams, D.; Adinolfi, M.; Ahmad, Z.; Ahmed, J.; Alion, T.; Alonso Monsalve, S.; et al. Supernova neutrino burst detection with the Deep underground neutrino Experiment. EPJ 2021, 81, 423. [Google Scholar] [CrossRef]
- Abi, B.; Acciarri, R.; Acero, M.A.; Adamov, G.; Adams, D.; Adinolfi, M.; Ahmad, Z.; Ahmed, J.; Alion, T.; Monsalve, S.A.; et al. Long-baseline neutrino oscillation physics potential of the DUNE experiment. EPJ 2020, 80, 978. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capolupo, A.; De Maria, G.; Monda, S.; Quaranta, A.; Serao, R. Quantum Field Theory of Neutrino Mixing in Spacetimes with Torsion. Universe 2024, 10, 170. https://doi.org/10.3390/universe10040170
Capolupo A, De Maria G, Monda S, Quaranta A, Serao R. Quantum Field Theory of Neutrino Mixing in Spacetimes with Torsion. Universe. 2024; 10(4):170. https://doi.org/10.3390/universe10040170
Chicago/Turabian StyleCapolupo, Antonio, Giuseppe De Maria, Simone Monda, Aniello Quaranta, and Raoul Serao. 2024. "Quantum Field Theory of Neutrino Mixing in Spacetimes with Torsion" Universe 10, no. 4: 170. https://doi.org/10.3390/universe10040170