Modeling and Disturbance Analysis of Spinning Satellites with Inflatable Protective Structures
Abstract
:1. Introduction
- (1)
- A novel IPS is implemented to guarantee the smooth operation of spinning satellites;
- (2)
- The dynamic model of the spinning satellite with IPSs in the inflatable stage is decoupled into two separate models: the spring hinge unfolding model and the spring expansion model;
- (3)
- The multi-body dynamics method based on the Newton–Euler equations is utilized to develop both the spring hinge unfolding model and the spring expansion model;
- (4)
- Various operating conditions are taken into consideration to thoroughly analyze the effects on the spinning satellite during the unfolding or expansion of IPSs.
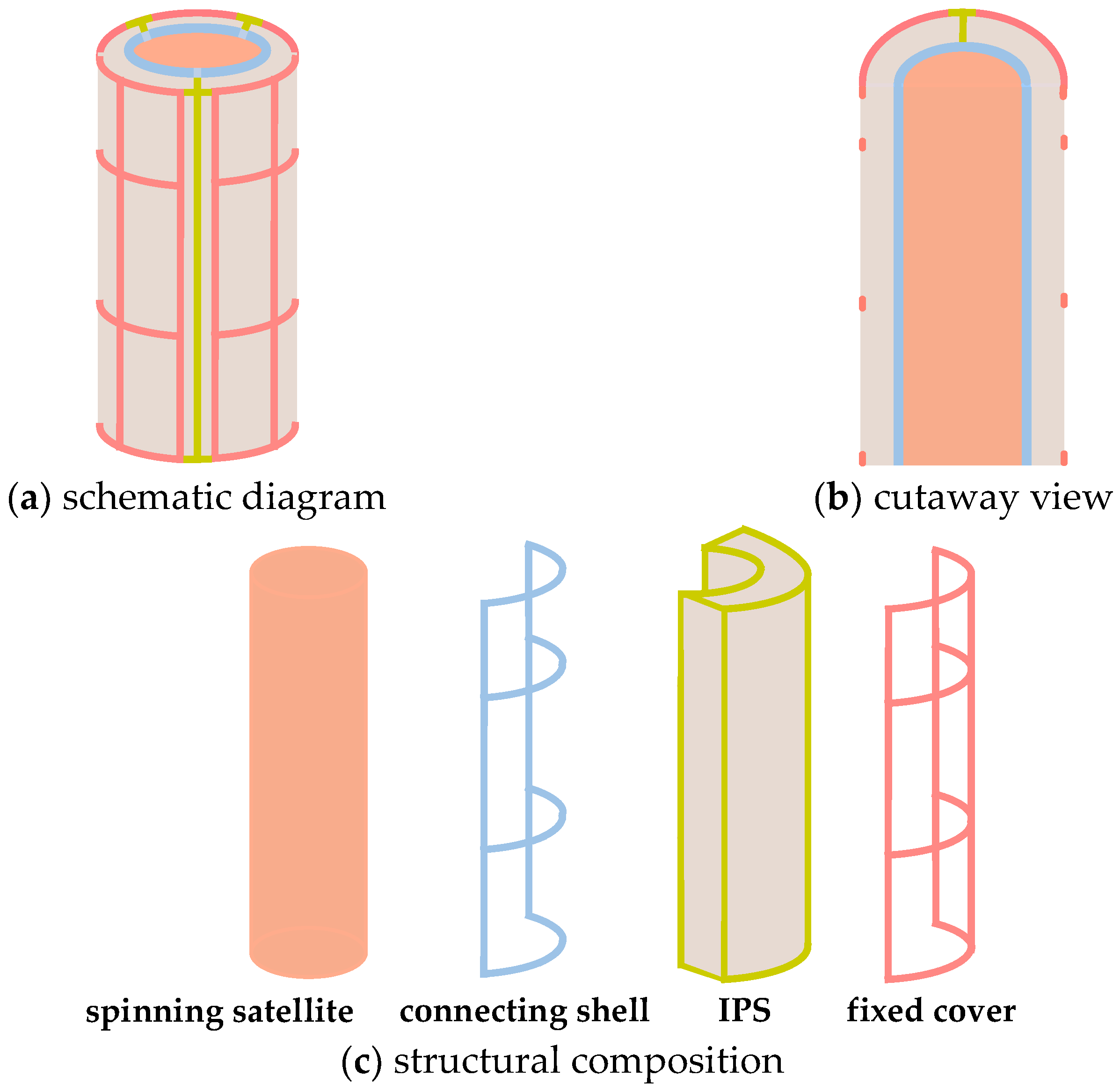
2. Description of the Spinning Satellite with IPSs
3. Dynamic Modeling of the Spinning Satellite with IPSs
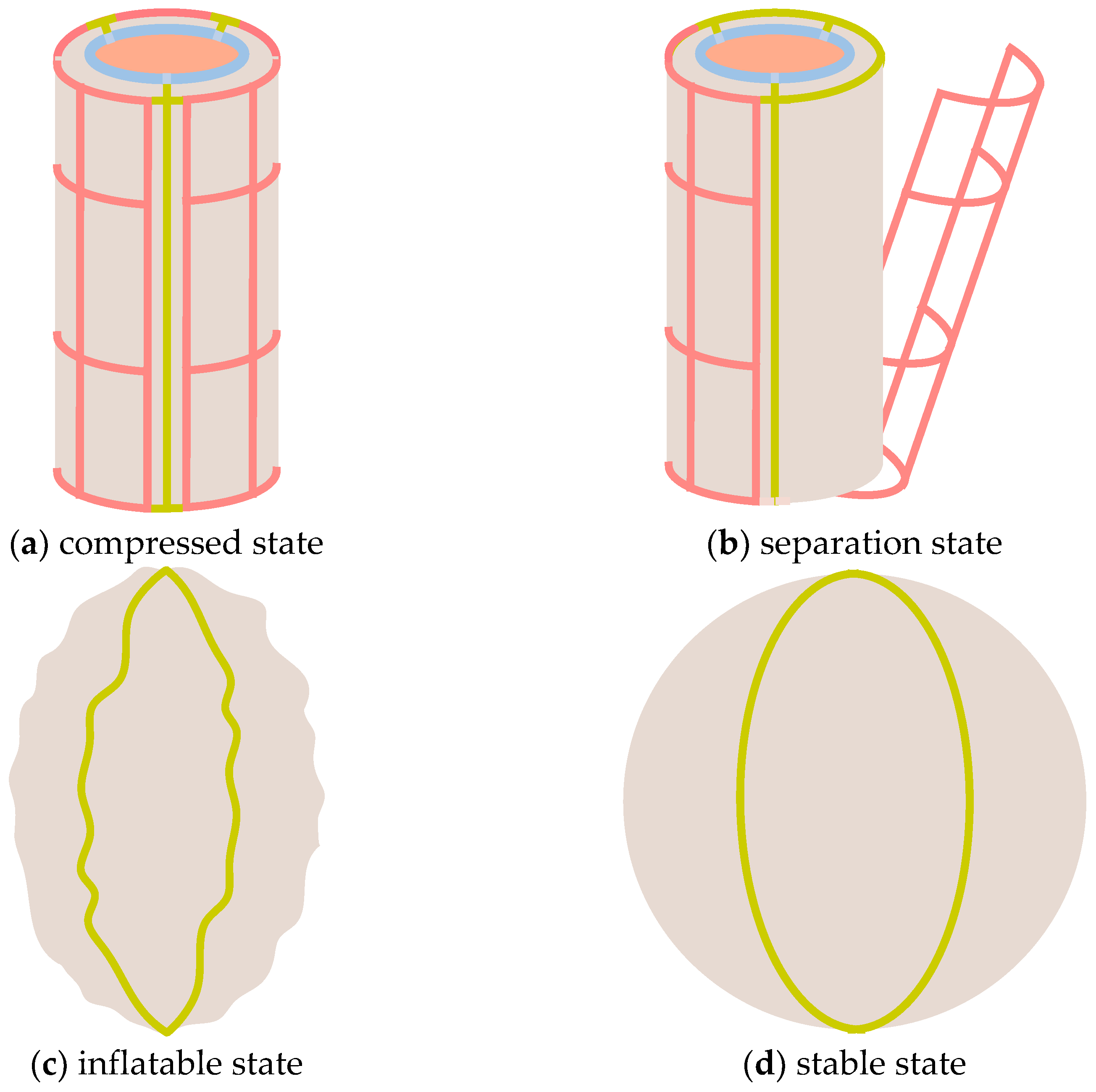
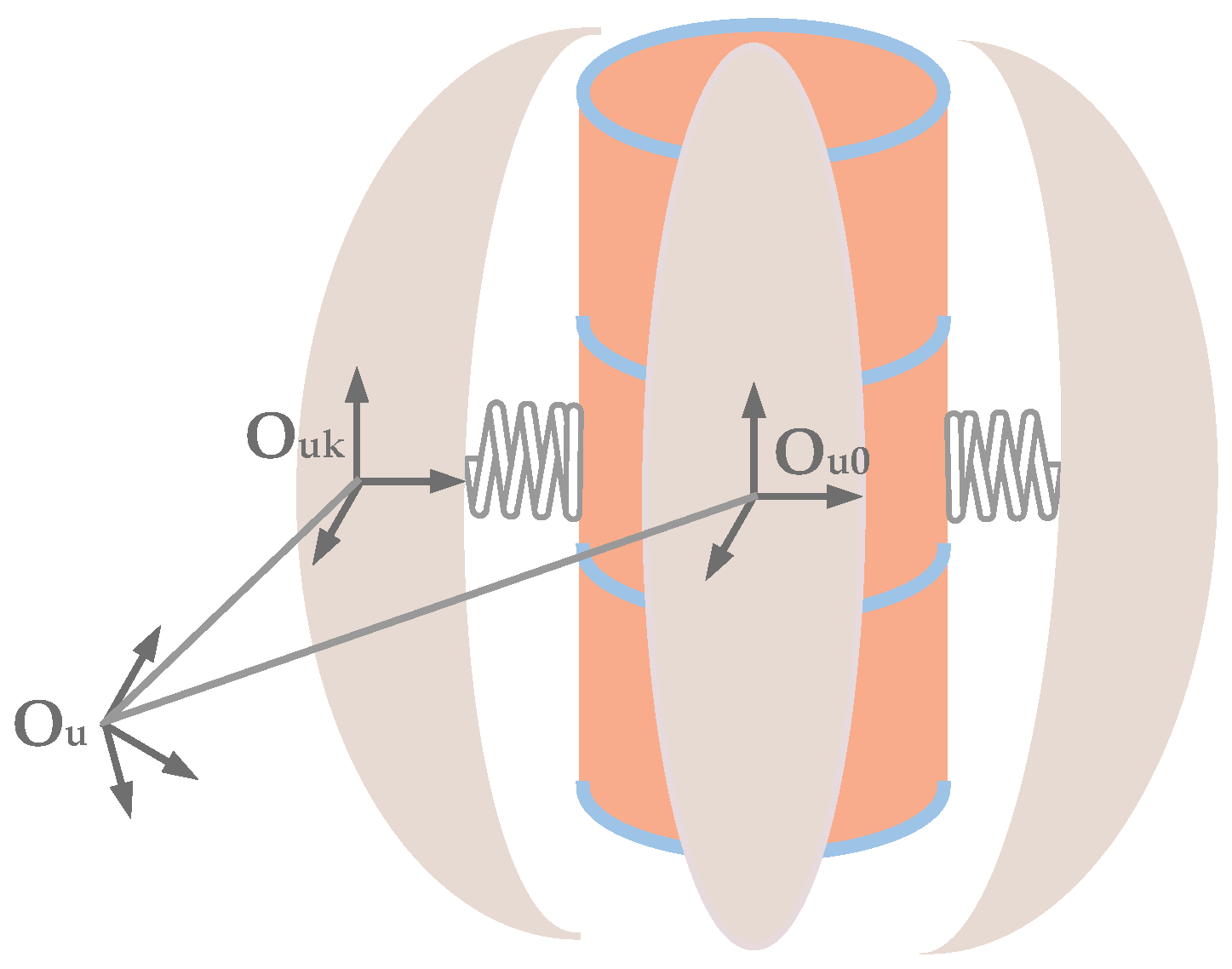
3.1. Spring Hinge Unfolding Model
3.2. Spring Expansion Model
4. Simulation Results and Analysis
4.1. IPS Unfolding Analysis
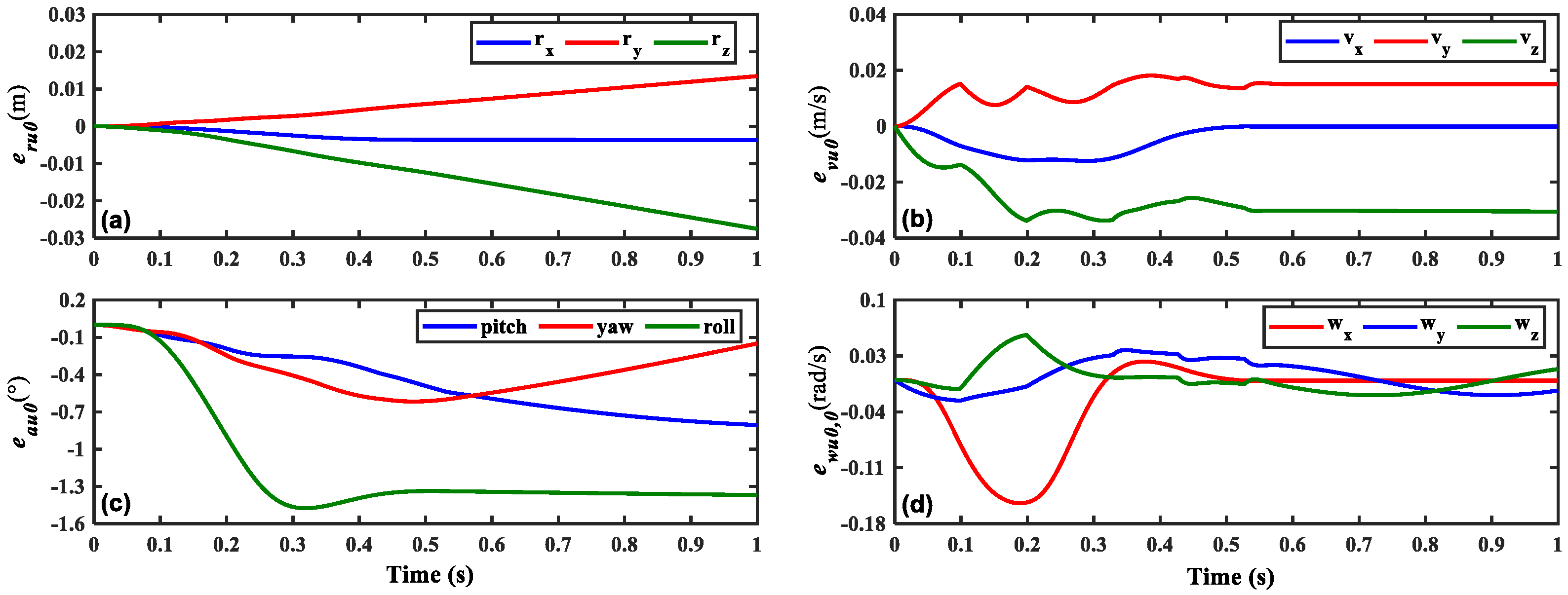
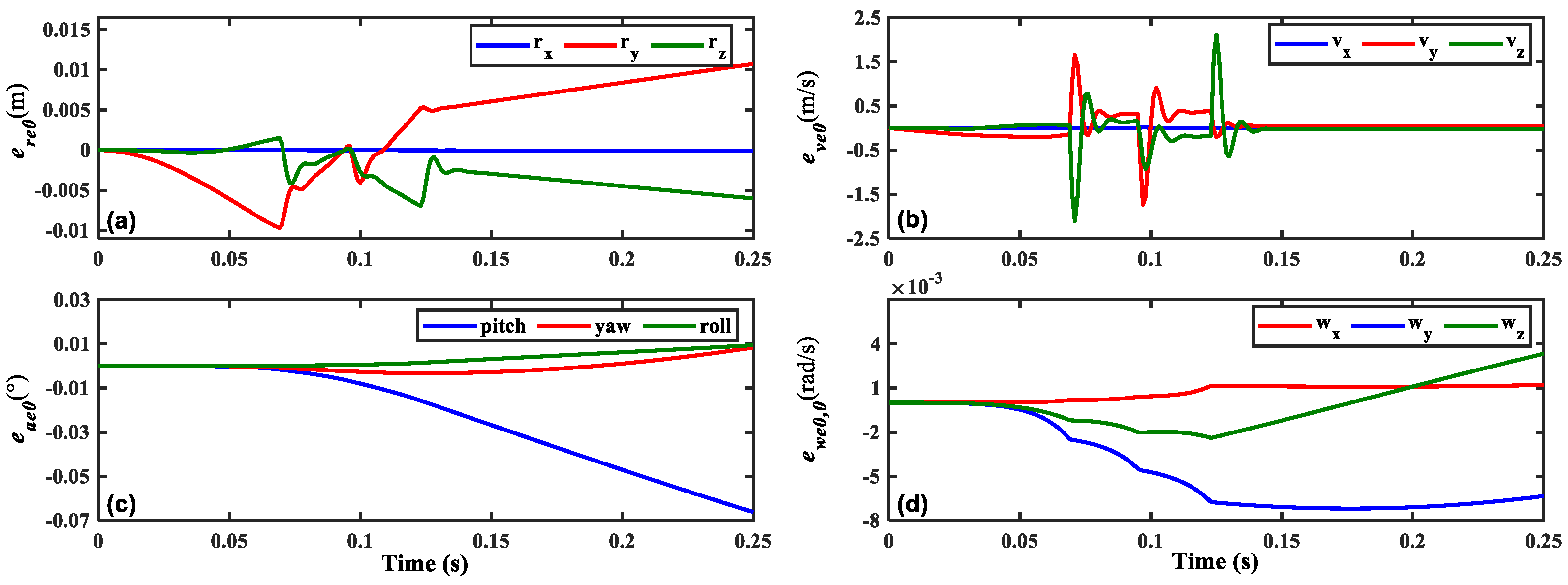
- The deviation motion curve of the spinning satellite between IPSs in the orbital coordinate system and free flight in the same orbital coordinate system is
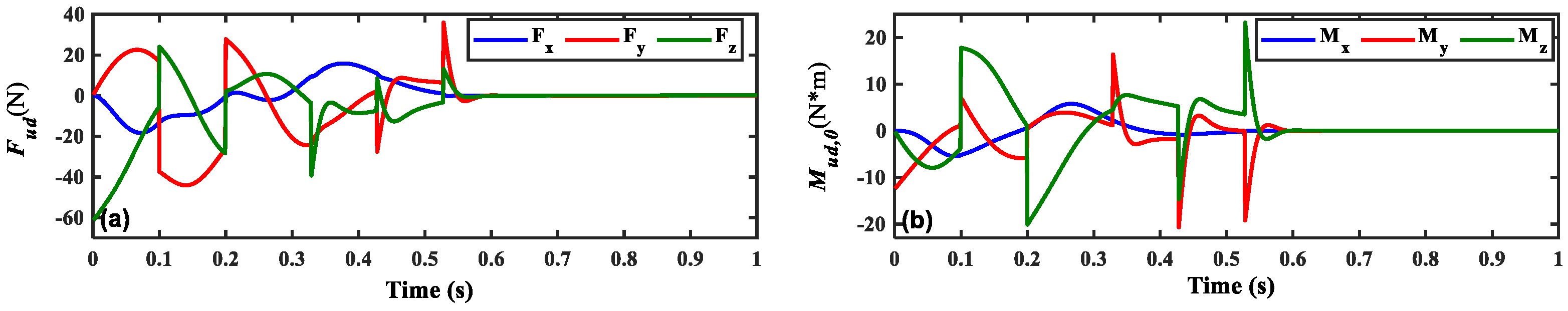
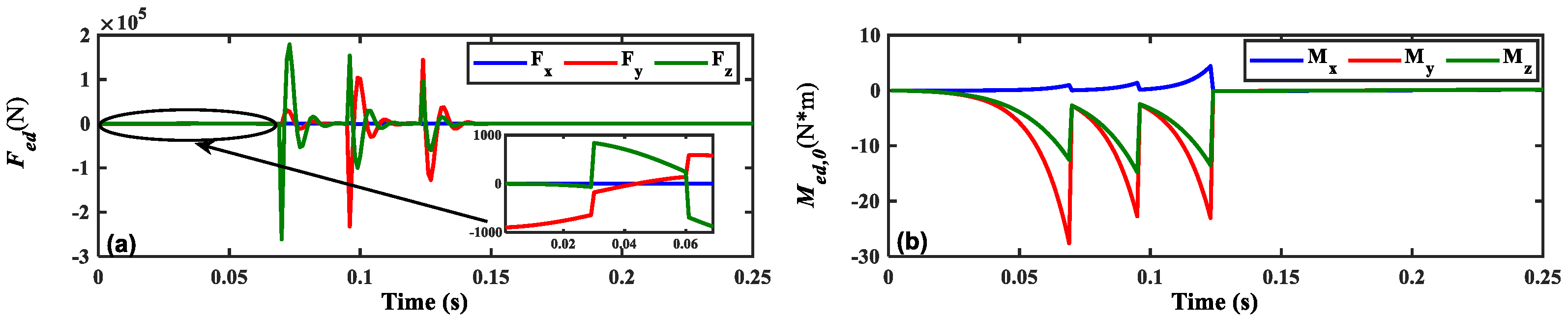
- The disturbance force and torque curve of the spinning satellite in the spinning satellite coordinate system are
4.1.1. Unfolding Interval of 0.01 s
4.1.2. Unfolding Interval of 0.4 s
4.2. IPS Expansion Analysis
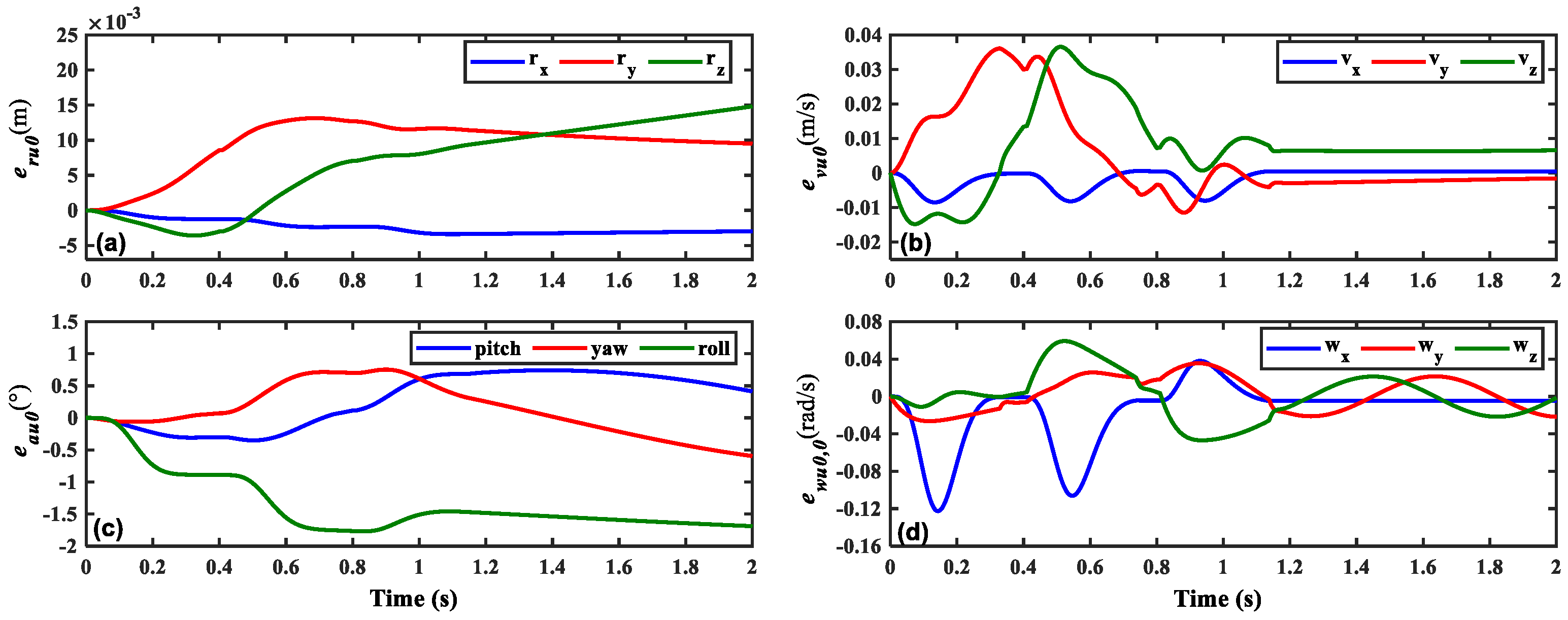
- The deviation motion curve of the spinning satellite between IPS expansion in the orbital coordinate system and free flight in the same orbital coordinate system is
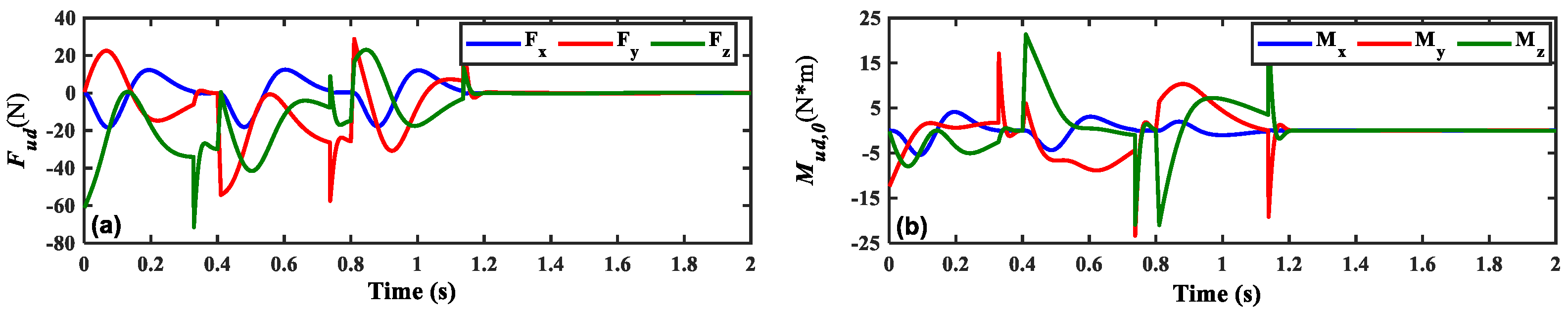
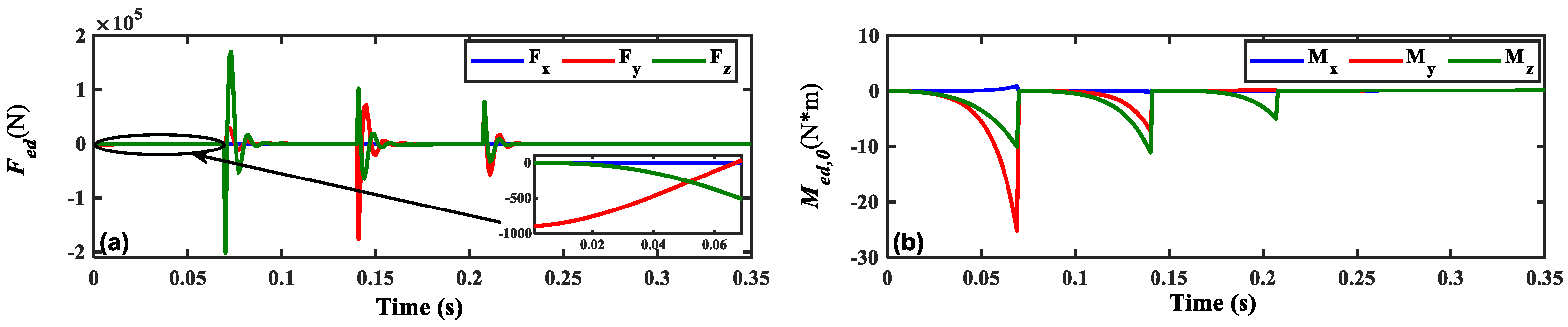
- The disturbance force and torque curve of the spinning satellite in the spinning satellite coordinate system are
4.2.1. Expansion Interval of 0.01 s
4.2.2. Expansion Interval of 0.08 s
4.3. Dynamic Model Verification
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| u | spring hinge unfolding | e | spring expansion |
| Ou | the composite center-of-mass orbital coordinate system (the reference frame) | Oe | the composite center-of-mass orbital coordinate system (the reference frame) |
| Ou0 | the spinning satellite coordinate system | Oe0 | the spinning satellite coordinate system |
| mu0 | the mass of the spinning satellite | me0 | the mass of the spinning satellite |
| Ju0 | the moment of inertia of the satellite | Je0 | the moment of inertia of the satellite |
| ru0 | the displacement of the spinning satellite | re0 | the displacement of the spinning satellite |
| qu0 | the quaternion of the spinning satellite | qe0 | the quaternion of the spinning satellite |
| vu0 | the velocity of the spinning satellite | ve0 | the velocity of the spinning satellite |
| wu0,0 | the angular velocity of the satellite in Ou0 | we0,0 | the angular velocity of the satellite in Oe0 |
| k | number of IPSs | k | number of IPSs |
| Ouk | the kth IPS coordinate system | Oek | the kth IPS coordinate system |
| muk | the mass of the kth IPS | mek | the mass of the kth IPS |
| Juk | the moment of inertia of the kth IPS | Jek | the moment of inertia of the kth IPS |
| ruk | the displacement of the kth IPS | rek | the displacement of the kth IPS |
| quk | the quaternion of the kth IPS | qek | the quaternion of the kth IPS |
| vuk | the velocity of the kth IPS | vek | the velocity of the kth IPS |
| wuk,k | the angular velocity of the kth IPS in Ouk | wek,k | the angular velocity of the kth IPS in Oek |
References
- Janssens, F.L.; van der Ha, J.C. On the stability of spinning satellites. Acta Astronaut. 2011, 68, 778–789. [Google Scholar] [CrossRef]
- Ergin, E.I.; Wheeler, P.C. Magnetic attitude control of a spinning satellite. J. Spacecr. Rocket. 1965, 2, 846–850. [Google Scholar] [CrossRef]
- Kane, T.R.; Barba, P.M. Effects of energy dissipation on a spinning satellite. AIAA J. 1966, 4, 1391–1394. [Google Scholar] [CrossRef]
- Janssens, F.L.; van der Ha, J.C. Stability of spinning satellite under axial thrust and internal mass motion. Acta Astronaut. 2014, 94, 502–514. [Google Scholar] [CrossRef]
- Greenbaum, D. Space debris puts exploration at risk. Science 2020, 370, 922. [Google Scholar] [CrossRef]
- Anttonen, A.; Kiviranta, M.; Höyhtyä, M. Space debris detection over intersatellite communication signals. Acta Astronaut. 2021, 187, 156–166. [Google Scholar] [CrossRef]
- Botta, E.M.; Sharf, I.; Misra, A.K. Simulation of tether-nets for capture of space debris and small asteroids. Acta Astronaut. 2019, 155, 448–461. [Google Scholar] [CrossRef]
- Svotina, V.V.; Cherkasova, M.V. Space debris removal–Review of technologies and techniques. Flexible or virtual connection between space debris and service spacecraft. Acta Astronaut. 2022, 204, 840–853. [Google Scholar] [CrossRef]
- Litteken, D.A. Inflatable technology: Using flexible materials to make large structures. Electroact. Polym. Actuators Devices 2019, 10966, 1096603. [Google Scholar]
- Harris, J. Advanced material and assembly methods for inflatable structures. In Proceedings of the 4th Aerodynamic Deceleration Systems Conference, Palm Springs, CA, USA, 21–23 May 1973. [Google Scholar]
- Cobb, R.G.; Black, J.T.; Swenson, E.D. Design and flight qualification of the rigidizable inflatable get-away-special experiment. J. Spacecr. Rocket. 2010, 47, 659–669. [Google Scholar] [CrossRef]
- Chandra, A.; Tonazzi, J.C.L.; Stetson, D.; Pat, T.; Walker, C.K. Inflatable membrane antennas for small satellites. In Proceedings of the 2020 IEEE Aerospace Conference, Big Sky, MT, USA, 7–14 March 2020. [Google Scholar]
- Freeland, R.E.; Helms, R.G.; Willis, P.B.; Mikulas, M.M.; Stuckey, W.; Steckel, G.; Watson, J. Inflatable space structures technology development for large radar antennas. In Proceedings of the 55th International Astronautical Congress, Vancouver, BC, Canada, 4–8 October 2004. [Google Scholar]
- Duan, T.; Wei, J.; Zhang, A.; Xu, Z.; Lim, T.C. Transmission error investigation of gearbox using rigid-flexible coupling dynamic model: Theoretical analysis and experiments. Mech. Mach. Theory 2021, 157, 104213. [Google Scholar] [CrossRef]
- Zhang, J.; Zhu, T.; Yang, B.; Wang, X.; Xiao, S.; Yang, G.; Liu, Y.; Che, Q. A rigid–flexible coupling finite element model of coupler for analyzing train instability behavior during collision. Railw. Eng. Sci. 2023, 4, 325–339. [Google Scholar] [CrossRef]
- Pagaimo, J.; Millan, P.; Ambrósio, J. Flexible multibody formulation using finite elements with 3 DoF per node with application in railway dynamics. Multibody Syst. Dyn. 2023, 30, 83–112. [Google Scholar] [CrossRef]
- Otsuka, K.; Wang, Y.; Palacios, R.; Makihara, K. Strain-based geometrically nonlinear beam formulation for rigid–flexible multibody dynamic analysis. AIAA J. 2022, 60, 4954–4968. [Google Scholar] [CrossRef]
- Luo, Y.; Qian, F.; Sun, H.; Wang, X.; Chen, A.; Zuo, L. Rigid-flexible coupling multi-body dynamics modeling of a semi-submersible floating offshore wind turbine. Ocean Eng. 2023, 281, 114648. [Google Scholar] [CrossRef]
- Htun, T.Z.; Suzuki, H.; García-Vallejo, D. On the theory and application of absolute coordinates-based multibody modelling of the rigid–flexible coupled dynamics of a deep-sea ROV-TMS (tether management system) integrated model. Ocean Eng. 2022, 258, 111748. [Google Scholar] [CrossRef]
- Eisa, A.I.; Shusen, L.; Helal, W.M. Study on the lateral and torsional vibration of single rotor-system using an integrated multi-body dynamics and finite element analysis. Adv. Mech. Eng. 2020, 12, 168. [Google Scholar] [CrossRef]
- Kashyzadeh, K.R.; Farrahi, G.H. Improvement of HCF life of automotive safety components considering a novel design of wheel alignment based on a Hybrid multibody dynamic, finite element, and data mining techniques. Eng. Fail Anal. 2023, 143, 106932. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, Z.; Wang, L.; Li, D.; Jin, Z. A patient-specific wear prediction framework for an artificial knee joint with coupled musculoskeletal multibody-dynamics and finite element analysis. Tribol. Int. 2017, 109, 382–389. [Google Scholar] [CrossRef]
- Xu, J.; Zhang, L.; Li, X.; Li, S.; Yang, K. A study of dynamic response of a wind turbine blade based on the multi-body dynamics method. Renew. Energy 2020, 155, 358–368. [Google Scholar] [CrossRef]
- Wu, X.; Cui, H.; Chen, W.; Xie, Y.; Zhao, H.; Bai, W. Integral dynamics modelling of chain-like space robot based on n-order dual number. Acta Astronaut. 2020, 177, 552–560. [Google Scholar]
- Gießler, M.; Waltersberger, B. Robust inverse dynamics by evaluating Newton–Euler equations with respect to a moving reference and measuring angular acceleration. Auton Robot. 2023, 47, 465–481. [Google Scholar]
- Caruso, M.; Bregant, L.; Gallina, P.; Seriani, S. Design and multi-body dynamic analysis of the Archimede space exploration rover. Acta Astronaut. 2022, 194, 229–241. [Google Scholar]
- Sarkar, S.; Fitzgerald, B. Use of kane’s method for multi-body dynamic modelling and control of spar-type floating offshore wind turbines. Energies 2021, 14, 6635. [Google Scholar]
- Sajeer, M.M.; Mitra, A.; Chakraborty, A. Multi-body dynamic analysis of offshore wind turbine considering soil-structure interaction for fatigue design of monopile. Soil Dyn. Earthq. Eng. 2021, 144, 106674. [Google Scholar]
- Zhang, Y.; Wu, G.; Zhao, G. Response of centrifugal pendulum vibration absorber considering gravity at low engine speed. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2022, 236, 274–290. [Google Scholar] [CrossRef]
- Wang, J.; Wang, S.; Le, H. Rigid-flexible coupled multi-body dynamics analysis of horizontal directional drilling rig system. J. Comput. Methods Sci. Eng. 2020, 20, 975–995. [Google Scholar]
- Zhang, Y.; Duan, B.; Li, T. A controlled deployment method for flexible deployable space antennas. Acta Astronaut. 2012, 81, 19–29. [Google Scholar] [CrossRef]
- Luo, C.; Sun, J.; Wen, H. Autonomous separation deployment dynamics of a space multi-rigid-body system with uncertain parameters. Mech. Mach. Theory 2023, 180, 104175. [Google Scholar]
- James, S.; James, P.; Giuseppe, L.; Vijay, K. IMU-Based Inertia Estimation for a Quadrotor Using Newton-Euler Dynamics. IEEE Robot. Autom. Lett. 2020, 5, 3861–3867. [Google Scholar]


| Parameter | Description | Value |
|---|---|---|
| m0 | the mass of the spinning satellite | 150 kg |
| mk | the mass of the kth IPS | 2 kg |
| r0 | the displacement of the spinning satellite | [0 m 0 m 0 m]T |
| v0 | the velocity of the spinning satellite | [0 m/s 0 m/s 0 m/s]T |
| a0 | the Euler angle of the spinning satellite | [0° 0° 0°]T |
| w0,0 | the angular velocity of the spinning satellite | [10 rad/s 0.03 rad/s 0.03 rad/s]T |
| h | the height of the spinning satellite | 1.7 m |
| r | the radius of the spinning satellite | 0.6 m |
| the Euler angle of the kth IPS | 0° | |
| wk | the angular velocity of the kth IPS | 0 rad/s |
| Parameter | The Finite Element Model | The Proposed Dynamic Model |
|---|---|---|
| Fx | 74.5123 N | 72.2888 N (2.9841%) |
| Fy | 15,395 N | 14,895 N (3.2478%) |
| Fz | 18,063 N | 17,463 N (3.3217%) |
| Mx | 2.6426 N*m | 2.5355 N*m (4.0528%) |
| My | 6.2578 N*m | 6.0007 N*m (4.1084%) |
| Mz | 8.2648 N*m | 8.1714 N*m (1.1301%) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shang, Y.; Deng, Y.; Cai, Y.; Chen, Y.; He, S.; Liao, X.; Jiang, H. Modeling and Disturbance Analysis of Spinning Satellites with Inflatable Protective Structures. Aerospace 2023, 10, 971. https://doi.org/10.3390/aerospace10110971
Shang Y, Deng Y, Cai Y, Chen Y, He S, Liao X, Jiang H. Modeling and Disturbance Analysis of Spinning Satellites with Inflatable Protective Structures. Aerospace. 2023; 10(11):971. https://doi.org/10.3390/aerospace10110971
Chicago/Turabian StyleShang, Yuting, Yifan Deng, Yuanli Cai, Yu Chen, Sirui He, Xuanchong Liao, and Haonan Jiang. 2023. "Modeling and Disturbance Analysis of Spinning Satellites with Inflatable Protective Structures" Aerospace 10, no. 11: 971. https://doi.org/10.3390/aerospace10110971





