Variable-Order Fractional Scale Calculus

Abstract

General variable-order fractional scale derivatives are introduced and studied. Both the stretching and the shrinking cases are considered for definitions of the derivatives of the GL type and of the Hadamard type. Their properties are deduced and discussed. Fractional variable-order systems of autoregressive–moving-average type are introduced and exemplified. The corresponding transfer functions are obtained and used to find the corresponding impulse responses.

1. Introduction

According to L. Nottale [1], scale is the resolution with which measurements are made. It is therefore a relative entity, since what is really important are the transformations introduced by appropriate operators such as scale derivatives. L. Cohen [2] considered scale to be a physical attribute similar to frequency. In most applications, the important factor is scale invariance [3,4], which has made it possible to establish bridges to other formulations such as Lamperti’s transformations for stochastic processes [5,6] and the operator theory [7]. Some interesting applications can be referred; for example: in scale relativity [8,9], in the study of the Schrödinger equation [10,11], in transport and relaxation [12,13], in economic phenomena [14,15], in the dynamics of spontaneous behaviour [3], in neurons [16], and so on. A very important tool in which scale is the fundamental notion is the multiscale/multiresolution analysis obtained from the wavelet transform [17,18,19,20,21] introduced in the 1980s in signal processing. This is mainly an analysis tool.

The concept of a scale-invariant system is not very old, although the term “scale” was being used for decades. In fact, it seems that the first tool that can be considered as a scale system was the Braccini and Gambardella “form-invariant” filter [22]. Linear scale invariant systems were really introduced by Yazici and Kashyap [23] to analyse and model $1/f$ phenomena and self-similar processes in general, namely scale stationary processes. However, their approach was based on an integer order Euler–Cauchy differential equation, without introducing any fractional derivative. A fractional scale-invariant linear system based on the generalization of the Euler–Cauchy equation using the fractional quantum derivative was introduced by M. Ortigueira [24]. The realization of the relationship between scale invariance and the Mellin convolution led to the discovery of the correct role played by the Hadamard derivative in the definitions of scale-invariant systems [25,26,27].

Hadamard derivatives, suggested by Hadamard [28] and studied, for the first time, in [29], were used in recent years in conjunction with other derivatives, or by substituting them in differential equations. However, they have no clear autonomy relatively to the usual Riemann–Liouville (RL) and Caputo (C) derivatives [30,31,32,33].

In [26], scale derivatives, in general, and Hadamard’s in particular, were obtained from the eigenvalue/eigenfunction associated with the Mellin convolution. This approach led to the discovery of stretching and shrinking derivatives expressed in two ways: integral and Grünwald–Letnikov-like. In this paper, the same methodology is used to generalise those results for the case where the fractional order is not constant, but variable. Indeed, when the order of a derivative is not restricted to integers, it can vary continuously: a situation far more interesting than the discontinuous variations which are the only possibility if orders are integer. In the development of the theory, we had to face the same problems we found some time ago when studying shift-invariant derivatives. In the context of shift-invariant systems and the associated Liouville formulation, variable-order fractional derivatives were introduced by Samko and Ross [34,35,36] from the Riemann–Liouville and Marchaud definitions, by Lorenzo and Hartley [37] from the Grünwald–Letnikov (GL) definition, and by Coimbra [38] from the Caputo definition. These possibilities were systematically explored in [39], and then developed in [40,41,42] to obtain recursive formulations, also for discrete-time systems [43].

However, we verified that not all of the operators reached in the above-quoted literature satisfy requirements that can be reasonably demanded of a differential operator [44,45,46]. Since it is possible to extend the results in [26] for variable orders with the application of a similar reasoning, we will perform as such in this paper, and show that the extension verifies the applicability of the criteria in [44,45,46] to the resulting operators. We must highlight an important fact: the variable order may depend on different parameters. We will assume that it varies with the same independent variable used in the functions at hand.

The rest of this paper is organised as follows: Section 2 sums up the results needed to present variable-order scale derivatives in Section 3, namely variable-order Grünwald–Letnikov-like derivatives in Section 3.1 and variable-order Hadamard derivatives in Section 3.2. Then Section 3.3 has a discussion of the desirable properties of a variable-order differential operator, and the extent to which they are verified with variable-order scale derivatives. Section 4 is concerned with variable-order scale-invariant systems, and Section 5 concludes the paper.

2. On Scale-Invariant Systems: Hadamard Derivatives

Fractional scale-invariant systems are based on the scale derivatives deduced from the Hadamard approach. The definitions and results in this section can be found with further details in [26].

We begin with some basic definitions:

Definition 1. 

A single-input, single-output (SISO) system T, i.e., a functional that transforms scalar input $x\left(t\right)$ into scalar output $y\left(t\right)$, is linear if

$$y\left(t\right)=T[a_1{x}_1\left(t\right)+a_2{x}_2\left(t\right)]=a_{1}T\left[x_1\left(t\right)\right]+a_{2}T\left[x_2\left(t\right)\right]=a_1{y}_1\left(t\right)+a_2{y}_2\left(t\right),$$

(1)

where $y_1\left(t\right)=T\left[x_1\left(t\right)\right]$ and $y_2\left(t\right)=T\left[x_2\left(t\right)\right].$

Definition 2. 

A linear SISO system T is scale-invariant or dilation-invariant (DI) if the input/output relation is stated by the Mellin convolution, denoted as ★:

$$\begin{array}{c}\hfill y\left(\tau \right)=x\left(\tau \right)★g\left(\tau \right)={\int }_0^{+\infty }x\left(\frac{\tau }{\eta }\right)g\left(\eta \right)\frac{\mathrm{d}\eta }{\eta },\end{array}$$

(2)

where τ is restricted to ${\mathbb{R}}^{+}$. When the input is a unit-scaled impulse, $x\left(\tau \right)=\delta (\tau -1)$, the output of the system is the impulse response, $y\left(\tau \right)=g\left(\tau \right)$.

Theorem 1 

(ref. [29,47]). The powers ${\tau }^v,\tau \in {\mathbb{R}}^{+},v\in \mathbb{C}$ are the eigenfunctions of the DI systems: if $x\left(\tau \right)={\tau }^v$, then

$$y\left(\tau \right)=G\left(v\right){\tau }^v,$$

(3)

where $G\left(v\right)$ is the transfer function of the system. It is given by the Mellin transform (MT) of the impulse response:

$$G\left(v\right)=\mathcal{M}\left[g\left(\tau \right)\right]={\int }_0^{+\infty }g\left(\eta \right){\eta }^{-v-1}\mathrm{d}\eta ,$$

(4)

that converges in a vertical strip of the complex plane called region of convergence (ROC).

Indeed, letting $x\left(\tau \right)={\tau }^v$ and using (2), we obtain

$$y\left(\tau \right)=x\left(\tau \right)★g\left(\tau \right)={\int }_0^{+\infty }\frac{{\tau }^v}{{\eta }^v}g\left(\eta \right)\frac{\mathrm{d}\eta }{\eta },$$

from where the result follows.

The inverse Mellin transform of $G\left(v\right)$, for $\tau \in {\mathbb{R}}^{+}$, is given by

$$g\left(\tau \right)={\mathcal{M}}^{-1}\left[G\left(v\right)\right]={\displaystyle \frac{1}{2\pi i}}{\int }_{\gamma }G\left(v\right){\tau }^v\mathrm{d}v,$$

(5)

where $\gamma$ is a vertical straight line lying in the region of convergence of the transform.

Remark 1. 

The MT is an integral transform, and its definition given in (4) has a parameter sign change $-v\to v$ relative to the usual MT [48,49,50]. This definition has the advantage of establishing a better parallelism with the bilateral Laplace transform, concerning the region of convergence [51].

Definition 3. 

The α-order scale derivative (SD) is an operator ${\mathfrak{D}}_s^{\alpha }$ such that

$${\mathfrak{D}}_s^{\alpha }{\tau }^v=v^{\alpha }{\tau }^v,$$

(6)

provided that

$$\begin{array}{cc}\hfill \tau & \in {\mathbb{R}}^{+},\hfill \\ \hfill v& \in \mathbb{C},\hfill \\ \hfill \alpha & \in \mathbb{R}.\hfill \end{array}$$

If $\Re \left(v\right)>0$, there is expansion (stretching); if $\Re \left(v\right)<0$, there is shrinkage.

Definition 4. 

More generally, the α-order SD of $x\left(\tau \right)$, having $X\left(v\right)=\mathcal{M}\left[x\left(\tau \right)\right]$, as MT, is given by

$$\begin{array}{cc}\hfill {\mathfrak{D}}_s^{\alpha }x\left(\tau \right)& =\frac{1}{2\pi i}{\int }_{\gamma }{v}^{\alpha }X\left(v\right){\tau }^v\mathrm{d}v,\hfill \end{array}$$

(7)

where γ is a vertical straight line in the ROC of $X\left(v\right)$.

Corollary 1. 

This definition means that

$$\mathcal{M}\left[{\mathfrak{D}}_{s\pm }^{\alpha }x\left(\tau \right)\right]=v^{\alpha }X\left(v\right),\pm \Re \left(v\right)>0.$$

(8)

Similarly to the shift-invariant case, (8) shows that the scale derivative is an elemental system with transfer function $v^{\alpha }$. The way we express it in the inverse scale domain leads to various expressions for the derivative, as we will see below.

Theorem 2. 

The stretching (+) and shrinking (-) GL-type derivatives are given by

$${\mathfrak{D}}_{s\pm }^{\alpha }x\left(\tau \right)=\underset{q\to 1^{+}}{lim}{ln}^{-\alpha }\left(q^{\pm 1}\right)\sum _{n=0}^{\infty }\frac{{(-\alpha )}_n}{n!}x\left(\tau q^{\mp n}\right),$$

(9)

for $q>1$.

Proof. 

The reasoning behind definition (9) is as follows [26]. From the series expansion of the exponential, we have

$$1-q^{-v}=1-e^{-vln\left(q\right)}=vln\left(q\right)-{(vln\left(q\right))}^2+\dots$$

(10)

This can be used to find the following limit for $\Re \left(v\right)>0$:

$$\begin{array}{cc}\hfill \underset{q\to 1}{lim}{ln}^{-\alpha }\left(q\right){(1-q^{-v})}^{\alpha }& =\underset{q\to 1}{lim}{\left[{\displaystyle \frac{vln\left(q\right)-{(vln\left(q\right))}^2+\dots }{lnq}}\right]}^{\alpha }.\hfill \end{array}$$

(11)

Neglecting higher-order terms, it is clear that

$$\begin{array}{cc}\hfill \underset{q\to 1}{lim}{ln}^{-\alpha }\left(q\right){(1-q^{-v})}^{\alpha }& =v^{\alpha }.\hfill \end{array}$$

(12)

Thanks to the binomial theorem

$$\begin{array}{c}\hfill {(1-q^{\pm v})}^{\alpha }=\sum _{n=0}^{\infty }{\displaystyle \frac{{(-\alpha )}_n}{n!}}{q}^{\pm nv},\end{array}$$

(13)

where ${\left(x\right)}_n=x(x+1)\dots (x+n-1)$ is the Pochhammer symbol, we obtain

$$\begin{array}{c}\hfill v^{\alpha }=\underset{q\to 1}{lim}{ln}^{-\alpha }\left(q\right)\sum _{n=0}^{\infty }{\displaystyle \frac{{(-\alpha )}_n}{n!}}{q}^{\pm nv}.\end{array}$$

(14)

Finally, we can use the dilation property of the MT

$$\begin{array}{cc}\hfill \mathcal{M}\left[x\left(a\tau \right)\right]& =a^{v}X\left(v\right)\hfill \\ \hfill \Rightarrow \mathcal{M}\left[x\left(\tau q^{-n}\right)\right]& =q^{-nv}X\left(v\right)a\in {\mathbb{R}}^{+}.\hfill \end{array}$$

(15)

Returning to (9), we reobtain (6), and if we constrain q to be greater than 1,

$$\begin{array}{cc}\hfill {\mathfrak{D}}_s^{\alpha }x\left(\tau \right)& =\underset{q\to 1}{lim}{ln}^{-\alpha }\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha )}_n}{n!}{q}^{-nv}x\left(\tau \right)\hfill \\ \hfill & =v^{\alpha }x\left(\tau \right),\hfill \end{array}$$

(16)

as expected. □

3. Variable-Order Scale Derivatives

3.1. GL-Like Derivatives

Some variable-order Hadamard derivatives have been proposed [32,52,53,54]. However, they reveal the same problems of the similar proposals for Liouville derivatives, as pointed out in [44]. A different alternative definition consists of a full transform approach proposed by Scarpi [55] that presents different difficulties. Although it can also be adapted to scale derivatives, we will not perform as such.

In this paper, we prefer to adhere closely to the approach introduced in [44] to obtain variable-order (VO) scale derivatives. We will describe the framework for both stretching and shrinking cases, although we only present the proofs for the former.

We return to (9) to obtain the following:

Definition 5. 

Let $\alpha \left(t\right)\in \mathbb{R}$ be a bounded piecewise continuous function and $q>1$. The VO stretching and shrinking GL-like scale derivatives are defined by

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}x\left(\tau \right)& =\underset{q\to 1^{+}}{lim}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_n}{n!}x\left(\tau q^{-n}\right),\hfill \end{array}$$

(17)

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s-}^{\alpha \left(\tau \right)}x\left(\tau \right)& =\underset{q\to 1^{+}}{lim}{(-1)}^{\alpha \left(\tau \right)}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_n}{n!}x\left(\tau q^n\right).\hfill \end{array}$$

(18)

These definitions generalise (9), while preserving the following important property of scale derivatives.

Theorem 3. 

Making $x\left(\tau \right)={\tau }^v$ in (17), with $v\in \mathbb{C}$, we obtain

$${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}{\tau }^v=\underset{q\to 1^{+}}{lim}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{k=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_k}{k!}{\tau }^v{q}^{\mp nv}=v^{\alpha \left(\tau \right)}{\tau }^v,for\Re (\pm v)>0.$$

(19)

Proof. 

This can be proven by replacing $x\left(\tau \right)$ with ${\tau }^v$ and using the results mentioned to justify the reasoning behind definition (9). □

This result can be used to define VO SD for functions with MT using the Bromwich inversion integral, in a manner similar to (7), as follows.

Definition 6. 

Let $x\left(\tau \right)$ be a function having MT $X\left(v\right)$, with a non-void ROC defined by $\Re (\pm v)>a\in \mathbb{R}$. Then, the VO scale derivative of $x\left(\tau \right)$ is given by

$${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}x\left(\tau \right)=\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{v}^{\alpha \left(\tau \right)}X\left(v\right){\tau }^v\mathrm{d}v,\tau \in {\mathbb{R}}^{+},$$

(20)

where $\pm \sigma >a$.

3.2. VO Hadamard Derivatives

To introduce variable-order Hadamard derivatives, we recover the result (8):

$$\mathcal{M}\left[{\mathfrak{D}}_{s\pm }^{\alpha }x\left(\tau \right)\right]=v^{\alpha }X\left(v\right),\pm \Re \left(v\right)>0.$$

As there exist two MT inverses of $v^{\alpha }$, according to the chosen ROC, we can obtain two integral versions of the scale derivative, that we will call Hadamard derivatives.

Theorem 4. 

The MT inverses of $v^{\alpha }$, for $\alpha <0$, are given by

$$\begin{array}{cc}\hfill {\mathcal{M}}^{-1}\left[v^{\alpha }\right]\left(\tau \right)& ={\displaystyle \frac{{ln}^{-\alpha -1}\left(\tau \right)}{\Gamma (-\alpha )}}\epsilon (\tau -1),\Re \left(v\right)>0,\hfill \end{array}$$

(21)

$$\begin{array}{cc}\hfill {\mathcal{M}}^{-1}\left[v^{\alpha }\right]\left(\tau \right)& ={\displaystyle \frac{{ln}^{-\alpha -1}(1/\tau )}{\Gamma (-\alpha )}}\epsilon (1-\tau ),\Re \left(v\right)<0,\hfill \end{array}$$

(22)

where $\epsilon \left(x\right)$ is the Heaviside function

$$\epsilon \left(x\right)=\left\{\begin{array}{cc}1\hfill & x\ge 0\hfill \\ 0\hfill & x<0.\hfill \end{array}\right.$$

Proof. 

The first expression is found, for any $\beta <0$, as

$$\begin{array}{c}\hfill \frac{1}{v^{\beta }}={\int }_0^{\infty }{\displaystyle \frac{{\eta }^{\beta -1}}{\Gamma \left(\beta \right)}}{e}^{-v\eta }\mathrm{d}\eta ={\int }_1^{\infty }{\displaystyle \frac{{ln}^{\beta -1}\left(\tau \right)}{\Gamma \left(\beta \right)}}{\tau }^{-v-1}\mathrm{d}\tau .\end{array}$$

(23)

The second is obtained by replacing $\tau$ with $\frac{1}{\tau }$. □

With these MT inverses and using the Mellin convolution, we obtain two new scale anti-derivatives:

Definition 7. 

For $\alpha <0$ and $\tau \in {\mathbb{R}}^{+}$, the Hadamard integrals (anti-derivatives) are given by [29,30]:

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{\alpha }x\left(\tau \right)& ={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_1^{\infty }x(\tau /\eta ){ln}^{-\alpha -1}\left(\eta \right){\displaystyle \frac{\mathrm{d}\eta }{\eta }}={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_0^{\tau }x\left(u\right){ln}^{-\alpha -1}(\tau /u){\displaystyle \frac{\mathrm{d}u}{u}},\hfill \end{array}$$

(24)

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s-}^{\alpha }x\left(\tau \right)& ={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_0^{1}x(\tau /\eta ){ln}^{-\alpha -1}(1/\eta ){\displaystyle \frac{\mathrm{d}\eta }{\eta }}={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_{\tau }^{\infty }x\left(u\right){ln}^{-\alpha -1}(u/\tau ){\displaystyle \frac{\mathrm{d}u}{u}}.\hfill \end{array}$$

(25)

These integrals become singular when $\alpha >0$, but can be regularised using logarithmic series, as shown in [26]:

Definition 8. 

Regularised Hadamard derivatives are given by

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{\alpha }x\left(\tau \right)& ={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_0^{\tau }\left[x\left(u\right)-\sum _{n=0}^{N-1}{(-1)}^n{\displaystyle \frac{{\mathfrak{D}}_{s+}^{n}x\left(\tau \right)}{n!}}{ln}^n(\tau /u)\right]{ln}^{-\alpha -1}(\tau /u){\displaystyle \frac{\mathrm{d}u}{u}},\hfill \end{array}$$

(26)

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s-}^{\alpha }x\left(\tau \right)& ={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_{\tau }^{\infty }\left[x\left(u\right)-\sum _{n=0}^{N-1}{\displaystyle \frac{{\mathfrak{D}}_{s-}^{n}x\left(\tau \right)}{n!}}{ln}^n(u/\tau )\right]{ln}^{-\alpha -1}(u/\tau ){\displaystyle \frac{\mathrm{d}u}{u}}.\hfill \end{array}$$

(27)

From here, Hadamard derivatives of variable order can be obtained as follows.

As $X\left(v\right)={\int }_0^{\infty }x\left(u\right)u^{-v-1}\mathrm{d}u$ and the inversion integral is uniformly convergent in the ROC, we can permute the integrations in (20):

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}x\left(\tau \right)& =\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{v}^{\alpha \left(\tau \right)}{\int }_0^{\infty }x\left(u\right)u^{-v-1}\mathrm{d}u{\tau }^v\mathrm{d}v\hfill \\ \hfill & ={\int }_0^{\infty }x\left(u\right)\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{v}^{\alpha \left(\tau \right)}{u}^{-v-1}{\tau }^v\mathrm{d}v\mathrm{d}u.\hfill \end{array}$$

(28)

Let us now assume that $\alpha \left(\tau \right)<0$. The results stated above in (21) allow us to write

$${\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}x\left(\tau \right)={\displaystyle \frac{1}{\Gamma (-\alpha (\tau \left)\right)}}{\int }_0^{\tau }x\left(u\right){ln}^{-\alpha \left(\tau \right)-1}(\tau /u){\displaystyle \frac{\mathrm{d}u}{u}}.$$

(29)

Let $\alpha \left(\tau \right)\le N\left(\tau \right)$. It is a simple matter to generalise (26) to obtain a VO Hadamard derivative:

$${\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}x\left(\tau \right)={\displaystyle \frac{1}{\Gamma (-\alpha (\tau \left)\right)}}{\int }_0^{\tau }\left[x\left(u\right)-{\sum }_{n=0}^{N\left(\tau \right)-1}{(-1)}^n{\displaystyle \frac{{\mathfrak{D}}_{s+}^{n}x\left(\tau \right)}{n!}}{ln}^n(\tau /u)\right]{ln}^{-\alpha \left(\tau \right)-1}(\tau /u){\displaystyle \frac{\mathrm{d}u}{u}}.$$

(30)

Since the summation is null if $N\left(t\right)\le 0$, in this expression, $\alpha \left(t\right)$ can be any real number. Notice that, if the variation of the order is bounded so that $N-1<\alpha \left(\tau \right)\le N,\forall \tau$, then the number of terms in the summation N is constant. As to (27), the corresponding VO Hadamard derivative is:

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s-}^{\alpha \left(\tau \right)}x\left(\tau \right)& ={\displaystyle \frac{1}{\Gamma (-\alpha (\tau \left)\right)}}{\int }_{\tau }^{\infty }\left[x\left(u\right)-\sum _{n=0}^{N\left(\tau \right)-1}{\displaystyle \frac{{\mathfrak{D}}_{s-}^{n}x\left(\tau \right)}{n!}}{ln}^n(u/\tau )\right]{ln}^{-\alpha \left(\tau \right)-1}(u/\tau ){\displaystyle \frac{\mathrm{d}u}{u}}.\hfill \end{array}$$

(31)

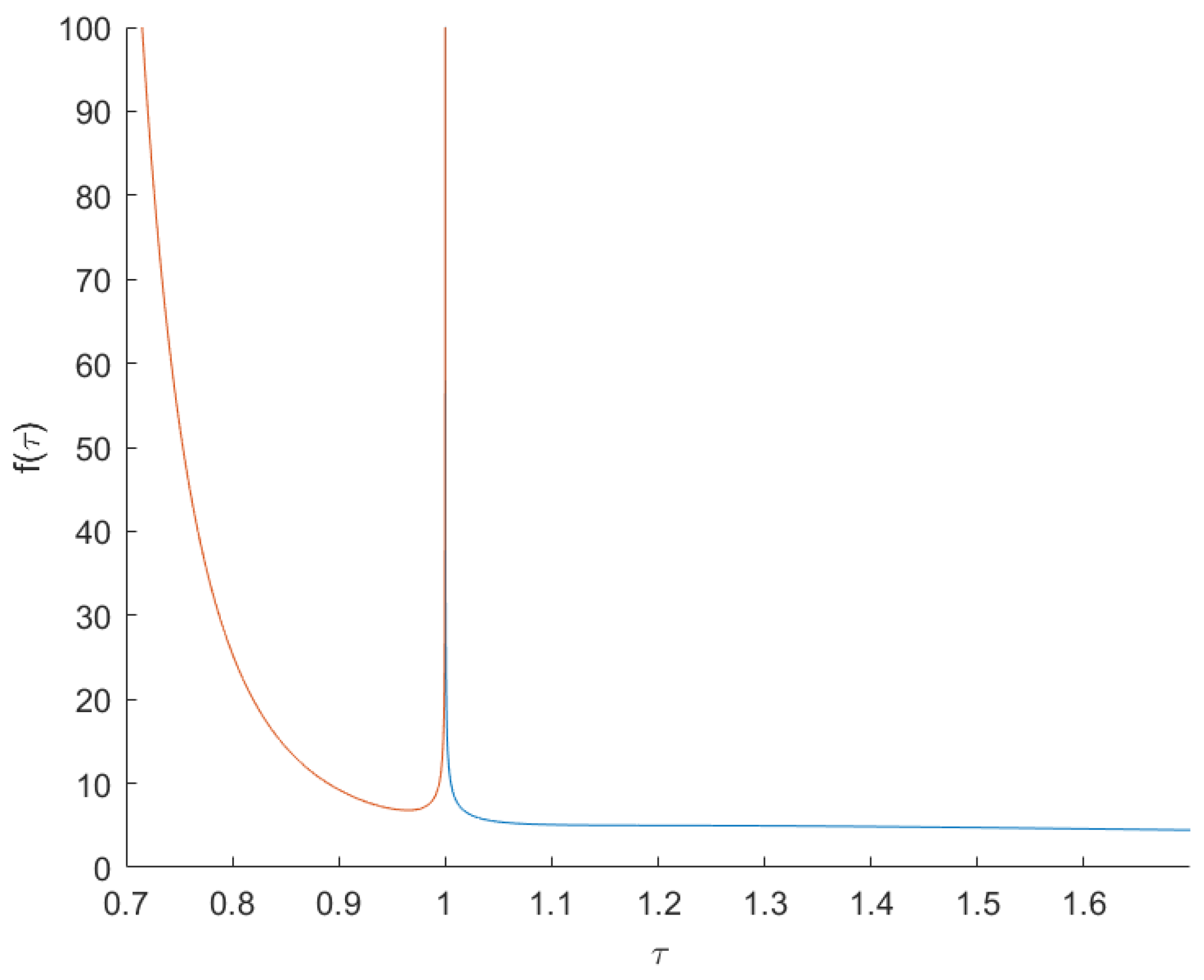
3.3. Derivative Properties

It would be desirable for the properties of variable-order derivatives to reproduce those required in [56]. This topic was studied in [44], where it was shown that most of the properties do not hold when the order becomes variable. Let us see what happens, starting by considering linearity. We will address the stretching case; the same result can be found for shrinking derivatives exactly in the same way.

Let $x\left(\tau \right)=x_1\left(\tau \right)+x_2\left(\tau \right)$ in (17). Then:

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}\left[x_1\left(\tau \right)+x_2\left(\tau \right)\right]& =\underset{q\to 1^{+}}{lim}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_n}{n!}\left[x_1\left(\tau q^{-n}\right)+x_2\left(\tau q^{-n}\right)\right]\hfill \\ \hfill & =\underset{q\to 1^{+}}{lim}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_n}{n!}{x}_1\left(\tau q^{-n}\right)+\hfill \\ & \underset{q\to 1^{+}}{lim}{ln}^{-\alpha \left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-\alpha \left(\tau \right))}_n}{n!}{x}_2\left(\tau q^{-n}\right)={\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}{x}_1\left(\tau \right)+{\mathfrak{D}}_{s+}^{\alpha \left(\tau \right)}{x}_2\left(\tau \right).\hfill \end{array}$$

(32)

Thus, this property does not create any difficulty. The next property is the very important “additivity and commutativity of the orders”. Let $\alpha \left(\tau \right)={\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)$ in (17). It is easy to see that there is no linearity on the orders:

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)}x\left(\tau \right)& =\underset{q\to 1^{+}}{lim}{ln}^{-{\alpha }_1\left(\tau \right)-{\alpha }_2\left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-{\alpha }_1\left(\tau \right)-{\alpha }_2\left(\tau \right))}_n}{n!}x\left(\tau q^{-n}\right)\hfill \\ \hfill & \ne \underset{q\to 1^{+}}{lim}{ln}^{-{\alpha }_1\left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-{\alpha }_1\left(\tau \right))}_n}{n!}x\left(\tau q^{-n}\right)+\hfill \\ & \underset{q\to 1^{+}}{lim}{ln}^{-{\alpha }_2\left(\tau \right)}\left(q\right)\sum _{n=0}^{\infty }\frac{{(-{\alpha }_2\left(\tau \right))}_n}{n!}x\left(\tau q^{-n}\right)={\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)}x\left(\tau \right)+{\mathfrak{D}}_{s+}^{{\alpha }_2\left(\tau \right)}x\left(\tau \right).\hfill \end{array}$$

(33)

This result points to the fact that the property does not hold. Let us study the situation by considering relation (20) again:

$${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}x\left(\tau \right)=\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{v}^{\alpha \left(\tau \right)}X\left(v\right){\tau }^v\mathrm{d}v,\tau \in {\mathbb{R}}^{+}.$$

We may observe that:

• This integral is not an inverse MT;
• This integral shows that ${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}x\left(\tau \right)$ can be considered a synthesis of elemental powers $v^{\alpha \left(\tau \right)}X\left(v\right){\tau }^v\mathrm{d}v$, which provides it sense and meaning;
• The expression $v^{\alpha \left(\tau \right)}X\left(v\right)$ is not an MT. In fact, its transform is given by:

  $${\overline{X}}_{\alpha }\left(u\right)=\mathcal{M}\left[{\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}x\left(\tau \right)\right]\left(u\right)=\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}\mathcal{M}\left[v^{\alpha \left(\tau \right)}{\tau }^v\right]X\left(v\right)\mathrm{d}v,\tau \in {\mathbb{R}}^{+}.$$

Therefore, while

$${\mathfrak{D}}_{s\pm }^{{\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)}x\left(\tau \right)=\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{v}^{{\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)}X\left(v\right){\tau }^v\mathrm{d}v,$$

we have

$${\mathfrak{D}}_{s\pm }^{{\alpha }_2\left(\tau \right)}{\mathfrak{D}}_{s\pm }^{{\alpha }_1\left(\tau \right)}x\left(\tau \right)=\frac{1}{2\pi i}\underset{\sigma -i\infty }{\overset{\sigma +i\infty }{\int }}{u}^{{\alpha }_2\left(\tau \right)}{\overline{X}}_{{\alpha }_1}\left(u\right){\tau }^u\mathrm{d}u,$$

which are therefore different expressions, since ${\overline{X}}_{{\alpha }_1}\left(u\right)\ne u^{{\alpha }_1\left(\tau \right)}X\left(u\right)$, unless ${\alpha }_1\left(\tau \right)$ is constant. We conclude that:

• In general,

  $$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{{\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)}x\left(\tau \right)\ne {\mathfrak{D}}_{s\pm }^{{\alpha }_2\left(\tau \right)}{\mathfrak{D}}_{s\pm }^{{\alpha }_1\left(\tau \right)}x\left(\tau \right);\end{array}$$

  (34)
• There is no recursivity

  $$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{n\alpha \left(\tau \right)}x\left(\tau \right)\ne {\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}{\mathfrak{D}}_{s\pm }^{(n-1)\alpha \left(\tau \right)}x\left(\tau \right);\end{array}$$

  (35)
• If ${\alpha }_1\left(\tau \right)$ is a real constant ${\alpha }_1\left(\tau \right)={\alpha }_0$, then ${\overline{X}}_{{\alpha }_0}\left(u\right)=u^{{\alpha }_0}X\left(u\right)$ and

  $$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{{\alpha }_0+{\alpha }_2\left(\tau \right)}x\left(\tau \right)={\mathfrak{D}}_{s\pm }^{{\alpha }_2\left(\tau \right)}{\mathfrak{D}}_{s\pm }^{{\alpha }_0}x\left(\tau \right);\end{array}$$

  (36)
• However,

  $$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{{\alpha }_0}{\mathfrak{D}}_{s\pm }^{{\alpha }_2\left(\tau \right)}x\left(\tau \right)\ne {\mathfrak{D}}_{s\pm }^{{\alpha }_2\left(\tau \right)}{\mathfrak{D}}_{s\pm }^{{\alpha }_0}x\left(\tau \right),\end{array}$$

  (37)

  since, after the first derivation, when carrying out the second, we are including ${\alpha }_2\left(\tau \right)$ in the computation;
• As it becomes clear, the anti-derivative of ${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}$ is not ${\mathfrak{D}}_{s\pm }^{-\alpha \left(\tau \right)}.$

Despite these results, and as strange as it may seem, Leibniz’s rule is valid. Let us show that it holds:

$${\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}\left[x\left(\tau \right)y\left(\tau \right)\right]=\sum _{k=0}^{\infty }\left(\genfrac{}{}{0pt}{}{\alpha \left(\tau \right)}{k}\right){\mathfrak{D}}_{s\pm }^{k}x\left(\tau \right){\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)-k}y\left(\tau \right).$$

(38)

To prove this relation, we first note that

$$\begin{array}{c}\hfill \mathcal{M}\left[x\left(\tau \right)y\left(\tau \right)\right]={\displaystyle \frac{1}{2\pi i}}X\left(v\right)\ast Y\left(v\right),\end{array}$$

(39)

where ∗ represents the usual shift-invariant convolution. Using the Bromwich inverse Mellin transform, we can write

$$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}\left[x\left(\tau \right)y\left(\tau \right)\right]={\displaystyle \frac{1}{{\left(2\pi i\right)}^2}}{\int }_{{\gamma }_1}{v}^{\alpha \left(\tau \right)}{\int }_{{\gamma }_2}X\left(u\right)Y(v-u)\mathrm{d}u{\tau }^v\mathrm{d}v,\end{array}$$

(40)

where ${\gamma }_1$ and ${\gamma }_2$ are vertical straight lines in the intersection of the region of convergence of both transforms. We now use equality

$$\begin{array}{cc}\hfill v^{\alpha \left(\tau \right)}& ={\left(v-u+\frac{v-u}{v-u}u\right)}^{\alpha \left(\tau \right)}={(v-u)}^{\alpha \left(\tau \right)}{\left[1+{\displaystyle \frac{u}{v-u}}\right]}^{\alpha \left(\tau \right)}\hfill \\ \hfill & =\sum _{k=0}^{\infty }\left(\genfrac{}{}{0pt}{}{\alpha \left(\tau \right)}{k}\right)k^k{(v-u)}^{\alpha \left(\tau \right)-k},\hfill \end{array}$$

(41)

and evident manipulations so as to obtain

$$\begin{array}{c}\hfill {\mathfrak{D}}_{s\pm }^{\alpha \left(\tau \right)}\left[x\left(\tau \right)y\left(\tau \right)\right]=\sum _{k=0}^{\infty }\left(\genfrac{}{}{0pt}{}{\alpha \left(\tau \right)}{k}\right){\displaystyle \frac{1}{{\left(2\pi i\right)}^2}}{\int }_{{\gamma }_1}{\int }_{{\gamma }_2}{u}^{k}X\left(u\right){(v-u)}^{\alpha \left(\tau \right)-k}Y(v-u)\mathrm{d}u{\tau }^v\mathrm{d}v,\end{array}$$

(42)

from where property (38) results.

Examples. Let

$$\begin{array}{cc}\hfill x\left(\tau \right)& ={\tau }^2,\hfill \end{array}$$

(43)

$$\begin{array}{cc}\hfill {\alpha }_1\left(\tau \right)& =0.1\tau ,\hfill \end{array}$$

(44)

$$\begin{array}{cc}\hfill {\alpha }_2\left(\tau \right)& =0.5.\hfill \end{array}$$

(45)

Applying (19), we obtain

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)}x\left(\tau \right)& ={\mathfrak{D}}_{s+}^{0.1\tau }{\tau }^2=2^{0.1\tau }{\tau }^2,\hfill \end{array}$$

(46)

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_2\left(\tau \right)}x\left(\tau \right)& ={\mathfrak{D}}_{s+}^{0.5}{\tau }^2=2^{0.5}{\tau }^2.\hfill \end{array}$$

(47)

As to the linearity of the orders (33),

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)+{\alpha }_2\left(\tau \right)}x\left(\tau \right)& ={\mathfrak{D}}_{s+}^{0.1\tau +0.5}{\tau }^2=2^{0.1\tau +0.5}{\tau }^2,\hfill \end{array}$$

(48)

which differs from

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)}x\left(\tau \right)+{\mathfrak{D}}_{s+}^{{\alpha }_2\left(\tau \right)}x\left(\tau \right)& =\left(2^{0.1\tau }+2^{0.5}\right){\tau }^2,\hfill \end{array}$$

(49)

but, as we could expect from (36), is equal to

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)}{\mathfrak{D}}_{s+}^{{\alpha }_2\left(\tau \right)}x\left(\tau \right)& ={\mathfrak{D}}_{s+}^{0.1\tau }{\mathfrak{D}}_{s+}^{0.5}{\tau }^2={\mathfrak{D}}_{s+}^{0.1\tau }{2}^{0.5}{\tau }^2\hfill \\ \hfill & =2^{0.5}{\mathfrak{D}}_{s+}^{0.1\tau +0.5}{\tau }^2=2^{0.5}{2}^{0.1\tau }{\tau }^2,\hfill \end{array}$$

(50)

which in turn is not, as seen in (37), the same as ${\mathfrak{D}}_{s+}^{{\alpha }_2\left(\tau \right)}{\mathfrak{D}}_{s+}^{{\alpha }_1\left(\tau \right)}x\left(\tau \right)$, that would have to be found using (38):

$$\begin{array}{cc}\hfill {\mathfrak{D}}_{s+}^{0.5}{\mathfrak{D}}_{s+}^{0.1\tau }{\tau }^2& ={\mathfrak{D}}_{s+}^{0.5}{2}^{0.1\tau }{\tau }^2=\sum _{k=0}^{\infty }\left(\genfrac{}{}{0pt}{}{0.5}{k}\right){\mathfrak{D}}_{s\pm }^k{2}^{0.1\tau }{\mathfrak{D}}_{s\pm }^{0.5-k}{\tau }^2.\hfill \end{array}$$

(51)

4. VO Scale-Invariant Systems

In agreement with the concepts introduced above, we define VO systems as follows.

Definition 9. 

A dilation scale-invariant variable-order autoregressive–moving-average (DI-VARMA) system is given by

$$\sum _{k=0}^{N_0}{a}_k{\mathfrak{D}}_{s\pm }^{{\alpha }_k\left(\tau \right)}y\left(\tau \right)=\sum _{k=0}^{M_0}{b}_k{\mathfrak{D}}_{s\pm }^{{\beta }_k\left(\tau \right)}x\left(\tau \right),\tau \in {\mathbb{R}}^{+}$$

(52)

where ${\mathfrak{D}}_{s\pm }^{{\alpha }_k\left(\tau \right)},{\mathfrak{D}}_{s\pm }^{{\beta }_k\left(\tau \right)},k=0,1,2,\dots$ are fractional ${\alpha }_k$-order and ${\beta }_k$-order scale derivatives, and constants $N_0$ and $M_0$ are the system orders. The parameters, $a_k,b_k,k=0,1,\cdots$, are constant real numbers. Without loosing generality, we set $a_{N_0}=1$.

Notice that, should the variable order (or orders) become fixed, we retrieve the results for scale-invariant systems (with constant orders) in [26]. The results in [44,57] can be easily adapted here. The name “autoregressive–moving-average” was borrowed from a current nomenclature used for shift-invariant systems [58]. We assume that variations in orders ${\alpha }_k\left(\tau \right)$ and ${\beta }_k\left(\tau \right)$ are significantly slower than the dynamic of the system. Therefore, as the power ${\tau }^v$ is the eigenfunction of (52), we easily obtain a VO transfer function [59]. Let us consider the commensurate case:

Definition 10. 

A DI-VARMA system (52) with orders that can be arranged so as to verify ${\alpha }_k\left(\tau \right)=k\alpha \left(\tau \right)$, $k=0,\dots ,M_0$ and ${\beta }_k\left(\tau \right)=k\alpha \left(\tau \right),k=0,\dots ,N_0$, for all values of $\tau \in {\mathbb{R}}^{+}$, is called commensurate, and $\alpha \left(\tau \right)$ is the variable commensurability order. Once more, system orders $M_0,N_0$ and parameters $a_k,b_k$ are assumed constant.

The transfer function corresponding to a commensurate case in (52) is

$$G\left(v\right)=\frac{{\displaystyle \sum _{k=0}^{M_0}{b}_k{v}^{k\alpha \left(\tau \right)}}}{{\displaystyle \sum _{k=0}^{N_0}{a}_k{v}^{k\alpha \left(\tau \right)}}}.$$

(53)

For simplicity and stability, we assume that $M_0<N_0$, and that $\forall \tau$ all the roots $p_k,k=1,2,\cdots ,$ of ${\displaystyle \sum _{k=0}^{N_0}}{a}_k{w}^k$ are simple. This allows us to write

$$G\left(v\right)=\sum _{k=1}^N\frac{A_k}{v^{\alpha \left(\tau \right)}-p_k},$$

(54)

where the $A_k$ are the residues obtained by substituting w for $v^{\alpha \left(\tau \right)}$ in (53). Notice that the residues are constant, inasmuch parameters $a_k,b_k$ are constant too. The impulse response results from the inversion of a combination of partial fractions such as

$$F\left(v\right)=\frac{1}{v^{\alpha \left(\tau \right)}-p}.$$

(55)

Assuming now that $\Re \left(v\right)>0$, we can use the series expansion [60]:

$$\frac{1}{v^{\alpha \left(\tau \right)}-p}=\sum _{n=1}^{\infty }{p}^{n-1}{v}^{-n\alpha \left(\tau \right)}\Re \left(v\right)>\left|p\right|.$$

(56)

The corresponding inverse MT can be found by replacing (21) in (56) [30,61]:

$$\begin{array}{cc}\hfill f\left(\tau \right)& =\sum _{n=1}^{\infty }{p}^{n-1}\frac{{\left(ln\left(\tau \right)\right)}^{n\alpha \left(t\right)-1}}{\Gamma \left(n\alpha \right(\tau \left)\right)}\epsilon (\tau -1)\hfill \\ \hfill & =\sum _{n=0}^{\infty }{p}^n\frac{{\left(ln\left(\tau \right)\right)}^{(n+1)\alpha \left(t\right)-1}}{\Gamma \left(\right(n+1\left)\alpha \right(\tau \left)\right)}\epsilon (\tau -1)\hfill \\ \hfill & ={ln}^{\alpha \left(\tau \right)-1}\left(\tau \right)\sum _{n=0}^{\infty }\frac{{\left(p{ln}^{\alpha \left(\tau \right)}\left(\tau \right)\right)}^n}{\Gamma \left(n\alpha \right(\tau )+\alpha (\tau \left)\right)},\tau \ge 1.\hfill \end{array}$$

(57)

Notice that the Mittag–Leffler function of a variable-order power $\alpha \left(\tau \right)$ of the logarithm is given by

$$E_{\alpha \left(\tau \right),\beta }\left({ln}^{\alpha \left(\tau \right)}\left(\tau \right)\right)=\sum _{n=0}^{\infty }\frac{{ln}^{n\alpha \left(\tau \right)}\left(\tau \right)}{\Gamma \left(n\alpha \right(\tau )+\beta )},\tau \ge 1,$$

(58)

and, consequently, we can rewrite (57) as:

$$f\left(\tau \right)={ln}^{\alpha \left(\tau \right)-1}\left(\tau \right)E_{\alpha \left(\tau \right),\alpha \left(\tau \right)}\left(p{ln}^{\alpha \left(\tau \right)}\left(\tau \right)\right),\tau \ge 1.$$

(59)

The solution corresponding to $\Re \left(v\right)<0$ is obtained using a similar procedure, accounting for (22):

$$\begin{array}{cc}\hfill f\left(\tau \right)& =\sum _{n=1}^{\infty }{p}^{n-1}\frac{{\left(ln\left(\frac{1}{\tau }\right)\right)}^{n\alpha \left(t\right)-1}}{\Gamma \left(n\alpha \right(\tau \left)\right)}\epsilon (1-\tau )\hfill \end{array}$$

$$\begin{array}{cc}\hfill & ={ln}^{\alpha \left(\tau \right)-1}\left(\frac{1}{\tau }\right)\sum _{n=0}^{\infty }\frac{{\left(p{ln}^{\alpha \left(\tau \right)}\left(\frac{1}{\tau }\right)\right)}^n}{\Gamma \left(n\alpha \right(\tau )+\alpha (\tau \left)\right)}\epsilon (1-\tau )\hfill \end{array}$$

(60)

$$\begin{array}{cc}\hfill & ={ln}^{\alpha \left(\tau \right)-1}\left(\frac{1}{\tau }\right)E_{\alpha \left(\tau \right),\alpha \left(\tau \right)}\left(p{ln}^{\alpha \left(\tau \right)}\left(\frac{1}{\tau }\right)\right),\tau \le 1.\hfill \end{array}$$

(61)

Remark 2. 

The poles with multiplicity greater than 1 can be treated through ordinary derivative computation relative to p.

Examples. Let

$$\begin{array}{c}\hfill F\left(v\right)=\frac{1}{v^{0.5\tau }-2}.\end{array}$$

(62)

Its stretching and shrinking impulse responses

$$\begin{array}{cc}\hfill f\left(\tau \right)& ={ln}^{0.5\tau -1}\left(\tau \right)E_{0.5\tau ,0.5\tau }\left(2{ln}^{0.5\tau }\left(\tau \right)\right)\hfill \end{array}$$

(63)

$$\begin{array}{cc}\hfill f\left(\tau \right)& ={ln}^{0.5\tau -1}\left(\frac{1}{\tau }\right)E_{0.5\tau ,0.5\tau }\left(2{ln}^{0.5\tau }\left(\frac{1}{\tau }\right)\right)\hfill \end{array}$$

(64)

are shown in Figure 1.

5. Conclusions

In this paper, we introduced and studied a special class of fractional derivatives: the variable order, stretching and shrinking, and scale derivatives. We proposed a GL-type formulation based on incremental ratio limits. Alternatively, we used Hadamard integral representations. We deduced and discussed a few of their properties. Finally, fractional variable-order systems of autoregressive–moving-average type were introduced and exemplified. For them, we presented the transfer function and showed how to obtain the corresponding impulse response.