Insight into Functional Boiti–Leon–Mana–Pempinelli Equation and Error Control: Approximate Similarity Solutions
Abstract
:1. Introduction
2. Preliminaries
2.1. Model Equation
2.1.1. Logarithmic BLMPE
2.1.2. Exponential BLMPE
2.1.3. Monomaniacal BLMPE
2.2. The Extended Unified Method
2.2.1. Polynomial Solutions
2.2.2. Rational Solutions
3. Logarithmic BLMPE
3.1. Polynomial Solutions
3.2. Rational Solutions
4. Exponential BLMPE
4.1. Polynomial Solutions
4.2. Rational Solutions
5. Monomaniacal BLMPE
5.1. Polynomial Solutions
5.2. Rational Solution
6. Discussion and Conclusions
- A functional Boiti–Leon–Mana–Pempinelli equation is considered in this work.
- Three particular cases (which are the logarithmic, exponential and monomaniacal versions) are investigated herein.
- Approximate similarity solutions in the three previous cases are obtained by using the extended unified method, which is presented here. According to this method, the residue terms are considered as errors in the solution.
- Here, in the case of self-similar solutions, the errors are time dependent.
- The maximum error is estimated and it is found that the presented method leads to a good accuracy, due to the possibility of controlling the maximum error.
- Thus, this method is efficient when studying evolution equations with time dependent coefficients.
- A class of similarity solutions for the three cases are established. It is found that the method used here gives rise to a high accuracy due to the parameters control.
- Furthermore, the extended unified method is of lower time cost in symbolic computations when compared, for instance, against Lie symmetries. Indeed, the later approach requires a hierarchy of long steps.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Animasaun, I.L.; Shah, N.A.; Wakif, A.; Mahanthesh, B.; Sivaraj, R.; Koríko, O.K. Ratio of Momentum Diffusivity to Thermal Diffusivity: Introduction, Meta-Analysis, and Scrutinization; Chapman and Hall/CRC Press: New York, NY, USA, 2022. [Google Scholar]
- Ma, H.; Bai, Y.; Deng, A. Exact three-wave solutions for the (3 + 1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Adv. Differ. Equ. 2013, 2013, 321. [Google Scholar] [CrossRef]
- Yuan, N. Rich analytical solutions of a new (3 + 1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Results Phys. 2021, 22, 103927. [Google Scholar] [CrossRef]
- Li, B.Q.; Ma, Y.L. Multiple-lump waves for a (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation arising from incompressible fluid. Comput. Math. Appl. 2018, 76, 204–214. [Google Scholar] [CrossRef]
- Darvishi, M.; Najafi, M.; Kavitha, L.; Venkatesh, M. Stair and step soliton solutions of the integrable (2 + 1) and (3 + 1)-dimensional Boiti—Leon—Manna—Pempinelli equations. Commun. Theor. Phys. 2012, 58, 785. [Google Scholar] [CrossRef]
- Osman, M.; Wazwaz, A.M. A general bilinear form to generate different wave structures of solitons for a (3 + 1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Math. Methods Appl. Sci. 2019, 42, 6277–6283. [Google Scholar] [CrossRef]
- Cui, C.J.; Tang, X.Y.; Cui, Y.J. New variable separation solutions and wave interactions for the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Appl. Math. Lett. 2020, 102, 106109. [Google Scholar] [CrossRef]
- Mabrouk, S.; Rashed, A. Analysis of (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation via Lax pair investigation and group transformation method. Comput. Math. Appl. 2017, 74, 2546–2556. [Google Scholar] [CrossRef]
- Liu, J.G.; Tian, Y.; Hu, J.G. New non-traveling wave solutions for the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Appl. Math. Lett. 2018, 79, 162–168. [Google Scholar] [CrossRef]
- Liu, J.G.; Du, J.Q.; Zeng, Z.F.; Nie, B. New three-wave solutions for the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Nonlinear Dyn. 2017, 88, 655–661. [Google Scholar] [CrossRef]
- Xu, G.q. Painlevé analysis, lump-kink solutions and localized excitation solutions for the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Appl. Math. Lett. 2019, 97, 81–87. [Google Scholar] [CrossRef]
- Peng, W.Q.; Tian, S.F.; Zhang, T.T. Breather waves and rational solutions in the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Comput. Math. Appl. 2019, 77, 715–723. [Google Scholar] [CrossRef]
- Yel, G.; Aktürk, T. A New Approach to (3 + 1) Dimensional Boiti–Leon–Manna–Pempinelli Equation. Appl. Math. Nonlinear Sci. 2020, 5, 309–316. [Google Scholar] [CrossRef]
- Samir, I.; Badra, N.; Ahmed, H.M.; Arnous, A.H. Solitary Wave Solutions for Generalized Boiti–Leon–Manna–Pempinelli Equation by Using Improved Simple Equation Method. Int. J. Appl. Comput. Math. 2022, 8, 102. [Google Scholar] [CrossRef]
- Zhou, A.J.; Guo, Y.R. Exact solutions of the (3 + 1)-dimensional generalized Boiti–Leon–Manna–Pempinelli equation. Mod. Phys. Lett. B 2022, 36, 2150578. [Google Scholar] [CrossRef]
- Ali, K.K.; Mehanna, M. On some new soliton solutions of (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation using two different methods. Arab. J. Basic Appl. Sci. 2021, 28, 234–243. [Google Scholar] [CrossRef]
- Tariq, K.U.; Bekir, A.; Zubair, M. On some new travelling wave structures to the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli model. J. Ocean. Eng. Sci. 2022, in press. [Google Scholar] [CrossRef]
- Wu, J.; Liu, Y.; Piao, L.; Zhuang, J.; Wang, D.S. Nonlinear localized waves resonance and interaction solutions of the (3 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Nonlinear Dyn. 2020, 100, 1527–1541. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Painlevé analysis for Boiti–Leon–Manna–Pempinelli equation of higher dimensions with time-dependent coefficients: Multiple soliton solutions. Phys. Lett. A 2020, 384, 126310. [Google Scholar] [CrossRef]
- Hosseini, K.; Ma, W.X.; Ansari, R.; Mirzazadeh, M.; Pouyanmehr, R.; Samadani, F. Evolutionary behavior of rational wave solutions to the (4 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Phys. Scr. 2020, 95, 065208. [Google Scholar] [CrossRef]
- Xu, G.Q.; Wazwaz, A.M. Integrability aspects and localized wave solutions for a new (4 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Nonlinear Dyn. 2019, 98, 1379–1390. [Google Scholar] [CrossRef]
- Li, Y.; Li, D. New exact solutions for the (2 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Appl. Math. Sci 2012, 6, 579–587. [Google Scholar]
- Seadawy, A.R.; Ali, A.; Helal, M.A. Analytical wave solutions of the (2 + 1)-dimensional Boiti–Leon–Pempinelli and Boiti–Leon–Manna–Pempinelli equations by mathematical methods. Math. Methods Appl. Sci. 2021, 44, 14292–14315. [Google Scholar] [CrossRef]
- Sun, L.; Qi, J.; An, H. Novel localized wave solutions of the (2 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation. Commun. Theor. Phys. 2020, 72, 125009. [Google Scholar] [CrossRef]
- Asadi, N.; Nadjafikhah, M. Geometry of Boiti-Leon-Manna-Pempinelli Equation. Indian J. Sci. Technol. 2015, 8, 33. [Google Scholar] [CrossRef]
- Hu, L.; Gao, Y.T.; Jia, S.L.; Su, J.J.; Deng, G.F. Solitons for the (2 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation for an irrotational incompressible fluid via the Pfaffian technique. Mod. Phys. Lett. B 2019, 33, 1950376. [Google Scholar] [CrossRef]
- Hu, L.; Gao, Y.T.; Jia, T.T.; Deng, G.F.; Li, L.Q. Higher-order hybrid waves for the (2 + 1)-dimensional Boiti–Leon–Manna–Pempinelli equation for an irrotational incompressible fluid via the modified Pfaffian technique. Z. für Angew. Math. und Phys. 2021, 72, 75. [Google Scholar] [CrossRef]
- Luo, L. New exact solutions and Bäcklund transformation for Boiti–Leon–Manna–Pempinelli equation. Phys. Lett. A 2011, 375, 1059–1063. [Google Scholar] [CrossRef]
- Bai, C.J.; Zhao, H. New solitary wave and Jacobi periodic wave excitations in (2 + 1)-dimensional Boiti–Leon–Manna–Pempinelli system. Int. J. Mod. Phys. B 2008, 22, 2407–2420. [Google Scholar] [CrossRef]
- Fu, Z.H.; Liu, J.G. Exact periodic cross-kink wave solutions for the (2 + 1)-dimensional Boiti-Leon-Manna-Pempinelli equation. Indian J. Pure Appl. Phys. (IJPAP) 2017, 55, 163–167. [Google Scholar]
- Shen, Y.; Tian, B.; Zhang, C.R.; Tian, H.Y.; Liu, S.H. Breather-wave, periodic-wave and traveling-wave solutions for a (2 + 1)-dimensional extended Boiti–Leon–Manna–Pempinelli equation for an incompressible fluid. Mod. Phys. Lett. B 2021, 35, 2150261. [Google Scholar] [CrossRef]
- Mu, L. A pressure-robust weak Galerkin finite element method for Navier–Stokes equations. Numer. Methods Partial. Differ. Equ. 2023, 39, 2327–2354. [Google Scholar] [CrossRef]
- Bayrak, M.A.; Demir, A.; Ozbilge, E. A novel approach for the solution of fractional diffusion problems with conformable derivative. Numer. Methods Partial. Differ. Equ. 2023, 39, 1870–1887. [Google Scholar] [CrossRef]
- Li, Z.; Xiao, L.; Li, M.; Chen, H. Error estimates for the finite element method of the chemotaxis-Navier–Stokes equations. J. Appl. Math. Comput. 2023, 69, 3039–3065. [Google Scholar] [CrossRef]
- Medina-Ramírez, I.; Puri, A. Numerical treatment of the spherically symmetric solutions of a generalized Fisher–Kolmogorov–Petrovsky–Piscounov equation. J. Comput. Appl. Math. 2009, 231, 851–868. [Google Scholar]
- Xiao, M.; Wang, Z.; Mo, Y. An implicit nonlinear difference scheme for two-dimensional time-fractional Burgers’ equation with time delay. J. Appl. Math. Comput. 2023, 69, 2919–2934. [Google Scholar] [CrossRef]
- Alderremy, A.; Abdel-Gawad, H.; Saad, K.M.; Aly, S. New exact solutions of time conformable fractional Klein Kramer equation. Opt. Quantum Electron. 2021, 53, 693. [Google Scholar] [CrossRef]
- Yang, X.; Wu, L.; Zhang, H. A space-time spectral order sinc-collocation method for the fourth-order nonlocal heat model arising in viscoelasticity. Appl. Math. Comput. 2023, 457, 128192. [Google Scholar] [CrossRef]
- Yang, X.; Zhang, Q.; Yuan, G.; Sheng, Z. On positivity preservation in nonlinear finite volume method for multi-term fractional subdiffusion equation on polygonal meshes. Nonlinear Dyn. 2018, 92, 595–612. [Google Scholar] [CrossRef]
- Gu, X.M.; Sun, H.W.; Zhang, Y.; Zhao, Y.L. Fast implicit difference schemes for time-space fractional diffusion equations with the integral fractional Laplacian. Math. Methods Appl. Sci. 2021, 44, 441–463. [Google Scholar] [CrossRef]
- Abdel-Gawad, H.I.; Elazab, N.S.; Osman, M. Exact solutions of space dependent Korteweg–de Vries equation by the extended unified method. J. Phys. Soc. Jpn. 2013, 82, 044004. [Google Scholar] [CrossRef]
- Abdel-Gawad, H.; Tantawy, M.; Abdelwahab, A.M. A new technique for solving Burgers-Kadomtsev-Petviashvili equation with an external source. Suppression of wave breaking and shock wave. Alex. Eng. J. 2022, 69, 167–176. [Google Scholar] [CrossRef]


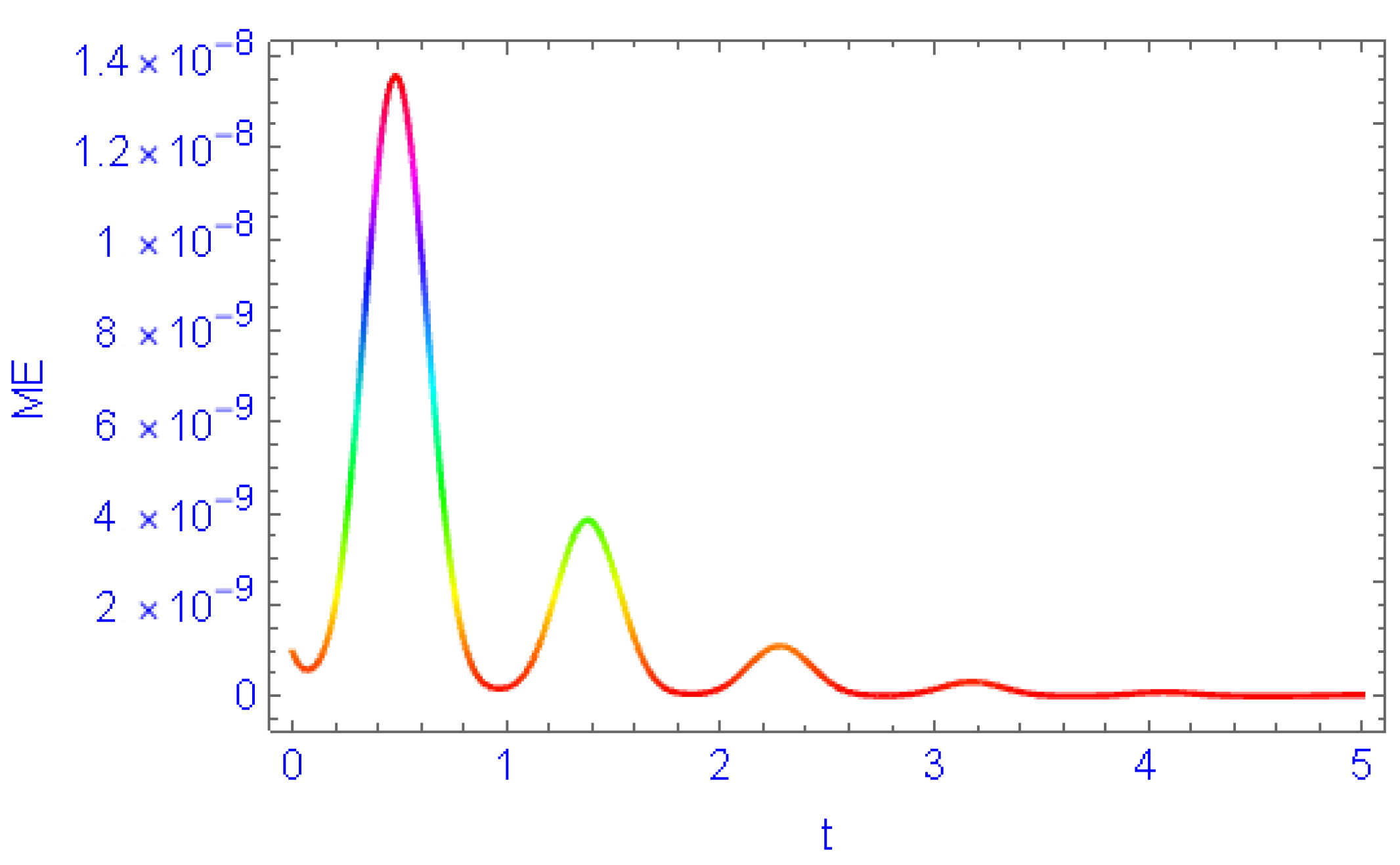
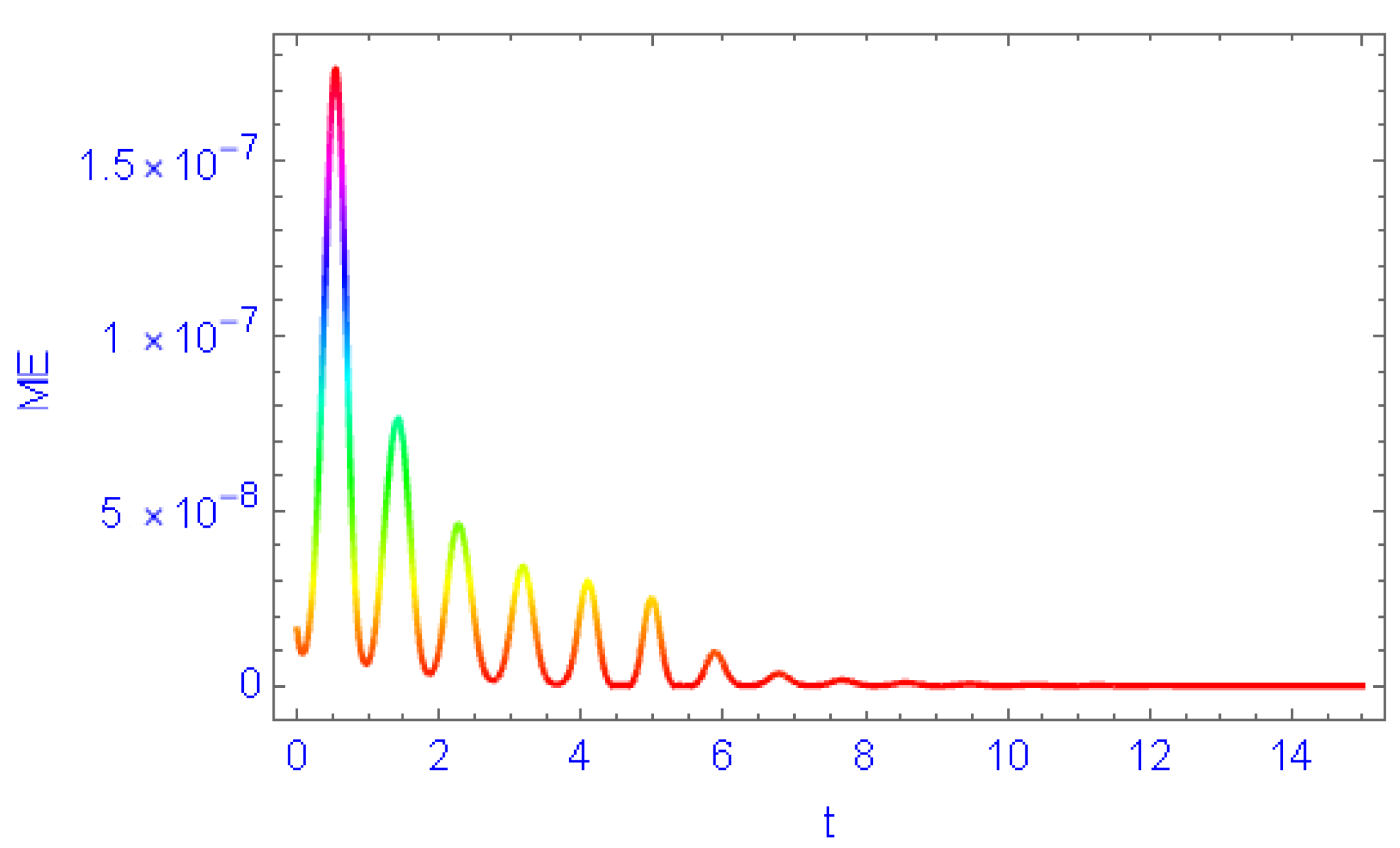

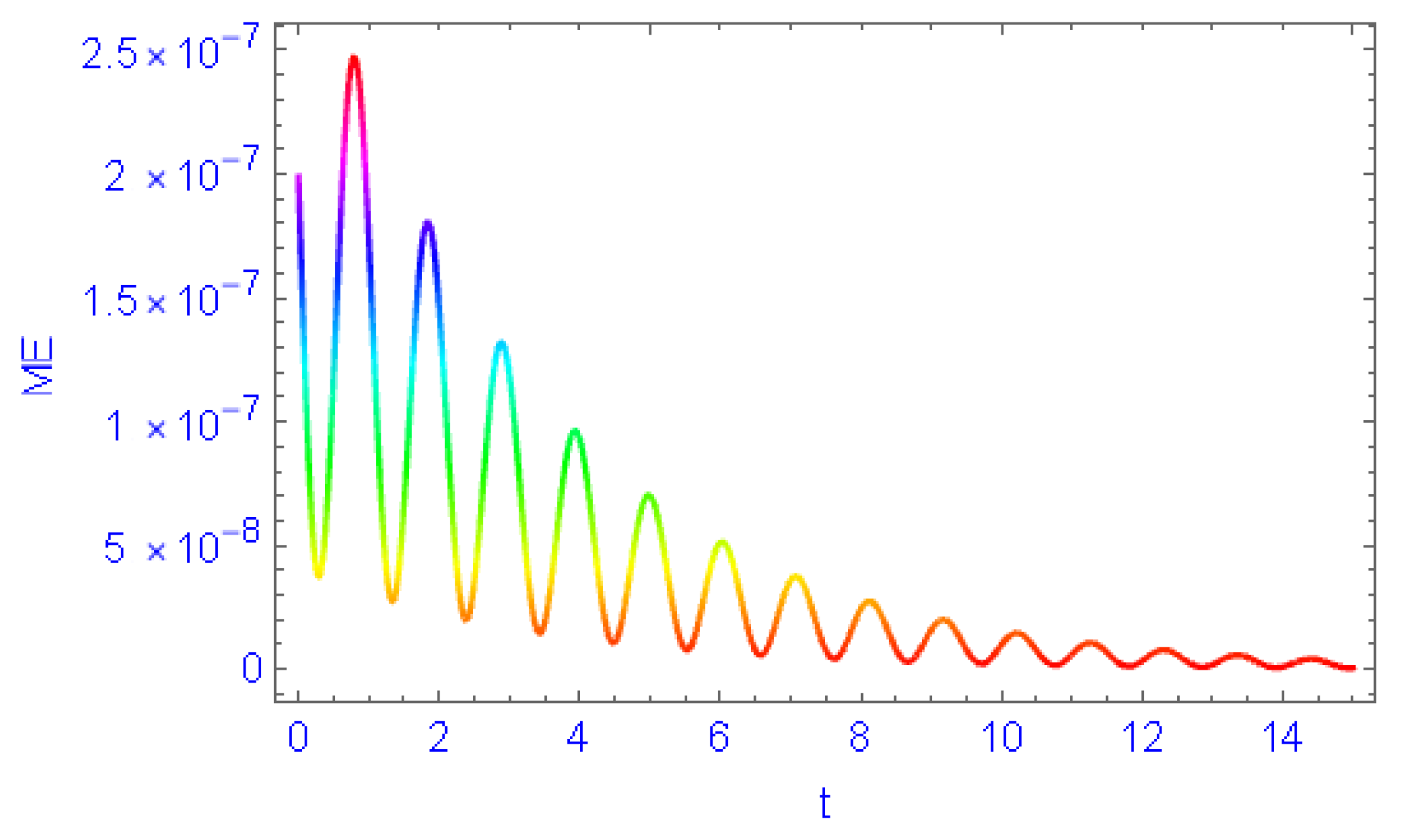


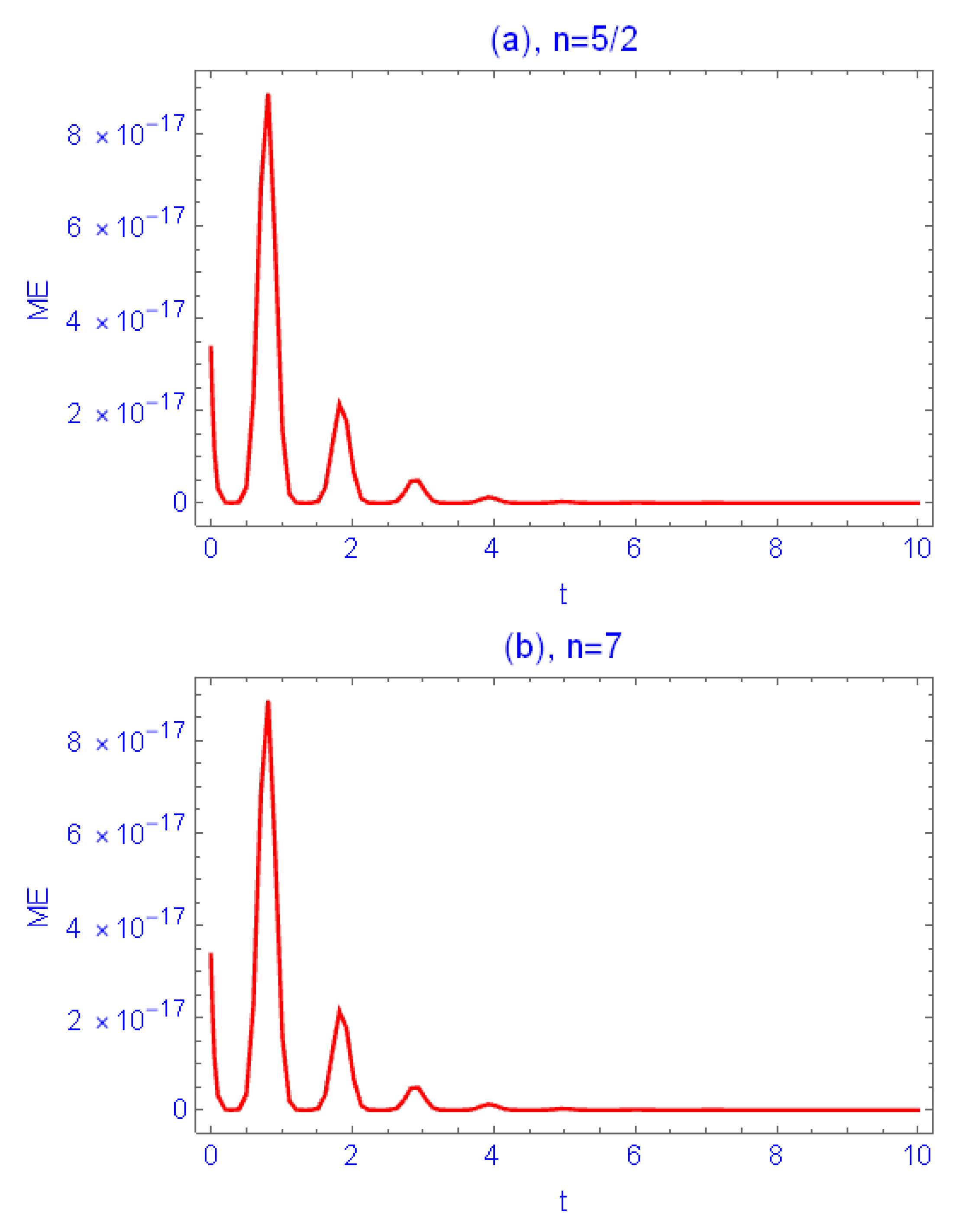

| RTs | Errors |
|---|---|
| RTs | Errors |
|---|---|
| RTs | Errors |
|---|---|
| 1.7777 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alqhtani, M.; Srivastava, R.; Abdel-Gawad, H.I.; Macías-Díaz, J.E.; Saad, K.M.; Hamanah, W.M. Insight into Functional Boiti–Leon–Mana–Pempinelli Equation and Error Control: Approximate Similarity Solutions. Mathematics 2023, 11, 4569. https://doi.org/10.3390/math11224569
Alqhtani M, Srivastava R, Abdel-Gawad HI, Macías-Díaz JE, Saad KM, Hamanah WM. Insight into Functional Boiti–Leon–Mana–Pempinelli Equation and Error Control: Approximate Similarity Solutions. Mathematics. 2023; 11(22):4569. https://doi.org/10.3390/math11224569
Chicago/Turabian StyleAlqhtani, Manal, Rekha Srivastava, Hamdy I. Abdel-Gawad, Jorge E. Macías-Díaz, Khaled M. Saad, and Waleed M. Hamanah. 2023. "Insight into Functional Boiti–Leon–Mana–Pempinelli Equation and Error Control: Approximate Similarity Solutions" Mathematics 11, no. 22: 4569. https://doi.org/10.3390/math11224569








