CFD Modeling and Experimental Validation of the Flow Processes of an External Gear Pump
Abstract
1. Introduction
2. CFD Model Development
2.1. Determination of the Pump Displacement Volume
- Gears without correction factors;
- Teeth number in range of z = 9–14;
- Center distance between the two gears a is equal to the pitch circle diameter Dm;
- The area of one tooth is equal to the area between two consecutive teeth.
2.1.1. Geometric Approach
2.1.2. Kinematic Approach
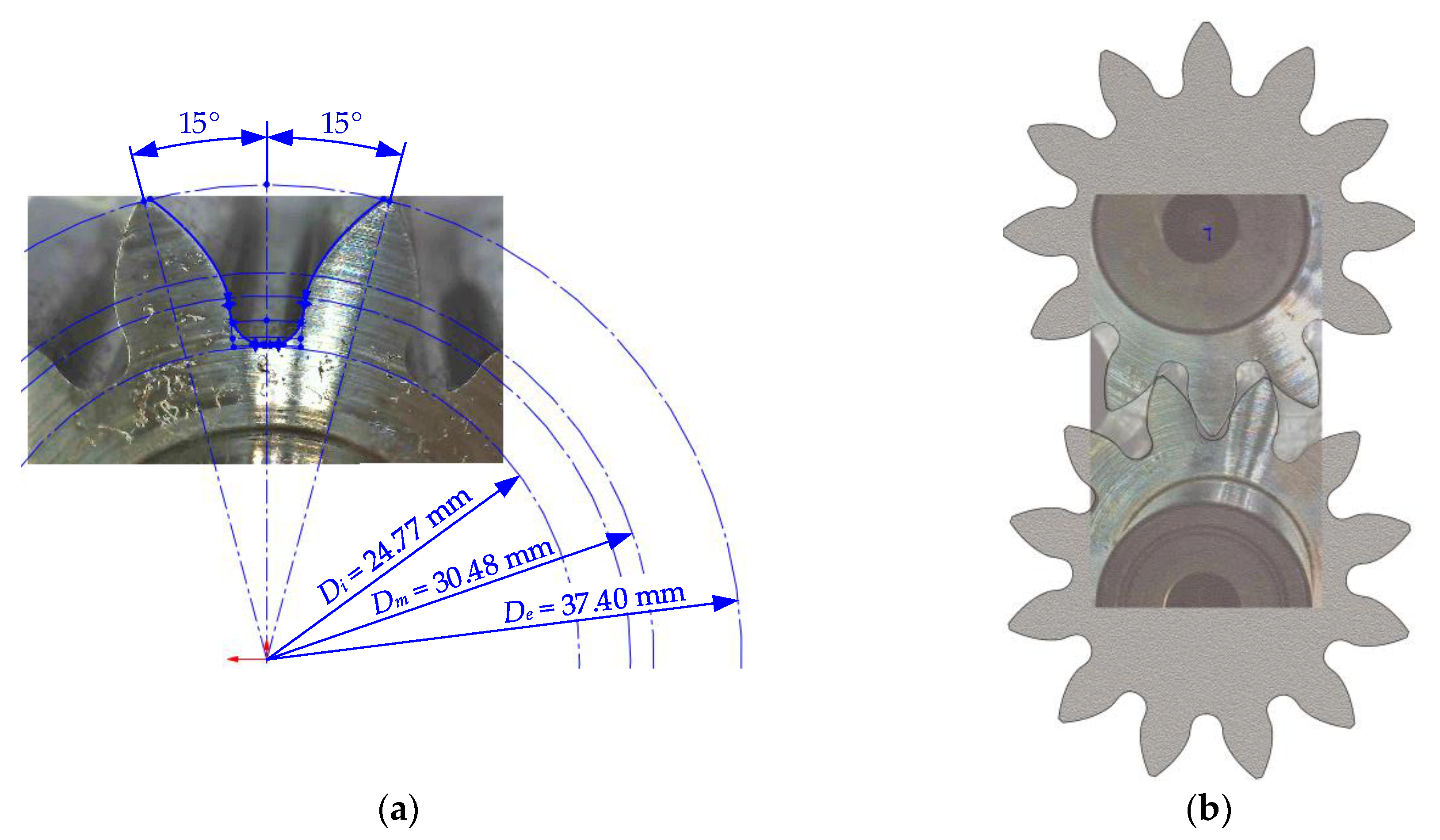
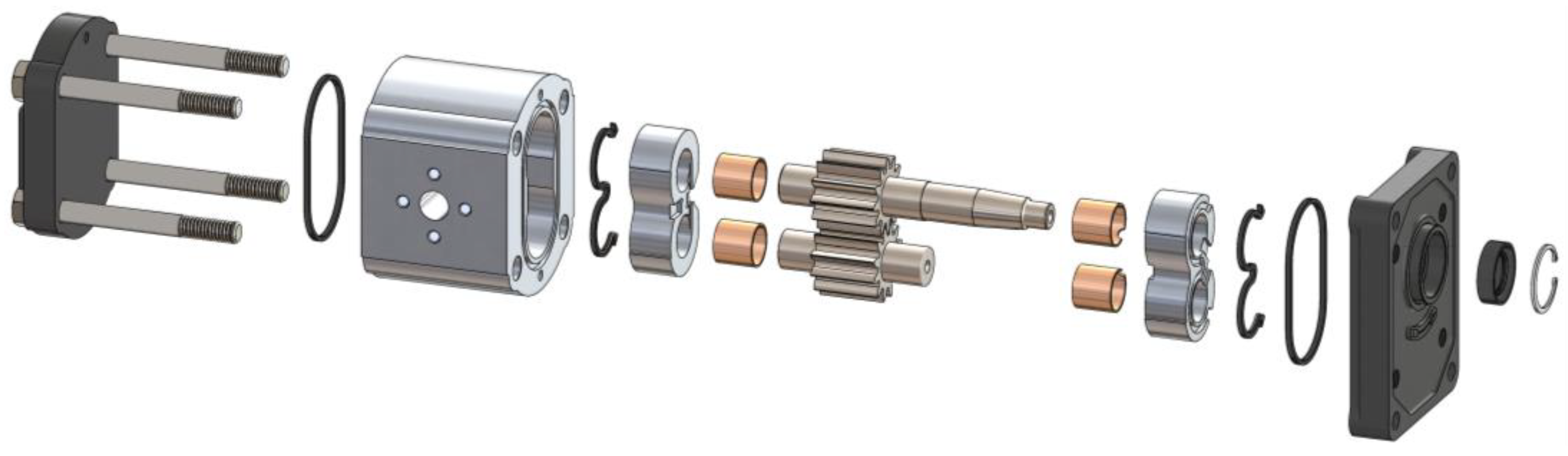
2.2. CAD Model
- Measurements with an accuracy of 0.01 mm;
- High-resolution photography with a digital microscope;
- The dimensions are partly calculated, and involutes are plotted according to the well-known formulas in machine element design theory [46]. They are derived from the number of teeth z and the diameters of the addendum and dedendum circles (De and Di);
- CAD model creation in SolidWorks® 2019 SP5.0 Education Edition environment with the imposition of sketches on the captured images (Figure 2a);
- A comparison of the resulting tooth profile with high-resolution photographs at the same scale shows satisfactory results—see Figure 2b.
- Radial gap: 0.02 mm;
- Axial gap: 0.025 mm.
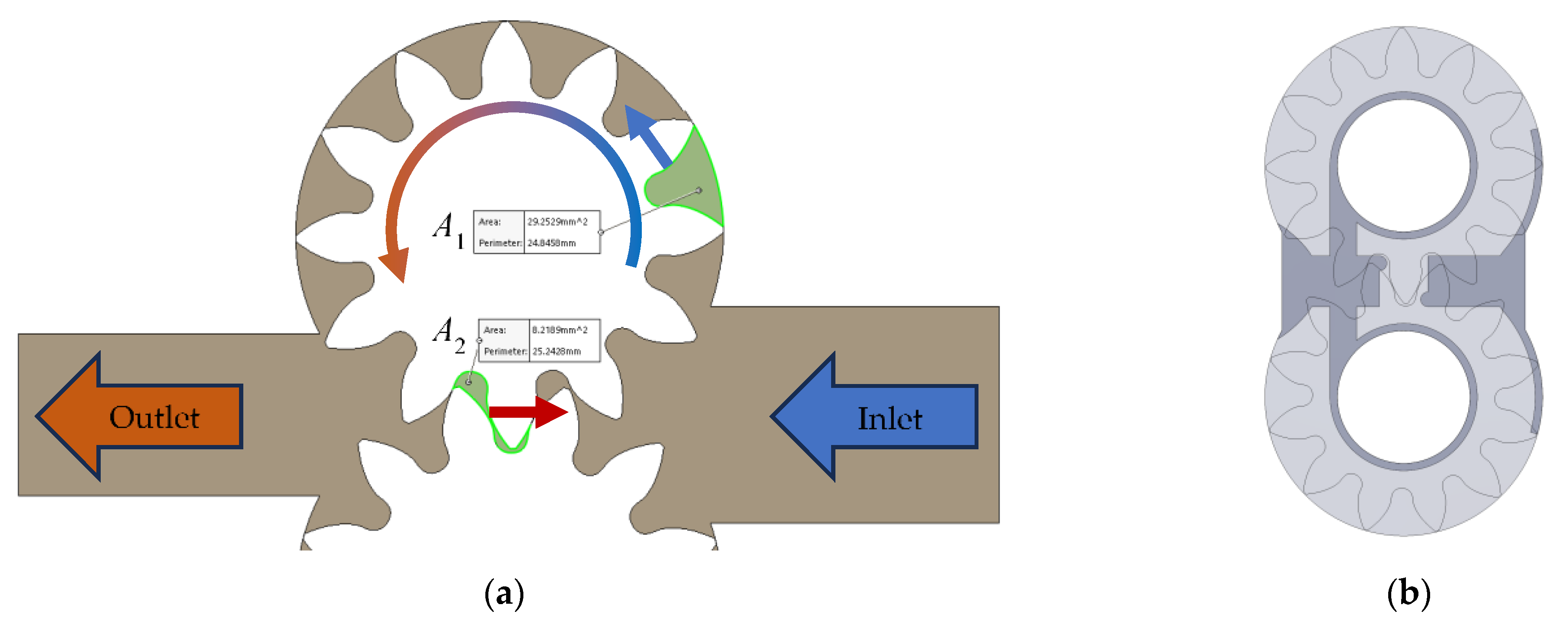
2.3. Development of a Simplified Geometrical Model for CFD Simulations
- Lubrication channels—with relatively small volume. They are not expected to influence the q(Δp) and q(t) characteristics;
- Channels to ensure more uniform deformation of the bearing bodies and more uniform wear of the bearing sleeves. These channels have a larger volume than the lubrication channels but are located away from the working chamber and are also not expected to have a significant impact on the q(Δp) and q(t) characteristics;
- Discharge channels. They ensure a more even pressure distribution, largely prevent the creation of areas of excessively high or excessively low pressure, reduce the risk of cavitation, and reduce wear. They also increase the displacement volume because they reduce the fluid transfer from the high- to the low-pressure area through the tooth meshing area. The discharge channels’ influence on q(Δp) and q(t) is significant, mainly because of their effect on the pump displacement volume.
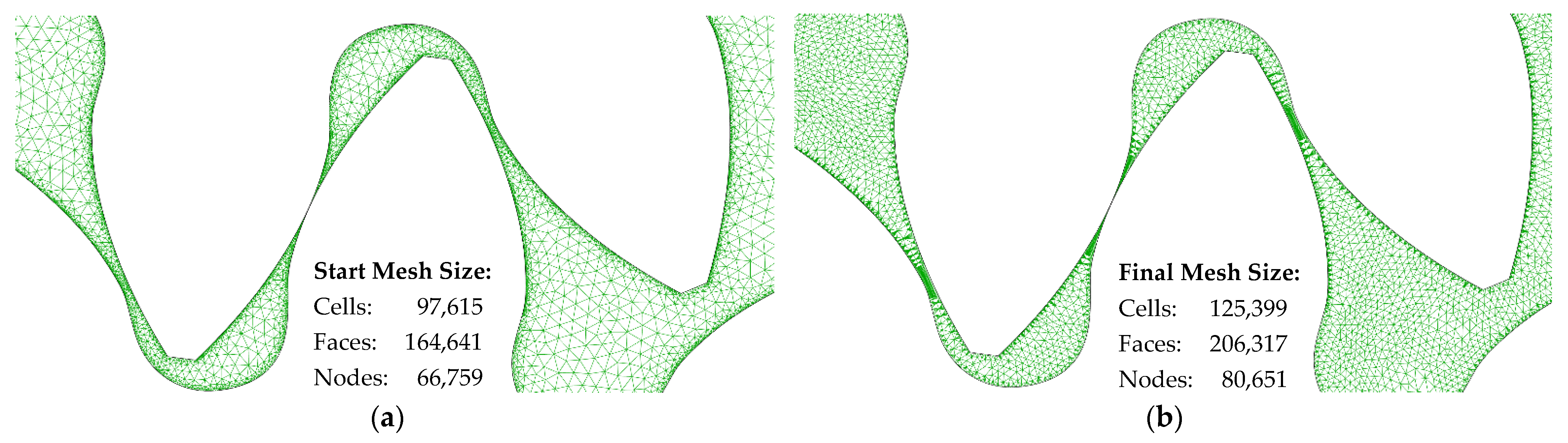
- Suction and delivery ports (Inlet Zone and Outlet Zone in Figure 4b). They do not change their shape and size during operation, which reduces the requirements for the finite elements mesh. It is appropriate to use a structured mesh of rectangular finite elements (quadrilaterals face meshing). This type of mesh gives faster and more stable solutions than other types of meshes, very often and more accurately, due to the even shape of the elements. Larger-size elements can be used without negatively affecting the solution.
- Work Zone. Due to the movement of the gears, this area changes its shape with each time step. When using a dynamic mesh CFD model, the mesh is regularly adjusted to fit the new geometry. This, in turn, with universal software packages, such as ANSYS Fluent® 2019 R3, requires the use of an unstructured mesh of triangular finite elements (all triangles method), which allows fast remeshing. To reduce the remeshing time and the risk of numerical errors (typically: floating point exception or negative cell volume), a finer mesh has to be used in this zone than in others.
2.4. Theoretical Background of the CFD Model
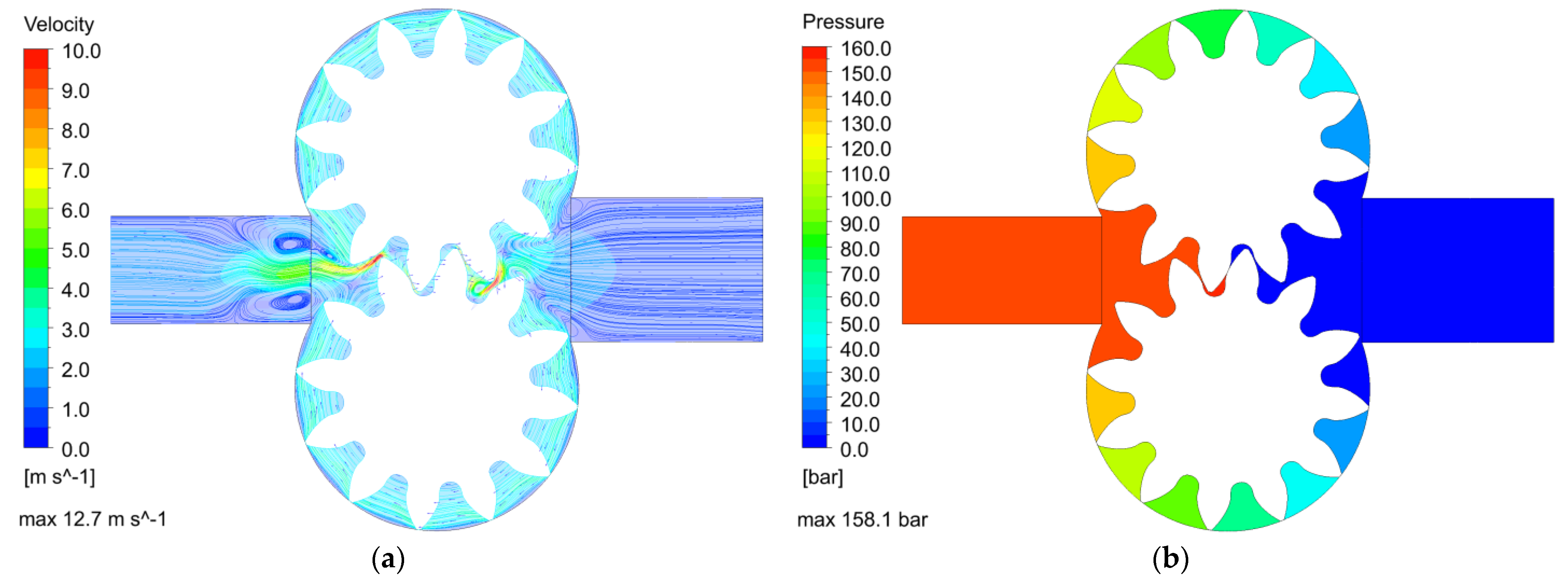
2.5. Creating a CFD Model
- Specialized gear pump modeling software. A typical representative is PumpLinx®;
- Universal CAE software containing modules for CFD simulations. A typical example is the ANSYS® software package.
- Immersed solid method and ANSYS CFX® module. Although there are not many, solutions using this approach are found in [24]. In this method, there are two separate meshes sharing the same volume: a fluid and a mobile solid immersed in it. The method has the huge advantage that it gives stable solutions on relatively large meshes, which allows us to quickly obtain results in 3D CFD simulations with significant geometries. The disadvantages are also not to be neglected, and they are the reason why this method is not preferred for modeling gear pumps. The main thing is that the phenomena near the walls, at the fluid/gear interface, cannot be accurately modeled.
- Dynamic mesh and ANSYS Fluent® module. Dynamic mesh is a feature that allows the mesh to deform and adapt to the motion of the boundaries. The method allows defining the size of the elements on the boundaries of the model, general control over the size of the elements generated during the solution (Minimum and Maximum Length Scale), partial control over the elements’ shape (Maximum Cell Skewness), and other settings. This is the preferred method in the literature for gear pump analyses, as it gives the most accurate solutions and allows maximum control in the boundary zones. Its main disadvantage is its resource intensity. Even in 2D, it requires a very fine mesh. In the areas with clearances, the elements have dimensions of the order of 5–20 μm. It requires frequent remeshing, usually at each time step. The time step is extremely small, typically 1 × 10−6 s in the literature [24], requiring thousands of time steps to be calculated. At least 20 iterations are usually used to calculate each time step. Additionally, using an unstructured mesh of pyramids (3D) or triangles (2D) results in a very large number of elements and nodes. This method is preferred in the present study. With the current setup, with a workstation with an Intel® Core™ i7-12800H processor and 32 GB RAM, the calculation speed is 100 min/30° in 2D, and the solution in 3D is practically impossible due to insufficient processing power.
2.6. General Settings of the CFD Model
- Solver type: Transient;
- Gravity: 9.81 m/s2 along Y;
- Viscose model: A standard κ-ε (two-equation type) with standard Wall Functions, as recommended in the literature, is chosen [32]. Model constants used values areCμ = 0.09; C1ε = 1.44; C2ε = 1.92; (TKE Prandtl Number) σk = 1.0; and (TDR Prandtl Number) σε = 1.3.
- Material: The working fluid is hydraulic oil (HLP 32), for which the following two material properties are set:
- -
- Density: 890 kg/m3;
- -
- Viscosity: 0.0712 kg/(m.s).
- Boundary conditions:
- -
- Inlet: Set to “Pressure Inlet” type, with a pressure of 1 bar, normal to the boundary;
- -
- Outlet: Set to “Pressure Outlet” type, with a pressure of 5, 25, 50, 75, 100, 125, or 150 bar, normal to the boundary;
- -
- Interface: Two contact regions are defined, Inlet Zone/Work Zone and Work Zone/Outlet Zone;
- -
- Internal: The three fluid regions mentioned above are defined;
- -
- Wall: The contours of all walls involved in the model are defined. There are a total of five groups: inlet walls, outlet walls, moving walls of the two gears, and work zone walls;
- -
- Mesh interfaces: The already mentioned two contact regions: Inlet Zone/Work Zone and Work Zone/Outlet Zone;
- -
- Dynamic mesh: In addition to the already-described settings, the dynamic mesh zones’ centers of rotation, as well as their rotational frequency through the User-Defined Function (UDF), are defined;
- -
- Solution Methods: Gradient—cell-based least squares; pressure—second order; momentum, turbulent kinetic energy, and turbulent dissipation rate—second order upwind;
- -
- Solution controls: Default values, except for absolute convergence criteria, which have been reduced from 1e-03 to 1e-06. This criterion is not met for 20 iterations; thus, the step never ends before all 20 iterations have been completed;
- -
- Time step size: For the purpose of the present study, the time step size is of the order of 2e-06 s, varying from 1e-06 to 3e-06, depending on the rotational frequency, as well as the stability of the solution.
- Initial condition at ;
- Velocity boundary condition on the casing wall: .
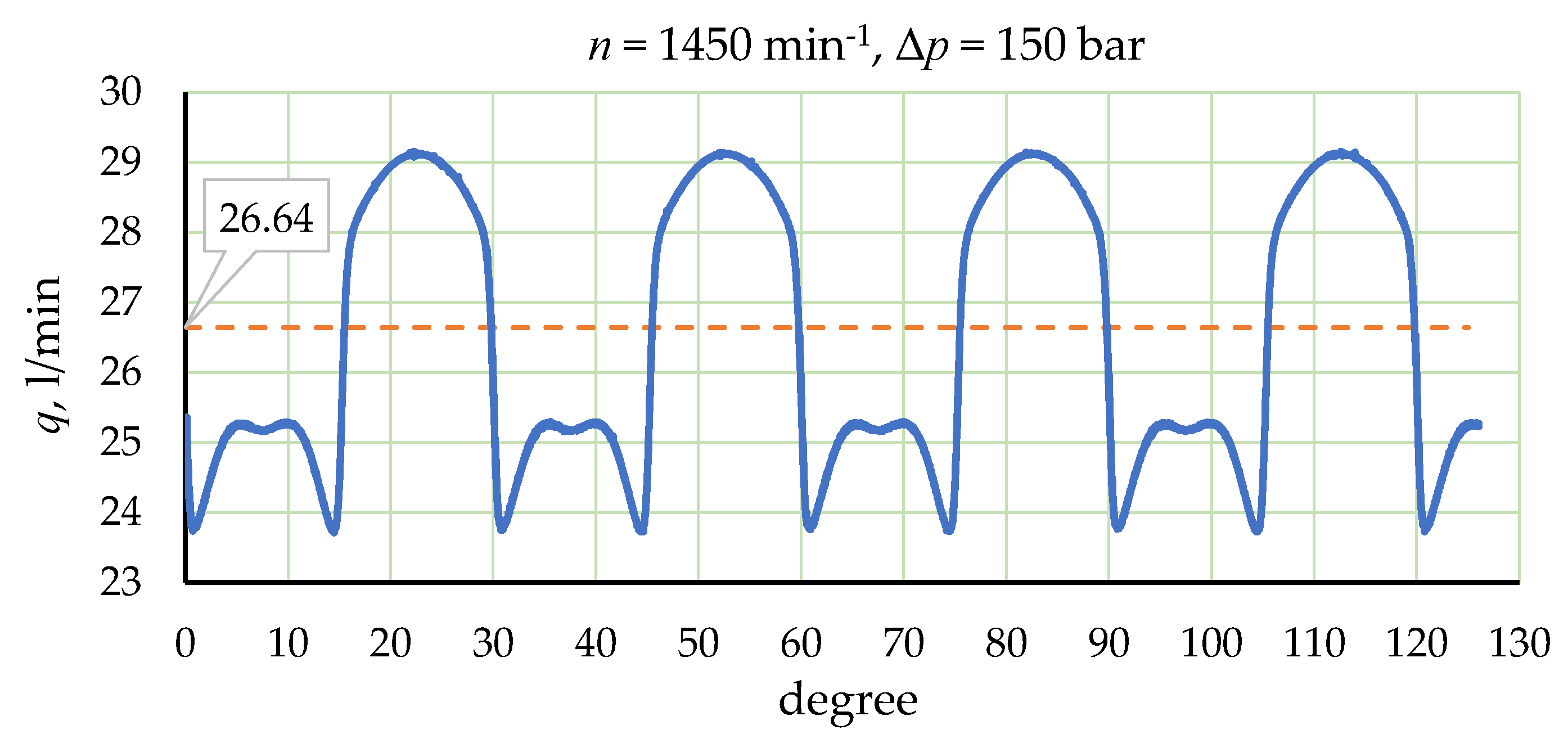
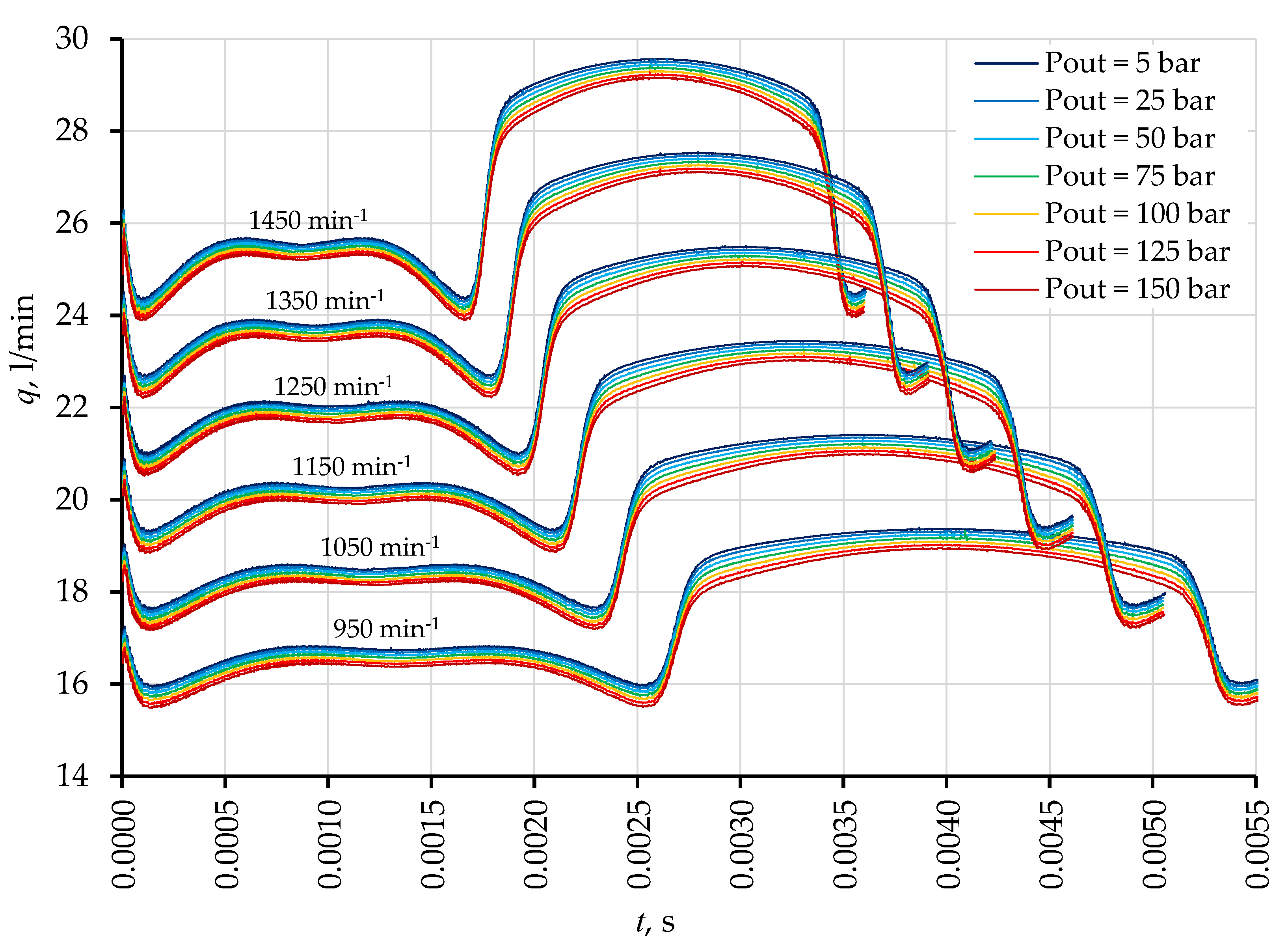
3. Numerical Results
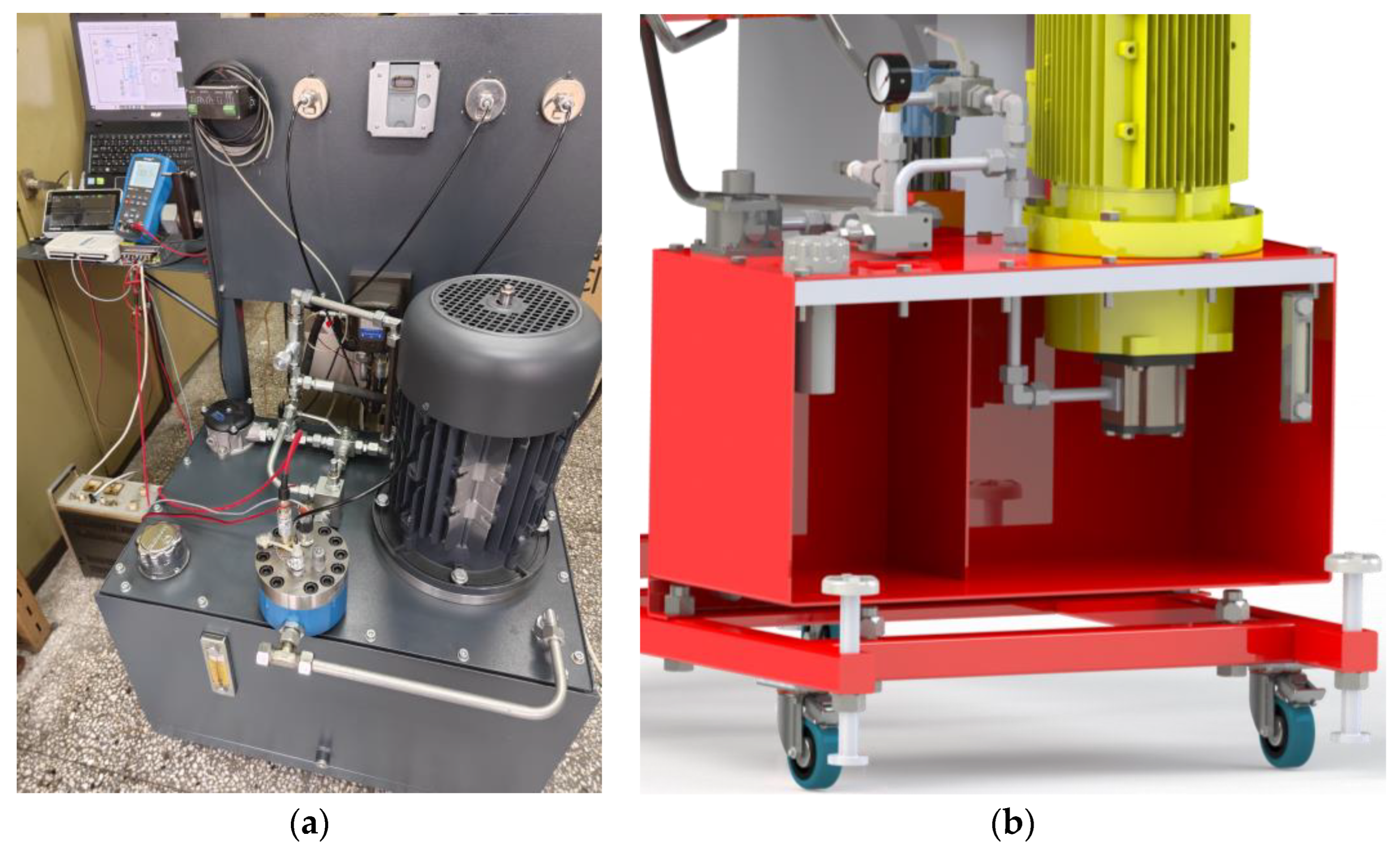
4. Experimental System Layout
5. Validation of Numerical Results and Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Findeisen, D.; Helduser, S. Ölhydraulik; Springer: Berlin, Germany, 2015. [Google Scholar]
- Ivantysyn, J.; Ivantysynova, M. Hydrostatic Pumps and Motors: Principles, Design, Performance, Modelling, Analysis, Control and Testing; Academia Books International: New Delhi, India, 2001. [Google Scholar]
- Manring, N.D.; Kasaragadda, S.B. The theoretical flow ripple of an external gear pump. J. Dyn. Syst. Meas. Control. Trans. ASME 2003, 125, 396–404. [Google Scholar] [CrossRef]
- Choudhuri, K.; Biswas, N.; Mandal, S.K.; Mitra, C.; Biswas, S. A numerical study of an external gear pump operating under different conditions. Mater. Today Proc. 2022, in press. [Google Scholar] [CrossRef]
- Zhao, X.; Vacca, A.; Dhar, S. Numerical Modeling of a Helical External Gear Pump with Continuous-Contact Gear Profile: A Comparison between a Lumped-Parameter and a 3D CFD Approach of Simulation. In Proceedings of the BATH/ASME 2018 Symposium on Fluid Power and Motion Control FPMC2018, Bath, UK, 12–14 September 2018. [Google Scholar]
- Orlandi, F.; Muzzioli, G.; Milani, M.; Paltrinieri, F.; Montorsi, L. Development of a numerical approach for the CFD simulation of a gear pump under actual operating conditions. Fluids 2023, 8, 244. [Google Scholar] [CrossRef]
- Corvaglia, A.; Rundo, M.; Bonati, S.; Rigosi, M. Simulation and experimental activity for the evaluation of the filling capability in external gear pumps. Fluids 2023, 8, 251. [Google Scholar] [CrossRef]
- Gafurov, S.; Rodionov, L.; Makaryants, G. Simulation of Gear Pump Noise Generation. In Proceedings of the 9th FPNI Ph.D. Symposium on Fluid Power FPNI 2016, Florianópolis, Brazil, 26–28 October 2016. [Google Scholar]
- Tang, C.; Wang, Y.S.; Gao, J.H.; Guo, H. Fluid-sound coupling simulation and experimental validation for noise characteristics of a variable displacement external gear pump. Noise Control. Eng. J. 2014, 62, 123–131. [Google Scholar] [CrossRef]
- Fiebig, W. Influence of the inter teeth volumes on the noise generation in external gear pumps. Arch. Acoust. 2014, 39, 261–266. [Google Scholar] [CrossRef]
- Mucchi, E.; Rivola, A.; Dalpiaz, G. Modelling dynamic behaviour and noise generation in gear pumps: Procedure and validation. Appl. Acoust. 2014, 77, 99–111. [Google Scholar] [CrossRef]
- Woo, S.; Opperwall, T.; Vacca, A.; Rigosi, M. Modeling noise sources and propagation in external gear pumps. Energies 2017, 10, 1068. [Google Scholar] [CrossRef]
- Fiebig, W. Noise Control of Fluid Power Units. In Proceedings of the 23rd International Congress on Sound and Vibration: From Ancient to Modern Acoustics, Athens, Greece, 10–14 July 2016. [Google Scholar]
- Carletti, E.; Pedrielli, F. Sound Power Levels of Hydraulic Pumps using Sound Intensity Techniques: Towards More Accurate Values? In Proceedings of the 12th International Congress on Sound and Vibration, Lisbon, Portuga, 11–14 July 2005. [Google Scholar]
- Osiński, P.; Deptuła, A.; Deptuła, A.M. Analysis of the Gear Pump’s Acoustic Properties Taking into Account the Classification of Induction Trees. Energies 2023, 16, 4460. [Google Scholar] [CrossRef]
- ISO 16902-1:2003; Hydraulic Fluid Power—Test Code for the Determination of Sound Power Levels of Pumps Using Sound Intensity Techniques: Engineering Method—Part 1: Pumps. ISO: Geneva, Switzerland, 2003.
- Mitov, A.; Nedelchev, K.; Kralov, I. Experimental Study of Sound Pressure Level in Hydraulic Power Unit with External Gear Pump. Processes 2023, 11, 2399. [Google Scholar] [CrossRef]
- Stan, L.C.; Gordes, A.N.; Agape, A.G. Numerical simulation of a gear pump. Int. J. Mod. Manuf. Technol. 2023, 15, 107–114. [Google Scholar]
- Patil, S. Numerical Simulation of Multi-Dimensional Flows in a Gear Pump. Master’s Thesis, Youngstown State University, Youngstown, OH, USA, 2006. [Google Scholar]
- Maccioni, L.; Concli, F. Computational fluid dynamics applied to lubricated mechanical components: Review of the approaches to simulate gears, bearings, and pumps. Appl. Sci. 2020, 10, 1–29. [Google Scholar] [CrossRef]
- Mali, P.S.; Joshi, G.S.; Patil, I.A. Performance improvement of external gear pump through CFD analysis. Int. Res. J. Eng. Technol. 2018, 5, 430–433. [Google Scholar]
- Del Campo, D. Analysis of the Suction Chamber of External Gear Pumps and Their Influence on Cavitation and Volumetric Efficiency. Ph.D. Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2012. [Google Scholar]
- Munih, J.; Hočevar, M.; Petrič, K.; Dular, M. Development of CFD-based procedure for 3D gear pump analysis. Eng. Appl. Comput. Fluid Mech. 2020, 14, 1023–1034. [Google Scholar] [CrossRef]
- Yoon, Y.; Park, B.-H.; Shim, J.; Han, Y.-O.; Hong, B.-J.; Yun, S.-H. Numerical simulation of three-dimensional external gear pump using immersed solid method. Appl. Therm. Eng. 2017, 118, 539–550. [Google Scholar] [CrossRef]
- Corvaglia, A.; Ferrari, A.; Rundo, M.; Vento, O. Three-dimensional model of an external gear pump with an experimental evaluation of the flow ripple. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 235, 1097–1105. [Google Scholar] [CrossRef]
- Frosina, E.; Senatore, A.; Rigosi, M. Study of a high-pressure external gear pump with a computational fluid dynamic modeling approach. Energies 2017, 10, 1113. [Google Scholar] [CrossRef]
- Močilan, M.; Husár, Š.; Labaj, J.; Žmindák, M. Non-Stationary CFD Simulation of a Gear Pump. In Proceedings of the 21st International Polish-Slovak Conference “Machine Modeling and Simulations 2016”, Hucisko, Poland, 8–11 September 2017. [Google Scholar]
- Jędraszczyk, P.; Fiebig, W. CFD model of an external gear pump. In RESRB 2016: Proceedings of the 13th International Scientific Conference, Wrocław, Poland, 22–24 June 2016; Springer: Berlin, Germany, 2017; pp. 221–231. [Google Scholar]
- Castilla, R.; Gamez-Montero, P.J.; Del Campo, D.; Raush, G.; Garcia-Vilchez, M.; Codina, E. Three-dimensional numerical simulation of an external gear pump with decompression slot and meshing contact point. J. Fluids Eng. Trans. ASME 2015, 137, 041105. [Google Scholar] [CrossRef]
- Mhana, W.; Popov, G. Method for Investigation of the Pressure Variation in the Chambers of Gear Pumps with Symmetric and Asymmetric Tooth Profiles Used in Electrohydraulic Drive Systems. In Proceedings of the 16th Conference on Electrical Machines, Drives and Power Systems, Varna, Bulgaria, 6–8 June 2019. [Google Scholar]
- Corvaglia, A.; Rundo, M.; Casoli, P.; Lettini, A. Evaluation of tooth space pressure and incomplete filling in external gear pumps by means of three-dimensional CFD simulations. Energies 2021, 14, 342. [Google Scholar] [CrossRef]
- Ouyang, T.; Mo, X.; Lu, Y.; Wang, J. CFD-vibration coupled model for predicting cavitation in gear transmissions. Int. J. Mech. Sci. 2022, 225, 107377. [Google Scholar] [CrossRef]
- Killedar, J.S. CFD Analysis of Gear Pump. Master’s Thesis, Youngstown State University, Youngstown, OH, USA, 2005. [Google Scholar]
- Heisler, A.S.; Moskwa, J.J.; Fronczak, F.J. Simulated Helical Gear Pump Analysis using a New CFD Approach. In Proceedings of the ASME Fluids Engineering Division Summer Conference FEDSM2009, Vail, CO, USA, 2–6 August 2009. [Google Scholar]
- Labaj, J.; Husar, S. Analysis of Gear Pump Designed for Manufacturing Processes. Appl. Mech. Mater. 2015, 803, 163–172. [Google Scholar] [CrossRef]
- Lee, J.-H.; Lee, S.-W. Numerical Simulations of Cavitation Flow in Volumetric Gear Pump. J. Korean Soc. Vis. 2011, 9, 28–34. [Google Scholar]
- Del Campo, D.; Castilla, R.; Raush, G.A.; Gamez Montero, P.J.; Codina, E. Numerical analysis of external gear pumps including cavitation. J. Fluids Eng. Trans. ASME 2012, 134, 081105. [Google Scholar] [CrossRef]
- Strasser, W. CFD Investigation of Gear Pump Mixing. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Orlando, FL, USA, 5–11 November 2005. [Google Scholar]
- Strasser, W. CFD investigation of gear pump mixing using deforming/agglomerating mesh. J. Fluids Eng. Trans. ASME 2007, 129, 476–484. [Google Scholar] [CrossRef]
- Ghazanfarian, J.; Ghanbari, D. Computational fluid dynamics investigation of turbulent flow inside a rotary double external gear pump. J. Fluids Eng. Trans. ASME 2015, 137, 021101. [Google Scholar] [CrossRef]
- Qi, F.; Dhar, S.; Nichani, V.H.; Srinivasan, C.; Wang, D.M.; Yang, L.; Bing, Z.; Yang, J.J. A CFD study of an Electronic Hydraulic Power Steering Helical External Gear Pump: Model Development, Validation and Application. SAE Int. J. Passeng. Cars—Mech. Syst. 2016, 9, 346–352. [Google Scholar] [CrossRef]
- Martínez, J. Mesh Handling for the CFD Simulation of External Gear Pumps. In Positive Displacement Machines: Modern Design Innovations and Tools, 1st ed.; Sultan, I., Phung, T., Eds.; Elsevier: Amsterdam, The Netherlands, 2019; pp. 345–368. [Google Scholar]
- Ull, A.R. Study of Mesh Deformation Features of an Open Source CFD Package and Application to a Gear Pump Simulation; Enginyeria Aeronàutica, Universitat Politècnica De Catalunya: Barcelona, Spain, 2012. [Google Scholar]
- Mucchi, E.; Dalpiaz, G.; Rivola, A. Dynamic behavior of gear pumps: Effect of variations in operational and design parameters. Meccanica 2011, 46, 1191–1212. [Google Scholar] [CrossRef]
- Marinaro, G.; Frosina, E.; Senatore, A. A Numerical Analysis of an Innovative Flow Ripple Reduction Method for External Gear Pumps. Energies 2021, 14, 471. [Google Scholar] [CrossRef]
- Mott, R. Machine Elements in Mechanical Design; Prentice Hall: Hoboken, NJ, USA, 2004. [Google Scholar]
- Will, D.; Ströhl, H. Einführung in die Hydraulik und Pneumatik; VEB Verlag Technik: Berlin, Germany, 1983. [Google Scholar]
- Findeisen, F.; Findeisen, D. Ölhydraulik in Theorie und Anwendung; Schweizer Verlag Haus: Zürich, Switzerland, 1968. [Google Scholar]
- Angelov, I. Hydraulic Transmissions; TU-Sofia: Sofia, Bulgaria, 2015. [Google Scholar]
- Bashta, T.M. Engineering Hydraulics: Handbook; Mashinostroenie: Moscow, Russia, 1963. [Google Scholar]
- Cengel, Y.A.; Cimbala, J.M. Fluid Mechanics: Fundamental and Applications; McGraw-Hill: New York, NY, USA, 2013. [Google Scholar]
- Szwemin, P.; Fiebig, W. The Influence of Radial and Axial Gaps on Volumetric Efficiency of External Gear Pumps. Energies 2021, 14, 4468. [Google Scholar] [CrossRef]
- Adake, D.G.; Dhote, N.D.; Khond, M.P. Experimentation and 2D Fluid Flow Simulation over an External Gear Pump. J. Phys. Conf. Ser. 2023, 2601, 012029. [Google Scholar] [CrossRef]
- Zhou, W.; Yu, D.; Wang, Y.; Shi, J.; Gan, B. Research on the Fluid-Induced Excitation Characteristics of the Centrifugal Pump Considering the Compound Whirl Effect. Facta Univ. Ser. Mech. Eng. 2023, 21, 223. [Google Scholar] [CrossRef]
- Bilalov, R.A.; Smetannikov, O.Y. Numerical Study of the Hydrodynamics of an External Gear Pump. J. Appl. Mech. Tech. Phys. 2022, 63, 1284–1293. [Google Scholar] [CrossRef]
- Li, X.; Zhang, L.; Zhang, Y. Numerical Study on the Influence of Different Operating Conditions on Mixing Uniformity of the Helical Gear Pump Mixer. Processes 2023, 11, 3223. [Google Scholar] [CrossRef]





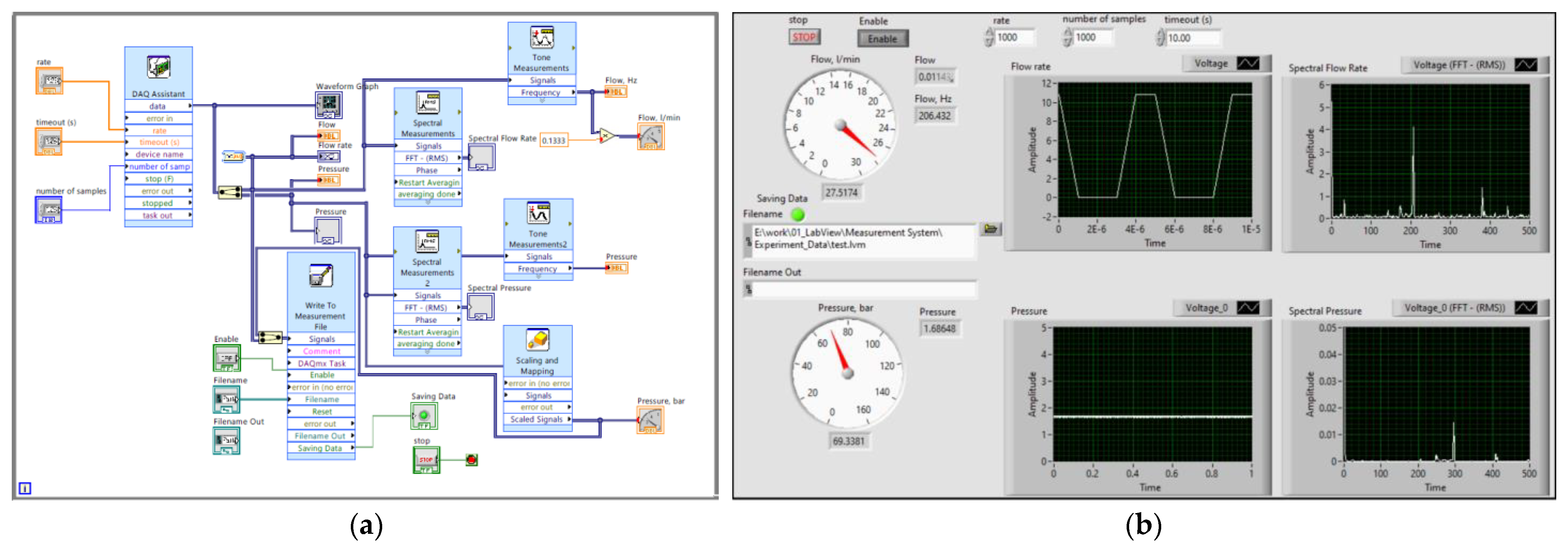
| Component | Model | Parameters |
|---|---|---|
| Tank | Custom design | V = 120 L |
| Electric Motor | Miksan 134 M4 | PEM = 7.5 kW n = 1500 min−1 |
| External Gear Pump | - | Vp = 19 cm3 |
| Direct Operated Pressure Relief Valve | CPL40/12 | qmax = 40 L/min ∆pmax = 25 MPa |
| Throttle Check Valve | VRFU9003 | qmax = 50 L/min ∆pmax = 35 MPa |
| 3-Way Ball “L”-type Valve | GB3VH | qmax = 50 L/min ∆pmax = 35 MPa |
| Return Filter Group | MPF1002AG3P25NBP01 | qmax = 50 L/min ƞ = 25 µm |
| Frequency Inverter | HNC HV 100 | U = 380 V P = 7.5 kW |
| Flow Meter | QG 100 HySense RS 300 | Gear type q = 0.7–70 L/min ∆pmax = 42 MPa |
| Pressure Transducer | MBS1250 | ∆p = 0–25 MPa Uout = 0.5–4.5 V |
| DAQ Device | NI USB 6211 | 8 Diff. or 16 SE ADC: 16 bits 250 kS/s |
| DC Power Supply | TEC 88 | Uin = 230 VAC Uout = 0.5–12 VDC I = 0–2 A |
| Low Pass Filter | Custom design | R = 100 kΩ L = 100 μH C = 4.7 μF |
| Digital Tachometer (Laser) | DT2234A | nmax = 100 000 min−1 |
| Design | Results | |||||
|---|---|---|---|---|---|---|
| nCFD min−1 | ΔpCFD bar | qCFD,AVG L/min | nEXP min−1 | qEXP,AVG, L/min | ΔpEXP,AVG bar | FIT % |
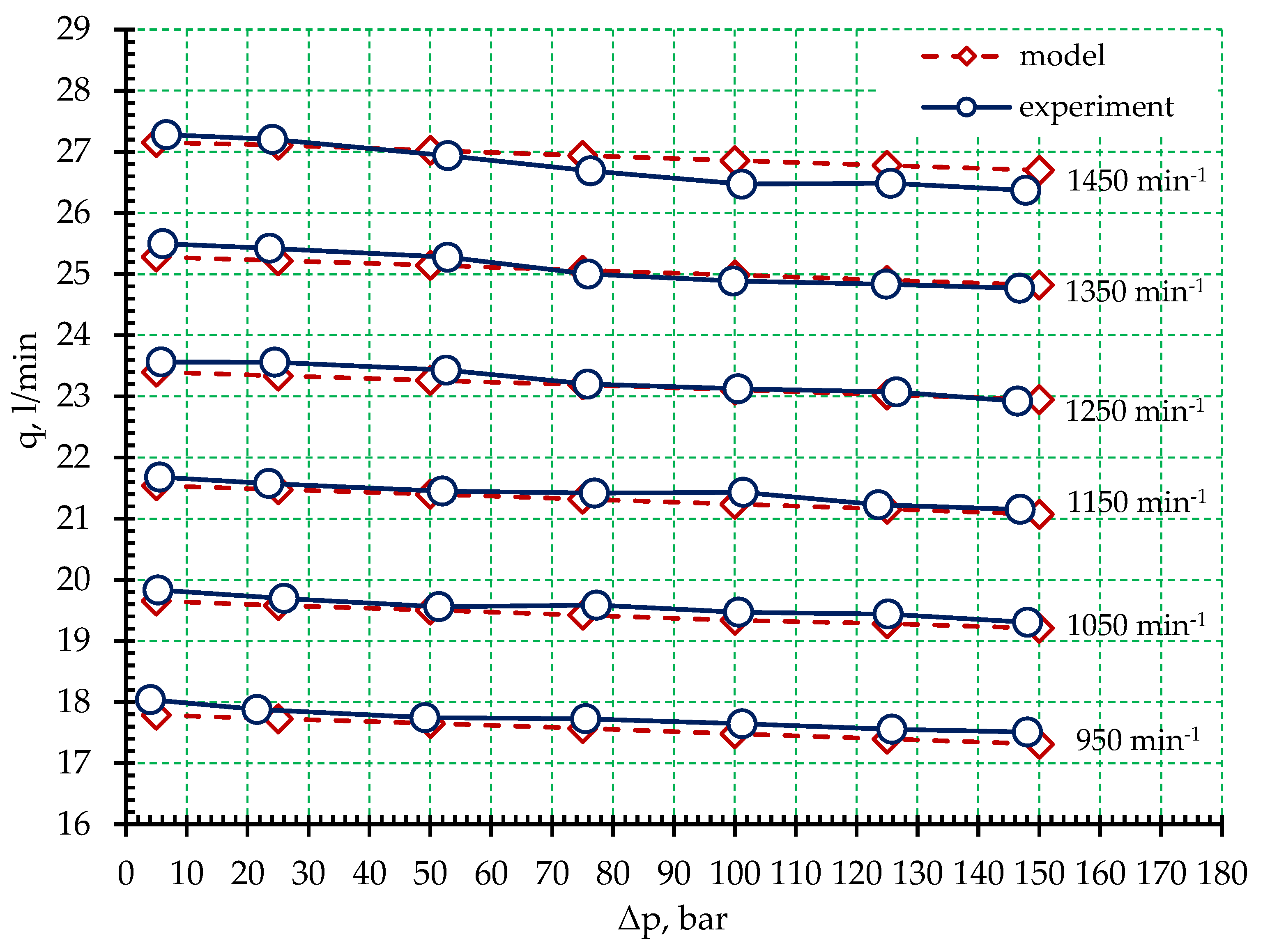
| 950 | 5 | 17.79 | 951 | 18.04 | 4.04 | 93.38 |
| 25 | 17.73 | 950 | 17.88 | 21.54 | ||
| 50 | 17.65 | 949 | 17.75 | 49.14 | ||
| 75 | 17.57 | 950 | 17.73 | 75.51 | ||
| 100 | 17.48 | 953 | 17.64 | 101.23 | ||
| 125 | 17.40 | 949 | 17.56 | 125.80 | ||
| 150 | 17.31 | 952 | 17.51 | 148.06 | ||
| 1050 | 5 | 19.66 | 1048 | 19.83 | 5.29 | 95.44 |
| 25 | 19.58 | 1049 | 19.69 | 25.98 | ||
| 50 | 19.51 | 1048 | 19.56 | 51.39 | ||
| 75 | 19.42 | 1050 | 19.59 | 77.32 | ||
| 100 | 19.34 | 1051 | 19.47 | 100.66 | ||
| 125 | 19.28 | 1053 | 19.44 | 125.21 | ||
| 150 | 19.21 | 1048 | 19.31 | 148.11 | ||
| 1150 | 5 | 21.54 | 1147 | 21.68 | 5.58 | 96.58 |
| 25 | 21.48 | 1148 | 21.57 | 23.49 | ||
| 50 | 21.40 | 1148 | 21.45 | 51.97 | ||
| 75 | 21.32 | 1152 | 21.42 | 76.97 | ||
| 100 | 21.24 | 1154 | 21.43 | 101.38 | ||
| 125 | 21.16 | 1149 | 21.23 | 123.62 | ||
| 150 | 21.07 | 1145 | 21.15 | 146.89 | ||
| 1250 | 5 | 23.40 | 1248 | 23.56 | 5.79 | 97.21 |
| 25 | 23.34 | 1254 | 23.56 | 24.46 | ||
| 50 | 23.26 | 1256 | 23.43 | 52.60 | ||
| 75 | 23.19 | 1248 | 23.20 | 75.86 | ||
| 100 | 23.11 | 1246 | 23.12 | 100.54 | ||
| 125 | 23.03 | 1251 | 23.07 | 126.57 | ||
| 150 | 22.95 | 1246 | 22.92 | 146.45 | ||
| 1350 | 5 | 25.28 | 1348 | 25.50 | 6.08 | 96.72 |
| 25 | 25.22 | 1352 | 25.42 | 23.62 | ||
| 50 | 25.15 | 1353 | 25.28 | 52.86 | ||
| 75 | 25.06 | 1348 | 25.00 | 75.91 | ||
| 100 | 24.99 | 1353 | 24.89 | 99.75 | ||
| 125 | 24.90 | 1348 | 24.84 | 124.87 | ||
| 150 | 24.82 | 1349 | 24.77 | 146.79 | ||
| 1450 | 5 | 27.15 | 1447 | 27.28 | 6.67 | 94.10 |
| 25 | 27.11 | 1448 | 27.20 | 24.07 | ||
| 50 | 27.02 | 1447 | 26.94 | 52.88 | ||
| 75 | 26.94 | 1446 | 26.69 | 76.31 | ||
| 100 | 26.86 | 1444 | 26.47 | 101.14 | ||
| 125 | 26.78 | 1450 | 26.49 | 125.59 | ||
| 150 | 26.70 | 1450 | 26.37 | 147.81 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mitov, A.; Nikolov, N.; Nedelchev, K.; Kralov, I. CFD Modeling and Experimental Validation of the Flow Processes of an External Gear Pump. Processes 2024, 12, 261. https://doi.org/10.3390/pr12020261
Mitov A, Nikolov N, Nedelchev K, Kralov I. CFD Modeling and Experimental Validation of the Flow Processes of an External Gear Pump. Processes. 2024; 12(2):261. https://doi.org/10.3390/pr12020261
Chicago/Turabian StyleMitov, Alexander, Nikolay Nikolov, Krasimir Nedelchev, and Ivan Kralov. 2024. "CFD Modeling and Experimental Validation of the Flow Processes of an External Gear Pump" Processes 12, no. 2: 261. https://doi.org/10.3390/pr12020261
APA StyleMitov, A., Nikolov, N., Nedelchev, K., & Kralov, I. (2024). CFD Modeling and Experimental Validation of the Flow Processes of an External Gear Pump. Processes, 12(2), 261. https://doi.org/10.3390/pr12020261






