Second-Order Sidebands and Group Delays in Coupled Optomechanical Cavity System with a Cubic Nonlinear Harmonic Oscillator
Abstract
:1. Introduction
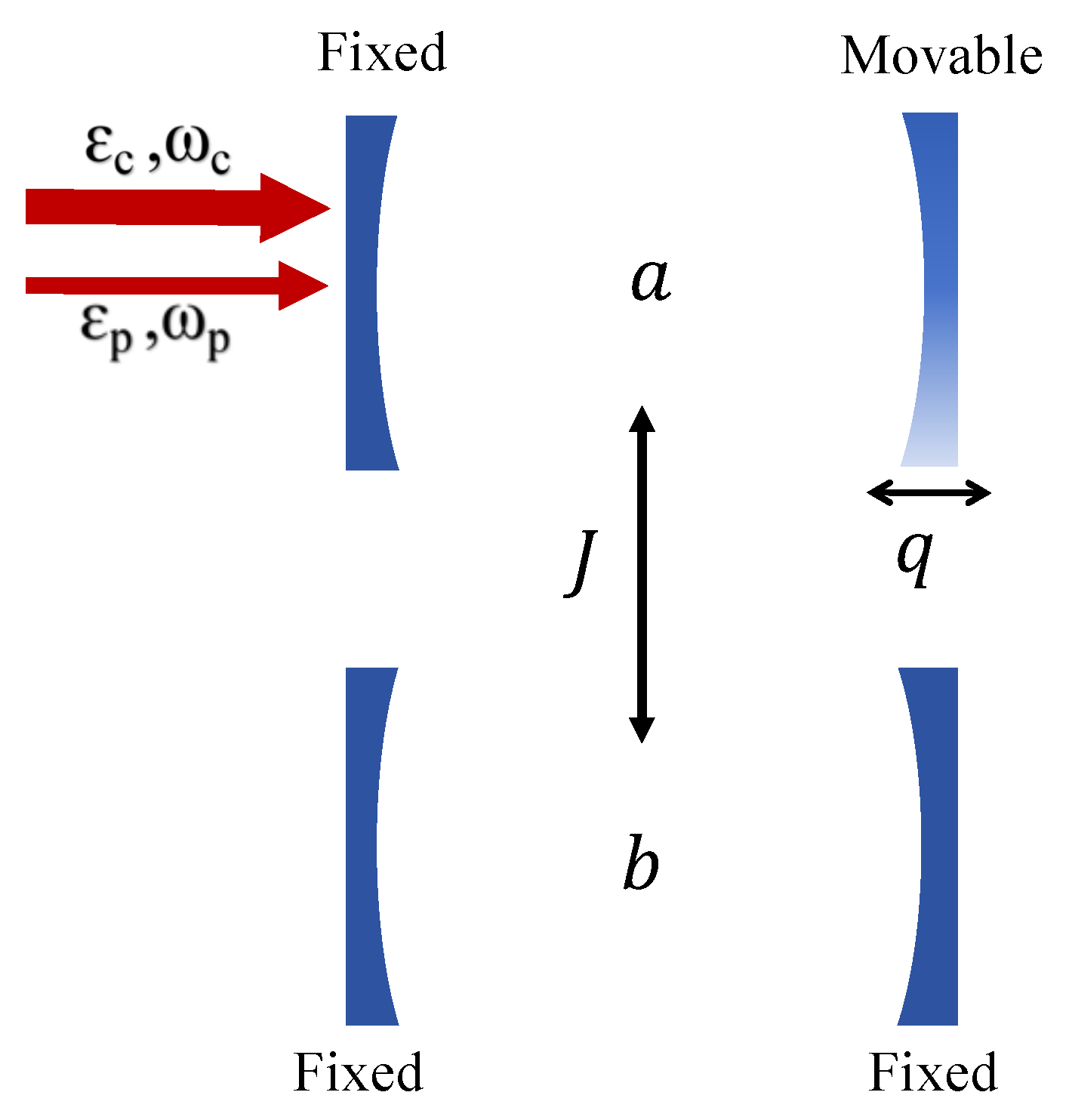
2. Theoretical Model
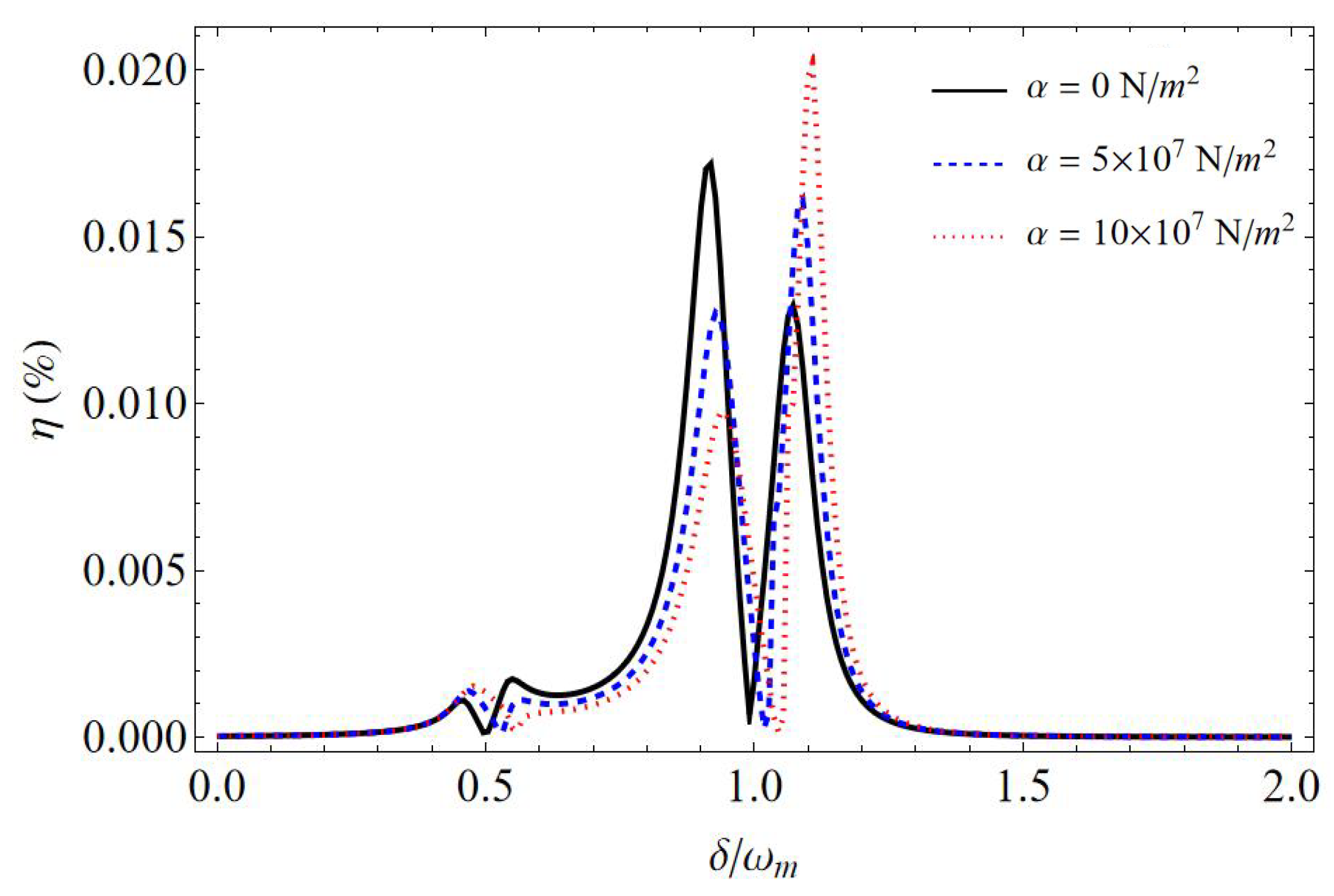
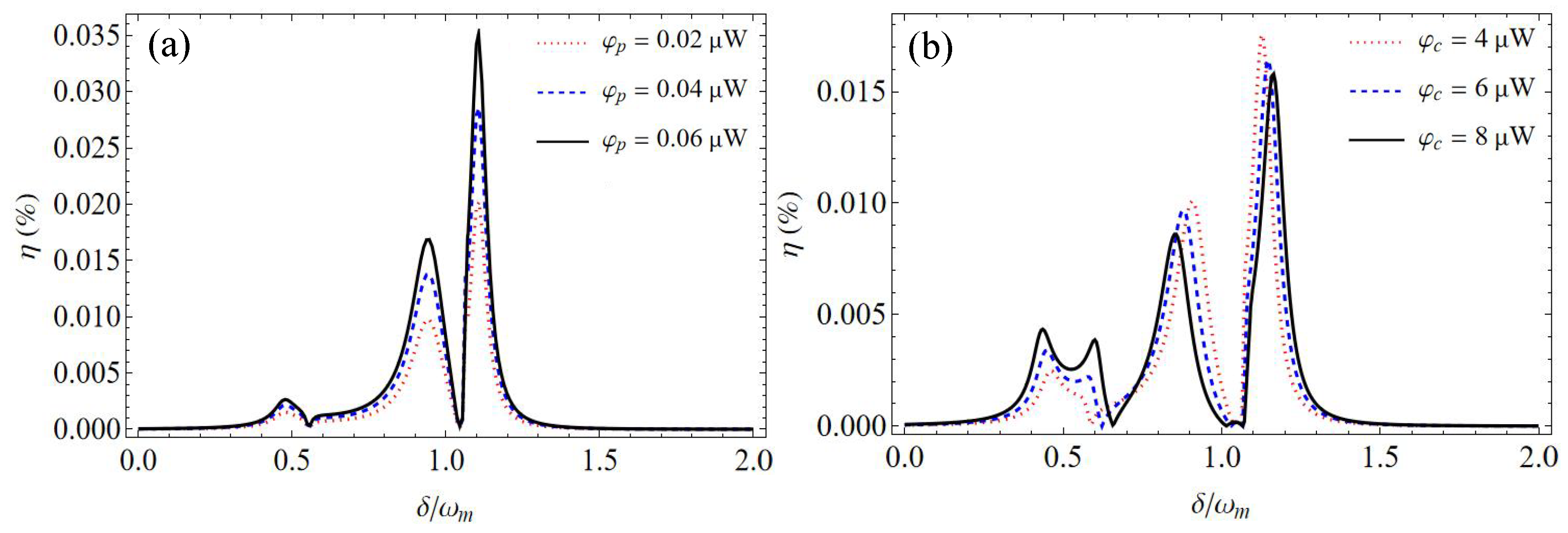
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Aspelmeyer, M.; Kippenberg, T.J.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391. [Google Scholar] [CrossRef]
- Stannigel, K.; Rabl, P.; Sørensen, A.S.; Zoller, P.; Lukin, M.D. Opto-mechanical transducers for long-distance quantum communication. Phys. Rev. Lett. 2010, 105, 220501. [Google Scholar] [CrossRef]
- Gavartin, E.; Verlot, P.; Kippenberg, T.J. A hybrid on-chip optonanomechanical transducer for ultra-sensitive force measurements. Nat. Nanotechnol. 2012, 7, 509–514. [Google Scholar] [CrossRef]
- Clark, J.B.; Lecocq, F.; Simmonds, R.W.; Aumentado, J.; Teufel, J.D. Sideband cooling beyond the quantum backaction limit with squeezed light. Nature 2017, 541, 191–195. [Google Scholar] [CrossRef]
- Qiu, L.; Shomroni, I.; Seidler, P.; Kippenberg, T.J. Laser cooling of a nanomechanical oscillator to its zero-point energy. Phys. Rev. Lett. 2020, 124, 173601. [Google Scholar] [CrossRef]
- Huang, S.; Hao, H.; Chen, A. The optomechanical response of a cubic anharmonic oscillator. Appl. Sci. 2020, 10, 5719. [Google Scholar] [CrossRef]
- Lemonde, M.A.; Didier, N.; Clerk, A.A. Nonlinear interaction effects in a strongly driven optomechanical cavity. Phys. Rev. Lett. 2013, 111, 053602. [Google Scholar] [PubMed]
- Børkje, K.; Nunnenkamp, A.; Teufel, J.D.; Girvin, S.M. Signatures of nonlinear cavity optomechanics in the weak coupling regime. Phys. Rev. Lett. 2013, 111, 053603. [Google Scholar] [CrossRef] [PubMed]
- Riedinger, R.; Hong, S.; Norte, R.A.; Slater, J.A.; Shang, J.; Krause, A.G.; Anant, V.; Aspelmeyer, M.; Groblacher, S. Non-classical correlations between single photons and phonons from a mechanical oscillator. Nature 2016, 530, 313–316. [Google Scholar] [CrossRef] [PubMed]
- Paternostro, M.; Vitali, D.; Gigan, S.; Kim, M.S.; Brukner, C.; Eisert, J.; Aspelmeyer, M. Creating and probing multipartite macroscopic entanglement with light. Phys. Rev. Lett. 2007, 99, 250401. [Google Scholar] [CrossRef] [PubMed]
- Palomaki, T.A.; Teufel, J.D.; Simmonds, R.W.; Lehnert, K.W. Entangling mechanical motion with microwave fields. Science 2013, 342, 710–713. [Google Scholar] [CrossRef] [PubMed]
- Grblacher, S.; Hammerer, K.; Vanner, M.R.; Aspelmeyer, M. Observation of strong coupling between a micromechanical resonator and an optical cavity field. Nature 2009, 460, 724–727. [Google Scholar] [CrossRef]
- Dobrindt, J.M.; Wilson-Rae, I.; Kippenberg, T.J. Parametric Normal-mode splitting in cavity optomechanics. Phys. Rev. Lett. 2008, 101, 263602. [Google Scholar] [CrossRef] [PubMed]
- Rossi, M.; Kralj, N.; Zippilli, S.; Natali, R.; Borrielli, A.; Pandraud, G.; Serra, E.; Di Giuseppe, G.; Vitali, D. Normal-mode splitting in a weakly coupled optomechanical system. Phys. Rev. Lett. 2018, 120, 073601. [Google Scholar] [CrossRef]
- Jaehne, K.; Genes, C.; Hammerer, K.; Wallquist, M.; Polzik, E.S.; Zoller, P. Cavity-assisted squeezing of a mechanical oscillator. Phys. Rev. A 2009, 79, 063819. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Huang, S. Strong mechanical squeezing and its detection. Phys. Rev. A 2016, 93, 043844. [Google Scholar] [CrossRef]
- Wollman, E.E.; Lei, C.U.; Weinstein, A.J.; Suh, J.; Kronwald, A.; Marquardt, F.; Clerk, A.A.; Schwab, K.C. Quantum squeezing of motion in a mechanical resonator. Science 2015, 349, 952–955. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Huang, S. Electromagnetically induced transparency in mechanical effects of light. Phys. Rev. A 2010, 81, 041803. [Google Scholar] [CrossRef]
- Weis, S.; Riviere, R.; Deleglise, S.; Gavartin, E.; Arcizet, O.; Schliesser, A.; Kippenberg, T.J. Optomechanically induced transparency. Science 2010, 330, 1520–1523. [Google Scholar] [CrossRef]
- Safavi-Naeini, A.H.; Alegre, T.M.; Chan, J.; Eichenfield, M.; Winger, M.; Lin, Q.; Hill, J.T.; Chang, D.E.; Painter, O. Electromagnetically induced transparency and slow light with optomechanics. Nature 2011, 472, 69–73. [Google Scholar] [CrossRef]
- Dong, C.; Fiore, V.; Kuzyk, M.C.; Wang, H. Transient optomechanically induced transparency in a silica microsphere. Phys. Rev. A 2013, 87, 055802. [Google Scholar] [CrossRef]
- Jain, M.; Xia, H.; Yin, G.Y.; Merriam, A.J.; Harris, S.E. Efficient nonlinear frequency conversion with maximal atomic coherence. Phys. Rev. Lett. 1996, 77, 4326. [Google Scholar] [CrossRef] [PubMed]
- Zhou, X.; Hocke, F.; Schliesser, A.; Marx, A.; Huebl, H.; Gross, R.; Kippenberg, T.J. Slowing, advancing and switching of microwave signals using circuit nanoelectromechanics. Nat. Phys. 2013, 9, 179–184. [Google Scholar] [CrossRef]
- Lvovsky, A.I.; Sanders, B.C.; Tittel, W. Optical quantum memory. Nat. Photonics 2009, 3, 706–714. [Google Scholar] [CrossRef]
- Agarwal, G.S.; Huang, S. Optomechanical systems as single photon routers. Phys. Rev. A 2012, 85, 021801(R). [Google Scholar] [CrossRef]
- Karuza, M.; Biancofiore, C.; Bawaj, M.; Molinelli, C.; Galassi, M.; Natali, R.; Tombesi, P.; Di Giuseppe, G.; Vitali, D. Optomechanically induced transparency in a membrane-in-the-middle setup at room temperature. Phys. Rev. A 2013, 88, 013804. [Google Scholar] [CrossRef]
- Xiong, H.; Wu, Y. Fundamentals and applications of optomechanically induced transparency. Appl. Phys. Rev. 2018, 5, 031305. [Google Scholar] [CrossRef]
- Chen, B.; Jiang, C.; Zhu, K.D. Slow light in a cavity optomechanical system with a Bose–Einstein condensate. Phys. Rev. A 2011, 83, 055803. [Google Scholar] [CrossRef]
- Akram, M.J.; Khan, M.M.; Saif, F. Tunable fast and slow light in a hybrid optomechanical system. Phys. Rev. A 2015, 92, 023846. [Google Scholar] [CrossRef]
- Lü, H.; Wang, C.; Yang, L.; Jing, H. Optomechanically induced transparency at exceptional points. Phys. Rev. Appl. 2018, 10, 014006. [Google Scholar] [CrossRef]
- Jiang, C.; Cui, Y.; Zhai, Z.; Yu, H.; Li, X.; Chen, G. Phase-controlled amplification and slow light in a hybrid optomechanical system. Opt. Express 2019, 27, 30473–30485. [Google Scholar] [CrossRef]
- Xu, Y.; Liu, W. Fano resonance and slow-to-fast light conversion in a Laguerre–Gaussian rovibrational cavity. J. Lightwave Technol. 2022, 41, 2246–2251. [Google Scholar] [CrossRef]
- Xiong, H.; Si, L.G.; Zheng, A.S.; Yang, X.X.; Wu, Y. Higher-order sidebands in optomechanically induced transparency. Phys. Rev. A 2012, 86, 013815. [Google Scholar] [CrossRef]
- Kronwald, A.; Marquardt, F. Optomechanically induced transparency in the nonlinear quantum regime. Phys. Rev. Lett. 2013, 111, 133601. [Google Scholar] [CrossRef] [PubMed]
- Jiao, Y.F.; Lu, T.X.; Jing, H. Optomechanical second-order sidebands and group delays in a Kerr resonator. Phys. Rev. A 2018, 97, 013843. [Google Scholar] [CrossRef]
- Wang, B.; Liu, Z.X.; Xiong, H.; Wu, Y. Highly sensitive mass sensing by means of the optomechanical nonlinearity. IEEE Photonics J. 2018, 10, 6803908. [Google Scholar] [CrossRef]
- Kong, C.; Xiong, H.; Wu, Y. Coulomb-interaction-dependent effect of high-order sideband generation in an optomechanical system. Phys. Rev. A 2017, 95, 033820. [Google Scholar] [CrossRef]
- Xiong, H.; Si, L.G.; Wu, Y. Precision measurement of electrical charges in an optomechanical system beyond linearized dynamics. Appl. Phys. Lett. 2017, 110, 171102. [Google Scholar] [CrossRef]
- Chen, B.; Shang, L.; Wang, X.F.; Chen, J.B.; Xue, H.B.; Liu, X.; Zhang, J. Atom-assisted second-order sideband generation in an optomechanical system with atom-cavity-resonator coupling. Phys. Rev. A 2019, 99, 063810. [Google Scholar] [CrossRef]
- Catalini, L.; Rossi, M.; Langman, E.C.; Schliesser, A. Modeling and observation of nonlinear damping in dissipation-diluted nanomechanical resonators. Phys. Rev. Lett. 2021, 126, 174101. [Google Scholar] [CrossRef]
- Lü, X.Y.; Liao, J.Q.; Tian, L.; Nori, F. Steady-state mechanical squeezing in an optomechanical system via Duffing nonlinearity. Phys. Rev. A 2015, 91, 013834. [Google Scholar] [CrossRef]
- Huang, P.; Zhou, J.; Zhang, L.; Hou, D.; Lin, S.; Deng, W.; Meng, C.; Duan, C.; Ju, C.; Zheng, X.; et al. Generating giant and tunable nonlinearity in a macroscopic mechanical resonator from a single chemical bond. Nat. Commun. 2016, 7, 11517. [Google Scholar] [CrossRef]
- Kaajakari, V.; Mattila, T.; Oja, A.; Seppa, H. Nonlinear limits for single-crystal silicon microresonators. J. Microelectromech. Syst. 2004, 13, 715–724. [Google Scholar] [CrossRef]
- Jacobs, K.; Landahl, A.J. Engineering giant nonlinearities in quantum nanosystems. Phys. Rev. Lett. 2009, 103, 067201. [Google Scholar] [CrossRef] [PubMed]
- Grimm, M.; Bruder, C.; Lörch, N. Optomechanical self-oscillations in an anharmonic potential: Engineering a nonclassical steady state. J. Opt. 2016, 18, 094004. [Google Scholar] [CrossRef]
- Djorwé, P.; Engo, S.N.; Woafo, P. Robustness of continuous-variable entanglement via geometrical nonlinearity. Phys. Rev. A 2014, 90, 024303. [Google Scholar] [CrossRef]
- Hao, H.; Huang, S.; Chen, A. Normal mode splitting in a cavity optomechanical system with a cubic anharmonic oscillator. Int. J. Theor. Phys. 2021, 60, 2766–2777. [Google Scholar] [CrossRef]
- Lv, W.; Deng, L.; Huang, S.; Chen, A. Optomechanically induced transparency in optomechanical system with a cubic anharmonic oscillator. Photonics 2023, 10, 407. [Google Scholar] [CrossRef]
- Saiko, A.P.; Fedaruk, R.; Markevich, S. Kerr-like nonlinearities in an optomechanical system with an asymmetric anharmonic mechanical resonator. JETP Lett. 2021, 113, 487–492. [Google Scholar] [CrossRef]
- Huang, S.; Wu, Y.; Chen, A. The stationary optomechanical entanglement between an optical cavity field and a cubic anharmonic oscillator. Int. J. Theor. Phys. 2021, 60, 3961–3972. [Google Scholar] [CrossRef]
- Latmiral, L.; Armata, F.; Genoni, M.G.; Pikovski, I.; Kim, M. Probing anharmonicity of a quantum oscillator in an optomechanical cavity. Phys. Rev. A 2016, 93, 052306. [Google Scholar] [CrossRef]
- Yaris, R.; Bendler, J.; Lovett, R.A.; Bender, C.M.; Fedders, P.A. Resonance calculations for arbitrary potentials. Phys. Rev. A 1978, 18, 1816. [Google Scholar] [CrossRef]
- Alvarez, G. Coupling-constant behavior of the resonances of the cubic anharmonic oscillator. Phys. Rev. A 1988, 37, 4079. [Google Scholar] [CrossRef] [PubMed]
- Cveticanin, L.; Zukovic, M.; Mester, G.; Biro, I.; Sarosi, J. Oscillators with symmetric and asymmetric quadratic nonlinearity. Acta Mech. 2016, 227, 1727–1742. [Google Scholar] [CrossRef]
- Bender, C.M.; Dunne, G.V. Large-order perturbation theory for a non-Hermitian PT-symmetric Hamiltonian. J. Math. Phys. 1998, 40, 4616–4621. [Google Scholar] [CrossRef]
- Bender, C.M.; Weniger, E.J. Numerical evidence that the perturbation expansion for a non-Hermitian PT-symmetric Hamiltonian is Stieltjes. J. Math. Phys. 2001, 42, 2167–2183. [Google Scholar] [CrossRef]
- Mera, H.; Pedersen, T.G.; Nikolić, B.K. Nonperturbative quantum physics from low-order perturbation theory. Phys. Rev. Lett. 2015, 115, 143001. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Q.; He, Y.; Yang, Y.; Zhang, H.; Xu, Y. Second-Order Sidebands and Group Delays in Coupled Optomechanical Cavity System with a Cubic Nonlinear Harmonic Oscillator. Photonics 2024, 11, 256. https://doi.org/10.3390/photonics11030256
Zhao Q, He Y, Yang Y, Zhang H, Xu Y. Second-Order Sidebands and Group Delays in Coupled Optomechanical Cavity System with a Cubic Nonlinear Harmonic Oscillator. Photonics. 2024; 11(3):256. https://doi.org/10.3390/photonics11030256
Chicago/Turabian StyleZhao, Qiwen, Ying He, Yanfang Yang, Huifang Zhang, and Yi Xu. 2024. "Second-Order Sidebands and Group Delays in Coupled Optomechanical Cavity System with a Cubic Nonlinear Harmonic Oscillator" Photonics 11, no. 3: 256. https://doi.org/10.3390/photonics11030256




