The Generation of Circularly Polarized Isolated Attosecond Pulses with Tunable Helicity from CO Molecules in Polarization Gating Laser Fields
Abstract
1. Introduction
2. Theoretical Model
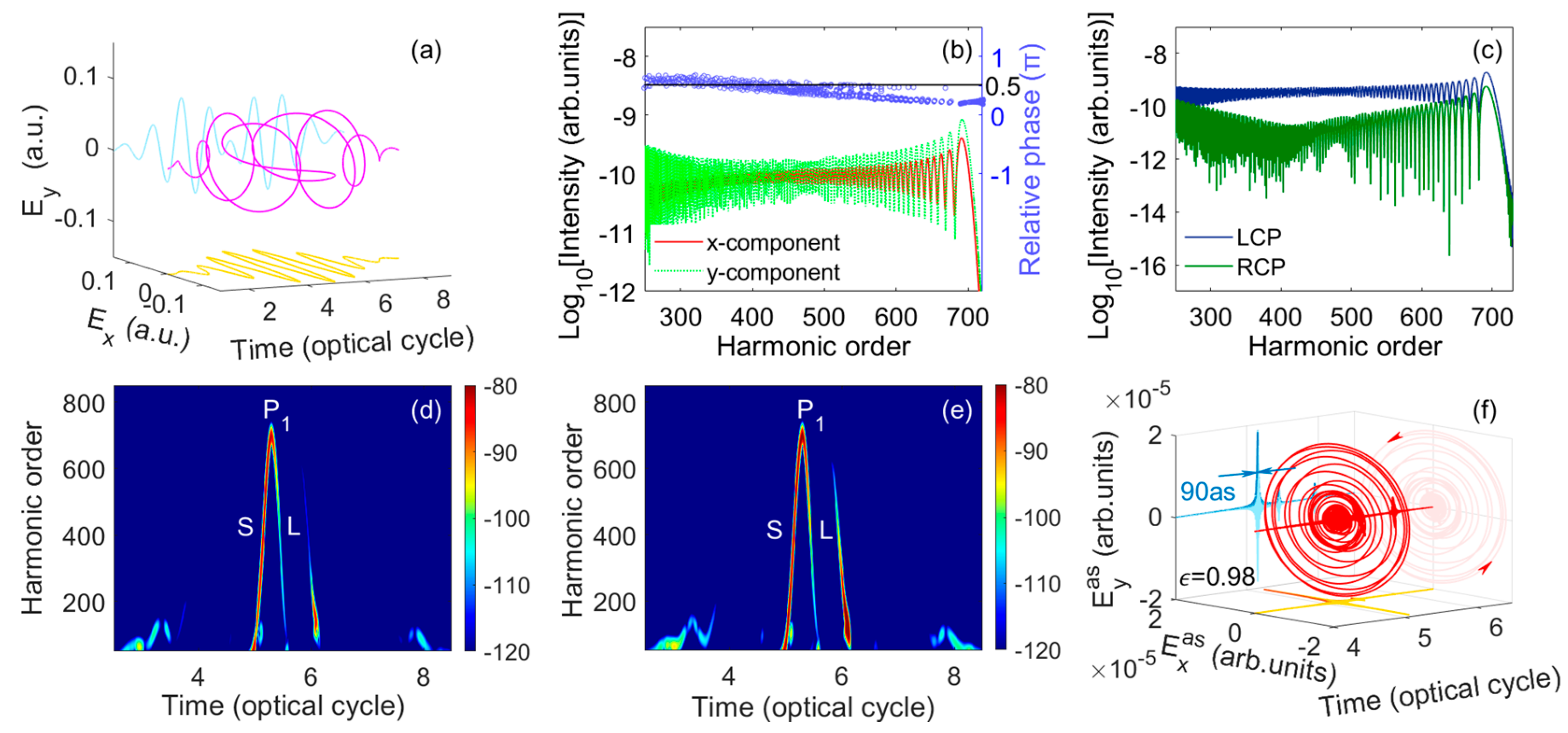
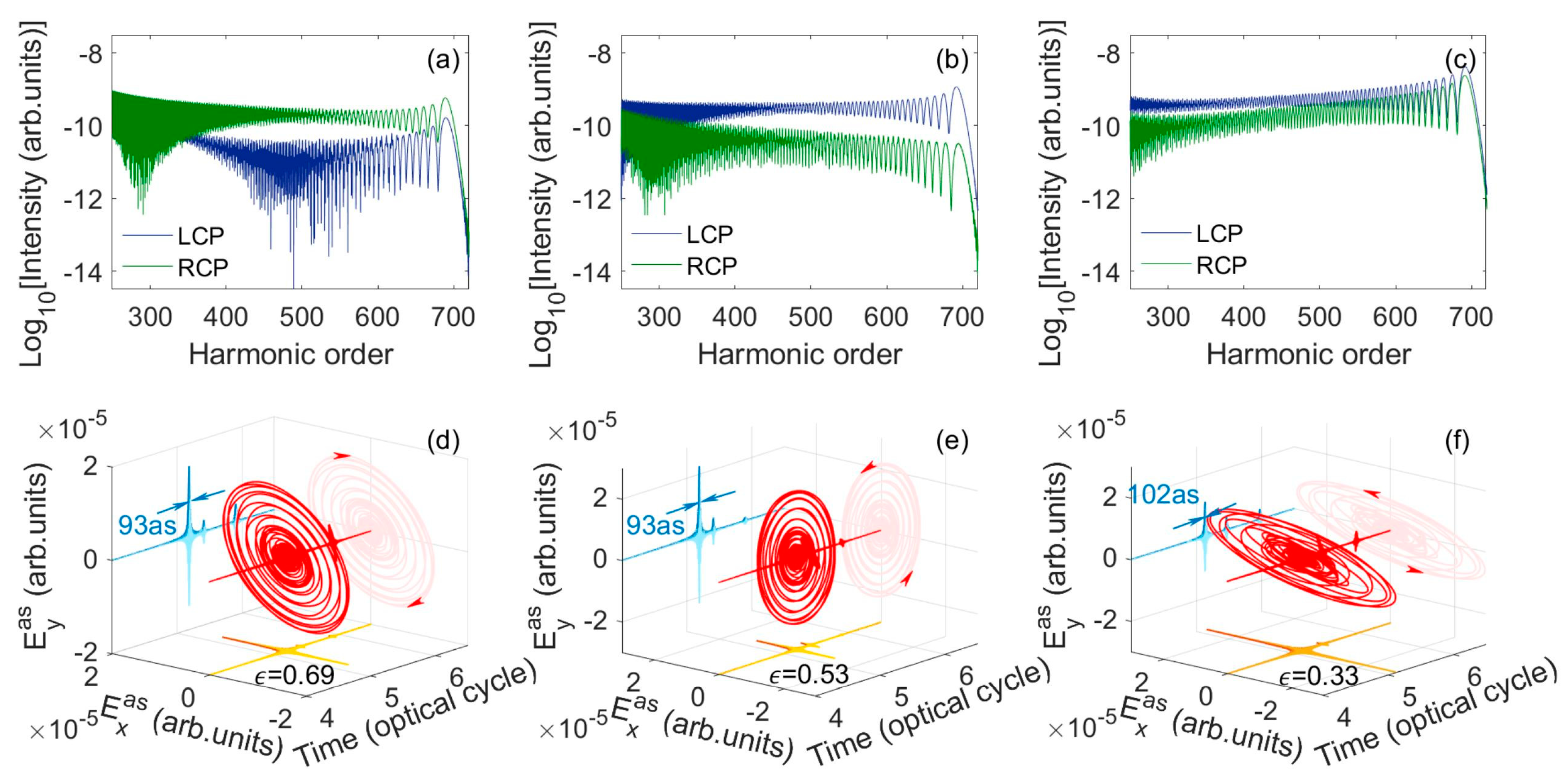
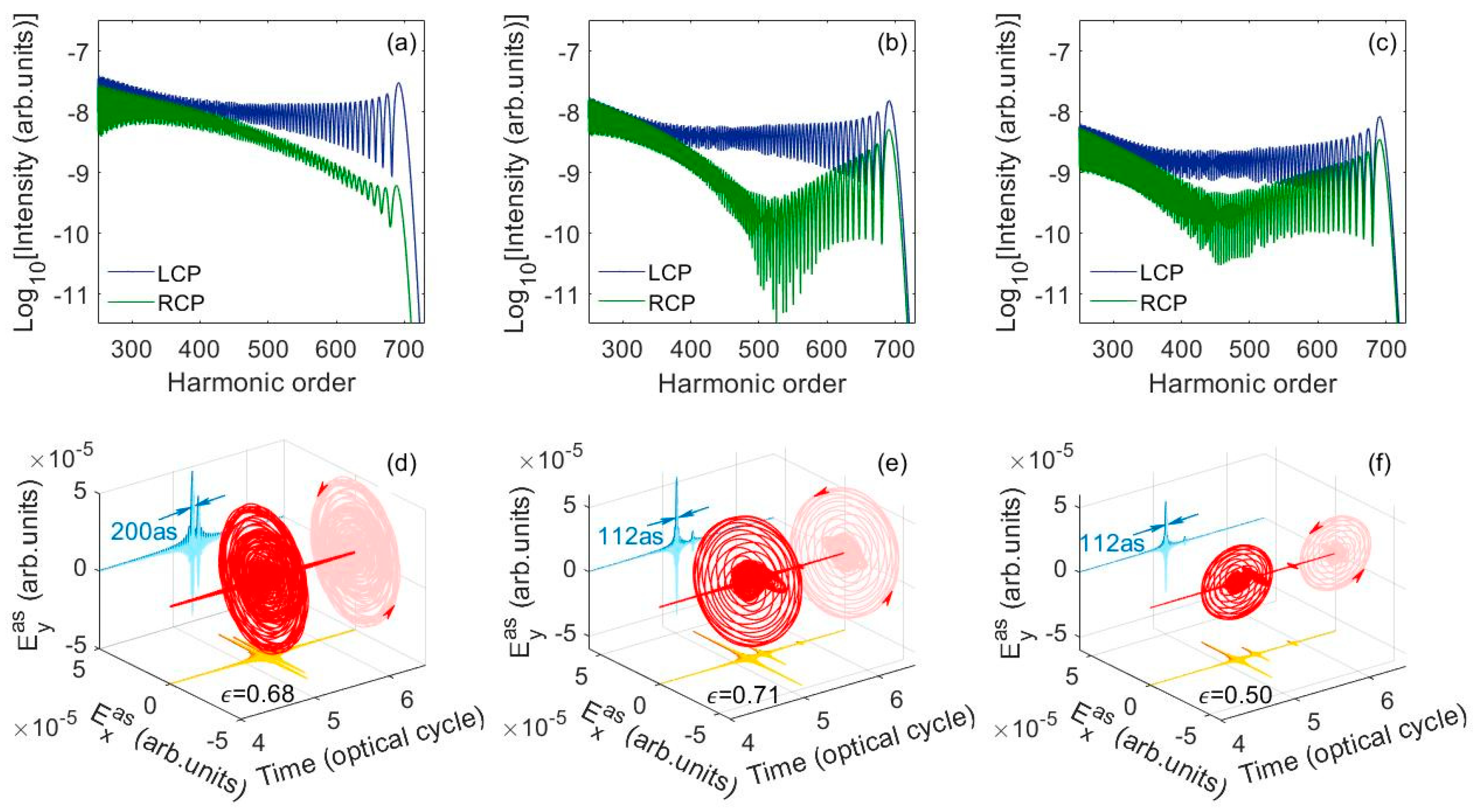
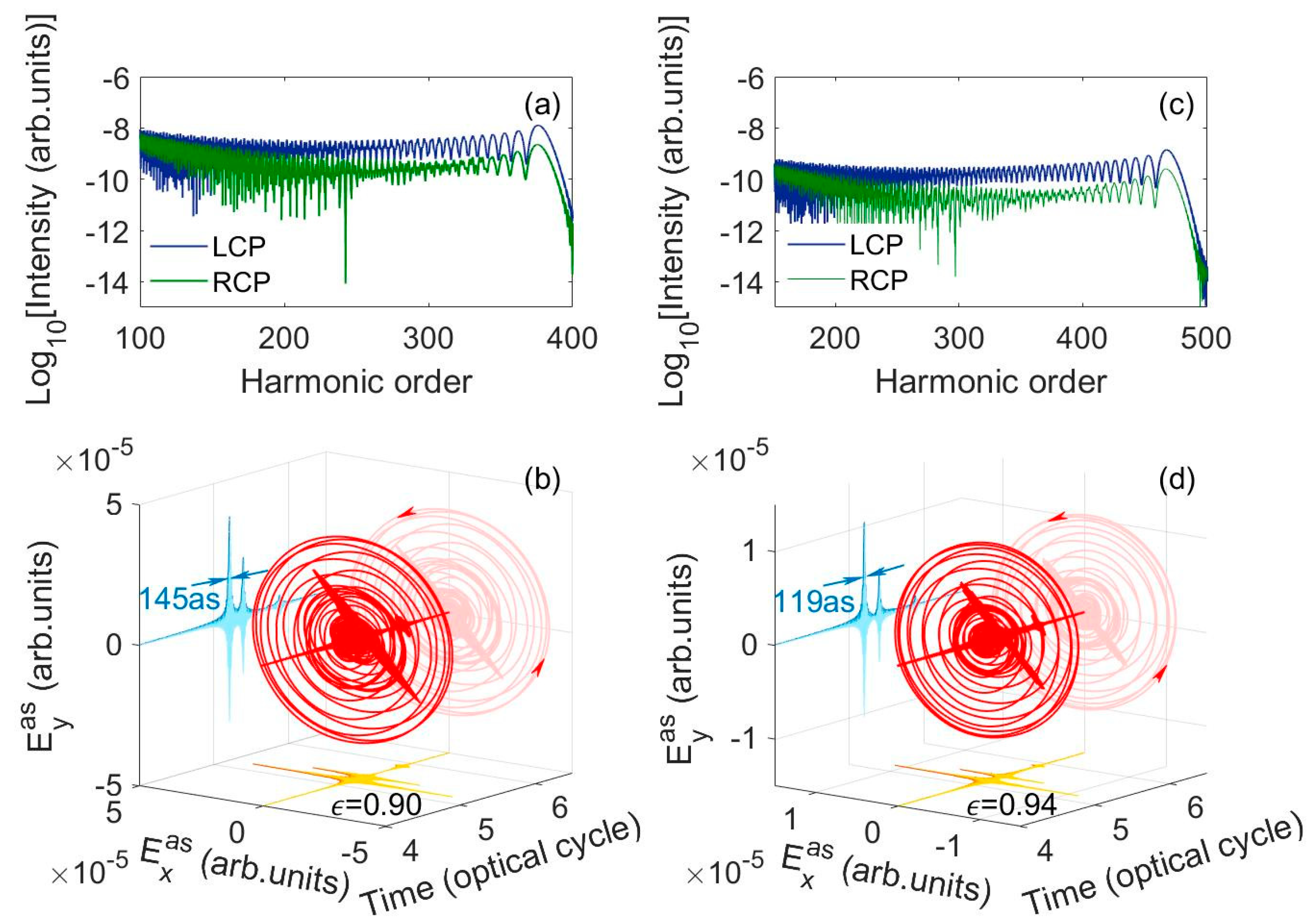
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Corkum, P.B.; Krausz, F. Attosecond science. Nat. Phys. 2007, 3, 381–387. [Google Scholar] [CrossRef]
- Chatziathanasiou, S.; Kahaly, S.; Skantzakis, E.; Sansone, G.; Lopez-Martens, R.; Haessler, S.; Varju, K.; Tsakiris, G.; Charalambidis, D.; Tzallas, P. Generation of Attosecond Light Pulses from Gas and Solid State Media. Photonics 2017, 4, 26. [Google Scholar] [CrossRef]
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994–1997. [Google Scholar] [CrossRef] [PubMed]
- Bartels, R.A.; Paul, A.; Green, H.; Kapteyn, H.C.; Murnane, M.M.; Backus, S.; Christov, I.P.; Liu, Y.; Attwood, D.; Jacobsen, C. Generation of Spatially Coherent Light at Extreme Ultraviolet Wavelengths. Science 2002, 297, 376–378. [Google Scholar] [CrossRef] [PubMed]
- Popmintchev, T.; Chen, M.-C.; Arpin, P.; Murnane, M.M.; Kapteyn, H.C. The attosecond nonlinear optics of bright coherent X-ray generation. Nat. Photonics 2010, 4, 822–832. [Google Scholar] [CrossRef]
- Itatani, J.; Quéré, F.; Yudin, G.L.; Ivanov, M.Y.; Krausz, F.; Corkum, P.B. Attosecond Streak Camera. Phys. Rev. Lett. 2002, 88, 173903. [Google Scholar] [CrossRef] [PubMed]
- Greening, D.; Weaver, B.; Pettipher, A.J.; Walke, D.J.; Larsen, E.W.; Marangos, J.P.; Tisch, J.W.G. Generation and measurement of isolated attosecond pulses with enhanced flux using a two colour synthesized laser field. Opt. Express 2020, 28, 23329. [Google Scholar] [CrossRef] [PubMed]
- Kienberger, R.; Goulielmakis, E.; Uiberacker, M.; Baltuska, A.; Yakovlev, V.; Bammer, F.; Scrinzi, A.; Westerwalbesloh, T.; Kleineberg, U.; Heinzmann, U.; et al. Atomic transient recorder. Nature 2004, 427, 817–821. [Google Scholar] [CrossRef]
- Goulielmakis, E.; Schultze, M.; Hofstetter, M.; Yakovlev, V.S.; Gagnon, J.; Uiberacker, M.; Aquila, A.L.; Gullikson, E.M.; Attwood, D.T.; Kienberger, R.; et al. Single-Cycle Nonlinear Optics. Science 2008, 320, 1614–1617. [Google Scholar] [CrossRef]
- Pfeifer, T.; Gallmann, L.; Abel, M.J.; Nagel, P.M.; Neumark, D.M.; Leone, S.R. Heterodyne mixing of laser fields for temporal gating of high-order harmonic generation. Phys. Rev. Lett. 2006, 97, 163901. [Google Scholar] [CrossRef]
- Lan, P.; Takahashi, E.J.; Midorikawa, K. Optimization of infrared two-color multicycle field synthesis for intense-isolated-attosecond-pulse generation. Phys. Rev. A 2010, 82, 053413. [Google Scholar] [CrossRef]
- Haessler, S.; Balčiūnas, T.; Fan, G.; Chipperfield, L.E.; Baltuška, A. Enhanced multi-colour gating for the generation of high-power isolated attosecond pulses. Sci. Rep. 2015, 5, 10084. [Google Scholar] [CrossRef] [PubMed]
- Yuan, H.; He, L.; Wang, F.; Wang, B.; Liu, W.; Hong, Z. Generation of isolated attosecond pulses in a multi-cycle inhomogeneous two-color field without CEP stabilization. Opt. Quantum Electron. 2017, 49, 214. [Google Scholar] [CrossRef]
- Xue, B.; Tamaru, Y.; Fu, Y.; Yuan, H.; Lan, P.; Mücke, O.D.; Suda, A.; Midorikawa, K.; Takahashi, E.J. Fully stabilized multi-TW optical waveform synthesizer: Toward gigawatt isolated attosecond pulses. Sci. Adv. 2020, 6, eaay2802. [Google Scholar] [CrossRef] [PubMed]
- Chang, Z. Chirp of the single attosecond pulse generated by a polarization gating. Phys. Rev. A 2005, 71, 023813. [Google Scholar] [CrossRef]
- Sansone, G.; Benedetti, E.; Calegari, F.; Vozzi, C.; Avaldi, L.; Flammini, R.; Poletto, L.; Villoresi, P.; Altucci, C.; Velotta, R.; et al. Isolated single-cycle attosecond pulses. Science 2006, 314, 443–446. [Google Scholar] [CrossRef]
- Sola, I.J.; Mével, E.; Elouga, L.; Constant, E.; Strelkov, V.; Poletto, L.; Villoresi, P.; Benedetti, E.; Caumes, J.P.; Stagira, S.; et al. Controlling attosecond electron dynamics by phase-stabilized polarization gating. Nat. Phys. 2006, 2, 319–322. [Google Scholar] [CrossRef]
- Zhang, Q.; Lu, P.; Lan, P.; Hong, W.; Yang, Z. Multi-cycle laser-driven broadband supercontinuum with a modulated polarization gating. Opt. Express 2008, 16, 9795–9803. [Google Scholar] [CrossRef] [PubMed]
- Gaumnitz, T.; Jain, A.; Pertot, Y.; Huppert, M.; Jordan, I.; Ardana-Lamas, F.; Wörner, H.J. Streaking of 43-attosecond soft-X-ray pulses generated by a passively CEP-stable mid-infrared driver. Opt. Express 2017, 25, 27506. [Google Scholar] [CrossRef]
- Li, J.; Ren, X.; Yin, Y.; Zhao, K.; Chew, A.; Cheng, Y.; Cunningham, E.; Wang, Y.; Hu, S.; Wu, Y.; et al. 53-attosecond X-ray pulses reach the carbon K-edge. Nat. Commun. 2017, 8, 186. [Google Scholar] [CrossRef]
- Böwering, N.; Lischke, T.; Schmidtke, B.; Müller, N.; Khalil, T.; Heinzmann, U. Asymmetry in Photoelectron Emission from Chiral Molecules Induced by Circularly Polarized Light. Phys. Rev. Lett. 2001, 86, 1187–1190. [Google Scholar] [CrossRef] [PubMed]
- López-Flores, V.; Arabski, J.; Stamm, C.; Halté, V.; Pontius, N.; Beaurepaire, E.; Boeglin, C. Time-resolved x-ray magnetic circular dichroism study of ultrafast demagnetization in a CoPd ferromagnetic film excited by circularly polarized laser pulse. Phys. Rev. B 2012, 86, 014424. [Google Scholar] [CrossRef]
- Siegrist, F.; Gessner, J.A.; Ossiander, M.; Denker, C.; Chang, Y.-P.; Schröder, M.C.; Guggenmos, A.; Cui, Y.; Walowski, J.; Martens, U.; et al. Light-wave dynamic control of magnetism. Nature 2019, 571, 240–244. [Google Scholar] [CrossRef] [PubMed]
- Baykusheva, D.; Wörner, H.J. Chiral Discrimination through Bielliptical High-Harmonic Spectroscopy. Phys. Rev. X 2018, 8, 031060. [Google Scholar] [CrossRef]
- Ferré, A.; Handschin, C.; Dumergue, M.; Burgy, F.; Comby, A.; Descamps, D.; Fabre, B.; Garcia, G.A.; Géneaux, R.; Merceron, L.; et al. A table-top ultrashort light source in the extreme ultraviolet for circular dichroism experiments. Nat. Photonics 2014, 9, 93–98. [Google Scholar] [CrossRef]
- Vodungbo, B.; Barszczak Sardinha, A.; Gautier, J.; Lambert, G.; Valentin, C.; Lozano, M.; Iaquaniello, G.; Delmotte, F.; Sebban, S.; Lüning, J.; et al. Polarization control of high order harmonics in the EUV photon energy range. Opt. Express 2011, 19, 4346–4356. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, S.; Huo, X.-X.; Xing, Y.-H.; Wang, F.; Liu, X.-S. Generation of ellipticity-tunable isolated attosecond pulses from diatomic molecules in intense laser fields. Opt. Commun. 2023, 530, 129152. [Google Scholar] [CrossRef]
- Sun, F.J.; Chen, C.; Li, W.Y.; Liu, X.; Li, W.; Chen, Y.J. High ellipticity of harmonics from molecules in strong laser fields of small ellipticity. Phys. Rev. A 2021, 103, 053108. [Google Scholar] [CrossRef]
- Fleischer, A.; Kfir, O.; Diskin, T.; Sidorenko, P.; Cohen, O. Spin angular momentum and tunable polarization in high-harmonic generation. Nat. Photonics 2014, 8, 543–549. [Google Scholar] [CrossRef]
- Kfir, O.; Grychtol, P.; Turgut, E.; Knut, R.; Zusin, D.; Popmintchev, D.; Popmintchev, T.; Nembach, H.; Shaw, J.M.; Fleischer, A.; et al. Generation of bright phase-matched circularly-polarized extreme ultraviolet high harmonics. Nat. Photonics 2014, 9, 99–105. [Google Scholar] [CrossRef]
- Fan, T.; Grychtol, P.; Knut, R.; Hernández-García, C.; Hickstein, D.D.; Zusin, D.; Gentry, C.; Dollar, F.J.; Mancuso, C.A.; Hogle, C.W.; et al. Bright circularly polarized soft X-ray high harmonics for X-ray magnetic circular dichroism. Proc. Natl. Acad. Sci. USA 2015, 112, 14206–14211. [Google Scholar] [CrossRef] [PubMed]
- Dorney, K.M.; Ellis, J.L.; Hernández-García, C.; Hickstein, D.D.; Mancuso, C.A.; Brooks, N.; Fan, T.; Fan, G.; Zusin, D.; Gentry, C.; et al. Helicity-Selective Enhancement and Polarization Control of Attosecond High Harmonic Waveforms Driven by Bichromatic Circularly Polarized Laser Fields. Phys. Rev. Lett. 2017, 119, 063201. [Google Scholar] [CrossRef] [PubMed]
- Jiménez-Galán, Á.; Zhavoronkov, N.; Ayuso, D.; Morales, F.; Patchkovskii, S.; Schloz, M.; Pisanty, E.; Smirnova, O.; Ivanov, M. Control of attosecond light polarization in two-color bicircular fields. Phys. Rev. A 2018, 97, 023409. [Google Scholar] [CrossRef]
- Huang, P.-C.; Hernández-García, C.; Huang, J.-T.; Huang, P.-Y.; Lu, C.-H.; Rego, L.; Hickstein, D.D.; Ellis, J.L.; Jaron-Becker, A.; Becker, A.; et al. Polarization control of isolated high-harmonic pulses. Nat. Photonics 2018, 12, 349–354. [Google Scholar] [CrossRef]
- Fang, Y.; Liu, Y. Optimal control over high-order-harmonic ellipticity in two-color cross-linearly-polarized laser fields. Phys. Rev. A 2021, 103, 033116. [Google Scholar] [CrossRef]
- Zhou, X.; Lock, R.; Wagner, N.; Li, W.; Kapteyn, H.C.; Murnane, M.M. Elliptically Polarized High-Order Harmonic Emission from Molecules in Linearly Polarized Laser Fields. Phys. Rev. Lett. 2009, 102, 073902. [Google Scholar] [CrossRef]
- Le, A.-T.; Lucchese, R.R.; Lin, C.D. Polarization and ellipticity of high-order harmonics from aligned molecules generated by linearly polarized intense laser pulses. Phys. Rev. A 2010, 82, 023814. [Google Scholar] [CrossRef]
- Zhai, C.; Wu, Y.; Liu, Y.; Zhang, K.; Kang, S.; Li, Z.; Wu, F.; Dong, X.; Cheng, X.; Li, Y.; et al. Near-circularly polarized isolated attosecond pulse generation from gas mixture with two-color multicycle laser fields. Results Phys. 2024, 58, 107518. [Google Scholar] [CrossRef]
- Van der Zwan, E.V.; Lein, M. Two-center interference and ellipticity in high-order harmonic generation from . Phys. Rev. A 2010, 82, 033405. [Google Scholar] [CrossRef]
- Strelkov, V.V.; Gonoskov, A.A.; Gonoskov, I.A.; Ryabikin, M.Y. Origin for ellipticity of high-order harmonics generated in atomic gases and the sublaser-cycle evolution of harmonic polarization. Phys. Rev. Lett. 2011, 107, 043902. [Google Scholar] [CrossRef]
- Lewenstein, M.; Balcou, P.; Ivanov, M.Y.; L’huillier, A.; Corkum, P.B. Theory of high-harmonic generation by low-frequency laser fields. Phys. Rev. A 1994, 49, 2117. [Google Scholar] [CrossRef]
- Tong, X.M.; Zhao, Z.X.; Lin, C.-D. Theory of molecular tunneling ionization. Phys. Rev. A 2002, 66, 033402. [Google Scholar] [CrossRef]
- Huang, Y.; Xie, T.; Li, J.; Yu, J.; Cong, S.-L. Efficiently field-free orientation of CO molecules by a femtosecond pulse and a THz pulse train. Laser Phys. 2014, 24, 016002. [Google Scholar] [CrossRef]
- Dang, H.-P.; Wang, S.; Zhan, W.; Han, X.-F.; Zai, J.-B. Field-free molecular orientation by two-color femtosecond laser pulse and time-delayed THz laser pulse. Laser Phys. 2015, 25, 075301. [Google Scholar] [CrossRef]
- Liao, S.-L.; Ho, T.-S.; Rabitz, H.; Chu, S.-I. Maximum attainable field-free molecular orientation of a thermal ensemble with near—Single-cycle THz pulses. Phys. Rev. A 2013, 87, 013429. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, S.; Yuan, H.; Wang, F.; Song, J.; Zhao, Y.; Yang, C.; Ou, T.; Zhang, R.; Chang, Q.; Sun, Y. The Generation of Circularly Polarized Isolated Attosecond Pulses with Tunable Helicity from CO Molecules in Polarization Gating Laser Fields. Photonics 2024, 11, 464. https://doi.org/10.3390/photonics11050464
Chen S, Yuan H, Wang F, Song J, Zhao Y, Yang C, Ou T, Zhang R, Chang Q, Sun Y. The Generation of Circularly Polarized Isolated Attosecond Pulses with Tunable Helicity from CO Molecules in Polarization Gating Laser Fields. Photonics. 2024; 11(5):464. https://doi.org/10.3390/photonics11050464
Chicago/Turabian StyleChen, Shiju, Hua Yuan, Feng Wang, Jiahang Song, Yue Zhao, Chunhui Yang, Tianxin Ou, Ru Zhang, Qiang Chang, and Yuping Sun. 2024. "The Generation of Circularly Polarized Isolated Attosecond Pulses with Tunable Helicity from CO Molecules in Polarization Gating Laser Fields" Photonics 11, no. 5: 464. https://doi.org/10.3390/photonics11050464
APA StyleChen, S., Yuan, H., Wang, F., Song, J., Zhao, Y., Yang, C., Ou, T., Zhang, R., Chang, Q., & Sun, Y. (2024). The Generation of Circularly Polarized Isolated Attosecond Pulses with Tunable Helicity from CO Molecules in Polarization Gating Laser Fields. Photonics, 11(5), 464. https://doi.org/10.3390/photonics11050464





