How Free Swimming Fosters the Locomotion of a Purely Oscillating Fish-like Body
Abstract
:1. Introduction
2. Materials and Methods
2.1. Mathematical Model
2.2. Numerical Method and Fish Kinematics
2.3. Prescribed Deformation
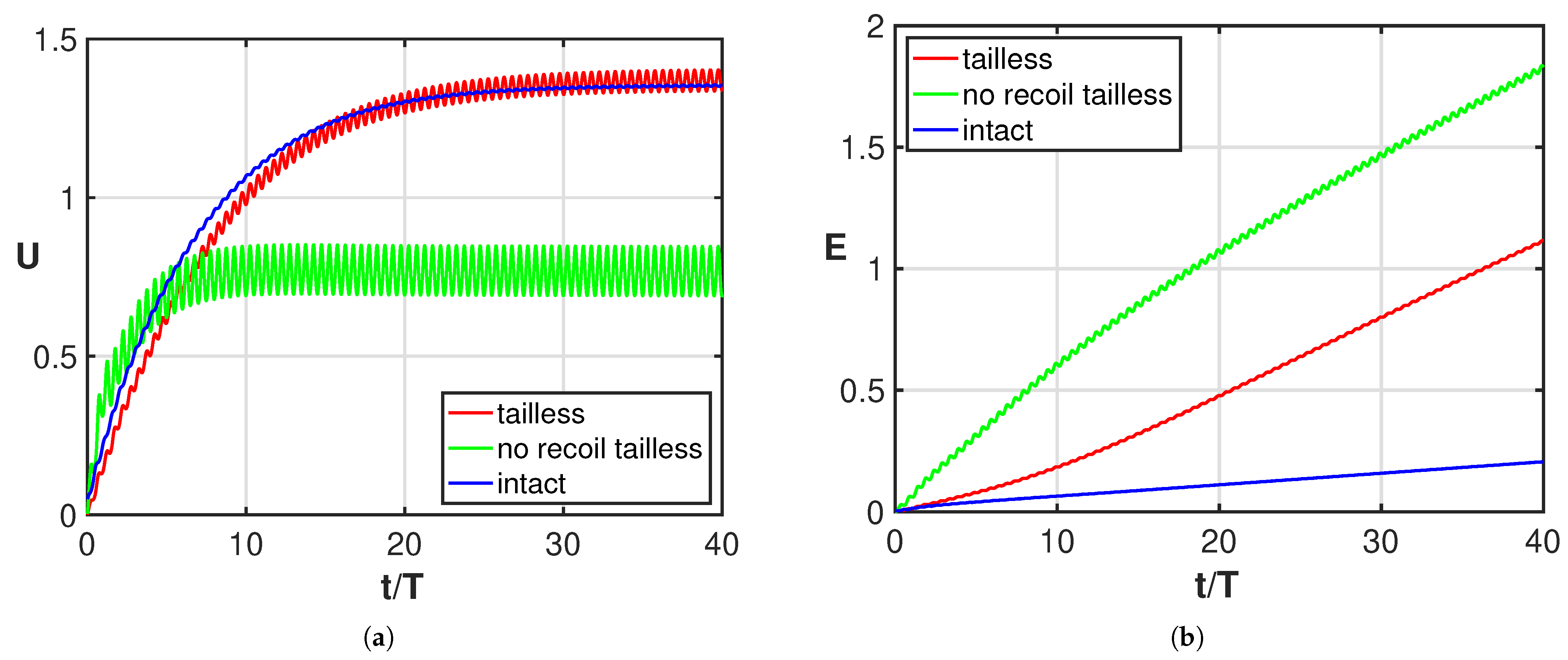
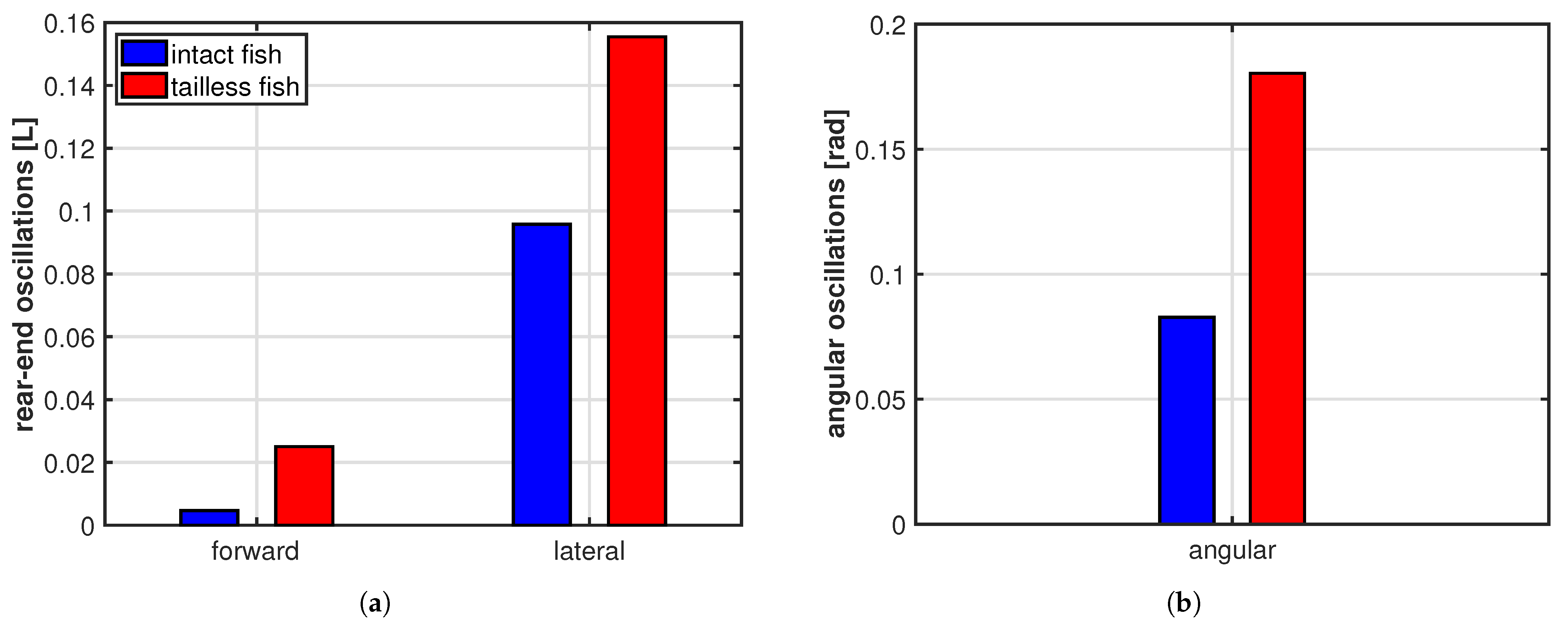
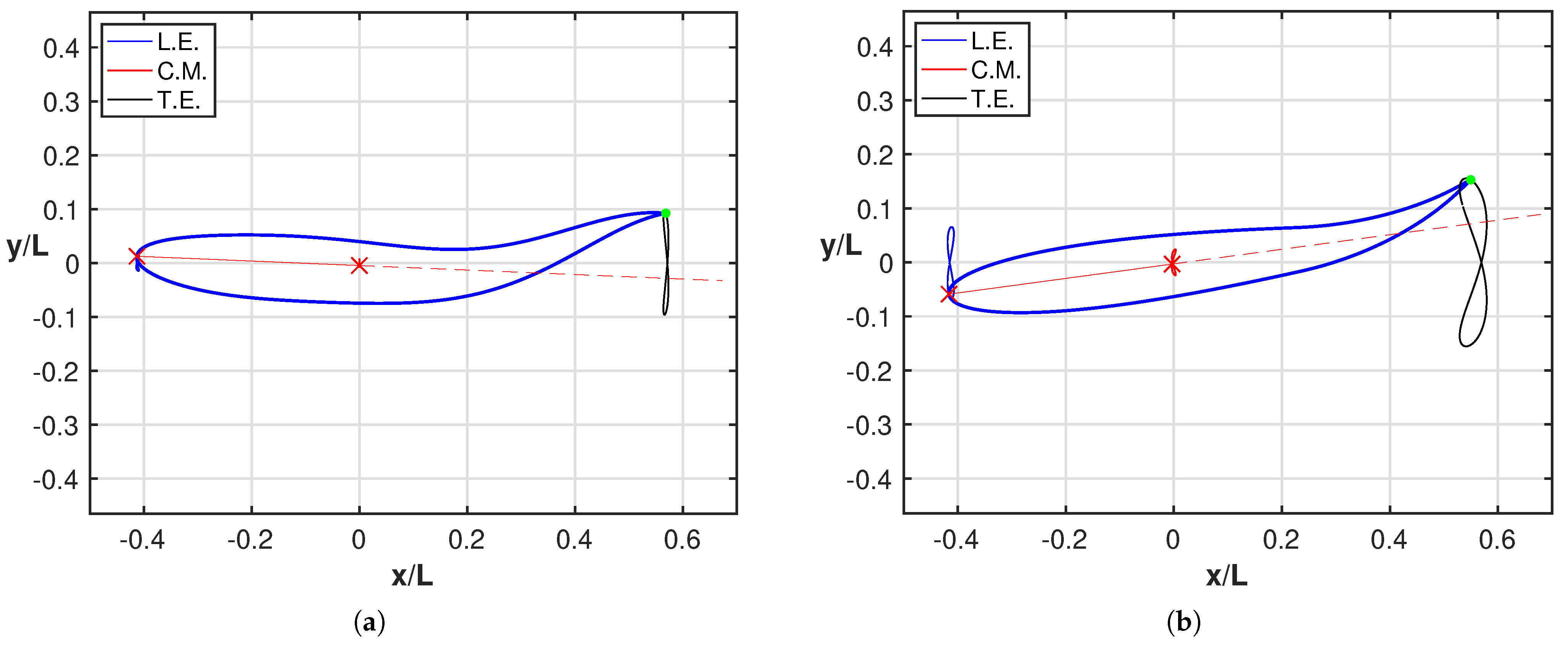
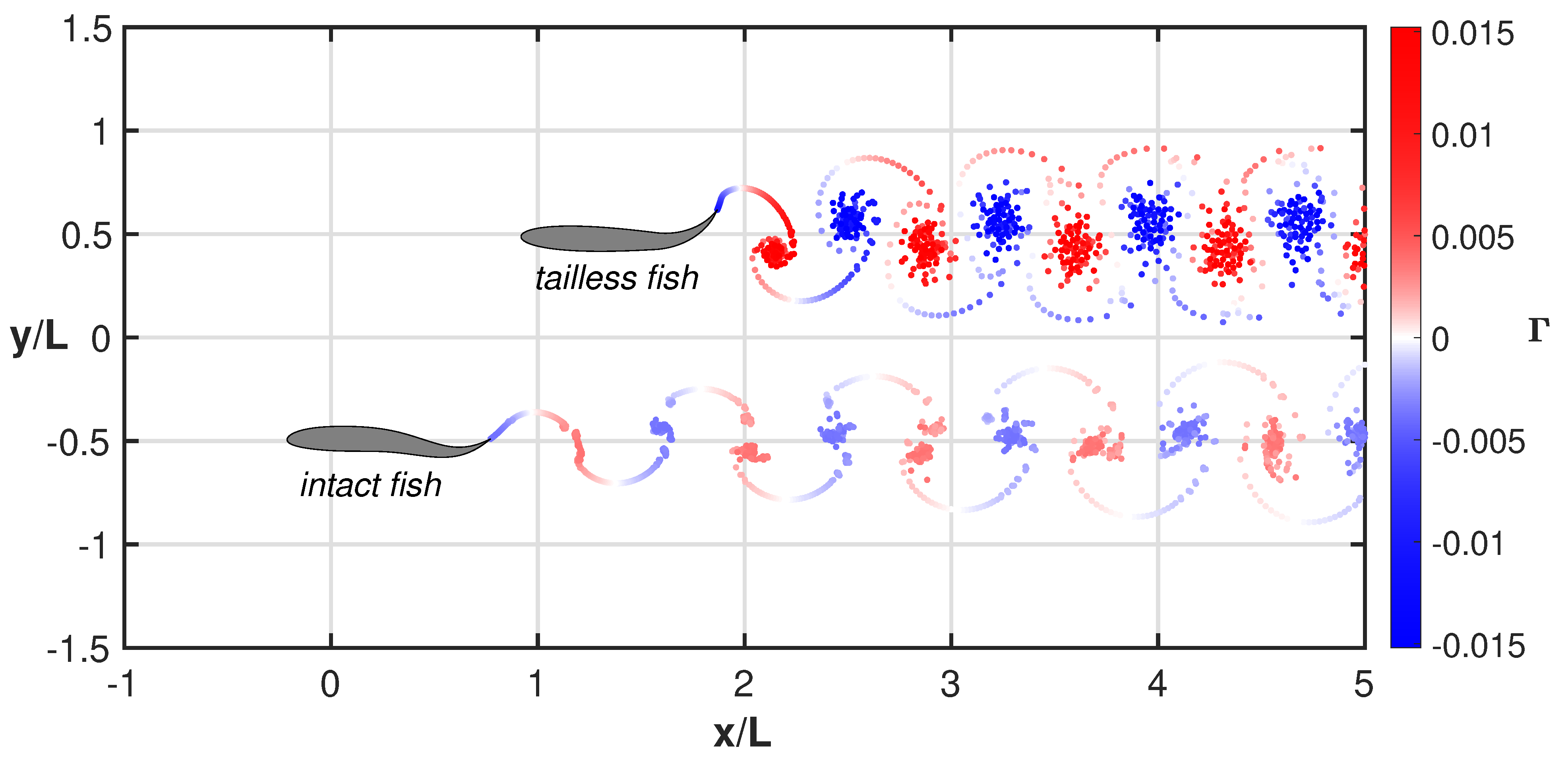
3. Results
4. Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1. Flapping–Undulation Analogy
Appendix A.2. Appendix Figures



References
- Breder, C. The locomotion of fishes. Zoologica 1926, 4, 159–295. [Google Scholar] [CrossRef]
- Gray, J. Studies in Animal Locomotion I. J. Exp. Biol. 1933, 10, 88–104. [Google Scholar] [CrossRef]
- Gray, J. Studies in Animal Locomotion II. J. Exp. Biol. 1933, 10, 386–390. [Google Scholar] [CrossRef]
- Gray, J. Studies in Animal Locomotion III. J. Exp. Biol. 1933, 10, 391–400. [Google Scholar] [CrossRef]
- Webb, P.W. Effects of Partial Caudal-Fin Amputation on the Kinematics and Metabolic Rate of Underyearling Sockeye Salmon (Oncorhynchus nerka) at Steady Swimming Speeds. J. Exp. Biol. 1973, 59, 565–582. [Google Scholar] [CrossRef]
- Plaut, I. Effects of fin size on swimming performance, swimming behaviour and routine activity of zebrafish Danio rerio. J. Exp. Biol. 2000, 203, 813–820. [Google Scholar] [CrossRef] [PubMed]
- Fu, C.; Cao, Z.D.; Fu, S.J. The effects of caudal fin loss and regeneration on the swimming performance of three cyprinid fish species with different swimming capacities. J. Exp. Biol. 2013, 216, 3164–3174. [Google Scholar] [CrossRef] [PubMed]
- Deakin, A.G.; Spencer, J.W.; Cossins, A.R.; Young, I.S.; Sneddon, L.U. Welfare Challenges Influence the Complexity of Movement: Fractal Analysis of Behaviour in Zebrafish. Fishes 2019, 4, 8. [Google Scholar] [CrossRef]
- Cai, L.; Chen, J.H.; Johnson, D.; Tu, Z.; Huang, Y.P. Effect of tail fin loss on swimming capability and tail beat frequency of juvenile black carp Mylopharyngodon piceus. Aquat. Biol. 2020, 29, 71–77. [Google Scholar] [CrossRef]
- Fish, F.E.; Rybczynski, N.; Lauder, G.V.; Duff, C.M. The Role of the Tail or Lack Thereof in the Evolution of Tetrapod Aquatic Propulsion. Integr. Comp. Biol. 2021, 61, 398–413. [Google Scholar] [CrossRef]
- Audira, G.; Suryanto, M.E.; Chen, K.H.; Vasquez, R.D.; Roldan, M.J.; Yang, C.C.; Hsiao, C.D.; Huang, J.C. Acute and Chronic Effects of Fin Amputation on Behavior Performance of Adult Zebrafish in 3D Locomotion Test Assessed with Fractal Dimension and Entropy Analyses and Their Relationship to Fin Regeneration. Biology 2022, 11, 969. [Google Scholar] [CrossRef]
- Lighthill, J. Note on the swimming of slender fish. J. Fluid Mech. 1960, 9, 305–317. [Google Scholar] [CrossRef]
- Wu, T.Y. Swimming of a waving plate. J. Fluid Mech. 1961, 10, 321–344. [Google Scholar] [CrossRef]
- Paniccia, D.; Padovani, L.; Graziani, G.; Piva, R. Locomotion performance for oscillatory swimming in free mode. Bioinspir. Biomim. 2023, 18, 015004. [Google Scholar] [CrossRef] [PubMed]
- Reid, D.A.P.; Hildenbrandt, H.; Padding, J.T.; Hemelrijk, C.K. Fluid dynamics of moving fish in a two-dimensional multiparticle collision dynamics model. Phys. Rev. E 2012, 85, 021901. [Google Scholar] [CrossRef]
- Paniccia, D.; Graziani, G.; Lugni, C.; Piva, R. The relevance of recoil and free swimming in aquatic locomotion. J. Fluids Struct. 2021, 103, 103290. [Google Scholar] [CrossRef]
- Noca, F. On the Evaluation of Time-Dependent Fluid Dynamic Forces on Bluff Bodies. Ph.D. Thesis, California Institute of Technology, Pasadena, CA, USA, 1997. [Google Scholar]
- Graziani, G.; Bassanini, P. Unsteady Viscous Flows about Bodies: Vorticity Release and Forces. Meccanica 2002, 37, 283–303. [Google Scholar] [CrossRef]
- Wu, J.Z.; Ma, H.Y.; Zhou, M.D. Vortical Flows; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Paniccia, D.; Graziani, G.; Lugni, C.; Piva, R. On the role of added mass and vorticity release for self propelled aquatic locomotion. J. Fluid Mech. 2021, 918, A45. [Google Scholar] [CrossRef]
- Saffman, P.G. The self-propulsion of a deformable body in a perfect fluid. J. Fluid Mech. 1967, 28, 385–389. [Google Scholar] [CrossRef]
- Kanso, E. Swimming due to transverse shape deformations. J. Fluid Mech. 2009, 631, 127–148. [Google Scholar] [CrossRef]
- Eldredge, J.D. A Reconciliation of Viscous and Inviscid Approaches to Computing Locomotion of Deforming Bodies. Exp. Mech. 2010, 50, 1349–1353. [Google Scholar] [CrossRef]
- Lamb, H. Hydrodynamics, 6th ed.; Cambridge University Press: Cambridge, UK, 1975. [Google Scholar]
- Hess, J.L.; Smith, A.M.O. Calculation of potential flow about arbitrary bodies. Prog. Aerosp. Sci. 1967, 8, 1–138. [Google Scholar] [CrossRef]
- Basu, B.C.; Hancock, G.J. The unsteady motion of a two-dimensional aerofoil in incompressible inviscid flow. J. Fluid Mech. 1978, 87, 159–178. [Google Scholar] [CrossRef]
- Hess, F.; Videler, J.J. Fast continuous swimming of Saithe (Pollachius virens): A dynamical analysis of bending moments and muscle power. J. Exp. Biol. 1984, 109, 229–251. [Google Scholar] [CrossRef]
- Videler, J.; Hess, F. Fast continuous swimming of two pelagic predators saithe (Pollachius virens) and mackerel (Scomber scombrius): A kinematic analysis. J. Exp. Biol. 1984, 109, 209–228. [Google Scholar] [CrossRef]
- Maertens, A.; Triantafyllou, M.; Yue, D. Efficiency of fish propulsion. Bioinspir. Biomim. 2015, 10, 046013. [Google Scholar] [CrossRef]
- Jurczyk, K.; Piskur, P.; Szymak, P. Parameters identification of the flexible fin kinematics model using vision and genetic algorithms. Pol. Marit. Res. 2020, 27, 39–47. [Google Scholar] [CrossRef]
- Li, N.; Liu, X.; Su, Y. Numerical study on the hydrodynamics of thunniform bio-inspired swimming under self-propulsion. PLoS ONE 2017, 12, e0174740. [Google Scholar] [CrossRef]
- Lighthill, J. Hydromechanics of aquatic animal propulsion: A survey. Annu. Rev. Fluid Mech. 1969, 1, 413–446. [Google Scholar] [CrossRef]
- Wu, T. Fish Swimming and Bird/Insect Flight. Annu. Rev. Fluid Mech. 2011, 43, 25–58. [Google Scholar] [CrossRef]
- Smits, A. Undulatory and oscillatory swimming. J. Fluid Mech. 2019, 874, 1–70. [Google Scholar] [CrossRef]
- Akoz, E.; Moored, K.W. Unsteady propulsion by an intermittent swimming gait. J. Fluid Mech. 2018, 834, 149–172. [Google Scholar] [CrossRef]
- Floryan, D.; Buren, T.V.; Rowley, C.W.; Smits, A.J. Scaling the propulsive performance of heaving and pitching foils. J. Fluid Mech. 2017, 822, 386–397. [Google Scholar] [CrossRef]
- Xiong, G.; Lauder, G.V. Center of mass motion in swimming fish: Effects of speed and locomotor mode during undulatory propulsion. Zoology 2014, 117, 269–281. [Google Scholar] [CrossRef] [PubMed]
- Lauder, G.V. Fish Locomotion: Recent Advances and New Directions. Annu. Rev. Mar. Sci. 2015, 7, 521–545. [Google Scholar] [CrossRef]
- Paniccia, D.; Padovani, L.; Graziani, G.; Piva, R. The performance of a flapping foil for a self-propelled fishlike body. Sci. Rep. 2021, 11, 22297. [Google Scholar] [CrossRef] [PubMed]
- Bale, R.; Hao, M.; Bhalla, A.P.S.; Patel, N.; Patankar, N.A. Gray’s paradox A fluid mechanical perspective. Sci. Rep. 2014, 4, 5904. [Google Scholar] [CrossRef]
- Zhang, J. Footprints of a flapping wing. J. Fluid Mech. 2017, 810, 1–4. [Google Scholar] [CrossRef]
- Ahlborn, B.; Harper, D.; Blake, R.; Ahlborn, D.; Cam, M. Fish without Footprints. J. Theor. Biol. 1991, 148, 521–533. [Google Scholar] [CrossRef]
- Paniccia, D.; Graziani, G.; Lugni, C.; Piva, R. The fish ability to accelerate and suddenly turn in fast maneuvers. Sci. Rep. 2022, 12, 4096. [Google Scholar] [CrossRef]
- Webb, P.W. The swimming energetics of trout. I. Thrust and power output at cruising speeds. J. Exp. Biol. 1971, 55, 489–520. [Google Scholar] [CrossRef]
- Chopra, M.G. Large amplitude lunate-tail theory of fish locomotion. J. Fluid Mech. 1976, 74, 161–182. [Google Scholar] [CrossRef]
- Webb, P.W. Is the High Cost of Body/Caudal Fin Undulatory Swimming due to increased Friction Drag or Inertial Recoil? J. Exp. Biol. 1992, 162, 157–166. [Google Scholar] [CrossRef]
- Webb, P.; Weihs, D. Stability versus Maneuvering: Challenges for Stability during Swimming by Fishes. Integr. Comp. Biol. 2015, 55, 753–764. [Google Scholar] [CrossRef]
- Borazjani, I. The functional role of caudal and anal/dorsal fins during the C-start of a bluegill sunfish. J. Exp. Biol. 2013, 216, 1658–1669. [Google Scholar] [CrossRef]
- Bhalla, A.P.S.; Bale, R.; Griffith, B.E.; Patankar, N.A. A unified mathematical framework and an adaptive numerical method for fluid-structure interaction with rigid deforming and elastic bodies. J. Comp. Phys. 2013, 250, 446–476. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Paniccia, D.; Padovani, L.; Graziani, G.; Lugni, C.; Piva, R. How Free Swimming Fosters the Locomotion of a Purely Oscillating Fish-like Body. Biomimetics 2023, 8, 401. https://doi.org/10.3390/biomimetics8050401
Paniccia D, Padovani L, Graziani G, Lugni C, Piva R. How Free Swimming Fosters the Locomotion of a Purely Oscillating Fish-like Body. Biomimetics. 2023; 8(5):401. https://doi.org/10.3390/biomimetics8050401
Chicago/Turabian StylePaniccia, Damiano, Luca Padovani, Giorgio Graziani, Claudio Lugni, and Renzo Piva. 2023. "How Free Swimming Fosters the Locomotion of a Purely Oscillating Fish-like Body" Biomimetics 8, no. 5: 401. https://doi.org/10.3390/biomimetics8050401
APA StylePaniccia, D., Padovani, L., Graziani, G., Lugni, C., & Piva, R. (2023). How Free Swimming Fosters the Locomotion of a Purely Oscillating Fish-like Body. Biomimetics, 8(5), 401. https://doi.org/10.3390/biomimetics8050401





