Anomalous Thermally Induced Deformation in Kelvin–Voigt Plate with Ultrafast Double-Strip Surface Heating
Abstract
:1. Introduction
2. Mathematical Model
- (i)
- Stress–strain constitutive relation
- (ii)
- The strain–displacement relation
- (iii)
- Conservation of momentum
- (iv)
- The balance equation for the entropy
3. Problem Formulation
- (i)
- The non-vanishing components of the stress tensor
- (ii)
- The non-vanishing components of the strain tensor
- (iii)
- The equations of motion in the absence of body forces along the x- and y-directions, respectively,
- (i)
- The heat conduction equation in the absence of a heat source
4. Solutions in the Integral-Transform Domain
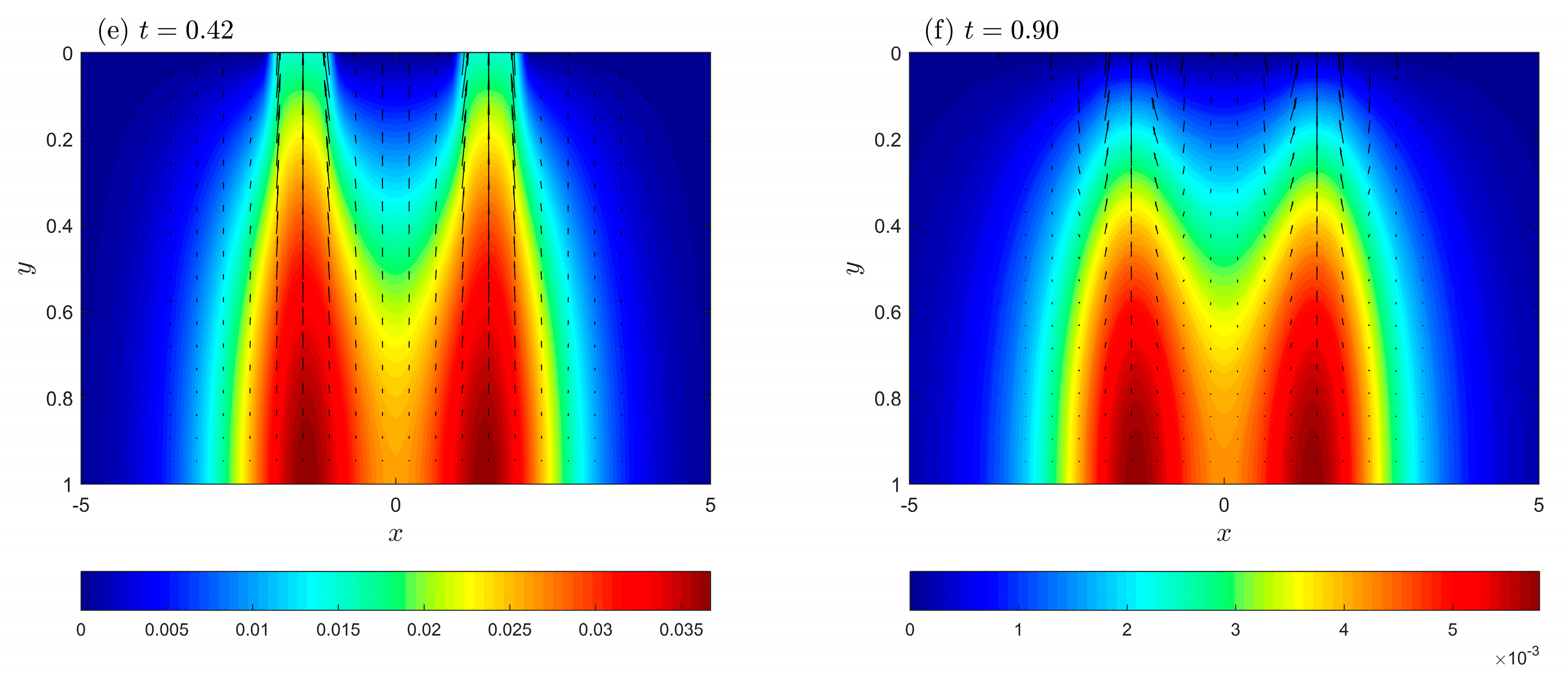
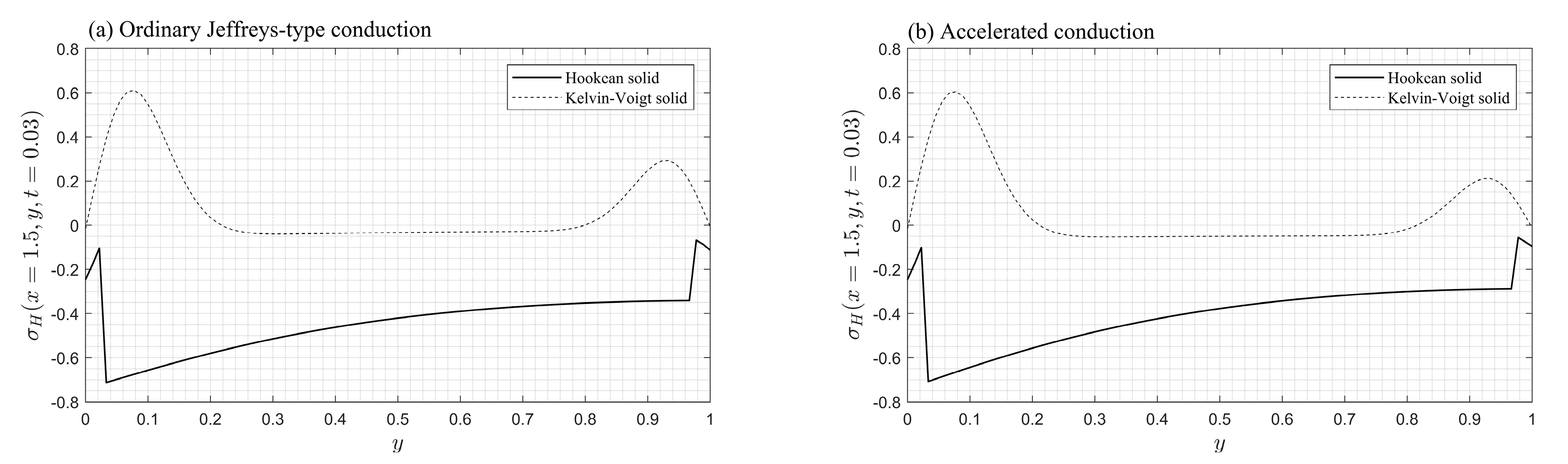
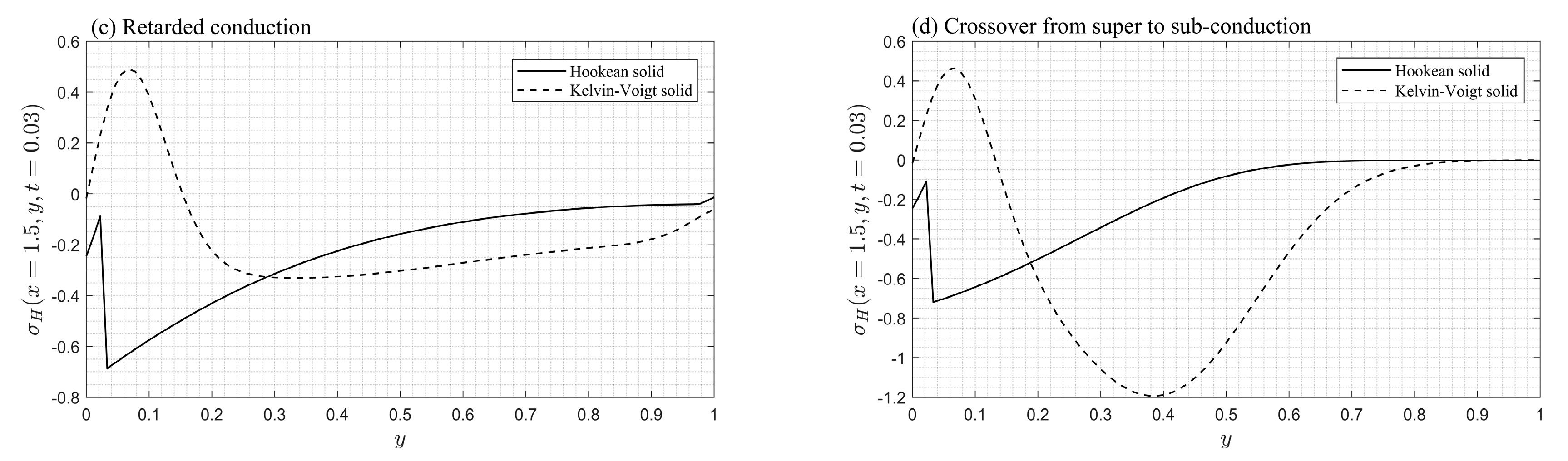
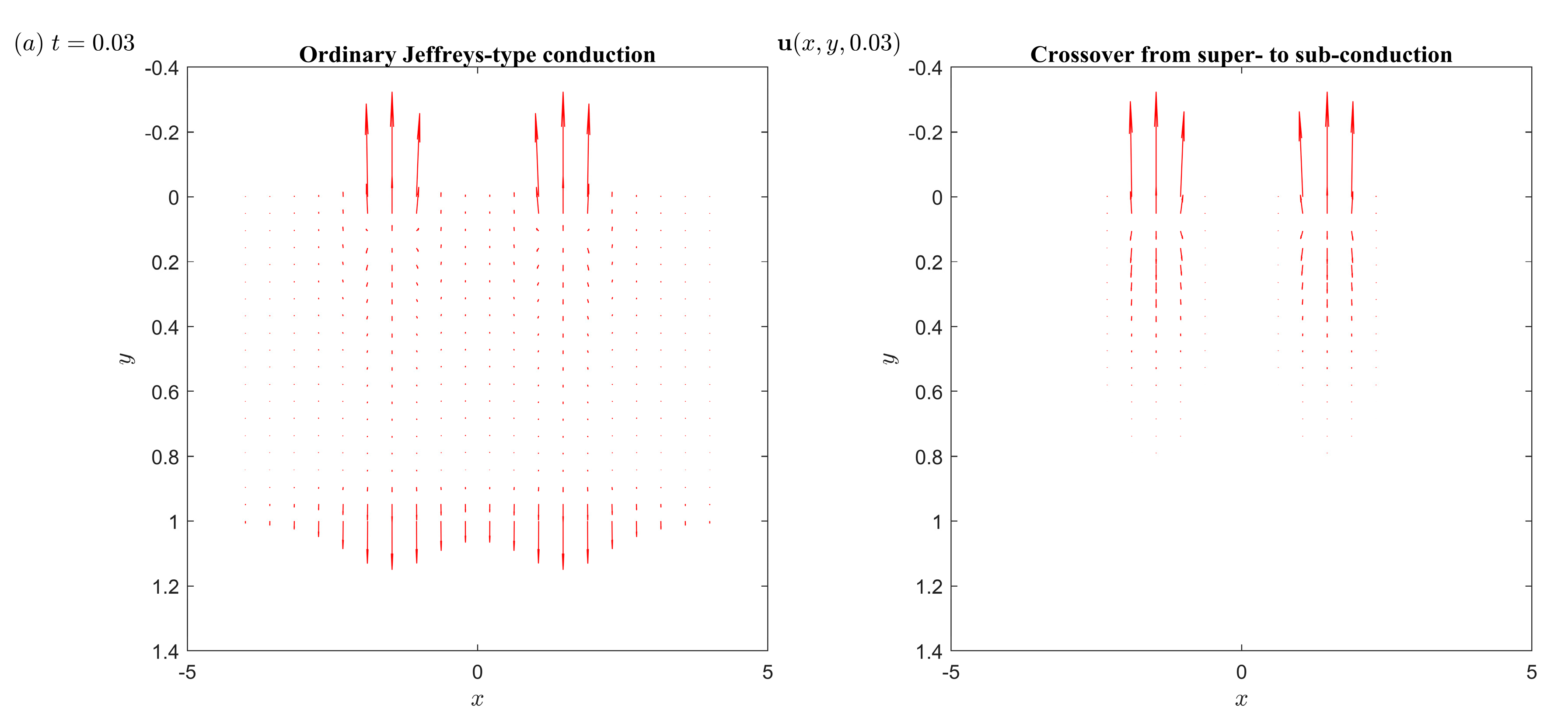
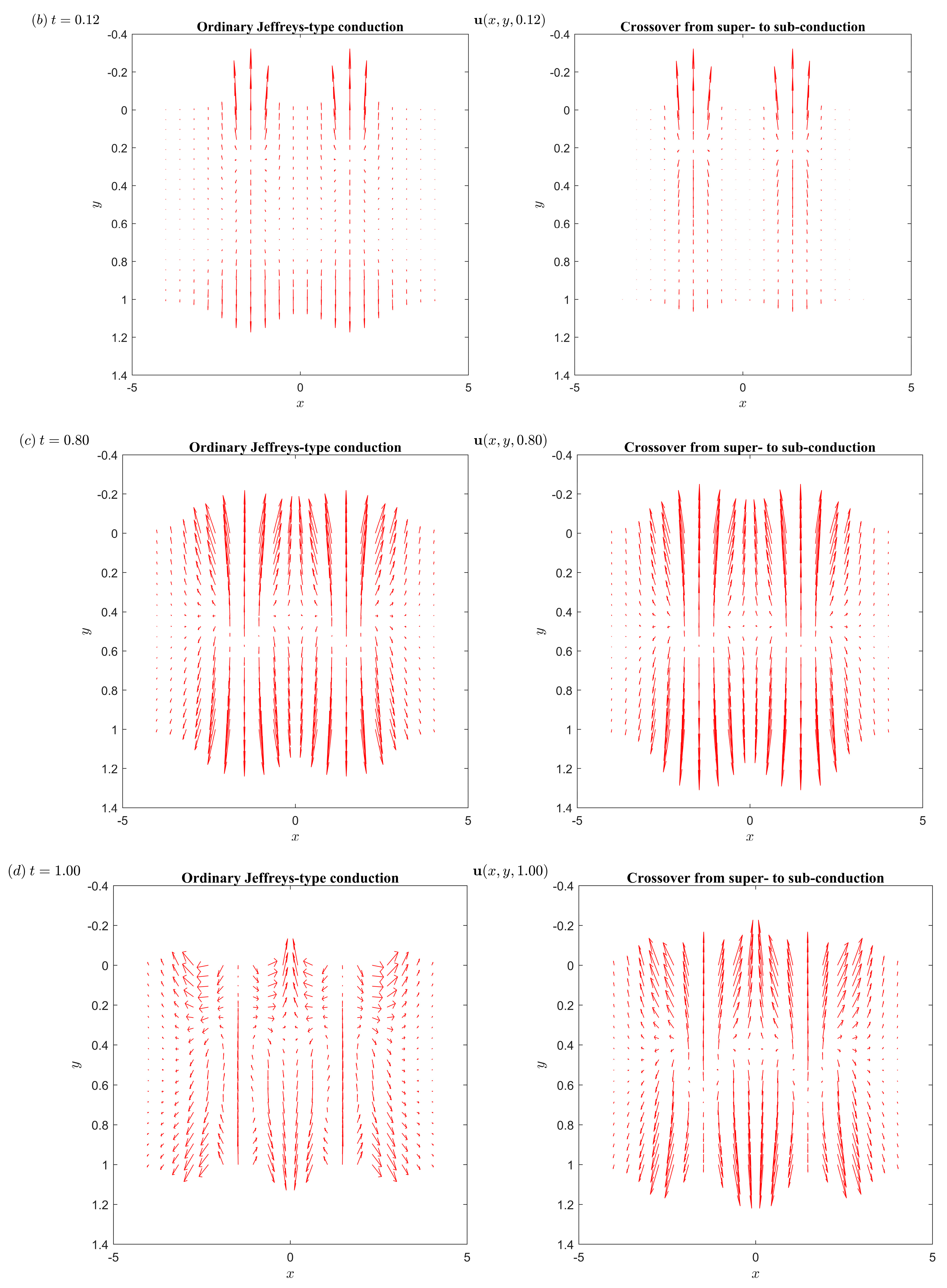
5. Numerical Results and Discussion
6. Summary
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Fournier, D.; Boccara, A. Heterogeneous media and rough surfaces: A fractal approach for heat diffusion studies. Phys. A Stat. Mech. Its Appl. 1989, 157, 587–592. [Google Scholar] [CrossRef]
- Tzou, D.Y.; Chen, J.K. Thermal lagging in random media. J. Thermophys. Heat Transf. 1998, 12, 567–574. [Google Scholar] [CrossRef]
- Choi, S.U.S.; Zhang, Z.G.; Yu, W.; Lockwood, F.E.; Grulke, E.A. Anomalous thermal conductivity enhancement in nanotube suspensions. Appl. Phys. Lett. 2001, 79, 2252–2254. [Google Scholar] [CrossRef]
- Li, B.; Wang, J. Anomalous heat conduction and anomalous diffusion in one-dimensional systems. Phys. Rev. Lett. 2003, 91, 044301. [Google Scholar] [CrossRef] [Green Version]
- Lee, V.; Wu, C.-H.; Lou, Z.-X.; Lee, W.-L.; Chang, C.-W. Divergent and ultrahigh thermal conductivity in millimeter-long nanotubes. Phys. Rev. Lett. 2017, 118, 135901. [Google Scholar] [CrossRef]
- Tzou, D.Y. Macro-to Microscale Heat Transfer: The Lagging Behavior, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Compte, A.; Metzler, R. The generalized Cattaneo equation for the description of anomalous transport processes. J. Phys. A Math. Gen. 1997, 30, 7277. [Google Scholar] [CrossRef]
- Barkai, E. Fractional Fokker-Planck equation, solution, and application. Phys. Rev. E 2001, 63, 046118. [Google Scholar] [CrossRef] [Green Version]
- Klafter, J.; Lim, S.; Metzler, R. Fractional Dynamics: Recent Advances; World Scientific: Singapore, 2012. [Google Scholar]
- Sierociuk, D.; Dzieliński, A.; Sarwas, G.; Petras, I.; Podlubny, I.; Skovranek, T. Modelling heat transfer in heterogeneous media using fractional calculus. Phil. Trans. R. Soc. A 2013, 371, 20120146. [Google Scholar] [CrossRef] [Green Version]
- Ji, C.-C.; Dai, W.; Sun, Z.-Z. Numerical Method for Solving the Time-Fractional Dual-Phase-Lagging Heat Conduction Equation with the Temperature-Jump Boundary Condition. J. Sci. Comput. 2018, 75, 1307–1336. [Google Scholar] [CrossRef]
- Ji, C.-C.; Dai, W.; Sun, Z.-Z. Numerical Schemes for Solving the Time-Fractional Dual-Phase-Lagging Heat Conduction Model in a Double-Layered Nanoscale Thin Film. J. Sci. Comput. 2019, 81, 1767–1800. [Google Scholar] [CrossRef]
- Bazhlekova, E.; Bazhlekov, I. Transition from Diffusion to Wave Propagation in Fractional Jeffreys-Type Heat Conduction Equation. Fractal Fract. 2020, 4, 32. [Google Scholar] [CrossRef]
- Awad, E.; Metzler, R. Crossover dynamics from superdiffusion to subdiffusion: Models and solutions. Fract. Calc. Appl. Anal. 2020, 23, 55–102. [Google Scholar] [CrossRef] [Green Version]
- Awad, E.; Sandev, T.; Metzler, R.; Chechkin, A. Closed-form multi-dimensional solutions and asymptotic behaviors for subdiffusive processes with crossovers: I. Retarding case. Chaos Solitons Fractals 2021, 152, 111357. [Google Scholar] [CrossRef]
- Awad, E.; Metzler, R. Closed-form multi-dimensional solutions and asymptotic behaviours for subdiffusive processes with crossovers: II. Accelerating case. J. Phys. A: Math. Gen. 2022, 55, 205003. [Google Scholar] [CrossRef]
- Górska, K.; Horzela, A. Subordination and memory dependent kinetics in diffusion and relaxation phenomena. Fract. Calc. Appl. Anal. 2023, 26, 480–512. [Google Scholar] [CrossRef]
- Liu, L.; Zheng, L.; Chen, Y.; Liu, F. Anomalous diffusion in comb model with fractional dual-phase-lag constitutive relation. Comput. Math. Appl. 2018, 76, 245–256. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Feng, L.; Xu, Q.; Chen, Y. Anomalous diffusion in comb model subject to a novel distributed order time fractional Cattaneo–Christov flux. Appl. Math. Lett. 2020, 102, 106116. [Google Scholar] [CrossRef]
- Awad, E. Dual-Phase-Lag in the balance: Sufficiency bounds for the class of Jeffreys’ equations to furnish physical solutions. Int. J. Heat Mass Trans. 2020, 158, 119742. [Google Scholar] [CrossRef]
- Awad, E.; Sandev, T.; Metzler, R.; Chechkin, A. From continuous-time random walks to the fractional Jeffreys equation: Solution and properties. Int. J. Heat Mass Transf. 2021, 181, 121839. [Google Scholar] [CrossRef]
- Hetnarski, R.B.; Ignaczak, J. Generalized thermoelasticity. J. Therm. Stress. 1999, 22, 451–476. [Google Scholar]
- Ignaczak, J.; Ostoja-Starzewski, M. Thermoelasticity with Finite Wave Speeds; Oxford University Press: New York, NY, USA, 2010. [Google Scholar]
- Chandrasekharaiah, D.S. Hyperbolic thermoelasticity: A review of recent literature. Appl. Mech. Rev. 1998, 51, 705–729. [Google Scholar] [CrossRef]
- Povstenko, V.Z. Fractional heat conduction equation and associated thermal stress. J. Therm. Stress. 2005, 28, 83–102. [Google Scholar] [CrossRef]
- Povstenko, Y.Z. Fractional Thermoelasticity; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Sherief, H.H.; El-Sayed, A.M.A.; Abd El-Latief, A.M. Fractional order theory of thermoelasticity. Int. J. Solids Struct. 2010, 47, 269–275. [Google Scholar] [CrossRef]
- Youssef, H.M. Theory of fractional order generalized thermoelasticity. J. Heat Transf. 2010, 132, 061301. [Google Scholar] [CrossRef]
- Ezzat, M.A.; Karamany, A.S.E. Fractional order heat conduction law in magneto-thermoelasticity involving two temperatures. Z. Fur. Angew. Math. Phys. 2011, 62, 937–952. [Google Scholar] [CrossRef]
- Awad, E. On the generalized thermal lagging behavior: Refined aspects. J. Therm. Stress. 2012, 35, 293–325. [Google Scholar] [CrossRef]
- Elhagary, M. Effect of Atangana–Baleanu fractional derivative on a two-dimensional thermoviscoelastic problem for solid sphere under axisymmetric distribution. Mech. Based Des. Struct. Mach. 2021, 51, 3295–3312. [Google Scholar] [CrossRef]
- Sherief, H.H.; Abd El-Latief, A.M.; Fayik, M.A. 2D hereditary thermoelastic application of a thick plate under axisymmetric temperature distribution. Math. Methods Appl. Sci. 2022, 45, 1080–1092. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: Singapore, 2010. [Google Scholar]
- Gurtin, M.E.; Sternberg, E. On the linear theory of viscoelasticity. Arch. Ration. Mech. Anal. 1962, 11, 291–356. [Google Scholar] [CrossRef]
- Mukhopadhyay, S. Effects of thermal relaxations on thermoviscoelastic interactions in an unbounded body with a spherical cavity subjected to a periodic loading on the boundary. J. Therm. Stress. 2000, 23, 675–684. [Google Scholar] [CrossRef]
- Youssef, H.M.; Al-Lehaibi, E.A. The vibration of viscothermoelastic static pre-stress nanobeam based on two-temperature dual-phase-lag heat conduction and subjected to ramp-type heat. J. Strain Anal. Eng. Des. 2022, 58, 410–421. [Google Scholar] [CrossRef]
- Abouelregal, A.; Zenkour, A. Fractional viscoelastic Voigt’s model for initially stressed microbeams induced by ultrashort laser heat source. Waves Random Complex Media 2020, 30, 687–703. [Google Scholar] [CrossRef]
- Magaña, A.; Quintanilla, R. On the uniqueness and analyticity of solutions in micropolar thermoviscoelasticity. J. Math. Anal. Appl. 2014, 412, 109–120. [Google Scholar] [CrossRef] [Green Version]
- Fabrizio, M.; Franchi, F.; Nibbi, R. Second gradient Green–Naghdi type thermo-elasticity and viscoelasticity. Mech. Res. Commun. 2022, 126, 104014. [Google Scholar] [CrossRef]
- Sherief, H.H.; Helmy, K.A. A two-dimensional generalized thermoelasticity problem for a half-space. J. Therm. Stress. 1999, 22, 897–910. [Google Scholar]
- Sherief, H.H.; Megahed, F.A. A two-dimensional thermoelasticity problem for a half space subjected to heat sources. Int. J. Solids Struct. 1999, 36, 1369–1382. [Google Scholar] [CrossRef]
- El-Maghraby, N.M. A two-dimensional problem for a thick plate with heat sources in generalized thermoelasticity. J. Therm. Stress. 2005, 28, 1227–1241. [Google Scholar] [CrossRef]
- El-Maghraby, N.M. Two-dimensional thermoelasticity problem for a thick plate under the action of a body force in two relaxation times. J. Therm. Stress. 2009, 32, 863–876. [Google Scholar] [CrossRef]
- Pal, P.; Kanoria, M. Thermoelastic wave propagation in a transversely isotropic thick plate under Green–Naghdi theory due to gravitational field. J. Therm. Stress. 2017, 40, 470–485. [Google Scholar] [CrossRef]
- Kalkal, K.K.; Deswal, S.; Yadav, R. Eigenvalue approach to fractional-order dual-phase-lag thermoviscoelastic problem of a thick plate. IJST-T Mech. Eng. 2019, 43, 917–927. [Google Scholar] [CrossRef]
- Tzou, D.Y.; Beraun, J.E.; Chen, J.K. Ultrafast deformation in femtosecond laser heating. J. Heat Transf. 2002, 124, 284–292. [Google Scholar] [CrossRef]
- Falkovsky, L.A.; Mishchenko, E.G. Electron-lattice kinetics of metals heated by ultrashort laser pulses. J. Exp. Theor. Phys. 1999, 88, 84–88. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.K.; Beraun, J.E.; Tham, C.L. Ultrafast thermoelasticity for short-pulse laser heating. Int. J. Eng. Sci. 2004, 42, 793–807. [Google Scholar] [CrossRef]
- Tzou, D.Y.; Chen, J.K.; Beraun, J.E. Recent development of ultrafast thermoelasticity. J. Therm. Stress. 2005, 28, 563–594. [Google Scholar] [CrossRef]
- Awad, E.; Fayik, M.; El-Dhaba, A. A comparative numerical study of a semi-infinite heat conductor subject to double-strip heating under non-Fourier models. Eur. Phys. J. Plus 2022, 137, 1303. [Google Scholar] [CrossRef]
- Awad, E.; Abo-Dahab, S.M.; Abdou, M.A. Exact solutions for a two-dimensional thermoelectric MHD flow with steady-state heat transfer on a vertical plate with two instantaneous infinite hot suction lines. arXiv 2022, arXiv:2212.01665. [Google Scholar] [CrossRef]
- Awad, E. On the time-fractional Cattaneo equation of distributed order. Phys. A Stat. Mech. Its Appl. 2019, 518, 210–233. [Google Scholar] [CrossRef]
- Gorenflo, R.; Mainardi, F. Fractional calculus. In Fractals and Fractional Calculus in Continuum Mechanics; Springer: Berlin/Heidelberg, Germany, 1997; pp. 223–276. [Google Scholar]
- Duffy, D.G. Transform Methods for Solving Partial Differential Equations; Chapman and Hall/CRC: Portsmouth, UK, 2004. [Google Scholar]
- Callister, W.D.; Rethwisch, D.G. Materials Science and Engineering: An Introduction; Wiley: New York, NY, USA, 2007. [Google Scholar]
- Thomas, L.C. Heat Transfer; Prentice Hall: Hoboken, NJ, USA, 1992. [Google Scholar]
- Fayik, M.; Alhazmi, S.E.; Abdou, M.A.; Awad, E. Transient Finite-Speed Heat Transfer Influence on Deformation of a Nanoplate with Ultrafast Circular Ring Heating. Mathematics 2023, 11, 1099. [Google Scholar] [CrossRef]
- Durbin, F. Numerical inversion of Laplace transforms: An efficient improvement to Dubner and Abate′s method. Comput. J. 1974, 17, 371–376. [Google Scholar] [CrossRef] [Green Version]
- Dubner, H.; Abate, J. Numerical inversion of Laplace transforms by relating them to the finite Fourier cosine transform. J. ACM 1968, 15, 115–123. [Google Scholar] [CrossRef]
- Honig, G.; Hirdes, U. A method for the numerical inversion of Laplace transforms. J. Comput. Appl. Math. 1984, 10, 113–132. [Google Scholar] [CrossRef] [Green Version]
- Press, W.H.; Teukolsky, S.A.; Flannery, B.P.; Vetterling, W.T. Numerical Recipes in Fortran 77: The Art of Scientific Computing, 2nd ed.; Cambridge University Press: Cambridge, UK, 1992; Volume 1. [Google Scholar]
- Rukolaine, S.A.; Samsonov, A.M. Local immobilization of particles in mass transfer described by a Jeffreys-type equation. Phys. Rev. E 2013, 88, 062116. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bora, A.; Dai, W.; Wilson, J.P.; Boyt, J.C. Neural network method for solving parabolic two-temperature microscale heat conduction in double-layered thin films exposed to ultrashort-pulsed lasers. Int. J. Heat Mass Transf. 2021, 178, 121616. [Google Scholar] [CrossRef]
- Bora, A.; Dai, W.; Wilson, J.P.; Boyt, J.C.; Sobolev, S. Neural network method for solving nonlocal two-temperature nanoscale heat conduction in gold films exposed to ultrashort-pulsed lasers. Int. J. Heat Mass Transf. 2022, 190, 122791. [Google Scholar] [CrossRef]


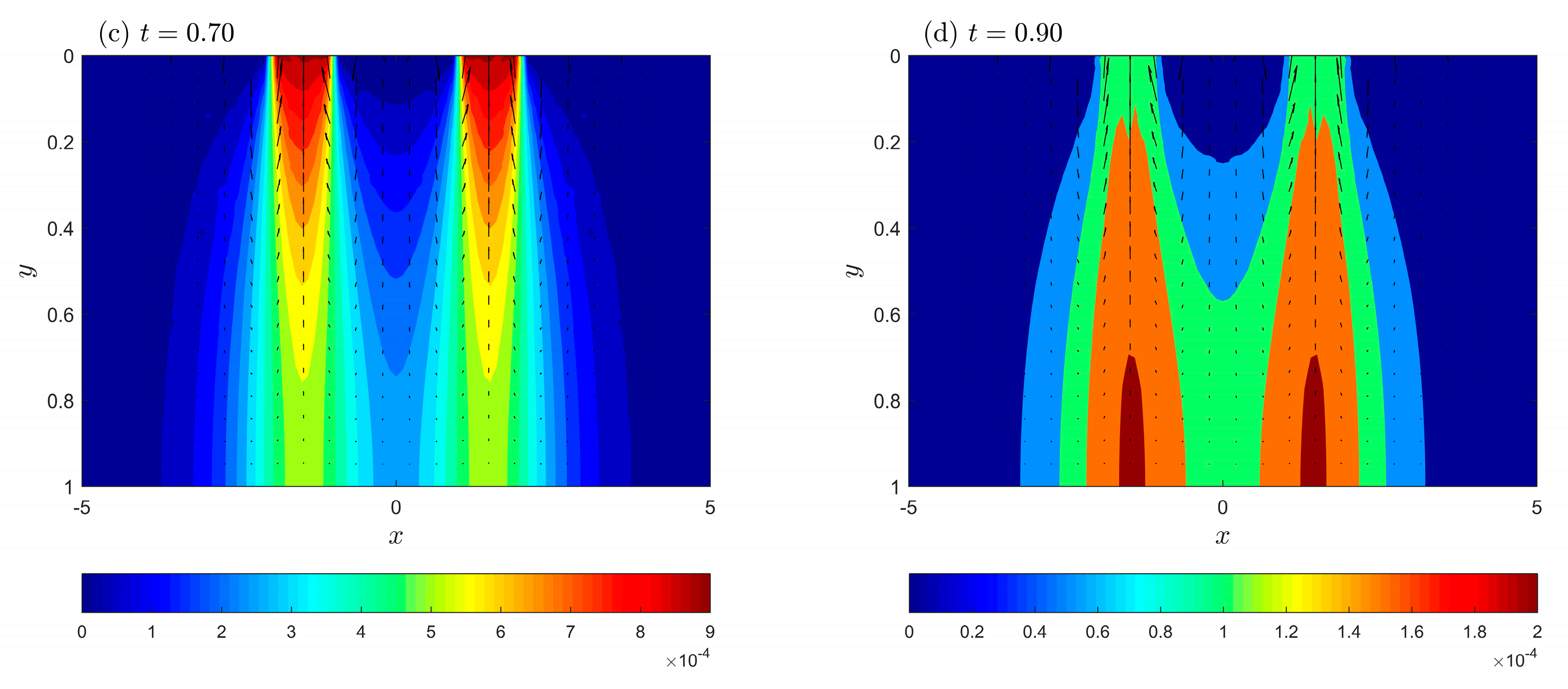
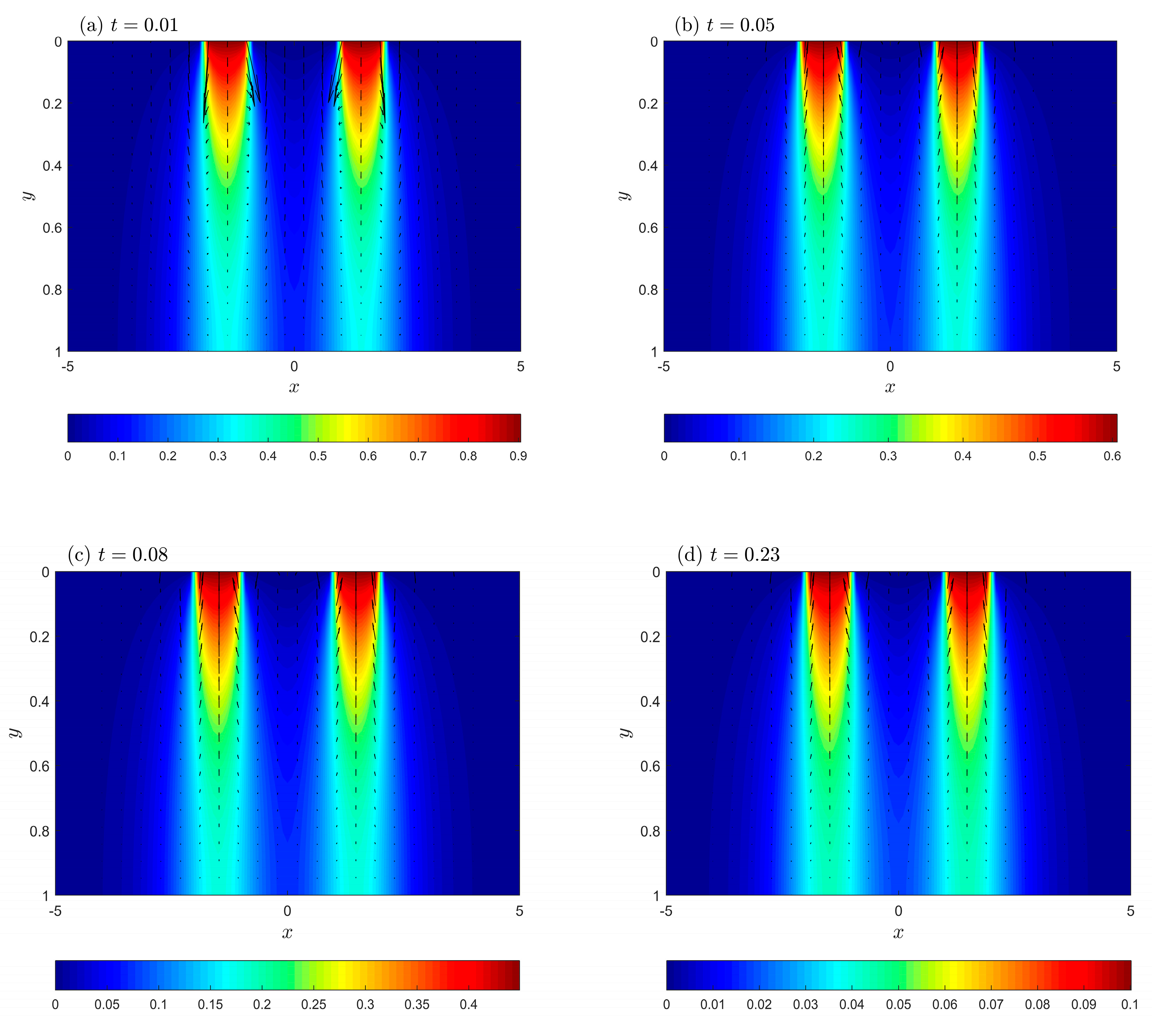

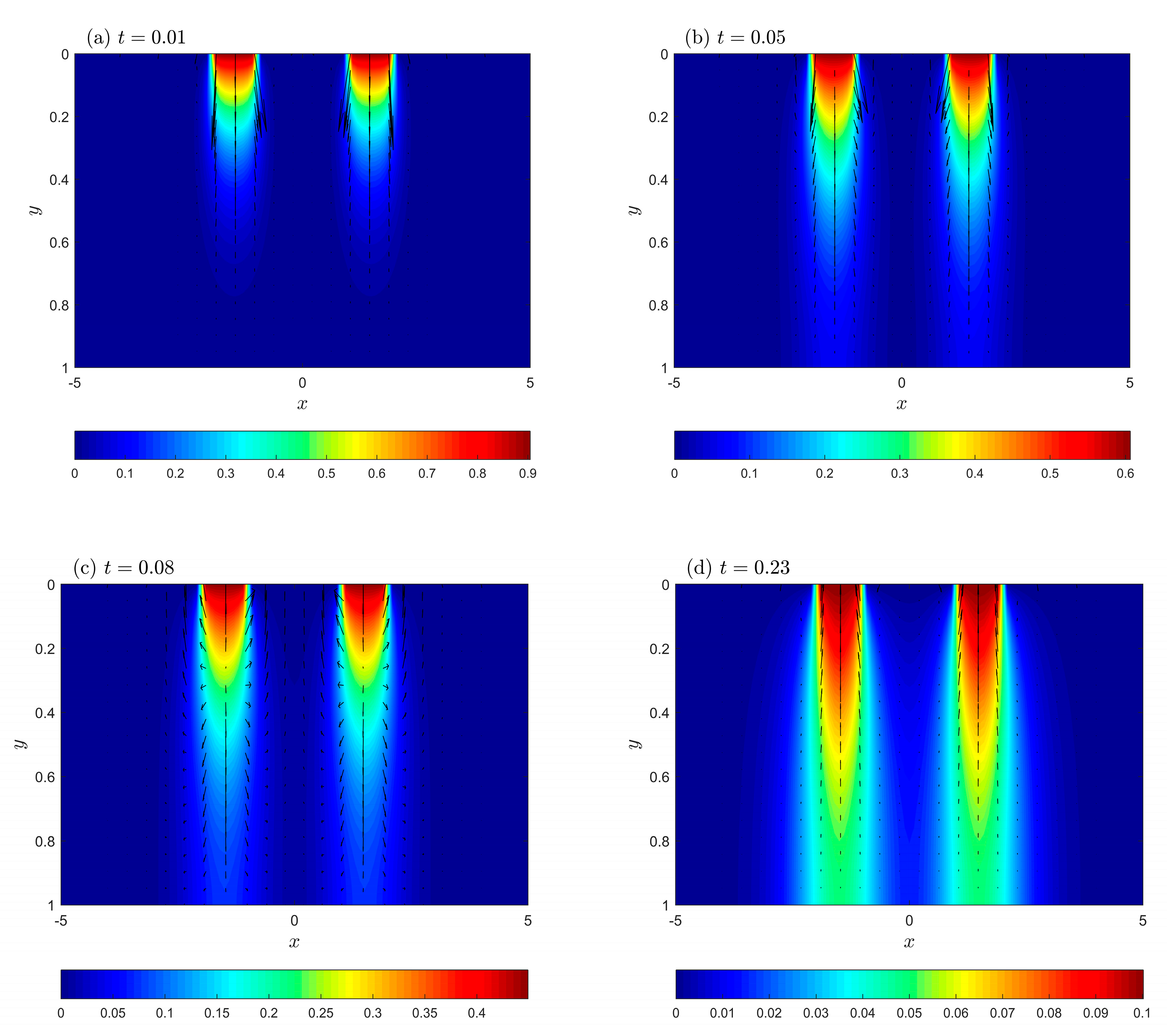

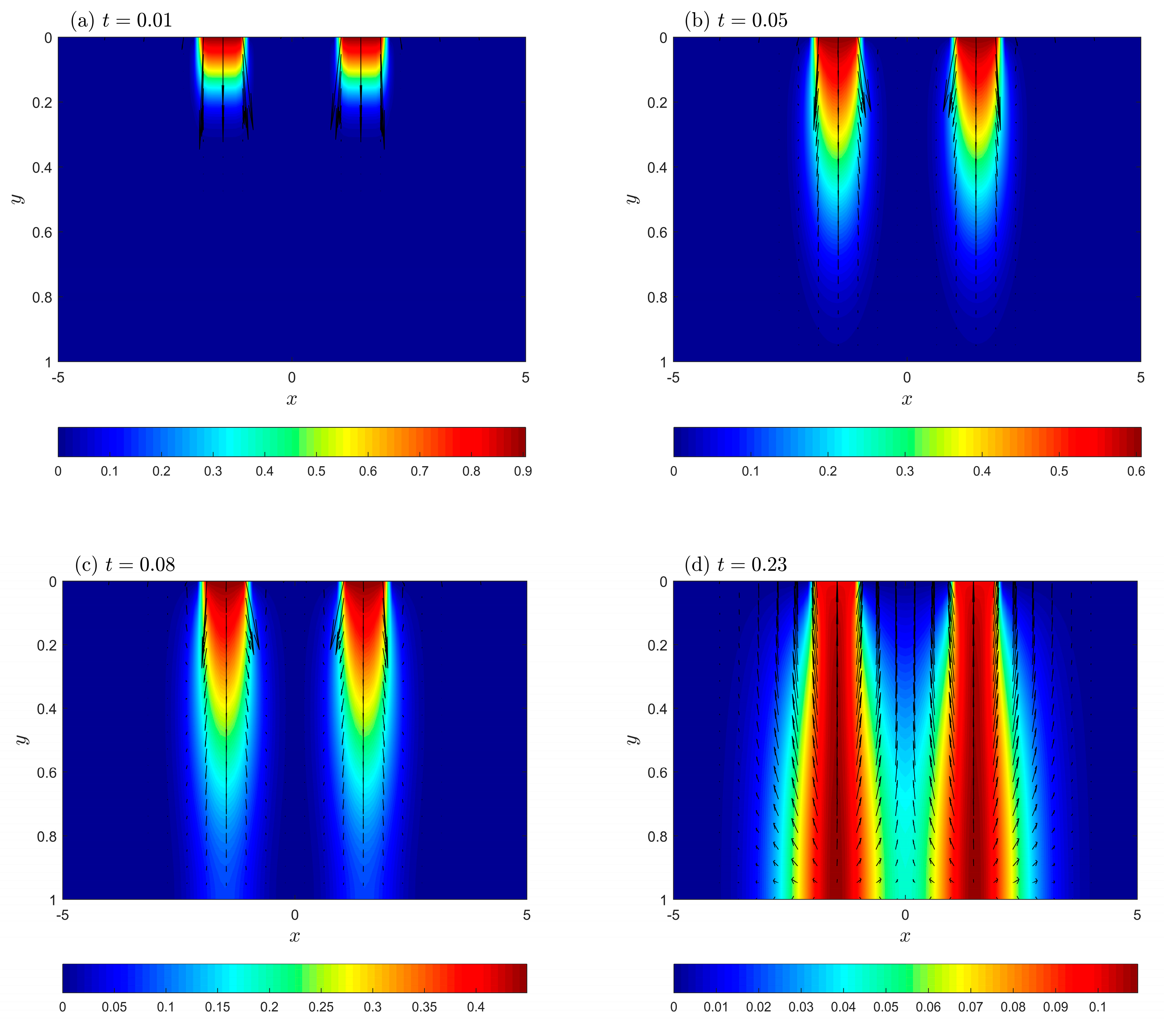

| Property | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Value | 8954 | 70.833 | |||||||
| SI unit | picoseconds | picoseconds | picoseconds |
| Ordinary Jeffreys Heat Conduction | The Fractional Jeffreys-Type Heat Conduction | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Accelerated Conduction | Retarded Conduction | Crossover from Super- to Sub-Conduction | |||||||||||
| x | |||||||||||||
| y | |||||||||||||
| 0.000009 | |||||||||||||
| Ordinary Jeffreys Heat Conduction | The Fractional Jeffreys-Type Heat Conduction | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Accelerated Conduction | Retarded Conduction | Crossover from Super- to Sub-Conduction | |||||||||||
| x | |||||||||||||
| y | |||||||||||||
| 0.000019 | |||||||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Awad, E.; Alhazmi, S.E.; Abdou, M.A.; Fayik, M. Anomalous Thermally Induced Deformation in Kelvin–Voigt Plate with Ultrafast Double-Strip Surface Heating. Fractal Fract. 2023, 7, 563. https://doi.org/10.3390/fractalfract7070563
Awad E, Alhazmi SE, Abdou MA, Fayik M. Anomalous Thermally Induced Deformation in Kelvin–Voigt Plate with Ultrafast Double-Strip Surface Heating. Fractal and Fractional. 2023; 7(7):563. https://doi.org/10.3390/fractalfract7070563
Chicago/Turabian StyleAwad, Emad, Sharifah E. Alhazmi, Mohamed A. Abdou, and Mohsen Fayik. 2023. "Anomalous Thermally Induced Deformation in Kelvin–Voigt Plate with Ultrafast Double-Strip Surface Heating" Fractal and Fractional 7, no. 7: 563. https://doi.org/10.3390/fractalfract7070563







