Comparison of Fracture Resistance of the Normal and High Strength Concrete Evaluated by Brazilian Disc Test †
Abstract
:1. Introduction
2. Theoretical Background
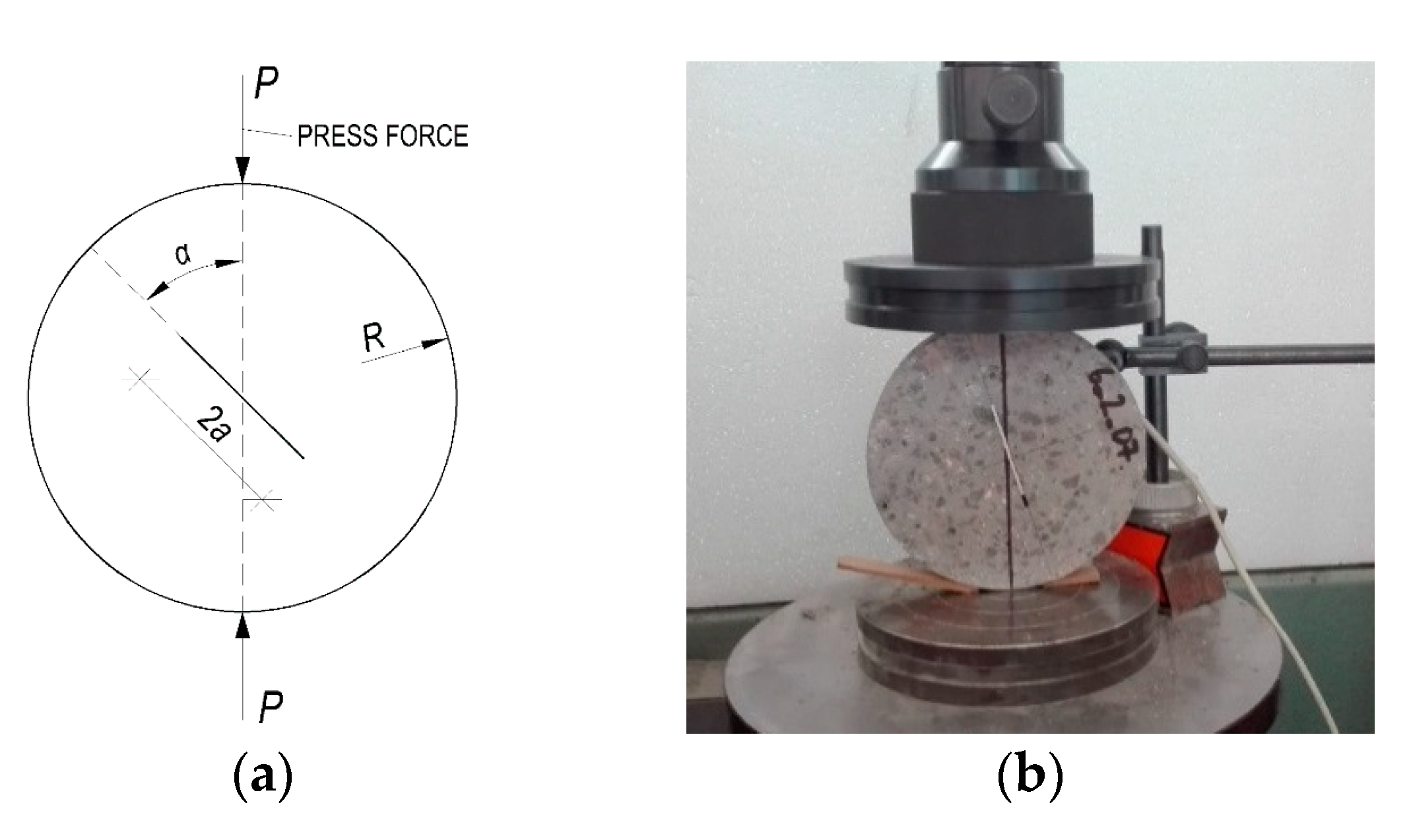
2.1. Brazilian Disc Test
2.2. GMTS Criterion
Application of the GMTS on Brazilian Disc Specimen
3. Materials
3.1. Normal Strength Concrete
3.2. High Strength Concrete
4. Experimental Measurement
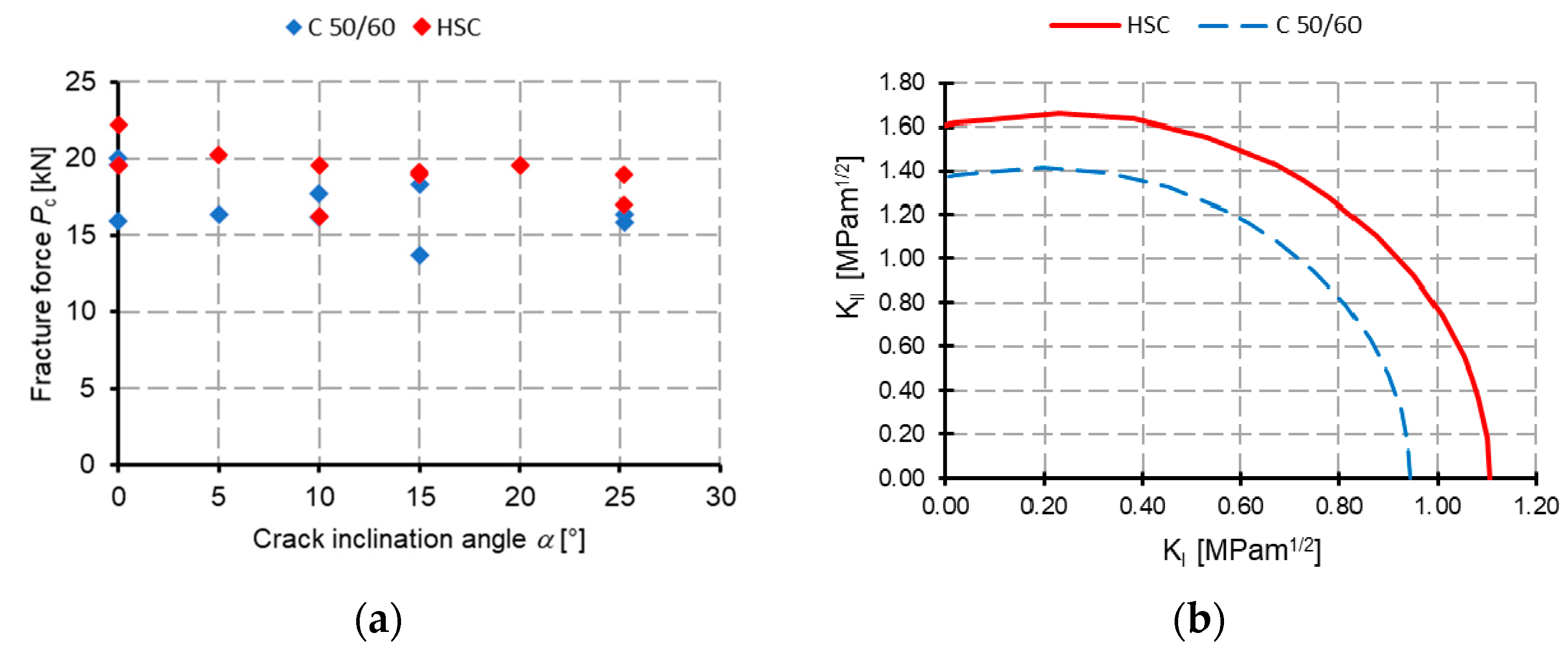
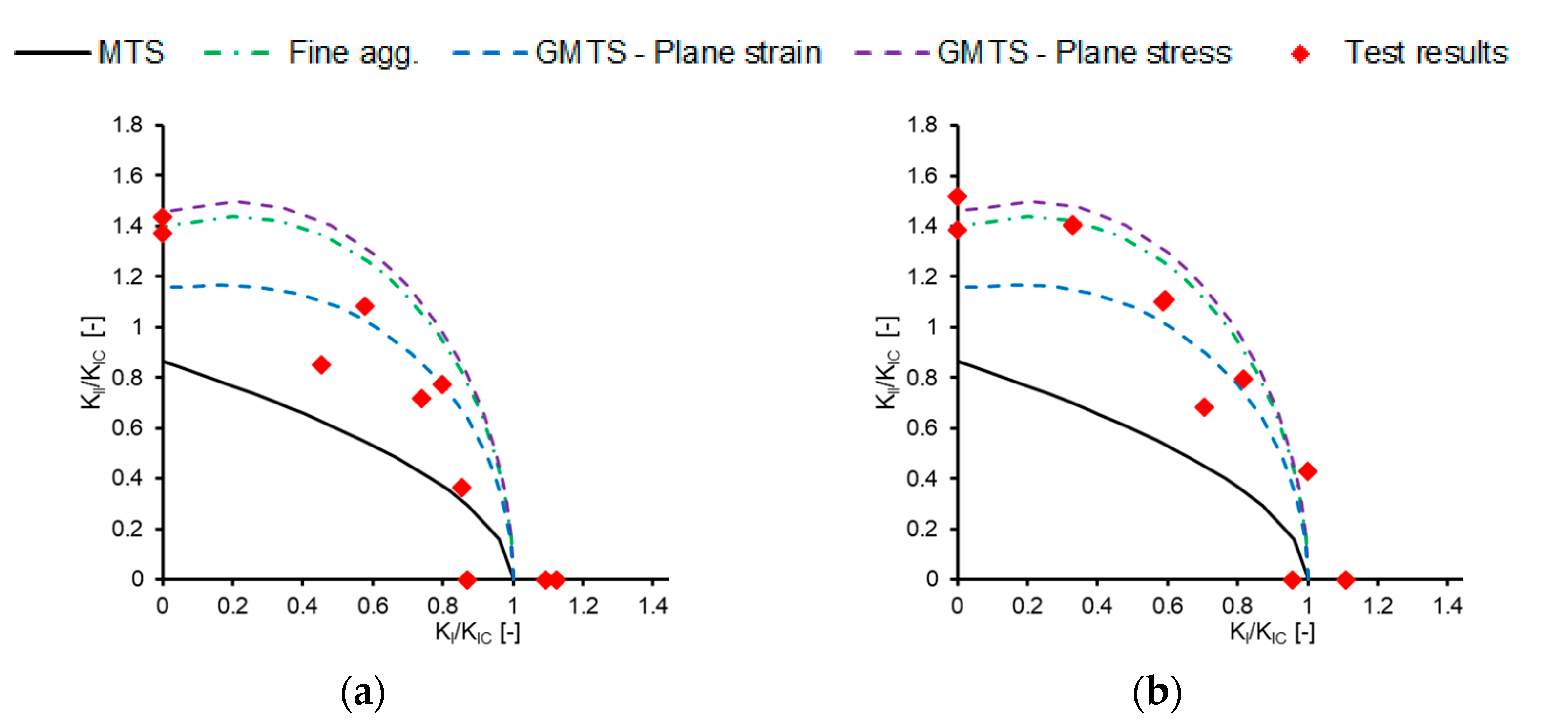
5. Results and Discussion
6. Conclusions
- The fracture toughness measured on the HSC material is higher in all investigated cases than for the traditional C 50/60 material.
- The experimental results done on the HSC material showed higher fracture resistance in mixed mode I/II than the traditional C 50/60 material.
- The fracture resistance of the C 50/60 material is characterized best by rC for plain strain, yet for HSC, it is better to use value of rC for fine aggregate.
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Nawy, E.G. Fundamentals of High-Performance Concrete; Wiley: Hoboken, NJ, USA, 2001. [Google Scholar]
- Malíková, L.; Veselý, V.; Seitl, S. Crack propagation direction in a mixed mode geometry estimated via multi-parameter fracture criteria. Int. J. Fatigue 2016, 89, 99–107. [Google Scholar] [CrossRef]
- Fett, T.; Gerteisen, G.; Hahnenberger, S.; Martin, G.; Munz, D. Fracture tests for ceramics under mode-I, mode-II and mixed-mode loading. J. Eur. Ceram. Soc. 1995, 15, 307–312. [Google Scholar] [CrossRef]
- Ayatollahi, M.R.; Aliha, M.R.M.; Saghafi, H. An improved semi-circular bend specimen for investigating mixed mode brittle fracture. Eng. Fract. Mech. 2011, 78, 110–123. [Google Scholar] [CrossRef]
- Li, D.; Wong, L.N.Y. The brazilian disc test for rock mechanics applications: Review and new insights. Rock Mech. Rock Eng. 2013, 46, 269–287. [Google Scholar] [CrossRef]
- Atkinson, C.; Smelser, R.E.; Sanchez, J. Combined mode fracture via the cracked brazilian disk test. Int. J. Fract. 1982, 18, 279–291. [Google Scholar] [CrossRef]
- Williams, M.L. On the stress distribution at the base of a stationary crack. J. Appl. Mech. 1956, 24, 6. [Google Scholar] [CrossRef]
- Ayatollahi, M.R.; Aliha, M.R.M. On the use of brazilian disc specimen for calculating mixed mode I-II fracture toughness of rock materials. Eng. Fract. Mech. 2008, 75, 4631–4641. [Google Scholar] [CrossRef]
- Seitl, S.; Miarka, P. Evaluation of mixed mode I/II fracture toughness of C 50/60 from Brazilian disc test. Frattura ed Integrita Strutturale 2017, 11, 119–127. [Google Scholar] [CrossRef]
- Seitl, S.; Miarka, P.; Bílek, V. The mixed-mode fracture resistance of C 50/60 and its suitability for use in precast elements as determined by the brazilian disc test and three-point bending specimens. Theor. Appl. Fract. Mech. 2018, in press. [Google Scholar] [CrossRef]
- Anderson, T.L. Fracture Mechanics: Fundamentals and Applications; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Tada, H.; Paris, P.C.; Irwin, G.R. The Stress Analysis of Cracks Handbook, 3rd ed.; ASM Press: New York, NY, USA, 2000. [Google Scholar]
- Yang, B.; Ravi-Chandar, K. Evaluation of elastic T-stress by the stress difference method. Eng. Fract. Mech. 1999, 64, 589–605. [Google Scholar] [CrossRef]
- Erdogan, F.; Sih, G.C. On the crack extension in plates under plane loading and transverse shear. J. Basic Eng. 1963, 85, 519–525. [Google Scholar] [CrossRef]
- Sih, G.C. Strain-energy-density factor applied to mixed mode crack problems. Int. J. Fract. 1974, 10, 305–321. [Google Scholar] [CrossRef]
- Smith, D.J.; Ayatollahi, M.R.; Pavier, M.J. The role of T-stress in brittle fracture for linear elastic materials under mixed-mode loading. Fatigue Fract. Eng. Mater. Struct. 2001, 24, 137–150. [Google Scholar] [CrossRef]
- Aliha, M.R.M.; Bahmani, A.; Akhondi, S. Mixed mode fracture toughness testing of pmma with different three-point bend type specimens. Eur. J. Mech.—A/Solids 2016, 58, 148–162. [Google Scholar] [CrossRef]
- Hou, C.; Wang, Z.; Liang, W.; Li, J. Determination of fracture parameters in center cracked circular discs of concrete under diametral loading: A numerical analysis and experimental results. Theor. Appl. Fract. Mech. 2016, 85, 355–366. [Google Scholar] [CrossRef]
- Adu-Amankwah, S.; Zajac, M.; Stabler, C.; Lothenbach, B. Influence of limestone on the hydration of ternary slag cements. Cem. Concr. Res. 2017, 100, 96–109. [Google Scholar] [CrossRef]
- Bilek, V.; Pytlik, D.; Bambuchova, M. High-Performance Concrete with Ternary Binders. Key Eng. Mater. 2018, 761, 120–123. [Google Scholar] [CrossRef]
| Specimen nmr. | Inclination Angle α [°] | Diameter D [mm] | Thickness B [mm] | Notch Length 2a [mm] | a/R [-] |
|---|---|---|---|---|---|
| 6_03 | 0 | 149.200 | 31.380 | 60.210 | 0.404 |
| 6_09 | 0 | 149.182 | 29.927 | 60.170 | 0.403 |
| 6_01 | 0 | 149.155 | 31.440 | 60.920 | 0.408 |
| 6_01 | 5 | 149.162 | 31.440 | 60.920 | 0.408 |
| 6_05 | 10 | 149.143 | 30.580 | 60.140 | 0.403 |
| 6_04 | 10 | 149.162 | 30.973 | 60.040 | 0.403 |
| 6_04 | 15 | 149.208 | 30.970 | 60.040 | 0.402 |
| 6_06 | 15 | 149.214 | 32.390 | 60.150 | 0.403 |
| 6_02 | 25.2 | 149.180 | 30.770 | 60.110 | 0.403 |
| 6_07 | 25.2 | 149.205 | 31.173 | 59.940 | 0.402 |
| Specimen nmr. | Inclination Angle α [°] | Diameter D [mm] | Thickness B [mm] | Notch Length 2a [mm] | a/R [-] |
|---|---|---|---|---|---|
| 6_2_02 | 0 | 149.09 | 29.43 | 59.70 | 0.400 |
| 6_2_01 | 0 | 149.15 | 29.99 | 59.44 | 0.399 |
| 6_2_05 | 5 | 149.23 | 28.35 | 59.91 | 0.401 |
| 6_2_10 | 10 | 149.32 | 28.48 | 59.27 | 0.396 |
| 6_2_11 | 10 | 149.01 | 27.57 | 60.13 | 0.403 |
| 6_2_08 | 15 | 149.18 | 28.09 | 60.06 | 0.403 |
| 6_2_09 | 15 | 149.28 | 28.70 | 59.96 | 0.402 |
| 6_2_06 | 20 | 149.21 | 28.33 | 60.01 | 0.402 |
| 6_2_07 | 20 | 149.12 | 28.45 | 60.03 | 0.403 |
| 6_2_03 | 25.2 | 149.18 | 28.45 | 59.81 | 0.400 |
| 6_2_04 | 25.2 | 149.23 | 28.96 | 59.93 | 0.402 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Miarka, P.; Seitl, S.; Bílek, V. Comparison of Fracture Resistance of the Normal and High Strength Concrete Evaluated by Brazilian Disc Test. Proceedings 2018, 2, 399. https://doi.org/10.3390/ICEM18-05236
Miarka P, Seitl S, Bílek V. Comparison of Fracture Resistance of the Normal and High Strength Concrete Evaluated by Brazilian Disc Test. Proceedings. 2018; 2(8):399. https://doi.org/10.3390/ICEM18-05236
Chicago/Turabian StyleMiarka, Petr, Stanislav Seitl, and Vlastimil Bílek. 2018. "Comparison of Fracture Resistance of the Normal and High Strength Concrete Evaluated by Brazilian Disc Test" Proceedings 2, no. 8: 399. https://doi.org/10.3390/ICEM18-05236
APA StyleMiarka, P., Seitl, S., & Bílek, V. (2018). Comparison of Fracture Resistance of the Normal and High Strength Concrete Evaluated by Brazilian Disc Test. Proceedings, 2(8), 399. https://doi.org/10.3390/ICEM18-05236





