Variable Stiffness Composites: Optimal Design Studies
Abstract
:1. Introduction
2. Materials and Methods
2.1. Static Buckling, Free Vibrations, and Static Analyses
Variable Stiffness Composites
2.2. First Order Shear Deformation Theory and Constitutive Relation
2.3. Optimization
| Algorithm 1: ASO |
| 1: Define optimization parameters |
| 2: While Candidates not stable Do |
| 3: OSF Procedure |
| 4: While Candidates not good Do |
| 5: Kriging response surface Procedure |
| 6: MISQP Procedure |
| 7: If Stop criteria is false Do |
| 8: Evaluate if candidates are good |
| 9: Else Stop (algorithm not converged) |
| 10: End |
| 11: End |
| 12: Evaluate candidate’s stability |
| 13: If Candidates not stable Do |
| 14: Domain reduction Procedure around candidates |
| 15: End |
| 16: End (algorithm converged) |
3. Verification Applications
3.1. Case 1: Natural Frequencies of Isotropic Plates
3.2. Case 2: Buckling Critical Loads of Constant Stiffness Composite Plates
3.3. Case 3: Natural Frequencies of Three-Layer Variable Stiffness Composite Plates
4. Numerical Applications
4.1. Static and Buckling Analyses of Three-Layer Variable Stiffness Composite Plates
4.2. Optimization
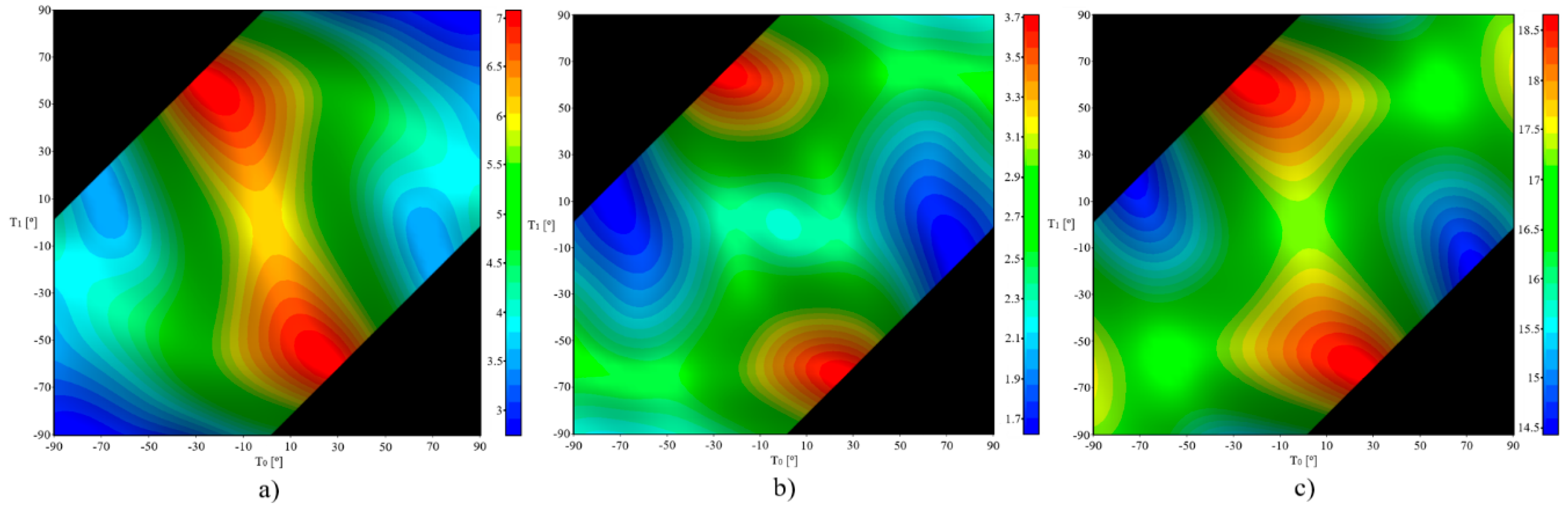
4.2.1. Single Layer Variable Stiffness Composite Plates
4.2.2. Three-Layer Variable Stiffness Composite Plates
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Xu, Y.; Zhu, J.; Wu, Z.; Cao, Y.; Zhao, Y.; Zhang, W. A review on the design of laminated composite structures: Constant and variable stiffness design and topology optimization. Adv. Compos. Hybrid Mater. 2018, 1, 460–477. [Google Scholar] [CrossRef]
- Lozano, G.G.; Tiwari, A.; Turner, C.; Astwood, S. A review on design for manufacture of variable stiffness composite laminates. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2015, 230, 981–992. [Google Scholar] [CrossRef]
- Setoodeh, S.; Gurdal, Z.; Watson, L.T. Design of variable-stiffness composite layers using cellular automata. Comput. Methods Appl. Mech. Eng. 2006, 195, 836–851. (In English) [Google Scholar] [CrossRef]
- Abdalla, M.M.; Setoodeh, S.; Gürdal, Z. Design of variable stiffness composite panels for maximum fundamental frequency using lamination parameters. Compos. Struct. 2007, 81, 283–291. [Google Scholar] [CrossRef]
- Setoodeh, S.; Abdalla, M.M.; Jsselmuiden, S.T.I.; Gürdal, Z. Design of variable-stiffness composite panels for maximum buckling load. Compos. Struct. 2009, 87, 109–117. [Google Scholar] [CrossRef]
- Lopes, C.S.; Gürdal, Z.; Camanho, P.P. Variable-stiffness composite panels: Buckling and first-ply failure improvements over straight-fibre laminates. Comput. Struct. 2008, 86, 897–907. (In English) [Google Scholar] [CrossRef]
- Gürdal, Z.; Tatting, B.F.; Wu, C.K. Variable stiffness composite panels: Effects of stiffness variation on the in-plane and buckling response. Compos. Part A Appl. Sci. Manuf. 2008, 39, 911–922. [Google Scholar] [CrossRef]
- Hao, P.; Yuan, X.; Liu, H.; Wang, B.; Liu, C.; Yang, D.; Zhan, S. Isogeometric buckling analysis of composite variable-stiffness panels. Compos. Struct. 2017, 165, 192–208. [Google Scholar] [CrossRef]
- Honda, S.; Oonishi, Y.; Narita, Y.; Sasaki, K. Vibration Analysis of Composite Rectangular Plates Reinforced along Curved Lines. J. Syst. Des. Dyn. 2008, 2, 76–86. [Google Scholar] [CrossRef] [Green Version]
- Houmat, A. Three-dimensional free vibration analysis of variable stiffness laminated composite rectangular plates. Compos. Struct. 2018, 194, 398–412. [Google Scholar] [CrossRef]
- Akhavan, H.; Ribeiro, P.; de Moura, M.F.S.F. Large deflection and stresses in variable stiffness composite laminates with curvilinear fibres. Int. J. Mech. Sci. 2013, 73, 14–26. [Google Scholar] [CrossRef]
- Venkatachari, A.; Natarajan, S.; Ganapathi, M. Variable stiffness laminated composite shells—Free vibration characteristics based on higher-order structural theory. Compos. Struct. 2018, 188, 407–414. [Google Scholar] [CrossRef]
- Sarvestani, H.Y.; Akbarzadeh, A.H.; Hojjati, M. Hygro-thermo-mechanical analysis of fiber-steered composite conical panels. Compos. Struct. 2017, 179, 146–160. [Google Scholar] [CrossRef]
- Demir, E.; Yousefi-Louyeh, P.; Yildiz, M. Design of variable stiffness composite structures using lamination parameters with fiber steering constraint. Compos. Part B Eng. 2019, 165, 733–746. [Google Scholar] [CrossRef]
- Shafighfard, T.; Demir, E.; Yildiz, M. Design of fiber-reinforced variable-stiffness composites for different open-hole geometries with fiber continuity and curvature constraints. Compos. Struct. 2019, 226, 111280. [Google Scholar] [CrossRef]
- Hao, P.; Liu, D.; Wang, Y.; Liu, X.; Wang, B.; Li, G.; Feng, S. Design of manufacturable fiber path for variable-stiffness panels based on lamination parameters. Compos. Struct. 2019, 219, 158–169. [Google Scholar] [CrossRef]
- Murugan, S.; Flores, E.I.S.; Adhikari, S.; Friswell, M.I. Optimal design of variable fiber spacing composites for morphing aircraft skins. Compos. Struct. 2012, 94, 1626–1633. [Google Scholar] [CrossRef]
- Murugan, S.; Friswell, M.I. Morphing wing flexible skins with curvilinear fiber composites. Compos. Struct. 2013, 99, 69–75. [Google Scholar] [CrossRef]
- Wu, Z.; Weaver, P.M.; Raju, G.; Kim, B.C. Buckling analysis and optimisation of variable angle tow composite plates. Thin-Walled Struct. 2012, 60, 163–172. [Google Scholar] [CrossRef] [Green Version]
- Reddy, J.N. Mechanics of Laminated Composite Plates and Shells: Theory and Analysis, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method, 5th ed.; Butterworth-Heinemann: Oxford, UK, 2000; Volume 1. [Google Scholar]
- Gürdal, Z.; Olmedo, R. Composite laminates with spatially varying fiber orientations—‘Variable stiffness panel concept’. In Proceedings of the 33rd Structures, Structural Dynamics and Materials Conference, Dallas, TX, USA, 13–15 April 1992. [Google Scholar]
- Waldhart, C.; Gurdal, Z.; Ribbens, C. Analysis of tow placed, parallel fiber, variable stiffness laminates. In Proceedings of the 37th Structure, Structural Dynamics and Materials Conference, Salt Lake City, UT, USA, 15–17 April 1996. [Google Scholar]
- Reissner, E. On the theory of transverse bending of elastic plates. Int. J. Solids Struct. 1976, 12, 545–554. [Google Scholar] [CrossRef]
- Mindlin, R.D. Influence of rotary inertia and shear on flexural motions of isotropic elastic plates. J. Appl. Mech. Asme 1951, 18, 31–38. [Google Scholar]
- Loja, M.A.R.; Barbosa, J.I.; Soares, C.M.M. Dynamic instability of variable stiffness composite plates. Compos. Struct. 2017, 182, 402–411. [Google Scholar] [CrossRef]
- Mota, A.F.; Loja, M.A.R.; Barbosa, J.I.; Rodrigues, J.A. Porous functionally graded plates: An assessment of shear correction factor influence on static behavior. Math. Comput. Appl. 2020, 25, 25. [Google Scholar]
- Moré, J.J.; Wright, S.J. Optimization Software Guide; Frontiers in Applied Mathematics; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1993. [Google Scholar]
- Yang, X.; He, X. Introduction to Optimization; Springer Nature: Basel, Switzerland, 2019. [Google Scholar]
- ANSYS DesignXplorer User’s Guide; ANSYS, Inc.: Fort Williams, PA, USA, 2020.
- Silva, T.A.N.; Loja, M.A.R.; Maia, N.M.M.; Barbosa, J.I. A hybrid procedure to identify the optimal stiffness coefficients of elastically restrained beams. Int. J. Appl. Math. Comput. Sci. 2015, 25, 245–257. [Google Scholar] [CrossRef] [Green Version]
- MathWorks. Available online: https://www.mathworks.com/ (accessed on 23 June 2020).
- Phan, N.D.; Reddy, J.N. Analysis of Laminated Composite Plates Using a Higher-Order Shear Deformation-Theory. Int. J. Numer. Methods Eng. 1985, 21, 2201–2219. [Google Scholar] [CrossRef]
- Akhavan, H.; Ribeiro, P. Natural modes of vibration of variable stiffness composite laminates with curvilinear fibers. Compos. Struct. 2011, 93, 3040–3047. (In English) [Google Scholar] [CrossRef]






| Mode | SSSS | CCCC | SFSF | CFCF | ||||
|---|---|---|---|---|---|---|---|---|
| CLPT | FSDT | CLPT | FSDT | CLPT | FSDT | CLPT | FSDT | |
| 1 | 19.737 (0.010) | 19.739 | 35.975 (0.031) | 36.006 | 3.367 (0.089) | 3.367 | 6.920 (0.058) | 6.923 |
| 2 | 49.337 (0.022) | 49.350 | 73.364 (0.042) | 73.483 | 17.318 (0.046) | 17.316 | 23.908 (0.063) | 23.914 |
| 3 | 49.337 (0.022) | 49.350 | 73.364 (0.042) | 73.483 | 19.293 (0.078) | 19.293 | 26.584 (0.026) | 26.590 |
| 4 | 78.922 (0.044) | 78.959 | 108.127 (0.084) | 108.629 | 38.214 (0.196) | 38.211 | 47.655 (0.031) | 47.675 |
| 5 | 98.666 (0.642) | 98.695 | 131.508 (0.206) | 131.755 | 51.044 (0.513) | 51.036 | 62.710 (0.223) | 62.718 |
| 6 | 98.666 (0.642) | 98.695 | 132.138 (0.205) | 132.471 | 53.492 (0.705) | 53.488 | 65.536 (0.227) | 65.557 |
| Mode | SSSS | CCCC | SFSF | CFCF | ||||
|---|---|---|---|---|---|---|---|---|
| CLPT | FSDT | CLPT | FSDT | CLPT | FSDT | CLPT | FSDT | |
| 1 | 71.552 (0.004) | 71.585 | 147.757 | 147.939 | 8.247 (0.243) | 8.247 | 24.830 (0.068) | 24.831 |
| 2 | 101.154 (0.010) | 101.202 | 173.758 | 173.957 | 29.568 (0.125) | 29.571 | 44.562 (0.079) | 44.565 |
| 3 | 150.490 (0.333) | 150.595 | 221.295 | 221.568 | 64.478 (0.820) | 64.492 | 81.496 (0.214) | 81.519 |
| 4 | 219.559 (1.530) | 219.812 | 291.595 | 292.084 | 98.684 (0.154) | 98.737 | 135.810 (1.769) | 135.910 |
| 5 | 256.587 (0.009) | 257.093 | 384.200 | 385.100 | 117.791 (2.473) | 117.857 | 142.475 (3.188) | 142.607 |
| 6 | 286.171 (0.017) | 286.711 | 394.201 | 395.538 | 125.624 (0.705) | 125.690 | 165.002 (0.236) | 165.144 |
| E1/E2 | Present FSDT | HSDT | |
|---|---|---|---|
| k Calculated | k = 5/6 | [33] | |
| 10 | 10.061 (2.936) | 10.076 (3.090) | 9.774 |
| 20 | 15.606 (2.013) | 15.633 (2.190) | 15.298 |
| 30 | 20.186 (1.147) | 20.235 (1.393) | 19.957 |
| 40 | 24.045 (3.021) | 24.131 (3.389) | 23.34 |
| a (m) | h (m) | E1 (GPa) | E2 (GPa) | G12 (GPa) | ν12 | ρ (kg·m−1) |
|---|---|---|---|---|---|---|
| 1 | 0.01 | 173 | 7.2 | 3.76 | 0.29 | 1540 |
| Stacking | Models | ω (rad·s−1) | |||||
|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | ||
| (<0|45>, <−45|−60>, <0|45>) | Present | 355.30 | 586.66 | 958.69 | 1070.28 | 1317.52 | 1464.42 |
| [26] | 347.1 (2.36) | 576.1 (1.83) | 949.31 (0.99) | 1066.64 (0.34) | 1300.47 (1.31) | 1457.21 (0.50) | |
| [34] | 358.49 (0.89) | 589.9 (0.55) | 960.36 (0.17) | 1075.21 (0.46) | 1327.88 (0.78) | 1474.67 (0.70) | |
| (<30|0>, <45|90>, <30|0>) | Present | 308.66 | 503.63 | 845.09 | 1130.41 | 1276.93 | 1305.21 |
| [26] | 308.03 (0.20) | 502.03 (0.32) | 842.79 (0.27) | 1133.79 (0.30) | 1277.26 (0.03) | 1300.73 (0.34) | |
| [34] | 308.8 (0.05) | 503.8 (0.03) | 845.51 (0.05) | 1131.31 (0.08) | 1279.85 (0.23) | 1307.4 (0.17) | |
| (<90|45>, <60|30>, <90|45>) | Present | 326.20 | 533.07 | 880.53 | 1082.91 | 1259.90 | 1388.02 |
| [26] | 320.05 (1.92) | 521.15 (2.29) | 877.23 (0.38) | 1084.42 (0.14) | 1238.85 (1.70) | 1395.8 (0.56) | |
| [34] | 329.69 (1.06) | 539.41 (1.18) | 886.39 (0.66) | 1091.2 (0.76) | 1279.9 (1.56) | 1401.87 (0.99) | |
| Layers | Boundary Condition | ||||||
|---|---|---|---|---|---|---|---|
| SSSS | CCCC | SSFF | CCFF | SFSF | CFCF | ||
| Pressure (kPa) | 1 | 1 | 1 | 0.1 | 0.1 | 0.01 | 0.1 |
| 3 | 10 | 10 | 1 | 1 | 0.1 | 1 | |
| Stacking | Boundary Condition | |||||
|---|---|---|---|---|---|---|
| SSSS | CCCC | SSFF | CCFF | SFSF | CFCF | |
| (<0|45>, <−45|−60>, <0|45>) | 7.894 | 3.005 | 1.456 | 0.411 | 8.571 | 10.834 |
| (<30|0>, <45|90>, <30|0>) | 10.83 | 2.285 | 2.167 | 0.339 | 15.657 | 7.100 |
| (<90|45>, <60|30>, <90|45>) | 9.736 | 2.016 | 18.951 | 1.938 | 10.382 | 9.388 |
| Stacking | Loading | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|---|
| (<0|45>, <−45|−60>, <0|45>) | Uniaxial | 2.12 | 3.865 | 5.519 | 6.625 | 7.337 | 8.122 |
| Biaxial | 1.091 | 1.208 | 1.678 | 2.384 | 3.08 | 3.203 | |
| (<30|0>, <45|90>, <30|0>) | Uniaxial | 1.451 | 3.091 | 4.765 | 5.651 | 6.895 | 7.987 |
| Biaxial | 0.794 | 0.831 | 1.218 | 1.742 | 2.327 | 2.880 | |
| (<90|45>, <60|30>, <90|45>) | Uniaxial | 1.006 | 1.132 | 1.674 | 1.937 | 2.684 | 3.153 |
| Biaxial | 0.658 | 0.750 | 1.148 | 1.585 | 2.083 | 2.295 |
| Properties | SSSS | SSFF | SFSF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 19.124 | 0 | 0.005 | −45 | −41.002 |
| T1 (°) | 0 | −62.531 | 0 | 0.017 | −45 | −50.329 |
| (mm) | 25.118 | 21.526 | 2.540 | 2.540 | 11.963 | 11.843 |
| Decrease (%) | - | 14.301 | - | 0 | - | 1.003 |
| Properties | CCCC | CCFF | CFCF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 0.015 | 0 | 0.000 | 78.174 | 30.998 |
| T1 (°) | 0 | −0.004 | 0 | −0.001 | 78.174 | −1.155 |
| (mm) | 5.109 | 5.109 | 0.501 | 0.501 | 20.101 | 19.193 |
| Decrease (%) | - | 0 | - | 0 | - | 4.517 |
| Properties | SSSS | SSFF | SFSF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 22.828 | 0 | 0 | −45 | −40.493 |
| T1 (°) | 0 | −62.043 | 0 | 0 | −45 | −51.166 |
| (Hz) | 17.224 | 18.630 | 16.032 | 16.032 | 2.932 | 2.950 |
| Increase (%) | - | 8.164 | - | 0 | - | 0.614 |
| Properties | CCCC | CCFF | CFCF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | −90 | 0 | 0 | 76.852 | −40.493 |
| T1 (°) | 0 | −1.680 | 0 | 0 | 76.852 | −51.166 |
| (Hz) | 37.664 | 39.498 | 36.324 | 36.324 | 6.671 | 2.950 |
| Increase (%) | - | 4.869 | - | 0 | - | 4.062 |
| Properties | SSSS | SSFF | SFSF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 23.057 | 0 | 0 | 43.256 | 34.701 |
| T1 (°) | 0 | −57.401 | 0 | 0 | 43.256 | 49.791 |
| 6.091 | 7.063 | 5.278 | 5.278 | 0.319 | 0.325 | |
| Increase (%) | - | 15.958 | - | 0 | - | 1.881 |
| Properties | CCCC | CCFF | CFCF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 0 | 0 | 0 | 12.386 | 15.750 |
| T1 (°) | 0 | 0 | 0 | 0 | 12.386 | 7.048 |
| 22.672 | 22.672 | 21.098 | 21.098 | 1.881 | 1.891 | |
| Increase (%) | - | 0 | - | 0 | - | 1.891 |
| Properties | SSSS | SSFF | SFSF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 69.748 | 27.047 | 0 | 0 | −45 | −43.145 |
| T1 (°) | 69.748 | −66.919 | 0 | 0 | −45 | −47.282 |
| 2.594 | 3.721 | 1.331 | 1.331 | 0.175 | 0.175 | |
| Increase (%) | - | 43.448 | - | 0 | - | 0 |
| Properties | CCCC | CCFF | CFCF | |||
|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | |
| T0 (°) | 0 | 10.852 | 16.874 | 79.914 | 45 | 60.389 |
| T1 (°) | 0 | −83.098 | 16.874 | −14.036 | 45 | 39.411 |
| 5.699 | 6.659 | 2.890 | 3.626 | 0.987 | 1.033 | |
| Increase (%) | - | 16.845 | - | 25.467 | - | 4.661 |
| Layer | Properties | SSSS | SSFF | SFSF | |||
|---|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | ||
| 1 | T0 (°) | −45 | −25.008 | 0 | 0 | 45 | 47.528 |
| T1 (°) | −45 | 62.886 | 0 | 0 | 45 | 42.625 | |
| 2 | T0 (°) | 45 | 26.499 | 0 | 0 | 45 | 49.833 |
| T1 (°) | 45 | 41.007 | 0 | 0 | 45 | 40.039 | |
| 3 | T0 (°) | −45 | −15.557 | 0 | 0 | −45 | −42.512 |
| T1 (°) | −45 | −54.835 | 0 | 0 | −45 | −47.796 | |
| (mm) | 8.529 | 7.569 | 0.945 | 0.945 | 2.718 | 2.704 | |
| Decrease (%) | - | 11.2578 | - | 0 | - | 0.515123 | |
| Layer | Properties | CCCC | CCFF | CFCF | |||
|---|---|---|---|---|---|---|---|
| CS | VS | CS | VS | CS | VS | ||
| 1 | T0 (°) | 0 | 90 | 0 | 0 | 12.422 | 36.051 |
| T1 (°) | 0 | 90 | 0 | 0 | 12.422 | 0.913 | |
| 2 | T0 (°) | 0 | −90 | 0 | 0 | 46.505 | −70.004 |
| T1 (°) | 0 | −90 | 0 | 0 | 46.505 | −35.080 | |
| 3 | T0 (°) | 0 | 90 | 0 | 0 | 12.419 | 36.047 |
| T1 (°) | 0 | 90 | 0 | 0 | 12.419 | 0.917 | |
| (mm) | 1.930 | 1.930 | 0.189 | 0.189 | 7.458 | 6.667 | |
| Decrease (%) | - | 0 | - | 0 | - | 10.605 | |
| Layer | Properties | Fundamental Frequency | |
|---|---|---|---|
| CS | VS | ||
| 1 | T0 (°) | −45 | 16.715 |
| T1 (°) | −45 | −60.572 | |
| 2 | T0 (°) | 45 | 57.990 |
| T1 (°) | 45 | 38.114 | |
| 3 | T0 (°) | −45 | 16.720 |
| T1 (°) | −45 | −60.573 | |
| (Hz) | 54.937 | 58.016 | |
| Increase (%) | - | 5.605 | |
| Layer | Properties | Uniaxial Buckling | Biaxial Buckling | ||
|---|---|---|---|---|---|
| CS | VS | CS | VS | ||
| 1 | T0 (°) | −6.925 | 30.476 | −45 | 23.369 |
| T1 (°) | −6.925 | −59.626 | −45 | −62.232 | |
| 2 | T0 (°) | 41.121 | 71.359 | 45 | −19.928 |
| T1 (°) | 41.121 | 41.604 | 45 | 73.375 | |
| 3 | T0 (°) | −6.925 | 30.473 | −45 | 23.355 |
| T1 (°) | −6.925 | −59.626 | −45 | −62.236 | |
| 1.698 | 2.565 | 0.818 | 1.293 | ||
| Increase (%) | - | 51.128 | - | 58.005 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marques, F.E.C.; Mota, A.F.S.d.; Loja, M.A.R. Variable Stiffness Composites: Optimal Design Studies. J. Compos. Sci. 2020, 4, 80. https://doi.org/10.3390/jcs4020080
Marques FEC, Mota AFSd, Loja MAR. Variable Stiffness Composites: Optimal Design Studies. Journal of Composites Science. 2020; 4(2):80. https://doi.org/10.3390/jcs4020080
Chicago/Turabian StyleMarques, Filipe Eduardo Correia, Ana Filipa Santos da Mota, and Maria Amélia Ramos Loja. 2020. "Variable Stiffness Composites: Optimal Design Studies" Journal of Composites Science 4, no. 2: 80. https://doi.org/10.3390/jcs4020080





