Simplified Approach to Nonlinear Vibration Analysis of Variable Stiffness Plates
Abstract
:1. Introduction
2. Formulation
2.1. Kinematics and Generalized Strains
2.2. Generalized Forces
2.3. Variational Framework
2.4. Boundary Conditions
3. Approximate Solution
3.1. Spatial Approximation via Ritz Method
3.2. Differential Quadrature Method
3.3. Arc-Length Harmonic Balance Method
3.4. Single-Mode Solution
4. Results
4.1. Validation
4.1.1. Linear Vibrations
4.1.2. Nonlinear Vibrations
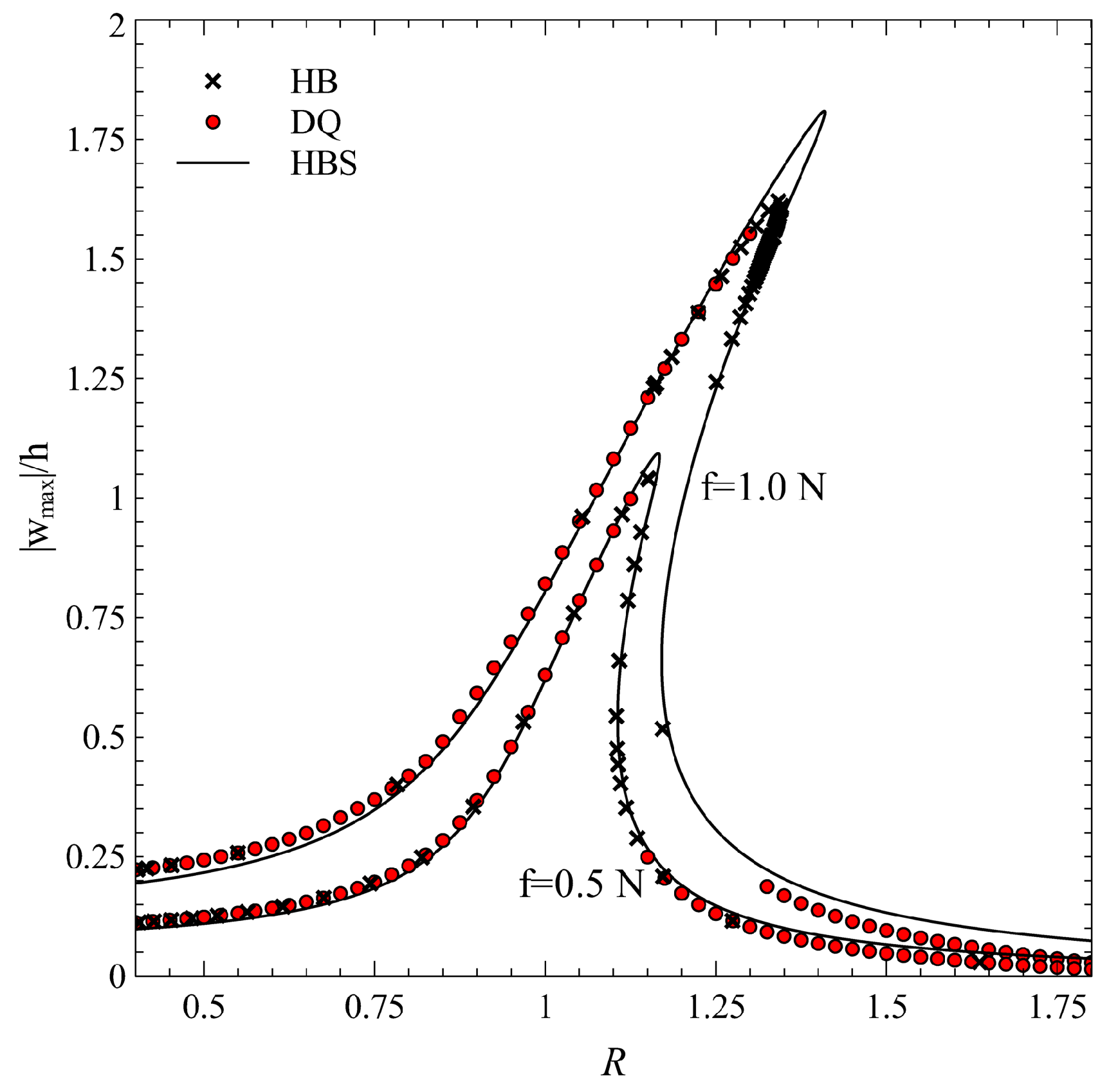
4.2. Comparison between Methods
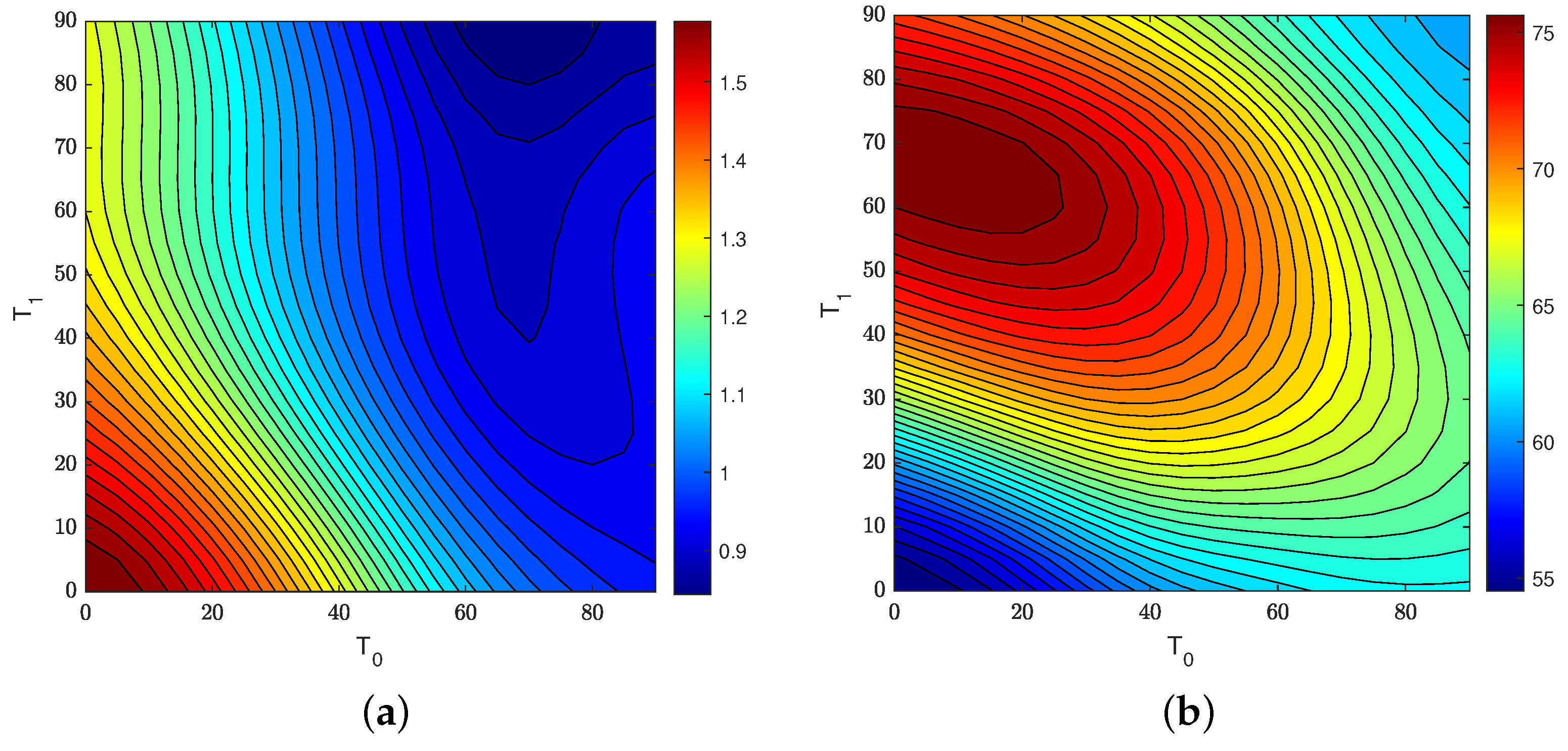
4.3. Parametric Studies
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Olmedo, R.; Gürdal, Z. Buckling response of laminates with spatially varying fiber orientations. In Proceedings of the 34th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Material Conference, AIAA-93-1567-CP, La Jolla, CA, USA, 19–22 April 1993; pp. 2261–2269. [Google Scholar]
- Wu, Z.; Weaver, P.; Raju, G.; Kim, B. Buckling analysis and optimisation of variable angle tow composite plates. Thin-Walled Struct. 2012, 60, 163–172. [Google Scholar] [CrossRef] [Green Version]
- Vescovini, R.; Dozio, L. Thermal buckling behaviour of thin and thick variable-stiffness panels. J. Compos. Sci. 2018, 2, 58. [Google Scholar] [CrossRef] [Green Version]
- Wu, Z.; Raju, G.; Weaver, P. Postbuckling analysis of variable angle tow composite plates. Int. J. Solids Struct. 2013, 50, 1770–1780. [Google Scholar] [CrossRef] [Green Version]
- Vescovini, R.; Spigarolo, E.; Jansen, E.; Dozio, L. Efficient post-buckling analysis of variable-stiffness plates using a perturbation approach. Thin-Walled Struct. 2019, 143, 106211. [Google Scholar] [CrossRef]
- Vescovini, R.; Dozio, L. A variable-kinematic model for variable stiffness plates: Vibration and buckling analysis. Compos. Struct. 2016, 142, 15–26. [Google Scholar] [CrossRef]
- Ribeiro, P.; Akhavan, H. Nonlinear vibrations of variable stiffness composite laminated plates. Compos. Struct. 2012, 94, 2424–2432. [Google Scholar] [CrossRef]
- Amabili, M. Nonlinear Vibrations and Stability of Shells and Plates; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Amabili, M. Nonlinear vibrations of rectangular plates with different boundary conditions: Theory and experiments. Comput. Struct. 2004, 82, 2587–2605. [Google Scholar] [CrossRef]
- Amabili, M. Nonlinear vibrations of laminated circular cylindrical shells: Comparison of different shell theories. Compos. Struct. 2011, 94, 207–220. [Google Scholar] [CrossRef]
- Farbod, F.; Amabili, M. Nonlinear vibrations of shells: A literature review from 2003 to 2013. Int. J. Non-Linear Mech. 2014, 58, 233–257. [Google Scholar]
- Chu, H.N.; Herrmann, G. Influence of large amplitude on free flexural vibrations of rectangular elastic plates. J. Appl. Mech. 1956, 23, 532–540. [Google Scholar] [CrossRef]
- Hui, D. Effects of geometric imperfections on large-amplitude vibrations of rectangular plates with hysteresis damping. J. Appl. Mech. 1984, 51, 216–220. [Google Scholar] [CrossRef]
- Rehfield, L. Nonlinear free vibrations of elastic structures. Int. J. Solids Struct. 1973, 9, 581–590. [Google Scholar] [CrossRef]
- Mei, C. Finite element displacement method for large amplitude free flexural vibrations of beams and plates. Comput. Struct. 1973, 3, 163–174. [Google Scholar] [CrossRef]
- Shi, Y.; Lee, R.; Mei, C. Finite element method for nonlinear free vibrations of composite plates. AIAA J. 1997, 35, 159–166. [Google Scholar] [CrossRef]
- Lau, L.; Cheung, Y.; Wu, S. Nonlinear vibration of thin elastic plates, Part 1: Generalized incremental Hamilton’s principle and element formulation. J. Appl. Mech. 1984, 51, 837–844. [Google Scholar] [CrossRef]
- Ribeiro, P.; Petyt, M. Geometrical nonlinear, steady state, forced, periodic vibration of plates, part I: Model and convergence studies. J. Sound Vib. 1999, 226, 955–983. [Google Scholar] [CrossRef]
- Ribeiro, P.; Petyt, M. Geometrical nonlinear, steady state, forced, periodic vibration of plates, part II: Stability study and analysis of multi-modal response. J. Sound Vib. 1999, 226, 985–1010. [Google Scholar] [CrossRef]
- Ribeiro, P. Nonlinear free periodic vibrations of variable stiffness composite laminated plates. Nonlinear Dyn. 2012, 70, 1535–1548. [Google Scholar] [CrossRef]
- Ribeiro, P.; Akhavan, H.; Teter, A.; Warmiński, J. A review on the mechanical behaviour of curvilinear fibre composite laminated panels. J. Compos. Mater. 2013, 48, 2761–2777. [Google Scholar] [CrossRef]
- Houmat, A. Nonlinear free vibration of laminated composite rectangular plates with curvilinear fibers. Compos. Struct. 2013, 106, 211–224. [Google Scholar] [CrossRef]
- Prabhakara, M.; Chia, C. Nonlinear flexural vibrations of orthotropic rectangular plates. J. Sound Vib. 1977, 52, 511–518. [Google Scholar] [CrossRef]
- Jansen, E. A comparison of analytical–numerical models for nonlinear vibrations of cylindrical shells. Comput. Struct. 2004, 82, 2647–2658. [Google Scholar] [CrossRef]
- Yan, C.; Vescovini, R.; Jansen, E. A semi-analytical framework for nonlinear vibration analysis of variable stiffness plates. Compos. Struct. 2021, 269, 1–16. [Google Scholar] [CrossRef]
- Rega, G.; Saetta, E.; Settimi, V. Modeling and nonlinear dynamics of thermomechanically coupled composite plates. Int. J. Mech. Sci. 2020, 187. [Google Scholar] [CrossRef]
- Settimi, V.; Saetta, F.; Rega, G. Nonlinear dynamics of a third-order reduced model of thermomechanically coupled plate under different thermal excitations. Meccanica 2020, 55, 2451–2473. [Google Scholar] [CrossRef]
- Wu, Z.; Raju, G.; Weaver, P. Buckling of VAT plates using energy methods. In Proceedings of the 53rd AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics and Material Conference, AIAA 2012-1463, Honolulu, HI, USA, 23–26 April 2012. [Google Scholar]
- Reddy, J. Mechanics of Laminated Composite Plates and Shells: Theory and Analysis; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Reddy, J. A simple higher-order theory for laminated composite plates. J. Appl. Mech. 1984, 51, 745–752. [Google Scholar] [CrossRef]
- Bisagni, C.; Vescovini, R. Analytical formulation for local buckling and post-buckling analysis of stiffened laminated panels. Thin-Walled Struct. 2009, 47, 318–334. [Google Scholar] [CrossRef]
- Raju, G.; Wu, Z.; Weaver, P. Buckling and postbuckling of variable angle tow composite plates under in-plane shear loading. Int. J. Solids Struct. 2015, 58, 270–287. [Google Scholar] [CrossRef] [Green Version]
- Giavotto, V. Sulla Meccanica dei Pannelli Anisotropi ed Eterogenei. Mem. Dell’ Ist. Lomb. Accad. Sci. Lett. 1969, XXV, 437–480. (In Italian) [Google Scholar]
- Librescu, L.; Reddy, J. A geometrically nonlinear theory of transversely isotropic laminated composite plates and its use in the post-buckling analysis. Thin-Walled Struct. 1991, 11, 177–201. [Google Scholar] [CrossRef]
- Giavotto, V. Variational Theory of Thin Shells and Panels. Meccanica 1966, 1, 98–107. [Google Scholar] [CrossRef]
- Vescovini, R.; Dozio, L.; D’Ottavio, M.; Polit, O. On the application of the Ritz method to free vibration and buckling analysis of highly anisotropic plates. Compos. Struct. 2018, 192, 460–474. [Google Scholar] [CrossRef]
- Tornabene, F.; Fantuzzi, N.; Viola, E.; Carrera, E. Static analysis of doubly-curved anisotropic shells and panels using CUF approach, differential geometry and differential quadrature method. Compos. Struct. 2014, 107, 675–697. [Google Scholar] [CrossRef]
- Xing, Y.; Guo, J. Differential quadrature time element method for structural dynamics. Acta Mech. Sin. 2012, 28, 782–792. [Google Scholar] [CrossRef]
- Wu, T.; Liu, G. A differential quadrature as a numerical method to solve differential equations. Comput. Mech. 1999, 24, 197–205. [Google Scholar] [CrossRef]
- Nayfeh, A.; Balachandran, B. Applied Nonlinear Dynamics: Analytical, Computational, and Experimental Methods; John Wiley & Sons: New York, NY, USA, 2008. [Google Scholar]
- Mickens, R. Comments on the method of harmonic balance. J. Sound Vib. 1984, 94, 456–460. [Google Scholar] [CrossRef] [Green Version]
- Borst, R.D.; Crisfield, M.; Remmers, J.; Verhoosel, C. Non-Linear Finite Element Analysis of Solids and Structures; John Wiley & Sons: New York, NY, USA, 2012. [Google Scholar]
- Akhavan, H.; Ribeiro, P. Natural modes of vibration of variable stiffness composite laminates with curvilinear fibers. Compos. Struct. 2011, 93, 3040–3047. [Google Scholar] [CrossRef]







| (MPa) | (MPa) | , (MPa) | (MPa) | ||
|---|---|---|---|---|---|
| M1 | 1.00 | 0.60 | 0.50 | 0.25 | |
| M2 | 120,500 | 9630 | 3580 | 3580 | 0.32 |
| M3 | 173,000 | 7200 | 3760 | 3760 | 0.29 |
| M4 | 131,700 | 9860 | 4210 | 4210 | 0.28 |
| n = 48 | n = 108 | n = 363 | Exact [29] | ||
|---|---|---|---|---|---|
| 10 | 5 | ||||
| 10 | |||||
| 20 | 5 | ||||
| 10 | |||||
| 40 | 5 | ||||
| 10 |
| Ref. [21] | Ref. [43] | Ritz | Ritz | |||||
|---|---|---|---|---|---|---|---|---|
| n = 500 | n = 500 | n = 506 | n = 156 | |||||
| Mode | SSSS | CCCC | SSSS | CCCC | SSSS | CCCC | SSSS | CCCC |
| 1 | 355.41 | 567.56 | 358.49 | 579.40 | 357.30 | 575.62 | 360.70 | 578.08 |
| 2 | 600.50 | 831.39 | 589.90 | 821.53 | 589.12 | 818.39 | 595.32 | 821.76 |
| 3 | 986.65 | 1253.18 | 960.36 | 1225.79 | 960.46 | 1222.74 | 980.81 | 1254.50 |
| 4 | 1027.55 | 1448.46 | 1075.21 | 1493.76 | 1073.03 | 1479.45 | 1086.21 | 1500.97 |
| 5 | 1309.92 | 1719.96 | 1327.88 | 1726.96 | 1322.84 | 1713.21 | 1350.30 | 1764.76 |
| 6 | 1506.90 | 1818.98 | 1474.67 | 1775.16 | 1466.67 | 1771.14 | 1690.45 | 1908.24 |
| 7 | 1743.33 | 2175.80 | 1726.71 | 2135.76 | 1718.08 | 2121.56 | 2265.94 | 2220.54 |
| 8 | 2106.31 | 2505.73 | 2137.13 | 2443.53 | 2085.50 | 2437.05 | 2324.50 | 2772.34 |
| 9 | 2171.03 | 2750.46 | 2262.35 | 2706.78 | 2227.60 | 2690.78 | 2591.59 | 2947.36 |
| 0.2 | 0.6 | 1.0 | ||||
|---|---|---|---|---|---|---|
| HBS | Ref. [22] | HBS | Ref. [22] | HBS | Ref. [22] | |
| 0.316 | 0.325 | 0.335 | 0.345 | 0.370 | 0.383 | |
| 0.310 | 0.318 | 0.328 | 0.338 | 0.362 | 0.375 | |
| 0.302 | 0.308 | 0.319 | 0.328 | 0.351 | 0.364 | |
| 0.292 | 0.297 | 0.308 | 0.316 | 0.338 | 0.350 | |
| 0.282 | 0.287 | 0.297 | 0.304 | 0.325 | 0.335 | |
| 0.273 | 0.278 | 0.286 | 0.294 | 0.311 | 0.322 | |
| 0.267 | 0.272 | 0.279 | 0.287 | 0.300 | 0.313 | |
| 0.264 | 0.269 | 0.275 | 0.283 | 0.296 | 0.309 | |
| Time (s) | |||
|---|---|---|---|
| DQ | f = 0.5 N | 1.0427 | |
| HB | 1.0451 | ||
| HBS | 1.0875 | ||
| DQ | f = 1.0 N | 1.6502 | |
| HB | 1.6668 | ||
| HBS | 1.8080 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Andérez González, J.; Vescovini, R. Simplified Approach to Nonlinear Vibration Analysis of Variable Stiffness Plates. J. Compos. Sci. 2023, 7, 30. https://doi.org/10.3390/jcs7010030
Andérez González J, Vescovini R. Simplified Approach to Nonlinear Vibration Analysis of Variable Stiffness Plates. Journal of Composites Science. 2023; 7(1):30. https://doi.org/10.3390/jcs7010030
Chicago/Turabian StyleAndérez González, Jorge, and Riccardo Vescovini. 2023. "Simplified Approach to Nonlinear Vibration Analysis of Variable Stiffness Plates" Journal of Composites Science 7, no. 1: 30. https://doi.org/10.3390/jcs7010030






