Net-Baryon Probability Distributions from Lattice Simulations
Abstract
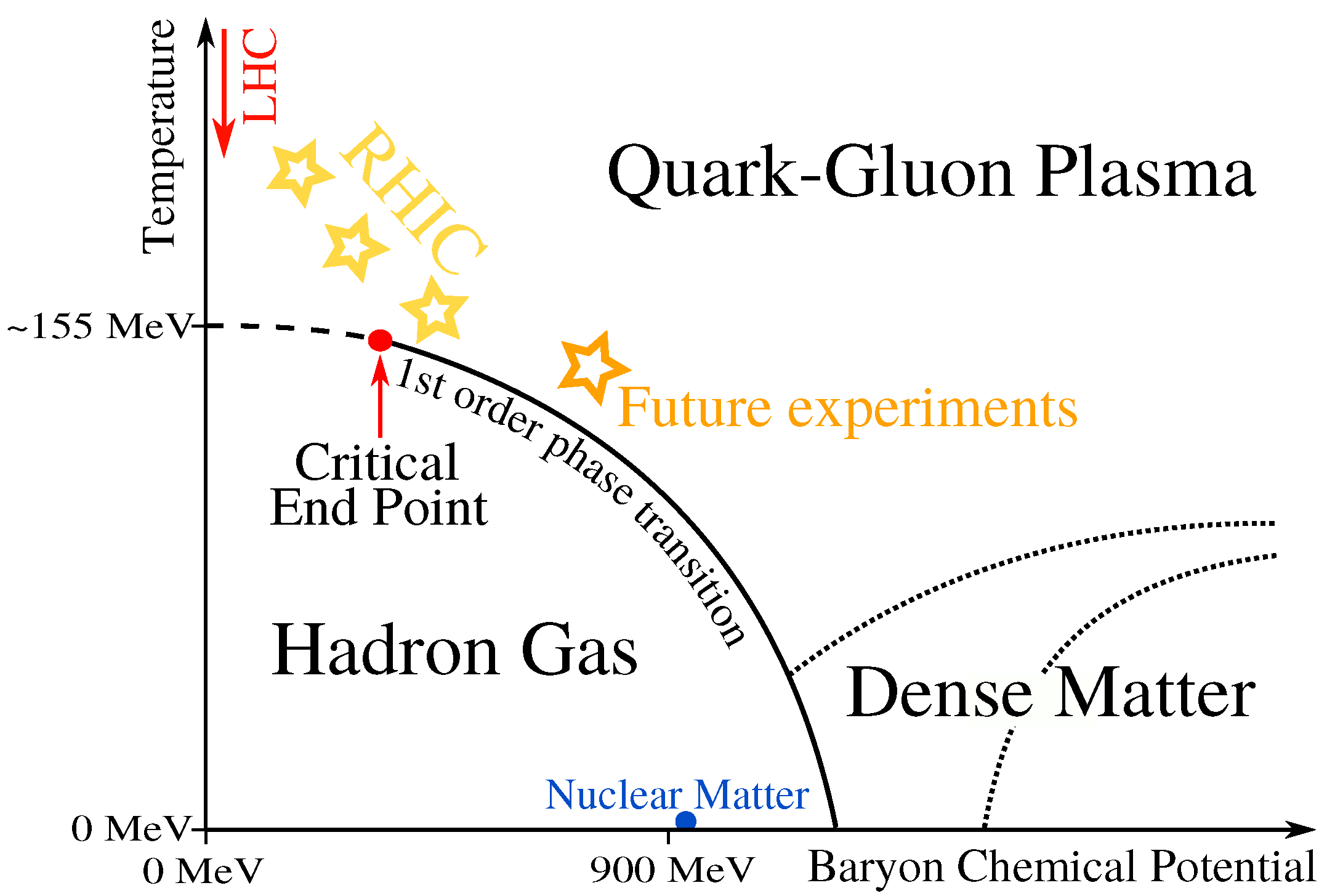
:1. Introduction
2. Definitions and Notation
3. Lattice Results
3.1.
3.2.
4. Equation of State
5. Asymptotic Behavior of the Net-Baryon Number Distributions
5.1.
5.2.
6. Discussion
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; Anderson, D.M.; Aparin, A.; et al. Nonmonotonic Energy Dependence of Net-Proton Number Fluctuations. Phys. Rev. Lett. 2021, 126, 092301. [Google Scholar] [CrossRef] [PubMed]
- Braun-Munzinger, P.; Friman, B.; Redlich, K.; Rustamov, A.; Stachel, J. Relativistic nuclear collisions: Establishing a non-critical baseline for fluctuation measurements. Nucl. Phys. A 2021, 1008, 122141. [Google Scholar] [CrossRef]
- Bornyakov, V.G.; Boyda, D.L.; Goy, V.A.; Molochkov, A.V.; Nakamura, A.; Nikolaev, A.A.; Zakharov, V.I. New approach to canonical partition functions computation in Nf = 2 lattice QCD at finite baryon density. Phys. Rev. D 2017, 95, 094506. [Google Scholar] [CrossRef]
- Roberge, A.; Weiss, N. Gauge Theories with Imaginary Chemical Potential and the Phases of QCD. Nucl. Phys. B 1986, 275, 734–745. [Google Scholar] [CrossRef]
- Nakamura, A.; Nagata, K. Probing QCD phase structure using baryon multiplicity distribution. Prog. Theor. Exp. Phys. 2016, 2016, 033D01. [Google Scholar] [CrossRef]
- Bzdak, A.; Koch, V. Net-baryon multiplicity distribution consistent with lattice QCD. Phys. Rev. C 2019, 99, 024913. [Google Scholar] [CrossRef]
- Begun, A.; Bornyakov, V.G.; Gerasimeniuk, N.V.; Goy, V.A.; Nakamura, A.; Rogalyov, R.N.; Vovchenko, V. Quark Density in Lattice QC2D at Imaginary and Real Chemical Potential. arXiv 2021, arXiv:2103.07442. [Google Scholar]
- Bazavov, A.; Bhattacharya, T.; DeTar, C.; Ding, H.T.; Gottlieb, S.; Gupta, R.; Hegde, P.; Heller, U.M.; Karsch, F.; Laermann, E.; et al. Equation of state in (2 + 1)-flavor QCD. Phys. Rev. D 2014, 90, 094503. [Google Scholar] [CrossRef]
- Taradiy, K.; Motornenko, A.; Vovchenko, V.; Gorenstein, M.I.; Stoecker, H. The analytic structure of thermodynamic systems with repulsive interactions. Phys. Rev. C 2019, 100, 065202. [Google Scholar] [CrossRef]
- Wakayama, M.; Nam, S.i.; Hosaka, A. Use of the canonical approach in effective models of QCD. Phys. Rev. D 2020, 102, 034035. [Google Scholar] [CrossRef]
- Bornyakov, V.G.; Gerasimeniuk, N.V.; Goy, V.A.; Korneev, A.A.; Molochkov, A.V.; Nakamura, A.; Rogalyov, R.N. Numerical study of the Roberge-Weiss transition. Phys. Rev. D 2023, 107, 014508. [Google Scholar] [CrossRef]
- Akhiezer, N.I. The Classical Moment Problem and Some Related Questions in Analysis; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2020. [Google Scholar] [CrossRef]
- Braun-Munzinger, P.; Redlich, K.; Stachel, J. Particle Production in Heavy Ion Collisions. In Quark–Gluon Plasma 3; Hwa, R., Wang, X.-N., Eds.; World Scientific: Singapore, 2004; pp. 491–599. [Google Scholar] [CrossRef]
- Braun-Munzinger, P.; Friman, B.; Karsch, F.; Redlich, K.; Skokov, V. Net-charge probability distributions in heavy ion collisions at chemical freeze-out. Nucl. Phys. A 2012, 880, 48–64. [Google Scholar] [CrossRef]
- Abdallah, M.S.; Adam, J.; Adamczyk, L.; Adams, J.R.; Adkins, J.K.; Agakishiev, G.; Aggarwal, I.; Aggarwal, M.M.; Ahammed, Z.; Alekseev, I.; et al. Cumulants and correlation functions of net-proton, proton, and antiproton multiplicity distributions in Au+Au collisions at energies available at the BNL Relativistic Heavy Ion Collider. Phys. Rev. C 2021, 104, 024902. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rogalyov, R.; Goy, V. Net-Baryon Probability Distributions from Lattice Simulations. Particles 2023, 6, 771-783. https://doi.org/10.3390/particles6030049
Rogalyov R, Goy V. Net-Baryon Probability Distributions from Lattice Simulations. Particles. 2023; 6(3):771-783. https://doi.org/10.3390/particles6030049
Chicago/Turabian StyleRogalyov, Roman, and Vladimir Goy. 2023. "Net-Baryon Probability Distributions from Lattice Simulations" Particles 6, no. 3: 771-783. https://doi.org/10.3390/particles6030049







