Analysing the Effect of Chassis Torsional Flexibility on the Rollover Threshold of a Multi-Purpose Agricultural Vehicle
Abstract
:1. Introduction
2. Materials and Methods
2.1. Reference Vehicle Description
2.2. Experimental Measurements Methodology
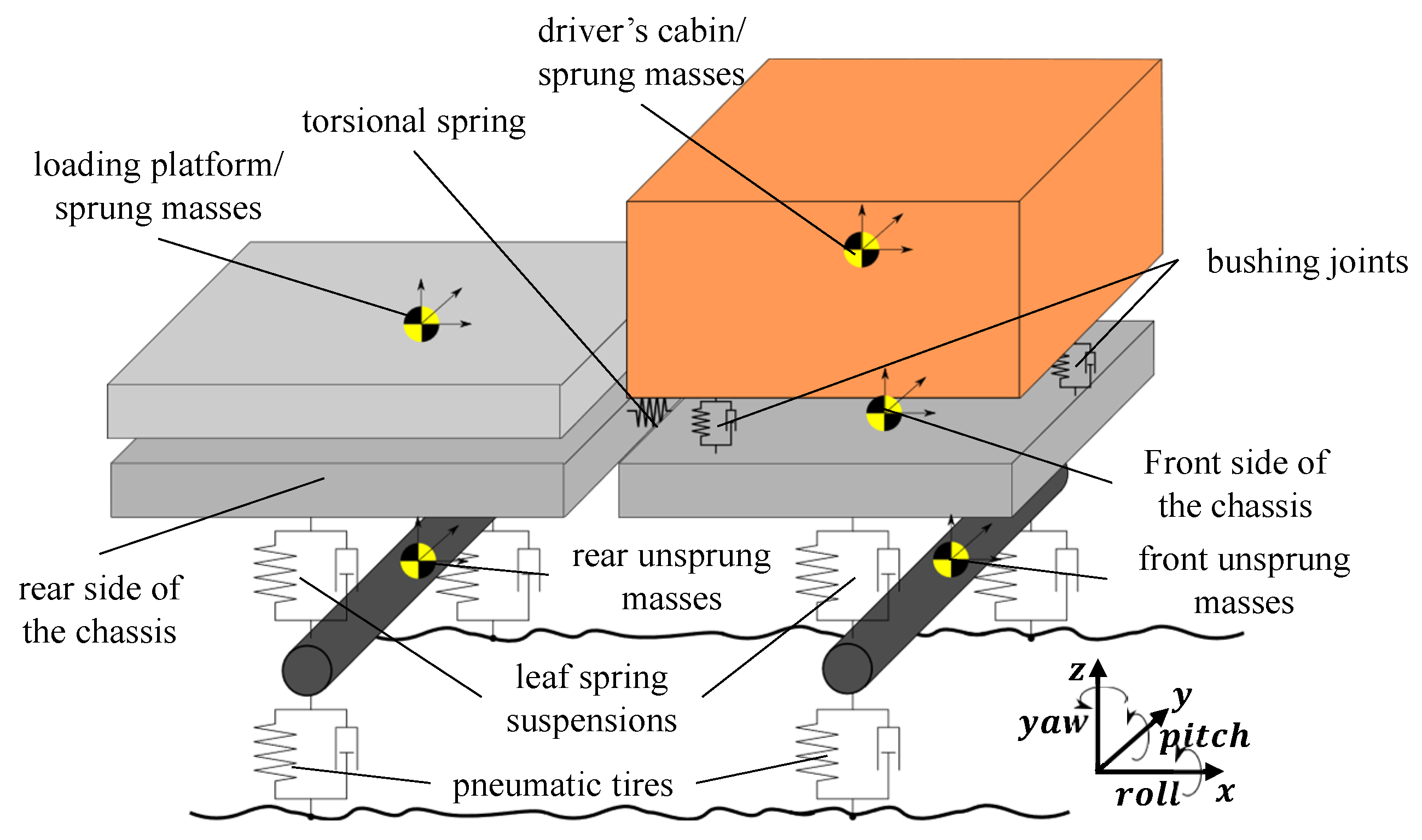
3. Vehicle Multi-Body Model
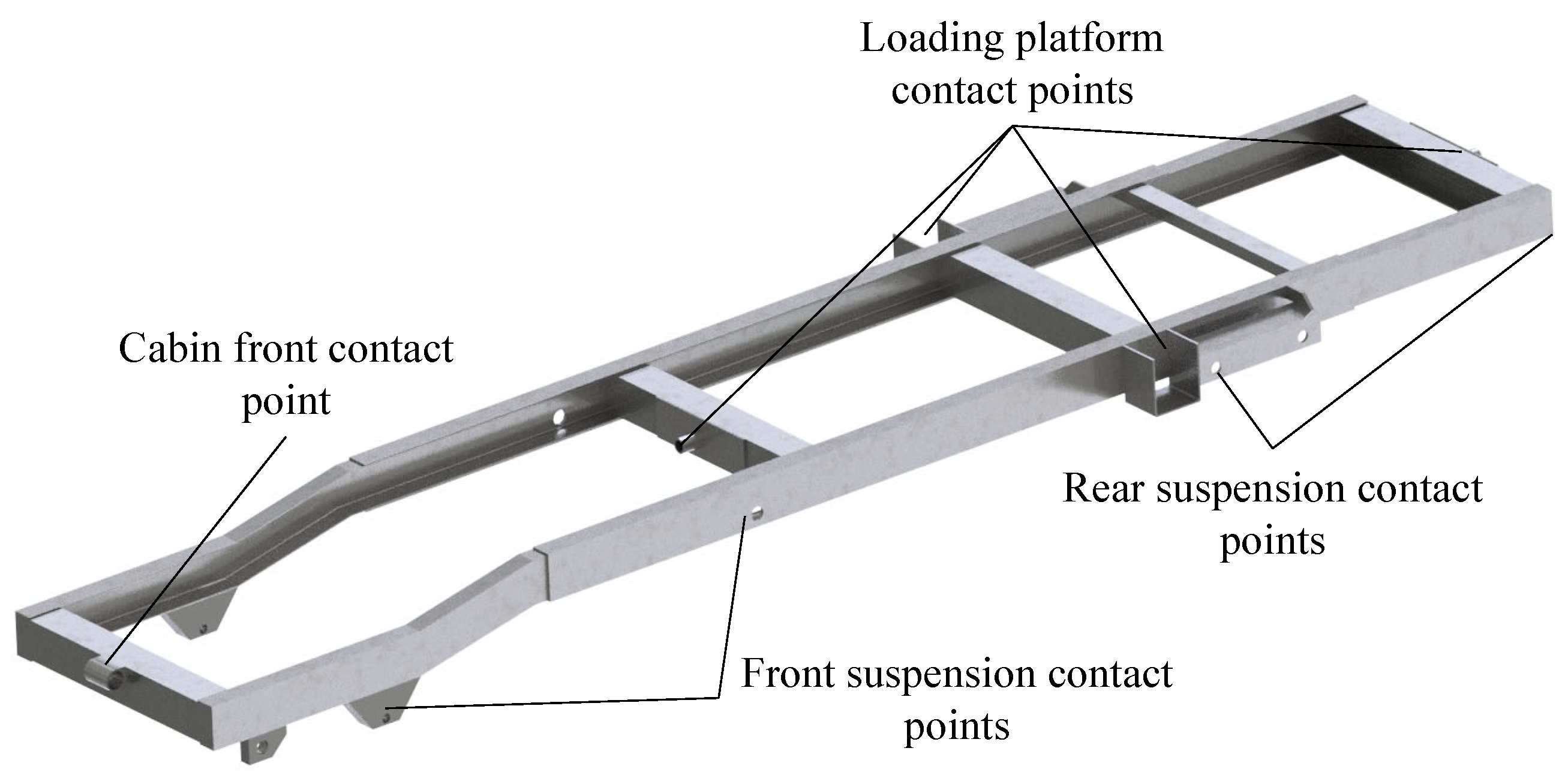
3.1. Vehicle Chassis Model
3.2. Vehicle Suspensions Model
3.3. Vehicle Tires Model
3.4. Modelling of Vehicle Unsprung Masses
4. Model Validation
4.1. Vehicle Model Mass Distribution Validation
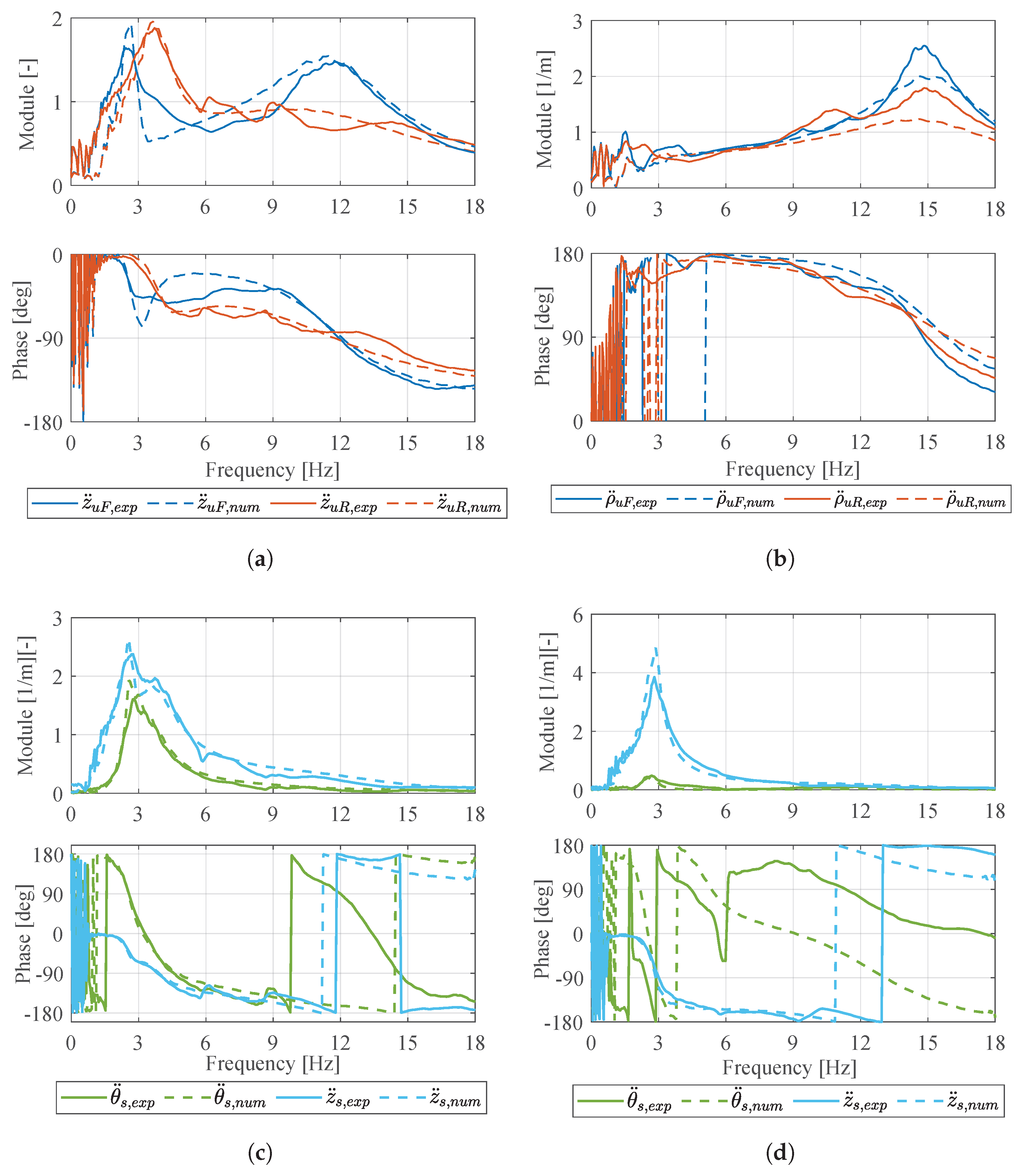
4.2. Validation of the Vehicle Model Dynamics
5. Results and Discussion
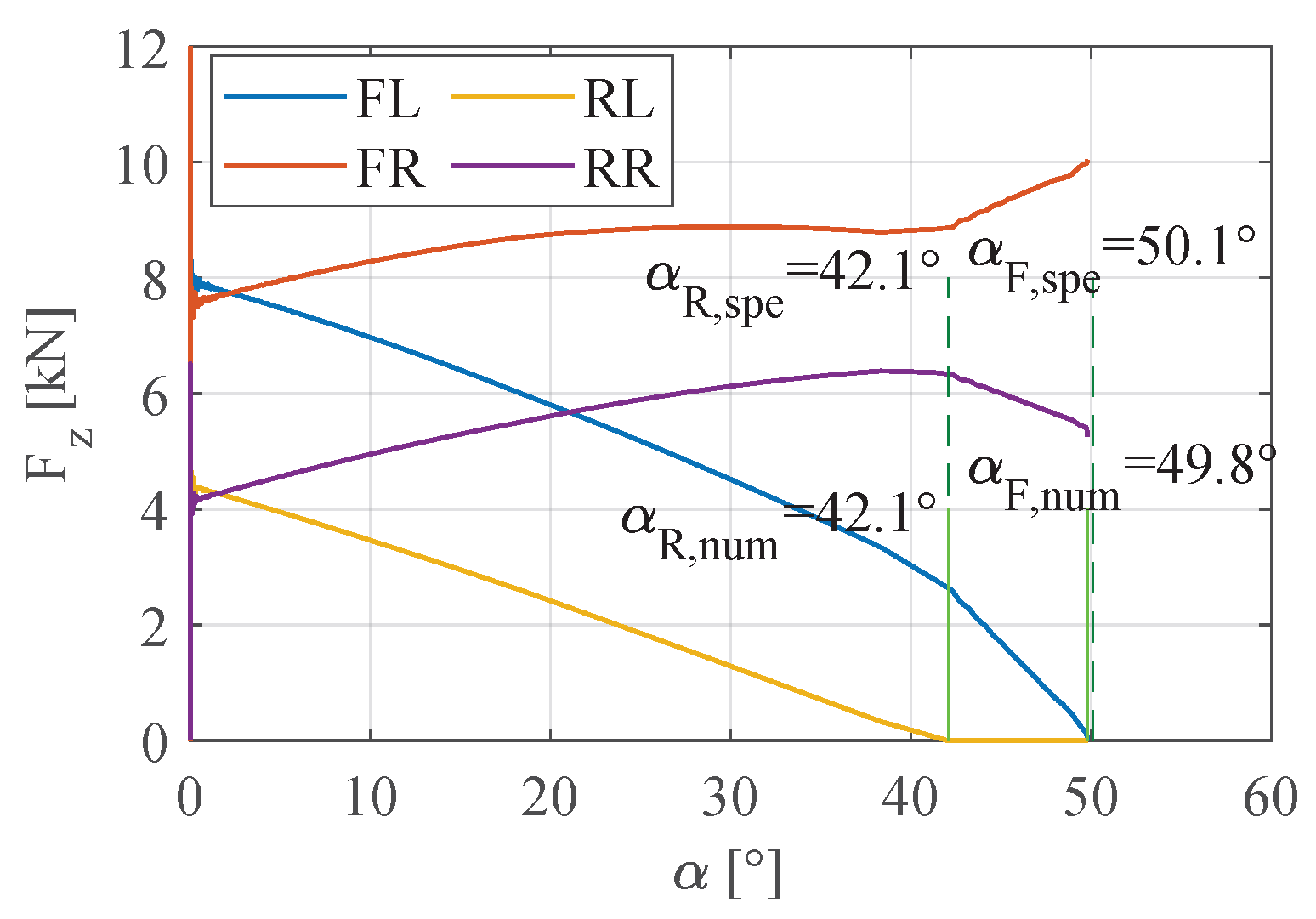
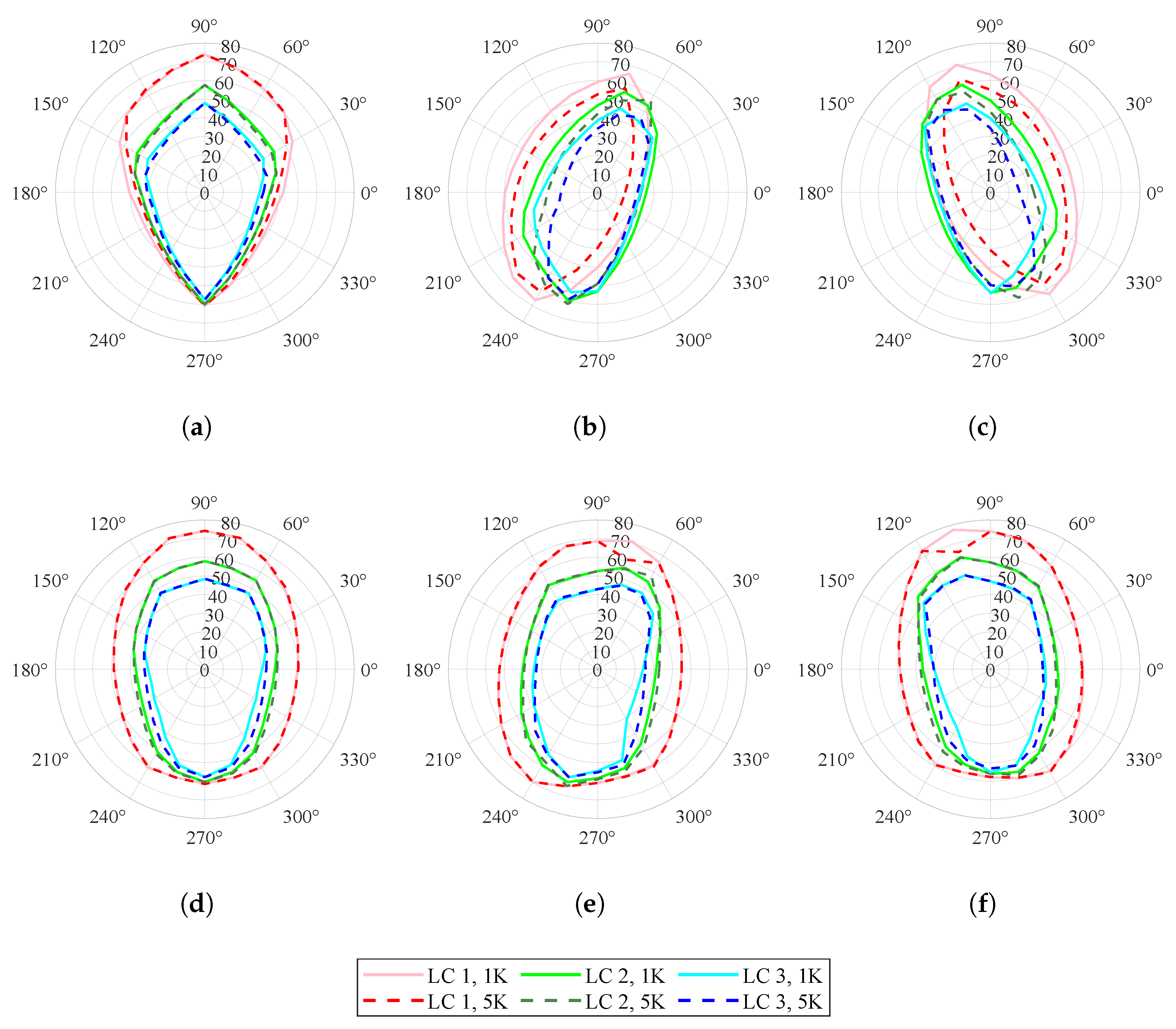
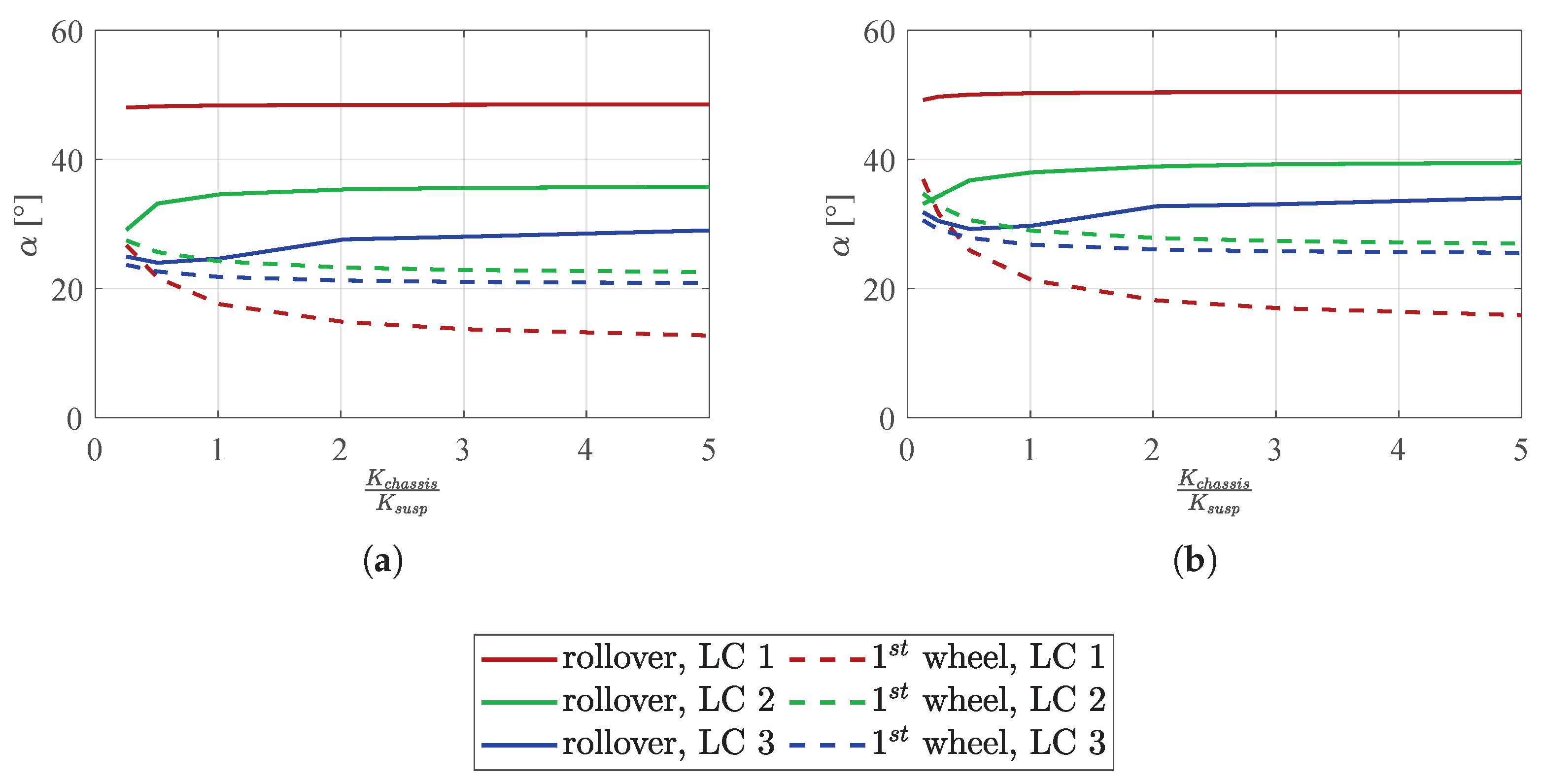
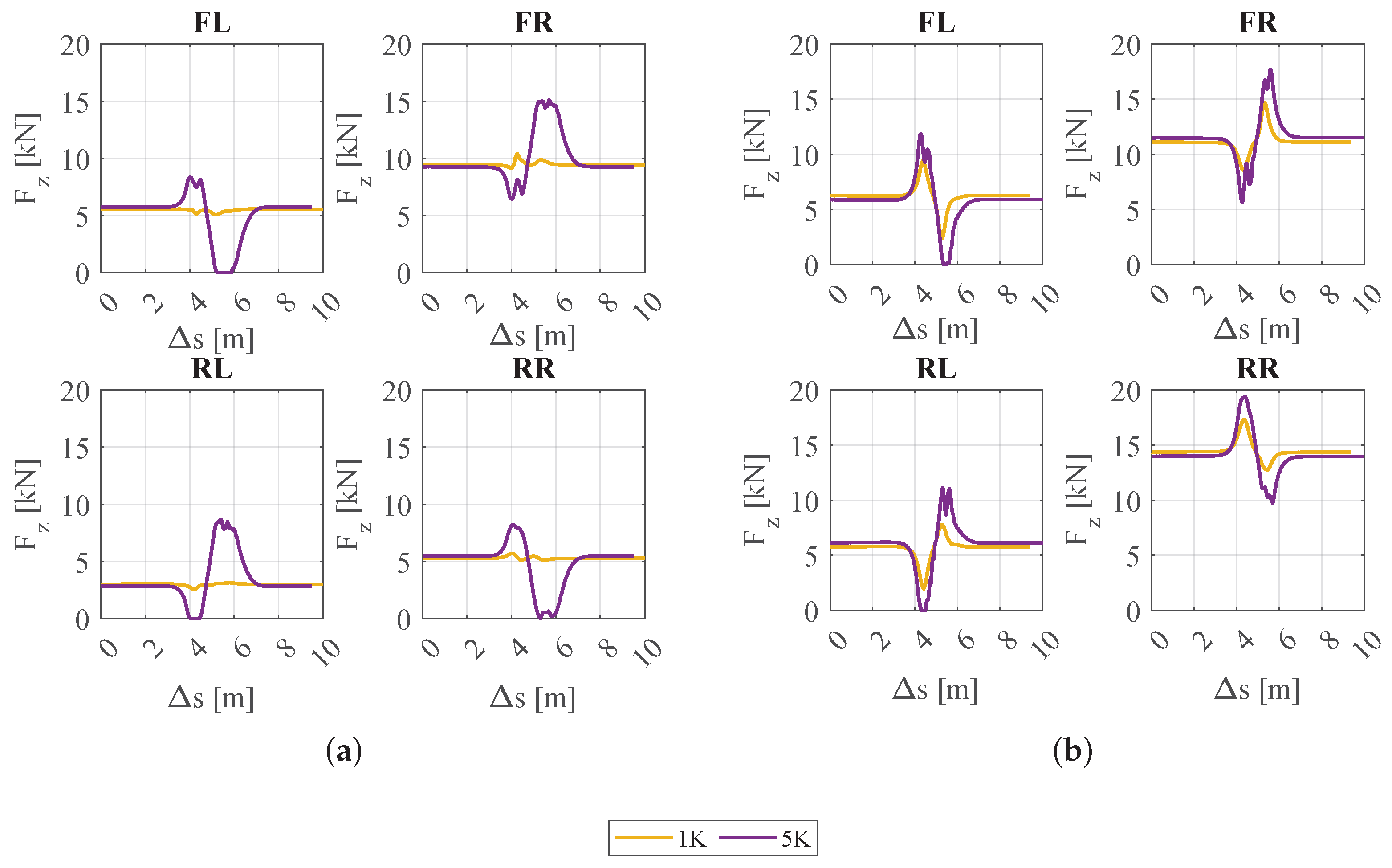
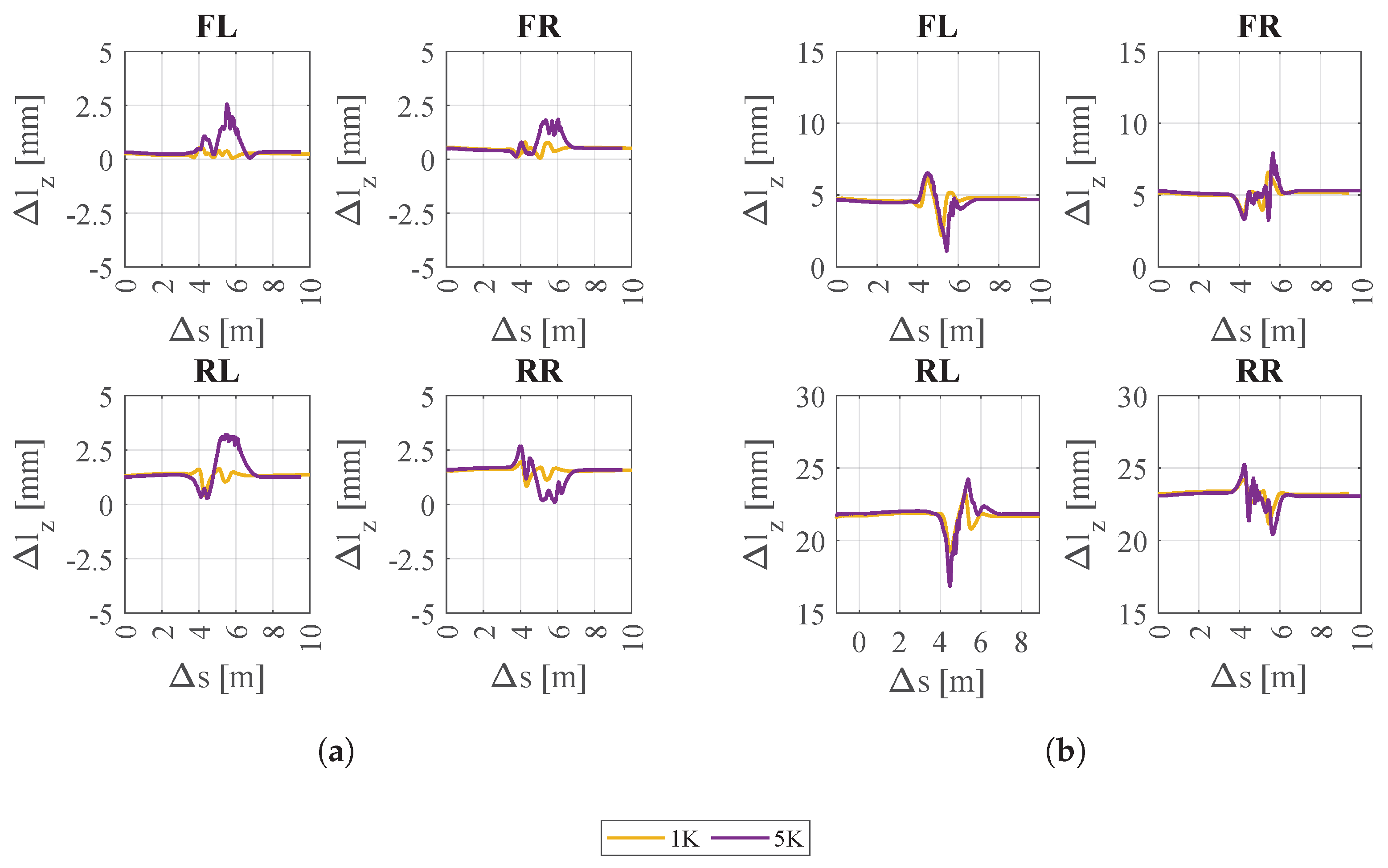
5.1. Static Numerical Simulations Results
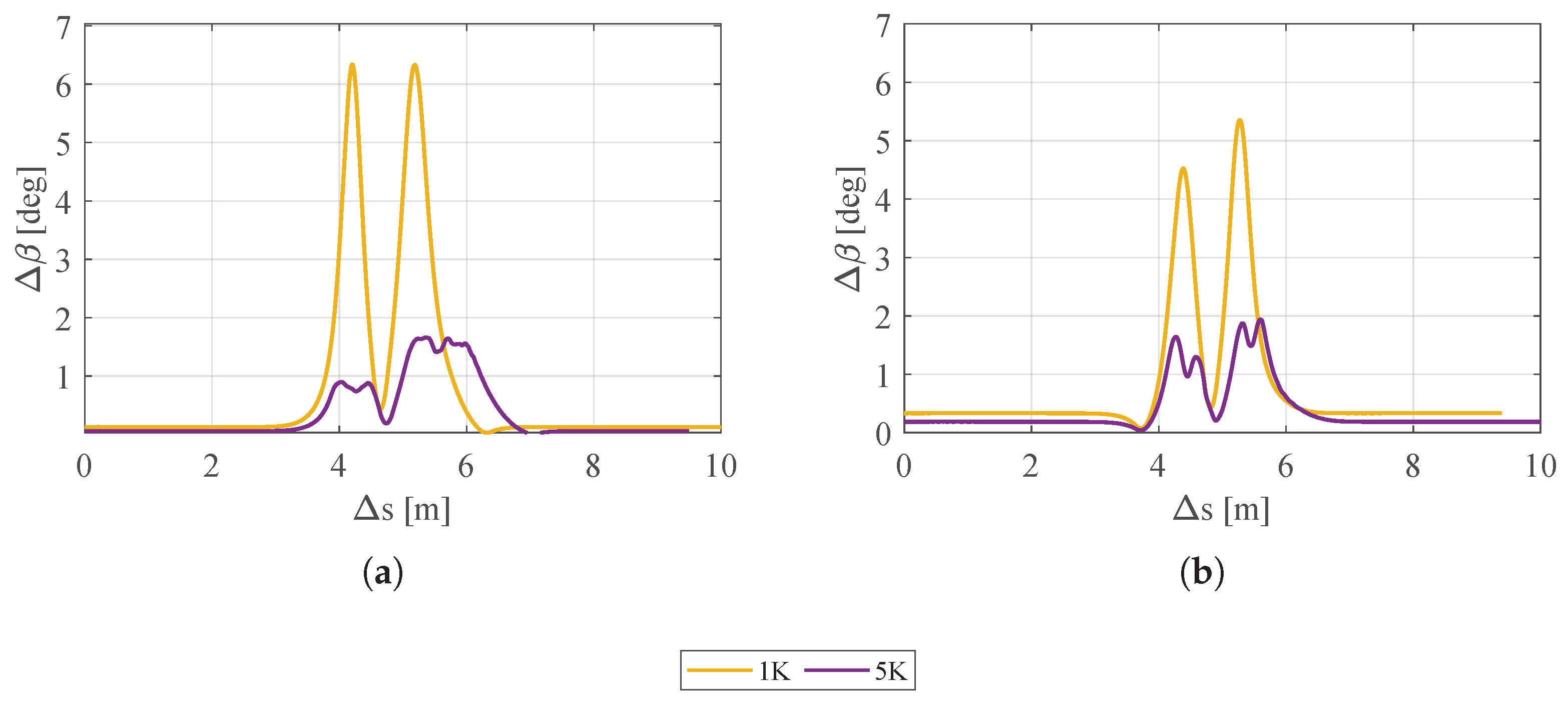
5.2. Dynamic Numerical Simulations Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jarén, C.; Ibarrola, A.; Mangado, T.; Adin, A.; Arnal, P.; López-Maestresalas, A.; Ríos, A.; Arazuri, S. Fatal Tractor Accidents in the Agricultural Sector in Spain during the Past Decade. Agronomy 2022, 12, 1694. [Google Scholar] [CrossRef]
- Bunn, T.L.; Slavova, S.; Hall, L. Narrative text analysis of Kentucky tractor fatality reports. Accid. Anal. Prev. 2008, 40, 419–425. [Google Scholar] [CrossRef] [PubMed]
- Rondelli, V.; Casazza, C.; Martelli, R. Tractor rollover fatalities, analyzing accident scenario. J. Saf. Res. 2018, 67, 99–106. [Google Scholar] [CrossRef] [PubMed]
- EC Directive 74/150/EEC (1974) on Tractors and Agricultural or Forestry Machinery: Approximation of the Laws. Available online: http://www.eur-lex.eu/ (accessed on 1 December 2023).
- EC Directive 2003/37/CE (2003). Available online: https://eur-lex.europa.eu/legal-content/IT/TXT/?uri=CELEX%3A32003L0037&qid=1708423163134 (accessed on 1 December 2023).
- EC Directive 2006/26/CE (2006). Available online: https://eur-lex.europa.eu/legal-content/IT/TXT/?uri=CELEX%3A32006L0096&qid=1708423106526 (accessed on 1 December 2023).
- SAE International Standard J1194; Rollover Protective Structures (ROPS) for Wheeled Agricultural Tractors. Society of Automotive Engineers Inc.: Warrendale, PA, USA, 2009.
- Gibson, H.G.; Elliott, K.C.; Persson, S.P.E. Side slope stability of articulated-frame logging tractors. J. Terramech. 1971, 8, 65–79. [Google Scholar] [CrossRef]
- Cavallo, E.; Görücü, S.; Murphy, D. Perception of side rollover hazards in a Pennsylvania rural population while operating an all-terrain vehicle (ATV). Work 2015, 51, 281–288. [Google Scholar] [CrossRef] [PubMed]
- Guzzomi, A.L.; Rondelli, V.; Guarnieri, A.; Molari, G.; Molari, P.G. Available energy during the rollover of narrow-track wheeled agricultural tractors. Biosyst. Eng. 2009, 104, 318–323. [Google Scholar] [CrossRef]
- Yang, H.; Yang, H.; Xia, C.; Han, J.; Chen, C.; Zhang, H.; Zhang, H. Analysis of Stability and Dynamic Model Simulation of Mountain Tractor Rollover. In Proceedings of the IOP Conference Series: Earth and Environmental Science, Hangzhou, China, 10–12 April 2020; Volume 512. [Google Scholar]
- Franceschetti, B.; Leinain, R.; Rondelli, V. Comparison between a rollover tractor dynamic model and actual lateral tests. Biosyst. Eng. 2014, 127, 79–91. [Google Scholar] [CrossRef]
- Liu, J.; Ayers, P. Off-road vehicle rollover and field testing of stability index. J. Agric. Saf. Health 1999, 5, 59–71. [Google Scholar] [CrossRef]
- Guzzomi, A.L. A revised kineto-static model for Phase I tractor rollover. Biosyst. Eng. 2012, 113, 65–75. [Google Scholar] [CrossRef]
- Jin, Z.; Li, J.; Huang, S. Rollover detection and prevention of a heavy-duty vehicle on banked and graded uneven road. Int. J. Veh. Des. 2022, 87, 218–248. [Google Scholar] [CrossRef]
- Yu, H.; Güvenç, L.; Özguner, Ü. Heavy duty vehicle rollover detection and active roll control. Int. J. Veh. Des. 2008, 46, 451–470. [Google Scholar] [CrossRef]
- Previati, G.; Mastinu, G.; Gobbi, M. Mathematical model for farm tractors towing single axle trailer rollover prediction. Int. J. Veh. Perform. 2022, 8, 132–146. [Google Scholar] [CrossRef]
- Melzi, S.; Sabbioni, E.; Vignati, M.; Cutini, M.; Brambilla, M.; Bisaglia, C.; Cavallo, E. Multibody model of fruit harvesting trucks: Comparison with experimental data and rollover analysis. J. Agric. Eng. 2018, 49, 92–99. [Google Scholar] [CrossRef]
- Jang, M.; Hwang, S.; Kim, J.; Nam, J. Overturning and rollover characteristics of a tractor through dynamic simulations: Effect of slope angle and obstacles on a hard surface. Biosyst. Eng. 2022, 219, 11–24. [Google Scholar] [CrossRef]
- Cutini, M.; Brambilla, M.; Bisaglia, C.; Melzi, S.; Vignati, M.; Cavallo, E.; Laurendi, V. A study of the lateral stability of self-propelled fruit harvesters. Agriculture 2017, 7, 92. [Google Scholar] [CrossRef]
- Sun, C.; Nakashima, H.; Shimizu, H.; Miyasaka, J.; Ohdoi, K. Physics engine application to overturning dynamics analysis on banks and uniform slopes for an agricultural tractor with a rollover protective structure. Biosyst. Eng. 2019, 185, 150–160. [Google Scholar] [CrossRef]
- Yoon, J.; Kim, D.; Yi, K. Design of a rollover index-based vehicle stability control scheme. User Model. User-Adapt. Interact. 2007, 45, 459–475. [Google Scholar] [CrossRef]
- Febo, P.; Pessina, D. The effect of tyre size on the non-continuous rolling test for narrow tractors. J. Agric. Eng. Res. 2001, 78, 147–152. [Google Scholar] [CrossRef]
- ISO 789-6:1982; Agricultural Tractors—Test Procedures—Part 6: Centre of Gravity. International Organization for Standardisation Publ.: Geneva, Switzerland, 1982.
- ISO 16321-1:2013; Self-Propelled Agricultural Machinery—Assessment of Stability—Part 1: Principles. International Organization for Standardisation Publ.: Geneva, Switzerland, 2013.
- ISO 16321-2:2015; Self-Propelled Agricultural Machinery—Assessment of Stability—Part 2: Determination of Static Stability and Test Procedures. International Organization for Standardisation Publ.: Geneva, Switzerland, 2015.
- Federico Cheli, G.D. Advanced Dynamics of Mechanical Systems; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
- Pacejka, H.B. Tire and Vehicle Dynamics, 3rd ed.; Butterworth-Heinemann: Oxford, UK, 2012. [Google Scholar]
- Braghin, F.; Cheli, F.; Genoese, A.; Sabbioni, E.; Bisaglia, C.; Cutini, M. Experimental modal analysis and numerical modelling of agricultural vehicles. In Proceedings of the IMAC-XXVII A Conference and Exposition on Structural Dynamics, Orlando, FL, USA, 9–12 February 2009; pp. 9–12. [Google Scholar]
- Bendat, J.S.; Piersol, A.G. Engineering Applications of Correlation and Spectral Analysis; Wiley-Interscience: Hoboken, NJ, USA, 2012. [Google Scholar]
- Belloni, M.; Vignati, M.; Sabbioni, E.; Cutini, M. Characterization of Vertical Dynamics of a Multi-Purpose Tractor with Static and Dynamic Experimental Tests; SAE WCX Technical Papers; SAE: Detroit, MI, USA, 2023. [Google Scholar]





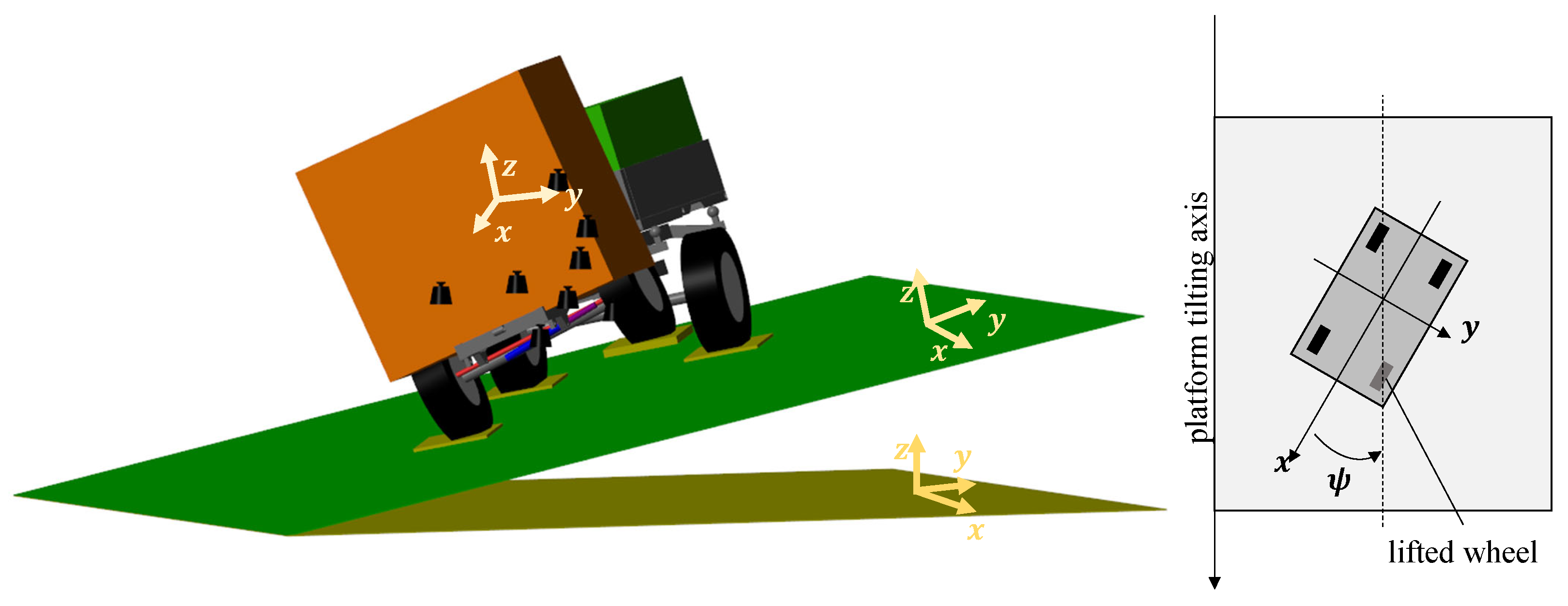
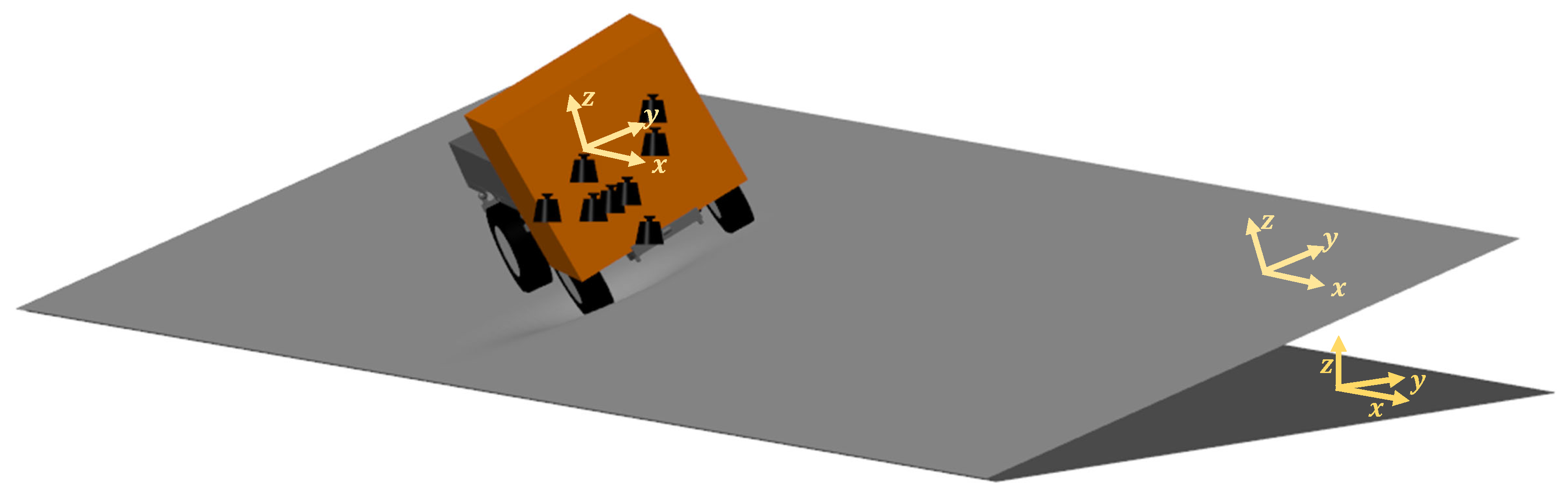
| Dimension | Value | Unit |
|---|---|---|
| Wheelbase | 2045 | mm |
| Front-wheel track | 1385 | mm |
| Rear-wheel track | 1400 | mm |
| Front-tire size | 225/75 R16 | |
| Rear-tire size | 225/75 R17.5 | |
| Overall length | 4060 | mm |
| Overall width | 1690 | mm |
| Unloaded vehicle mass | 2450 | kg |
| Payload | 2500 | kg |
| Sensor Type | Full Scale | Sensitivity | Unit |
|---|---|---|---|
| loading cells | 12,000 | ±5 | kg |
| laser transducers | 30 | ±0.1 | mm |
| accelerometers | ±250 | ±0.00025 | g |
| Dimension | Experimental Measurement | Numerical Model | ||
|---|---|---|---|---|
| Loaded | Unloaded | Loaded | Unloaded | |
| lateral distance from the longitudinal axis (positive left) (mm) | 23 | 20 | 19 | 20 |
| height from the ground (mm) | 701 | 754 | 705 | 760 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belloni, M.; Vignati, M.; Sabbioni, E. Analysing the Effect of Chassis Torsional Flexibility on the Rollover Threshold of a Multi-Purpose Agricultural Vehicle. Vehicles 2024, 6, 415-432. https://doi.org/10.3390/vehicles6010018
Belloni M, Vignati M, Sabbioni E. Analysing the Effect of Chassis Torsional Flexibility on the Rollover Threshold of a Multi-Purpose Agricultural Vehicle. Vehicles. 2024; 6(1):415-432. https://doi.org/10.3390/vehicles6010018
Chicago/Turabian StyleBelloni, Mattia, Michele Vignati, and Edoardo Sabbioni. 2024. "Analysing the Effect of Chassis Torsional Flexibility on the Rollover Threshold of a Multi-Purpose Agricultural Vehicle" Vehicles 6, no. 1: 415-432. https://doi.org/10.3390/vehicles6010018







