Dynamics of Fricke–Painlevé VI Surfaces
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Fricke–Painlevé VI Surface
The Cayley’s Nodal Cubic Surface
3. Algebraic Solutions of Painlevé VI Equation Mapping to Algebraic Surfaces
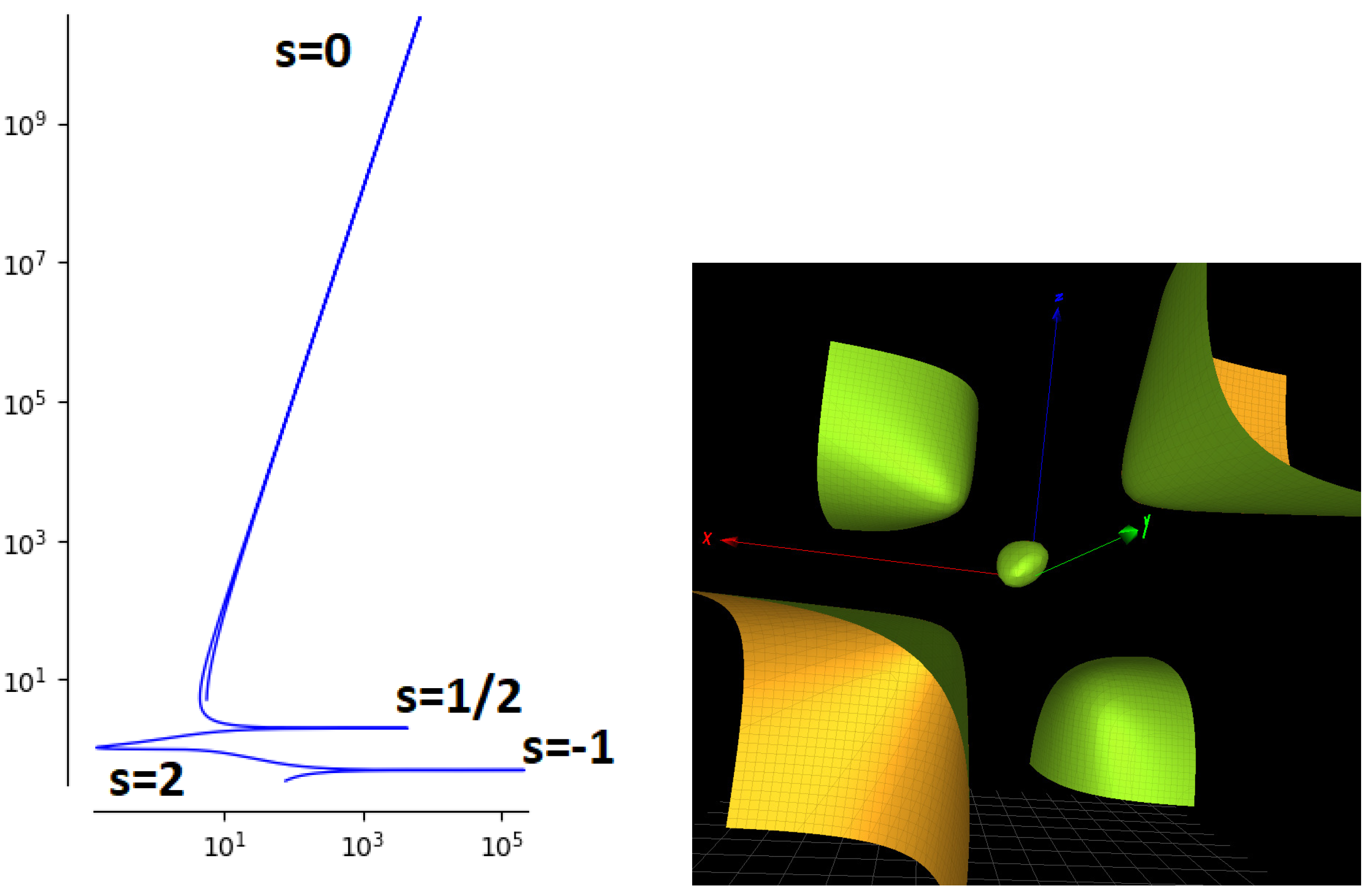
3.1. The Klein Solution
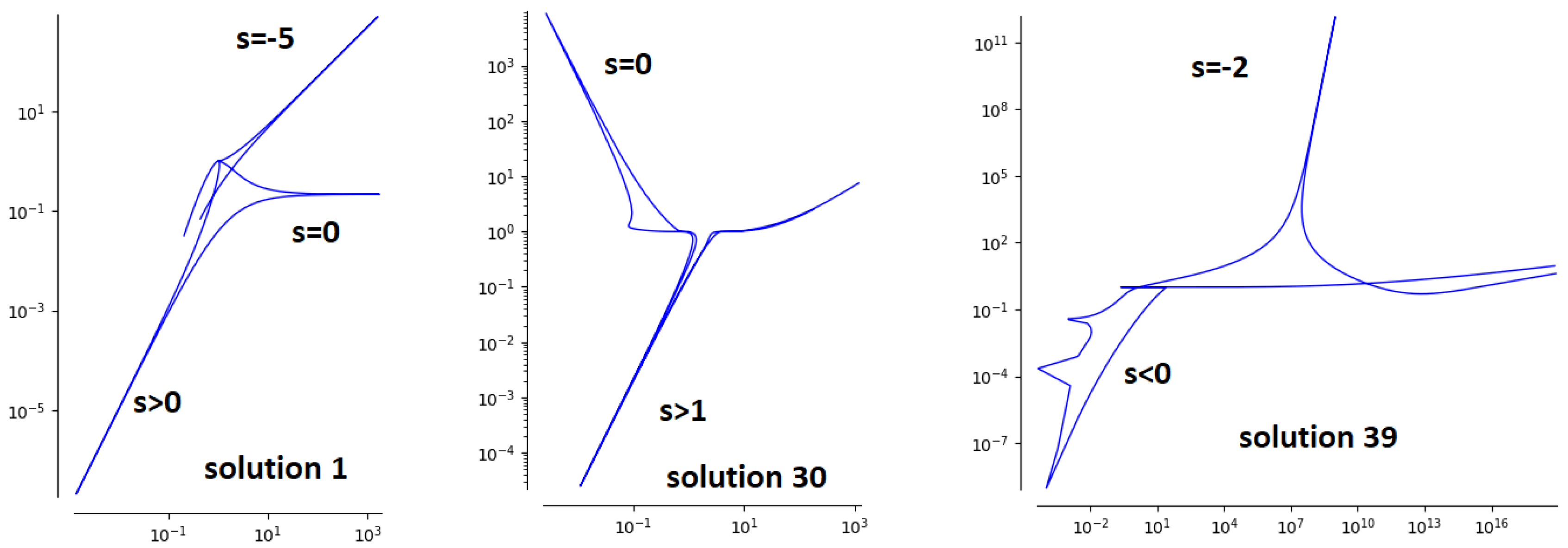
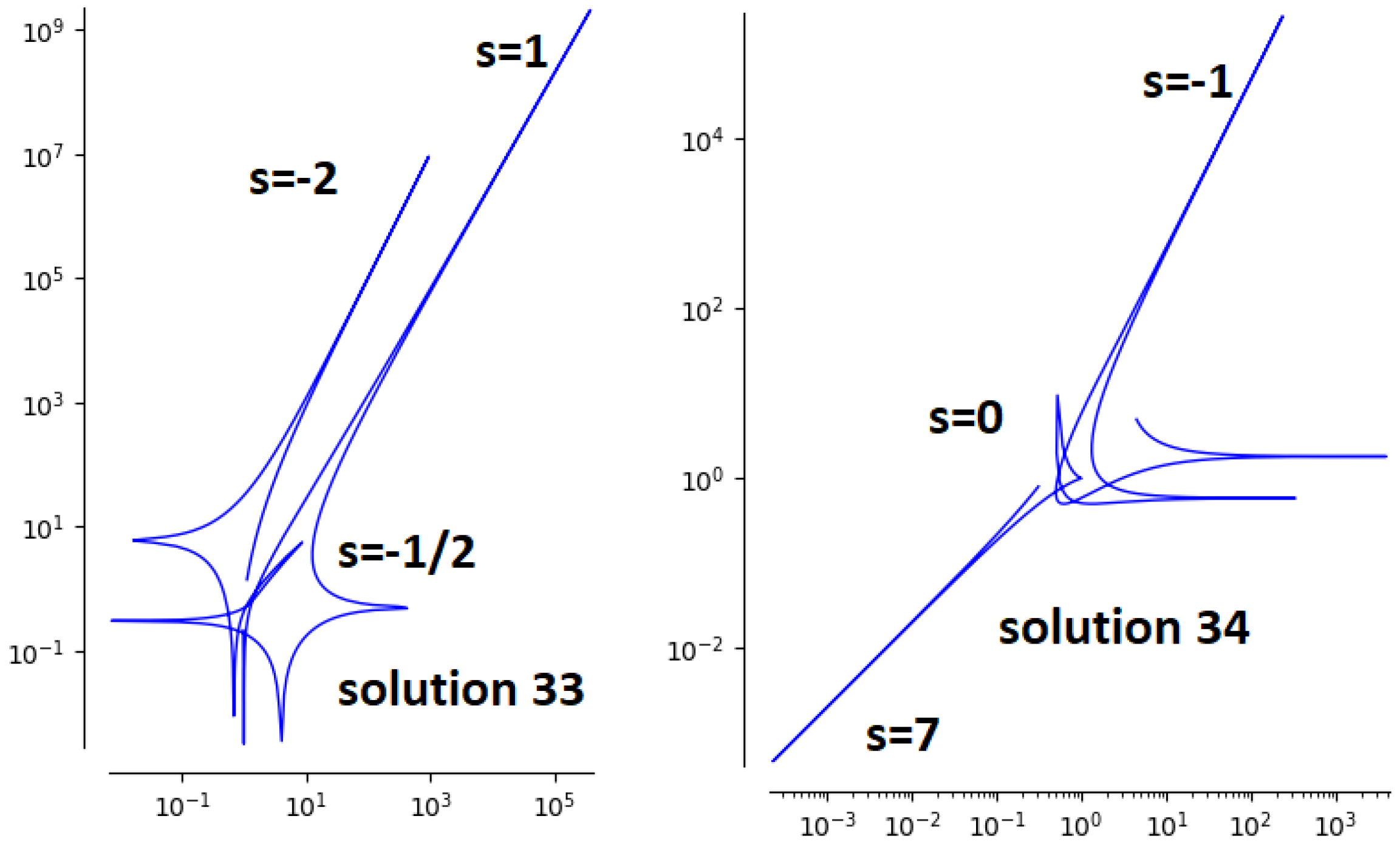
3.2. Solutions with Parameters
3.3. Solutions with Parameters
3.4. The Great Dodecahedron Solution
3.5. Three Extra Solutions Leading to an Algebraic FRICKE-Painlevé Surface
4. Further Algebraic Solutions of Painlevé VI Equation
4.1. The Icosahedral Solution 7
4.2. Dubrovin–Mazzocco Platonic Solutions
4.3. Solutions Related to the Valentiner Group
4.4. Two Extra Icosahedral Solutions
5. Discussion
5.1. Application to Character Varieties of Finitely Generated Groups
A Specific Example: (-Methyladenosine) Modifications
5.2. Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Planat, M.; Chester, D.; Amaral, M.M.; Irwin, K. Fricke topological qubits. Quant. Rep. 2022, 4, 523–532. [Google Scholar] [CrossRef]
- Planat, M.; Amaral, M.; Irwin, K. Algebraic morphology of DNA-RNA transcription and regulation. Symmetry 2023, 15, 770. [Google Scholar] [CrossRef]
- Asselmeyer-Maluga, T. Topological quantum computing and 3-manifolds. Quant. Rep. 2021, 3, 153. [Google Scholar] [CrossRef]
- Clarkson, P.A.; Joshi, N.; Mazzocco, M.; Nijhoff, F.W.; Noumi, M. One hundred years of PVI, the Fuchs—Painlevé equation. J. Phys. A Math. Gen. 2006, 39, EO1. [Google Scholar] [CrossRef]
- Boalch, P. From Klein to Painlevé via Fourier, Laplace and Jimbo. Proc. Lond. Math. Soc. 2005, 90, 167–208. [Google Scholar] [CrossRef]
- Goldman, W.M. Trace coordinates on Fricke spaces of some simple hyperbolic surfaces. Theor. Phys. 2009, 13, 611–684. [Google Scholar]
- Di Francesco, P.; Mathieu, P. Sénéchal, D. Conformal Field Theory; Graduate Texts in Contemporary Physics; Springer: New York, NY, USA, 1997. [Google Scholar]
- Biquard, O. AdS/CFT Correspondence: Einstein Metrics and Their Conformal Boundaries; EMS IRMA Lectures in Mathematics and Theoretical Physics; European Mathematical Society: Strasbourg, France, 2005; ISBN 978-3-03719-013-5. [Google Scholar]
- Isomonodromic Deformation. Available online: https://en.wikipedia.org/wiki/Isomonodromic_deformation (accessed on 1 August 2023).
- Gromak, V.I. The Painlevé Property: One Century Later; CRM Series in Mathematical Physics; Conte, R., Ed.; Springer: New York, NY, USA, 1999; pp. 687–734. [Google Scholar]
- Garnier Integrable System. Available online: https://en.wikipedia.org/wiki/Garnier_integrable_system (accessed on 1 December 2023).
- Mason, L.J.; Woodhouse, N.M.J. Integrability, Self-Duality, and Twistor Theory; London Mathematical Society Monographs; Oxford University Press: Oxford, UK, 1997. [Google Scholar]
- Forrester, P.J. Log Gases and Random Matrices; Princeton University Press: Princeton, NJ, USA, 2010. [Google Scholar]
- Gamayun, O.; Iorgov, N.; Lisovyy, O. Conformal field theory of Painlevé VI. J. High Energy Phys. 2012, 10, 38. [Google Scholar] [CrossRef]
- Jimbo, M. Monodromy problem and the boundary condition for some Painlevé equations. Publ. RIMS Kyoto Univ. 1982, 18, 1137. [Google Scholar] [CrossRef]
- Iorgov, N.; Lisovyy, O.; Tykhyyy, Y. Painlevé VI connection problem and monodromy of c = 1 conformal blocks. JHEP 2012, 10, 038. [Google Scholar] [CrossRef]
- Planat, M.; Amaral, M.M.; Fang, F.; Chester, D.; Aschheim, R.; Irwin, K. Character varieties and algebraic surfaces for the topology of quantum computing. Symmetry 2022, 14, 915. [Google Scholar] [CrossRef]
- Lisovyy, O.; Tykhyy, Y. Algebraic solutions of the sixth Painlevé equation. J. Geom. Phys. 2014, 85, 124–163. [Google Scholar] [CrossRef]
- Cantat, S. Bers and Hénon, Painlevé and Schrödinger. Duke Math. J. 2009, 149, 411–460. [Google Scholar] [CrossRef]
- Benedetto, R.L.; Goldman, W.M. The topology of the relative character varieties of a quadruply-punctured sphere. Exp. Math. 1999, 8, 85–103. [Google Scholar] [CrossRef]
- Iwasaki, K. An area-preserving action of the modular group on cubic surfaces and the Painlevé VI. Comm. Math. Phys. 2003, 242, 185–219. [Google Scholar] [CrossRef]
- Inaba, M.; Iwasaki, K.; Saito, M.H. Dynamics of the sixth Painlevé equation. arXiv 2005, arXiv:math.AG/0501007. [Google Scholar]
- Mazzocco, M. Picard and Chazy solutions to the Painlevé VI equation. Math. Ann. 2001, 321, 157–195. [Google Scholar] [CrossRef]
- Boalch, P. Towards a nonlinear Schwarz’s list. arXiv 2007, arXiv:0707.3375. [Google Scholar]
- Boalch, P. The fifty-two icosahedral solutions of Painlevé VI. J. Reine Angew. Math. 2006, 596, 183–214. [Google Scholar] [CrossRef]
- Dubrovin, B.; Mazzocco, M. Monodromy of certain Painlevé-VI transcendents and reflection groups. Invent. Math. 2000, 141, 55–147. [Google Scholar] [CrossRef]
- Hitchin, N. A lecture on the octahedron. Bull. Lond. Math. Soc. 2003, 35, 577–600. [Google Scholar] [CrossRef]
- Kitaev, L.V. Remarks towards the classification of (3)-transformations and algebraic solutions of the sixth Painlevé equation. Semin. Congr. Soc. Math. Fr. 2006, 14, 199–227. [Google Scholar]
- Boalch, P. Painlevé, Klein & the Icosahedron. Available online: https://webusers.imj-prg.fr/philip.boalch/abs/nlsl.html (accessed on 20 December 2023).
- Boalch, P. The Klein solution to Painlevé’s sixth equation. arXiv 2003, arXiv:math.AG/0308221. [Google Scholar]
- Planat, M.; Amaral, M.M.; Chester, D.; Irwin, K. SL(2, ℂ) scheme processsing of singularities in quantum computing and genetics. Axioms 2023, 12, 233. [Google Scholar] [CrossRef]
- Vissers, C.; Sinha, A.; Ming, G.L.; Song, H. The epitranscriptome in stem cell biology and neural development. Neurobiol. Dis 2020, 146, 105139. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Lv, W.; Li, T.; Zhang, S.; Wang, H.; Li, X.; Wang, L.; Ma, D.; Zang, Y.; Shen, J.; et al. Dynamic regulation and functions of mRNA m6A modification. Cancer Cell Int. 2022, 22, 48. [Google Scholar] [CrossRef] [PubMed]
- Widagdo, J.; Wong, J.J.L.; Anggono, V. The m6A-epitranscriptome in brain plasticity, learning and memory. Semin. Cell Dev. Biol. 2022, 125, 110–121. [Google Scholar] [CrossRef] [PubMed]
- Planat, M.; Amaral, M.M.; Fang, F.; Chester, D.; Aschheim, R. Irwin, K. Group theory of messenger RNA metabolism and disease. Gene Expr. 2023, in press. [Google Scholar] [CrossRef]
- Cantat, S.; Loray, F. Holomorphic dynamics, Painlevé VI equation and character varieties. arXiv 2007, arXiv:1207.0154. [Google Scholar]
- Connes, A.; Marcolli, M. Quantum fieds and motives. J. Geom. Phys. 2006, 56, 55–85. [Google Scholar] [CrossRef]
- Cartier, P. A mad day’s work: From Grothendieck to Connes and Kontsevich. The evolution of concepts of space and symmetry. Bull. Amer. Math. Soc. 2001, 38, 389–408. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Planat, M.; Chester, D.; Irwin, K. Dynamics of Fricke–Painlevé VI Surfaces. Dynamics 2024, 4, 1-13. https://doi.org/10.3390/dynamics4010001
Planat M, Chester D, Irwin K. Dynamics of Fricke–Painlevé VI Surfaces. Dynamics. 2024; 4(1):1-13. https://doi.org/10.3390/dynamics4010001
Chicago/Turabian StylePlanat, Michel, David Chester, and Klee Irwin. 2024. "Dynamics of Fricke–Painlevé VI Surfaces" Dynamics 4, no. 1: 1-13. https://doi.org/10.3390/dynamics4010001






