You seem to have javascript disabled. Please note that many of the page functionalities won't work as expected without javascript enabled.

Search for Articles:

Title / Keyword

Author / Affiliation / Email

Journal

Article Type

Advanced Search

Section

Special Issue

Volume

Issue

Number

Page

Logical OperatorOperator

Search Text

Search Type

Journal Description

Mathematical and Computational Applications

Mathematical and Computational Applications is an international, peer-reviewed, open access journal on applications of mathematical and/or computational techniques, published bimonthly online by MDPI. The South African Association for Theoretical and Applied Mechanics (SAAM) is affiliated with the journal Mathematical and Computational Applications and its members receive discounts on the article processing charges.

Open Access— free for readers, with article processing charges (APC) paid by authors or their institutions.
High Visibility: indexed within Scopus, ESCI (Web of Science), Inspec, and other databases.
Journal Rank: JCR - Q2 (Mathematics, Interdisciplinary Applications)
Rapid Publication: manuscripts are peer-reviewed and a first decision is provided to authors approximately 25.4 days after submission; acceptance to publication is undertaken in 2.6 days (median values for papers published in this journal in the second half of 2024).
Recognition of Reviewers: reviewers who provide timely, thorough peer-review reports receive vouchers entitling them to a discount on the APC of their next publication in any MDPI journal, in appreciation of the work done.
Testimonials: See what our editors and authors say about MCA.

Impact Factor: 1.9 (2023); 5-Year Impact Factor: 1.6 (2023)

Imprint Information Journal Flyer Open Access ISSN: 2297-8747

Latest Articles

16 pages, 2341 KiB

Open AccessArticle

TAE Predict: An Ensemble Methodology for Multivariate Time Series Forecasting of Climate Variables in the Context of Climate Change

by Juan Frausto Solís, Erick Estrada-Patiño, Mirna Ponce Flores, Juan Paulo Sánchez-Hernández, Guadalupe Castilla-Valdez and Javier González-Barbosa

Math. Comput. Appl. 2025, 30(3), 46; https://doi.org/10.3390/mca30030046 - 25 Apr 2025

Climate change presents significant challenges due to the increasing frequency and intensity of extreme weather events. Mexico, with its diverse climate and geographic position, is particularly vulnerable, underscoring the need for robust strategies to predict atmospheric variables. This work presents TAE Predict (Time [...] Read more.

Climate change presents significant challenges due to the increasing frequency and intensity of extreme weather events. Mexico, with its diverse climate and geographic position, is particularly vulnerable, underscoring the need for robust strategies to predict atmospheric variables. This work presents TAE Predict (Time series Analysis and Ensemble-based Prediction with relevant feature selection) based on relevant feature selection and ensemble models of machine learning. Dimensionality in multivariate time series is reduced through Principal Component Analysis, ensuring interpretability and efficiency. Additionally, data remediation techniques improve data set quality. The ensemble combines Long Short-Term Memory neural networks, Random Forest regression, and Support Vector Machines, optimizing their contributions using heuristic algorithms such as Particle Swarm Optimization. Experimental results from meteorological time series in key Mexican cities demonstrate that the proposed strategy outperforms individual models in accuracy and robustness. This methodology provides a replicable framework for climate variable forecasting, delivering analytical tools that support decision-making in critical sectors, such as agriculture and water resource management. The findings highlight the potential of integrating modern techniques to address complex, high-dimensional problems. By combining advanced prediction models and feature selection strategies, this study advances the reliability of climate forecasts and contributes to the development of effective adaptation and mitigation measures in response to climate change challenges. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

Figure 1

35 pages, 1960 KiB

Open AccessArticle

Bio-Inspired Multiobjective Optimization for Designing Content Distribution Networks

by Gerardo Goñi, Sergio Nesmachnow, Diego Rossit, Pedro Moreno-Bernal and Andrei Tchernykh

Math. Comput. Appl. 2025, 30(2), 45; https://doi.org/10.3390/mca30020045 - 21 Apr 2025

This article studies the effective design of content distribution networks over cloud computing platforms. This problem is relevant nowadays to provide fast and reliable access to content on the internet. A bio-inspired evolutionary multiobjective optimization approach is applied as a viable alternative to [...] Read more.

This article studies the effective design of content distribution networks over cloud computing platforms. This problem is relevant nowadays to provide fast and reliable access to content on the internet. A bio-inspired evolutionary multiobjective optimization approach is applied as a viable alternative to solve realistic problem instances where exact optimization methods are not applicable. Ad hoc representation and search operators are applied to optimize relevant metrics from the point of view of both system administrators and users. In the evaluation of problem instances built using real data, the evolutionary multiobjective optimization approach was able to compute more accurate solutions in terms of cost and quality of service when compared to the exact resolution method. The obtained results represent an improvement over greedy heuristics from 47.6% to 93.3% in terms of cost while maintaining competitive quality of service. In addition, the computed solutions had different tradeoffs between the problem objectives. This can provide different options for content distribution network design, allowing for a fast configuration that fulfills specific quality of service demands. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

Figure 1

13 pages, 4627 KiB

Open AccessArticle

Detection of Abnormal Pedestrian Flows with Automatic Contextualization Using Pre-Trained YOLO11n

by Adrián Núñez-Vieyra, Juan C. Olivares-Rojas, Rogelio Ferreira-Escutia, Arturo Méndez-Patiño, José A. Gutiérrez-Gnecchi and Enrique Reyes-Archundia

Math. Comput. Appl. 2025, 30(2), 44; https://doi.org/10.3390/mca30020044 - 17 Apr 2025

Recently, video surveillance systems have evolved from expensive, human-operated monitoring systems that were only useful after the crime was committed to systems that monitor 24/7, in real time, and with less and less human involvement. This is partly due to the use of [...] Read more.

Recently, video surveillance systems have evolved from expensive, human-operated monitoring systems that were only useful after the crime was committed to systems that monitor 24/7, in real time, and with less and less human involvement. This is partly due to the use of smart cameras, the improvement of the Internet, and AI-based algorithms that allow the classifying and tracking of objects in images and in some cases identifying them as threats. Threats are often associated with abnormal or unexpected situations such as the presence of unauthorized persons in a given place or time, the manifestation of a different behavior by one or more persons compared to the behavior of the majority, or simply an unexpected number of people in the place, which depends largely on the available information of their context, i.e., place, date, and time of capture. In this work, we propose a model to automatically contextualize video capture scenarios, generating data such as location, date, time, and flow of people in the scene. A strategy to measure the accuracy of the data generated for such contextualization is also proposed. The pre-trained YOLO11n algorithm and the Bot-SORT algorithm gave the best results in person detection and tracking, respectively. Full article

(This article belongs to the Special Issue New Trends in Computational Intelligence and Applications 2024)

► Show Figures

Figure 1

15 pages, 674 KiB

Open AccessArticle

Thau Observer for Insulin Estimation Considering the Effect of Beta-Cell Dynamics for a Diabetes Mellitus Model

by Diana Gamboa, Tonalli C. Galicia and Paul J. Campos

Math. Comput. Appl. 2025, 30(2), 43; https://doi.org/10.3390/mca30020043 - 17 Apr 2025

In this work, a Thau observer is designed based on a nonlinear third-order mathematical model described by ODEs, which captures the dynamics among insulin levels,

β

-cells, and glucose concentration. The novelty of this research lies in its interdisciplinary approach to understanding a [...] Read more.

In this work, a Thau observer is designed based on a nonlinear third-order mathematical model described by ODEs, which captures the dynamics among insulin levels,

β

-cells, and glucose concentration. The novelty of this research lies in its interdisciplinary approach to understanding a complex biological system. The observer’s mathematical validation is established using the Localization of Compact Invariant Sets to determine the domain of attraction and global knowledge about the system’s dynamic bounds. These bounds are used to compute the Lipschitz constant and the elements of the free gain matrix that satisfy the constraints for designing a Thau observer, such as the stability matrix and asymptotic stability. This analysis provides insights into how insulin levels evolve over time at various glucose concentrations, an essential step toward hardware implementation due to the system’s chaotic behavior. It also establishes a mathematical background that could contribute to treatment planning in future Digital Twins studies. Numerical simulations demonstrate that the observer can accurately track the dynamic behavior of the Diabetes Mellitus model analyzed in this work through in silico methods. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

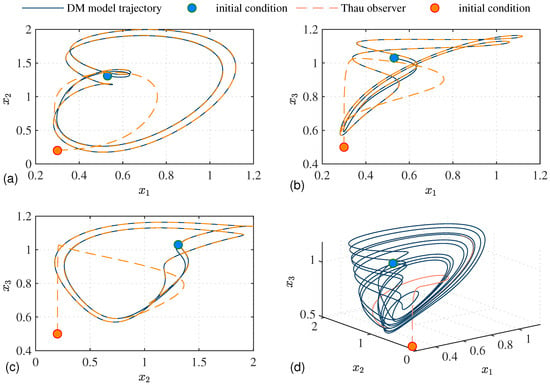
Figure 1

24 pages, 485 KiB

Open AccessArticle

The Weighted Flexible Weibull Model: Properties, Applications, and Analysis for Extreme Events

by Ziaurrahman Ramaki, Morad Alizadeh, Saeid Tahmasebi, Mahmoud Afshari, Javier E. Contreras-Reyes and Haitham M. Yousof

Math. Comput. Appl. 2025, 30(2), 42; https://doi.org/10.3390/mca30020042 - 16 Apr 2025

The weighted flexible Weibull distribution focuses on its unique point of flaunting a bathtub-shaped hazard rate, characterized by an initial increase followed by a drop over time. This property plays a major role in reliability analysis. In this paper, this distribution and its [...] Read more.

The weighted flexible Weibull distribution focuses on its unique point of flaunting a bathtub-shaped hazard rate, characterized by an initial increase followed by a drop over time. This property plays a major role in reliability analysis. In this paper, this distribution and its main properties are examined, and the parameters are estimated using several estimation methods. In addition, a simulation study is done for different sample sizes. The performance of the proposed model is illustrated through two real-world applications: component failure times and COVID-19 mortality. Moreover, the value-at-risk (VaR), tail value-at-risk (TVaR), peaks over a random threshold VaR (PORT-VaR), the mean of order P (

{MOP}_{[P]}

) analysis, and optimal order of P due to the true mean value can help identify and characterize critical events or outliers in failure events and COVID-19 death data across different counties. Finally, the PORT-VaR estimators are provided under a risk analysis for both applications. Full article

(This article belongs to the Section Social Sciences)

► Show Figures

Figure 1

23 pages, 5543 KiB

Open AccessArticle

Enhanced Synchrosqueezing Transform for Detecting Non-Traditional Flight Modes in High Angle of Attack Maneuvers

by Seyed Amin Bagherzadeh, Hamed Mohammadkarimi and Mohammad Hossein Alizadeh

Math. Comput. Appl. 2025, 30(2), 41; https://doi.org/10.3390/mca30020041 - 12 Apr 2025

Due to nonlinear aerodynamics, “non-traditional” flight modes may appear in longitudinal and lateral/directional dynamics once an aircraft experiences a high angle of attack and rapid maneuvers. Signal decomposition techniques are required to uncover these modes since they are hidden in flight characteristics. This [...] Read more.

Due to nonlinear aerodynamics, “non-traditional” flight modes may appear in longitudinal and lateral/directional dynamics once an aircraft experiences a high angle of attack and rapid maneuvers. Signal decomposition techniques are required to uncover these modes since they are hidden in flight characteristics. This study represents the Enhanced SynchroSqueezing Transform (ESST) for the extraction of “non-traditional” flight modes from flight data. Developed in the framework of the SynchroSqueezing Transform (SST), the ESST decomposes an Amplitude- and Frequency-Modulated (AMFM) signal into Intrinsic Mode Functions (IMFs). This process is optimized using the Genetic Algorithm (GA). Numerical investigations are performed to confirm the validity of the ESST. Both quantitative criteria for the fitness of the IMFs and qualitative study of the Time–Frequency Representations (TFRs) suggest that the ESST may perform better than the SST in decomposing nonlinear and non-stationary signals. Then, a method is proposed to find the instantaneous characteristics of the flight modes obtained by the ESST. The ESST analyzes an aircraft’s longitudinal and lateral flight data in post-stall maneuvers. The TFRs of flight parameters verify the existence of identical flight modes at different flight conditions. The IMFs are separated, and their instantaneous characteristics are computed. In addition, the ESST modes are compared to conventional modes. The results indicate that the ESST is capable of obtaining both classical oscillatory modes, such as Short Period (SP) and Dutch Roll (DR), and “non-traditional” modes. In the end, coupled modes are identified by comparing longitudinal and lateral IMFs. Full article

(This article belongs to the Section Engineering)

► Show Figures

Figure 1

21 pages, 1482 KiB

Open AccessArticle

Fractional Diffusion: A Structured Approach to Application with Examples

by Kathrin Kulmus, Christopher Essex, Karl Heinz Hoffmann and Janett Prehl

Math. Comput. Appl. 2025, 30(2), 40; https://doi.org/10.3390/mca30020040 - 9 Apr 2025

Time-fractional evolution equations for probability distributions provide a means of representing an important class of stochastic processes. Their solutions have features that are important in modeling anomalous diffusion and a variety of other real-world applications, like the search patterns of predators or queuing [...] Read more.

Time-fractional evolution equations for probability distributions provide a means of representing an important class of stochastic processes. Their solutions have features that are important in modeling anomalous diffusion and a variety of other real-world applications, like the search patterns of predators or queuing problems. However, these equations are usually not included in current physics education, as the underlying mathematics might be considered too advanced. In the following, we present a novel approach to understanding time-fractional diffusion equations and their solutions for practical applications. This approach shifts the focus to the physical rather than the in-depth mathematical properties typically studied for this topic. We introduce the fractional differential operator simply as an identity operation on generalizations of exponential functions. This shifts the emphasis to the actual functions of fractional derivatives instead of what they are. This concept is applied to a discrete one-dimensional time-fractional diffusion equation on a finite interval modeling anomalous subdiffusion. Examples and tasks are provided for readers to allow interactive learning. Full article

(This article belongs to the Section Natural Sciences)

► Show Figures

Figure 1

21 pages, 3138 KiB

Open AccessArticle

An Evolutionary Strategy Based on the Generalized Mallows Model Applied to the Mixed No-Idle Permutation Flow Shop Scheduling Problem

by Elvi M. Sánchez Márquez, Ricardo Pérez-Rodríguez, Manuel Ornelas-Rodriguez and Héctor J. Puga-Soberanes

Math. Comput. Appl. 2025, 30(2), 39; https://doi.org/10.3390/mca30020039 - 3 Apr 2025

The Mixed No-Idle Permutation Flow Shop Scheduling Problem (MNPFSSP) represents a specific case within regular flow scheduling problems. In this problem, some machines allow idle times between consecutive jobs or operations while other machines do not. Traditionally, the MNPFSSP has been addressed using [...] Read more.

The Mixed No-Idle Permutation Flow Shop Scheduling Problem (MNPFSSP) represents a specific case within regular flow scheduling problems. In this problem, some machines allow idle times between consecutive jobs or operations while other machines do not. Traditionally, the MNPFSSP has been addressed using the metaheuristics and exact methods. This work proposes an Evolutionary Strategy Based on the Generalized Mallows Model (ES-GMM) to solve the issue. Additionally, its advanced version, ES-GMMc, is developed, incorporating operating conditions to improve execution times without compromising solution quality. The proposed approaches are compared with algorithms previously used for the problem under study. Statistical tests of the experimental results show that the ES-GMMc achieved reductions in execution time, especially standing out in large instances, where the shortest computing times were obtained in 23 of 30 instances, without affecting the quality of the solutions. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

Figure 1

32 pages, 5759 KiB

Open AccessReview

Related Standards and Certifications in the Architecture of Service-Oriented System in Welding Technology: A Systematic Review

by Bálint Molnár, József Szőlősi, Attila Gludovátz and Mátyás Andó

Math. Comput. Appl. 2025, 30(2), 38; https://doi.org/10.3390/mca30020038 - 31 Mar 2025

IT (Information Technology) support plays a major role in CPSs (cyber-physical systems). More and more IT solutions and CIS (complex information system) modules are being developed to help engineering systems to a higher level of efficiency. The different specificities of different technological environments [...] Read more.

IT (Information Technology) support plays a major role in CPSs (cyber-physical systems). More and more IT solutions and CIS (complex information system) modules are being developed to help engineering systems to a higher level of efficiency. The different specificities of different technological environments require a very different IT approach. Increasing the efficiency of different manufacturing processes requires an appropriate architecture. The Zachman framework guidelines were applied to design a suitable framework architecture for the welding process. A literature search was conducted to explore the conditions for component matching to a complex information system, in which advanced data management and data protection are important. In order to effectively manage the standards, a dedicated module needs to be created that can be integrated into the MES-ERP (Manufacturing Execution System-Enterprise Resource Planning) architecture. The result of the study is the creation of business UML (Unified Modeling Language) and BPMN (Business Process Model and Notation) diagrams and a roadmap to start a concrete application development. The paper concludes with an example to illustrate ideas for the way forward. Full article

(This article belongs to the Section Engineering)

► Show Figures

Figure 1

17 pages, 562 KiB

Open AccessArticle

A New Higher-Order Convergence Laplace–Fourier Method for Linear Neutral Delay Differential Equations

by Gilbert Kerr and Gilberto González-Parra

Math. Comput. Appl. 2025, 30(2), 37; https://doi.org/10.3390/mca30020037 - 28 Mar 2025

In this article, a new higher-order convergence Laplace–Fourier method is developed to obtain the solutions of linear neutral delay differential equations. The proposed method provides more accurate solutions than the ones provided by the pure Laplace method and the original Laplace–Fourier method. We [...] Read more.

In this article, a new higher-order convergence Laplace–Fourier method is developed to obtain the solutions of linear neutral delay differential equations. The proposed method provides more accurate solutions than the ones provided by the pure Laplace method and the original Laplace–Fourier method. We develop and show the crucial modifications of the Laplace–Fourier method. As with the original Laplace–Fourier method, the new method combines the Laplace transform method with Fourier series theory. All of the beneficial features from the original Laplace–Fourier method are retained. The solution still includes a component that accounts for the terms in the tail of the infinite series, allowing one to obtain more accurate solutions. The Laplace–Fourier method requires us to approximate the formula for the residues with an asymptotic expansion. This is essential to enable us to use the Fourier series results that enable us to account for the tail. The improvement is achieved by deriving a new asymptotic expansion which minimizes the error between the actual residues and those which are obtained from this asymptotic expansion. With both the pure Laplace and improved Laplace–Fourier methods, increasing the number of terms in the truncated series obviously increases the accuracy. However, with the pure Laplace method, this improvement is small. As we shall show, with the improved higher-order convergence Laplace–Fourier method, the improvement is significantly larger. We show that the convergence rate of the new Laplace–Fourier solution has a remarkable order of convergence. The validity of the new technique is corroborated by means of illustrative examples. Comparisons of the solutions of the new method with those generated by the pure Laplace method and the unmodified Laplace–Fourier approach are presented. Full article

► Show Figures

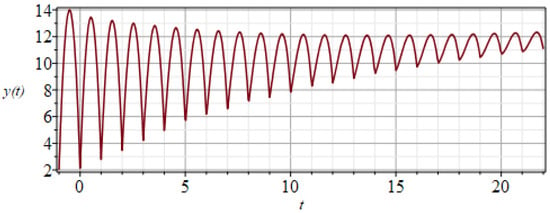
Figure 1

13 pages, 1333 KiB

Open AccessArticle

Internet-of-Things-Based CO₂ Monitoring and Forecasting System for Indoor Air Quality Management

by Marya J. Marquez-Zepeda, Ildeberto Santos-Ruiz, Esvan-Jesús Pérez-Pérez, Adrián Navarro-Díaz and Jorge-Alejandro Delgado-Aguiñaga

Math. Comput. Appl. 2025, 30(2), 36; https://doi.org/10.3390/mca30020036 - 28 Mar 2025

This study presents a low-cost and scalable CO₂ monitoring system that leverages NDIR sensors and a Long Short-Term Memory (LSTM) neural network to predict indoor CO₂ concentrations over both short- and long-term horizons. The proposed system aims to anticipate air quality [...] Read more.

This study presents a low-cost and scalable CO₂ monitoring system that leverages NDIR sensors and a Long Short-Term Memory (LSTM) neural network to predict indoor CO₂ concentrations over both short- and long-term horizons. The proposed system aims to anticipate air quality deterioration in shared spaces, enabling proactive ventilation strategies. Various LSTM configurations were evaluated, optimizing the number of layers, neurons per layer, and input delays to enhance forecasting accuracy. The optimal model consisted of two LSTM layers with 128 neurons each and a time window of 10 previous observations. This model achieved an RMSE of approximately 57 ppm for an 8 h forecast in a classroom setting. Experimental results demonstrate the reliability of the proposed approach for CO₂ prediction and its potential impact on indoor air quality management. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

Figure 1

55 pages, 998 KiB

Open AccessArticle

Nonlinear Finite Element Model for FGM Porous Circular and Annular Micro-Plates Under Thermal and Mechanical Loads Using Modified Couple Stress-Based Third-Order Plate Theory

by Enrique Nava and Jinseok Kim

Math. Comput. Appl. 2025, 30(2), 35; https://doi.org/10.3390/mca30020035 - 26 Mar 2025

A nonlinear finite element model for circular and annular micro-plates under thermal and mechanical loading was developed using a third-order shear deformation theory. In the kinematic assumptions, a change in plate thickness is allowed, and no transverse shear strains are considered on the [...] Read more.

A nonlinear finite element model for circular and annular micro-plates under thermal and mechanical loading was developed using a third-order shear deformation theory. In the kinematic assumptions, a change in plate thickness is allowed, and no transverse shear strains are considered on the top and bottom surfaces. A power-law distribution was utilized to account for variations in two constituents through the thickness of the plate. Three different types of porosity distributions are considered. The strain gradient effect in micro-scale structures is accounted for by using the modified couple stress theory. Hamilton’s principle is used to obtain the equations of motion, and conforming plate elements are used in the development of the finite element model. The developed finite element model was verified against the available literature and analytical solutions. The effects of the material and porosity distribution, microstructure-dependency, geometric nonlinearity, and various boundary conditions on the bending response of functionally graded and porous circular and annular micro-plates were studied using the developed nonlinear finite element model. Full article

(This article belongs to the Special Issue Mathematical and Computational Approaches in Applied Mechanics: A Themed Issue Dedicated to Professor J.N. Reddy)

► Show Figures

Figure 1

23 pages, 3658 KiB

Open AccessArticle

MASIP: A Methodology for Assets Selection in Investment Portfolios

by José Purata-Aldaz, Juan Frausto-Solís, Guadalupe Castilla-Valdez, Javier González-Barbosa and Juan Paulo Sánchez Hernández

Math. Comput. Appl. 2025, 30(2), 34; https://doi.org/10.3390/mca30020034 - 24 Mar 2025

This paper proposes a Methodology for Assets Selection in Investment Portfolios (MASIP) focused on creating investment portfolios using heuristic algorithms based on the Markowitz and Sharpe models. MASIP selects and allocates financial assets by applying heuristic methods to accomplish three assignments: (a) Select [...] Read more.

This paper proposes a Methodology for Assets Selection in Investment Portfolios (MASIP) focused on creating investment portfolios using heuristic algorithms based on the Markowitz and Sharpe models. MASIP selects and allocates financial assets by applying heuristic methods to accomplish three assignments: (a) Select the stock candidates in an initial portfolio; (b) Forecast the asset values for the short and medium term; and (c) Optimize the investment portfolio by using the Sharpe metric. Once MASIP creates the initial portfolio and forecasts its assets, an optimization process is started in which a set with the best weights determines the participation of each asset. Moreover, a rebalancing process is carried out to enhance the portfolio value. We show that the improvement achieved by MASIP can reach 147% above the SP500 benchmark. We use a dataset of SP500 to compare MASIP with state-of-the-art methods, obtaining superior performance and an outstanding Sharpe Ratio and returns compared to traditional investment approaches. The heuristic algorithms proved effective in asset selection and allocation, and the forecasting process and rebalancing contributed to further improved results. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

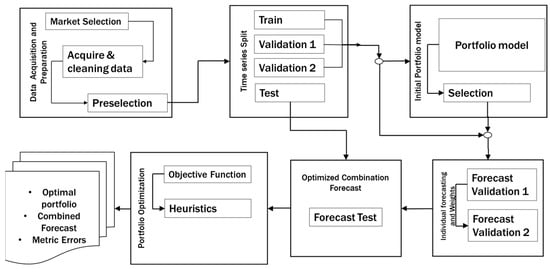
Figure 1

30 pages, 8417 KiB

Open AccessArticle

Toward a Blood Sensor for an IoT Monitoring: A New Approach for the Design and Implementation of Blood Light Absorption Systems Based on the Finite Element Method and the Diffusion Equation

by Mouna Dhmiri, Yassine Manai and Tahar Ezzedine

Math. Comput. Appl. 2025, 30(2), 33; https://doi.org/10.3390/mca30020033 - 24 Mar 2025

Non-invasive blood analysis has the power to completely change how doctors identify and track illnesses. This study presents a novel approach for the non-invasive monitoring of red blood cell (RBC) mobility and concentration within capillaries, using photon absorption as a key diagnostic tool. [...] Read more.

Non-invasive blood analysis has the power to completely change how doctors identify and track illnesses. This study presents a novel approach for the non-invasive monitoring of red blood cell (RBC) mobility and concentration within capillaries, using photon absorption as a key diagnostic tool. The research combines optical modeling with the diffusion equation for light propagation, leveraging COMSOL simulations to create a comprehensive framework for understanding RBC dynamics. A two-dimensional geometric model of capillaries with RBCs is developed, where blood flow is modeled as a laminar, incompressible fluid. The Arbitrary Lagrangian–Eulerian (ALE) formulation is employed to account for the fluid–structure interactions, while photon attenuation by the RBCs is analyzed to investigate wavelength-dependent absorption characteristics. The methodology is implemented through a workflow developed with MATLAB’s S-Function builder, consisting of three main components: mesh generation, fluence computing, and Software-in-the-Loop (SIL) verification. The mesh generation process adapts to the target architecture using COMSOL Multiphysics for fluid–structure interaction (FSI) modeling. The fluence computing function solves the diffusion equation to model light intensity attenuation due to RBCs, and the SIL function compares computed results with real-time measurements, ensuring accuracy for potential real-time embedded system applications. The results demonstrate significant wavelength-dependent variations in photon absorption by RBCs, providing insights into the optical behavior of blood in microvascular structures. The findings have important implications for medical imaging, photodynamic therapy, and diagnostic tools, emphasizing the potential of integrating computational models with real-time systems for enhanced performance in biomedical applications. Full article

(This article belongs to the Topic Numerical Methods for Partial Differential Equations)

► Show Figures

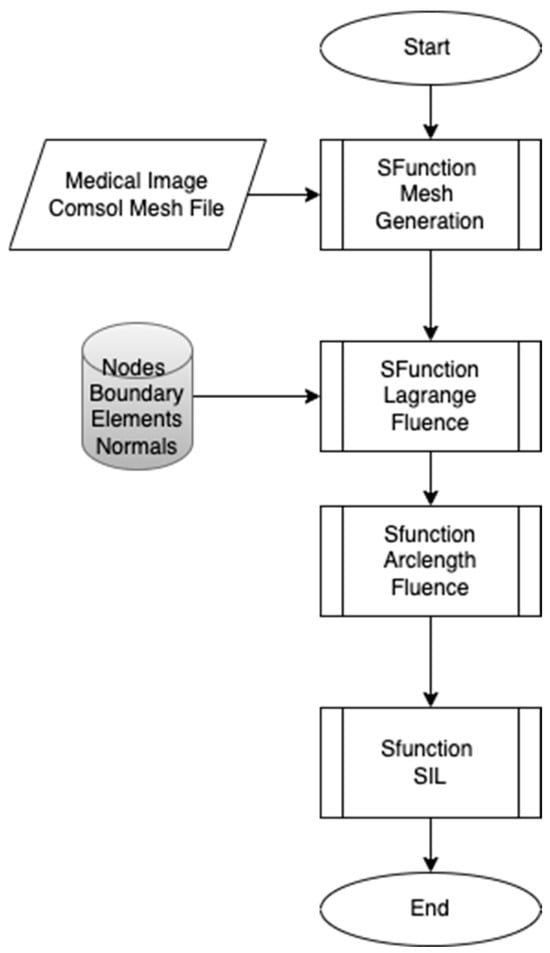
Figure 1

2 pages, 159 KiB

Open AccessCorrection

Correction: Zambou et al. Optimized Nonlinear PID Control for Maximum Power Point Tracking in PV Systems Using Particle Swarm Optimization. Math. Comput. Appl. 2024, 29, 88

by Maeva Cybelle Zoleko Zambou, Alain Soup Tewa Kammogne, Martin Siewe Siewe, Ahmad Taher Azar, Saim Ahmed and Ibrahim A. Hameed

Math. Comput. Appl. 2025, 30(2), 32; https://doi.org/10.3390/mca30020032 - 21 Mar 2025

Because of the uncertain meaning in the original publication [...] Full article

25 pages, 668 KiB

Open AccessArticle

An Experimental Study of Strategies to Control Diversity in Grouping Mutation Operators: An Improvement to the Adaptive Mutation Operator for the GGA-CGT for the Bin Packing Problem

by Stephanie Amador-Larrea, Marcela Quiroz-Castellanos and Octavio Ramos-Figueroa

Math. Comput. Appl. 2025, 30(2), 31; https://doi.org/10.3390/mca30020031 - 18 Mar 2025

Grouping Genetic Algorithms (GGAs) are among the most outstanding methods for solving NP-hard combinatorial optimization problems by efficiently grouping sets of items. Their performance depends on problem-specific heuristics and a balance between exploration and exploitation. The mutation operator plays a crucial role in [...] Read more.

Grouping Genetic Algorithms (GGAs) are among the most outstanding methods for solving NP-hard combinatorial optimization problems by efficiently grouping sets of items. Their performance depends on problem-specific heuristics and a balance between exploration and exploitation. The mutation operator plays a crucial role in exploring new solutions, but improper mutation control can lead to premature convergence. This work introduces adaptive mutation strategies for the GGA-CGT applied to the One-Dimensional Bin Packing Problem (1D-BPP). These strategies control the level of change that will be introduced to each solution dynamically by using feedback on population diversity, enabling better exploration. The proposed approach resulted in a 4.08% increase in optimal solutions (2227 across all classes) and a severe reduction in the average of individuals with equal fitness (from over 50% to less than 1%), enhancing diversity and avoiding local optima. The adaptive strategies were particularly effective in problem instances with larger item weights, where improvements were the most significant. Furthermore, statistical analysis confirmed the adaptive mutation approach’s superior performance compared with the original one. These findings demonstrate the potential of adaptive mechanisms to improve genetic algorithms, offering a robust strategy for tackling complex optimization problems. Full article

(This article belongs to the Special Issue Numerical and Evolutionary Optimization 2024)

► Show Figures

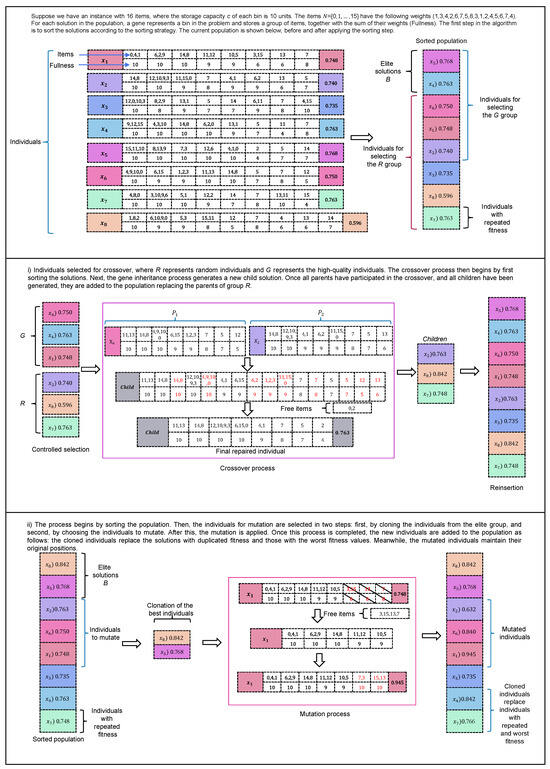
Figure 1

20 pages, 8271 KiB

Open AccessArticle

Deciding on the Regularity of a Planar Coons Map

by Maharavo Randrianarivony and Guido Brunnett

Math. Comput. Appl. 2025, 30(2), 30; https://doi.org/10.3390/mca30020030 - 17 Mar 2025

We consider the construction of a regular map from the unit square to a general four-sided domain, a problem that arises in several applications, e.g., in the numerical solution of integral equations or when dealing with trimmed surfaces in CAD. In our approach, [...] Read more.

We consider the construction of a regular map from the unit square to a general four-sided domain, a problem that arises in several applications, e.g., in the numerical solution of integral equations or when dealing with trimmed surfaces in CAD. In our approach, we consider the problem in as general a form as possible, which means that initially no assumptions are made about the type of mathematical representation of the boundary curves. This approach becomes possible by using planar Coons maps to describe the parameterization. We show that the regularity of a Coons map depends both on the waviness and the similarity of the boundary curves. Constraining these properties allows us to formulate sufficient conditions for regularity and to specify special cases in which the regularity of the Coons map is obvious. For the case of polynomial boundary curves, we present a regularity criterion that is both necessary and sufficient and can thus be used to characterize regular polynomial mappings. Our decision algorithm implements this criterion and provides a powerful tool not only for deciding the regularity of a given Coons map, but also for determining the transition between a regular and a non-regular Coons map depending on the curvature of the boundary curves. Full article

► Show Figures

Figure 1

21 pages, 519 KiB

Open AccessArticle

Learning Deceptive Tactics for Defense and Attack in Bayesian–Markov Stackelberg Security Games

by Julio B. Clempner

Math. Comput. Appl. 2025, 30(2), 29; https://doi.org/10.3390/mca30020029 - 17 Mar 2025

In this paper, we address the challenges posed by limited knowledge in security games by proposing a novel system grounded in Bayesian–Markov Stackelberg security games (SSGs). These SSGs involve multiple defenders and attackers and serve as a framework for managing incomplete information effectively. [...] Read more.

In this paper, we address the challenges posed by limited knowledge in security games by proposing a novel system grounded in Bayesian–Markov Stackelberg security games (SSGs). These SSGs involve multiple defenders and attackers and serve as a framework for managing incomplete information effectively. To tackle the complexity inherent in these games, we introduce an iterative proximal-gradient approach to compute the Bayesian Equilibrium, which captures the optimal strategies of both defenders and attackers. This method enables us to navigate the intricacies of the game dynamics, even when the specifics of the Markov games are unknown. Moreover, our research emphasizes the importance of Bayesian approaches in solving the reinforcement learning (RL) algorithm, particularly in addressing the exploration–exploitation trade-off. By leveraging Bayesian techniques, we aim to minimize the expected total discounted costs, thus optimizing decision-making in the security domain. In pursuit of effective security game implementation, we propose a novel random walk approach tailored to fulfill the requirements of the scenario. This innovative methodology enhances the adaptability and responsiveness of defenders and attackers, thereby improving overall security outcomes. To validate the efficacy of our proposed strategy, we provide a numerical example that demonstrates its benefits in practice. Through this example, we showcase how our approach can effectively address the challenges posed by limited knowledge, leading to more robust and efficient security solutions. Overall, our paper contributes to advancing the understanding and implementation of security strategies in scenarios characterized by incomplete information. By combining Bayesian and Markov Stackelberg games, reinforcement learning algorithms, and innovative random walk techniques, we offer a comprehensive framework for enhancing security measures in real-world applications. Full article

(This article belongs to the Special Issue Applied Optimization in Automatic Control and Systems Engineering)

► Show Figures

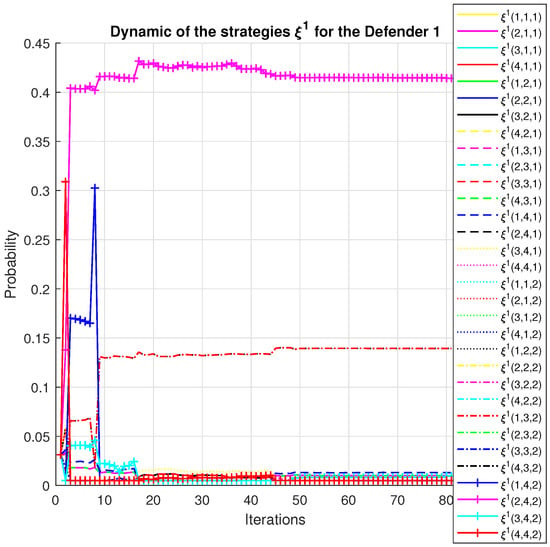
Figure 1

15 pages, 1443 KiB

Open AccessArticle

Characterization of the Appointment’s Reasons for “P—Psychological” on the ICPC-2 Scale in Primary Health Care Services

by Filipa Rocha, Cristiana J. Silva, Sofia J. Pinheiro, Vera Afreixo, Rui Pedro Leitão and Miguel Felgueiras

Math. Comput. Appl. 2025, 30(2), 28; https://doi.org/10.3390/mca30020028 - 14 Mar 2025

(1) Background: Mental health significantly impacts personal relationships and societal integration. Portugal faces a high prevalence of psychiatric illnesses and psychological distress, which the COVID-19 pandemic might have exacerbated. Therefore, this study aims to study risk factors that lead to psychological problems, using [...] Read more.

(1) Background: Mental health significantly impacts personal relationships and societal integration. Portugal faces a high prevalence of psychiatric illnesses and psychological distress, which the COVID-19 pandemic might have exacerbated. Therefore, this study aims to study risk factors that lead to psychological problems, using data available in the primary health care centers of the region of Aveiro. (2) Methods: This observational and retrospective study analyzes data from 2009 to 2022 on psychological consultations in the Aveiro municipalities. Variables considered are municipality, International Classification of Primary Care problem, sex, and comorbidities (cancer, obesity, and diabetes). Summary statistics and graphs were employed for data understanding, with R software used for analysis. Regression models, odds ratios, and association tests were calculated. Also, cluster analysis was performed on municipalities. (3) Results: A new, significant increase in the appointment growth rate was observed in 2021 and 2022. Anxiety and depressive disorders contribute to the identified growth. Women reported more problems than men. Cancer was the most present comorbidity. (4) Conclusions: The study reveals increased mental health problems, with primary health care users in Aveiro experiencing worsened psychosocial health, resulting in more medical consultations for psychological reasons. Risk factors included being female and having chronic conditions such as cancer. The findings provide insights into the burden of mental health issues in the region, highlighting the need for effective mental health interventions and resources to address health inequalities and support at-risk groups. Full article

(This article belongs to the Special Issue Feature Papers in Mathematical and Computational Applications 2025)

► Show Figures

Figure 1

21 pages, 597 KiB

Open AccessArticle

Common Attractor for Hutchinson θ-Contractive Operators in Partial Metric Spaces

by Naila Shabir, Ali Raza, Manuel De la Sen, Mujahid Abbas and Shahbaz Ahmad

Math. Comput. Appl. 2025, 30(2), 27; https://doi.org/10.3390/mca30020027 - 14 Mar 2025

This paper investigates the existence of common attractors for generalized

θ

-Hutchinson operators within the framework of partial metric spaces. Utilizing a finite iterated function system composed of

θ

-contractive mappings, we establish theoretical results on common attractors, generalizing numerous existing results in [...] Read more.

This paper investigates the existence of common attractors for generalized

θ

-Hutchinson operators within the framework of partial metric spaces. Utilizing a finite iterated function system composed of

θ

-contractive mappings, we establish theoretical results on common attractors, generalizing numerous existing results in the literature. Additionally, to enhance understanding, we present intuitive and easily comprehensible examples in one-, two-, and three-dimensional Euclidean spaces. These examples are accompanied by graphical representations of attractor images for various iterated function systems. As a practical application, we demonstrate how our findings contribute to solving a functional equation arising in a dynamical system, emphasizing the broader implications of the proposed approach. Full article

► Show Figures

Figure 1

More Articles...

Submit to MCA Review for MCA

Journal Menu

Journal Browser

► Journal Browser

Highly Accessed Articles

View More...

Latest Books

More Books and Reprints...

E-Mail Alert

News

3 April 2025
Meet Us at Math 2 Product (M2P), 4–6 June 2025, Valencia, Spain

2 April 2025
MDPI INSIGHTS: The CEO's Letter #21 - Annual Report, Swiss Consortium, IWD, ICARS, Serbia

5 March 2025
Meet Us at the XI International Conference on Coupled Problems in Science and Engineering (COUPLED PROBLEMS 2025), 25–28 May 2025, Villasimius, Italy

More News & Announcements...

Topics

Propose a Topic

Topic in AppliedMath, Axioms, Fractal Fract, MCA, Mathematics, Symmetry

Fractional Calculus, Symmetry Phenomenon and Probability Theory for PDEs, and ODEs Topic Editors: Renhai Wang, Junesang Choi
Deadline: 30 April 2025

Topic in Axioms, Computation, Entropy, MCA, Mathematics, Symmetry

Numerical Methods for Partial Differential Equations Topic Editors: Pengzhan Huang, Yinnian He
Deadline: 30 June 2025

Conferences

Announce Your Conference

15–16 October 2025 The 1st International Electronic Conference on Games (IECGA 2025)

More Conferences...

Special Issues

Propose a Special Issue

Special Issue in MCA

Advanced Computing Methods for Environmental Sustainability Guest Editors: Rana Muhammad Adnan, Ozgur Kisi, Mo Wang
Deadline: 15 May 2025

Special Issue in MCA

Numerical and Symbolic Computation: Developments and Applications 2025 Guest Editor: Maria Amélia Ramos Loja
Deadline: 15 August 2025

Special Issue in MCA

AI Text: Text Mining, Tools, Algorithms, Applications, and Challenges Guest Editors: Xuequn Shang, Samer Waheeb
Deadline: 15 August 2025

Special Issue in MCA

Latest Research in Mathematical Modeling in Cancer Research Guest Editor: Ophir Nave
Deadline: 30 November 2025

More Special Issues

Topical Collections

Topical Collection in MCA

Mathematical Modelling of COVID-19 Collection Editor: Cristiana da Silva

Topical Collection in MCA

Numerical Optimization Reviews Collection Editor: Oliver Schütze

Back to TopTop