A Dynamic Contest Model of Platform Competition in Two-Sided Markets
Abstract
:1. Introduction
2. Related Literature
3. Model
4. Results
4.1. Optimality Conditions
4.1.1. Optimal Behavior in Period 2
4.1.2. Optimal Behavior in Period 1
- In the open-loop equilibrium, managers do not take into account—by implication of the equilibrium concept—their strategic option in Period 1 to change the opponent’s incentive to invest in the platform in Period 2 by changing own platform investments in Period 1. In this case, such that holds.
- In the closed-loop equilibrium, however, this strategic option is considered, and the terms and do not have to be zero. See [45], who provide a formal description of the two equilibrium concepts.
4.2. Linear Costs and Heterogeneity
- (i)
- A unique equilibrium exists, and the closed-loop equilibrium coincides with the open-loop equilibrium.
- (ii)
- The optimal asset stocks of platformin Period 2 are given by the following:
- (iii)
- The optimal asset stocks of platformin Period 1 are given by the following:
- (i)
- The asset stocks for both platforms are larger in Period 1 than in Period 2, i.e.,.
- (ii)
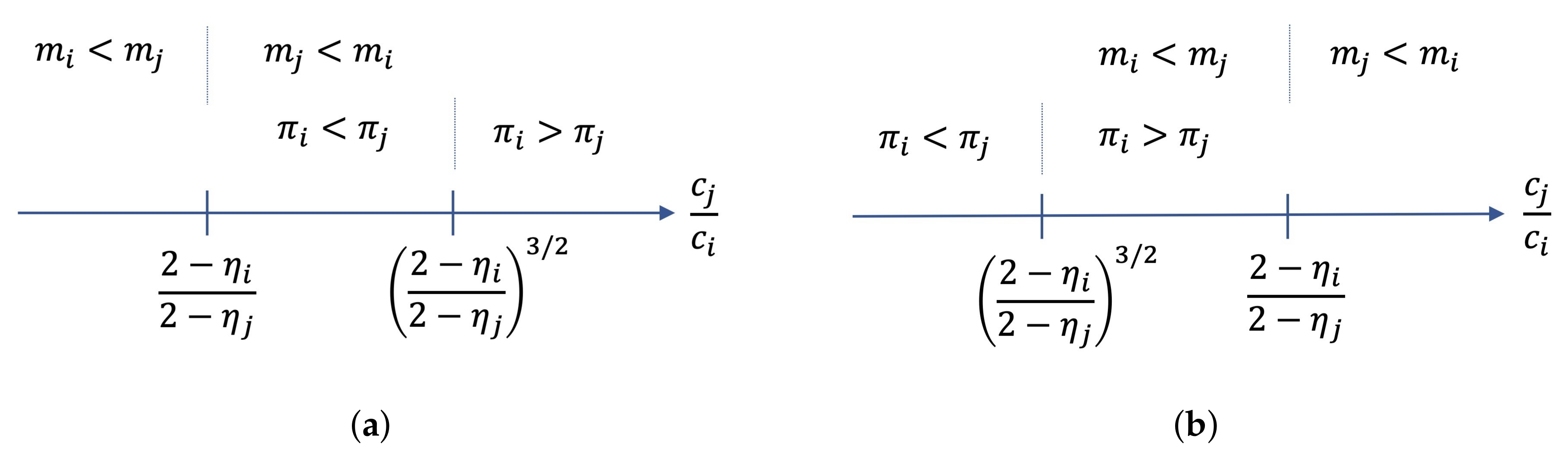
- The asset stock of platform i is larger than the asset stock of platform j in periodiff.
- (iii)
- Stronger network effects on platform i always increase the asset stock of platform i, while stronger network effects on platform j increase the asset stock of platform i iff.
- (iv)
- The winning probability of platform i increases with larger network effects on the own platform and it decreases with stronger network effects on the other platform, i.e.,and. Hence, larger network effectson platform i increase the balance of the contest if.
- (i)
- In Period 1, the manager of platform i invests the following:to build up asset stock given by.
- (ii)
- In Period 2, the manager of platform i invests the following:to build up asset stock given by
- (i)
- Expected profits of platformin equilibrium are given by the following:withand.
- (ii)
- We derive the following comparative statics:
4.3. Quadratic Costs and Homogeneity
- (i)
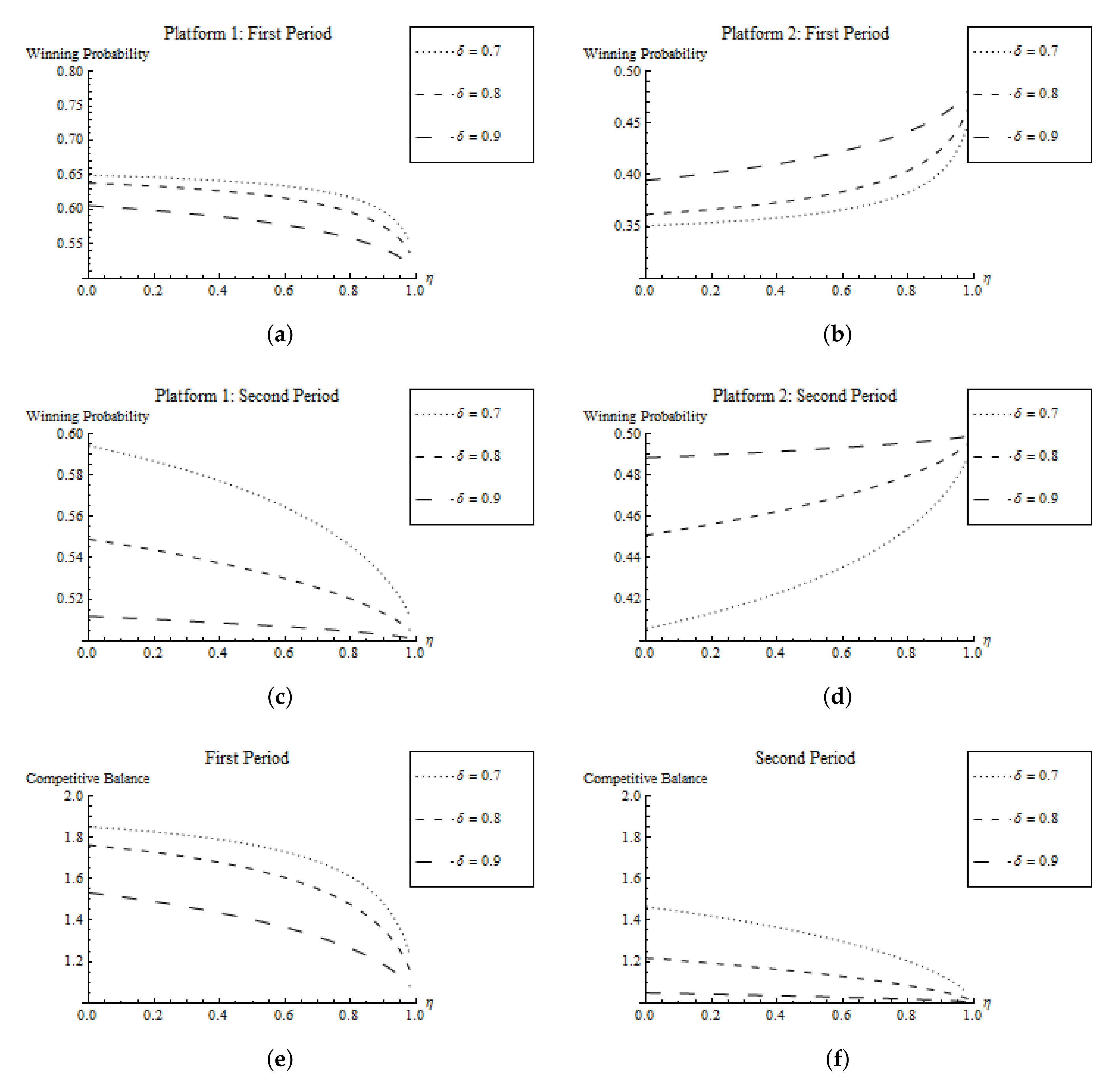
- A lower depreciation rate δ or stronger network effects η increase asset stocksfor platform i in period t.
- (ii)
- A higher depreciation rate δ or stronger network effects η increase the speed of convergence of asset stocksfor platform i in period t.
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PC | Personal Computer |
| VHS | Video Home System |
| OTT | Over-The-Top |
| CSF | Contest Success Function |
| CB | Competitive Balance |
Appendix A
Appendix A.1. Proof of Lemma 1
Appendix A.2. Proof of Lemma 4
References
- Wright, J. One-Sided Logic in Two-Sided Markets. Rev. Netw. Econ. 2004, 3, 44–64. [Google Scholar] [CrossRef]
- Rysman, M. The Economics of Two-Sided Markets. J. Econ. Perspect. 2009, 23, 125–143. [Google Scholar] [CrossRef] [Green Version]
- Ihlström Eriksson, C.; Akesson, M.; Lund, J. Designing Ubiquitous Media Services-Exploring the Two-Sided Market of Newspapers. J. Theor. Appl. Electron. Commer. Res. 2016, 11, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Landsman, V.; Stremersch, S. Multihoming in Two-Sided Markets: An Empirical Inquiry in the Video Game Console Industry. J. Mark. 2011, 75, 39–54. [Google Scholar] [CrossRef]
- Netflix Is Still Growing Wildly, but Its Market Share Has Fallen to an Estimated 19% as New Competitors Emerge. Available online: https://www.businessinsider.com/netflix-market-share-of-global-streaming-subscribers-dropping-ampere-2020-1 (accessed on 1 May 2021).
- Staykova, K.; Damsgaard, J. A 2020 Perspective on “the Race to Dominate the Mobile Payments Platform: Entry and Expansion Strategies”. Electron. Commer. Res. Appl. 2020, 41, 100954. [Google Scholar] [CrossRef]
- Staykova, K.S.; Damsgaard, J. Adoption of Mobile Payment Platforms: Managing Reach and Range. J. Theor. Appl. Electron. Commer. Res. 2016, 11, 65–84. [Google Scholar] [CrossRef] [Green Version]
- Evans, D.; Schmalensee, R. The Antitrust Analysis of Multisided Platform Businesses. In Oxford Handbook on International Antitrust Economics; Blair, R.D., Sokol, D.D., Eds.; Oxford University Press: Oxford, UK, 2014. [Google Scholar]
- Pontual Ribeiro, E.; Golovanova, S. A Unified Presentation Of Competition Analysis In Two-Sided Markets. J. Econ. Surv. 2020, 34, 548–571. [Google Scholar] [CrossRef]
- Wilbur, K.C. A Two-Sided, Empirical Model of Television Advertising and Viewing Markets. Mark. Sci. 2008, 27, 356–378. [Google Scholar] [CrossRef] [Green Version]
- Liu, H. Dynamics of Pricing in the Video Game Console Market: Skimming or Penetration? J. Mark. Res. 2010, 47, 428–443. [Google Scholar] [CrossRef]
- Chu, J.; Manchanda, P. Quantifying Cross and Direct Network Effects in Online Consumer-to-Consumer Platforms. Mark. Sci. 2016, 35, 870–893. [Google Scholar] [CrossRef]
- Hinz, O.; Otter, T.; Skiera, B. Estimating Network Effects in Two-Sided Markets. J. Manag. Inform. Syst. 2020, 37, 12–38. [Google Scholar] [CrossRef]
- Rochet, J.C.; Tirole, J. Two-Sided Markets: A Progress Report. RAND J. Econ. 2006, 37, 645–667. [Google Scholar] [CrossRef] [Green Version]
- Weyl, E.G. A Price Theory of Multi-Sided Platforms. Am. Econ. Rev. 2010, 100, 1642–1672. [Google Scholar] [CrossRef]
- Roger, G.; Vasconcelos, L. Platform Pricing Structure and Moral Hazard. J. Econ. Manag. Strategy 2014, 23, 527–547. [Google Scholar] [CrossRef] [Green Version]
- Ribeiro, V.M.; Bao, L. Professionalization of Online Gaming? Theoretical and Empirical Analysis for a Monopoly-Holding Platform. J. Theor. Appl. Electron. Commer. Res. 2021, 16, 682–708. [Google Scholar] [CrossRef]
- Caillaud, B.; Jullien, B. Competing Cybermediaries. Eur. Econ. Rev. 2001, 45, 797–808. [Google Scholar] [CrossRef] [Green Version]
- Jullien, B. Competition in multi-sided markets: Divide and conquer. Am. Econ. J. Macroecon. 2011, 3, 186–220. [Google Scholar] [CrossRef]
- Hossain, T.; Morgan, J. When Do Markets Tip? A Cognitive Hierarchy Approach. Mark. Sci. 2013, 32, 431–453. [Google Scholar] [CrossRef] [Green Version]
- Caillaud, B.; Jullien, B. Chicken & Egg: Competition among Intermediation Service Providers. RAND J. Econ. 2003, 34, 309–328. [Google Scholar]
- Rochet, J.C.; Tirole, J. Platform Competition in Two-Sided Markets. J. Eur. Econ. Assoc. 2003, 1, 990–1029. [Google Scholar] [CrossRef] [Green Version]
- Armstrong, M. Competition in Two-Sided Markets. RAND J. Econ. 2006, 37, 668–691. [Google Scholar] [CrossRef] [Green Version]
- Liu, Q.; Serfes, K. Price Discrimination in Two-Sided Markets. J. Econ. Manag. Strategy 2013, 22, 768–786. [Google Scholar] [CrossRef]
- Lee, R.S. Competing Platforms. J. Econ. Manag. Strategy 2014, 23, 507–526. [Google Scholar] [CrossRef]
- Hałaburda, H.; Yehezkel, Y. The Role of Coordination Bias in Platform Competition. J. Econ. Manag. Strategy 2016, 25, 274–312. [Google Scholar] [CrossRef] [Green Version]
- Liu, Q. Stability and Bayesian Consistency in Two-Sided Markets. Am. Econ. Rev. 2020, 110, 2625–2666. [Google Scholar] [CrossRef]
- Konrad, K.A. Strategy and Dynamics in Contests; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Clark, D.J.; Nilssen, T. Learning by Doing in Contests. Public Choice 2013, 156, 329–343. [Google Scholar] [CrossRef]
- Grossmann, M.; Lang, M.; Dietl, H.M. Transitional Dynamics in a Tullock Contest with a General Cost Function. BE J. Theor. Econ. 2011, 11. [Google Scholar] [CrossRef] [Green Version]
- Grossmann, M.; Dietl, H.M. Investment Behaviour in a Two-Period Contest Model. J. Inst. Theor. Econ. 2009, 165, 401–417. [Google Scholar] [CrossRef]
- Yildirim, H. Contests with Multiple Rounds. Games Econ. Behav. 2005, 51, 213–227. [Google Scholar] [CrossRef] [Green Version]
- Baik, K.H.; Lee, S. Two-Stage Rent-Seeking Contests with Carryovers. Public Choice 2000, 103, 285–296. [Google Scholar] [CrossRef]
- Lee, S. Two-Stage Contests with Additive Carryovers. Int. Econ. J. 2003, 17, 83–99. [Google Scholar] [CrossRef]
- Keskin, K.; Saglam, C. Investment on Human Capital in a Dynamic Contest Model. Stud. Nonlinear Dyn. Econom. 2019, 23, 20170095. [Google Scholar] [CrossRef] [Green Version]
- Matsumoto, A.; Szidarovszky, F. Dynamic Contest Games with Time Delays. Int. Game Theory Rev. 2020, 22, 1950017. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, G.; Xu, J.; Qu, C. Dynamic Contest Model with Bounded Rationality. Appl. Math. Comput. 2020, 370, 124909. [Google Scholar] [CrossRef]
- Tullock, G. Efficient Rent Seeking. In Efficient Rent-Seeking: Chronicle of an Intellectual Quagmire; Lockard, A.A., Tullock, G., Eds.; Springer US: Boston, MA, USA, 1980; pp. 3–16. [Google Scholar]
- Skaperdas, S. Contest Success Functions. Econ. Theory 1996, 7, 283–290. [Google Scholar] [CrossRef]
- Clark, D.J.; Riis, C. Contest Success Functions: An Extension. Econ. Theory 1998, 11, 201–204. [Google Scholar] [CrossRef]
- Lazear, E.P.; Rosen, S. Rank-Order Tournaments as Optimum Labor Contracts. J. Pol. Econ. 1981, 89, 841–864. [Google Scholar] [CrossRef] [Green Version]
- Dixit, A. Strategic Behavior in Contests. Am. Econ. Rev. 1987, 77, 891–898. [Google Scholar]
- Hirshleifer, J. Conflict and Rent-Seeking Success Functions: Ratio Vs. Difference Models of Relative Success. Public Choice 1989, 63, 101–112. [Google Scholar] [CrossRef]
- Runkel, M. Total Effort, Competitive Balance and the Optimal Contest Success Function. Eur. J. Political Econ. 2006, 22, 1009–1013. [Google Scholar] [CrossRef]
- Fudenberg, D.; Tirole, J. Game Theory; The MIT Press: Cambridge, MA, USA, 1991. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grossmann, M.; Lang, M.; Dietl, H.M. A Dynamic Contest Model of Platform Competition in Two-Sided Markets. J. Theor. Appl. Electron. Commer. Res. 2021, 16, 2091-2109. https://doi.org/10.3390/jtaer16060117
Grossmann M, Lang M, Dietl HM. A Dynamic Contest Model of Platform Competition in Two-Sided Markets. Journal of Theoretical and Applied Electronic Commerce Research. 2021; 16(6):2091-2109. https://doi.org/10.3390/jtaer16060117
Chicago/Turabian StyleGrossmann, Martin, Markus Lang, and Helmut M. Dietl. 2021. "A Dynamic Contest Model of Platform Competition in Two-Sided Markets" Journal of Theoretical and Applied Electronic Commerce Research 16, no. 6: 2091-2109. https://doi.org/10.3390/jtaer16060117
APA StyleGrossmann, M., Lang, M., & Dietl, H. M. (2021). A Dynamic Contest Model of Platform Competition in Two-Sided Markets. Journal of Theoretical and Applied Electronic Commerce Research, 16(6), 2091-2109. https://doi.org/10.3390/jtaer16060117





