Thermoelectrics of Interacting Nanosystems—Exploiting Superselection Instead of Time-Reversal Symmetry
Abstract
:1. Introduction
1.1. Motivation and General Outline
1.2. Overview of Main Ideas and Results
- (1)
- The duality relation maps the eigenmodes of the system of interest to the amplitudes for a different physical system—the dual system. We will refer to the latter as the inverted system because the duality transformation inverts the interaction (as well as other energies), going from repulsive to attractive and vice versa. For quantum dots, this can be easily visualized by inverting the energy landscape in Figure 1a to that of Figure 1c, whose details will be discussed later on. This mapping already explains the seemingly strange occurrence of features of attractive interaction in quantities computed for repulsive systems, as first noted in [29,34]. The straightforward interpretation of such puzzling properties is done resorting to the inverted stationary state, which allows to understand the nontrivial dependence on the original system’s parameters from the—often simple and well-understood [41]—physics of the attractive dual model as in Figure 1c.
- (2)
- Another reason why the duality clarifies interaction effects is that the “essential” correlating parts of the Coulomb interactions, say, between two orbitals i and j with occupation operators and , respectively, is simply given by parity operators, . In fact, correlated electron model Hamiltonians are often directly formulated in terms of the operators on the left hand side. The duality reveals that the total parity operator always corresponds to a special eigenmode of open fermion-system dynamics [36,37], and is hence protected. In simple yet relevant situations, one thereby cleanly separates, throughout the entire calculation, the contributions of the Coulomb interaction into an “essential” correlating part and a nontrivial “average” contribution carried by a charge mode. Since Coulomb interaction is an important source of energy dependence and energy storage in quantum dots, thermoelectrics is thus seen to be intimately tied up with fermion parity and the corresponding duality.
- (3)
- Finally, in the context of thermoelectricity, it is important to emphasize that the duality—in the simple form used here—requires energy-independent coupling between system and reservoir (wide-band limit). This does not mean that it is irrelevant to thermoelectric transport, where properly engineered energy-dependence of the coupling can be of interest for the device operation, see, e.g., References [20,21,23,24,25]. Here, the nanoscale system itself provides the strong energy dependence required for thermoelectric effects, both through strong size-quantization and Coulomb interaction. Models of this kind are relevant in many thermoelectric studies [38,42,43,44,45] and the duality applies to their description, even when the energy-independent coupling is strong and the temperature is low [29], cf. [36,37]. Also, effective energy-dependent couplings as realized in multi-dot systems [43,44] can be treated in terms of the duality relation presented here. Finally, the duality considerations can be extended [46] systematically to account for the energy-dependence of the coupling.
2. Model, Master Equation, and Duality
2.1. Model, Assumptions, and Notation
2.2. Master Equation and Non-Equilibrium Currents
2.3. Fermion-Parity Duality and Its Use in Thermoelectrics
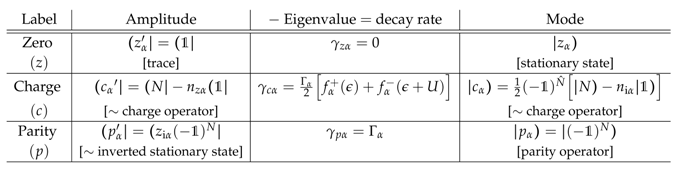
2.4. Charge and Energy Currents
3. Linear Response Regime
3.1. Electric Response
3.2. Thermo-Electric Response and Seebeck Thermopower
3.3. Peltier Coefficient
3.4. Thermal Response and Fourier Heat Conductance
4. Nonlinear Regime
4.1. Thermo-Electric Response and Seebeck Thermopower
4.2. Electro-Thermal Response and Peltier Coefficient
4.3. Thermal Response and Fourier Coefficient
5. Conclusions and Outlook
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Non-Equilibrium Master Equation Kernel from Physical Principles and Symmetries
Appendix A.1. Kernel for a Single Lead

Appendix A.2. Full Multi-Lead Kernel
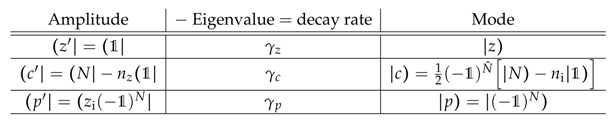
Appendix B. Explicit Expressions for the Kernel
Appendix C. Derivation of the Linear Response Coefficients
Appendix C.1. First Derivatives at Equilibrium
Appendix C.2. Linearized Charge Current and Conductance
Appendix C.3. Charge-Energy Correlation and Seebeck Effect
Appendix C.4. Peltier Effect and Fourier Heat
Appendix D. Non-Equilibrium Relations for Current-Balanced System
References and Notes
- Sothmann, B.; Sánchez, R.; Jordan, A.N. Thermoelectric energy harvesting with quantum dots. Nanotechnology 2015, 26, 032001. [Google Scholar] [CrossRef] [PubMed]
- Benenti, G.; Casati, G.; Saito, K.; Whitney, R.S. Fundamental aspects of steady-state conversion of heat to work at the nanoscale. Phys. Rep. 2017, 694, 1–124. [Google Scholar] [CrossRef]
- Scheibner, R.; König, M.; Reuter, D.; Wieck, A.D.; Gould, C.; Buhmann, H.; Molenkamp, L.W. Quantum dot as thermal rectifier. New J. Phys. 2008, 10, 083016. [Google Scholar] [CrossRef]
- Esposito, M.; Lindenberg, K.; Van den Broeck, C. Thermoelectric efficiency at maximum power in a quantum dot. EPL 2009, 85, 60010. [Google Scholar] [CrossRef]
- Wierzbicki, M.; Świrkowicz, R. Electric and thermoelectric phenomena in a multilevel quantum dot attached to ferromagnetic electrodes. Phys. Rev. B 2010, 82, 165334. [Google Scholar] [CrossRef]
- Kennes, D.M.; Schuricht, D.; Meden, V. Efficiency and power of a thermoelectric quantum dot device. EPL 2013, 102, 57003. [Google Scholar] [CrossRef]
- Sánchez, D.; López, R. Scattering Theory of Nonlinear Thermoelectric Transport. Phys. Rev. Lett. 2013, 110, 026804. [Google Scholar] [CrossRef] [PubMed]
- Meair, J.; Jacquod, P. Scattering theory of nonlinear thermoelectricity in quantum coherent conductors. J. Phys. Condens. Matter 2013, 25, 082201. [Google Scholar] [CrossRef] [PubMed]
- Svensson, S.F.; Hoffmann, E.A.; Nakpathomkun, N.; Wu, P.M.; Xu, H.Q.; Nilsson, H.A.; Sánchez, D.; Kashcheyevs, V.; Linke, H. Nonlinear thermovoltage and thermocurrent in quantum dots. New J. Phys. 2013, 15, 105011. [Google Scholar] [CrossRef]
- Sánchez, D.; López, R. Nonlinear phenomena in quantum thermoelectrics and heat. C.R. Phys. 2016, 17, 1060–1071. [Google Scholar] [CrossRef]
- Erdman, P.A.; Mazza, F.; Bosisio, R.; Benenti, G.; Fazio, R.; Taddei, F. Thermoelectric properties of an interacting quantum dot based heat engine. Phys. Rev. B 2017, 95, 245432. [Google Scholar] [CrossRef]
- Shiraishi, N. Stationary engines in and beyond the linear response regime at the Carnot efficiency. Phys. Rev. E 2017, 95, 052128. [Google Scholar] [CrossRef] [PubMed]
- Josefsson, M.; Svilans, A.; Burke, A.M.; Hoffmann, E.A.; Fahlvik, S.; Thelander, C.; Leijnse, M.; Linke, H. A quantum-dot heat engine operated close to thermodynamic efficiency limits. arXiv, 2017; arXiv:1710.00742. [Google Scholar]
- Saito, K.; Utsumi, Y. Symmetry in full counting statistics, fluctuation theorem, and relations among nonlinear transport coefficients in the presence of a magnetic field. Phys. Rev. B 2008, 78, 115429. [Google Scholar] [CrossRef]
- Förster, H.; Büttiker, M. Fluctuation Relations Without Microreversibility in Nonlinear Transport. Phys. Rev. Lett. 2008, 101, 136805. [Google Scholar] [CrossRef] [PubMed]
- Esposito, M.; Harbola, U.; Mukamel, S. Nonequilibrium fluctuations, fluctuation theorems, and counting statistics in quantum systems. Rev. Mod. Phys. 2009, 81, 1665–1702. [Google Scholar] [CrossRef]
- Silaev, M.; Heikkilä, T.T.; Virtanen, P. Lindblad-equation approach for the full counting statistics of work and heat in driven quantum systems. Phys. Rev. E 2014, 90, 022103. [Google Scholar] [CrossRef] [PubMed]
- Utsumi, Y.; Entin-Wohlman, O.; Aharony, A.; Kubo, T.; Tokura, Y. Fluctuation theorem for heat transport probed by a thermal probe electrode. Phys. Rev. B 2014, 89, 205314. [Google Scholar] [CrossRef]
- Karlström, O.; Linke, H.; Karlström, G.; Wacker, A. Increasing thermoelectric performance using coherent transport. Phys. Rev. B 2011, 84, 113415. [Google Scholar] [CrossRef]
- Sánchez, R.; Büttiker, M. Optimal energy quanta to current conversion. Phys. Rev. B 2011, 83, 085428. [Google Scholar] [CrossRef]
- Sothmann, B.; Sánchez, R.; Jordan, A.N.; Büttiker, M. Rectification of thermal fluctuations in a chaotic cavity heat engine. Phys. Rev. B 2012, 85, 205301. [Google Scholar] [CrossRef]
- Whitney, R.S.; Sánchez, R.; Haupt, F.; Splettstoesser, J. Thermoelectricity without absorbing energy from the heat sources. Phys. E 2016, 75, 257–265. [Google Scholar] [CrossRef]
- Thierschmann, H.; Sánchez, R.; Sothmann, B.; Arnold, F.; Heyn, C.; Hansen, W.; Buhmann, H.; Molenkamp, L.W. Three-terminal energy harvester with coupled quantum dots. Nat. Nanotechnol. 2015, 10, 854–858. [Google Scholar] [CrossRef] [PubMed]
- Hartmann, F.; Pfeffer, P.; Höfling, S.; Kamp, M.; Worschech, L. Voltage Fluctuation to Current Converter with Coulomb-Coupled Quantum Dots. Phys. Rev. Lett. 2015, 114, 146805. [Google Scholar] [CrossRef] [PubMed]
- Roche, B.; Roulleau, P.; Jullien, T.; Jompol, Y.; Farrer, I.; Ritchie, D.A.; Glattli, D.C. Harvesting dissipated energy with a mesoscopic ratchet. Nat. Commun. 2015, 6, 7738. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Butcher, P.N. Thermal and electrical transport formalism for electronic microstructures with many terminals. J. Phys. Condens. Matter 1990, 2, 4869. [Google Scholar] [CrossRef]
- Jacquod, P.; Whitney, R.S.; Meair, J.; Büttiker, M. Onsager relations in coupled electric, thermoelectric, and spin transport: The tenfold way. Phys. Rev. B 2012, 86, 155118. [Google Scholar] [CrossRef]
- Schulenborg, J.; Saptsov, R.B.; Haupt, F.; Splettstoesser, J.; Wegewijs, M.R. Fermion-parity duality and energy relaxation in interacting open systems. Phys. Rev. B 2016, 93, 081411. [Google Scholar] [CrossRef]
- Wick, G.C.; Wightman, A.S.; Wigner, E.P. The Intrinsic Parity of Elementary Particles. Phys. Rev. 1952, 88, 101–105. [Google Scholar] [CrossRef]
- Aharonov, Y.; Susskind, L. Charge Superselection Rule. Phys. Rev. 1967, 155, 1428–1431. [Google Scholar] [CrossRef]
- Streater, R.F.; Wightman, A.S. PCT, Spin and Statistics, and All That; Princeton University Press: Princeton, NJ, USA, 2000. [Google Scholar]
- Bogolubov, N.N.; Logunov, A.A.; Oksak, A.I.; Todorov, I. General Principles of Quantum Field Theory; Springer: Dordrecht, The Netherlands, 1990. [Google Scholar]
- Vanherck, J.; Schulenborg, J.; Saptsov, R.B.; Splettstoesser, J.; Wegewijs, M.R. Relaxation of quantum dots in a magnetic field at finite bias—Charge, spin, and heat currents. Phys. Status Solidi B 2017, 254, 1600614. [Google Scholar] [CrossRef]
- Andergassen, S.; Costi, T.A.; Zlatić, V. Mechanism for large thermoelectric power in molecular quantum dots described by the negative-U Anderson model. Phys. Rev. B 2011, 84, 241107. [Google Scholar] [CrossRef]
- Saptsov, R.B.; Wegewijs, M.R. Fermionic superoperators for zero-temperature nonlinear transport: Real-time perturbation theory and renormalization group for Anderson quantum dots. Phys. Rev. B 2012, 86, 235432. [Google Scholar] [CrossRef]
- Saptsov, R.B.; Wegewijs, M.R. Time-dependent quantum transport: Causal superfermions, exact fermion-parity protected decay modes, and Pauli exclusion principle for mixed quantum states. Phys. Rev. B 2014, 90, 045407. [Google Scholar]
- Sierra, M.A.; Saiz-Bretín, M.; Domínguez-Adame, F.; Sánchez, D. Interactions and thermoelectric effects in a parallel-coupled double quantum dot. Phys. Rev. B 2016, 93, 235452. [Google Scholar] [CrossRef]
- Vasquez Jaramillo, J.D.; Fransson, J. Magnetic control of heat and charge transport in a paramagnetic molecular dimer. arXiv, 2017; arXiv:1702.05389. [Google Scholar]
- Rosselló, G.; López, R.; Sánchez, R. Dynamical Coulomb blockade of thermal transport. Phys. Rev. B 2017, 95, 235404. [Google Scholar] [CrossRef]
- Anderson, P.W. Model for the Electronic Structure of Amorphous Semiconductors. Phys. Rev. Lett. 1975, 34, 953. [Google Scholar] [CrossRef]
- Crépieux, A.; Šimkovic, F.; Cambon, B.; Michelini, F. Enhanced thermopower under a time-dependent gate voltage. Phys. Rev. B 2011, 83, 153417. [Google Scholar] [CrossRef]
- Juergens, S.; Haupt, F.; Moskalets, M.; Splettstoesser, J. Thermoelectric performance of a driven double quantum dot. Phys. Rev. B 2013, 87, 245423. [Google Scholar] [CrossRef]
- Jordan, A.N.; Sothmann, B.; Sánchez, R.; Büttiker, M. Powerful and efficient energy harvester with resonant-tunneling quantum dots. Phys. Rev. B 2013, 87, 075312. [Google Scholar] [CrossRef]
- Zhou, H.; Thingna, J.; Hänggi, P.; Wang, J.S.; Li, B. Boosting thermoelectric efficiency using time-dependent control. Sci. Rep. 2015, 5, 14870. [Google Scholar] [CrossRef] [PubMed]
- Schulenborg, J.; Saptsov, R.B.; Splettstoesser, J.; Wegewijs, M. Unpublished work. 2017.
- Previously, our duality was similarly combined with the independent well-known Iche-duality [48,49,50] based on an electron-hole transformation, yielding new relations for quantum dots in a magnetic field [34].
- Iche, G.; Zawadowski, A. Partial cancellation in the electron-hole and electron-electron correlation in the symmetric anderson model. Solid State Commun. 1972, 10, 1001–1004. [Google Scholar] [CrossRef]
- Taraphder, A.; Coleman, P. Heavy-fermion behavior in a negative-U Anderson model. Phys. Rev. Lett. 1991, 66, 2814–2817. [Google Scholar] [CrossRef] [PubMed]
- Koch, J.; Sela, E.; Oreg, Y.; von Oppen, F. Nonequilibrium charge-Kondo transport through negative-U molecules. Phys. Rev. B 2007, 75, 195402. [Google Scholar] [CrossRef]
- Ashcroft, N.W.; Mermin, N.D. Solid State Physics; Brooks Cole: Boston, MA, USA, 1976. [Google Scholar]
- Schulenborg, J. Time-Dependent Relaxation of Charge and Energy in Electronic Nanosystems. Master’s Thesis, Chalmers University of Technology Göteborg, Göteborg, Sweden, 2016. [Google Scholar]
- Vanherck, J. Time-Dependent Particle and Energy Currents through Interacting Quantum Dots. Master’s Thesis, Applied Quantum Physics Laboratory, Gothenburg, Sweden, 2016. [Google Scholar]
- The rotational symmetry of the full Hamiltonian (2) causes the master equation for the probabilities in the states (8) to completely decouple from the local spin-dynamics, including the coherences.
- Gergs, N.; Schuricht, D. Unpublished work. 2017.
- Beenakker, C.W.J. Theory of Coulomb-blockade oscillations in the conductance of a quantum dot. Phys. Rev. B 1991, 44, 1646. [Google Scholar] [CrossRef]
- Beenakker, C.W.J.; Staring, A.A.M. Theory of the thermopower of a quantum dot. Phys. Rev. B 1992, 46, 9667. [Google Scholar] [CrossRef]
- If one considered more than two leads and obtained 3 linearly-independent vectors already from all |zα), one could formally expand |z) in these vectors. However, this would not be beneficial, since it would still require knowledge of the explicit form of |z), and the weights λα would be functions of all system parameters, including the temperature and chemical potential of all other reservoirs.
- Cutler, M.; Mott, N.F. Observation of Anderson Localization in an Electron Gas. Phys. Rev. 1969, 181, 1336. [Google Scholar] [CrossRef]
- Matveev, K.A. Thermopower in quantum dots. In Statistical and Dynamical Aspects of Mesoscopic Systems; Reguera, D., Platero, G., Bonilla, L.L., Rubi, J.M., Eds.; Springer: Berlin/Heidelberg, Germany, 1999; Chapter 1; pp. 3–15. [Google Scholar]
- The linearization of the particle current (22a) in either x = μα, Tα is proportional to terms of the form (. By Equation (24) the ΔT-derivative of |z) translates to a temperature derivative of the Boltzmann factor which pulls down both and , giving correlators and ).
- Reference [63] also links the thermoelectric conversion efficiency to mixed charge-heat noise, which in essence relates to the time-nonlocal version of the mixed particle-energy correlator appearing here.
- Crépieux, A.; Michelini, F. Heat-charge mixed noise and thermoelectric efficiency fluctuations. J. Stat. Mech. Theory Exp. 2016, 2016, 054015. [Google Scholar] [CrossRef]
- In the vicinity of a single resonance, the linear behavior of the Seebeck coefficient can simply be understood from the exact solution of a single noninteracting level with transmission probability (ω) peaked around ω = ϵ. Then, I = ∫ dω(ω)(fL(ω) − fR(ω)) ≈ ∫ dω(ω)(∂f/∂x)|(x=ϵ−μ)/T [−(ω − μ)ΔT/T2 + V/T], when linearizing with respect to ΔT and V. Approximating the transmission probability to be proportional to a δ function, this yields I ≈ (G/T)[(ϵ − μ)ΔT/T − V]. Getting a vanishing current requires V = SΔT = (ϵ − μ)ΔT/T, such that S is linear in ϵ.
- Leijnse, M.; Wegewijs, M.R.; Hettler, M.H. Pair Tunneling Resonance in the Single-Electron Transport Regime. Phys. Rev. Lett. 2009, 103, 156803. [Google Scholar] [CrossRef] [PubMed]
- Note that, when increasing Γ/T, the slope at the crossover is expected to be modified by renormalization due to higher-order effects [67].
- Kubala, B.; König, J. Quantum-fluctuation effects on the thermopower of a single-electron transistor. Phys. Rev. B 2006, 73, 195316. [Google Scholar] [CrossRef]
- Dzurak, A.S.; Smith, C.G.; Barnes, C.H.W.; Pepper, M.; Martín-Moreno, L.; Liang, C.T.; Ritchie, D.A.; Jones, G.A.C. Thermoelectric signature of the excitation spectrum of a quantum dot. Phys. Rev. B 1997, 55, R10197. [Google Scholar] [CrossRef]
- Staring, A.A.M.; Molenkamp, L.W.; Alphenaar, B.W.; van Houten, H.; Buyk, O.J.A.; Mabesoone, M.A.A.; Beenakker, C.W.J.; Foxon, C.T. Coulomb-Blockade Oscillations in the Thermopower of a Quantum Dot. EPL 2007, 22, 57. [Google Scholar] [CrossRef]
- Svensson, S.F.; Persson, A.I.; Hoffmann, E.A.; Nakpathomkun, N.; Nilsson, H.A.; Xu, H.Q.; Samuelson, L.; Linke, H. Lineshape of the thermopower of quantum dots. New J. Phys. 2012, 14, 033041. [Google Scholar] [CrossRef]
- Contreras-Pulido, L.D.; Splettstoesser, J.; Governale, M.; König, J.; Büttiker, M. Time scales in the dynamics of an interacting quantum dot. Phys. Rev. B 2012, 85, 075301. [Google Scholar] [CrossRef]
- For U = 0 there are no further contribution mechanisms to thermal transport in the absence of charge transport to the leading order in tunnel coupling Γ. Higher orders in the tunnel coupling would, however, allow for processes in which energy is transferred in a multi-particle tunneling process, while no net charge transport occurs.
- López, R.; Lim, J.S.; Sánchez, D. Fluctuation Relations for Spintronics. Phys. Rev. Lett. 2012, 108, 246603. [Google Scholar] [CrossRef] [PubMed]
- Sierra, M.A.; Sánchez, D. Strongly nonlinear thermovoltage and heat dissipation in interacting quantum dots. Phys. Rev. B 2014, 90, 115313. [Google Scholar] [CrossRef]
- Dorda, A.; Ganahl, M.; Andergassen, S.; von der Linden, W.; Arrigoni, E. Thermoelectric response of a correlated impurity in the nonequilibrium Kondo regime. Phys. Rev. B 2016, 94, 245125. [Google Scholar] [CrossRef]
- Sierra, M.A.; López, R.; Sánchez, D. Fate of the spin-12 Kondo effect in the presence of temperature gradients. Phys. Rev. B 2017, 96, 085416. [Google Scholar] [CrossRef]
- Karki, D.B.; Kiselev, M.N. Thermoelectric transport through a SU(N) Kondo impurity. Phys. Rev. B 2017, 96, 121403. [Google Scholar] [CrossRef]
- Otherwise, it would always be possible to find an initial state |ρ0) for which the master equation predicts energy eigenstate projections (i|ρ(t)) < 0 or even Im [(i|ρ(t))] ≠ 0 at some time t, which obviously forbids a probability interpretation.
- For energy-dependent bare couplings Γ(E), this situation would change if Γ(E) had roots on the real axis!







© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schulenborg, J.; Di Marco, A.; Vanherck, J.; Wegewijs, M.R.; Splettstoesser, J. Thermoelectrics of Interacting Nanosystems—Exploiting Superselection Instead of Time-Reversal Symmetry. Entropy 2017, 19, 668. https://doi.org/10.3390/e19120668
Schulenborg J, Di Marco A, Vanherck J, Wegewijs MR, Splettstoesser J. Thermoelectrics of Interacting Nanosystems—Exploiting Superselection Instead of Time-Reversal Symmetry. Entropy. 2017; 19(12):668. https://doi.org/10.3390/e19120668
Chicago/Turabian StyleSchulenborg, Jens, Angelo Di Marco, Joren Vanherck, Maarten R. Wegewijs, and Janine Splettstoesser. 2017. "Thermoelectrics of Interacting Nanosystems—Exploiting Superselection Instead of Time-Reversal Symmetry" Entropy 19, no. 12: 668. https://doi.org/10.3390/e19120668
APA StyleSchulenborg, J., Di Marco, A., Vanherck, J., Wegewijs, M. R., & Splettstoesser, J. (2017). Thermoelectrics of Interacting Nanosystems—Exploiting Superselection Instead of Time-Reversal Symmetry. Entropy, 19(12), 668. https://doi.org/10.3390/e19120668



