Robust Diabatic Grover Search by Landau–Zener–Stückelberg Oscillations
Abstract
:1. Introduction
2. Background
2.1. LZS Hamiltonians
2.2. Grover as a Two-Level System
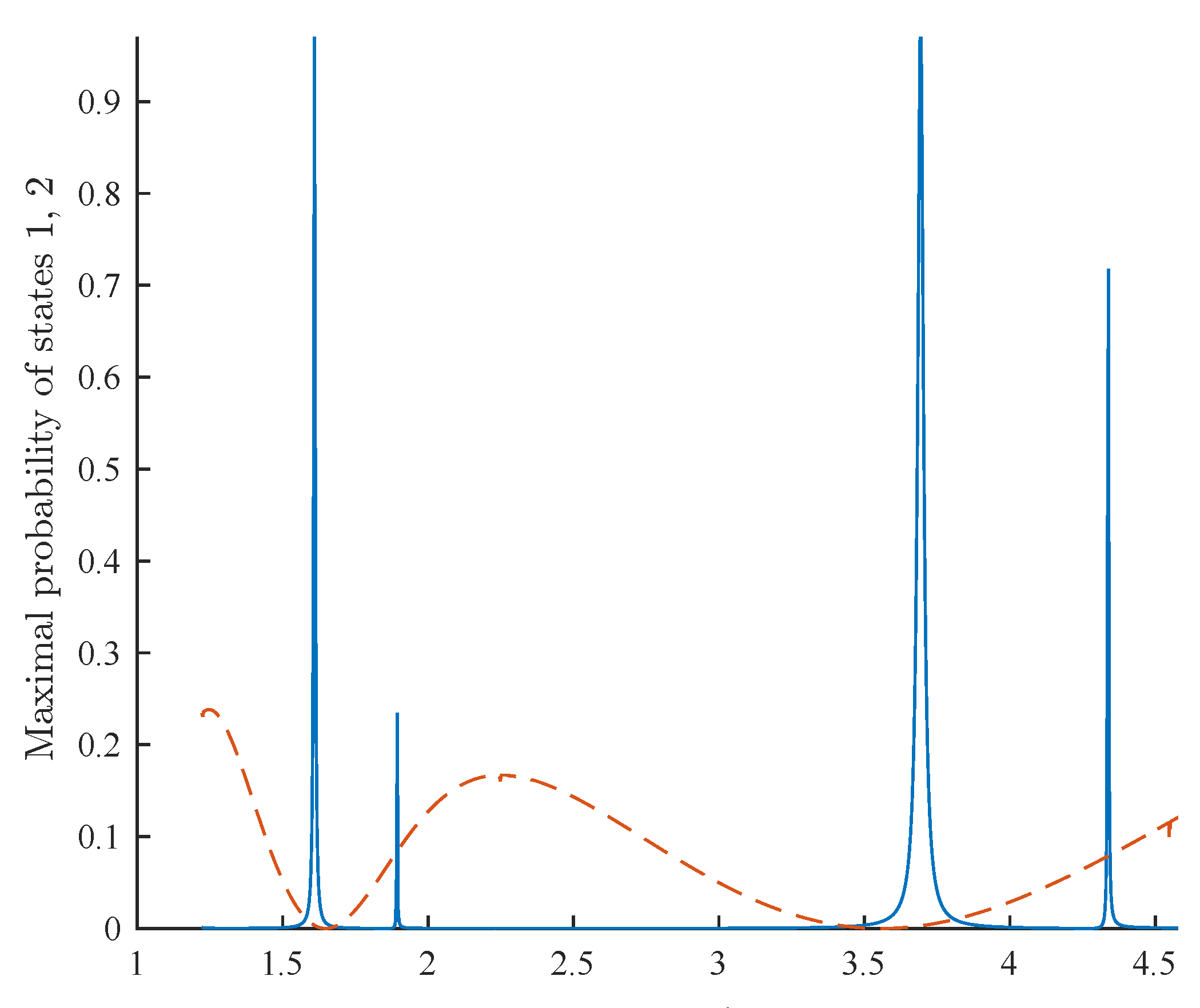
3. Results
3.1. Algorithm
3.2. Algorithm
3.3. Robustness Comparison
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Dimensionless Units
Appendix B. Analysis of LZS Oscillations Using the Rotating Wave Approximation
Appendix C. Invariant Subspace in HG(s)
Appendix D. Proof of Claim 1
Appendix E. Analysis of Algorithm Using the Rotating Wave Approximation
References
- Farhi, E.; Goldstone, J.; Gutmann, S.; Sipser, M. Quantum computation by adiabatic evolution. arXiv 2000, arXiv:quant-ph/0001106. [Google Scholar]
- Albash, T.; Lidar, D.A. Adiabatic quantum computation. Rev. Mod. Phys. 2018, 90, 015002. [Google Scholar] [CrossRef] [Green Version]
- Kato, T. On the adiabatic theorem of quantum mechanics. J. Phys. Soc. Jpn. 1950, 5, 435–439. [Google Scholar] [CrossRef]
- Messiah, A. Quantum Mechanics [Vol 1–2]; North-Holland Publishing Company: Amsterdam, The Netherlands, 1964. [Google Scholar]
- Grover, L.K. A Fast Quantum Mechanical Algorithm for Database Search. In Proceedings of the 28th Annual ACM Symposium on Theory of Computing, Philadelphia, PA, USA, 22–24 May 1996; pp. 212–219. [Google Scholar]
- Bennett, C.H.; Bernstein, E.; Brassard, G.; Vazirani, U. Strengths and Weaknesses of Quantum Computing. SIAM J. Comp. 1997, 26, 1510–1523. [Google Scholar] [CrossRef]
- Somma, R.D.; Nagaj, D.; Kieferová, M. Quantum Speedup by Quantum Annealing. Phys. Rev. Lett. 2012, 109, 050501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hen, I. Period finding with adiabatic quantum computation. EPL (Europhys. Lett.) 2014, 105, 50005. [Google Scholar] [CrossRef] [Green Version]
- Subasi, Y.; Somma, R.D.; Orsucci, D. Quantum algorithms for linear systems of equations inspired by adiabatic quantum computing. arXiv 2018, arXiv:1805.10549. [Google Scholar]
- Atia, Y.; Aharonov, D. Fast-forwarding of Hamiltonians and exponentially precise measurements. Nat. Commun. 2017, 8, 1572. [Google Scholar] [CrossRef] [PubMed]
- Van Dam, W.; Mosca, M.; Vazirani, U. How powerful is adiabatic quantum computation? In Proceedings of the 42nd IEEE Symposium on Foundations of Computer Science, Newport Beach, CA, USA, 8–11 October 2001; pp. 279–287. [Google Scholar]
- Roland, J.; Cerf, N.J. Quantum search by local adiabatic evolution. Phys. Rev. A 2002, 65, 042308. [Google Scholar] [CrossRef] [Green Version]
- Landau, L.D. Zur theorie der energieubertragung. II. Phys. Sov. Union 1932, 2, 1–13. [Google Scholar]
- Zener, C. Non-adiabatic crossing of energy levels. Proc. R. Soc. Lond. A 1932, 137, 696–702. [Google Scholar] [CrossRef]
- Stückelberg, E. Theory of Inelastic Collisions between Atoms. Helv. Phys. Acta 1932, 5, 369–432. [Google Scholar]
- Oliver, W.D.; Valenzuela, S.O. Large-amplitude driving of a superconducting artificial atom. Quantum Inf. Process. 2009, 8, 261–281. [Google Scholar] [CrossRef] [Green Version]
- Shevchenko, S.; Ashhab, S.; Nori, F. Landau–zener–stückelberg interferometry. Phys. Rep. 2010, 492, 1–30. [Google Scholar] [CrossRef]
- Di Giacomo, F.; Nikitin, E.E. The Majorana formula and the Landau–Zener–Stückelberg treatment of the avoided crossing problem. Phys.-Uspekhi 2005, 48, 515. [Google Scholar] [CrossRef]
- Ashhab, S.; Johansson, J.; Zagoskin, A.; Nori, F. Two-level systems driven by large-amplitude fields. Phys. Rev. A 2007, 75, 063414. [Google Scholar] [CrossRef] [Green Version]
- Grossmann, F.; Dittrich, T.; Jung, P.; Hänggi, P. Coherent destruction of tunneling. Phys. Rev. Lett. 1991, 67, 516. [Google Scholar] [CrossRef]
- Villas-Bôas, J.; Ulloa, S.E.; Studart, N. Selective coherent destruction of tunneling in a quantum-dot array. Phys. Rev. B 2004, 70, 041302. [Google Scholar] [CrossRef] [Green Version]
- Lignier, H.; Sias, C.; Ciampini, D.; Singh, Y.; Zenesini, A.; Morsch, O.; Arimondo, E. Dynamical control of matter-wave tunneling in periodic potentials. Phys. Rev. Lett. 2007, 99, 220403. [Google Scholar] [CrossRef]
- Zueco, D.; Galve, F.; Kohler, S.; Hänggi, P. Quantum router based on ac control of qubit chains. Phys. Rev. A 2009, 80, 042303. [Google Scholar] [CrossRef] [Green Version]
- Farhi, E.; Gutmann, S. Analog analogue of a digital quantum computation. Phys. Rev. A 1998, 57, 2403. [Google Scholar] [CrossRef]
- Morley, J.G.; Chancellor, N.; Bose, S.; Kendon, V. Quantum search with hybrid adiabatic–quantum-walk algorithms and realistic noise. Phys. Rev. A 2019, 99, 022339. [Google Scholar] [CrossRef]
- Huang, X.R.; Ding, Z.X.; Hu, C.S.; Shen, L.T.; Li, W.; Wu, H.; Zheng, S.B. Robust Rydberg gate via Landau-Zener control of Förster resonance. Phys. Rev. A 2018, 98, 052324. [Google Scholar] [CrossRef]
- Meyer, D.A. From quantum cellular automata to quantum lattice gases. J. Stat. Phys. 1996, 85, 551–574. [Google Scholar] [CrossRef] [Green Version]
- Farhi, E.; Gutmann, S. Quantum computation and decision trees. Phys. Rev. A 1998, 58, 915. [Google Scholar] [CrossRef]
- Shenvi, N.; Kempe, J.; Whaley, K.B. Quantum random-walk search algorithm. Phys. Rev. A 2003, 67, 052307. [Google Scholar] [CrossRef] [Green Version]
- Childs, A.M.; Goldstone, J. Spatial search by quantum walk. Phys. Rev. A 2004, 70, 022314. [Google Scholar] [CrossRef]
- Hein, B.; Tanner, G. Quantum search algorithms on the hypercube. J. Phys. A: Math. Theor. 2009, 42, 085303. [Google Scholar] [CrossRef]
- Wong, T.G.; Meyer, D.A. Irreconcilable difference between quantum walks and adiabatic quantum computing. Phys. Rev. A 2016, 93, 062313. [Google Scholar] [CrossRef] [Green Version]
- Chakraborty, S.; Novo, L.; Di Giorgio, S.; Omar, Y. Optimal quantum spatial search on random temporal networks. Phys. Rev. Lett. 2017, 119, 220503. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Bernstein, E.; Vazirani, U. Quantum Complexity Theory†. SIAM J. Comp. 1997, 26, 1411–1473. [Google Scholar] [CrossRef]
- Roland, J.; Cerf, N.J. Noise resistance of adiabatic quantum computation using random matrix theory. Phys. Rev. A 2005, 71, 032330. [Google Scholar] [CrossRef] [Green Version]
- Ashhab, S.; Johansson, J.; Nori, F. Decoherence in a scalable adiabatic quantum computer. Phys. Rev. A 2006, 74, 052330. [Google Scholar] [CrossRef] [Green Version]
- Shirley, J.H. Solution of the Schrödinger equation with a Hamiltonian periodic in time. Phys. Rev. 1965, 138, B979. [Google Scholar] [CrossRef]
- Young, K.C.; Sarovar, M.; Blume-Kohout, R. Error suppression and error correction in adiabatic quantum computation: Techniques and challenges. Phys. Rev. X 2013, 3, 041013. [Google Scholar] [CrossRef]
- Gottesman, D. Stabilizer codes and quantum error correction. arXiv 1997, arXiv:quant-ph/9705052. [Google Scholar]
- Lidar, D.A. Towards fault tolerant adiabatic quantum computation. Phys. Rev. Lett. 2008, 100, 160506. [Google Scholar] [CrossRef]
- Jordan, S.P.; Farhi, E.; Shor, P.W. Error-correcting codes for adiabatic quantum computation. Phys. Rev. A 2006, 74, 052322. [Google Scholar] [CrossRef] [Green Version]
- Paz-Silva, G.A.; Rezakhani, A.; Dominy, J.M.; Lidar, D. Zeno effect for quantum computation and control. Phys. Rev. Lett. 2012, 108, 080501. [Google Scholar] [CrossRef]
- Facchi, P.; Lidar, D.; Pascazio, S. Unification of dynamical decoupling and the quantum Zeno effect. Phys. Rev. A 2004, 69, 032314. [Google Scholar] [CrossRef] [Green Version]
- Preskill, J. Quantum Computing in the NISQ era and beyond. arXiv 2018, arXiv:1801.00862. [Google Scholar] [CrossRef]
- Muthukrishnan, S.; Albash, T.; Lidar, D.A. Tunneling and speedup in quantum optimization for permutation-symmetric problems. Phys. Rev. X 2016, 6, 031010. [Google Scholar] [CrossRef]
- Brady, L.; van Dam, W. Evolution-Time Dependence in Near-Adiabatic Quantum Evolutions. arXiv 2018, arXiv:1801.04349. [Google Scholar]
- Peruzzo, A.; McClean, J.; Shadbolt, P.; Yung, M.H.; Zhou, X.Q.; Love, P.J.; Aspuru-Guzik, A.; O’brien, J.L. A variational eigenvalue solver on a photonic quantum processor. Nat. Commun. 2014, 5, 4213. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wecker, D.; Hastings, M.B.; Troyer, M. Progress towards practical quantum variational algorithms. Phys. Rev. A 2015, 92, 042303. [Google Scholar] [CrossRef]
- McClean, J.R.; Romero, J.; Babbush, R.; Aspuru-Guzik, A. The theory of variational hybrid quantum-classical algorithms. New J. Phys. 2016, 18, 023023. [Google Scholar] [CrossRef]
- Yang, Z.C.; Rahmani, A.; Shabani, A.; Neven, H.; Chamon, C. Optimizing variational quantum algorithms using pontryagin’s minimum principle. Phys. Rev. X 2017, 7, 021027. [Google Scholar] [CrossRef]
- Farhi, E.; Goldstone, J.; Gutmann, S. A quantum approximate optimization algorithm. arXiv 2014, arXiv:1411.4028. [Google Scholar]
- Farhi, E.; Goldstone, J.; Gutmann, S. A Quantum Approximate Optimization Algorithm Applied to a Bounded Occurrence Constraint Problem. arXiv 2014, arXiv:1412.6062. [Google Scholar]
- Dodds, B.; Kendon, V.; Adams, C.S.; Chancellor, N. Practical designs for permutation symmetric problem Hamiltonians on hypercubes. arXiv 2018, arXiv:1812.07885. [Google Scholar] [CrossRef]
- Pegg, D.; Series, G.W. On the reduction of a problem in magnetic resonance. Proc. R. Soc. Lond. A 1973, 332, 281–289. [Google Scholar] [CrossRef]
- Joachain, C.J. Quantum Collision Theory; Elsevier: Amsterdam, The Netherlands, 1975. [Google Scholar]
- Ono, K.; Shevchenko, S.; Mori, T.; Moriyama, S.; Nori, F. Quantum interferometry with a high-temperature single-spin qubit. arXiv 2018, arXiv:1809.02326. [Google Scholar]




© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Atia, Y.; Oren, Y.; Katz, N. Robust Diabatic Grover Search by Landau–Zener–Stückelberg Oscillations. Entropy 2019, 21, 937. https://doi.org/10.3390/e21100937
Atia Y, Oren Y, Katz N. Robust Diabatic Grover Search by Landau–Zener–Stückelberg Oscillations. Entropy. 2019; 21(10):937. https://doi.org/10.3390/e21100937
Chicago/Turabian StyleAtia, Yosi, Yonathan Oren, and Nadav Katz. 2019. "Robust Diabatic Grover Search by Landau–Zener–Stückelberg Oscillations" Entropy 21, no. 10: 937. https://doi.org/10.3390/e21100937
APA StyleAtia, Y., Oren, Y., & Katz, N. (2019). Robust Diabatic Grover Search by Landau–Zener–Stückelberg Oscillations. Entropy, 21(10), 937. https://doi.org/10.3390/e21100937



