About Universality and Thermodynamics of Turbulence
Abstract
1. Introduction
2. Experimental and Numerical Setup
2.1. Experimental Facilities and Parameters
2.2. Direct Numerical Simulation
3. Theoretical Background
3.1. Velocity Increments vs. Wavelet Transform (WT) of Velocity Gradients
3.2. K41 and K62 Universality
3.3. Multifractal and Fluctuating Dissipation Length
3.4. General Universality
4. Check of Universality Using Data Analysis
4.1. Check of K41 Universality
4.2. Check of K62 Universality
4.3. Check of General Universality
4.4. Function
4.5. Scaling Exponents
4.6. Multifractal Spectrum
5. Thermodynamics and Turbulence
5.1. Thermodynamical Analogy
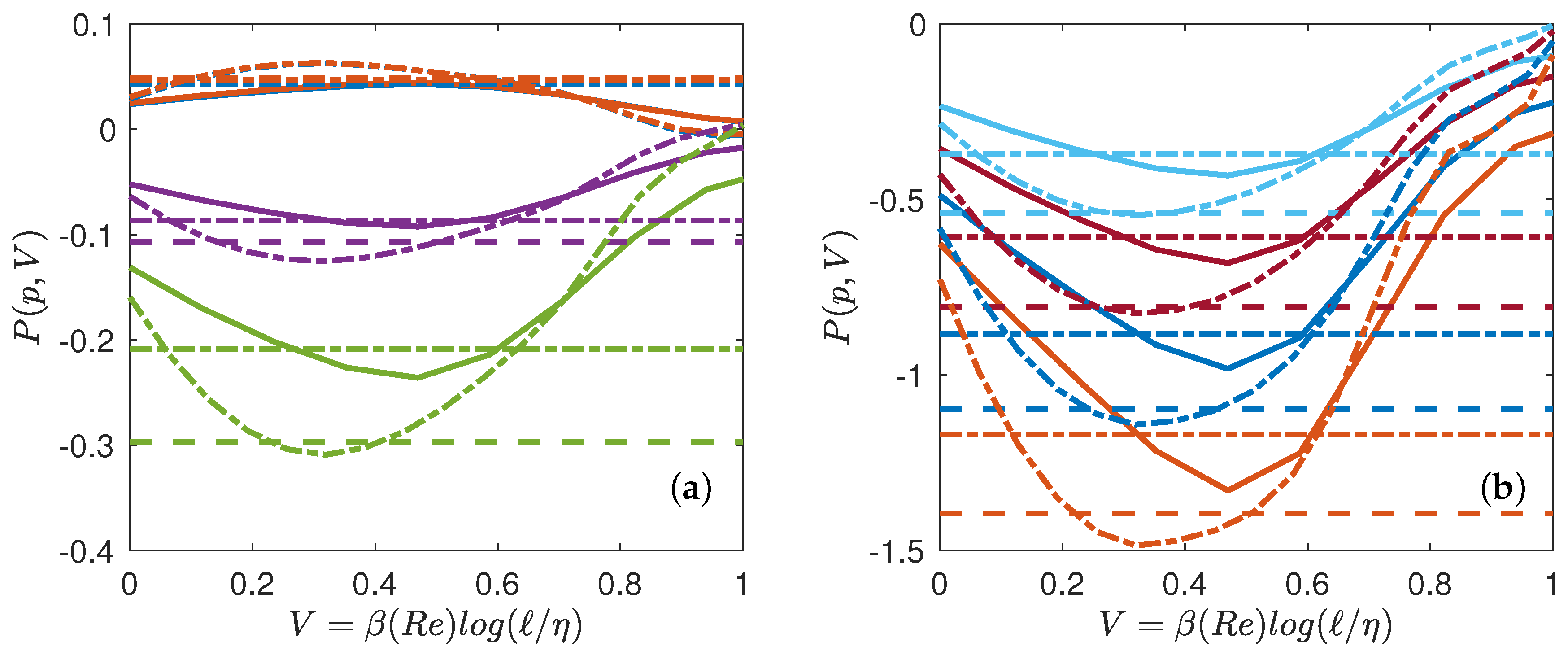
5.2. Multifractal Pressure and Phase Transition
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Dubrulle, B. Beyond Kolmogorov cascades. J. Fluid Mech. Perspect. 2019. [Google Scholar] [CrossRef]
- Castaing, B. Conséquences d’un principe d’extremum en turbulence. J. Phys. Fr. 1989, 50, 147–156. [Google Scholar] [CrossRef]
- Frisch, U.; Parisi, G. On the singularity structure of fully developed turbulence. In Turbulence and Predictability in Geophysical Fluid Dynamics and ClimateDynamics; Gil, M., Benzi, R., Parisi, G., Eds.; Elsevier: Amsterdam, The Netherlands, 1985; pp. 84–88. [Google Scholar]
- Eyink, G.L. Turbulence Theory. Course Notes, The Johns Hopkins University. 2007–2008. Available online: http://www.ams.jhu.edu/~eyink/Turbulence/notes/ (accessed on 24 September 2018).
- Paladin, G.; Vulpiani, A. Anomalous scaling laws in multifractal objects. Phys. Rev. 1987, 156, 147–225. [Google Scholar] [CrossRef]
- Frisch, U.; Vergassola, M. A Prediction of the Multifractal Model: The Intermediate Dissipation Range. Europhys. Lett. (EPL) 1991, 14, 439–444. [Google Scholar] [CrossRef]
- Castaing, B.; Gagne, Y.; Marchand, M. Log-similarity for turbulent flows? Phys. D Nonlinear Phenom. 1993, 68, 387–400. [Google Scholar] [CrossRef]
- Muzy, J.F.; Bacry, E.; Arneodo, A. Wavelets and multifractal formalism for singular signals: Application to turbulence data. Phys. Rev. Lett. 1991, 67, 3515. [Google Scholar] [CrossRef] [PubMed]
- Saw, E.W.; Debue, P.; Kuzzay, D.; Daviaud, F.; Dubrulle, B. On the universality of anomalous scaling exponents of structure functions in turbulent flows. J. Fluid Mech. 2018, 837, 657–669. [Google Scholar] [CrossRef]
- Debue, P.; Shukla, V.; Kuzzay, D.; Faranda, D.; Saw, E.W.; Daviaud, F.; Dubrulle, B. Dissipation, intermittency, and singularities in incompressible turbulent flows. Phys. Rev. E 2018, 97, 053101. [Google Scholar] [CrossRef] [PubMed]
- Kolmogorov, A.N. The local structure of turbulence in incompressible viscous fluids for very large Reynolds number. Dokl. Akad. Nauk SSSR [Sov. Phys.-Dokl.] 1941, 30, 913. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. A refinement of previous hypotheses concerning the local structure of turbulence in a viscous incompressible fluid at high Reynolds number. J. Fluid Mech. 1962, 13, 82. [Google Scholar] [CrossRef]
- Touchette, H. The large deviation approach to statistical mechanics. Phys. Rep. 2009, 478, 1–69. [Google Scholar] [CrossRef]
- Frisch, U. Turbulence: The Legacy of A.N. Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Benzi, R.; Ciliberto, S.; Tripiccione, R.; Baudet, C.; Massaioli, F.; Succi, S. Extended self-similarity in turbulent flows. Phys. Rev. E 1993, 48, R29–R32. [Google Scholar] [CrossRef]
- Bohr, T.; Rand, D. The entropy function for characteristic exponents. Phys. D Nonlinear Phenom. 1987, 25, 387–398. [Google Scholar] [CrossRef]
- Rinaldo, A.; Maritan, A.; Colaiori, F.; Flammini, A.; Rigon, R.; Rodriguez-Iturbe, I.; Banavar, J.R. Thermodynamics of fractal networks. Phys. Rev. Lett. 1996, 76, 3364. [Google Scholar] [CrossRef] [PubMed]
- Arneodo, A.; Benzi, R.; Berg, J.; Biferale, L.; Bodenschatz, E.; Busse, A.; Calzavarini, E.; Castaing, B.; Cencini, M.; Chevillard, L.; et al. Universal Intermittent Properties of Particle Trajectories in Highly Turbulent Flows. Phys. Rev. Lett. 2008, 100, 254504. [Google Scholar] [CrossRef] [PubMed]
- Biferale, L.; Boffetta, G.; Celani, A.; Devenish, B.J.; Lanotte, A.; Toschi, F. Multifractal Statistics of Lagrangian Velocity and Acceleration in Turbulence. Phys. Rev. Lett. 2004, 93, 064502. [Google Scholar] [CrossRef] [PubMed]







| Symbol | Mathematical Definition | Interpretation |
|---|---|---|
| Velocity field | ||
| k | Wavenumber | |
| FT | Energy spectrum | |
| Forcing wavenumber | ||
| Grid size in direction x | ||
| Kinematic viscosity | ||
| Mean dissipation power per unit mass | ||
| Kolmogorov scale | ||
| Kolmogorov velocity | ||
| Characteristic velocity | ||
| Characteristic length | ||
| Re | Reynolds number | |
| Taylor length | ||
| Root mean squared velocity | ||
| Taylor Reynolds number | ||
| SPIV spatial resolution | ||
| p | Power | |
| ℓ | Scale | |
| L | Inertial large scale | |
| Velocity increment at scale ℓ | ||
| Wavelet filter | ||
| Wavelet filter at scale ℓ | ||
| Wavelet transform of | ||
| Wavelet velocity increment | ||
| Velocity structure function | ||
| Relative structure function | ||
| Local Hölder exponent | ||
| Multifractal Spectrum | ||
| Multifractal regularization scale | ||
| Intermittency parameter | ||
| Lognormal Intermittency correction | ||
| Scaling exponent | ||
| Rescaled length | ||
| General intermittency correction | ||
| General intermittency correction | ||
| , | Fitting functions | |
| G | General function from Castaing [2] | |
| , | Universal parameters | |
| H | New general function | |
| Universal parameter | ||
| a, b | Parabolic fit | |
| Parameter | ||
| for ℓ in Inertial range | Intermittency correction from general rescaling | |
| Spatial scale dependent measure | ||
| Large deviation function of | ||
| Boltzmann constant | ||
| T | Temperature | |
| E | Energy | |
| N | Number of degrees of freedom | |
| V | Volume | |
| P | Pressure | |
| F | Free energy |
| Case | Frequency (Hz) | Glycerol Part | Re | (mm) | Frames | Symbol | ||
|---|---|---|---|---|---|---|---|---|
| A | 5 | 0% | 0.02 | 2.4 | ○ | |||
| B | 5 | 0% | 0.02 | 0.48 | □ | |||
| C | 5 | 0% | 0.02 | 0.24 | ◊ | |||
| D | 1 | 0% | 0.08 | 0.48 | △ | |||
| E | 1.2 | 59% | 0.37 | 0.24 | ⋆ |
| Samples | Symbol | |||||
|---|---|---|---|---|---|---|
| 25 | 0.079 | 3.35 | 0.635 | 5000 | ⋆ | |
| 53 | 0.034 | 8.5 | 0.31 | 105,000 | △ | |
| 80 | 0.020 | 1.68 | 1.22 | 270,000 | □ | |
| 90 | 0.017 | 5.7 | 0.36 | 10,000 | ◊ | |
| 138 | 0.009 | 1.55 | 1.37 | 12,000 | ○ |
| Exponent\Order | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 0.36 | 0.69 | 1 | 1.29 | 1.55 | 1.78 | 1.98 | 2.17 | 2.33 | |
| 0.31 | 0.58 | 0.80 | 0.98 | 1.12 | 1.23 | 1.26 | 1.25 | 1.23 | |
| 0.32 | 0.58 | 0.80 | 0.98 | 1.12 | 1.23 | 1.32 | 1.39 | 1.44 | |
| 0.04 | 0.05 | 0 | |||||||
| 0.05 | 0.05 | 0 |
| Thermodynamics | Turbulence | |
|---|---|---|
| Temperature | ||
| Energy | E | |
| Number of d.f. | N | |
| Volume | V | |
| Pressure | P | |
| Free energy | F |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Geneste, D.; Faller, H.; Nguyen, F.; Shukla, V.; Laval, J.-P.; Daviaud, F.; Saw, E.-W.; Dubrulle, B. About Universality and Thermodynamics of Turbulence. Entropy 2019, 21, 326. https://doi.org/10.3390/e21030326
Geneste D, Faller H, Nguyen F, Shukla V, Laval J-P, Daviaud F, Saw E-W, Dubrulle B. About Universality and Thermodynamics of Turbulence. Entropy. 2019; 21(3):326. https://doi.org/10.3390/e21030326
Chicago/Turabian StyleGeneste, Damien, Hugues Faller, Florian Nguyen, Vishwanath Shukla, Jean-Philippe Laval, Francois Daviaud, Ewe-Wei Saw, and Bérengère Dubrulle. 2019. "About Universality and Thermodynamics of Turbulence" Entropy 21, no. 3: 326. https://doi.org/10.3390/e21030326
APA StyleGeneste, D., Faller, H., Nguyen, F., Shukla, V., Laval, J.-P., Daviaud, F., Saw, E.-W., & Dubrulle, B. (2019). About Universality and Thermodynamics of Turbulence. Entropy, 21(3), 326. https://doi.org/10.3390/e21030326





