A New Logit-Based Gini Coefficient
Abstract
:1. Introduction
2. Motivation for Our Analysis
2.1. Derivation of the Gini Coefficient with a Linear Projection Function
2.2. Derivation of a Share Function from the Given Gini Coefficient
3. Derivation of the LBGC and the Corresponding IDF
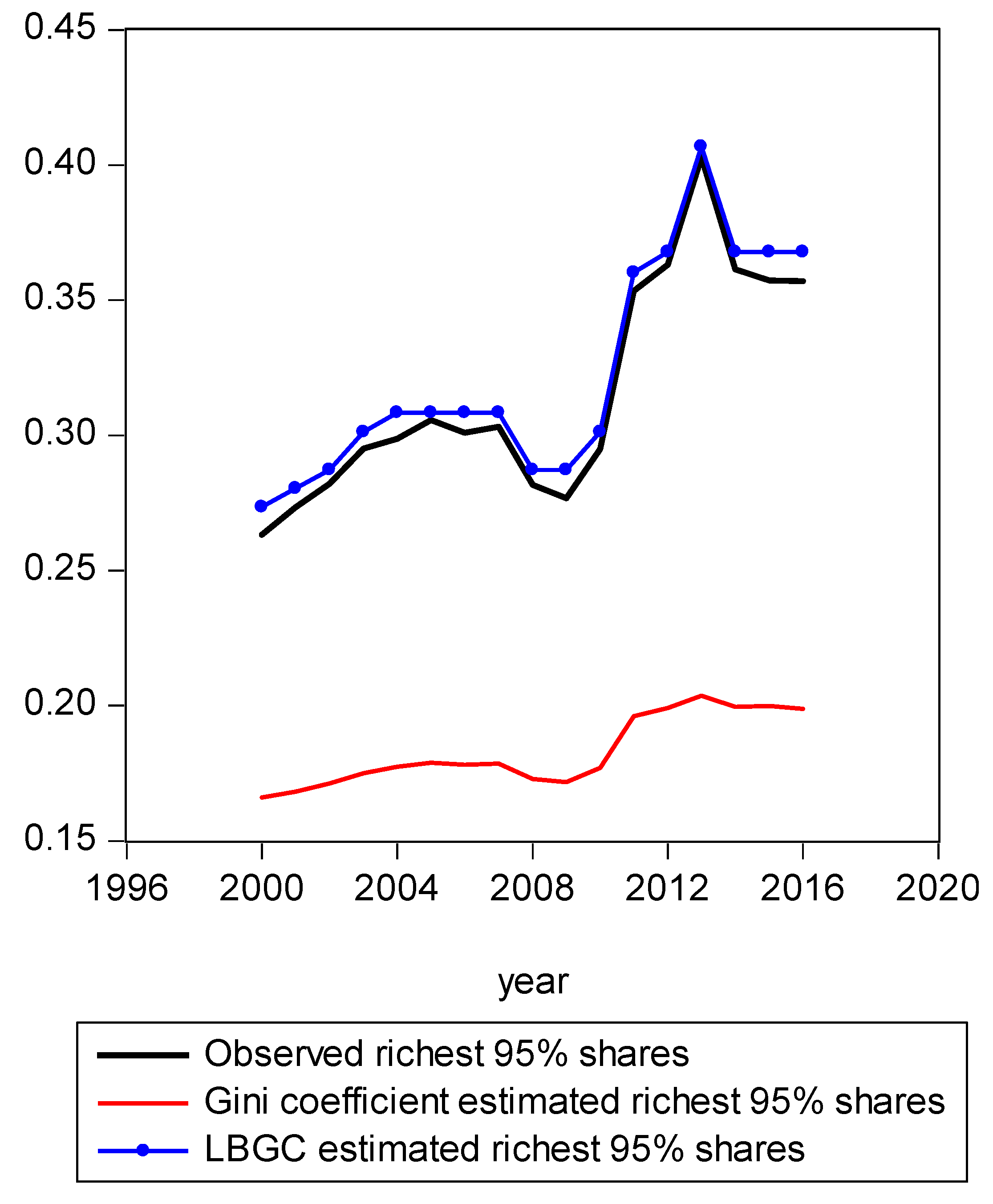
4. Applications
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Desirable Properties of an Income Inequality Index
Appendix B. Various Weighting Functions for Inequality Measures
Appendix B.1. Derivation of an Inequality Measure and Corresponding Share Function from the Given Second Moment
Appendix B.2. Derivation of an Inequality Measure and Corresponding Share Function from the Given Third Moment
Appendix B.3. Derivation of an Inequality Measure and Corresponding Share Function from the Given Square Root Moment
Appendix B.4. Derivation of an Inequality Measure and Corresponding share Function from the Given Exponent MOMENT
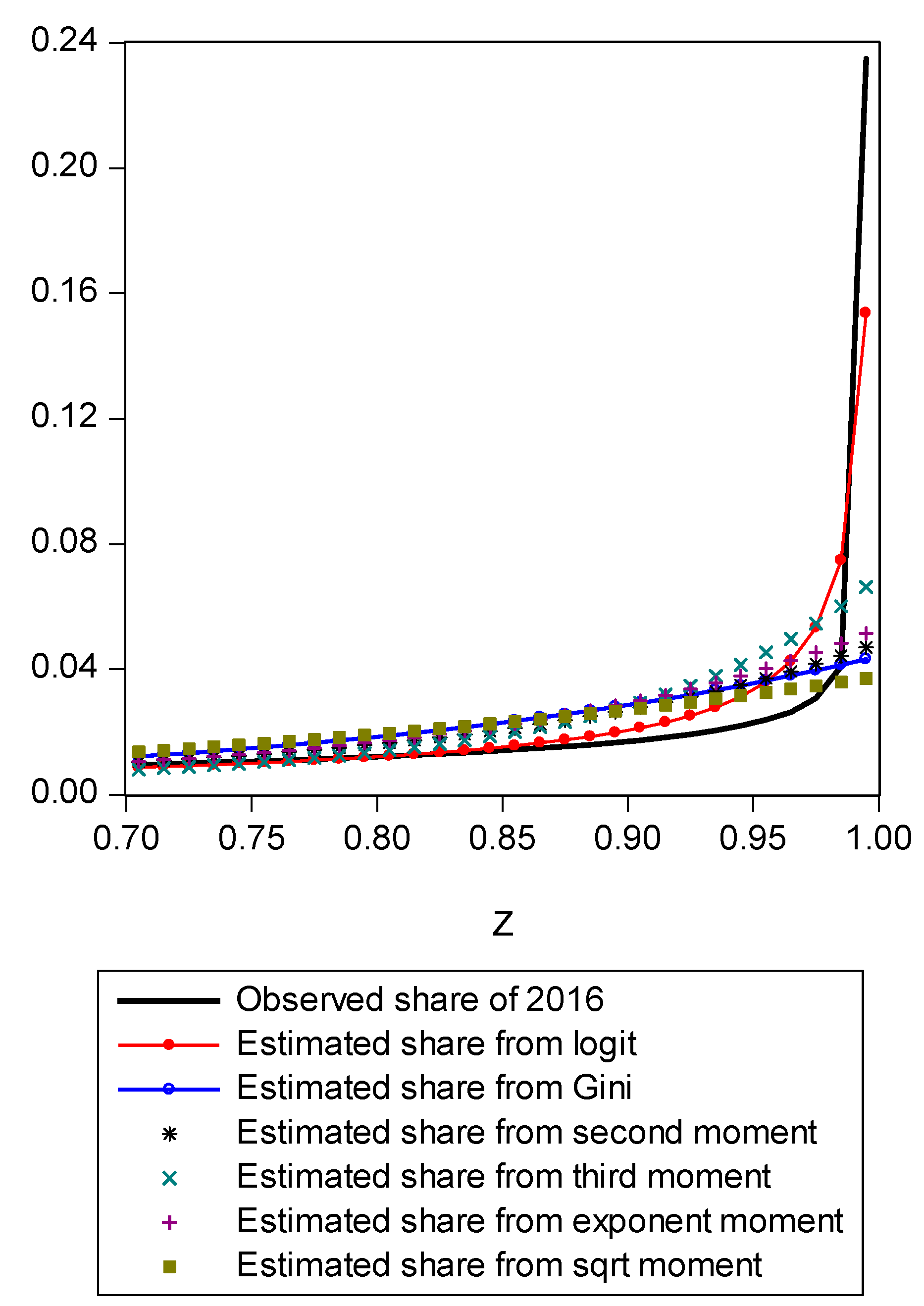
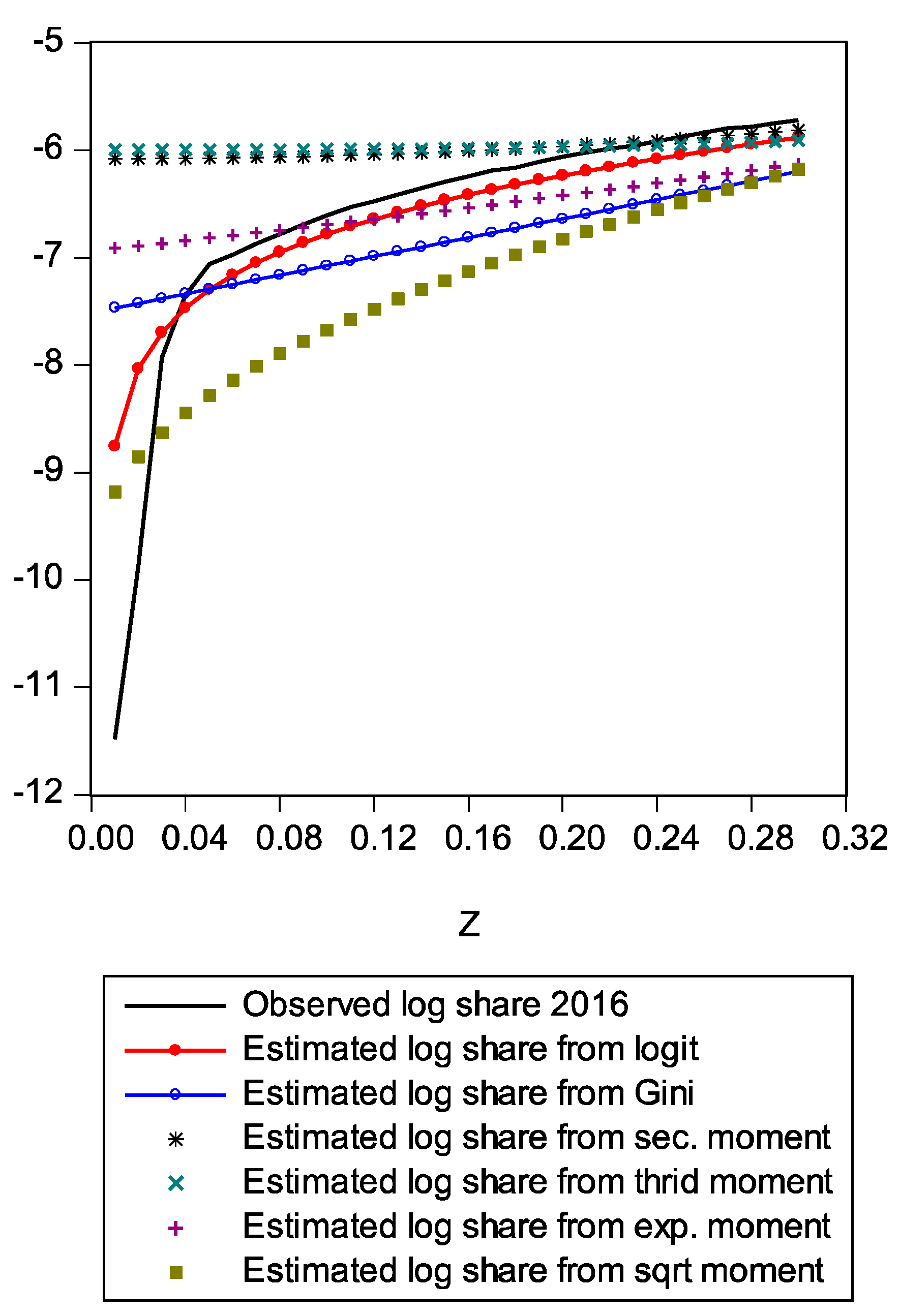


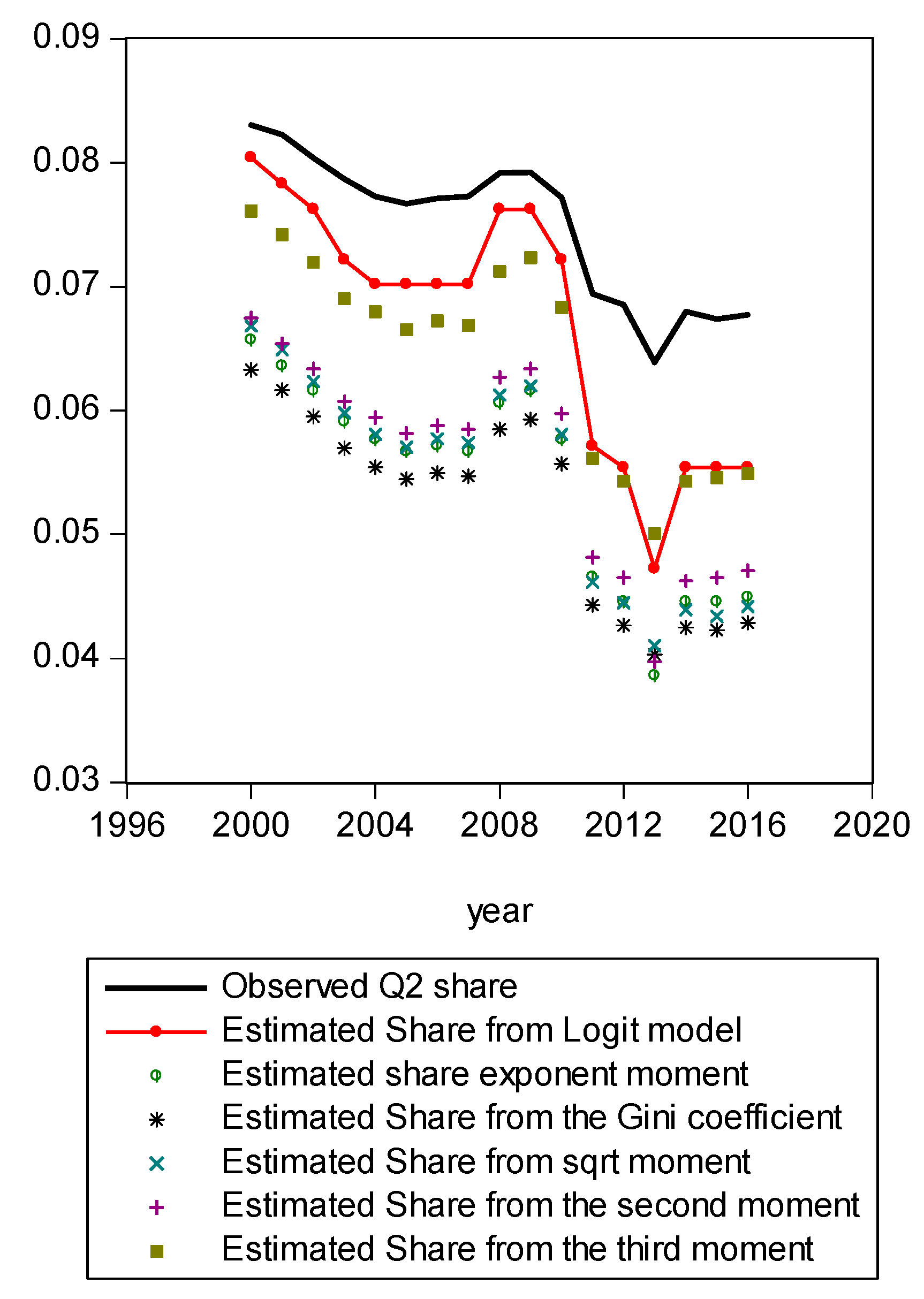
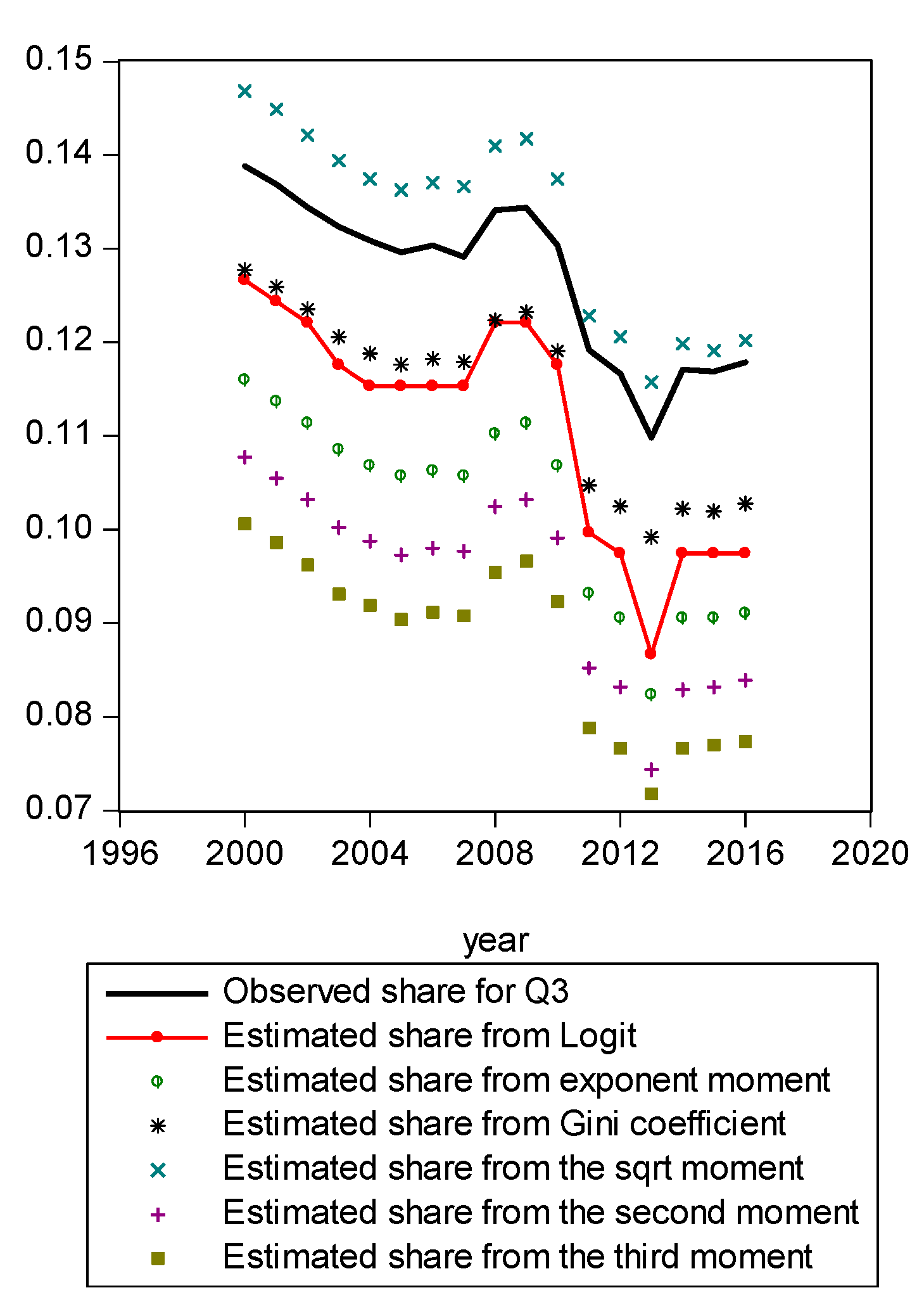
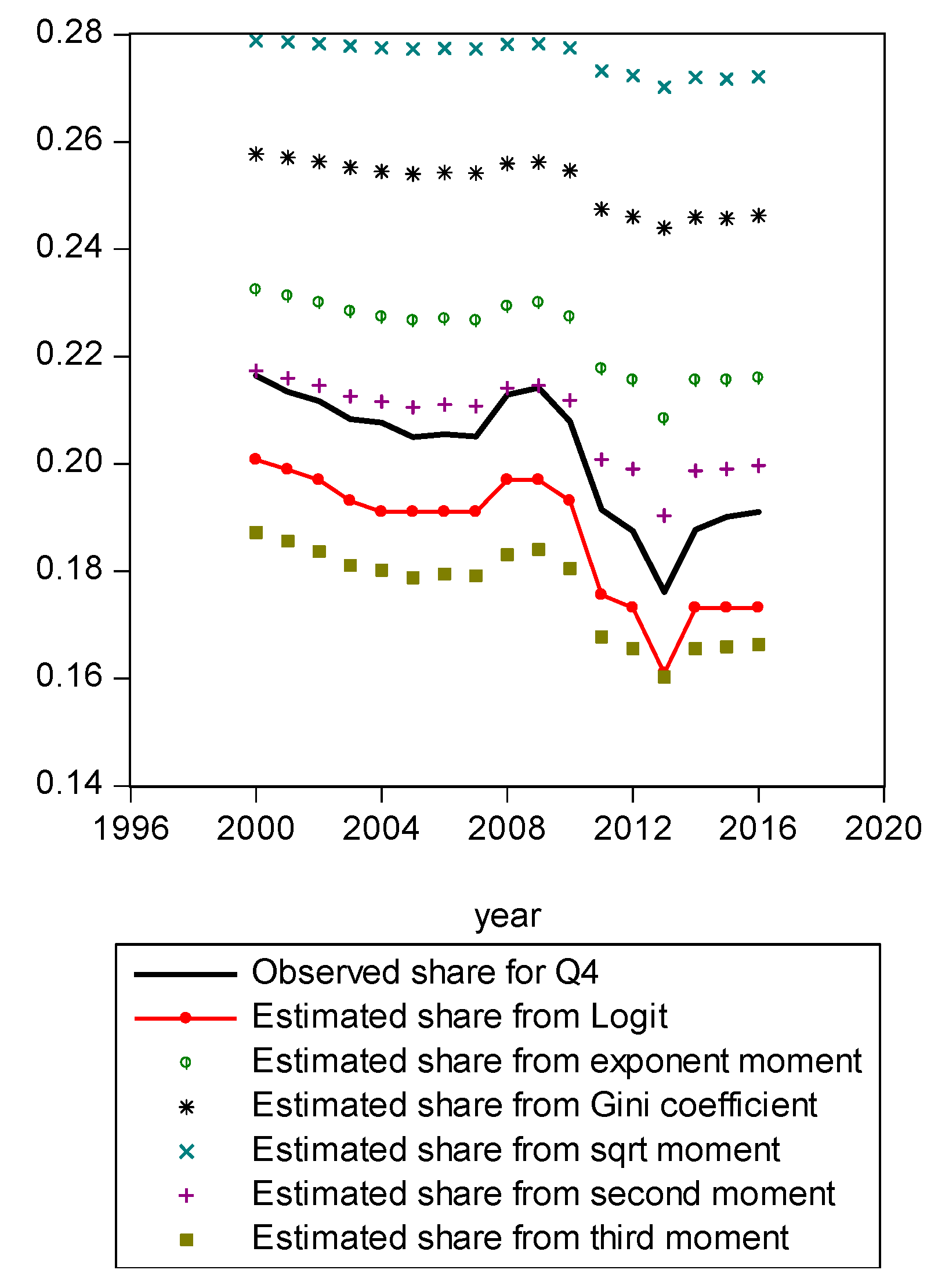
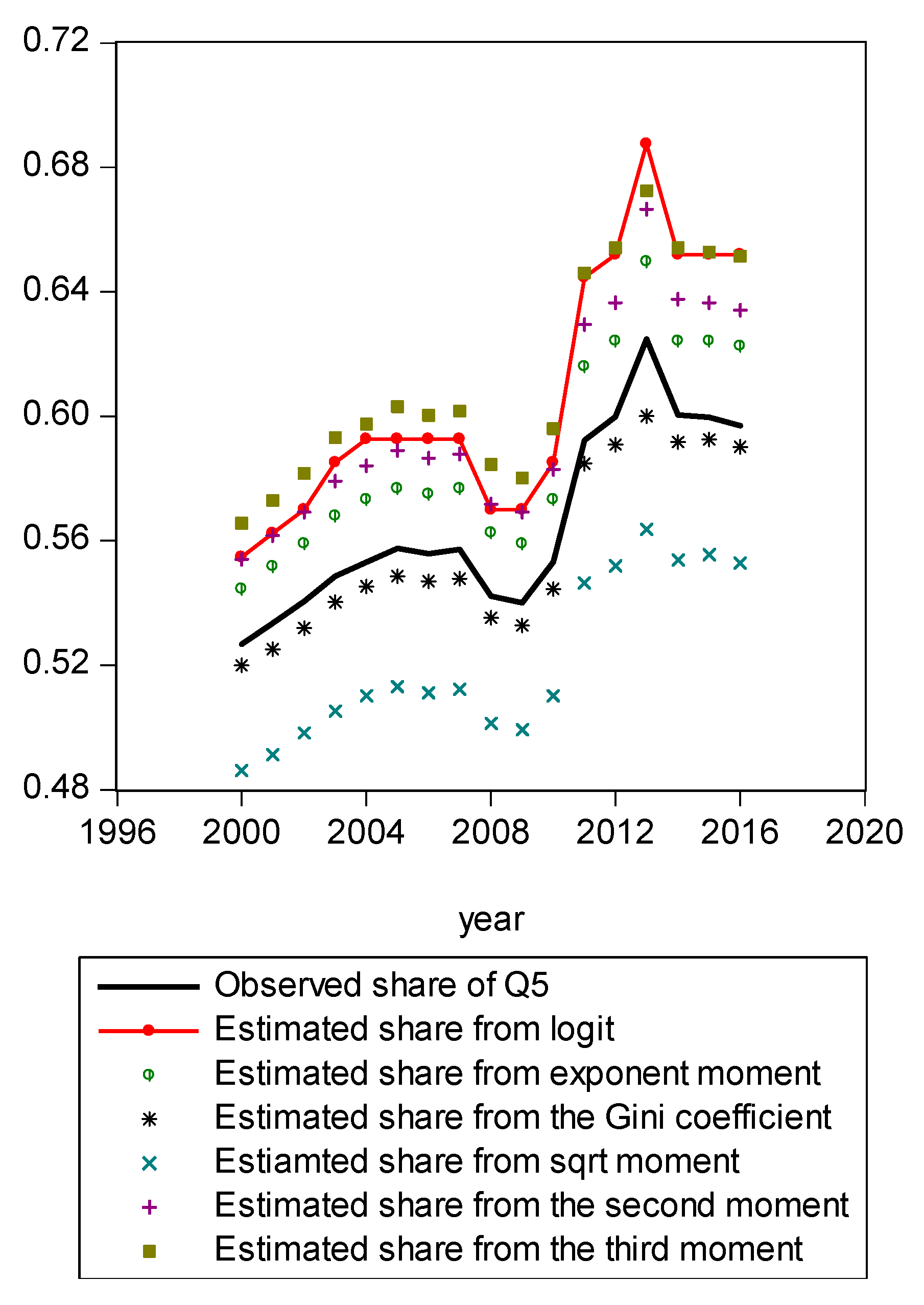
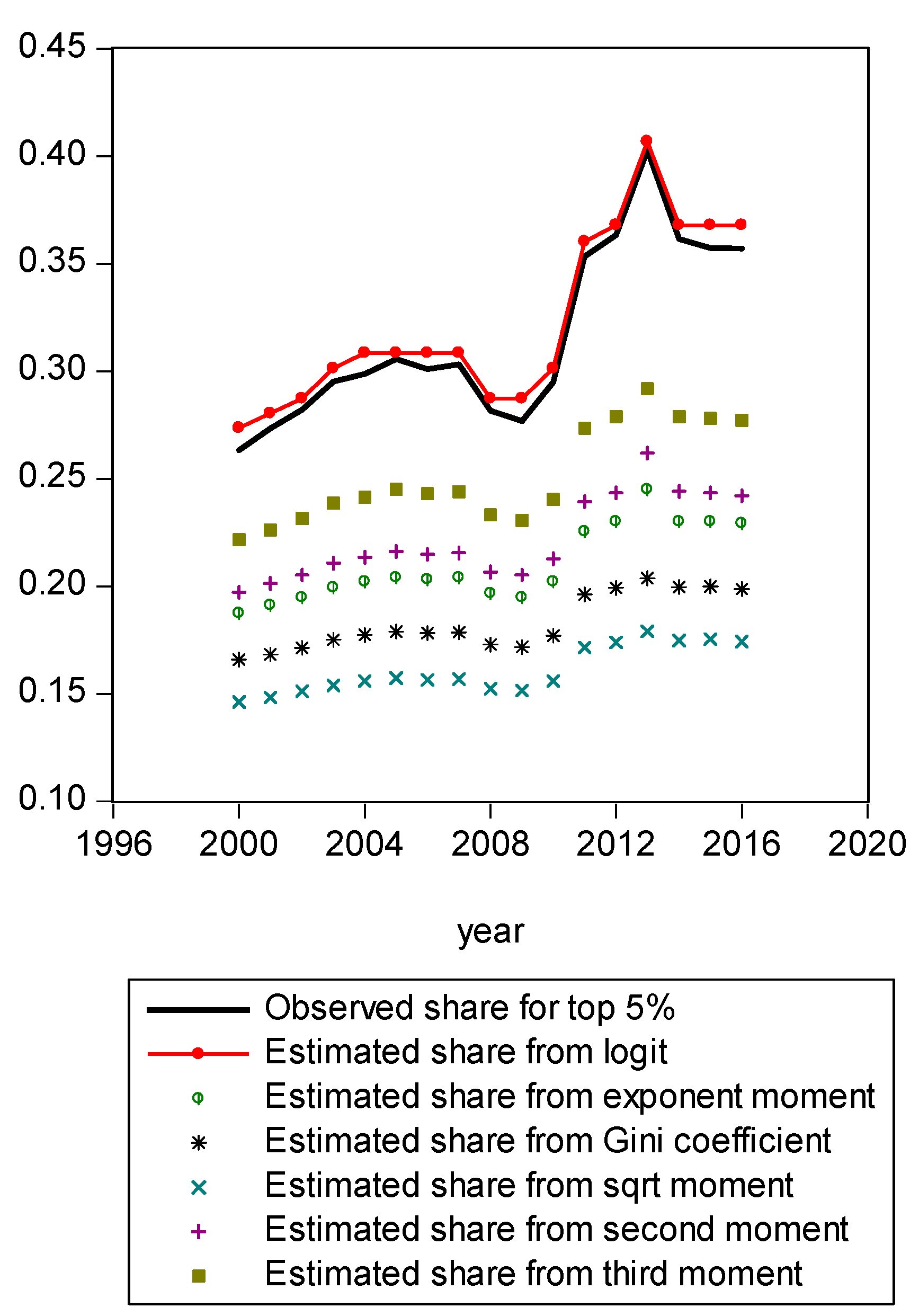

References
- Cowell, F. Measuring Inequality, 3rd ed.; Oxford University Press: Oxford, UK, 2011. [Google Scholar]
- Ryu, H. Maximum Entropy Estimation of Density and Regression Functions. J. Econ. 1993, 56, 397–440. [Google Scholar] [CrossRef]
- Tanak, A.K.; Borzadaran, G.R.M.; Ahmadi, J. New Functional Forms of Lorenz Curves by Maximizing Tsallis Entropy of Income Share Function under the Constraint on Generalized Index. Physica A 2018, 511, 280–288. [Google Scholar] [CrossRef]
- Rad, N.; Borzadaran, G.R.M.; Yari, G.H. Maximum Entropy Estimation of Income Share Function from Generalized Gini Index. J. Appl. Stat. 2016, 43, 2910–2921. [Google Scholar]
- Arnold, B.; Sarabia, J.M. Majorization and the Lorenz Order with Applications in Applied Mathematics and Economics; Springer: New York, NY, USA, 2018. [Google Scholar]
- Arnold, B.; Castillo, E.; Sarabia, J.M. Conditional Specification of Statistical Models; Springer: New York, NY, USA, 1999. [Google Scholar]
- Giorgi, G.M. A Fresh Look at the Topical Interest of the Gini Concentration Ratio. Econometrics 1993, 51, 83–98. [Google Scholar]
- Giorgi, G.M.; Gubbiotti, S. Celebrating the memory of Corrado Gini: A personality out of the ordinary. Int. Stat. Rev. 2017, 85, 325–339. [Google Scholar] [CrossRef]
- Nygard, F.; Sandstrom, A. Measuring Inequality; Almqvist and Wiksell: Stockholm, Sweden, 1981. [Google Scholar]
- Nygard, F.; Sandstrom, A. Income Inequality Measures based on Sample Surveys. J. Econom. 1989, 42, 81–96. [Google Scholar] [CrossRef]
- Lerman, R.; Yitzhaki, S. A Note on the Calculation and Interpretation of the Gini Index. Econom. Lett. 1984, 15, 363–368. [Google Scholar] [CrossRef]
- Yitzhaki, S. More than a dozen ways of spelling Gini. Res. Income Inequal. 1998, 8, 13–33. [Google Scholar]
- Gastwirth, J. Is the Gini Index Overly Sensitive to changes in the Middle of the Income distribution? Stat. Public Policy 2017, 4, 1–11. [Google Scholar] [CrossRef]
- Arfken, G. Mathematical Methods for Physicists, 3rd ed.; Academic Press, Inc.: San Diego, CA, USA, 1985. [Google Scholar]
- Milne, W. Numerical Calculations; Princeton University Press: Princeton, NJ, USA, 1973. [Google Scholar]
- Ryu, H.; Slottje, D. Income Inequality vs Utility Inequality. Commun. Stat. 2017, 46, 3631–3640. [Google Scholar]
- Ryu, H.; Slottje, D. Maximum Entropy Estimation of Income Distributions from Basmann’s WGM Class. J. Econom. 2017, 199, 221–231. [Google Scholar] [CrossRef]
- Shannon, C. The Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423, reprinted in Shannon, C.E.; Weaver, W.; Shannon, C.E.; Weaver, W. The Mathematical Theory of Communication; University of Illinois Press: Urbana, IL, USA, 1949; pp. 3–91. [Google Scholar] [CrossRef]
- Sen, A. On Economic Inequality, 3rd ed.; Oxford University Press: Oxford, UK, 2003. [Google Scholar]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryu, H.K.; Slottje, D.J.; Kwon, H.Y. A New Logit-Based Gini Coefficient. Entropy 2019, 21, 488. https://doi.org/10.3390/e21050488
Ryu HK, Slottje DJ, Kwon HY. A New Logit-Based Gini Coefficient. Entropy. 2019; 21(5):488. https://doi.org/10.3390/e21050488
Chicago/Turabian StyleRyu, Hang K., Daniel J. Slottje, and Hyeok Y. Kwon. 2019. "A New Logit-Based Gini Coefficient" Entropy 21, no. 5: 488. https://doi.org/10.3390/e21050488
APA StyleRyu, H. K., Slottje, D. J., & Kwon, H. Y. (2019). A New Logit-Based Gini Coefficient. Entropy, 21(5), 488. https://doi.org/10.3390/e21050488




