A Simple Mechanism Causing Wealth Concentration
Abstract
:1. Introduction
1.1. Some Stylised Facts Related to Empirical Wealth Distribution
1.2. The General Structure of Kinetic Exchange Models
2. Model
- (i)
- agents are equal in the sense that each of them has the same access to the market and the same knowledge about it.
- (ii)
- agent trade only when it is profitable from their perspective.
- The first agent i is chosen randomly with the probability equal to its wealth .
- The second agent j is chosen randomly with the probability equal to its wealth .
- If or agent i has traded with agent j in this cycle, go to point 1. Otherwise, make the trade.
3. Results and Discussion
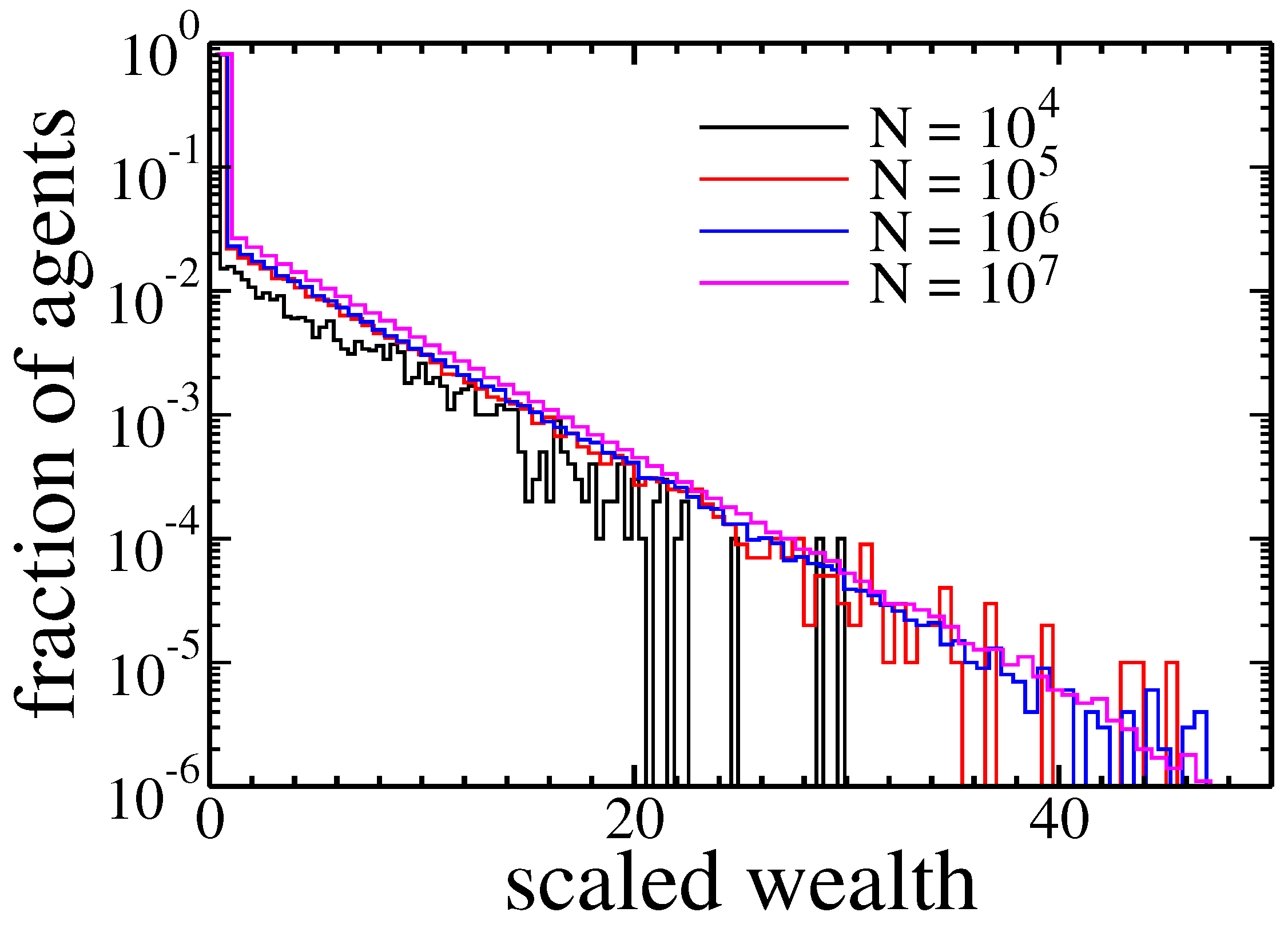
3.1. Wealth Condensation
- (a)
- delta distribution—all agents started with the same amount of money;
- (b)
- uniform distribution—the initial wealth of each agent was uniformly distributed on the interval ;
- (c)
- exponential distribution—the initial wealth was drawn according to the exponential distribution of the unit mean and variance;
- (d)
- Gaussian distribution—the initial wealth of each agent was an absolute value of a number drawn according to the normal distribution of the zero mean value and unit variance;
- (e)
- Cauchy distribution—the initial wealth of each agent was an absolute value of a number drawn according to the following probability distribution function
- (f)
- of richest agents possessed 100 times more money than the remaining of poorer agents.
- (i)
- linear preferences utility function: —gains from individual transaction depend on assets of both sides of trade process.
- (ii)
- Cobb–Douglas utility function: —similar as in the above case but gains were much lower when agents assets differed significantly.
- (iii)
- Koopmans and Leontieff utility function: —gains are determined by a poorer trader.
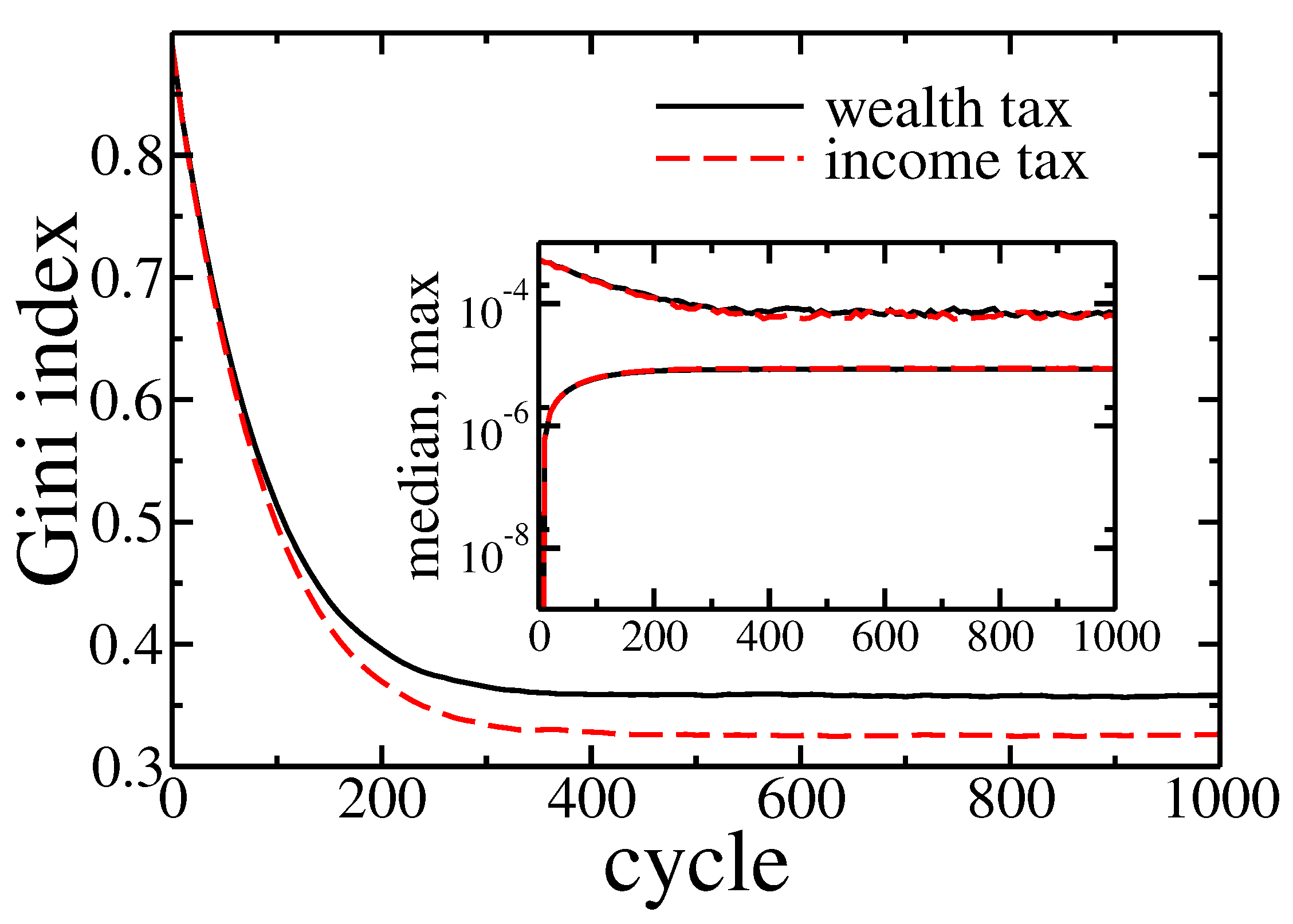
3.2. Income and Wealth Tax Influence on the Model
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Pareto, V. Cours d’économie Politique; Librairie Droz: Geneva, Switzerland, 1964; Volume 1. [Google Scholar]
- Heathcote, J.; Perri, F.; Violante, G.L. Unequal we stand: An empirical analysis of economic inequality in the United States, 1967–2006. Rev. Econ. Dyn. 2010, 13, 15–51. [Google Scholar] [CrossRef] [Green Version]
- Ljungqvist, L.; Sargent, T.J. Recursive Macroeconomic Theory, 2nd ed.; MIT Press: Cambridge, MA, USA, 2004. [Google Scholar]
- Huggett, M. Wealth distribution in life-cycle economies. J. Monet. Econ. 1996, 38, 469–494. [Google Scholar] [CrossRef]
- Golosov, M.; Lorenzoni, G.; Tsyvinski, A. Decentralized trading with private information. Econometrica 2014, 82, 1055–1091. [Google Scholar]
- Bentley, R.A.; Lake, M.W.; Shennan, S.J. Specialisation and wealth inequality in a model of a clustered economic network. J. Archaeol. Sci. 2005, 32, 1346–1356. [Google Scholar] [CrossRef]
- Mohanty, P. Why only few are so successful? Physica A 2007, 384, 75–79. [Google Scholar] [CrossRef] [Green Version]
- De Grauwe, P. Animal spirits and monetary policy. Econ. Theory 2011, 47, 423–457. [Google Scholar] [CrossRef] [Green Version]
- Patriarca, M.; Heinsalu, E.; Chakraborti, A. Basic kinetic wealth-exchange models: Common features and open problems. Eur. Phys. J. B 2010, 73, 145–153. [Google Scholar] [CrossRef] [Green Version]
- Angle, J. The surplus theory of social stratification and the size distribution of personal wealth. Soc. Forces 1986, 65, 293–326. [Google Scholar] [CrossRef]
- Bennati, E. Un metodo di simulazione statistica nell’analisi della distribuzione del reddito. Riv. Internazionale Sci. Econ. Commer. 1988, 35, 735–756. [Google Scholar]
- Chakraborti, A.; Chakrabarti, B.K. Statistical mechanics of money: How saving propensity affects its distribution. Eur. Phys. J. Condens. Matter Complex Syst. 2000, 17, 167–170. [Google Scholar] [CrossRef] [Green Version]
- Dragulescu, A.; Yakovenko, V.M. Statistical mechanics of money. EPJ B 2000, 17, 723–729. [Google Scholar] [CrossRef] [Green Version]
- Vallejos, H.A.; Nutaro, J.J.; Perumalla, K.S. An agent-based model of the observed distribution of wealth in the United States. J. Econ. Interact. Coord. 2018, 13, 641–656. [Google Scholar] [CrossRef] [Green Version]
- Lim, G.; Min, S. Analysis of solidarity effect for entropy, Pareto, and Gini indices on two-class society using kinetic wealth exchange model. Entropy 2020, 22, 386. [Google Scholar] [CrossRef] [Green Version]
- Cowell, F.; Karagiannaki, E.; McKnight, A. The changing distribution of wealth in the pre-crisis US and UK: The role of socio-economic factors. Oxf. Econ. Pap. 2019, 71, 1–24. [Google Scholar] [CrossRef]
- Cressie, N.; Read, T.R. Multinomial goodness-of-fit tests. J. R. Stat. Soc. Ser. Methodol. 1984, 46, 440–464. [Google Scholar] [CrossRef]
- Judge, G.G.; Mittelhammer, R.C. Implications of the Cressie-Read family of additive divergences for information recovery. Entropy 2012, 14, 2427–2438. [Google Scholar] [CrossRef]
- Rigby, R.A.; Stasinopoulos, D.M. Generalized additive models for location, scale and shape. J. R. Stat. Soc. Ser. Appl. Stat. 2005, 54, 507–554. [Google Scholar] [CrossRef] [Green Version]
- Angle, J. The inequality process as a wealth maximizing process. Phys. A Stat. Mech. Its Appl. 2006, 367, 388–414. [Google Scholar] [CrossRef] [Green Version]
- Bouchaud, J.P.; Mézard, M. Wealth condensation in a simple model of economy. Physica A 2000, 282, 536–545. [Google Scholar] [CrossRef] [Green Version]
- Burda, Z.; Johnston, D.; Jurkiewicz, J.; Kamiński, M.; Nowak, M.A.; Papp, G.; Zahed, I. Wealth condensation in pareto macroeconomies. Phys. Rev. E 2002, 65, 026102. [Google Scholar] [CrossRef] [Green Version]
- Vatn, A. Cooperative behavior and institutions. J. Socio-Econ. 2009, 38, 188–196. [Google Scholar] [CrossRef]
- Silberberg, E.; Suen, W.C. The Structure of Economics: A Mathematical Analysis, 3rd ed.; McGraw-Hill: New York, NY, USA, 2000. [Google Scholar]
- Goswami, S.; Sen, P. Agent based models for wealth distribution with preference in interaction. Physica A 2014, 415, 514–524. [Google Scholar] [CrossRef] [Green Version]
- Bertotti, M.L.; Modanese, G. From microscopic taxation and redistribution models to macroscopic income distributions. Phys. A Stat. Mech. Its Appl. 2011, 390, 3782–3793. [Google Scholar] [CrossRef] [Green Version]
- Kulp, C.W.; Kurtz, M.; Wilston, N.; Quigley, L. The effect of various tax and redistribution models on the Gini coefficient of simple exchange games. Chaos Interdiscip. J. Nonlinear Sci. 2019, 29, 083118. [Google Scholar] [CrossRef] [PubMed]
- Diniz, M.; Mendes, F. Effects of taxation on money distribution. Int. Rev. Financ. Anal. 2012, 23, 81–85. [Google Scholar] [CrossRef]
- Piketty, T.; Saez, E. A theory of optimal inheritance taxation. Econometrica 2013, 81, 1851–1886. [Google Scholar]








| Distribution | 2010 | 2018 | ||||
|---|---|---|---|---|---|---|
| Gini | Aic | Bic | Gini | Aic | Bic | |
| Generalised Beta of the Second Kind | 0.4504 | 825,368.9827 | 825,407.7613 | 0.4656 | 914,590.8355 | 914,629.8928 |
| Generalised Gamma | 0.4485 | 825,598.8432 | 825,627.9271 | 0.4526 | 915,341.0957 | 915,370.3886 |
| Beta of the Second Kind | 0.4545 | 825,501.8130 | 825,530.8969 | 0.4636 | 915,233.3365 | 915,262.6295 |
| Dagum | 1,258,915.0143 | 1,258,944.0982 | 0.4693 | 914,642.1348 | 914,671.4277 | |
| Singmad | 0.4531 | 827,239.3961 | 827,268.4800 | 0.4600 | 914,833.1633 | 914,862.4562 |
| Lognormal | 0.5013 | 832,408.2444 | 832,427.6337 | 0.5206 | 924,094.3485 | 924,113.8772 |
| Weibull | 0.4432 | 827,065.1604 | 827,084.5496 | 0.4462 | 916,179.0877 | 916,198.6163 |
| Gamma | 0.4409 | 826,112.8345 | 826,132.2238 | 0.4467 | 915,559.4152 | 915,578.9439 |
| Doubly lognormal | 1,375,275.6949 | 1,375,295.0841 | 920,090.4281 | 920,109.9568 | ||
| Pareto | 0.5047 | 832,191.8408 | 832,211.2301 | 0.5061 | 920,845.1144 | 920,864.6431 |
| Distribution | 2010 | 2018 | ||||||
|---|---|---|---|---|---|---|---|---|
| Generalised Beta of the Second Kind | 108,564.1708 | 1.7786 | 0.7034 | 2.0083 | 113,253.3847 | 2.1917 | 0.5323 | 1.2229 |
| Generalised Gamma | 60,663.6500 | 0.9001 | 0.7612 | 81,899.1321 | 0.9057 | 0.8421 | ||
| Beta of the Second Kind | 283,044.3149 | 1.5608 | 7.5992 | 372,137.5280 | 1.4877 | 7.2873 | ||
| Dagum | 1,012,451,669.9591 | 0.9721 | 0.1021 | 105,486.1708 | 2.4436 | 0.4689 | ||
| Singmad | 1,012,451,669.9591 | 1.1348 | 53,697.3940 | 190,335.2758 | 1.3413 | 3.3841 | ||
| Lognormal | 10.6958 | 0.9900 | 10.9507 | 1.0373 | ||||
| Weibull | 69,527.7650 | 1.1699 | 89,843.3170 | 1.1589 | ||||
| Gamma | 6,5806.3882 | 0.8652 | 85,510.9515 | 0.8789 | ||||
| Doubly lognormal | 1,012,451,669.9591 | 0.1514 | 60,235.5085 | 1.7064 | ||||
| Pareto | 1,549,526.7678 | 24.2996 | ||||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cieśla, M.; Snarska, M. A Simple Mechanism Causing Wealth Concentration. Entropy 2020, 22, 1148. https://doi.org/10.3390/e22101148
Cieśla M, Snarska M. A Simple Mechanism Causing Wealth Concentration. Entropy. 2020; 22(10):1148. https://doi.org/10.3390/e22101148
Chicago/Turabian StyleCieśla, Michał, and Małgorzata Snarska. 2020. "A Simple Mechanism Causing Wealth Concentration" Entropy 22, no. 10: 1148. https://doi.org/10.3390/e22101148
APA StyleCieśla, M., & Snarska, M. (2020). A Simple Mechanism Causing Wealth Concentration. Entropy, 22(10), 1148. https://doi.org/10.3390/e22101148





