Quantum Finite-Time Thermodynamics: Insight from a Single Qubit Engine
Abstract
:1. Introduction
- Finite heat transport.
- Friction.
- Heat leaks.
- Cost of switching contacts between subsystems.
2. Some Preliminaries
2.1. Classical Engines Operating in Finite-Time
2.2. Qubit Engine Model
- (A)
- Hot bath thermalization.
- (B)
- Unitary expansion from hot to cold
- (C)
- Cold bath thermalization
- (D)
- Unitary compression from cold to hot
3. Frictionless Engines
3.1. Elementary Cycles
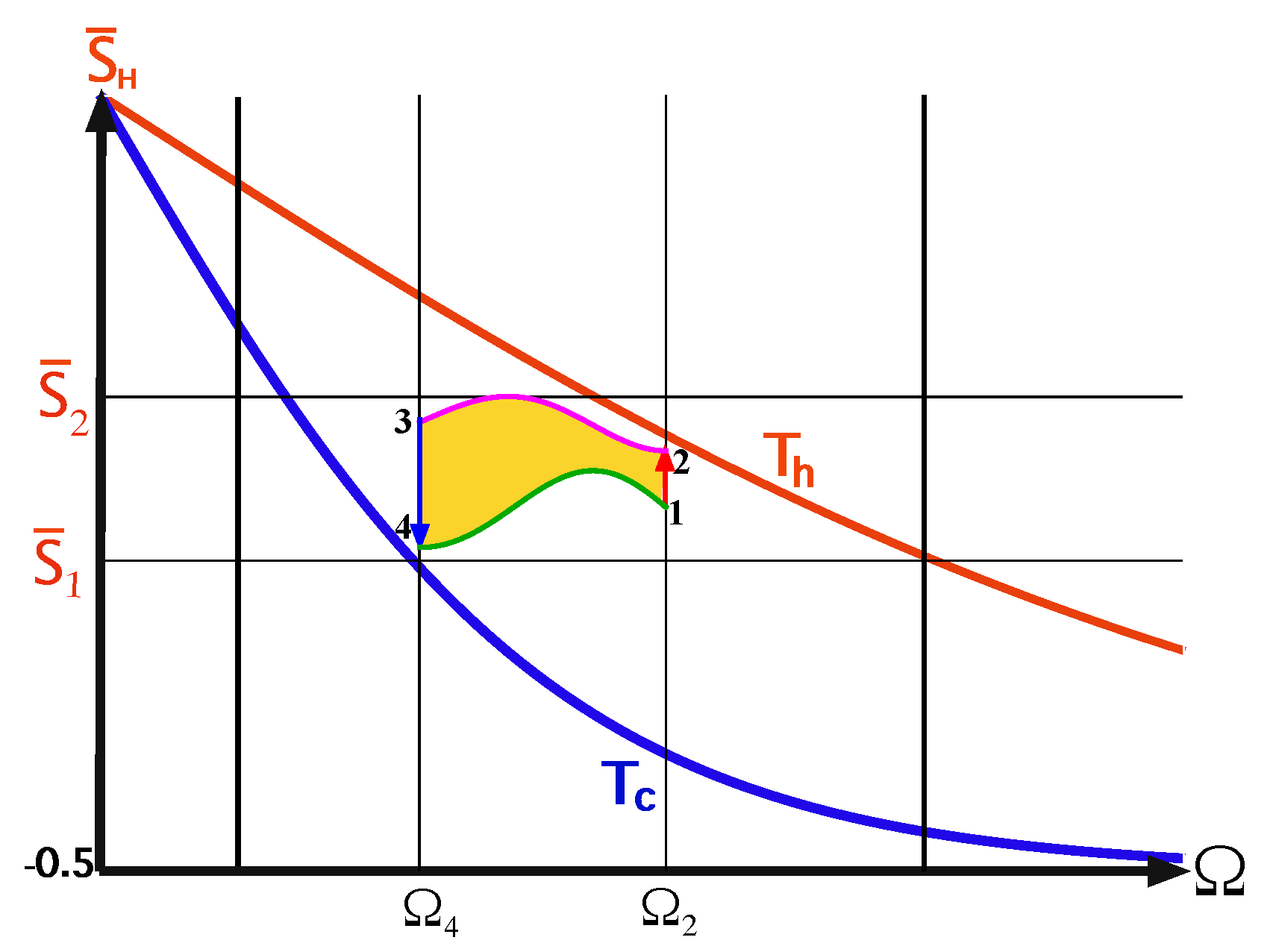
3.2. Elementary Carnot-Type Cycle
3.3. Elementary Otto Cycle
3.4. Optimization of the Elementary Otto Cycle
4. The Quantum Origin of Friction
4.1. Slow Driving Regime
4.2. Sudden Limit
4.3. Shortcuts to Adiabaticity
5. Thermalization
5.1. Isochoric Thermalization
5.2. Isothermal Thermalization
5.3. Shortcut to Equilibrium Protocols
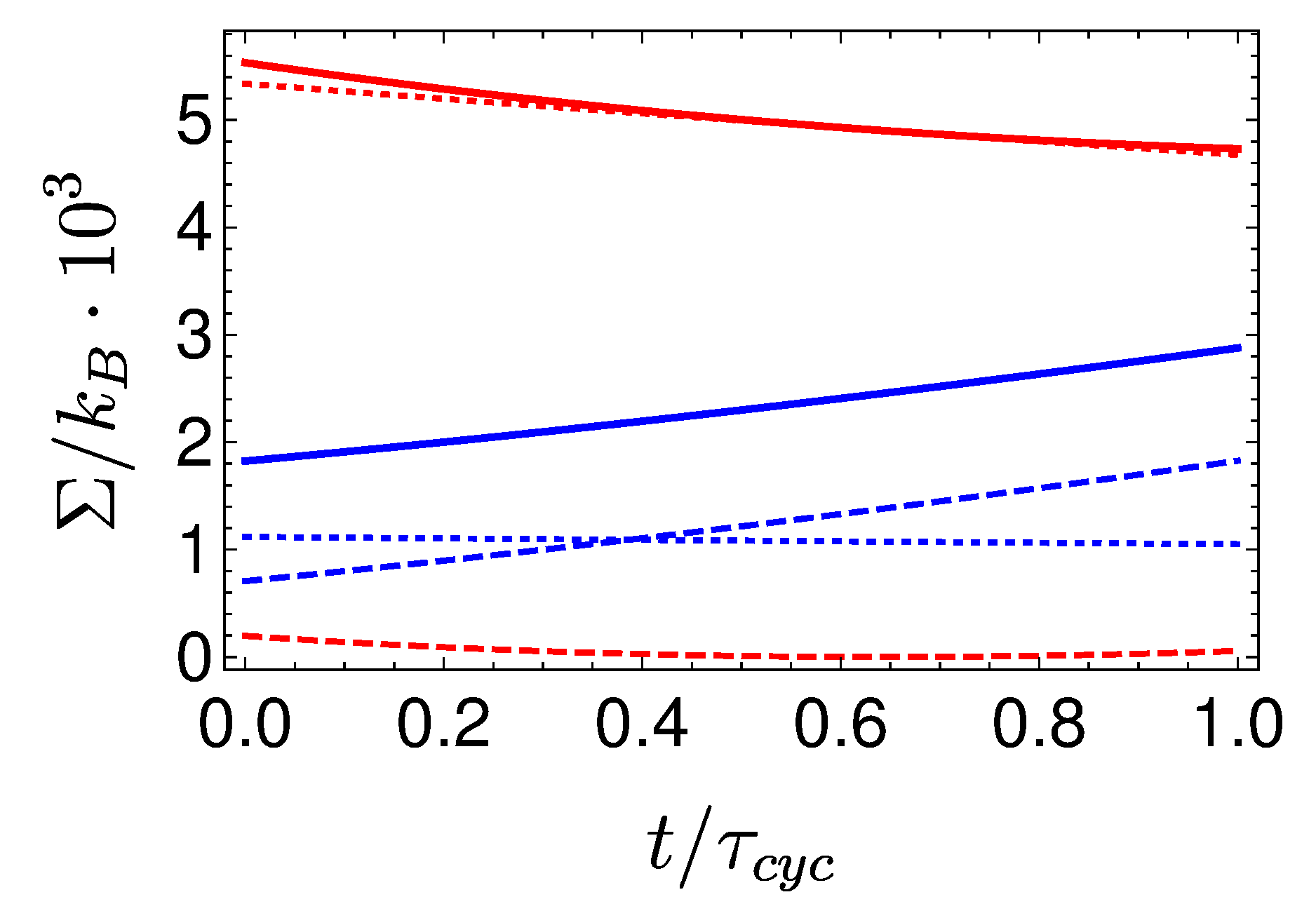
5.4. Thermodynamic Cost of Finite-Time Thermalization
6. Local Cycles
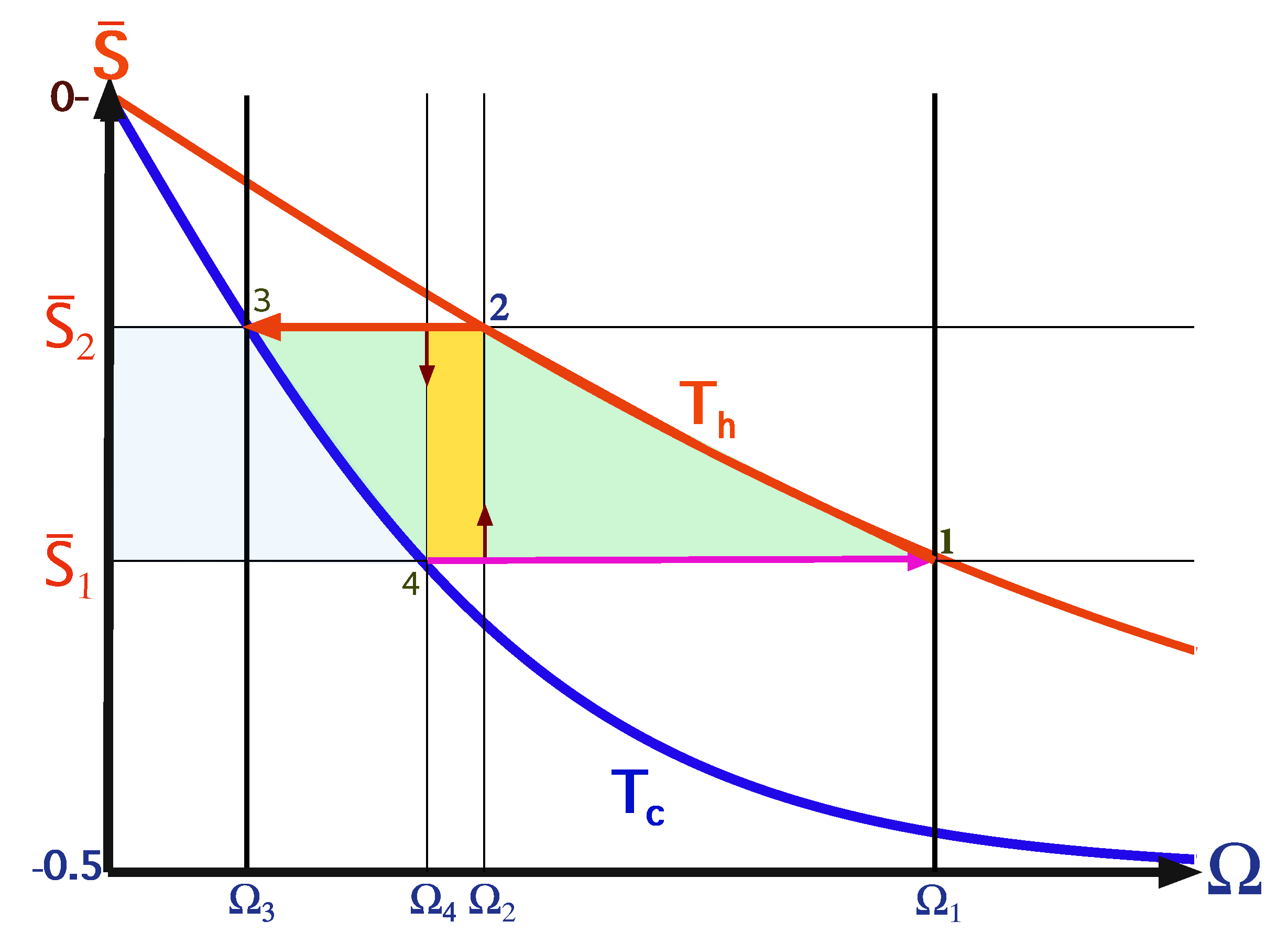
6.1. Local Otto Cycle
6.2. Local Carnot Cycle
7. Global Cycles
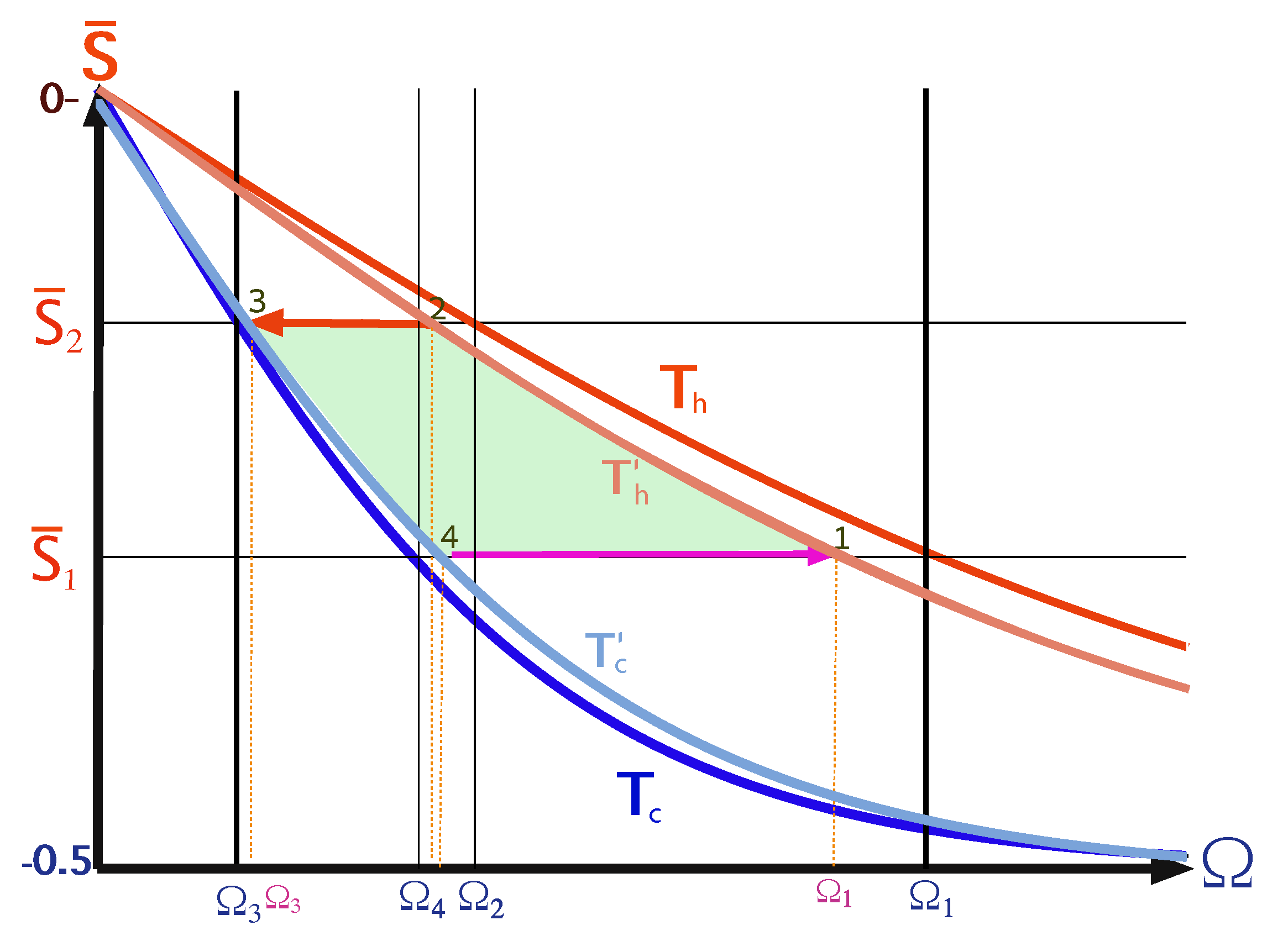
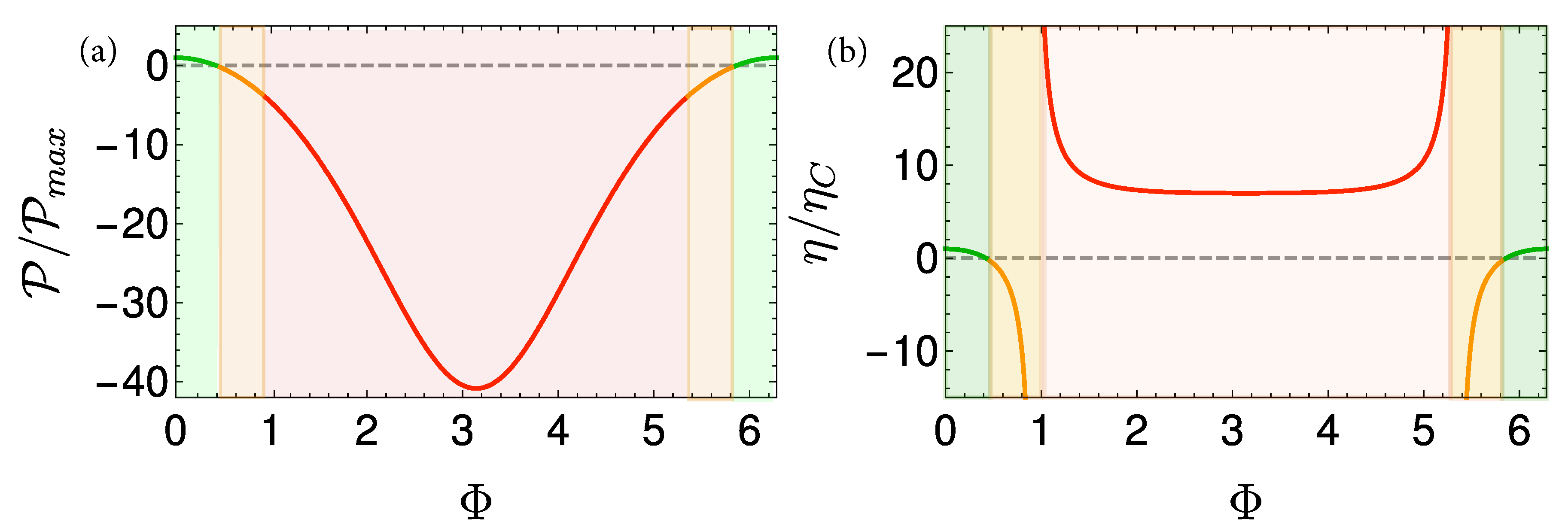
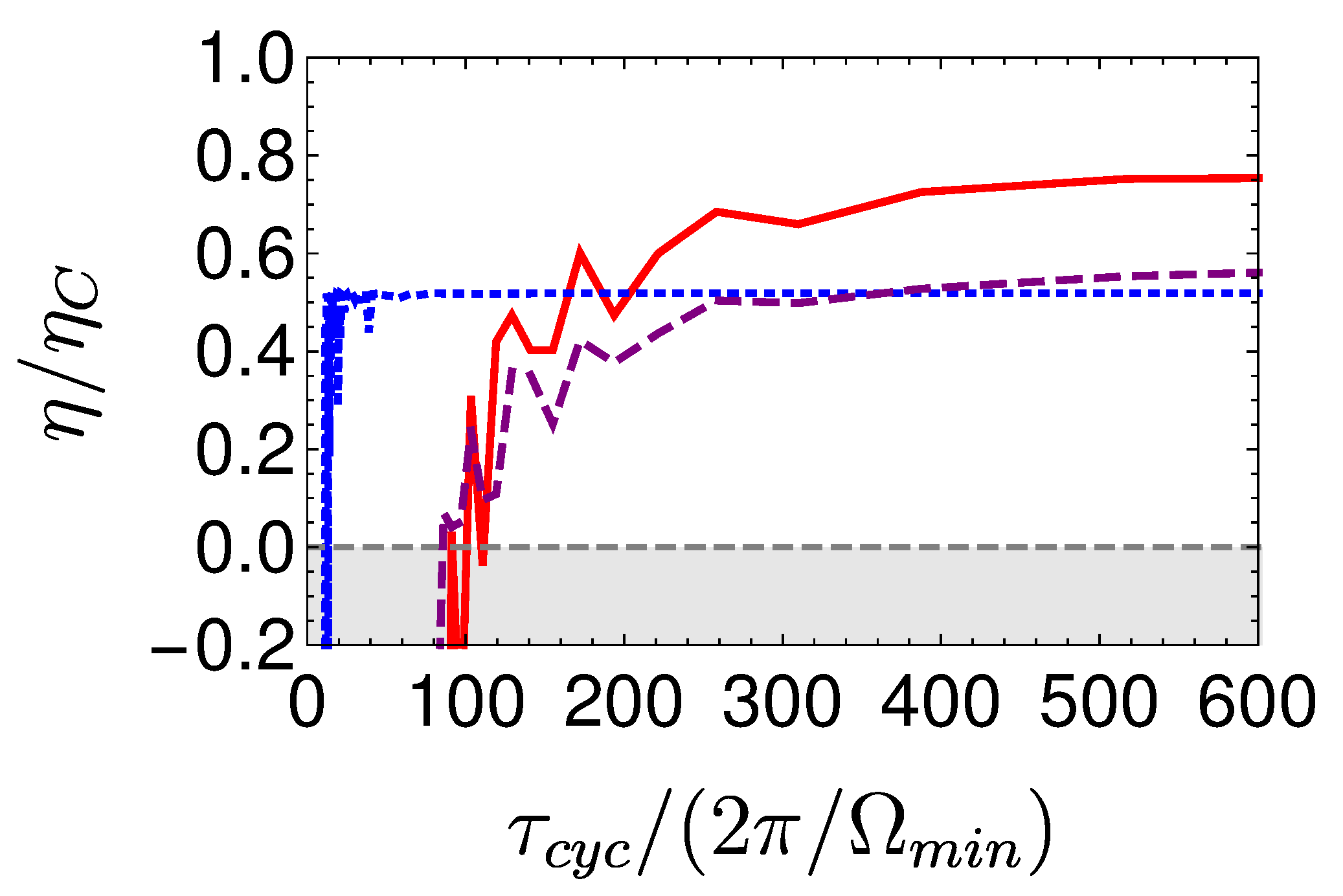
7.1. Global Otto Cycle and the Sudden Limit
7.2. Global Carnot-Type Constant Adiabatic Parameter Cycle
| Parameters | Local Carnot | Globally Coherent Carnot | Local Otto | Globally Coherent Otto |
|---|---|---|---|---|
| 12 | 10 | 8 | 9 | |
| 8 | 9 () | 8 | 9 | |
| 4 | 6 | 6 | 6 | |
| 6 | 6 () | 6 | 6 | |
| Hot bath temperature | ||||
| Cold bath temperature |
| Parameters | Value |
|---|---|
| Coupling constant | |
| Integration step size |
8. Quantum Signature: Constant Adiabatic Parameter Cycles Maintaining Global Coherence
9. Discussion
9.1. What the Qubit Can and Cannot Do
9.2. Further Considerations
9.3. Comparing to the Harmonic Working Fluid
9.4. High Temperature Limit
9.5. Dissipation
9.6. Experimental Connections
10. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| GKLS | Gorini, Kossakowski, Linblad, Sudarshan; Master equation |
| CPTP | Completely Positive Trace Preserving map |
| NAME | Non-Adiabatic Master Equation |
| FEAT | Fastest Effectively Adiabatic transition |
| STA | Shortcut To Adiabticity |
| STE | Shortcut To Equilibrium |
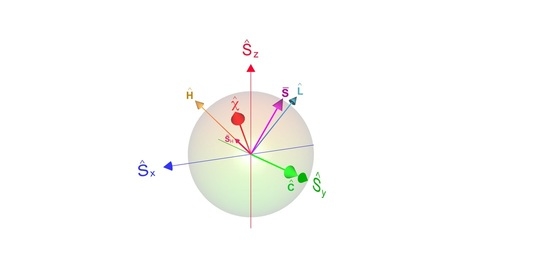
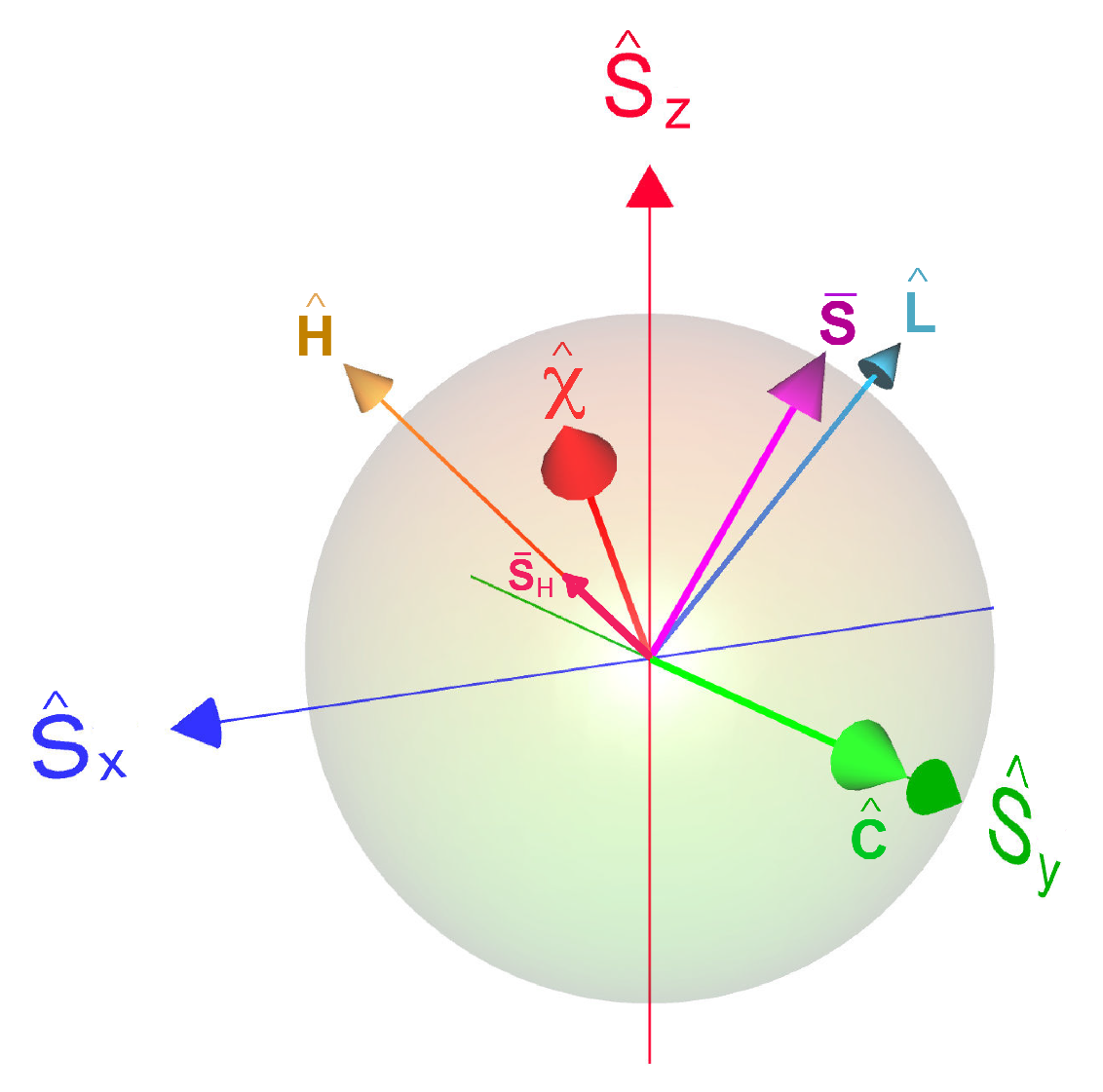
Appendix A. Representations of the Qubit State
Appendix B. Explicit Expressions
| Parameters | Description |
|---|---|
| ; | dynamical operator basis; associated vector in Liouville space |
| ; | polarization operator basis; vector in Liouville space |
| ; | eigenoperator basis; vector in Liouville space |
| ; | eigenoperator basis; vector in Liouville space |
| Heisenberg picture | |
| interaction picture | |
| and | control parameters |
| generalized Rabi frequency | |
| polarization vector | |
| polarization | |
| projection of the polarization vector on the energy axis | |
| thermal polarization | |
| T | bath temperature |
| dynamical propagators | |
| von-Neumann entropy | |
| energy entropy | |
| entropy production per cycle | |
| entropy production rate | |
| decay rate | |
| and | efficiency and work of the Carnot cycle |
| , , and | efficiency, work, power and heat of the i’th cycle |
| adiabatic parameter | |
| Inertial scaling factor | |
| effective frequency | |
| C | coherence |
| work to counter friction | |
| P | transformation matrix between and |
| D | eigenvalue matrix of the eigenoperators |
| effective temperatures | |
| thermodynamic fluxes | |
| thermodynamic force |
References
- Carnot, S. Réflexions sur la Puissance Motrice du feu et sur les Machines Propres à Développer Cette Puissance; Bachelier: Paris, France, 1824. [Google Scholar]
- Salamon, P.; Nulton, J.; Siragusa, G.; Andersen, T.R.; Limon, A. Principles of control thermodynamics. Energy 2001, 26, 307–319. [Google Scholar] [CrossRef] [Green Version]
- Andresen, B.; Berry, R.S.; Ondrechen, M.J.; Salamon, P. Thermodynamics for processes in finite time. Acc. Chem. Res. 1984, 17, 266–271. [Google Scholar] [CrossRef]
- Andresen, B.; Salamon, P.; Berry, R.S. Thermodynamics in finite time: Extremals for imperfect heat engines. J. Chem. Phys. 1977, 66, 1571–1577. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Burzler, J.M.; Schubert, S. Endoreversible Thermodynamics. J. Non-Equilib. Thermodyn. 1997, 22, 311–355. [Google Scholar]
- Andresen, B. Current trends in finite-time thermodynamics. Angew. Chem. Int. Ed. 2011, 50, 2690–2704. [Google Scholar] [CrossRef]
- Curzon, F.; Ahlborn, B. Efficiency of a Carnot engine at maximum power output. Am. J. Phys. 1975, 43, 22–24. [Google Scholar] [CrossRef]
- Salamon, P.; Nitzan, A. Finite time optimizations of a Newton’s law Carnot cycle. J. Chem. Phys. 1981, 74, 3546–3560. [Google Scholar] [CrossRef]
- Kraus, K. General state changes in quantum theory. Ann. Phys. 1971, 64, 311–335. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Commun. Math. Phys. 1976, 48, 119–130. [Google Scholar] [CrossRef]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of N-level systems. J. Math. Phys. 1976, 17, 821–825. [Google Scholar] [CrossRef]
- Alicki, R.; Kosloff, R. Introduction to Quantum Thermodynamics: History and Prospects. In Thermodynamics in the Quantum Regime; Binder, F., Correa, L.A., Gogolin, C., Anders, J., Adesso, G., Eds.; Fundamental Theories of Physics; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar] [CrossRef] [Green Version]
- Davies, E.B. Markovian master equations. Commun. Math. Phys. 1974, 39, 91–110. [Google Scholar] [CrossRef]
- Levy, A.; Kosloff, R. The local approach to quantum transport may violate the second law of thermodynamics. EPL Europhys. Lett. 2014, 107, 20004. [Google Scholar] [CrossRef] [Green Version]
- Barra, F. The thermodynamic cost of driving quantum systems by their boundaries. Sci. Rep. 2015, 5, 14873. [Google Scholar] [CrossRef] [Green Version]
- Hofer, P.P.; Perarnau-Llobet, M.; Miranda, L.D.M.; Haack, G.; Silva, R.; Brask, J.B.; Brunner, N. Markovian master equations for quantum thermal machines: Local versus global approach. New J. Phys. 2017, 19, 123037. [Google Scholar] [CrossRef]
- González, J.O.; Correa, L.A.; Nocerino, G.; Palao, J.P.; Alonso, D.; Adesso, G. Testing the validity of the ‘local’and ‘global’GKLS master equations on an exactly solvable model. Open Syst. Inf. Dyn. 2017, 24, 1740010. [Google Scholar] [CrossRef]
- De Chiara, G.; Landi, G.; Hewgill, A.; Reid, B.; Ferraro, A.; Roncaglia, A.J.; Antezza, M. Reconciliation of quantum local master equations with thermodynamics. New J. Phys. 2018, 20, 113024. [Google Scholar] [CrossRef]
- Hewgill, A.; De Chiara, G.; Imparato, A. Quantum thermodynamically consistent local master equations. arXiv 2020, arXiv:2008.04742. [Google Scholar]
- Allahverdyan, A.; Nieuwenhuizen, T.M. Extraction of work from a single thermal bath in the quantum regime. Phys. Rev. Lett. 2000, 85, 1799. [Google Scholar] [CrossRef] [Green Version]
- Hilt, S.; Shabbir, S.; Anders, J.; Lutz, E. Landauer’s principle in the quantum regime. Phys. Rev. E 2011, 83, 030102. [Google Scholar] [CrossRef] [Green Version]
- Boukobza, E.; Ritsch, H. Breaking the Carnot limit without violating the second law: A thermodynamic analysis of off-resonant quantum light generation. Phys. Rev. A 2013, 87, 063845. [Google Scholar] [CrossRef]
- Uzdin, R.; Levy, A.; Kosloff, R. Equivalence of quantum heat machines, and quantum-thermodynamic signatures. Phys. Rev. X 2015, 5, 031044. [Google Scholar] [CrossRef] [Green Version]
- Roßnagel, J.; Abah, O.; Schmidt-Kaler, F.; Singer, K.; Lutz, E. Nanoscale heat engine beyond the Carnot limit. Phys. Rev. Lett. 2014, 112, 030602. [Google Scholar] [CrossRef]
- Alicki, R.; Fannes, M. Entanglement boost for extractable work from ensembles of quantum batteries. Phys. Rev. E 2013, 87, 042123. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mandal, D.; Jarzynski, C. Work and information processing in a solvable model of Maxwell’s demon. Proc. Natl. Acad. Sci. USA 2012, 109, 11641–11645. [Google Scholar] [CrossRef] [Green Version]
- Vidrighin, M.D.; Dahlsten, O.; Barbieri, M.; Kim, M.; Vedral, V.; Walmsley, I.A. Photonic Maxwell’s demon. Phys. Rev. Lett. 2016, 116, 050401. [Google Scholar] [CrossRef] [Green Version]
- Geva, E.; Kosloff, R.; Skinner, J. On the relaxation of a two-level system driven by a strong electromagnetic field. J. Chem. Phys. 1995, 102, 8541–8561. [Google Scholar] [CrossRef]
- Geva, E.; Kosloff, R. The quantum heat engine and heat pump: An irreversible thermodynamic analysis of the three-level amplifier. J. Chem. Phys. 1996, 104, 7681–7699. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Palao, J.P.; Adesso, G.; Alonso, D. Performance bound for quantum absorption refrigerators. Phys. Rev. E 2013, 87, 042131. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Palao, J.P.; Alonso, D.; Adesso, G. Quantum-enhanced absorption refrigerators. Sci. Rep. 2014, 4, 3949. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Alicki, R.; Gelbwaser-Klimovsky, D.; Kurizki, G. Periodically driven quantum open systems: Tutorial. arXiv 2012, arXiv:1205.4552. [Google Scholar]
- Albash, T.; Boixo, S.; Lidar, D.A.; Zanardi, P. Quantum adiabatic Markovian master equations. New J. Phys. 2012, 14, 123016. [Google Scholar] [CrossRef]
- Dann, R.; Levy, A.; Kosloff, R. Time-dependent Markovian quantum master equation. Phys. Rev. A 2018, 98, 052129. [Google Scholar] [CrossRef] [Green Version]
- Kosloff, R. Quantum thermodynamics: A dynamical viewpoint. Entropy 2013, 15, 2100–2128. [Google Scholar] [CrossRef] [Green Version]
- Mandelstam, L.; Tamm, I. The energy–time uncertainty relation in non-relativistic quantum mechanics. Izv. Akad. Nauk SSSR 1945, 9, 122. [Google Scholar]
- Jones, P.J.; Kok, P. Geometric derivation of the quantum speed limit. Phys. Rev. A 2010, 82, 022107. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti, V.; Lloyd, S.; Maccone, L. The speed limit of quantum unitary evolution. J. Opt. B Quantum Semiclassical Opt. 2004, 6, S807. [Google Scholar] [CrossRef]
- Del Campo, A.; Egusquiza, I.; Plenio, M.B.; Huelga, S.F. Quantum speed limits in open system dynamics. Phys. Rev. Lett. 2013, 110, 050403. [Google Scholar] [CrossRef] [Green Version]
- Uzdin, R.; Kosloff, R. Speed limits in Liouville space for open quantum systems. EPL Europhys. Lett. 2016, 115, 40003. [Google Scholar] [CrossRef] [Green Version]
- Funo, K.; Shiraishi, N.; Saito, K. Speed limit for open quantum systems. New J. Phys. 2019, 21, 013006. [Google Scholar] [CrossRef]
- von Lindenfels, D.; Gräb, O.; Schmiegelow, C.T.; Kaushal, V.; Schulz, J.; Mitchison, M.T.; Goold, J.; Schmidt-Kaler, F.; Poschinger, U.G. Spin Heat Engine Coupled to a Harmonic-Oscillator Flywheel. Phys. Rev. Lett. 2019, 123, 080602. [Google Scholar] [CrossRef] [Green Version]
- Ono, K.; Shevchenko, S.; Mori, T.; Moriyama, S.; Nori, F. Analog of a quantum heat engine using a single-spin qubit. Phys. Rev. Lett. 2020, 125, 166802. [Google Scholar] [CrossRef]
- Bouton, Q.; Nettersheim, J.; Burgardt, S.; Adam, D.; Lutz, E.; Widera, A. An endoreversible quantum heat engine driven by atomic collisions. arXiv 2020, arXiv:2009.10946. [Google Scholar]
- Bejan, A.; Kestin, J. Entropy Generation Through Heat and Fluid Flow. J. Appl. Mech. 1983, 50, 475. [Google Scholar] [CrossRef]
- Szargut, J. International progress in second law analysis. Energy 1980, 5, 709–718. [Google Scholar] [CrossRef]
- Gouy, M. Sur l’energie utilisable. J. Phys. 1889, 8, 501. [Google Scholar] [CrossRef]
- Stodola, A. Steam and Gas Turbines; McGraw-Hill: New York, NY, USA, 1927. [Google Scholar]
- Feldmann, T.; Kosloff, R. Characteristics of the limit cycle of a reciprocating quantum heat engine. Phys. Rev. E 2004, 70, 046110. [Google Scholar] [CrossRef] [Green Version]
- Lindblad, G. Expectations and entropy inequalities for finite quantum systems. Commun. Math. Phys. 1974, 39, 111–119. [Google Scholar] [CrossRef]
- Feldmann, T.; Kosloff, R. Quantum four-stroke heat engine: Thermodynamic observables in a model with intrinsic friction. Phys. Rev. E 2003, 68, 016101. [Google Scholar] [CrossRef] [Green Version]
- Baumgratz, T.; Cramer, M.; Plenio, M.B. Quantifying coherence. Phys. Rev. Lett. 2014, 113, 140401. [Google Scholar] [CrossRef] [Green Version]
- Girolami, D. Observable Measure of Quantum Coherence in Finite Dimensional Systems. Phys. Rev. Lett. 2014, 113, 170401. [Google Scholar] [CrossRef] [Green Version]
- Feldmann, T.; Kosloff, R. Transitions between refrigeration regions in extremely short quantum cycles. Phys. Rev. E 2016, 93, 052150. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, J. Divergence measures based on the Shannon entropy. IEEE Trans. Inf. Theory 1991, 37, 145–151. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Messiah, A. Quantum Mechanics: Translated [from the French] by J. Potter; North-Holland: Amsterdam, The Netherlands, 1962. [Google Scholar]
- Spohn, H.; Lebowitz, J.L. Irreversible thermodynamics for quantum systems weakly coupled to thermal reservoirs. Adv. Chem. Phys. 1978, 38, 109–142. [Google Scholar]
- Alicki, R. The quantum open system as a model of the heat engine. J. Phys. A Math. Gen. 1979, 12, L103. [Google Scholar] [CrossRef]
- Abiuso, P.; Miller, H.J.; Perarnau-Llobet, M.; Scandi, M. Geometric optimisation of quantum thermodynamic processes. Entropy 2020, 22, 1076. [Google Scholar] [CrossRef]
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef] [Green Version]
- Sekimoto, K. Stochastic Energetics; Springer: Berlin/Heidelberg, Germany, 2010; Volume 799. [Google Scholar]
- Feldmann, T.; Geva, E.; Kosloff, R.; Salamon, P. Heat engines in finite time governed by master equations. Am. J. Phys. 1996, 64, 485–492. [Google Scholar] [CrossRef]
- Geva, E.; Kosloff, R. A quantum-mechanical heat engine operating in finite time. A model consisting of spin-1/2 systems as the working fluid. J. Chem. Phys. 1992, 96, 3054–3067. [Google Scholar] [CrossRef] [Green Version]
- Novikov, I. The efficiency of atomic power stations (a review). J. Nucl. Energy (1954) 1958, 7, 125–128. [Google Scholar] [CrossRef]
- Esposito, M.; Kawai, R.; Lindenberg, K.; Van den Broeck, C. Efficiency at maximum power of low-dissipation Carnot engines. Phys. Rev. Lett. 2010, 105, 150603. [Google Scholar] [CrossRef]
- Abiuso, P.; Perarnau-Llobet, M. Optimal cycles for low-dissipation heat engines. Phys. Rev. Lett. 2020, 124, 110606. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abiuso, P.; Giovannetti, V. Non-Markov enhancement of maximum power for quantum thermal machines. Phys. Rev. A 2019, 99, 052106. [Google Scholar] [CrossRef] [Green Version]
- Erdman, P.A.; Cavina, V.; Fazio, R.; Taddei, F.; Giovannetti, V. Maximum power and corresponding efficiency for two-level heat engines and refrigerators: Optimality of fast cycles. New J. Phys. 2019, 21, 103049. [Google Scholar] [CrossRef] [Green Version]
- Karimi, B.; Pekola, J. Otto refrigerator based on a superconducting qubit: Classical and quantum performance. Phys. Rev. B 2016, 94, 184503. [Google Scholar] [CrossRef] [Green Version]
- Pekola, J.P.; Karimi, B.; Thomas, G.; Averin, D.V. Supremacy of incoherent sudden cycles. Phys. Rev. B 2019, 100, 085405. [Google Scholar] [CrossRef] [Green Version]
- Feldmann, T.; Kosloff, R. Performance of discrete heat engines and heat pumps in finite time. Phys. Rev. E 2000, 61, 4774. [Google Scholar] [CrossRef] [Green Version]
- Scully, M.O.; Zubairy, M.S.; Agarwal, G.S.; Walther, H. Extracting work from a single heat bath via vanishing quantum coherence. Science 2003, 299, 862–864. [Google Scholar] [CrossRef]
- Francica, G.; Goold, J.; Plastina, F. Role of coherence in the nonequilibrium thermodynamics of quantum systems. Phys. Rev. E 2019, 99, 042105. [Google Scholar] [CrossRef] [Green Version]
- Dann, R.; Kosloff, R. Quantum signatures in the quantum Carnot cycle. New J. Phys. 2020, 22, 013055. [Google Scholar] [CrossRef]
- Kosloff, R.; Feldmann, T. Discrete four-stroke quantum heat engine exploring the origin of friction. Phys. Rev. E 2002, 65, 055102. [Google Scholar] [CrossRef] [Green Version]
- Boldt, F.; Nulton, J.D.; Andresen, B.; Salamon, P.; Hoffmann, K.H. Casimir companion: An invariant of motion for Hamiltonian systems. Phys. Rev. A 2013, 87, 022116. [Google Scholar] [CrossRef] [Green Version]
- Brandner, K.; Saito, K. Thermodynamic geometry of microscopic heat engines. Phys. Rev. Lett. 2020, 124, 040602. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Ruschhaupt, A.; Schmidt, S.; del Campo, A.; Guéry-Odelin, D.; Muga, J.G. Fast optimal frictionless atom cooling in harmonic traps: Shortcut to adiabaticity. Phys. Rev. Lett. 2010, 104, 063002. [Google Scholar] [CrossRef]
- Guéry-Odelin, D.; Ruschhaupt, A.; Kiely, A.; Torrontegui, E.; Martínez-Garaot, S.; Muga, J.G. Shortcuts to adiabaticity: Concepts, methods, and applications. Rev. Mod. Phys. 2019, 91, 045001. [Google Scholar] [CrossRef]
- Torrontegui, E.; Lizuain, I.; González-Resines, S.; Tobalina, A.; Ruschhaupt, A.; Kosloff, R.; Muga, J.G. Energy consumption for shortcuts to adiabaticity. Phys. Rev. A 2017, 96, 022133. [Google Scholar] [CrossRef]
- Zheng, Y.; Campbell, S.; De Chiara, G.; Poletti, D. Cost of counterdiabatic driving and work output. Phys. Rev. A 2016, 94, 042132. [Google Scholar] [CrossRef] [Green Version]
- Campbell, S.; Deffner, S. Trade-off between speed and cost in shortcuts to adiabaticity. Phys. Rev. Lett. 2017, 118, 100601. [Google Scholar] [CrossRef] [Green Version]
- Abah, O.; Lutz, E. Performance of shortcut-to-adiabaticity quantum engines. Phys. Rev. E 2018, 98, 032121. [Google Scholar] [CrossRef] [Green Version]
- Çakmak, B.; Müstecaplıoğlu, Ö.E. Spin quantum heat engines with shortcuts to adiabaticity. Phys. Rev. E 2019, 99, 032108. [Google Scholar] [CrossRef] [Green Version]
- Boldt, F.; Hoffmann, K.; Salamon, P.; Kosloff, R. Time-optimal processes for interacting spin systems. EPL Europhys. Lett. 2012, 99, 40002. [Google Scholar] [CrossRef]
- Boldt, F.; Salamon, P.; Hoffmann, K.H. Fastest effectively adiabatic transitions for a collection of harmonic oscillators. J. Phys. Chem. A 2016, 120, 3218–3224. [Google Scholar] [CrossRef] [PubMed]
- Funo, K.; Lambert, N.; Karimi, B.; Pekola, J.P.; Masuyama, Y.; Nori, F. Speeding up a quantum refrigerator via counterdiabatic driving. Phys. Rev. B 2019, 100, 035407. [Google Scholar] [CrossRef] [Green Version]
- Tobalina, A.; Lizuain, I.; Muga, J. Vanishing efficiency of a speeded-up ion-in-Paul-trap Otto engine. EPL Europhys. Lett. 2019, 127, 20005. [Google Scholar] [CrossRef]
- Dann, R.; Tobalina, A.; Kosloff, R. Shortcut to equilibration of an open quantum system. Phys. Rev. Lett. 2019, 122, 250402. [Google Scholar] [CrossRef] [Green Version]
- Dann, R.; Tobalina, A.; Kosloff, R. Fast route to equilibration. Phys. Rev. A 2020, 101, 052102. [Google Scholar] [CrossRef]
- Pancotti, N.; Scandi, M.; Mitchison, M.T.; Perarnau-Llobet, M. Speed-Ups to Isothermality: Enhanced Quantum Thermal Machines through Control of the System-Bath Coupling. Phys. Rev. X 2020, 10, 031015. [Google Scholar]
- Villazon, T.; Polkovnikov, A.; Chandran, A. Swift heat transfer by fast-forward driving in open quantum systems. Phys. Rev. A 2019, 100, 012126. [Google Scholar] [CrossRef] [Green Version]
- Henrich, M.J.; Rempp, F.; Mahler, G. Quantum thermodynamic Otto machines: A spin-system approach. Eur. Phys. J. Spec. Top. 2007, 151, 157–165. [Google Scholar] [CrossRef]
- Kosloff, R.; Rezek, Y. The quantum harmonic Otto cycle. Entropy 2017, 19, 136. [Google Scholar] [CrossRef] [Green Version]
- Wu, F.; Chen, L.; Sun, F.; Wu, C. Finite-time exergoeconomic performance bound for a quantum Stirling engine. Int. J. Eng. Sci. 2000, 38, 239–247. [Google Scholar] [CrossRef]
- Yin, Y.; Chen, L.; Wu, F. Optimal power and efficiency of quantum Stirling heat engines. Eur. Phys. J. Plus 2017, 132, 1–10. [Google Scholar] [CrossRef]
- Dong, C.; Lefkidis, G.; Hübner, W. Magnetic quantum diesel engine in Ni 2. Phys. Rev. B 2013, 88, 214421. [Google Scholar] [CrossRef]
- Dann, R.; Kosloff, R. The Inertial Theorem. arXiv 2018, arXiv:1810.12094. [Google Scholar]
- Scandi, M.; Miller, H.J.; Anders, J.; Perarnau-Llobet, M. Quantum work statistics close to equilibrium. Phys. Rev. Res. 2020, 2, 023377. [Google Scholar] [CrossRef]
- Alhassid, Y.; Levine, R. Connection between the maximal entropy and the scattering theoretic analyses of collision processes. Phys. Rev. A 1978, 18, 89. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal relations in irreversible processes. I. Phys. Rev. 1931, 37, 405. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal relations in irreversible processes. II. Phys. Rev. 1931, 38, 2265. [Google Scholar] [CrossRef] [Green Version]
- De Groot, S.R.; Mazur, P. Non-Equilibrium Thermodynamics; North-Holland: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Hoffmann, K.; Salamon, P.; Rezek, Y.; Kosloff, R. Time-optimal controls for frictionless cooling in harmonic traps. EPL Europhys. Lett. 2011, 96, 60015. [Google Scholar] [CrossRef]
- Del Campo, A.; Goold, J.; Paternostro, M. More bang for your buck: Super-adiabatic quantum engines. Sci. Rep. 2014, 4, 6208. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rezek, Y.; Kosloff, R. Irreversible performance of a quantum harmonic heat engine. New J. Phys. 2006, 8, 83. [Google Scholar] [CrossRef]
- Ma, Y.H.; Xu, D.; Dong, H.; Sun, C.P. Universal constraint for efficiency and power of a low-dissipation heat engine. Phys. Rev. E 2018, 98, 042112. [Google Scholar] [CrossRef] [Green Version]
- Ryabov, A.; Holubec, V. Maximum efficiency of steady-state heat engines at arbitrary power. Phys. Rev. E 2016, 93, 050101. [Google Scholar] [CrossRef] [Green Version]
- Solfanelli, A.; Falsetti, M.; Campisi, M. Nonadiabatic single-qubit quantum Otto engine. Phys. Rev. B 2020, 101, 054513. [Google Scholar] [CrossRef] [Green Version]
- Alecce, A.; Galve, F.; Gullo, N.L.; Dell’Anna, L.; Plastina, F.; Zambrini, R. Quantum Otto cycle with inner friction: Finite-time and disorder effects. New J. Phys. 2015, 17, 075007. [Google Scholar] [CrossRef]
- Ma, Y.H.; Xu, D.; Dong, H.; Sun, C.P. Optimal operating protocol to achieve efficiency at maximum power of heat engines. Phys. Rev. E 2018, 98, 022133. [Google Scholar] [CrossRef] [Green Version]
- Lostaglio, M. Certifying quantum signatures in thermodynamics and metrology via contextuality of quantum linear response. arXiv 2020, arXiv:2004.01213. [Google Scholar]
- Diosi, L. Weak measurements in quantum mechanics. arXiv 2005, arXiv:quant-ph/0505075. [Google Scholar]
- Facchi, P.; Gorini, V.; Marmo, G.; Pascazio, S.; Sudarshan, E. Quantum zeno dynamics. Phys. Lett. A 2000, 275, 12–19. [Google Scholar] [CrossRef] [Green Version]
- Uzdin, R.; Gasparinetti, S.; Ozeri, R.; Kosloff, R. Markovian heat sources with the smallest heat capacity. New J. Phys. 2018, 20, 063030. [Google Scholar] [CrossRef] [Green Version]
- Feldmann, T.; Kosloff, R. Quantum lubrication: Suppression of friction in a first-principles four-stroke heat engine. Phys. Rev. E 2006, 73, 025107. [Google Scholar] [CrossRef] [Green Version]
- Elouard, C.; Herrera-Martí, D.A.; Clusel, M.; Auffèves, A. The role of quantum measurement in stochastic thermodynamics. Npj Quantum Inf. 2017, 3, 1–10. [Google Scholar] [CrossRef]
- Elouard, C.; Jordan, A.N. Efficient quantum measurement engines. Phys. Rev. Lett. 2018, 120, 260601. [Google Scholar] [CrossRef] [Green Version]
- Guryanova, Y.; Friis, N.; Huber, M. Ideal projective measurements have infinite resource costs. Quantum 2020, 4, 222. [Google Scholar] [CrossRef] [Green Version]
- Zhang, T.; Liu, W.T.; Chen, P.X.; Li, C.Z. Four-level entangled quantum heat engines. Phys. Rev. A 2007, 75, 062102. [Google Scholar] [CrossRef]
- Wang, H.; Liu, S.; He, J. Thermal entanglement in two-atom cavity QED and the entangled quantum Otto engine. Phys. Rev. E 2009, 79, 041113. [Google Scholar] [CrossRef]
- He, J.-Z.; He, X.; Zheng, J. Entangled quantum heat engine based on two-qubit Heisenberg XY model. Chin. Phys. B 2012, 21, 050303. [Google Scholar] [CrossRef]
- Halpern, N.Y.; White, C.D.; Gopalakrishnan, S.; Refael, G. Quantum engine based on many-body localization. Phys. Rev. B 2019, 99, 024203. [Google Scholar] [CrossRef] [Green Version]
- Hardal, A.Ü.; Müstecaplıoğlu, Ö.E. Superradiant quantum heat engine. Sci. Rep. 2015, 5, 12953. [Google Scholar] [CrossRef] [Green Version]
- Campisi, M.; Fazio, R. The power of a critical heat engine. Nat. Commun. 2016, 7, 11895. [Google Scholar] [CrossRef]
- Niedenzu, W.; Kurizki, G. Cooperative many-body enhancement of quantum thermal machine power. New J. Phys. 2018, 20, 113038. [Google Scholar] [CrossRef] [Green Version]
- Mukherjee, V.; Divakaran, U.; del Campo, A. Universal finite-time thermodynamics of many-body quantum machines from Kibble-Zurek scaling. arXiv 2020, arXiv:2003.06607. [Google Scholar]
- Jaramillo, J.; Beau, M.; del Campo, A. Quantum supremacy of many-particle thermal machines. New J. Phys. 2016, 18, 075019. [Google Scholar] [CrossRef]
- Jaseem, N.; Hajdusek, M.; Vedral, V.; Fazio, R.; Kwek, L.C.; Vinjanampathy, S. Quantum synchronization in nanoscale heat engines. Phys. Rev. E 2020, 101, 020201. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Palao, J.P.; Alonso, D. Internal dissipation and heat leaks in quantum thermodynamic cycles. Phys. Rev. E 2015, 92, 032136. [Google Scholar] [CrossRef] [Green Version]
- Newman, D.; Mintert, F.; Nazir, A. Performance of a quantum heat engine at strong reservoir coupling. Phys. Rev. E 2017, 95, 032139. [Google Scholar] [CrossRef] [Green Version]
- Perarnau-Llobet, M.; Wilming, H.; Riera, A.; Gallego, R.; Eisert, J. Strong coupling corrections in quantum thermodynamics. Phys. Rev. Lett. 2018, 120, 120602. [Google Scholar] [CrossRef] [Green Version]
- Shiraishi, N.; Saito, K.; Tasaki, H. Universal trade-off relation between power and efficiency for heat engines. Phys. Rev. Lett. 2016, 117, 190601. [Google Scholar] [CrossRef]
- Pietzonka, P.; Seifert, U. Universal trade-off between power, efficiency, and constancy in steady-state heat engines. Phys. Rev. Lett. 2018, 120, 190602. [Google Scholar] [CrossRef] [Green Version]
- Funo, K.; Ueda, M.; Sagawa, T. Thermodynamics in the Quantum Regime; Binder, F., Correa, L.A., Gogolin, C., Anders, J., Adesso, G., Eds.; Fundamental Theories of Physics; Springer: Berlin/Heidelberg, Germany, 2018; pp. 249–273. [Google Scholar] [CrossRef] [Green Version]
- Denzler, T.; Lutz, E. Power fluctuations in a finite-time quantum Carnot engine. arXiv 2020, arXiv:2007.01034. [Google Scholar]
- Silaev, M.; Heikkilä, T.T.; Virtanen, P. Lindblad-equation approach for the full counting statistics of work and heat in driven quantum systems. Phys. Rev. E 2014, 90, 022103. [Google Scholar] [CrossRef] [Green Version]
- Tsirlin, A.; Sukin, I. Averaged Optimization and Finite-Time Thermodynamics. Entropy 2020, 22, 912. [Google Scholar] [CrossRef]
- Tondeur, D.; Kvaalen, E. Equipartition of entropy production. An optimality criterion for transfer and separation processes. Ind. Eng. Chem. Res. 1987, 26, 50–56. [Google Scholar] [CrossRef]
- Korzekwa, K.; Lostaglio, M.; Oppenheim, J.; Jennings, D. The extraction of work from quantum coherence. New J. Phys. 2016, 18, 023045. [Google Scholar] [CrossRef]
- Camati, P.A.; Santos, J.F.; Serra, R.M. Coherence effects in the performance of the quantum Otto heat engine. Phys. Rev. A 2019, 99, 062103. [Google Scholar] [CrossRef] [Green Version]
- Geva, E.; Kosloff, R. On the classical limit of quantum thermodynamics in finite time. J. Chem. Phys. 1992, 97, 4398–4412. [Google Scholar] [CrossRef] [Green Version]
- Abah, O.; Rossnagel, J.; Jacob, G.; Deffner, S.; Schmidt-Kaler, F.; Singer, K.; Lutz, E. Single-ion heat engine at maximum power. Phys. Rev. Lett. 2012, 109, 203006. [Google Scholar] [CrossRef]
- Roßnagel, J.; Dawkins, S.T.; Tolazzi, K.N.; Abah, O.; Lutz, E.; Schmidt-Kaler, F.; Singer, K. A single-atom heat engine. Science 2016, 352, 325–329. [Google Scholar] [CrossRef] [Green Version]
- Abah, O.; Paternostro, M. Shortcut-to-adiabaticity Otto engine: A twist to finite-time thermodynamics. Phys. Rev. E 2019, 99, 022110. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Insinga, A.; Andresen, B.; Salamon, P. Thermodynamical analysis of a quantum heat engine based on harmonic oscillators. Phys. Rev. E 2016, 94, 012119. [Google Scholar] [CrossRef] [Green Version]
- Insinga, A.; Andresen, B.; Salamon, P.; Kosloff, R. Quantum heat engines: Limit cycles and exceptional points. Phys. Rev. E 2018, 97, 062153. [Google Scholar] [CrossRef] [Green Version]
- Deffner, S. Efficiency of harmonic quantum Otto engines at maximal power. Entropy 2018, 20, 875. [Google Scholar] [CrossRef] [Green Version]
- Salamon, P.; Berry, R.S. Thermodynamic length and dissipated availability. Phys. Rev. Lett. 1983, 51, 1127. [Google Scholar] [CrossRef]
- Peterson, J.P.; Batalhão, T.B.; Herrera, M.; Souza, A.M.; Sarthour, R.S.; Oliveira, I.S.; Serra, R.M. Experimental characterization of a spin quantum heat engine. arXiv 2018, arXiv:1803.06021. [Google Scholar] [CrossRef] [Green Version]
- Pekola, J.P.; Khaymovich, I.M. Thermodynamics in single-electron circuits and superconducting qubits. Annu. Rev. Condens. Matter Phys. 2019, 10, 193–212. [Google Scholar] [CrossRef]
- Klatzow, J.; Becker, J.N.; Ledingham, P.M.; Weinzetl, C.; Kaczmarek, K.T.; Saunders, D.J.; Nunn, J.; Walmsley, I.A.; Uzdin, R.; Poem, E. Experimental demonstration of quantum effects in the operation of microscopic heat engines. Phys. Rev. Lett. 2019, 122, 110601. [Google Scholar] [CrossRef] [Green Version]
- Hofer, P.P.; Brask, J.B.; Perarnau-Llobet, M.; Brunner, N. Quantum thermal machine as a thermometer. Phys. Rev. Lett. 2017, 119, 090603. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharjee, S.; Bhattacharya, U.; Niedenzu, W.; Mukherjee, V.; Dutta, A. Quantum magnetometry using two-stroke thermal machines. New J. Phys. 2020, 22, 013024. [Google Scholar] [CrossRef]
- Bhattacharjee, S.; Dutta, A. Quantum thermal machines and batteries. arXiv 2020, arXiv:2008.07889. [Google Scholar]
- Levy, A.; Göb, M.; Deng, B.; Singer, K.; Torrontegui, E.; Wang, D. Single-atom heat engine as a sensitive thermal probe. New J. Phys. 2020, 22, 093020. [Google Scholar] [CrossRef]
- Ronzani, A.; Karimi, B.; Senior, J.; Chang, Y.C.; Peltonen, J.T.; Chen, C.; Pekola, J.P. Tunable photonic heat transport in a quantum heat valve. Nat. Phys. 2018, 14, 991–995. [Google Scholar] [CrossRef] [Green Version]
- Wei, J.; Norman, E. On global representations of the solutions of linear differential equations as a product of exponentials. Proc. Am. Math. Soc. 1964, 15, 327–334. [Google Scholar] [CrossRef]

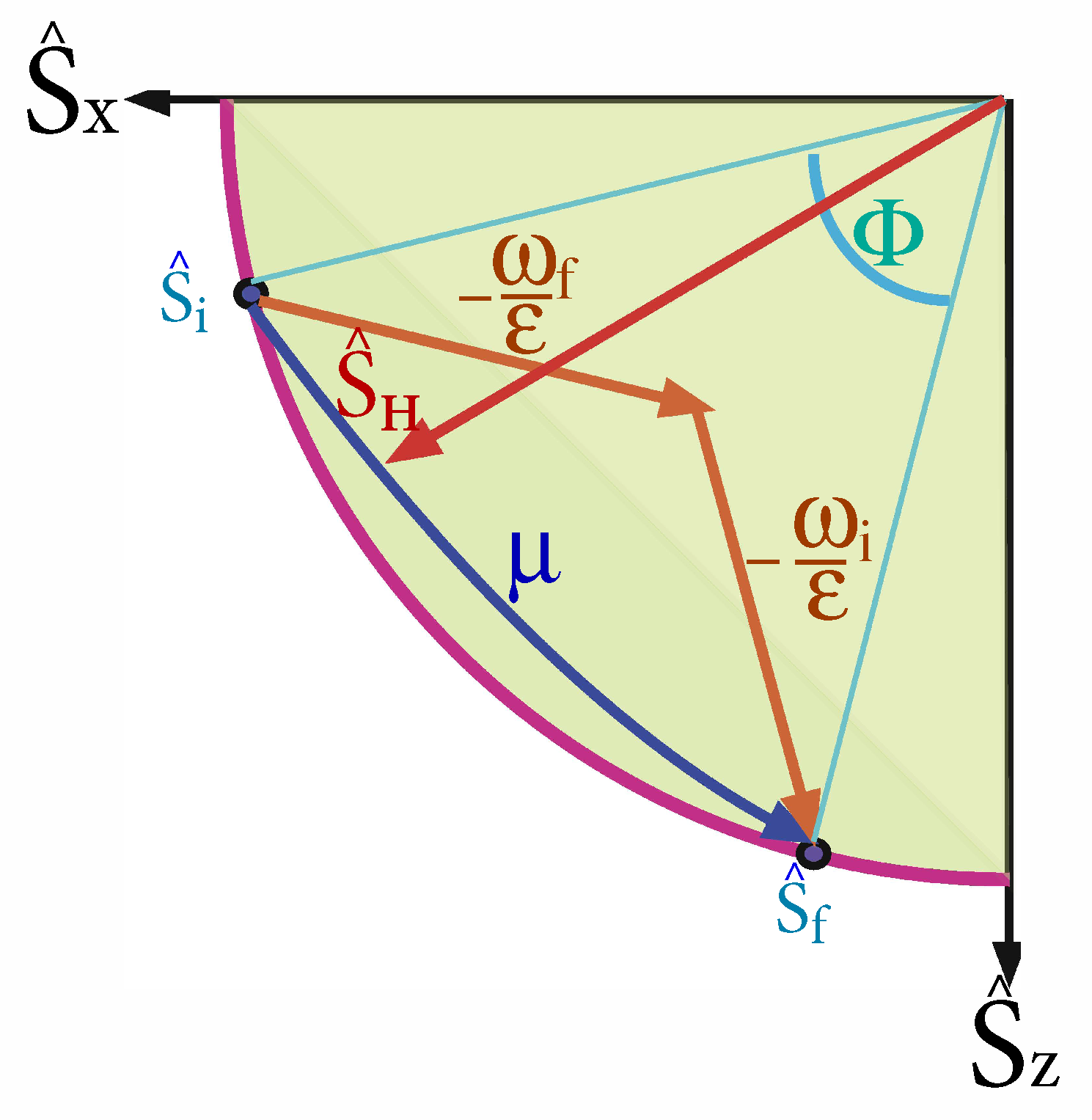
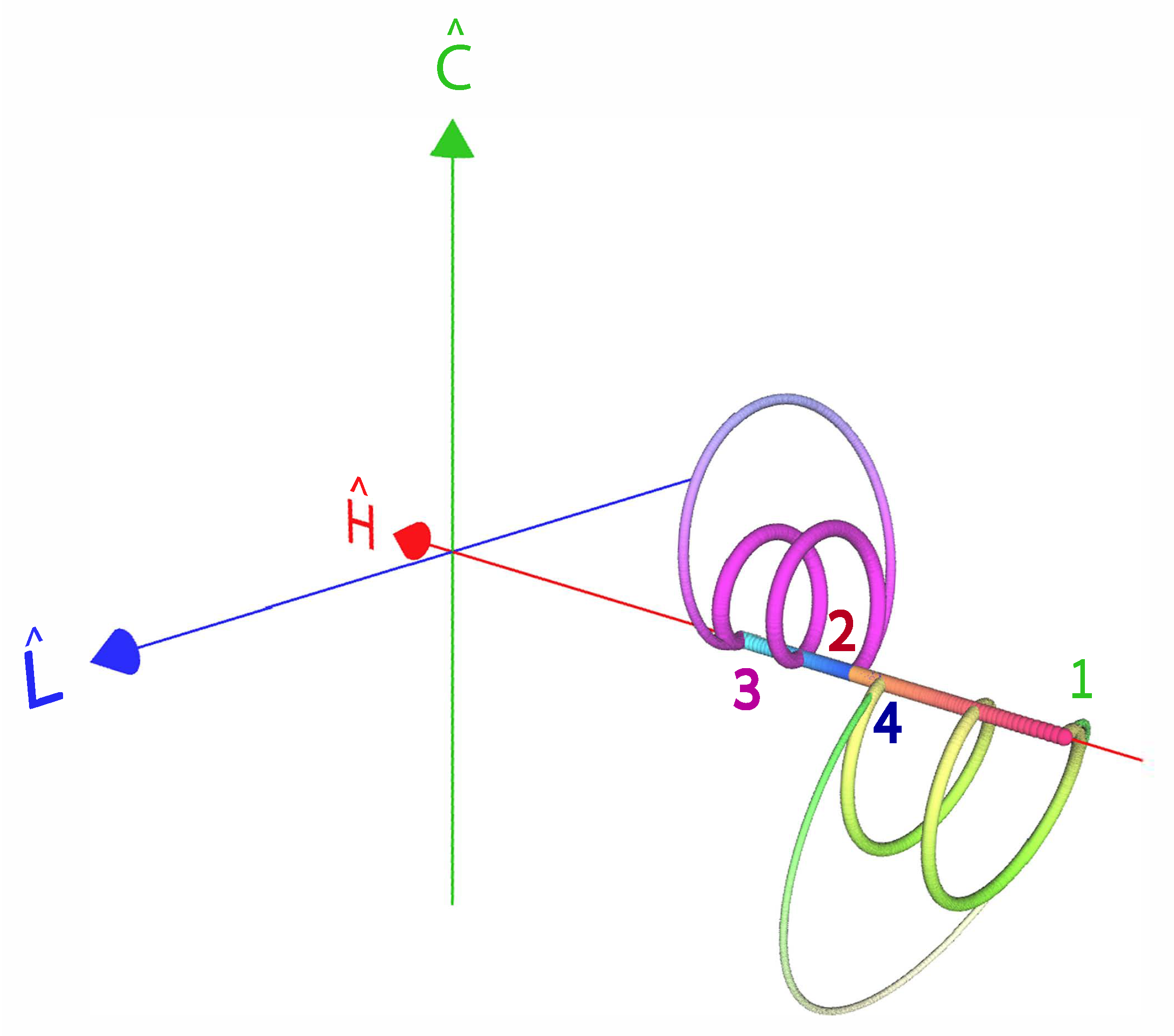




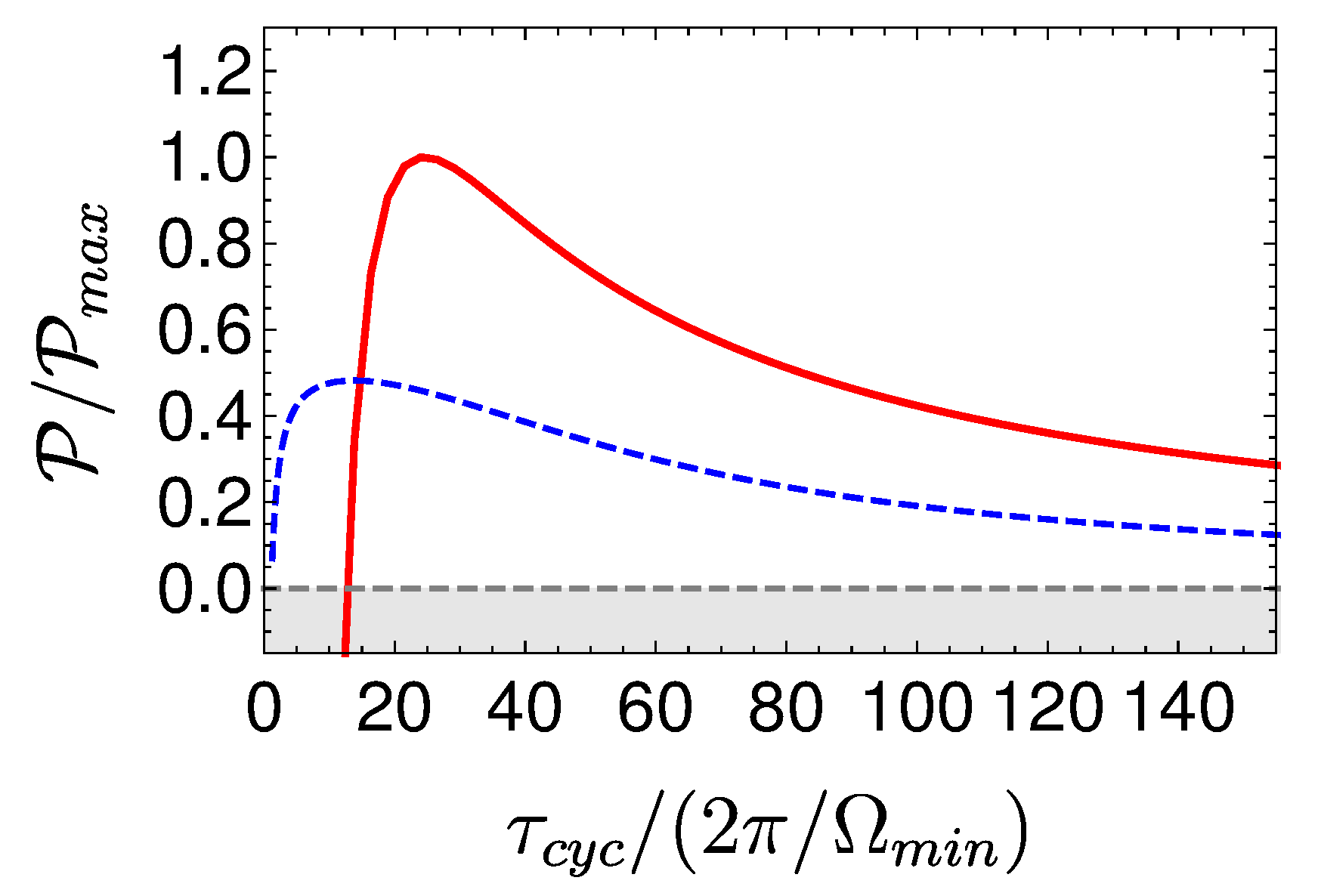
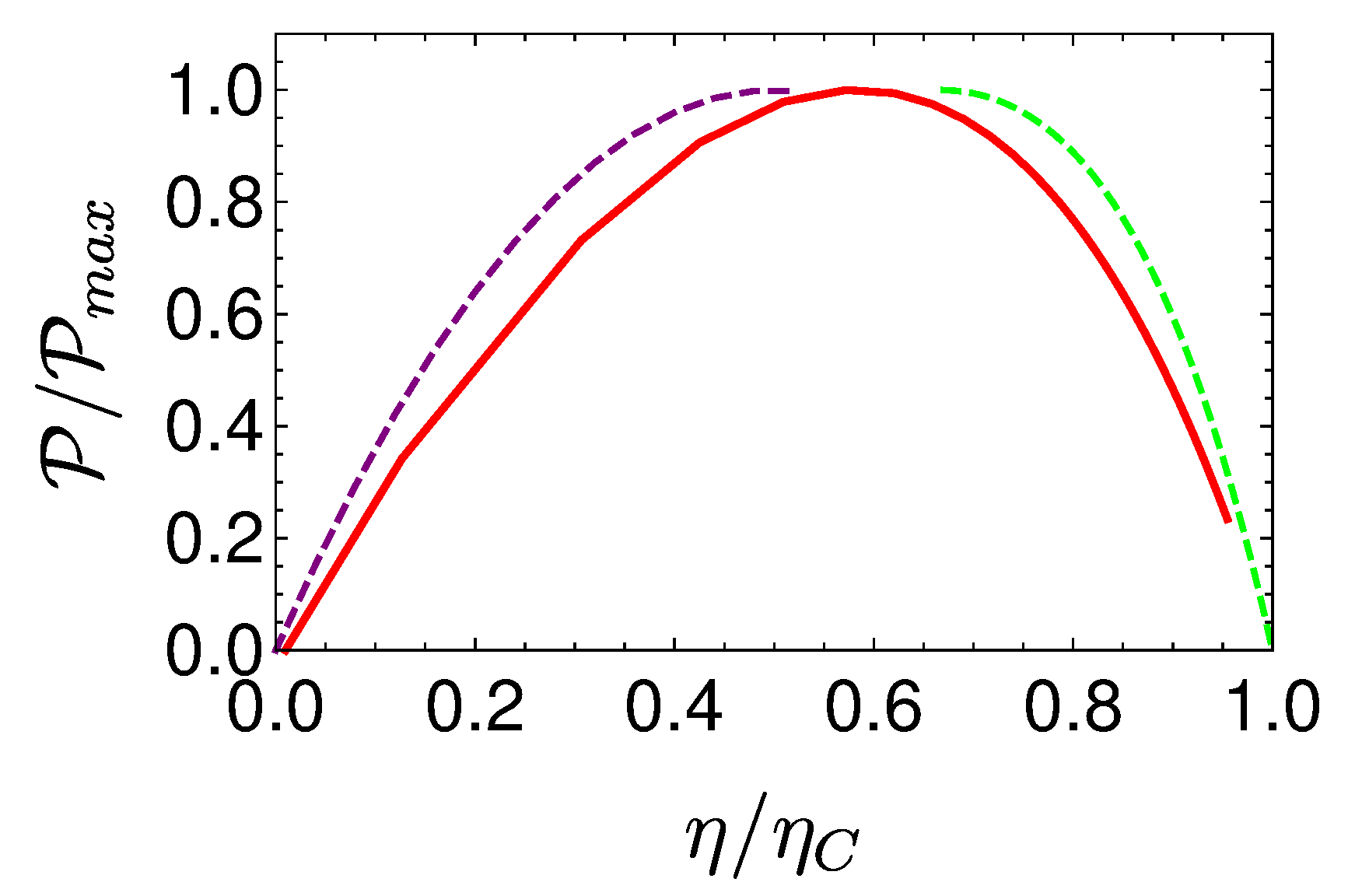

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dann, R.; Kosloff, R.; Salamon, P. Quantum Finite-Time Thermodynamics: Insight from a Single Qubit Engine. Entropy 2020, 22, 1255. https://doi.org/10.3390/e22111255
Dann R, Kosloff R, Salamon P. Quantum Finite-Time Thermodynamics: Insight from a Single Qubit Engine. Entropy. 2020; 22(11):1255. https://doi.org/10.3390/e22111255
Chicago/Turabian StyleDann, Roie, Ronnie Kosloff, and Peter Salamon. 2020. "Quantum Finite-Time Thermodynamics: Insight from a Single Qubit Engine" Entropy 22, no. 11: 1255. https://doi.org/10.3390/e22111255
APA StyleDann, R., Kosloff, R., & Salamon, P. (2020). Quantum Finite-Time Thermodynamics: Insight from a Single Qubit Engine. Entropy, 22(11), 1255. https://doi.org/10.3390/e22111255