A Discretization Approach for the Nonlinear Fractional Logistic Equation
Abstract
:1. Introduction
2. Fractional Calculus
- (1)
- (2)
- (3)
3. Discretized LDG Formulation
Algebraic Formulation
4. Numerical Stability and Error Estimates
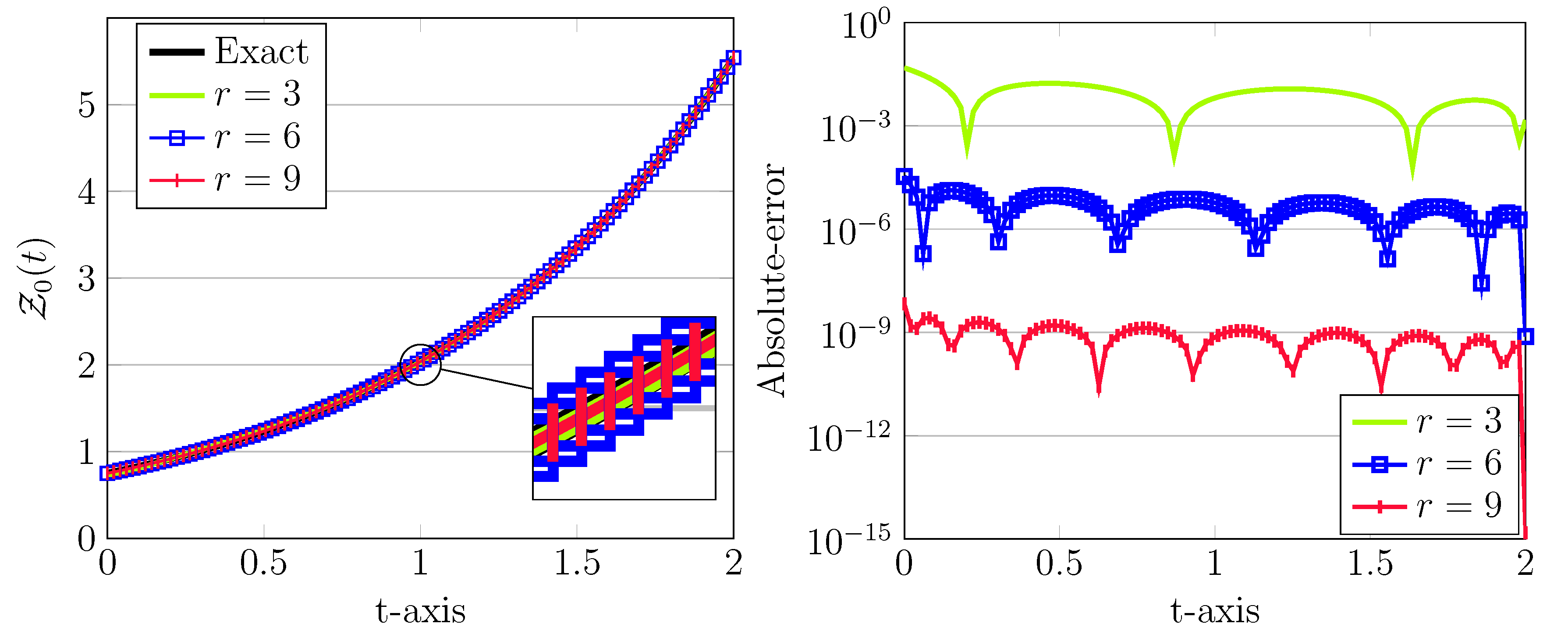
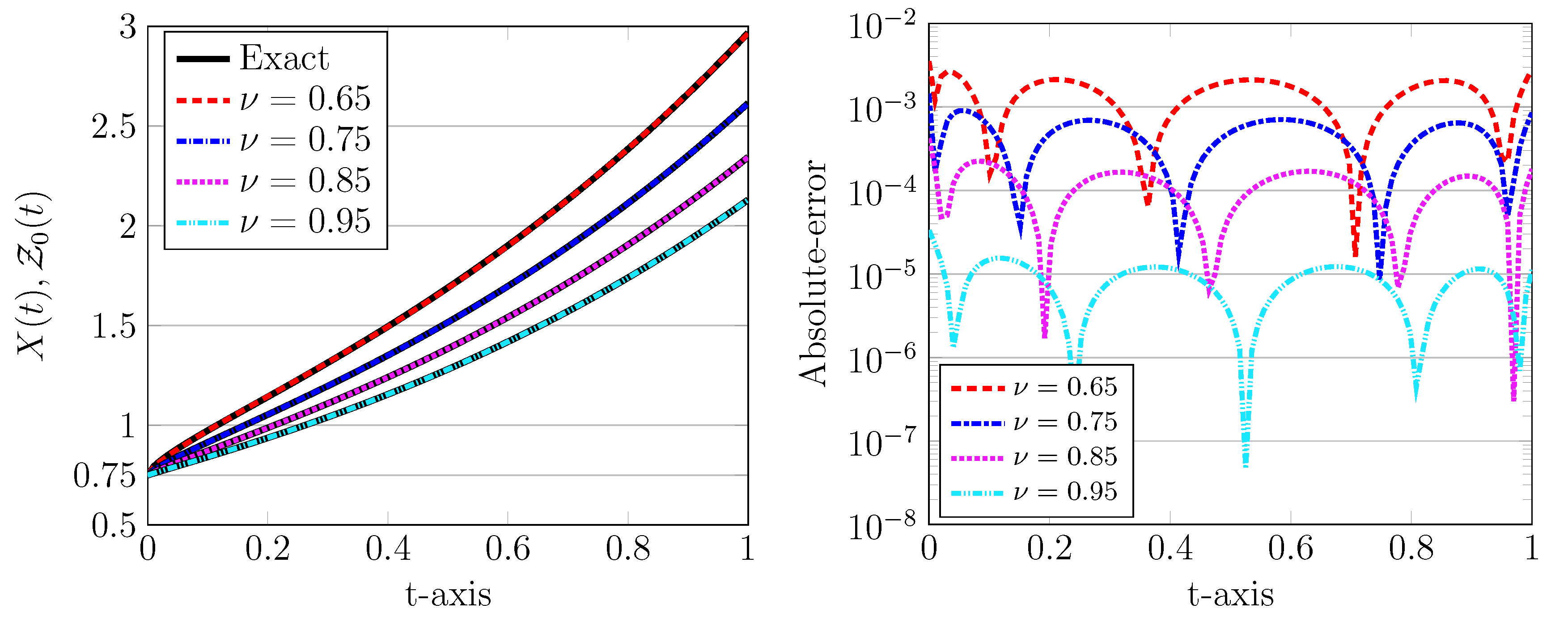
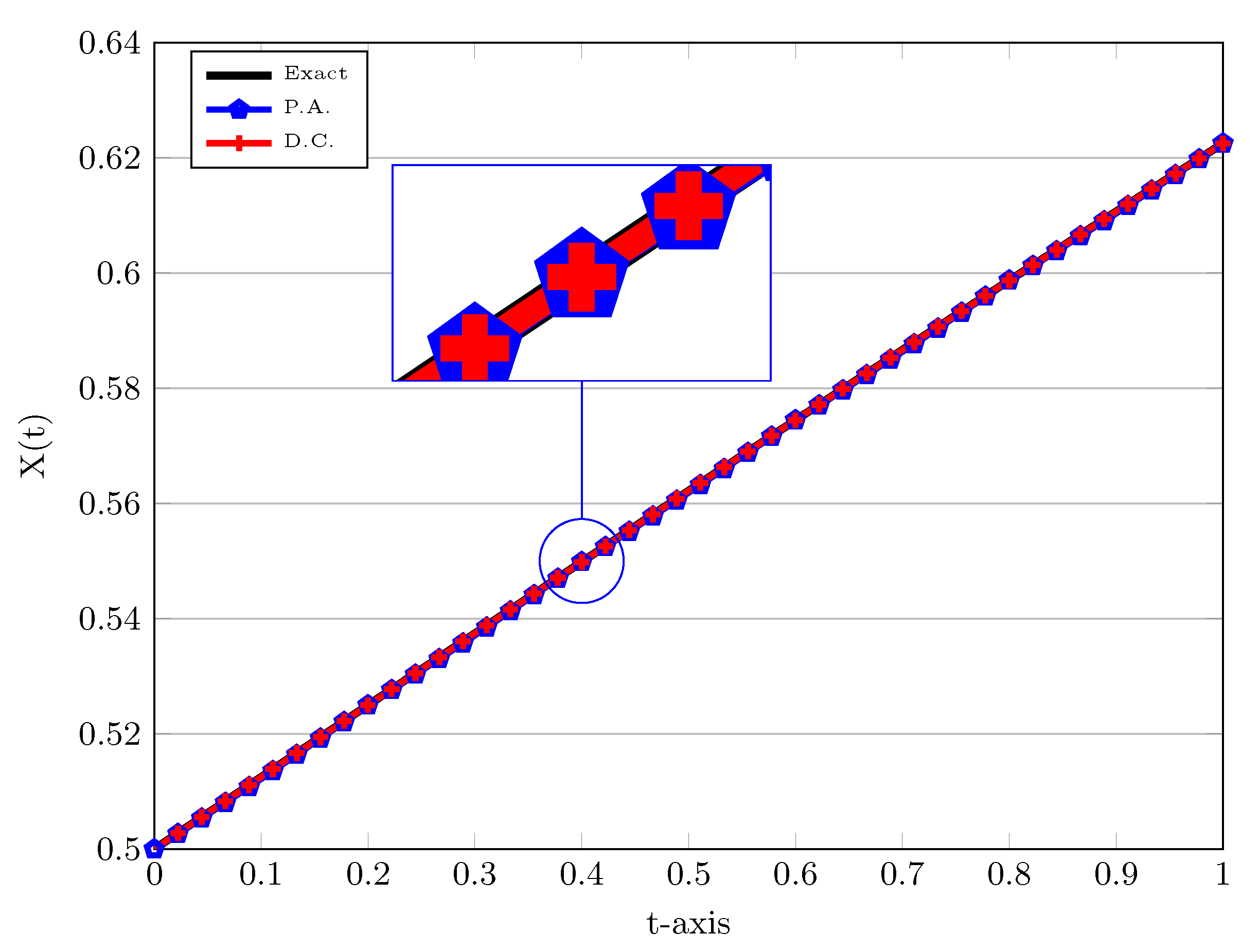
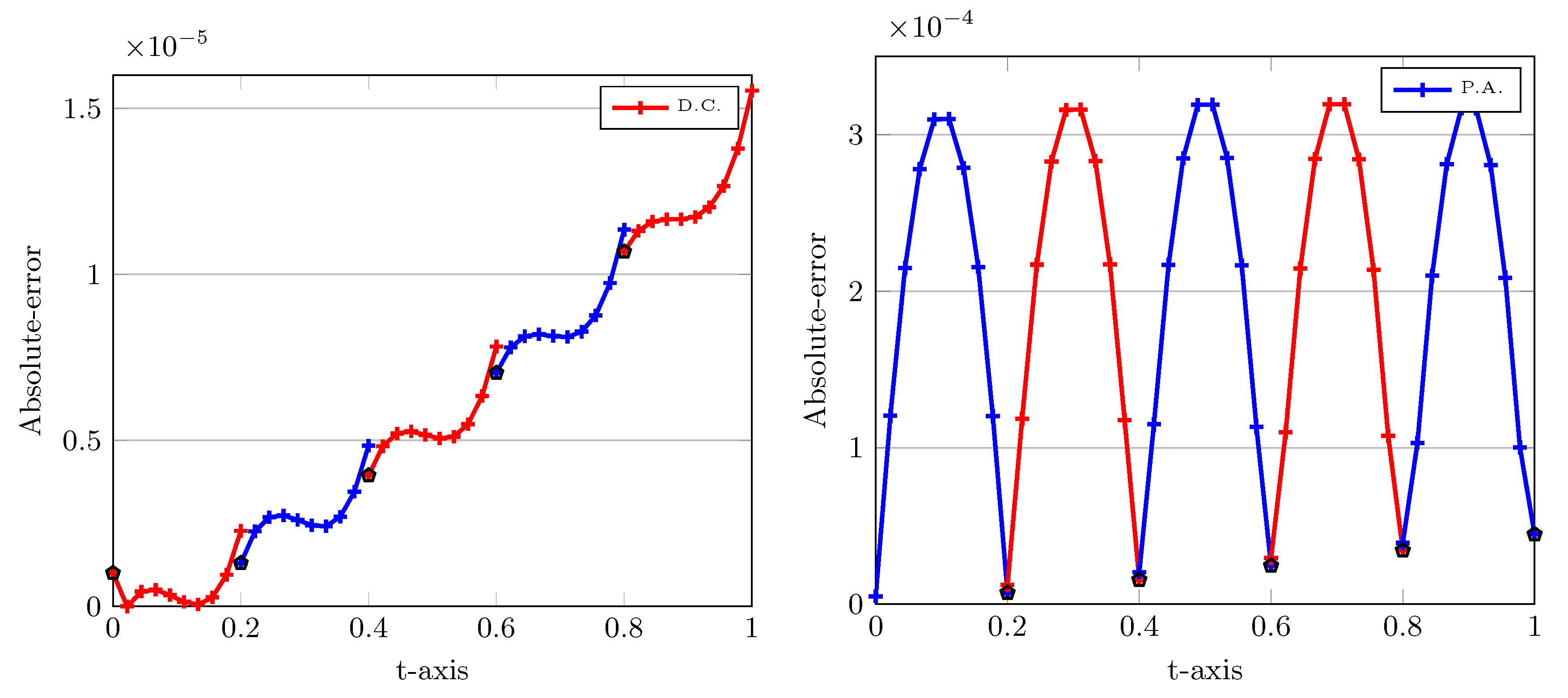
5. Numerical Results and Discussions
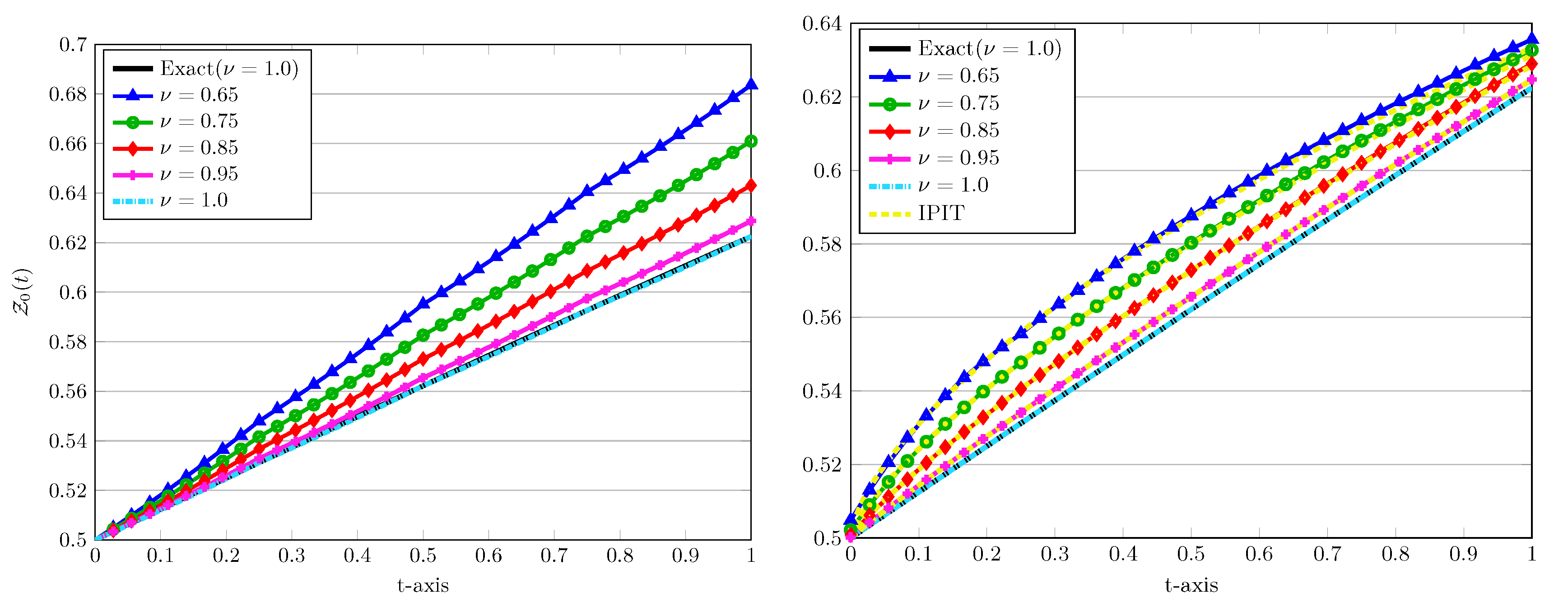
5.1. Linear Model
5.2. Nonlinear Model
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Malthus, T.R. Population: The First Essay (1798); University of Michigan Press: Ann Arbor, MI, USA, 1959. [Google Scholar]
- Verhulst, P.F. Notice sur la loi que la population sint dons son accroissement. Math. Phys. 1838, 10, 113–121. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, North-Holland Mathematical Studies; Elsevier (North-Holland) Science Publishers: Amsterdam, The Netherlands; London, UK; New York, NY, USA, 2006; Volume 204. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Foryś, U.; Marciniak-Czochra, A. Logistic equations in tumor growth modelling. Int. J. Appl. Math. Comput. Sci. 2003, 13, 317–325. [Google Scholar]
- Krishna, B.T. Binary phase coded sequence generation using fractional order logistic equation. Circuits Syst. Signal Process. 2012, 31, 401–411. [Google Scholar]
- Torresia, R.M.; de Torresib, S.I.C.; Gonzaleza, E.R. On the use of the quadratic logistic differential equation for the interpretation of electrointercalation processes. J. Electroanal. Chem. 1999, 461, 161–166. [Google Scholar]
- Pastijn, H. Chaotic growth with the logistic model of P.-F. Verhulst in The Logistic Map and the Route to Chaos. In Understanding Complex Systems; Springer: Berlin, Germany, 2006; pp. 3–11. [Google Scholar]
- El-Sayed, A.M.A.; El-Mesiry, A.E.M.; El-Saka, H.A.A. On the fractional-order logistic equation. Appl. Math. Lett. 2007, 20, 817–823. [Google Scholar]
- West, B.J. Exact solution to fractional logistic equation. Phys. A Stat. Mech. Its Appl. 2015, 429, 103–108. [Google Scholar]
- Area, I.; Losada, J.; Nieto, J.J. A note on the fractional logistic equation. Phys. A Stat. Mech. Its Appl. 2016, 444, 182–187. [Google Scholar]
- Ortigueira, M.; Bengochea, G. A new look at the fractionalization of the logistic equation. Phys. A Stat. Mech. Its Appl. 2017, 467, 554–561. [Google Scholar]
- D’Ovidio, M.; Loreti, P. Solutions of fractional logistic equations by Euler’s numbers. Phys. A Stat. Mech. Its Appl. 2018, 506, 1081–1092. [Google Scholar]
- Bhalekar, S.; Daftardar-Gejji, V. Solving fractional-order logistic equation using a new iterative method. Int. J. Differ. Equ. 2012, 2012, 975829. [Google Scholar]
- Garrappa, R. On linear stability of predictor-corrector algorithms for fractional differential equations. Int. J. Comput. Math. 2010, 87, 2281–2290. [Google Scholar]
- Khader, M.M. Numerical treatment for solving fractional logistic differential equation. Differ. Equ. Dyn. Syst. 2016, 24, 99–107. [Google Scholar]
- Izadi, M. A comparative study of two Legendre-collocation schemes applied to fractional logistic equation. Int. J. Appl. Comput. Math. 2020, 6, 71. [Google Scholar]
- Turalska, M.; West, B.J. A search for a spectral technique to solve nonlinear fractional differential equations. Chaos Solitons Fractals 2017, 102, 387–395. [Google Scholar]
- Yuzbasi, S. A collocation method for numerical solutions of fractional-order logistic population model. Int. J. Biomath. 2016, 9, 1650031–1650045. [Google Scholar]
- Khader, M.M.; Adel, M. Chebyshev wavelet procedure for solving FLDEs. Acta Appl. Math. 2018, 158, 1–10. [Google Scholar]
- Khader, M.M.; Babatin, M.M. On approximate solutions for fractional logistic differential equation. Math. Probl. Eng. 2013, 2013, 391901. [Google Scholar]
- Pitolli, F.; Pezza, L. A Fractional Spline Collocation Method for the Fractional Order Logistic Equation. In Approximation Theory XV, San Antonio 2016; Proceedings in Mathematics & Statistics; Fasshauer, G., Schumaker, L., Eds.; Springer: Cham, Switzerland, 2017; Volume 201, pp. 307–318. [Google Scholar]
- Baleanu, D.; Shiri, B.; Srivastava, H.M.; Qurashi, M.A. A Chebyshev spectral method based on operational matrix for fractional differential equations involving non-singular Mittag-Leffler kernel. Adv. Differ. Equ. 2018, 2018, 353. [Google Scholar]
- Garrappa, R. Numerical solution of fractional differential equations: A survey and a software tutorial. Mathematics 2018, 6, 16. [Google Scholar]
- Izadi, M. Fractional polynomial approximations to the solution of fractional Riccati equation. Punjab Univ. J. Math. 2019, 51, 123–141. [Google Scholar]
- Abd-Elhameed, W.M.; Youssri, Y.H. A novel operational matrix of Caputo fractional derivatives of Fibonacci polynomials: Spectral solutions of fractional differential equations. Entropy 2016, 18, 345. [Google Scholar]
- Srivastava, H.M. Fractional-order derivatives and integrals: Introductory overview and recent developments. Kyungpook Math. J. 2020, 60, 73–116. [Google Scholar]
- Srivastava, H.M.; Saad, K.M.; Khader, M.M. An efficient spectral collocation method for the dynamic simulation of the fractional epidemiological model of the Ebola virus. Chaos Solitons Fractals 2020, 140, 110174. [Google Scholar]
- Singh, H.; Srivastava, H.M. Jacobi collocation method for the approximate solution of some fractional-order Riccati differential equations with variable coefficients. Phys. A Statist. Mech. Appl. 2019, 523, 1130–1149. [Google Scholar]
- Abd-Elhameed, W.M.; Youssri, Y.H. Explicit shifted second-kind Chebyshev spectral treatment for fractional Riccati differential equation. Comput. Model. Eng. Sci. 2019, 121, 1029–1049. [Google Scholar]
- Srivastava, H.M.; Dubey, V.P.; Kumar, R.; Singh, J.; Kumar, D.; Baleanu, D. An efficient computational approach for a fractional-order biological population model with carrying capacity. Chaos Solitons Fractals 2020, 138, 109880. [Google Scholar]
- Izadi, M. An accurate approximation method for solving fractional order boundary value problems. Acta Univ. M. Belii Ser. Math. 2020, 2020, 52–67. [Google Scholar]
- Izadi, M.; Cattani, C. Generalized Bessel polynomial for multi-order fractional differential equations. Symmetry 2020, 12, 1260. [Google Scholar]
- Srivastava, H.M.; Shah, F.A.; Irfan, M. Generalized wavelet quasi-linearization method for solving population growth model of fractional order. Math. Methods Appl. Sci. 2020, 43, 8753–8762. [Google Scholar]
- Reed, W.H.; Hill, T.R. Triangular Mesh Methods for the Neutron Transport Equation; Tech. Report LA-UR-73-479; Los Alamos Scientific Laboratory: Los Alamos, NM, USA, 1973. [Google Scholar]
- Delfour, M.; Hager, W.; Trochu, F. Discontinuous Galerkin methods for ordinary differential equations. Math. Comput. 1981, 36, 455–473. [Google Scholar]
- Cockburn, B.; Karniadakis, G.E.; Shu, C.W. (Eds.) Discontinuous Galerkin Methods: Theory, Computation and Applications, Lecture Notes in Computational Science and Engineering; Springer: Berlin, Germany, 2000; Volume 11. [Google Scholar]
- Deng, W.; Hesthaven, J.S. Local discontinuous Galerkin method for fractional ordinary differential equations. BIT Numer. Math. 2015, 55, 967–985. [Google Scholar]
- Izadi, M. Application of LDG scheme to solve semi-differential equations. J. Appl. Math. Comput. Mech. 2019, 18, 29–37. [Google Scholar]
- Izadi, M.; Negar, M.R. Local discontinuous Galerkin approximations to fractional Bagley-Torvik equation. Math. Meth. Appl. Sci. 2020, 43, 4798–4813. [Google Scholar]
- Izadi, M.; Afshar, M. Solving the Basset equation via Chebyshev collocation and LDG methods. J. Math. Model. 2020. [Google Scholar] [CrossRef]
- Christie, I.; Griffths, D.F.; Mitchell, A.R.; Sanz-Serna, J.M. Product approximations for nonlinear problems in finite element methods. IMA J. Numer. Anal. 1981, 1, 253–266. [Google Scholar]
- Diethelm, K.; Freed, A.D. The Frac PECE Subroutine for the Numerical Solution of Differential Equations of Fractional Order. In Forschung und Wissenschaftliches Rechnen 1998; Heinzel, S., Plesser, T., Eds.; Gessellschaft fur Wissenschaftliche Datenverarbeitung: Göttingen, Germany, 1999; pp. 57–71. [Google Scholar]
- Zhang, Y.; Sun, Z.; Liao, H. Finite difference methods for the time fractional diffusion equation on non-uniform meshes. J. Comput. Phys. 2014, 265, 195–210. [Google Scholar]
- Jiang, S.; Zhang, J.; Zhang, Q.; Zhang, Z. Fast evaluation of the Caputo fractional derivative and its applications to fractional diffusion equations. Commun. Comput. Phys. 2017, 21, 650–678. [Google Scholar]

| LDG | PECE | |||||
|---|---|---|---|---|---|---|
| EOC | Numerical | Error | EOC | |||
| 1 | 5.625000000000 | − | − | |||
| 2 | 5.543701171875 | |||||
| 4 | 5.229675292969 | |||||
| 8 | 5.446685392454 | |||||
| 16 | ||||||
| 32 | ||||||
| 64 | ||||||
| 128 | ||||||
| LDG | L1 | Fast L1 | Exact | LDG | L1 | Fast L1 | Exact | |
| 0.4 | ||||||||
| P.A. | D.C. | |||||
|---|---|---|---|---|---|---|
| EOC | EOC | |||||
| 1 | 0.6234038976 | − | − | |||
| 2 | 0.6226973939 | |||||
| 4 | ||||||
| P.A. | D.C. | |||||
|---|---|---|---|---|---|---|
| EOC | EOC | |||||
| 1 | 0.6233820141 | − | − | |||
| 2 | 0.6226943815 | |||||
| 4 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Izadi, M.; Srivastava, H.M. A Discretization Approach for the Nonlinear Fractional Logistic Equation. Entropy 2020, 22, 1328. https://doi.org/10.3390/e22111328
Izadi M, Srivastava HM. A Discretization Approach for the Nonlinear Fractional Logistic Equation. Entropy. 2020; 22(11):1328. https://doi.org/10.3390/e22111328
Chicago/Turabian StyleIzadi, Mohammad, and Hari M. Srivastava. 2020. "A Discretization Approach for the Nonlinear Fractional Logistic Equation" Entropy 22, no. 11: 1328. https://doi.org/10.3390/e22111328
APA StyleIzadi, M., & Srivastava, H. M. (2020). A Discretization Approach for the Nonlinear Fractional Logistic Equation. Entropy, 22(11), 1328. https://doi.org/10.3390/e22111328






