Unification of the Nature’s Complexities via a Matrix Permanent—Critical Phenomena, Fractals, Quantum Computing, ?P-Complexity
Abstract
:1. Introduction
2. The Matrix Permanent: Quantum Computing and ♯P-Complete Oracle
2.1. The Permanent’s Complexity of the Quantum Information Processing and Computing
2.2. The Permanent as the ♯P-Complete Oracle for the Universal Quantum Computing and the Toda’s Theorem
3. Reduction of the Critical Phenomena to Computing a Matrix Permanent
3.1. The Constrained Spin Bosons in the Holstein-Primakoff Representation
3.2. The Order Parameter and Correlation Functions via the True Probabilities of Spin-Boson Occupations
3.3. The Unconstrained Probabilities of Spin-Boson Occupations via the Unconstrained Correlation Matrix
3.4. The Partition Function and the True Probabilities of Spin-Boson Occupations
3.5. The Exact Solution for the Total Irreducible Self-Energy via the Unconstrained Correlation Matrix
3.6. The Exact Closed Self-Consistency Equation for the Unconstrained Correlation Matrix
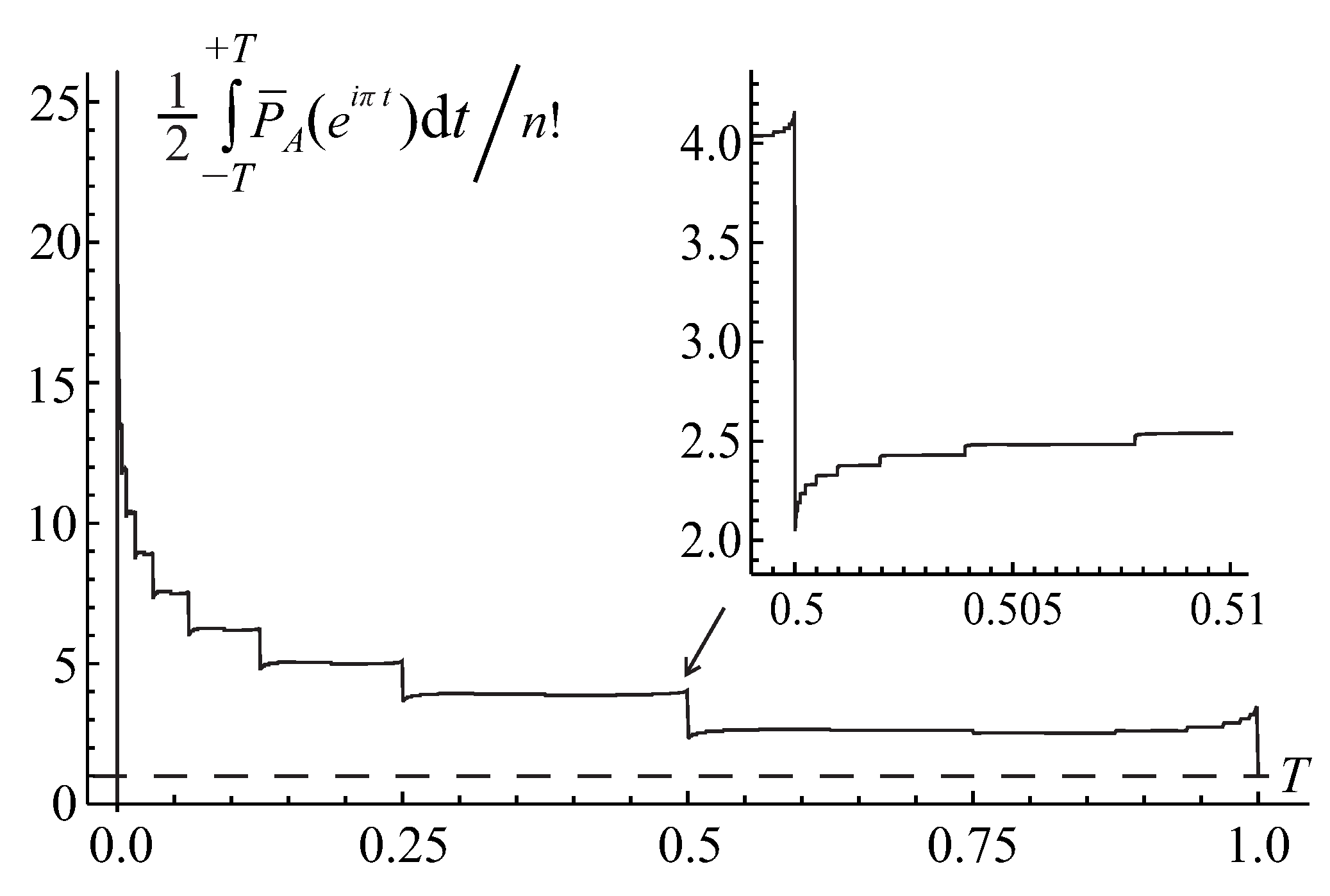
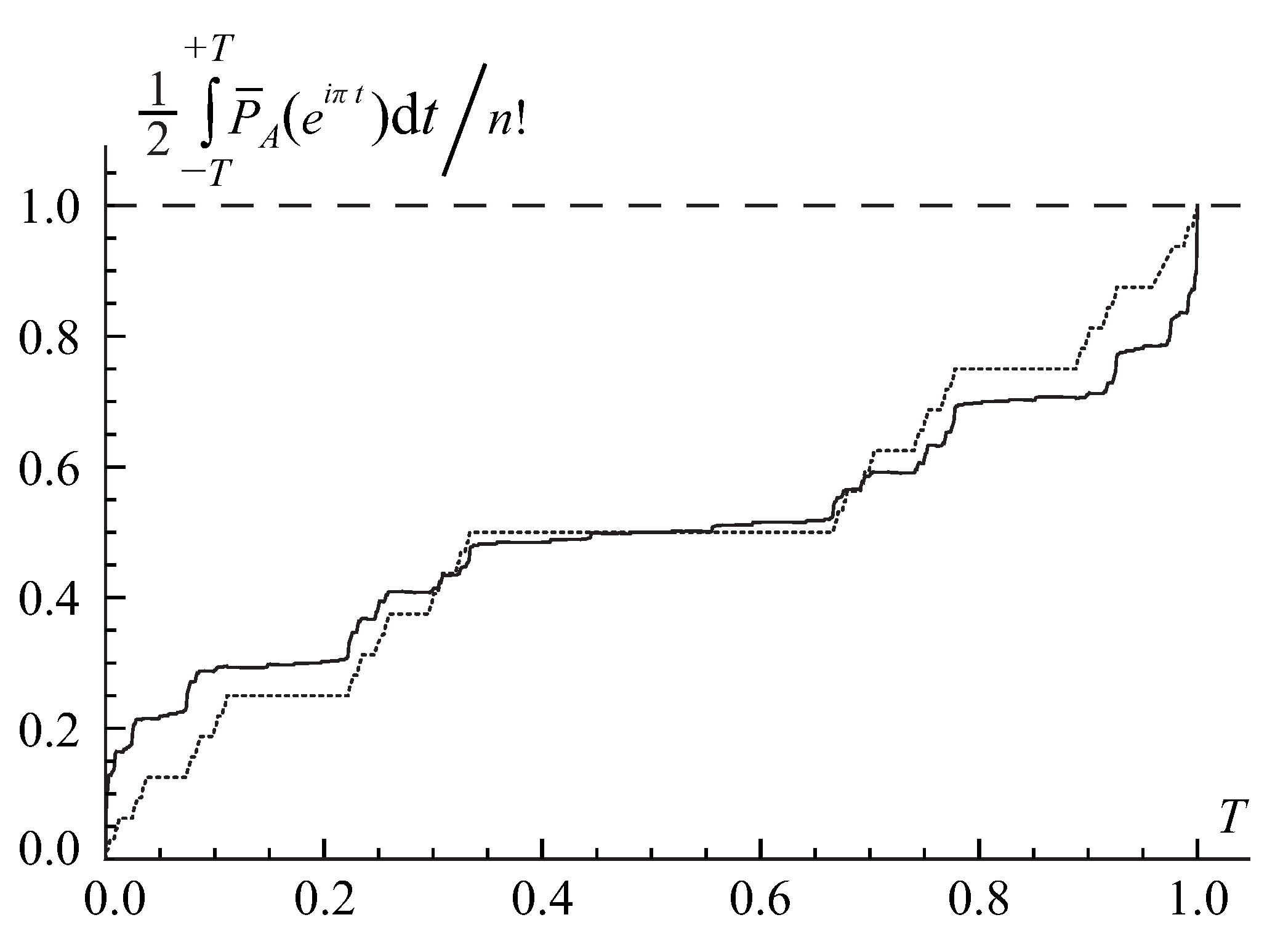
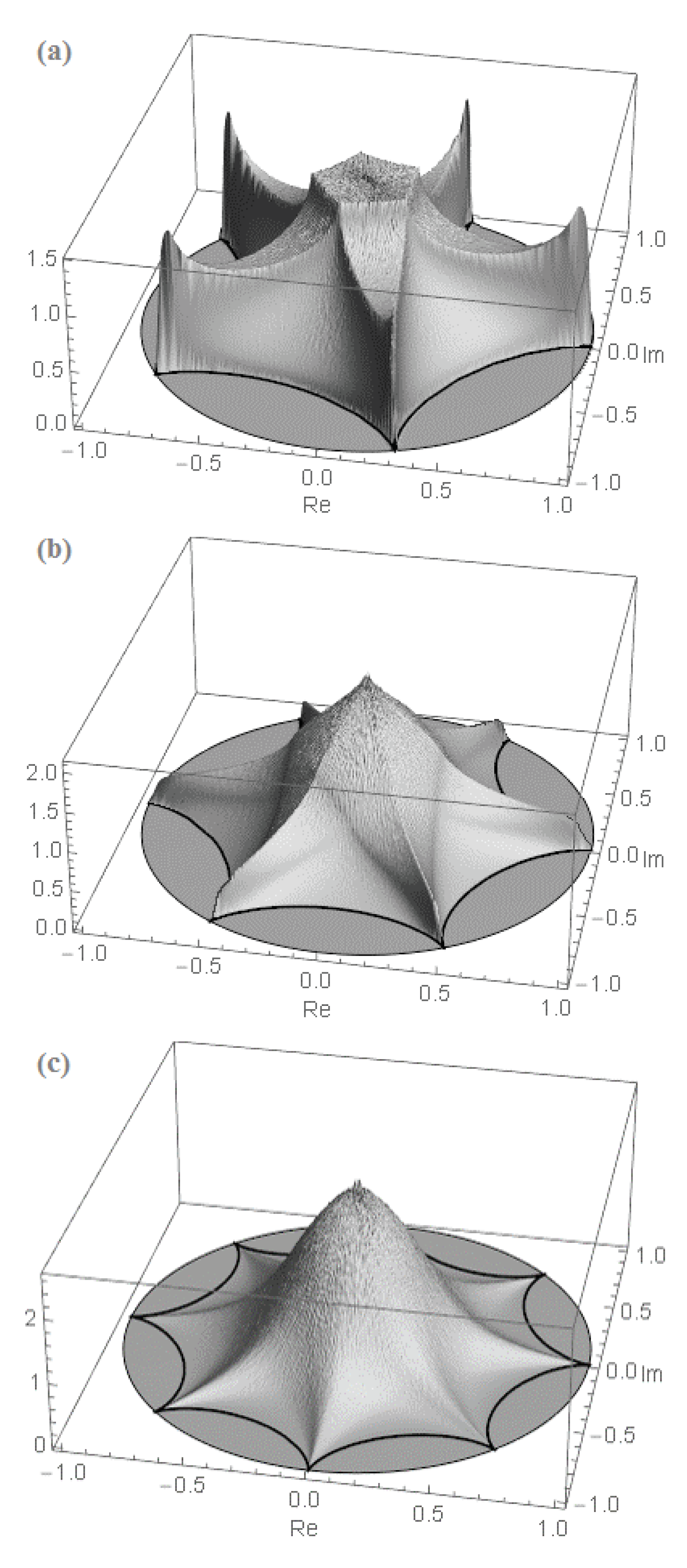
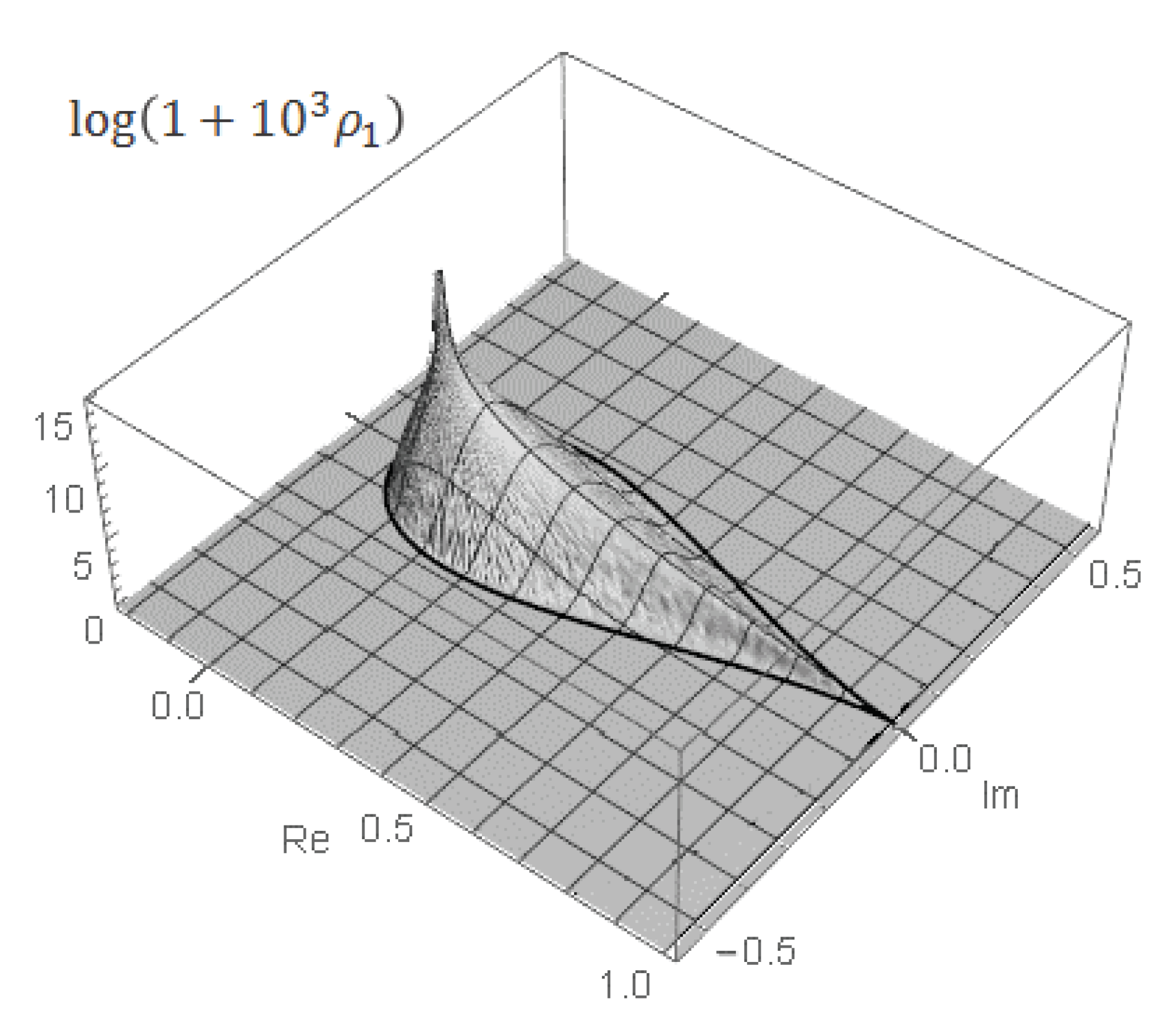
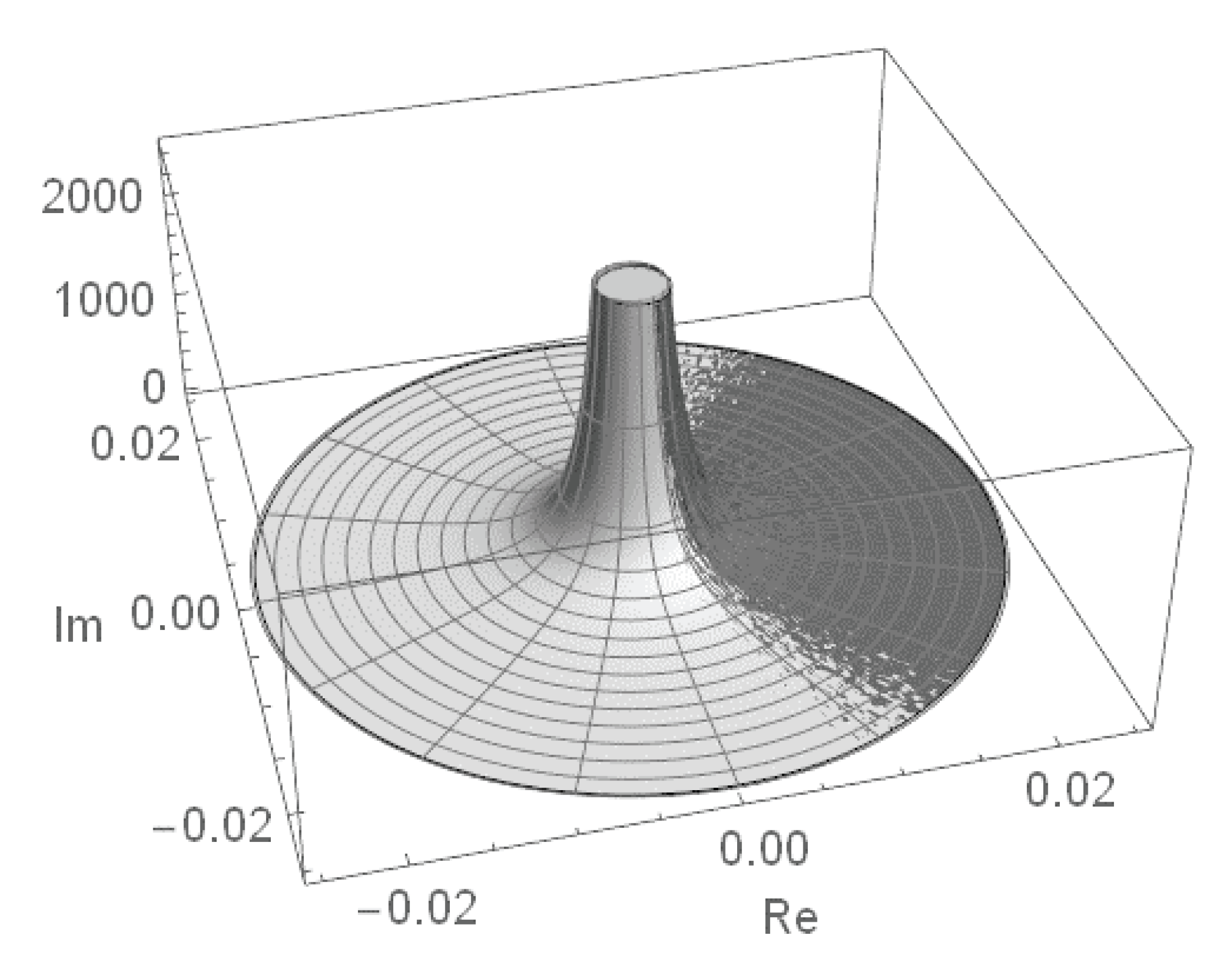
4. The Permanent and the Fractals
4.1. The 1d Integral Representation of the Permanent: A Fractal Integrand and a Weierstrass Function
4.2. A Fractal Nature of the Matrix Permanent
4.3. Permanent’s Fractal: The Case of the Integer Base
4.4. Permanent’s Fractal: The Case of the Non-Integer Base
5. Multivariate Representations of the Matrix Permanent
5.1. The Integral Representation of the Permanent via a Multivariate Polynomial of Complex Variables
5.2. Discrete Analogs of the Permanent’s Integral Representations: BBFG Formula & Its Generalization
5.3. Permanent vs. Determinant: The MacMahon Master Theorem
5.4. Permanent’s Fractal vs. Complex Stochastic Multivariate Polynomial
6. Manifestation of a Number-Theoretic Complexity in the Permanent of Schur/Fourier Matrices
6.1. Permanent’s Representation via Laplace Integrals
6.2. The Permanent of the Schur/Fourier and Circulant Matrices vs. the Number Theory
7. Asymptotics of the Permanent and the Szegő Limit Theorems
7.1. The Circulant Determinant vs. the Toeplitz Determinant
7.2. McCullagh Asymptotics of the Permanent and Two Opposite Limits for the Circulant Determinant
7.3. An Example of the Permanent’s Asymptotics: Circulant Matrix with Exponentially Varying Entries
7.4. The Permanent’s Asymptotics for the Circulant Matrices with the Power-Law Varying Entries
7.5. An Example of the Exact Analytic Solution for the Permanent of the Doubly Stochastic Circulant Matrix
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Minc, H. Permanents (Encyclopedia of Mathematics and Its Applications); Addison - Wesley: Reading, MA, USA, 1978; Volume 6. [Google Scholar]
- Minc, H. Theory of Permanents 1982–1985. Linear Multilinear Algebr. 1987, 21, 109–148. [Google Scholar] [CrossRef]
- Stanley, R.P. Enumerative Combinatorics; Cambridge University Press: Cambridge, MA, USA, 2012; Volume 1. [Google Scholar]
- Barvinok, A. Combinatorics and Complexity of Partition Functions. Algorithms and Combinatorics; Springer International Publishing: Berlin/Heidelberg, Germany, 2016; Volume 30. [Google Scholar]
- Scheel, S. Permanents in linear optical networks. arXiv 2004, arXiv:0406127v1. [Google Scholar]
- Aaronson, S.; Arkhipov, A. The computational complexity of linear optics. Theory Comput. 2013, 9, 143–252. [Google Scholar] [CrossRef]
- Kalai, G. The quantum computer puzzle (expanded version). arXiv 2016, arXiv:1605.00992v1. [Google Scholar]
- Wu, J.; Liu, Y.; Zhang, B.; Jin, X.; Wang, Y.; Wang, H.; Yang, X. Computing Permanents for Boson Sampling on Tianhe-2 Supercomputer. arXiv 2016, arXiv:1606.05836v1. [Google Scholar]
- Wang, H.; Qin, J.; Ding, X.; Chen, M.; Chen, S.; You, X.; He, Y.; Jiang, X.; You, L.; Wang, Z.; et al. Boson Sampling with 20 input photons and a 60-mode interferometer in a 1014-dimensional Hilbert space. Phys. Rev. Lett. 2019, 123, 250503. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Drummond, P.D.; Opanchuk, B.; Rosales-Zárate, L.; Reid, M.D.; Forrester, P.J. Scaling of boson sampling experiments. Phys. Rev. A 2016, 94, 042339. [Google Scholar] [CrossRef]
- Opanchuk, B.; Rosales-Zárate, L.; Reid, M.D.; Drummond, P.D. Simulating and assessing boson sampling experiments with phase-space representations. Phys. Rev. A 2018, 97, 042304. [Google Scholar] [CrossRef] [Green Version]
- Opanchuk, B.; Rosales-Zárate, L.; Reid, M.D.; Drummond, P.D. Robustness of quantum Fourier transform interferometry. Opt. Lett. 2019, 44, 343–346. [Google Scholar] [CrossRef]
- Shchesnovich, V.S. Partial distinguishability and photon counting probabilities in linear multiport devices. arXiv 2017, arXiv:1712.03191v2. [Google Scholar]
- Chin, S.; Huh, J. Generalized concurrence in boson sampling. Sci. Rep. 2018, 8, 6101. [Google Scholar] [CrossRef] [PubMed]
- Yung, M.-H.; Gao, X.; Huh, J. Universal bound on sampling bosons in linear optics and its computational implications. Natl. Sci. Rev. 2019, 6, 719–729. [Google Scholar] [CrossRef]
- Kim, Y.; Hong, K.-H.; Kim, Y.-H.; Huh, J. Connection between BosonSampling with quantum and classical input states. Opt. Express 2020, 28, 6929–6936. [Google Scholar] [CrossRef]
- Rudolph, T. Simple encoding of a quantum circuit amplitude as a matrix permanent. Phys. Rev. A 2009, 80, 054302. [Google Scholar] [CrossRef] [Green Version]
- Gurvits, L. Classical complexity and quantum entanglement. J. Comput. Syst. Sci. 2004, 69, 448–484. [Google Scholar] [CrossRef] [Green Version]
- Dolev, S.; Fandina, N.; Gutfreund, D. Succinct permanent is NEXP-hard with many hard instances. In Algorithms and Complexity: 8th International Conference, CIAC 2013, LNCS 7878; Spirakis, P.G., Serna, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2013; pp. 183–196. [Google Scholar]
- Valiant, L.G. The complexity of computing the permanent. Theor. Comput. Sci. 1979, 8, 189–201. [Google Scholar] [CrossRef] [Green Version]
- Aaronson, S. A linear-optical proof that the permanent is ♯P-hard. Proc. R. Soc. A 2011, 467, 3393–3405. [Google Scholar] [CrossRef] [Green Version]
- Ryser, H.J. Combinatorial Mathematics, The Carus Mathematical Monographs, No. 14; The Mathematical Association of America: Washington, DC, USA, 1963. [Google Scholar]
- Glynn, D.G. The permanent of a square matrix. Eur. J. Combinatorics 2010, 31, 1887–1891. [Google Scholar] [CrossRef] [Green Version]
- Goldreich, O. P, NP, and NP-Completeness: The Basics of Complexity Theory; Cambridge University Press: Cambridge, MA, USA, 2010. [Google Scholar]
- Bernstein, E.; Vazirani, U. Quantum complexity theory. SIAM J. Comput. 1997, 26, 1411–1473. [Google Scholar] [CrossRef]
- Toda, S. PP is as hard as the polynomial-time hierarchy. SIAM J. Comput. 1991, 20, 865–877. [Google Scholar] [CrossRef] [Green Version]
- Basu, S. A complex analog of Toda’s theorem. Found. Comput. Math. 2012, 12, 327–362. [Google Scholar] [CrossRef] [Green Version]
- Bernien, H.; Schwartz, S.; Keesling, A.; Levine, H.; Omran, A.; Pichler, H.; Choi, S.; Zibrov, A.S.; Endres, M.; Greiner, M.; et al. Probing many-body dynamics on a 51-atom quantum simulator. Nature 2017, 551, 579–584. [Google Scholar] [CrossRef] [PubMed]
- McMahon, P.L.; Marandi, A.; Haribara, Y.; Hamerly, R.; Langrock, C.; Tamate, S.; Inagaki, T.; Takesue, H.; Utsunomiya, S.; Aihara, K.; et al. A fully-programmable 100-spin coherent Ising machine with all-to-all connections. Science 2016, 354, 614–617. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pierangeli, D.; Marcucci, G.; Conti, C. Large-scale photonic Ising machine by spatial light modulation. Phys. Rev. Lett. 2019, 122, 213902. [Google Scholar] [CrossRef] [Green Version]
- Lucas, A. Ising formulations of many NP problems. Front. Phys. 2014, 2, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Kocharovsky, V.V.; Kocharovsky, V.V. Towards an exact solution for the three-dimensional Ising model: A method of the recurrence equations for partial contractions. Phys. Lett. A 2015, 379, 2520–2523. [Google Scholar] [CrossRef]
- Kocharovsky, V.V.; Kocharovsky, V.V. Exact general solution to the three-dimensional Ising model and a self-consistency equation for the nearest-neighbors’ correlations. arXiv 2016, arXiv:1510.07327v3. [Google Scholar]
- Caianiello, E.R. Combinatorics and Renormalization in Quantum Field Theory. Frontiers in Physics; W. A. Benjamin Inc.: Reading, PA, USA; London, UK; Amsterdam, The Netherlands, 1973. [Google Scholar]
- Huo, Y.; Liang, H.; Liu, S.-Q.; Bai, F. Computing the monomer-dimer systems through matrix permanent. arXiv 2007, arXiv:0708.1641v2. [Google Scholar] [CrossRef] [Green Version]
- Jerrum, M.; Sinclair, A. Approximating the permanent. SIAM J. Comput. 1989, 18, 1149–1174. [Google Scholar] [CrossRef]
- Jerrum, M.; Sinclair, A. Polynomial-time approximation algorithms for the Ising model. SIAM J. Comput. 1993, 22, 1087–1116. [Google Scholar] [CrossRef] [Green Version]
- Jerrum, M.; Sinclair, A.; Vigoda, E. A polynomial-time approximation algorithm for the permanent of a matrix with nonnegative entries. J. ACM 2004, 51, 671–697. [Google Scholar] [CrossRef]
- Goldberg, L.A.; Jerrum, M. A complexity classification of spin systems with an external field. Proc. Nat. Acad. Sci. USA 2015, 112, 13161–13166. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Huber, M.; Law, J. Simulation reduction of the Ising model to general matchings. Electron. J. Probab. 2012, 17, 1–15. [Google Scholar] [CrossRef]
- Kocharovsky, V.V.; Kocharovsky, V.V. Microscopic theory of a phase transition in a critical region: Bose–Einstein condensation in an interacting gas. Phys. Lett. A 2015, 379, 466–470. [Google Scholar] [CrossRef] [Green Version]
- Holstein, T.; Primakoff, H. Field dependence of the intrinsic domain magnetization of a ferromagnet. Phys. Rev. 1940, 58, 1098–1113. [Google Scholar] [CrossRef]
- Schwinger, J. Quantum Theory of Angular Momentum; Academic Press: New York, NY, USA, 1965. [Google Scholar]
- Kadanoff, L.P. Statistical Physics: Statics, Dynamics and Renormalization; World Scientific: Singapore, 2000. [Google Scholar]
- Dyson, F.J. General theory of spin-wave interactions. Phys. Rev. 1956, 102, 1217–1230. [Google Scholar] [CrossRef]
- Abrikosov, A.A.; Gorkov, L.P.; Dzyaloshinskii, I.E. Methods of Quantum Field Theory in Statistical Physics; Prentice-all: Englewood Cliffs, NJ, USA, 1963. [Google Scholar]
- Onsager, L. Crystal statistics. I. A two-dimensional model with an order-disorder transition. Phys. Rev. 1944, 65, 117–149. [Google Scholar] [CrossRef]
- Montroll, E.W.; Potts, R.B.; Ward, J.C. Correlations and spontaneous magnetization of the two-dimensional Ising model. J. Math. Phys. 1963, 4, 308–322. [Google Scholar] [CrossRef]
- Wu, T.T.; McCoy, B.M.; Tracy, C.A.; Barouch, E. Spin-spin correlation functions for the two-dimensional Ising model: Exact theory in the scaling region. Phys. Rev. B 1976, 13, 316–374. [Google Scholar] [CrossRef]
- Baxter, R.J. Exactly Solved Models in Statistical Mechanics; Academic Press: London, UK, 1989. [Google Scholar]
- Gray, R.M. Toeplitz and circulant matrices: A review. Found. Trends Commun. Inf. Theory 2006, 2, 155–239. [Google Scholar] [CrossRef]
- Deift, P.; Its, A.; Krasovsky, I. Toeplitz matrices and Toeplitz determinants under the impetus of the Ising model: Some history and some recent results. Commun. Pure Appl. Math. 2013, 66, 1360–1438. [Google Scholar] [CrossRef] [Green Version]
- Percus, J.K. Combinatorial Methods; Springer: New York, NY, USA; Heidelberg/Berlin, Germany, 1971. [Google Scholar]
- Louck, J.D. Unitary Symmetry and Combinatorics; World Scientific: Singapore, 2008. [Google Scholar]
- Baranski, K. Dimension of the Graphs of the Weierstrass-Type Functions. In Fractal Geometry and Stochastics V, Progress in Probability; Bandt, C., Falconer, K., Zahle, M., Eds.; Springer International Publishing: Cham, Switzerland, 2015; Volume 70, pp. 77–91. [Google Scholar]
- Falconer, K.J. Fractal Geometry. Mathematical Foundations and Applications, 2nd ed.; Wiley: Hoboken, NJ, USA, 2003. [Google Scholar]
- Balasubramanian, K. Combinatorics and Diagonals of Matrices. Ph.D. Thesis, Department of Statistics, Loyola College, Indian Statistical Institute, Calcutta, India, 1980. [Google Scholar]
- Bax, E.; Franklin, J. A finite-difference sieve to compute the permanent. In Caltech-CS-TR-96-04; California Institute of Technology: Pasadena, CA, USA, 1996. [Google Scholar]
- Kocharovsky, V.V.; Kocharovsky, V.V. On the permanents of circulant and degenerate Schur matrices. Linear Algebra Appl. 2017, 519, 366–381. [Google Scholar] [CrossRef]
- Ernst, T. A Comprehensive Treatment of Q-Calculus; Springer: Basel, Swizerland, 2012. [Google Scholar]
- Exton, H. Q-Hypergeometric Functions and Applications; Halsted Press: New York, NY, USA, 1983. [Google Scholar]
- Graham, R.L.; Lehmer, D.H. On the permanent of Schur’s matrix. J. Austral. Math. Soc. A 1976, 21, 487–497. [Google Scholar] [CrossRef] [Green Version]
- Thomas, H. The number of terms in the permanent and the determinant of a generic circulant matrix. J. Algebr. Comb. 2004, 20, 55–60. [Google Scholar] [CrossRef] [Green Version]
- McCullagh, P. An asymptotic approximation for the permanent of a doubly stochastic matrix. J. Stat. Comput. Simul. 2014, 84, 404–414. [Google Scholar] [CrossRef] [Green Version]
- Böttcher, A.; Silbermann, B. Analysis of Toeplitz Operators; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Garey, M.R.; Johnson, D.S. Computers and Intractability: A Guide to the Theory of NP-Completeness. A Series of Books in the Mathematical Sciences; Klee, V., Ed.; W. H. Freeman and Co.: San Francisco, CA, USA, 1979. [Google Scholar]

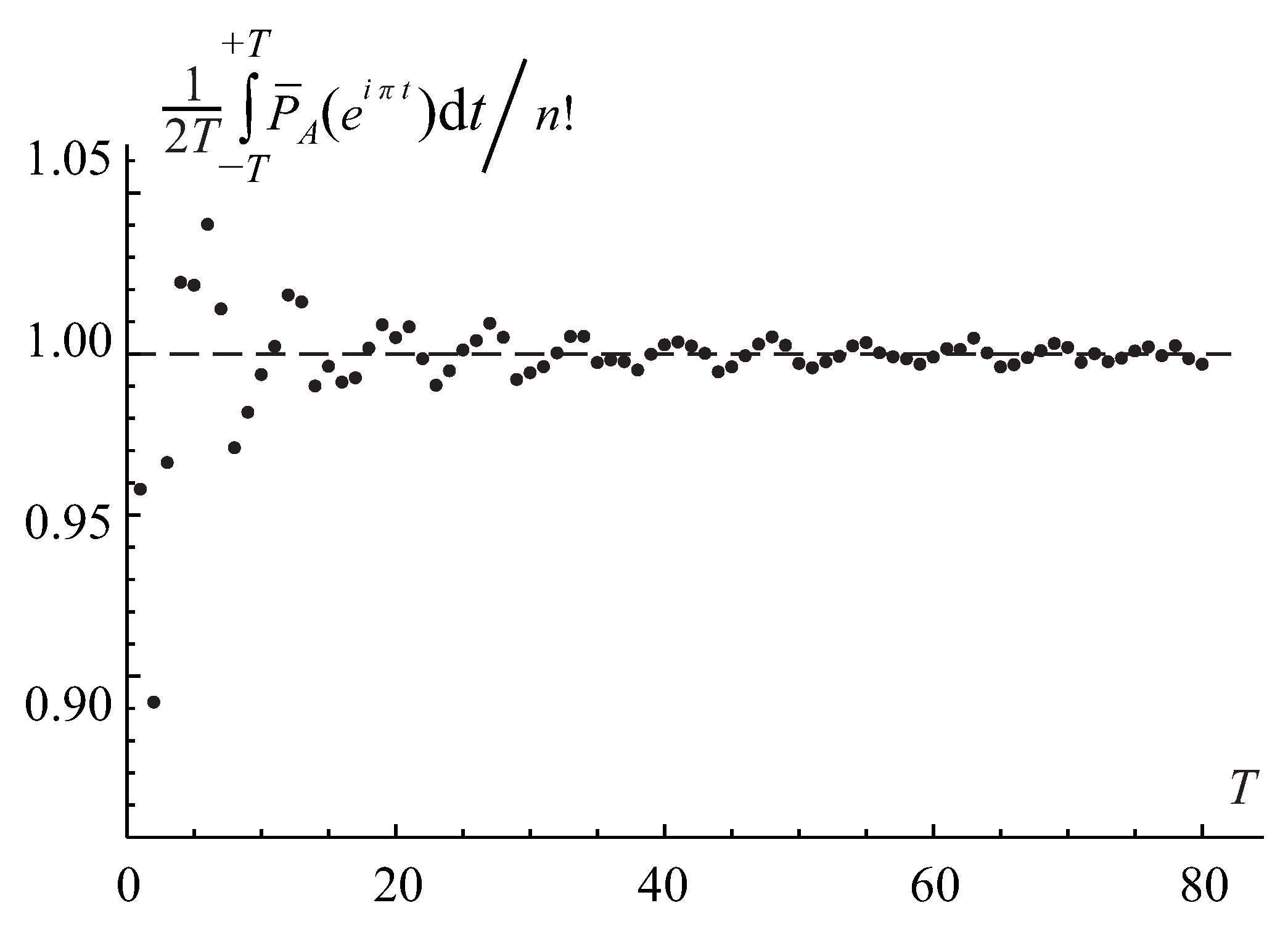




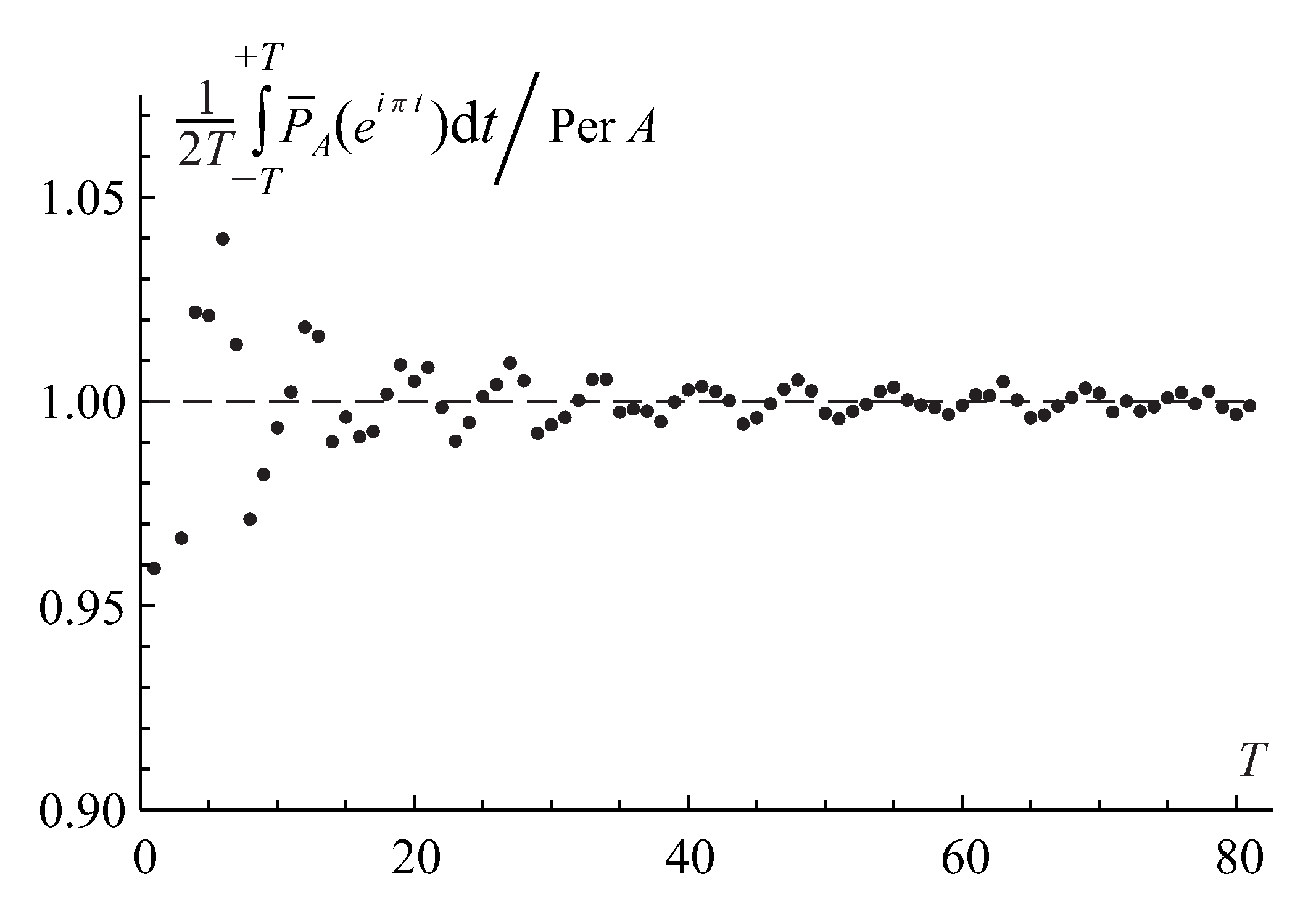



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kocharovsky, V.; Kocharovsky, V.; Tarasov, S. Unification of the Nature’s Complexities via a Matrix Permanent—Critical Phenomena, Fractals, Quantum Computing, ?P-Complexity. Entropy 2020, 22, 322. https://doi.org/10.3390/e22030322
Kocharovsky V, Kocharovsky V, Tarasov S. Unification of the Nature’s Complexities via a Matrix Permanent—Critical Phenomena, Fractals, Quantum Computing, ?P-Complexity. Entropy. 2020; 22(3):322. https://doi.org/10.3390/e22030322
Chicago/Turabian StyleKocharovsky, Vitaly, Vladimir Kocharovsky, and Sergey Tarasov. 2020. "Unification of the Nature’s Complexities via a Matrix Permanent—Critical Phenomena, Fractals, Quantum Computing, ?P-Complexity" Entropy 22, no. 3: 322. https://doi.org/10.3390/e22030322
APA StyleKocharovsky, V., Kocharovsky, V., & Tarasov, S. (2020). Unification of the Nature’s Complexities via a Matrix Permanent—Critical Phenomena, Fractals, Quantum Computing, ?P-Complexity. Entropy, 22(3), 322. https://doi.org/10.3390/e22030322





