Regularization Methods Based on the Lq-Likelihood for Linear Models with Heavy-Tailed Errors
Abstract
:1. Introduction
2. Preliminaries
2.1. Normal Linear Model and Sparse Estimation
2.2. -Likelihood
2.3. q-Normal Model
3. Problem and Estimation Method
3.1. Linear Model with q-Normal Error
3.2. -Likelihood-Based Regularization Methods
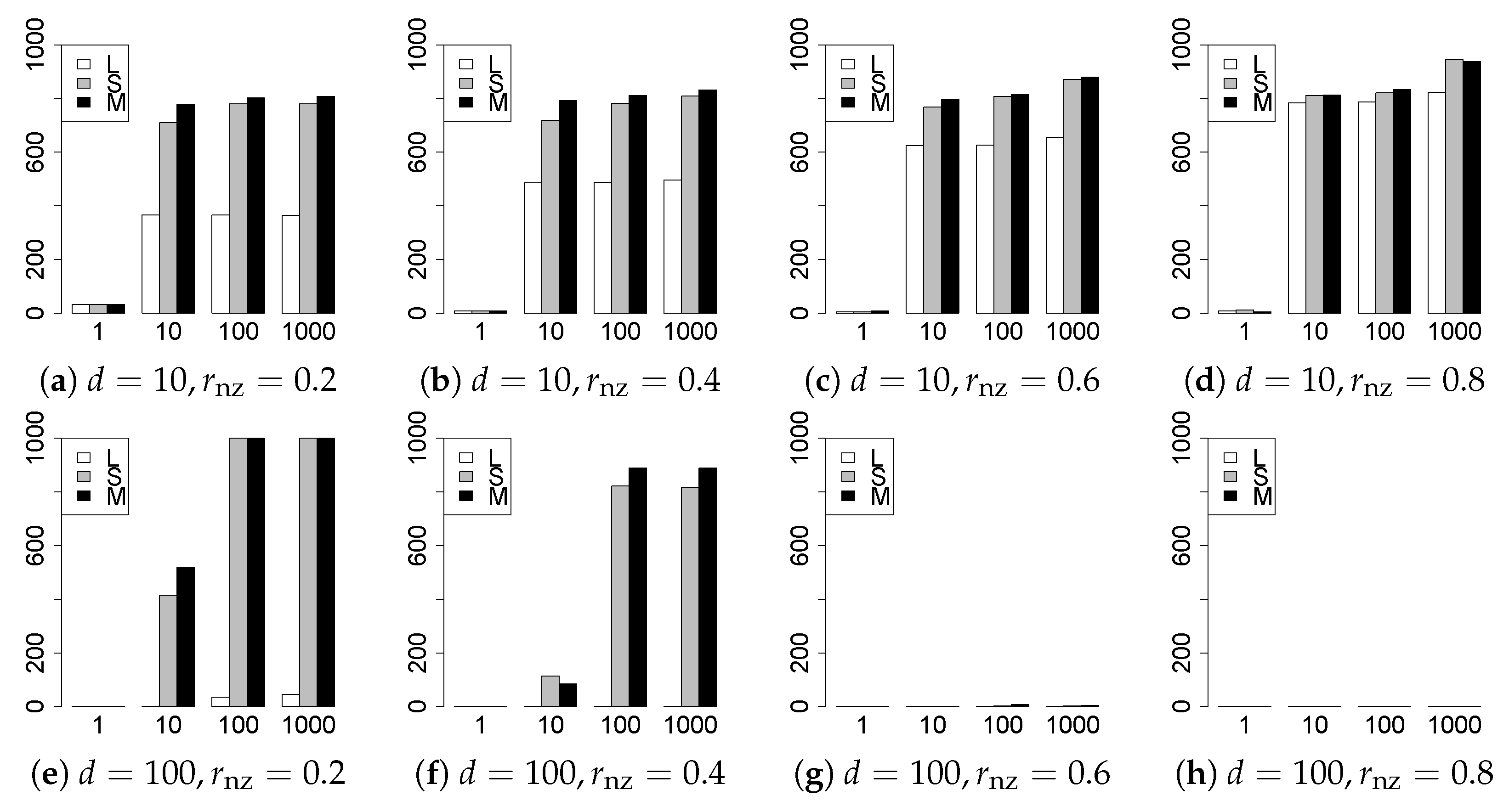
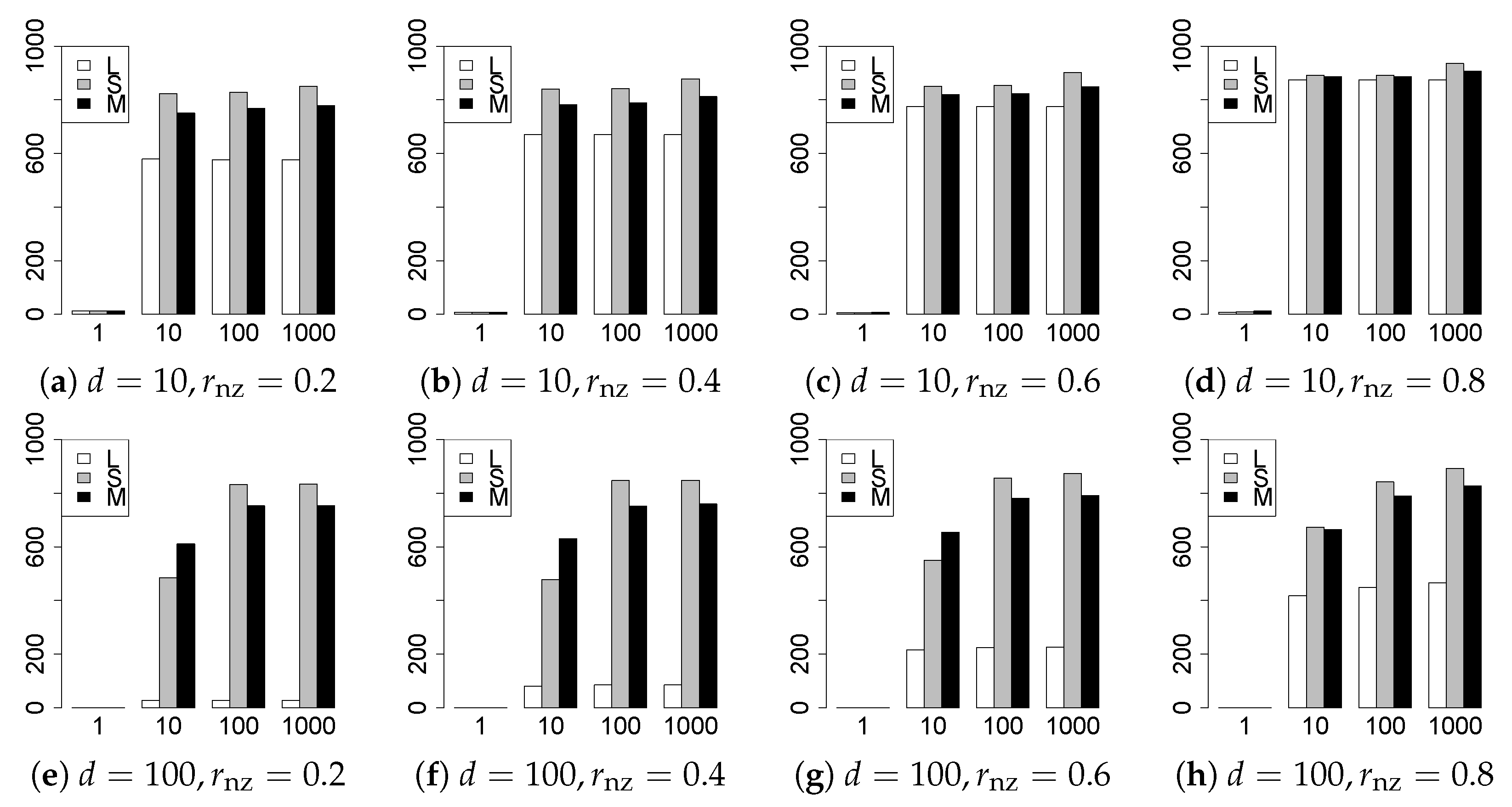
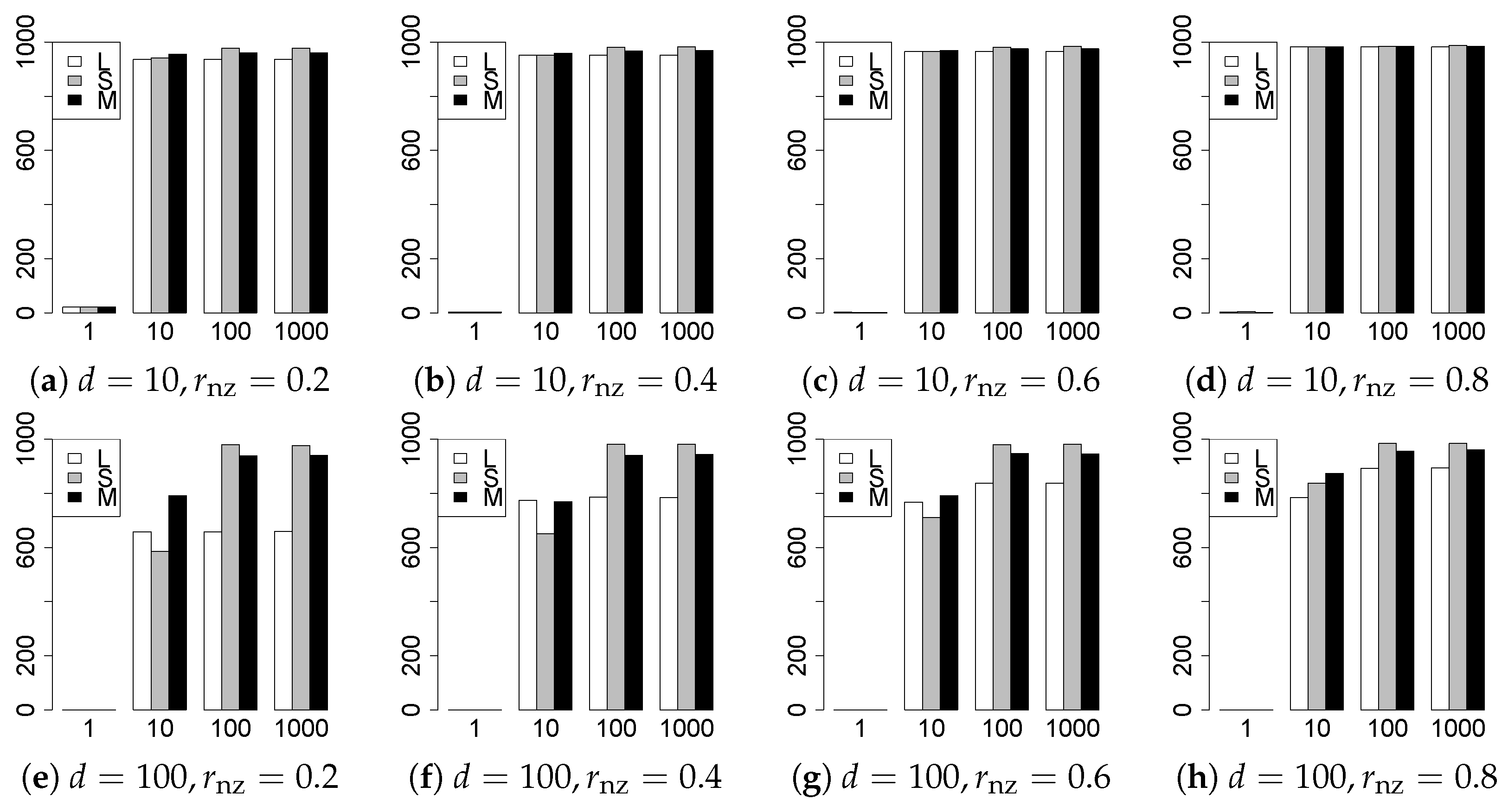
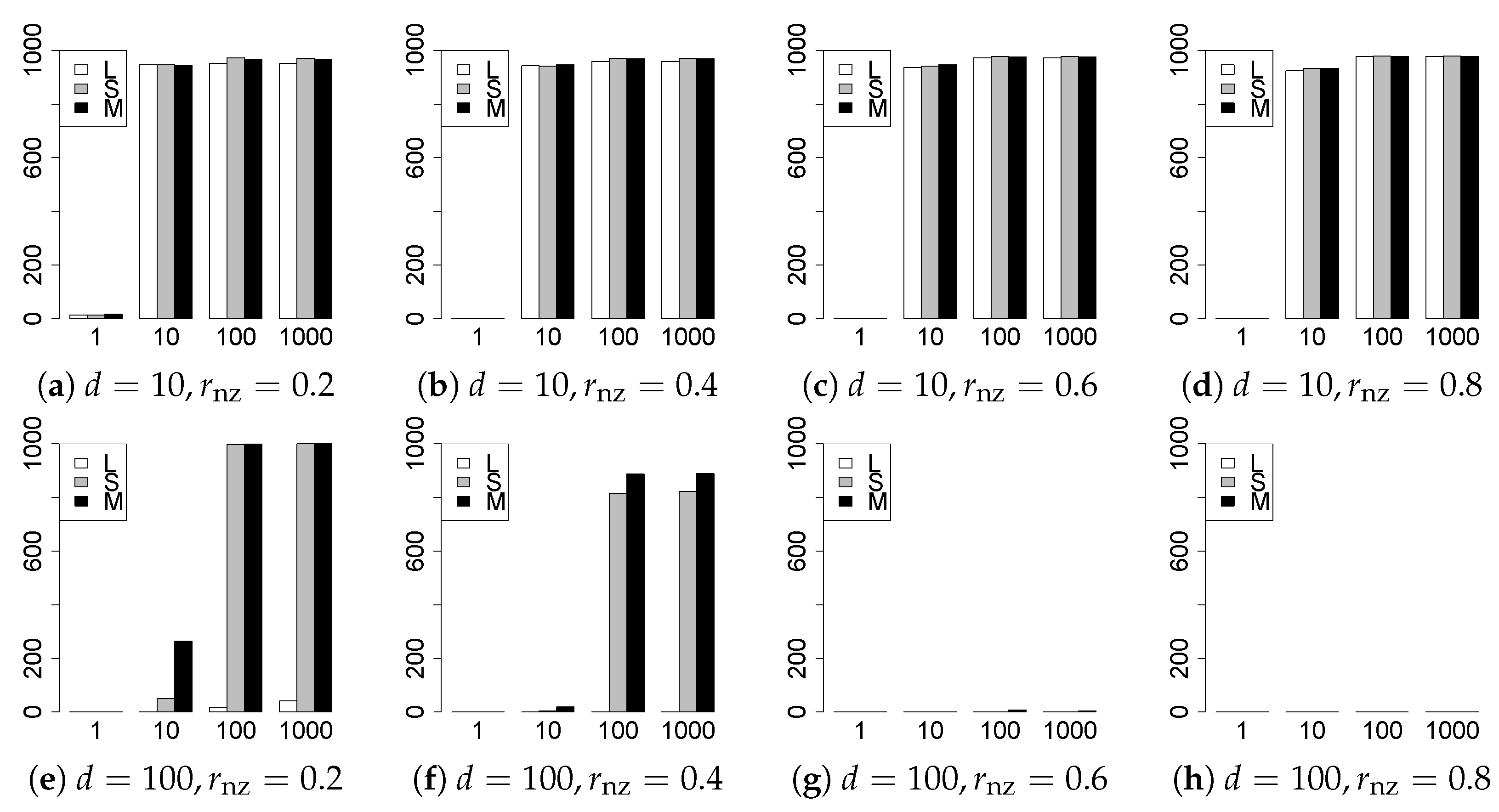
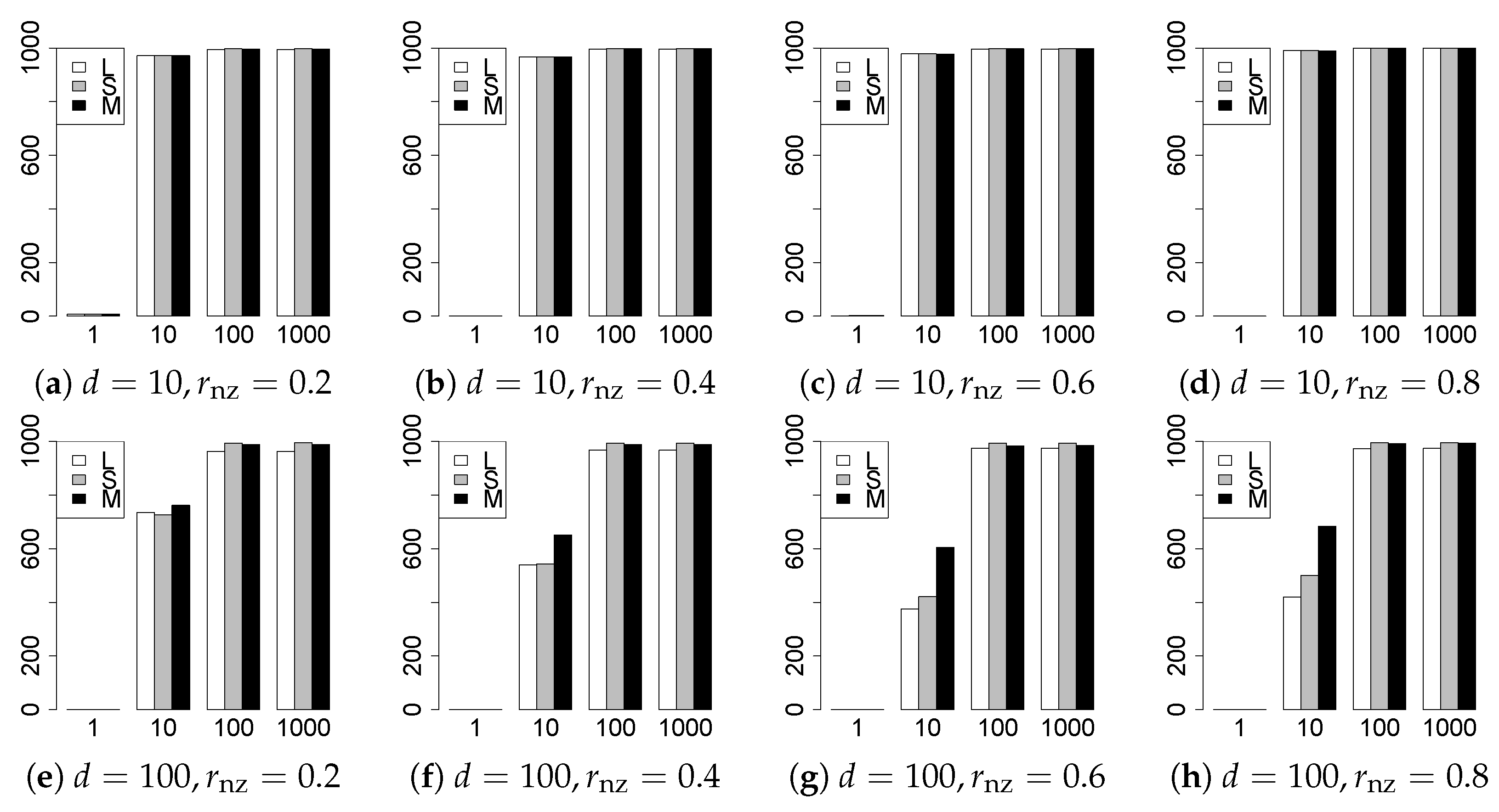
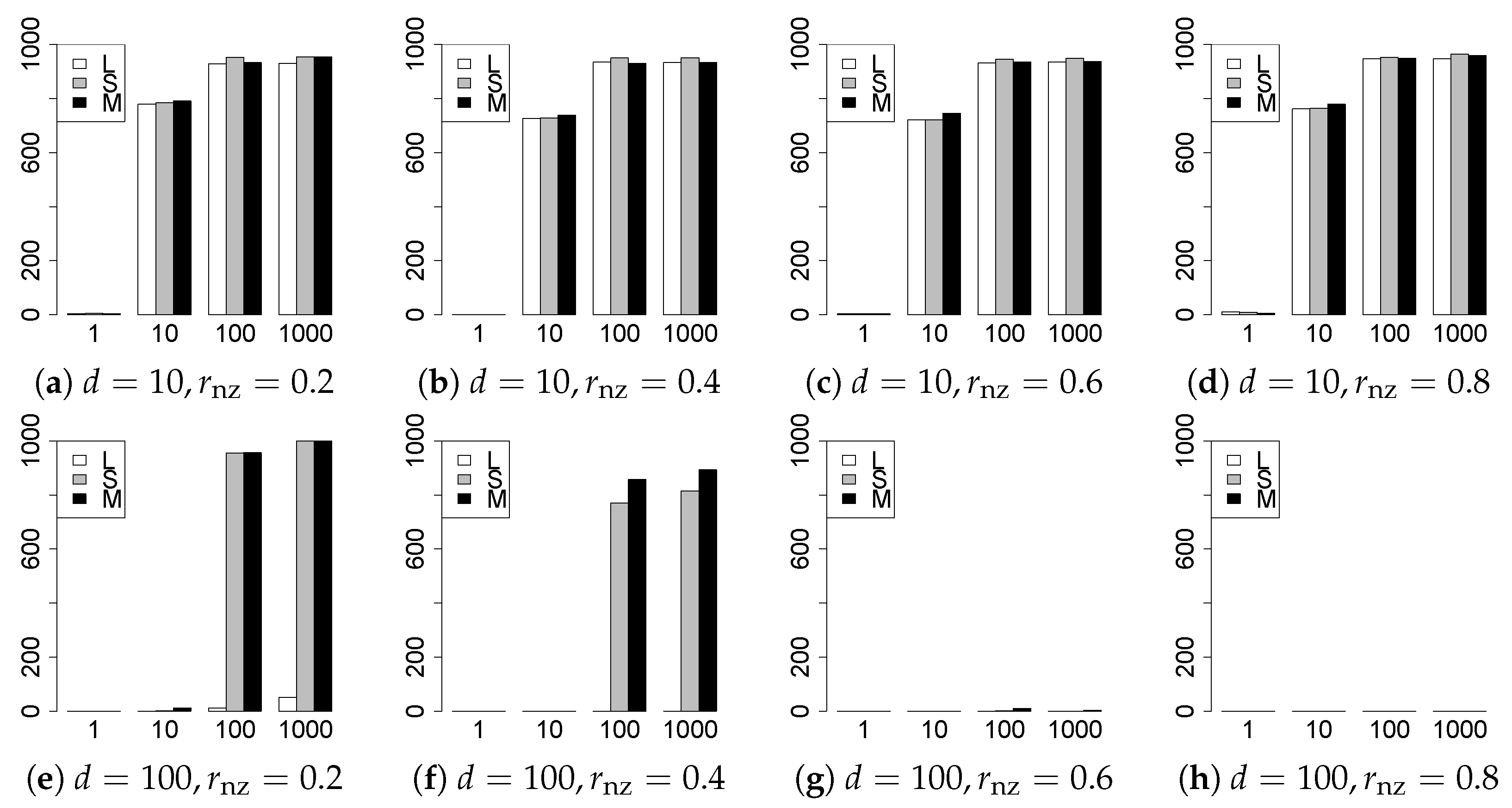
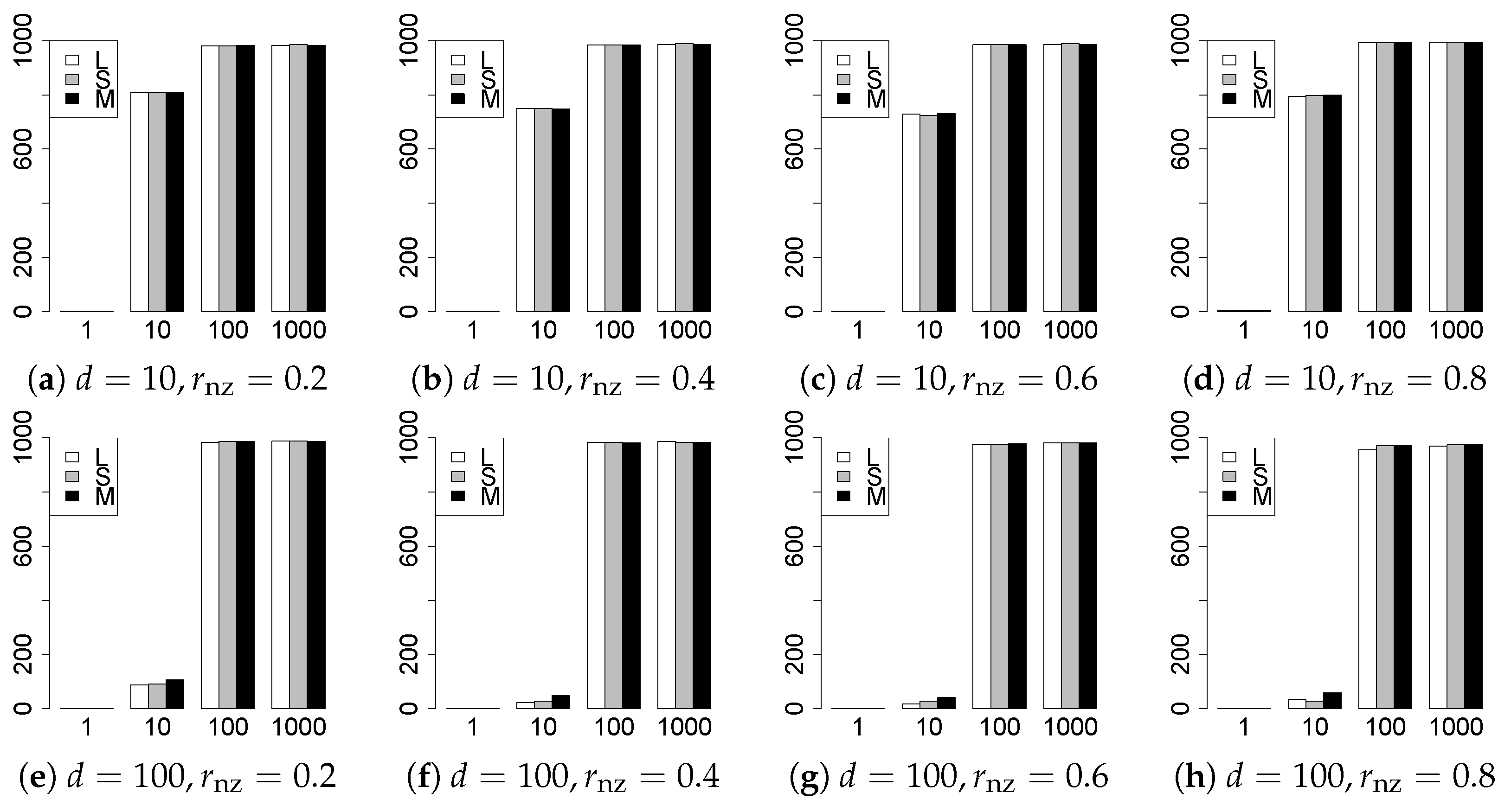
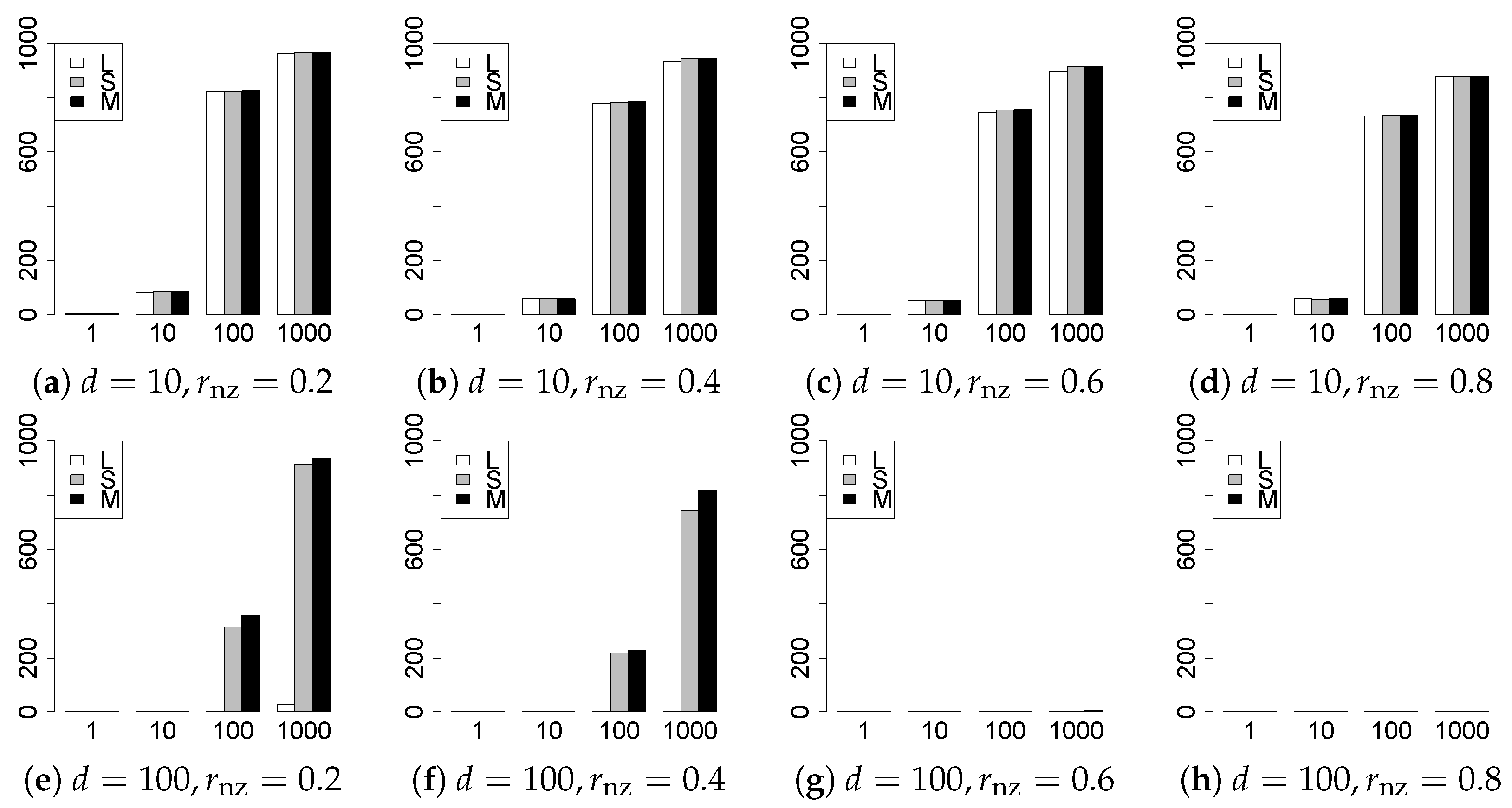
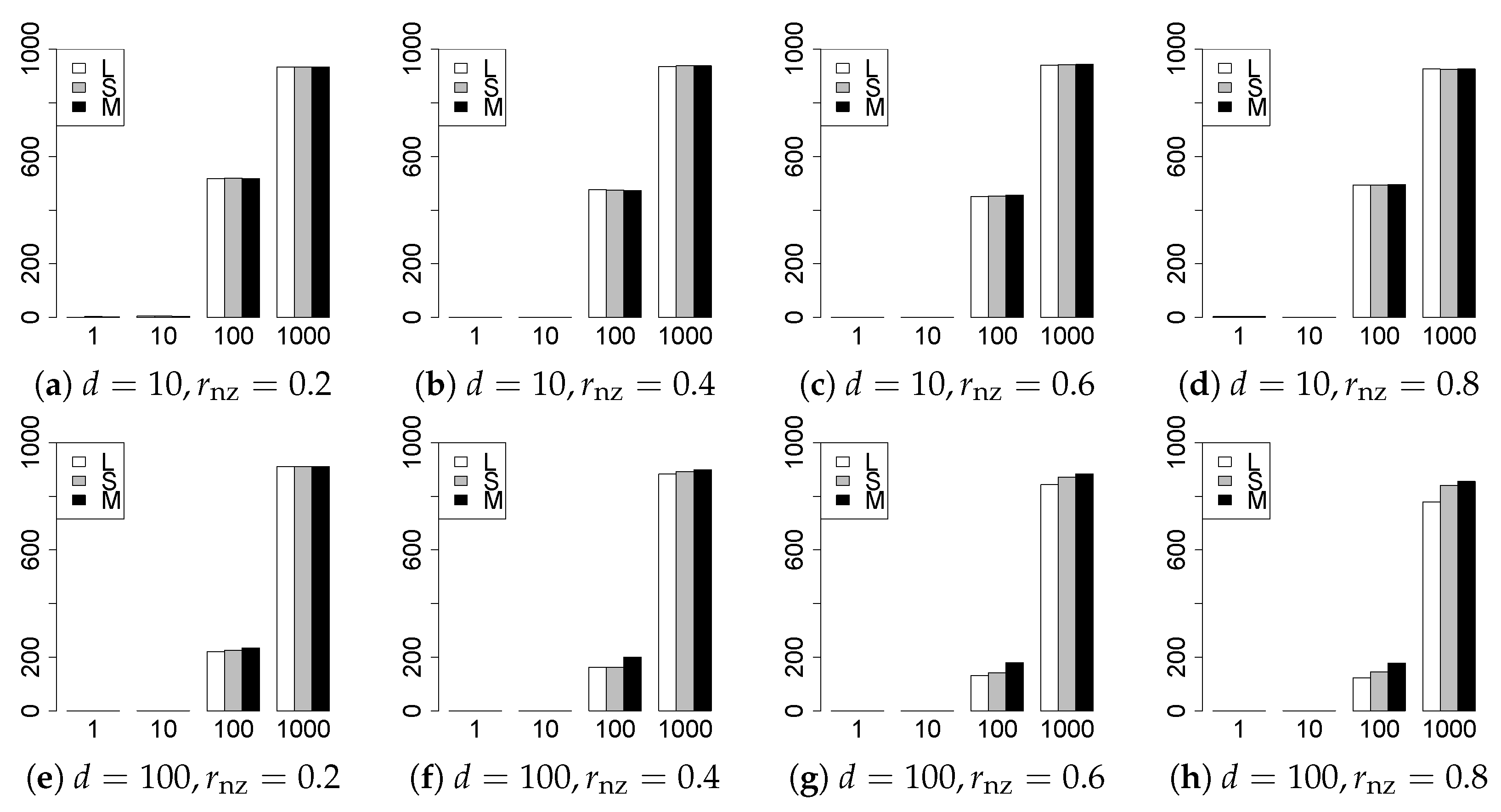
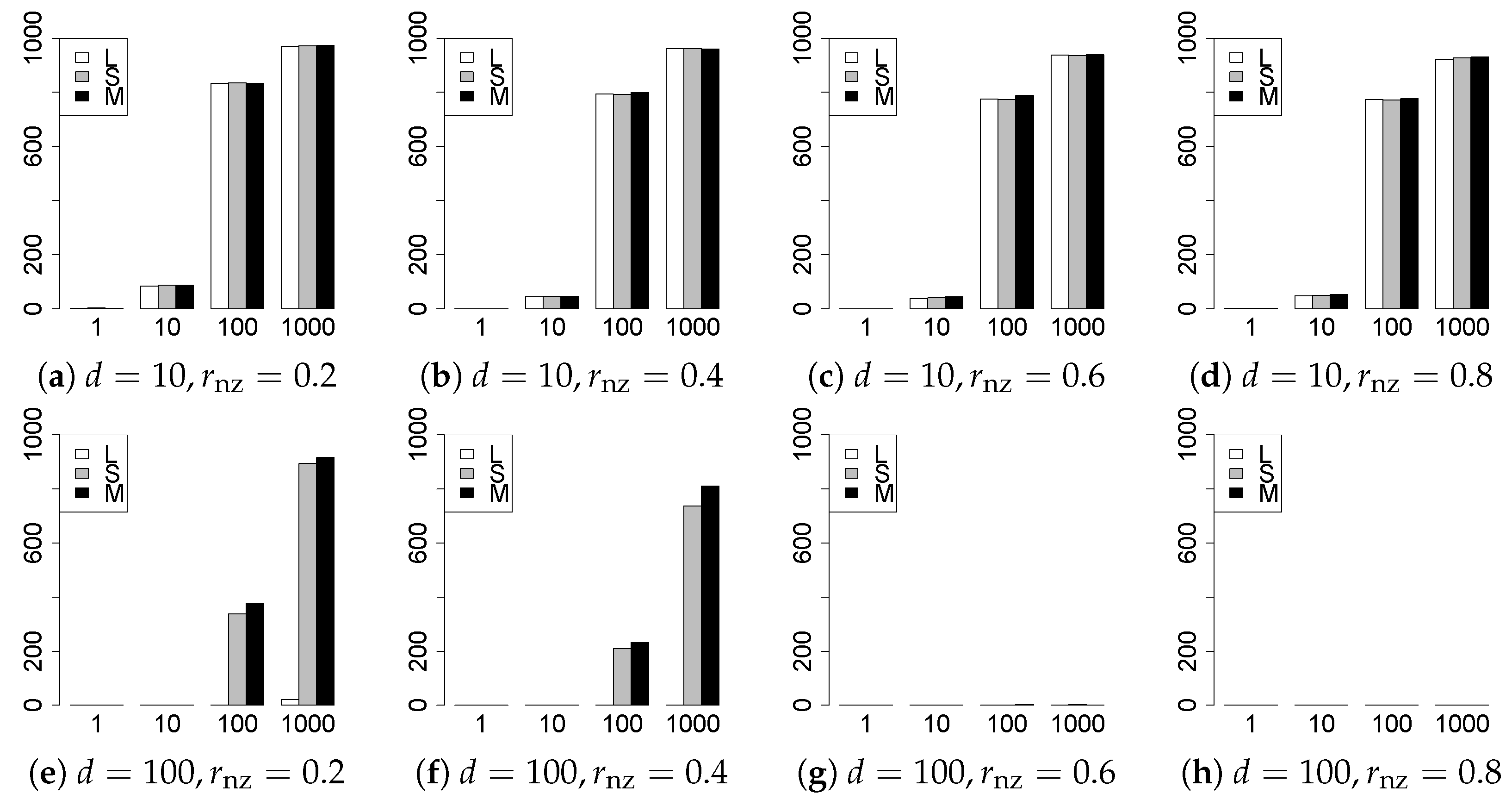
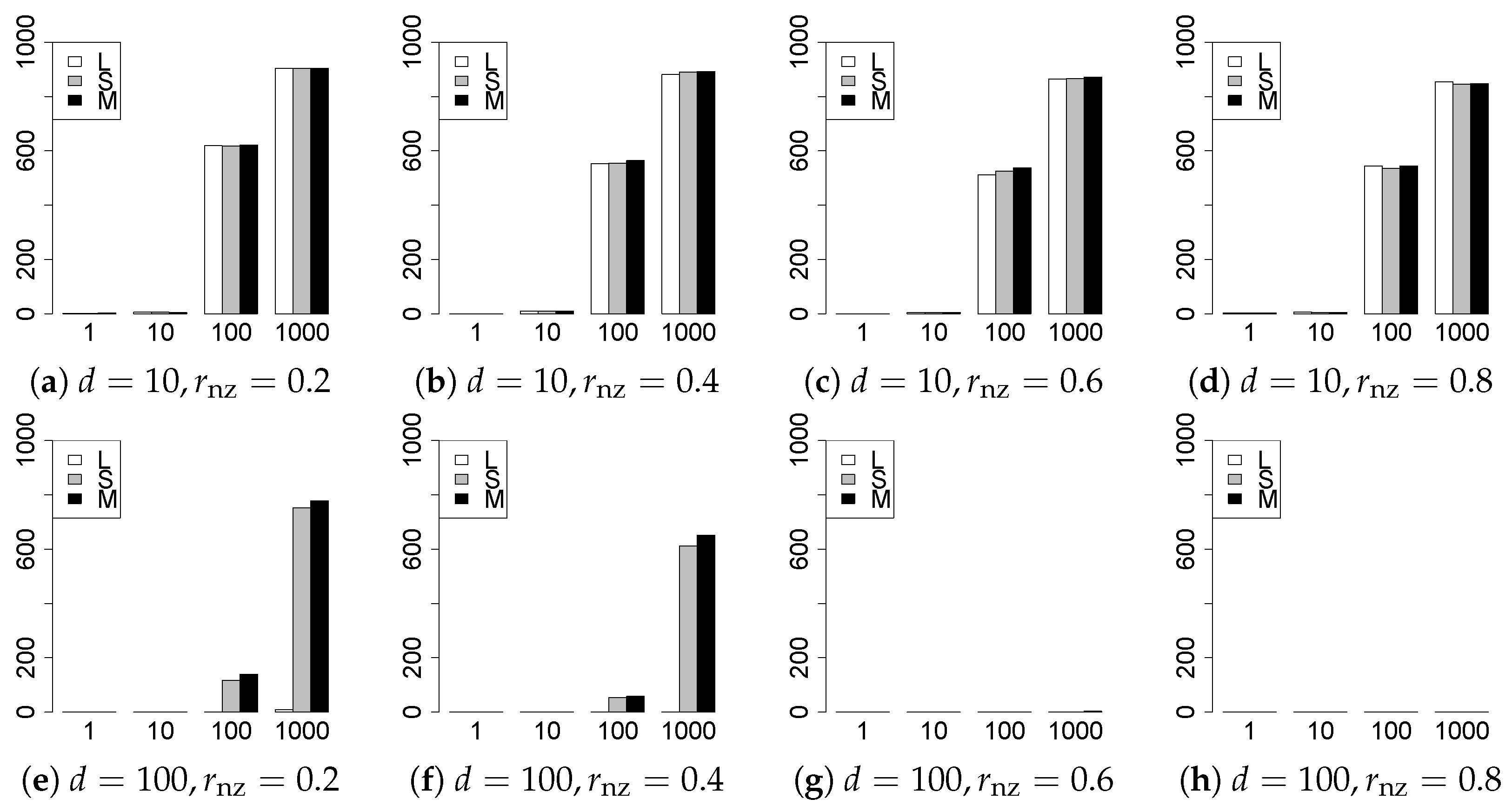
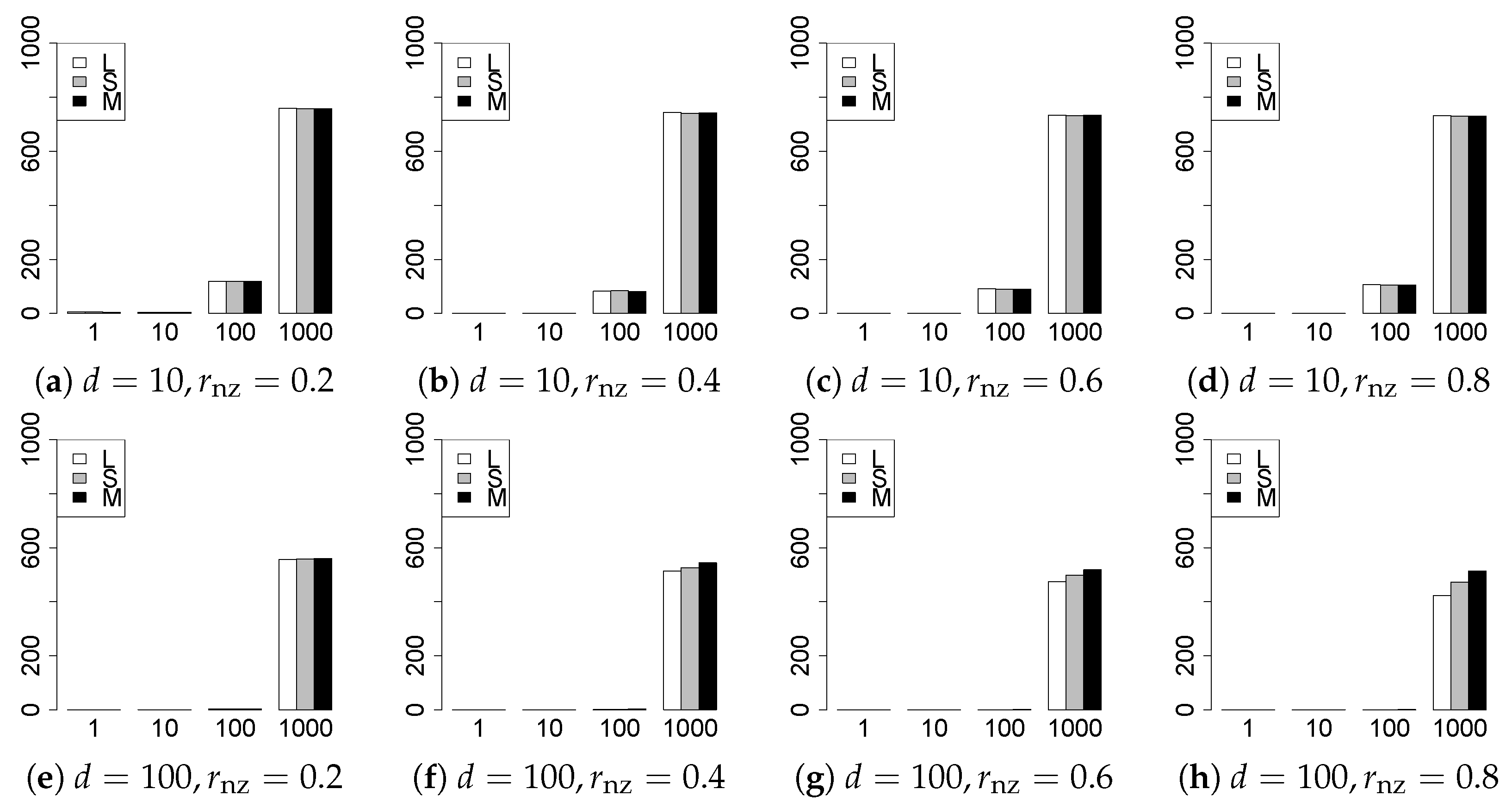
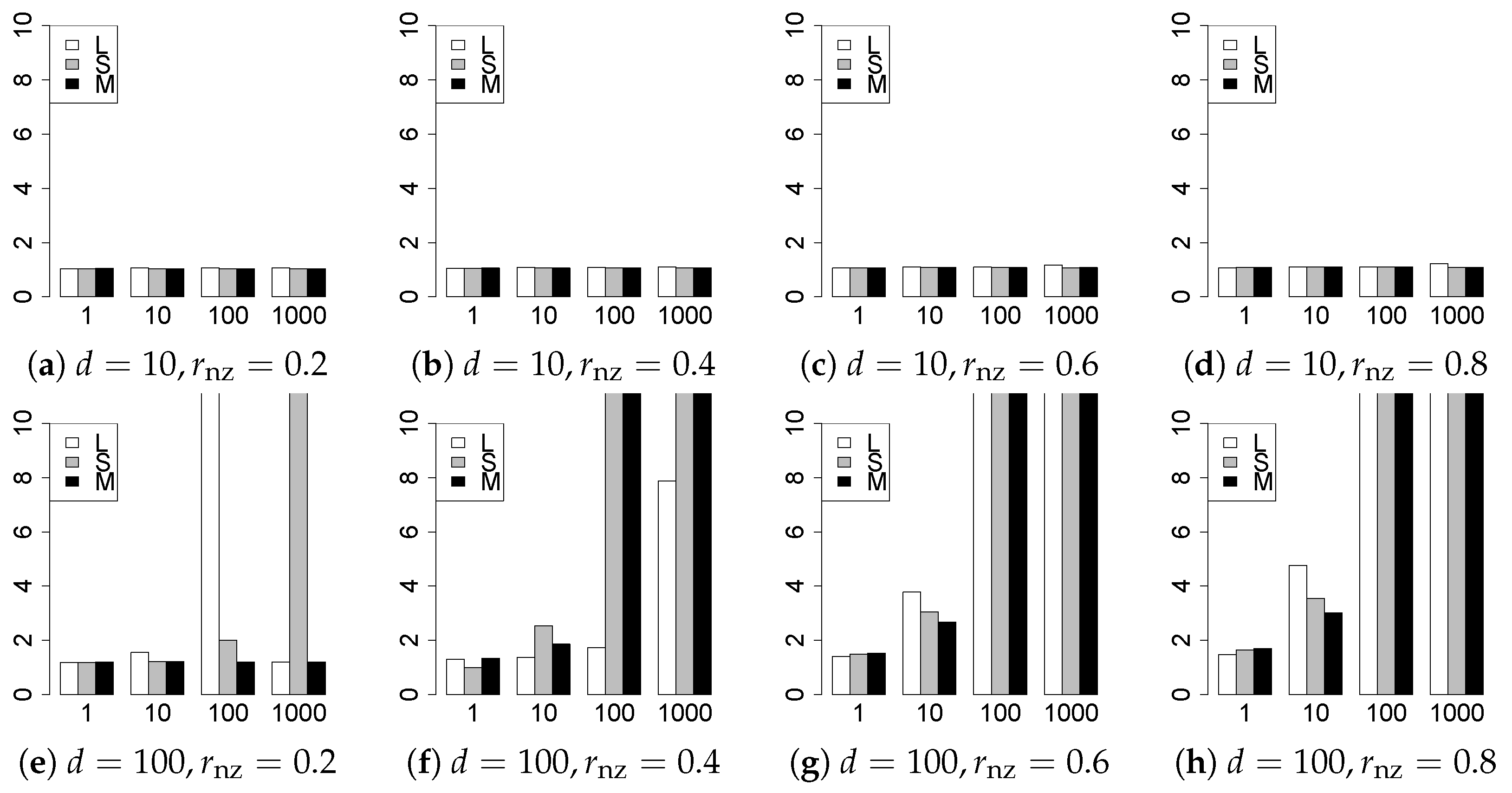
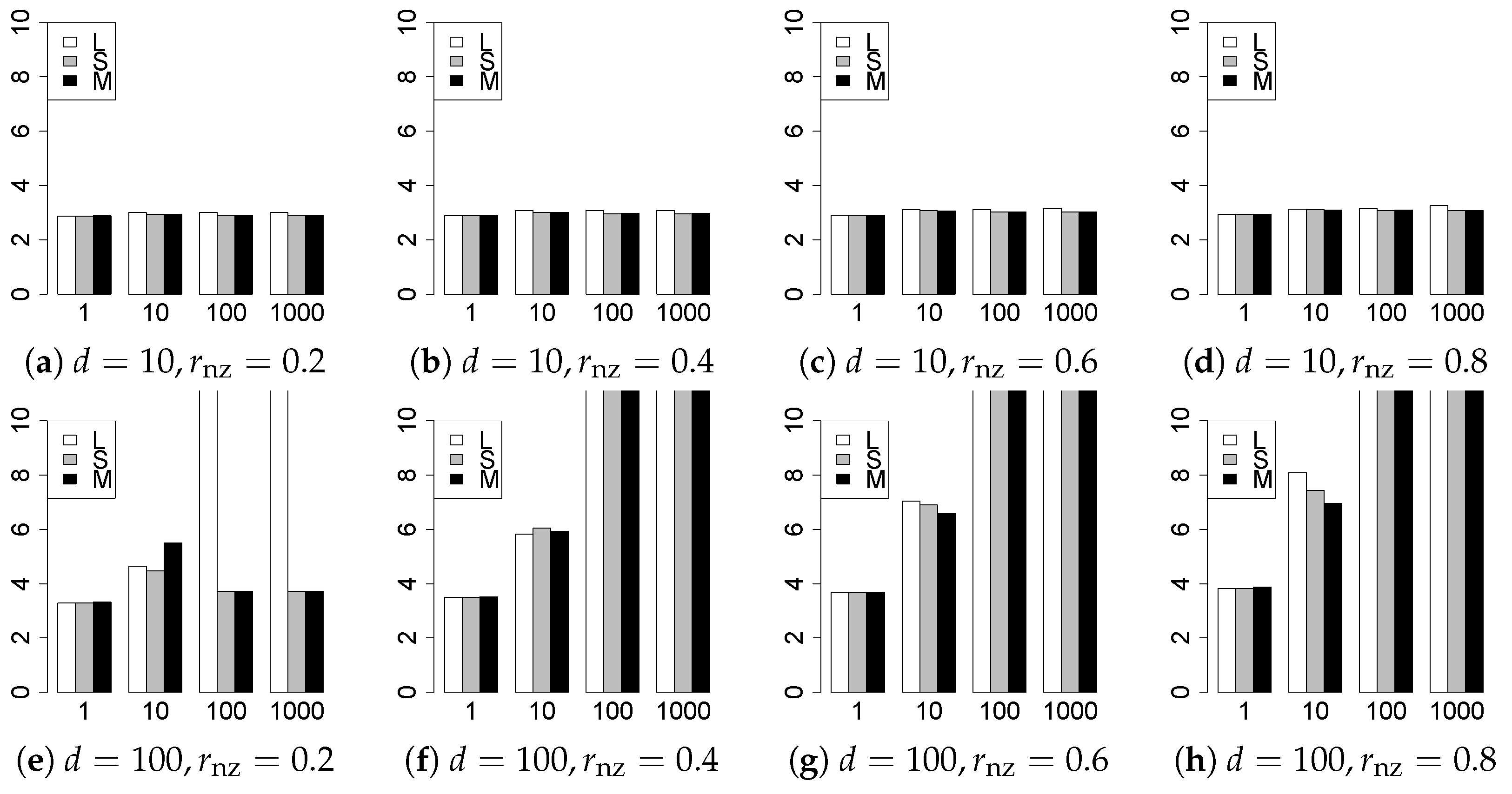
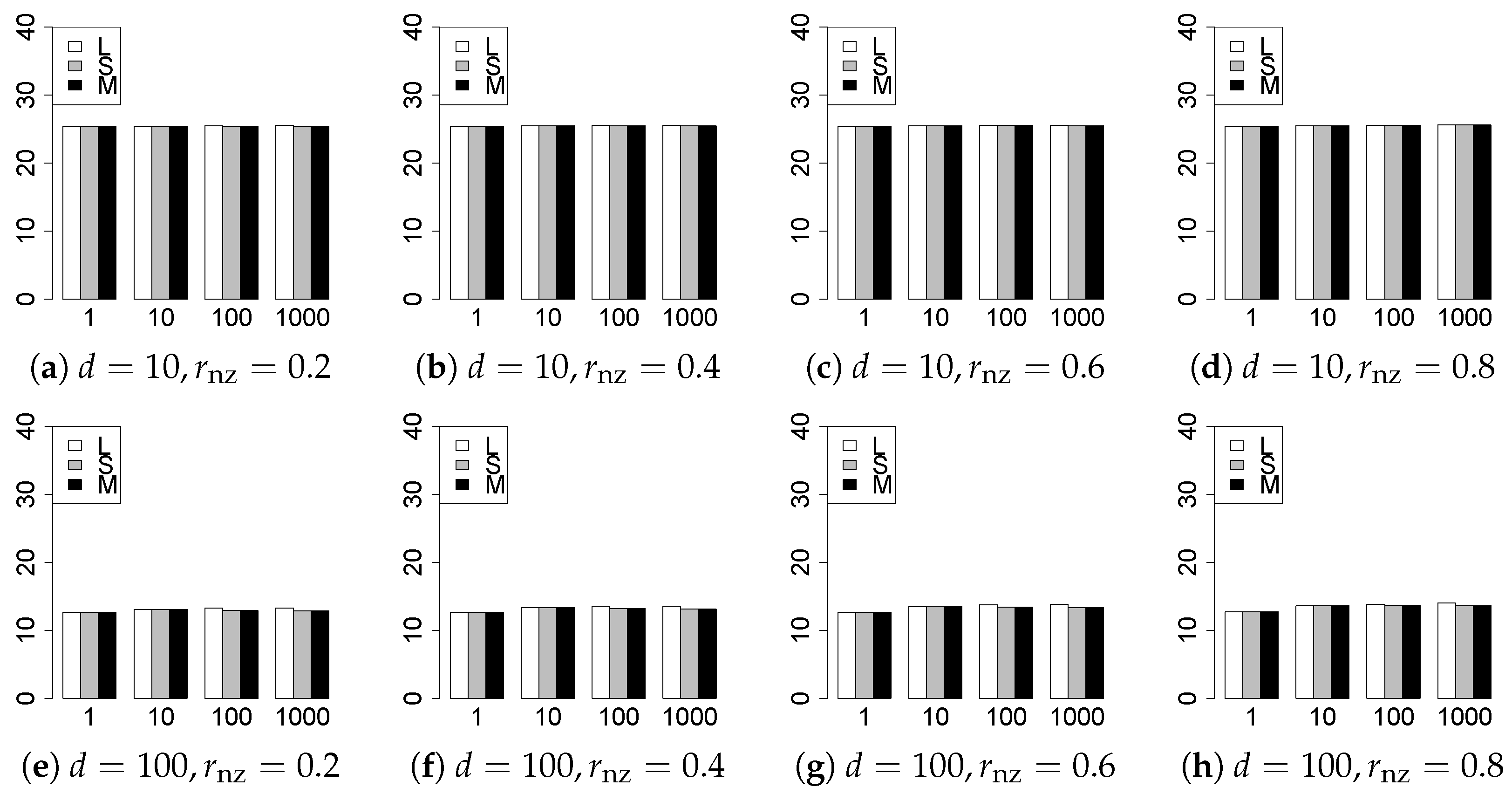
4. Numerical Experiments
4.1. Setting
4.2. Result
5. Conclusions
Supplementary Materials
Funding
Conflicts of Interest
References
- Tibshirani, R. Regression Shrinkage and Selection via the Lasso. J. R. Stat. Soc. B 1996, 58, 267–288. [Google Scholar] [CrossRef]
- Fan, J.; Li, R. Variable Selection via Nonconcave Penalized Likelihood and its Oracle Properties. J. Am. Stat. Assoc. 2001, 96, 1348–1360. [Google Scholar] [CrossRef]
- Candes, E.; Tao, T. The Dantzig selector: Statistical estimation when p is much larger than n. Ann. Stat. 2007, 36, 2313–2351. [Google Scholar] [CrossRef] [Green Version]
- Zhang, C.H. Nearly unbiased variable selection under minimax concave penalty. Ann. Stat. 2010, 38, 894–942. [Google Scholar] [CrossRef] [Green Version]
- Park, M.Y.; Hastie, T. L1-Regularization Path Algorithm for Generalized Linear Models. J. R. Stat. Soc. B 2007, 69, 659–677. [Google Scholar] [CrossRef]
- Ahmed, S.E.; Kim, H.; Yildirim, G.; Yüzbasi, B. High-Dimensional Regression Under Correlated Design: An Extensive Simulation Study. In International Workshop on Matrices and Statistics; Springer: Cham, Switzerland, 2016; pp. 145–175. [Google Scholar]
- Furuichi, S. On the maximum entropy principle and the minimization of the Fisher information in Tsallis statistics. J. Math. Phys. 2009, 50, 013303. [Google Scholar] [CrossRef] [Green Version]
- Prato, D.; Tsallis, C. Nonextensive foundation of Lévy distributions. Phys. Rev. E 2000, 60, 2398–2401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tsallis, C. Introduction to Nonextensive Statistical Mechanics; Springer: New York, NY, USA, 2009. [Google Scholar]
- Alzaatreh, A.; Lee, C.; Famoye, F.; Ghosh, I. The Generalized Cauchy Family of Distributions with Applications. J. Stat. Distrib. Appl. 2016, 3, 12. [Google Scholar] [CrossRef] [Green Version]
- Bassiou, N.; Kotropoulos, C.; Koliopoulou, E. Symmetric α-Stable Sparse Linear Regression for Musical Audio Denoising. In Proceedings of the 8th International Symposium on Image and Signal Processing and Analysis (ISPA 2013), Trieste, Italy, 4–6 September 2013; pp. 382–387. [Google Scholar]
- Carrillo, R.E.; Aysal, T.C.; Barner, K.E. Generalized Cauchy Distribution Based Robust Estimation. In Proceedings of the International Conference on Acoustics, Speech, and Signal Processing (ICASSP) 2008, Las Vegas, NV, USA, 31 March–4 April 2008; pp. 3389–3392. [Google Scholar]
- Carrillo, R.E.; Aysal, T.C.; Barner, K.E. A Generalized Cauchy Distribution Framework for Problems Requiring Robust Behavior. EURASIP J. Adv. Signal Process. 2010, 2010, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Ferrari, D.; Yang, Y. Maximum Lq-Likelihood Estimation. Ann. Stat. 2010, 38, 753–783. [Google Scholar] [CrossRef] [Green Version]
- Hastie, T.; Tibshirani, R.; Friedman, J. The Elements of Statistical Learning, 2nd ed.; Springer: New York, NY, USA, 2009. [Google Scholar]
- Efron, B.; Hastie, T.; Johnstone, I.; Tibshirani, R. Least Angle Regression. Ann. Stat. 2004, 32, 407–499. [Google Scholar]
- Hinich, M.J.; Talwar, P.P. A Simple Method for Robust Regression. J. Am. Stat. Assoc. 1975, 70, 113–119. [Google Scholar] [CrossRef]
- Holland, P.W.; Welsch, R.E. Robust Regression Using Iteratively Reweighted Least-Squares. Commun. Stat. Theory Methods 1977, 6, 813–827. [Google Scholar] [CrossRef]
- Kadiyala, K.R.; Murthy, K.S.R. Estimation of regression equation with Cauchy disturbances. Can. J. Stat. 1977, 5, 111–120. [Google Scholar] [CrossRef]
- Smith, V.K. Least squares regression with Cauchy errors. Oxf. Bull. Econ. Stat. 1973, 35, 223–231. [Google Scholar] [CrossRef]







| 1 | 5 | 9 | 13 | 17 | 21 | 25 | 29 | |
| 2 | 6 | 10 | 14 | 18 | 22 | 26 | 30 | |
| 3 | 7 | 11 | 15 | 19 | 23 | 27 | 31 | |
| 4 | 8 | 12 | 16 | 20 | 24 | 28 | 32 | |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hirose, Y. Regularization Methods Based on the Lq-Likelihood for Linear Models with Heavy-Tailed Errors. Entropy 2020, 22, 1036. https://doi.org/10.3390/e22091036
Hirose Y. Regularization Methods Based on the Lq-Likelihood for Linear Models with Heavy-Tailed Errors. Entropy. 2020; 22(9):1036. https://doi.org/10.3390/e22091036
Chicago/Turabian StyleHirose, Yoshihiro. 2020. "Regularization Methods Based on the Lq-Likelihood for Linear Models with Heavy-Tailed Errors" Entropy 22, no. 9: 1036. https://doi.org/10.3390/e22091036
APA StyleHirose, Y. (2020). Regularization Methods Based on the Lq-Likelihood for Linear Models with Heavy-Tailed Errors. Entropy, 22(9), 1036. https://doi.org/10.3390/e22091036





