Uncertainty of Interval Type-2 Fuzzy Sets Based on Fuzzy Belief Entropy
Abstract
:1. Introduction
2. Preliminaries
2.1. Interval Type-2 Fuzzy Sets
2.2. Basic Concepts in Belief Structure
2.2.1. Frame of Discernment (FOD)
2.2.2. Basic Probability Assignment (BPA)
2.2.3. Belief Function
2.2.4. Plausibility Function
2.3. The Relation among IT2 FS, Z-Valuations, and Evidence Theory
2.4. Shannon Entropy
2.5. Existing Belief Entropy
3. Fuzzy Belief Entropy
4. Numerical Example
4.1. Example 1
4.2. Example 2
4.3. Example 3
4.4. Example 4
4.5. Example 5
4.6. Example 6
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Deng, Y. Information Volume of Mass Function. Int. J. Comput. Commun. Control 2020, 15, 3983. [Google Scholar] [CrossRef]
- Pal, N.R.; Bezdek, J.C. Measuring Fuzzy uncertainty. IEEE Trans. Fuzzy Syst. 1994, 2, 107–118. [Google Scholar] [CrossRef]
- Klir, G.; Yuan, B. Fuzzy Sets and Fuzzy Logic: Theory and Applications; World Scientific: Singapore, 1995. [Google Scholar]
- Zadeh, L.A. Fuzzy sets, information and control. Inf. Control 1965, 8, 338–353. [Google Scholar] [CrossRef] [Green Version]
- Dempster, A.P. Upper and Lower Probabilities Induced by a Multivalued Mapping. Ann. Math. Stat. 1967, 38, 325–339. [Google Scholar] [CrossRef]
- Shafer, G.A. A Mathematical Theory of Evidence. Technometrics 1978, 20, 106. [Google Scholar]
- Pawlak, Z. Rough sets. Int. J. Comput. Inf. Sci. 1982, 11, 341–356. [Google Scholar] [CrossRef]
- Denoeux, T. Modeling vague beliefs using fuzzy-valued belief structures. Fuzzy Sets Syst. 2000, 116, 167–199. [Google Scholar] [CrossRef]
- Yager, R.R. Dempster–Shafer belief structures with interval valued focal weights. Int. J. Intell. Syst. 2001, 16, 497–512. [Google Scholar] [CrossRef]
- Song, Y.; Wang, X.; Lei, L.; Xue, A. Combination of interval-valued belief structures based on intuitionistic fuzzy set. Knowl.-Based Syst. 2014, 67, 61–70. [Google Scholar] [CrossRef]
- Xue, Y.; Deng, Y. Interval-valued belief entropies for Dempster Shafer structures. Soft Comput. 2021, 25, 8063–8071. [Google Scholar] [CrossRef]
- Zadeh, L.A. A Note on Z-numbers. Inf. Sci. 2011, 181, 2923–2932. [Google Scholar] [CrossRef]
- Alcantud, J.; Feng, F.; Yager, R.R. An N-Soft Set Approach to Rough Sets. IEEE Trans. Fuzzy Syst. 2019, 28, 2996–3007. [Google Scholar] [CrossRef]
- Liu, P.; Zhang, X. A novel approach to multi-criteria group decision-making problems based on linguistic D numbers. Comput. Appl. Math. 2020, 39, 132. [Google Scholar] [CrossRef]
- Li, Y.; Pelusi, D.; Deng, Y. Generate two-dimensional belief function based on an improved similarity measure of trapezoidal fuzzy numbers. Comput. Appl. Math. 2020, 39, 326. [Google Scholar] [CrossRef]
- Dymova, L.; Kaczmarek, K.; Sevastjanov, P.; Kulawik, J. A Fuzzy Multiple Criteria Decision Making Approach with a Complete User Friendly Computer Implementation. Entropy 2021, 23, 203. [Google Scholar] [CrossRef]
- Pelusi, D.; Mascella, R.; Tallini, L.; Nayak, J.; Naik, B.; Abraham, A. Neural Network and Fuzzy System for the tuning of Gravitational Search Algorithm parameters. Expert Syst. Appl. 2018, 102, 234–244. [Google Scholar] [CrossRef]
- Cao, Z.; Lin, C.T. Inherent fuzzy entropy for the improvement of EEG complexity evaluation. IEEE Trans. Fuzzy Syst. 2018, 26, 1032–1035. [Google Scholar] [CrossRef] [Green Version]
- Zadeh, L.A. The concept of a linguistic variable and its application to approximate reasoning—I. Inf. Sci. 1975, 8, 199–249. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K.; Gargov, G. Interval valued intuitionistic fuzzy sets. Fuzzy Sets Syst. 1989, 31, 343–349. [Google Scholar] [CrossRef]
- Mendel, J.M.; John, R.I.; Liu, F. Interval Type-2 Fuzzy Logic Systems Made Simple. IEEE Trans. Fuzzy Syst. 2006, 14, 808–821. [Google Scholar] [CrossRef] [Green Version]
- Mendel, J.M.; John, R. Type-2 fuzzy sets made simple. IEEE Trans. Fuzzy Syst. 2002, 10, 117–127. [Google Scholar] [CrossRef]
- Tang, G.; Chiclana, F.; Lin, X.; Liu, P. Interval type-2 fuzzy multi-attribute decision-making approaches for evaluating the service quality of Chinese commercial banks. Knowl.-Based Syst. 2020, 193, 105438. [Google Scholar] [CrossRef]
- Ozen, T.; Garibaldi, J.M. Effect of type-2 fuzzy membership function shape on modelling variation in human decision making. In Proceedings of the 2004 IEEE International Conference on Fuzzy Systems (IEEE Cat. No.04CH37542), Budapest, Hungary, 25–29 July 2004. [Google Scholar]
- Chen, S.M.; Yang, M.W.; Lee, L.W.; Yang, S.W. Fuzzy multiple attributes group decision-making based on ranking interval type-2 fuzzy sets. Expert Syst. Appl. Int. J. 2012, 39, 5295–5308. [Google Scholar] [CrossRef]
- Baguley, P.; Page, T.; Koliza, V.; Maropoulos, P. Time to market prediction using type-2 fuzzy sets. J. Manuf. Technol. Manag. 2006, 17, 513–520. [Google Scholar] [CrossRef]
- Jia, Z.; Liu, Z.Q. Type-2 fuzzy hidden Markov models and their application to speech recognition. IEEE Trans. Fuzzy Syst. 2006, 14, 454–467. [Google Scholar]
- Dymova, L.; Sevastjanov, P.; Tikhonenko, A. An interval type-2 fuzzy extension of the TOPSIS method using alpha cuts. Knowl.-Based Syst. 2015, 83, 116–127. [Google Scholar] [CrossRef]
- Pan, Y.; Zhang, L.; Li, Z.W.; Ding, L. Improved Fuzzy Bayesian Network-Based Risk Analysis With Interval-Valued Fuzzy Sets and D-S Evidence Theory. IEEE Trans. Fuzzy Syst. 2019, 28, 2063–2077. [Google Scholar] [CrossRef]
- Cao, Z.; Lin, C.T.; Lai, K.L.; Ko, L.W.; King, J.T.; Liao, K.K.; Fuh, J.L.; Wang, S.J. Extraction of SSVEPs-based Inherent Fuzzy Entropy Using a Wearable Headband EEG in Migraine Patients. IEEE Trans. Fuzzy Syst. 2020, 28, 14–27. [Google Scholar] [CrossRef] [Green Version]
- Liu, P.; Zhang, X.; Wang, Z.; Shi, Y. An Extended VIKOR Method for Multiple Attribute Decision Making with Linguistic D Numbers Based on Fuzzy Entropy. Int. J. Inf. Technol. Decis. Mak. (IJITDM) 2020, 19, 143–167. [Google Scholar] [CrossRef]
- Feifei, J.; Lidan, P.; Huayou, C.; Ligang, Z. Interval-valued intuitionistic fuzzy continuous weighted entropy and its application to multi-criteria fuzzy group decision making. Knowl.-Based Syst. 2014, 59, 132–141. [Google Scholar]
- Vlachos, I.K.; Sergiadis, G.D. Subsethood, entropy, and cardinality for interval-valued fuzzy sets—An algebraic derivation. Fuzzy Sets Syst. 2007, 158, 1384–1396. [Google Scholar] [CrossRef]
- Burillo, P.; Bustince, H. Entropy on intuitionistic fuzzy sets and on interval-valued fuzzy sets. Fuzzy Sets Syst. 1996, 78, 305–316. [Google Scholar] [CrossRef]
- Wu, D.; Mendel, J.M. Uncertainty measures for interval type-2 fuzzy sets. Inf. Sci. 2007, 177, 5378–5393. [Google Scholar] [CrossRef]
- Greenfield, S. Uncertainty Measurement for the Interval Type-2 Fuzzy Set. In Proceedings of the International Conference on Artificial Intelligence and Soft Computing, Zakopane, Poland, 12–16 June 2016. [Google Scholar]
- Yager, R.R. An intuitionistic view of the Dempster–Shafer belief structure. Soft Comput. 2014, 18, 2091–2099. [Google Scholar] [CrossRef]
- Türkşen, I. Belief, plausibility, and probability measures on interval-valued type 2 fuzzy sets. Int. J. Intell. Syst. 2004, 19, 681–699. [Google Scholar] [CrossRef]
- Dymova, L.; Sevastjanov, P. The Definition of Interval-Valued Intuitionistic Fuzzy Sets in the Framework of Dempster-Shafer Theory. In Proceedings of the International Conference on Parallel Processing and Applied Mathematics, Warsaw, Poland, 8–11 September 2014. [Google Scholar]
- Yager, R.R. On Z-valuations using Zadeh’s Z-numbers. Int. J. Intell. Syst. 2012, 27, 259–278. [Google Scholar] [CrossRef]
- Song, Y.; Deng, Y. Entropic Explanation of Power Set. Int. J. Comput. Commun. Control 2021, 16, 4413. [Google Scholar] [CrossRef]
- Jousselme, A.L.; Liu, C.; Grenier, D.; Bosse, E. Measuring ambiguity in the evidence theory. IEEE Trans. Syst. Man Cybern. Part A Syst. Hum. 2006, 36, 890–903. [Google Scholar] [CrossRef]
- Yang, Y.; Han, D. A new distance-based total uncertainty measure in the theory of belief functions. Knowl.-Based Syst. 2016, 94, 114–123. [Google Scholar] [CrossRef]
- Zhang, H.; Deng, Y. Entropy Measure for Orderable Sets. Inf. Sci. 2021, 561, 141–151. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 623–656. [Google Scholar] [CrossRef]
- Tsallis, C. Possible generalization of Boltzmann-Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Klir, G.J.; Folger, T.A. Fuzzy sets, uncertainty and information. J. Oper. Res. Soc. 1990, 41. [Google Scholar] [CrossRef]
- Cao, Z.; Ding, W.; Wang, Y.K.; Hussain, F.; Al-Jumaily, A.; Lin, C.T. Effects of Repetitive SSVEPs on EEG Complexity using Multiscale Inherent Fuzzy Entropy. Neurocomputing 2019, 389, 198–206. [Google Scholar] [CrossRef] [Green Version]
- Tsallis, C. Nonadditive entropy: The concept and its use. Eur. Phys. J. A 2008, 40, 257–266. [Google Scholar] [CrossRef] [Green Version]
- Deng, Y. Deng entropy. Chaos Solitons Fractals Appl. Sci. Eng. Interdiscip. J. Nonlinear Sci. 2016, 91, 549–553. [Google Scholar] [CrossRef]
- Pan, L.; Deng, Y. A New Belief Entropy to Measure Uncertainty of Basic Probability Assignments Based on Belief Function and Plausibility Function. Entropy 2018, 20, 842. [Google Scholar] [CrossRef] [Green Version]
- Song, Y.; Wang, X.; Lei, L.; Yue, S. Uncertainty measure for interval-valued belief structures. Measurement 2016, 80, 241–250. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, Y.; Wu, X. Information fusion for probabilistic risk analysis in complex projects under uncertainty. Appl. Soft Comput. 2021, 104, 107189. [Google Scholar] [CrossRef]
- Chen, L.; Deng, Y.; Cheong, K.H. Probability transformation of mass function: A weighted network method based on the ordered visibility graph. Eng. Appl. Artif. Intell. 2021, 105, 104438. [Google Scholar] [CrossRef]
- Higashi, M.; Klir, G.J. Measures of uncertainty and information based on possibility distributions. Read. Fuzzy Sets Intell. Syst. 1993, 9, 217–232. [Google Scholar] [CrossRef]
- Yager, R.R. Entropy and specificity in a mathematical theory of evidence. In Classic Works of the Dempster-Shafer Theory of Belief Functions; Springer: Berlin/Heidelberg, Germany, 2008; pp. 291–310. [Google Scholar]
- Klir, G.J.; Ramer, A. Uncertainty in the Dempster-Shafer theory: A critical re-examination. Int. J. Gen. Syst. 1990, 18, 155–166. [Google Scholar] [CrossRef]
- George, T.; Pal, N.R. Quantification of conflict in Dempster-Shafer framework: A new approach. Int. J. Gen. Syst. 1996, 24, 407–423. [Google Scholar] [CrossRef]
- Xiao, F. Multi-sensor data fusion based on the belief divergence measure of evidences and the belief entropy. Inf. Fusion 2019, 46, 23–32. [Google Scholar] [CrossRef]
- Wang, X.; Song, Y. Uncertainty measure in evidence theory with its applications. Appl. Intell. 2017, 48, 1672–1688. [Google Scholar] [CrossRef]

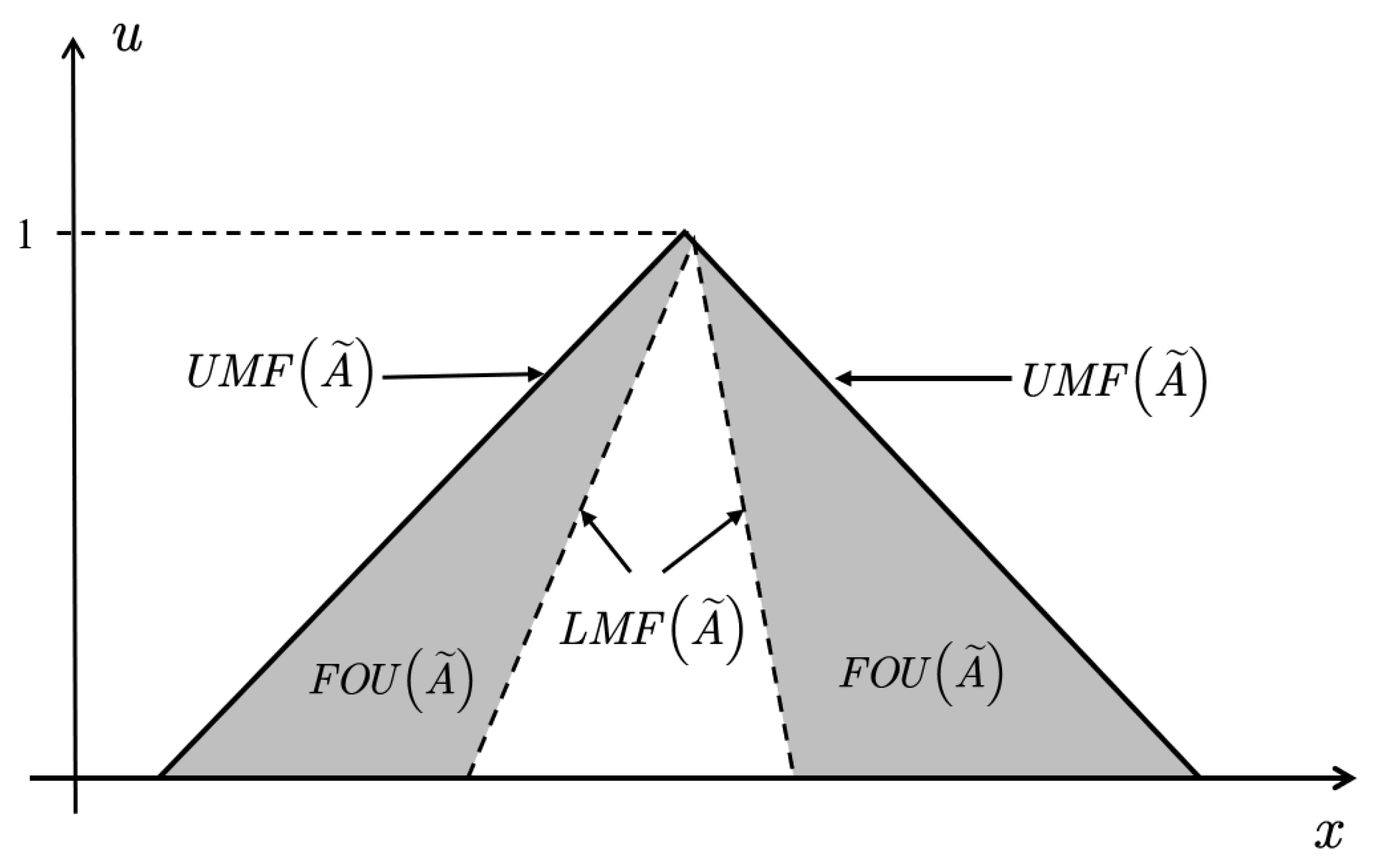
| Items | Uncertainty Expression |
|---|---|
| Hole’s UM [56] | |
| Yager’s UM [57] | |
| Dubois and Prade’s UM [58] | |
| Klir & Parviz’s strife [59] | |
| George & Pal’s conflict measure [60] | |
| Wang & Song’s UM [61] | |
| Deng entropy [51] | |
| Pan & Deng’s entropy [52] |
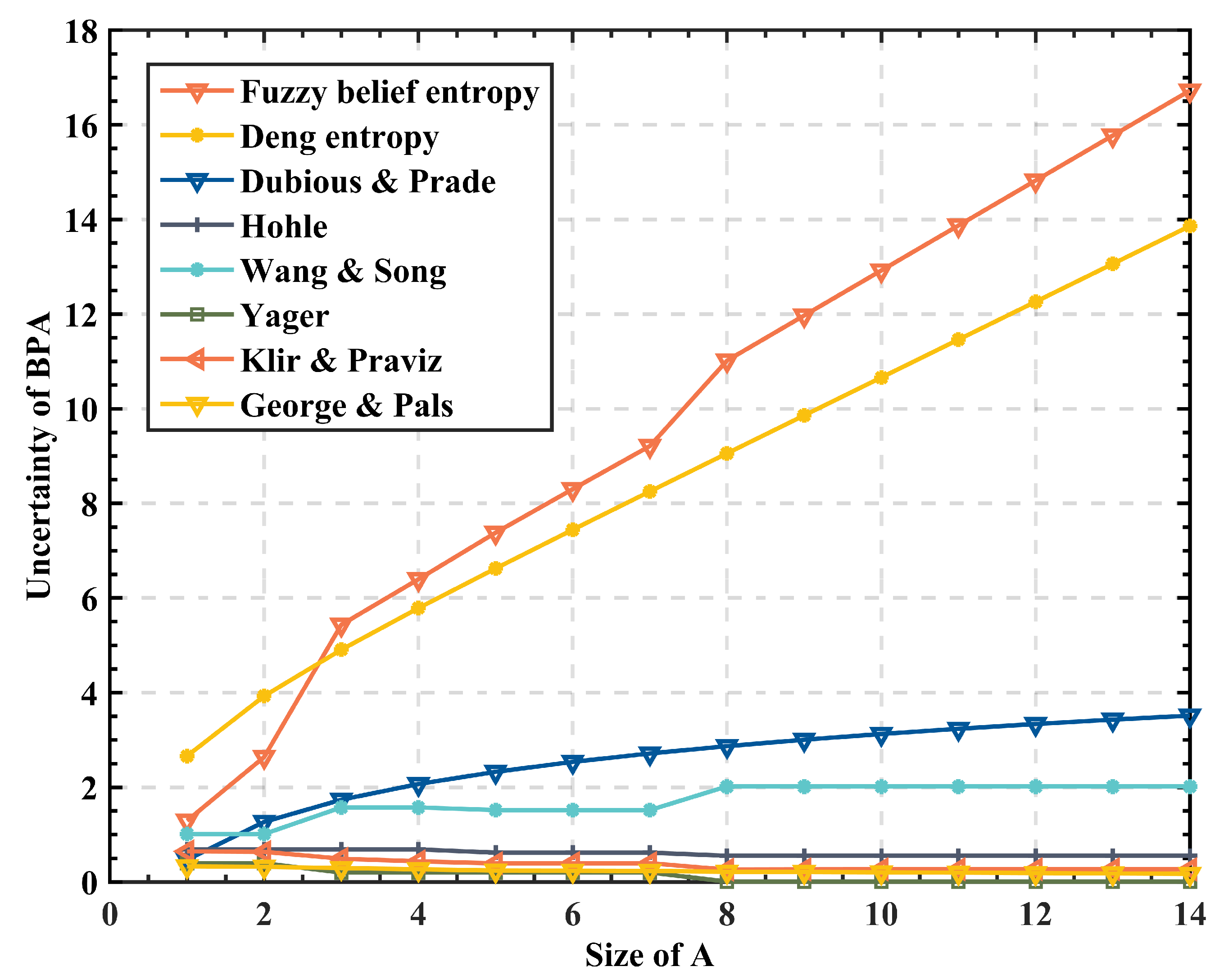
| Cases | Wang & Song [61] | Pan & Deng [52] | Fuzzy Belief Entropy |
|---|---|---|---|
| 1.8787 | 2.3176 | 2.7176 | |
| 1.8787 | 3.6648 | 4.0648 | |
| 1.8787 | 4.7038 | 5.1038 | |
| 1.8787 | 5.6384 | 6.0384 | |
| 1.8787 | 6.5286 | 6.9286 | |
| 1.8787 | 7.3983 | 7.7983 | |
| 1.8787 | 8.2580 | 8.6580 | |
| 1.8787 | 9.1128 | 9.5128 | |
| 1.8787 | 9.9652 | 10.3652 | |
| 1.8787 | 10.8164 | 11.2164 | |
| 1.8787 | 11.6670 | 12.0670 | |
| 1.8787 | 12.5173 | 12.9173 | |
| 1.8787 | 13.3674 | 13.7674 | |
| 1.8787 | 14.2175 | 14.6175 |
| Cases | Fuzzy Belief Entropy |
|---|---|
| 1.2944 | |
| 2.6416 | |
| 5.4359 | |
| 6.3980 | |
| 7.3814 | |
| 8.3022 | |
| 9.2125 | |
| 11.0186 | |
| 11.9713 | |
| 12.9226 | |
| 13.8733 | |
| 14.8236 | |
| 15.7738 | |
| 16.7239 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Cai, R. Uncertainty of Interval Type-2 Fuzzy Sets Based on Fuzzy Belief Entropy. Entropy 2021, 23, 1265. https://doi.org/10.3390/e23101265
Liu S, Cai R. Uncertainty of Interval Type-2 Fuzzy Sets Based on Fuzzy Belief Entropy. Entropy. 2021; 23(10):1265. https://doi.org/10.3390/e23101265
Chicago/Turabian StyleLiu, Sicong, and Rui Cai. 2021. "Uncertainty of Interval Type-2 Fuzzy Sets Based on Fuzzy Belief Entropy" Entropy 23, no. 10: 1265. https://doi.org/10.3390/e23101265
APA StyleLiu, S., & Cai, R. (2021). Uncertainty of Interval Type-2 Fuzzy Sets Based on Fuzzy Belief Entropy. Entropy, 23(10), 1265. https://doi.org/10.3390/e23101265






