Improving the Performance of Continuous-Variable Measurement-Device-Independent Quantum Key Distribution via a Noiseless Linear Amplifier
Abstract
:1. Introduction
2. The Scheme of CV-MDI-QKD with NLA
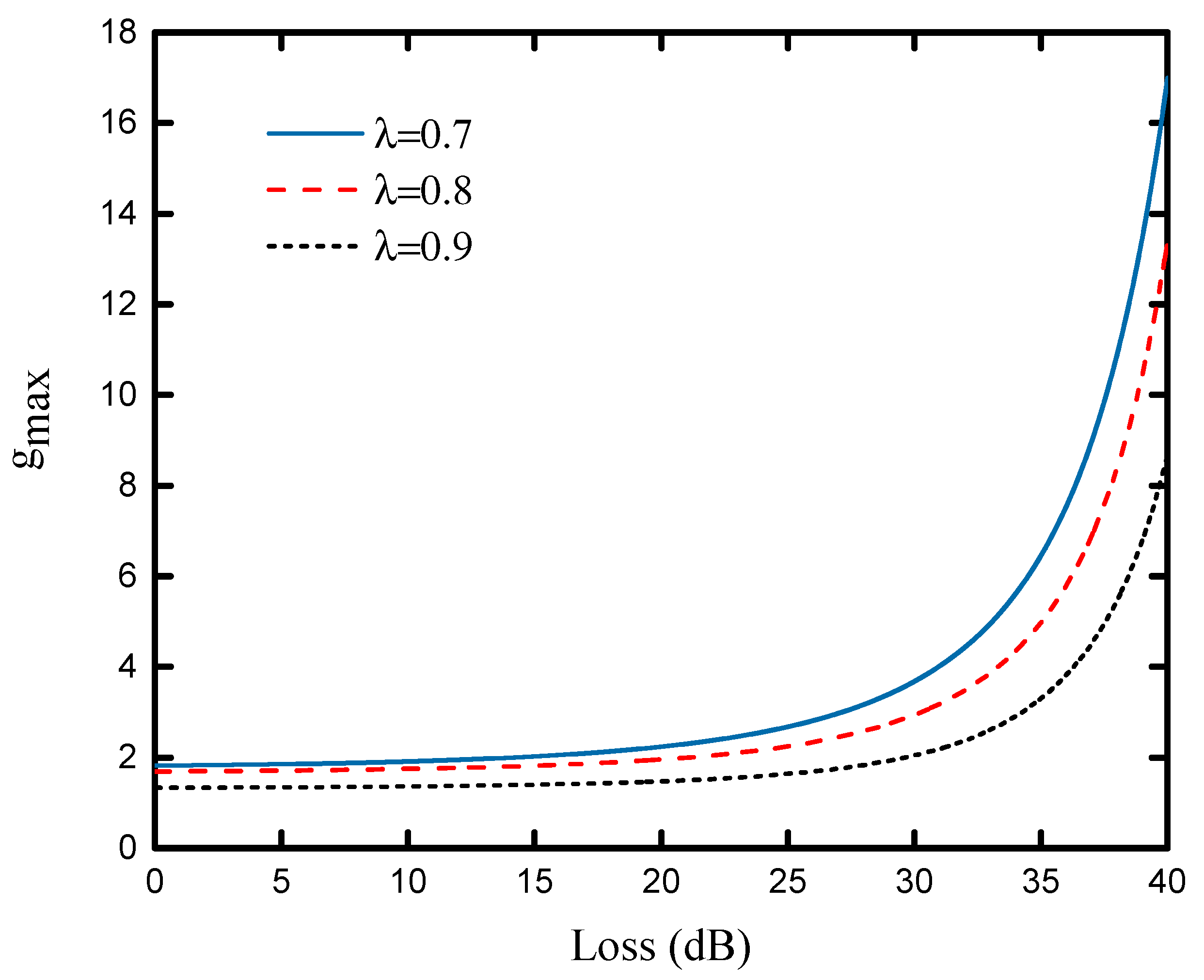
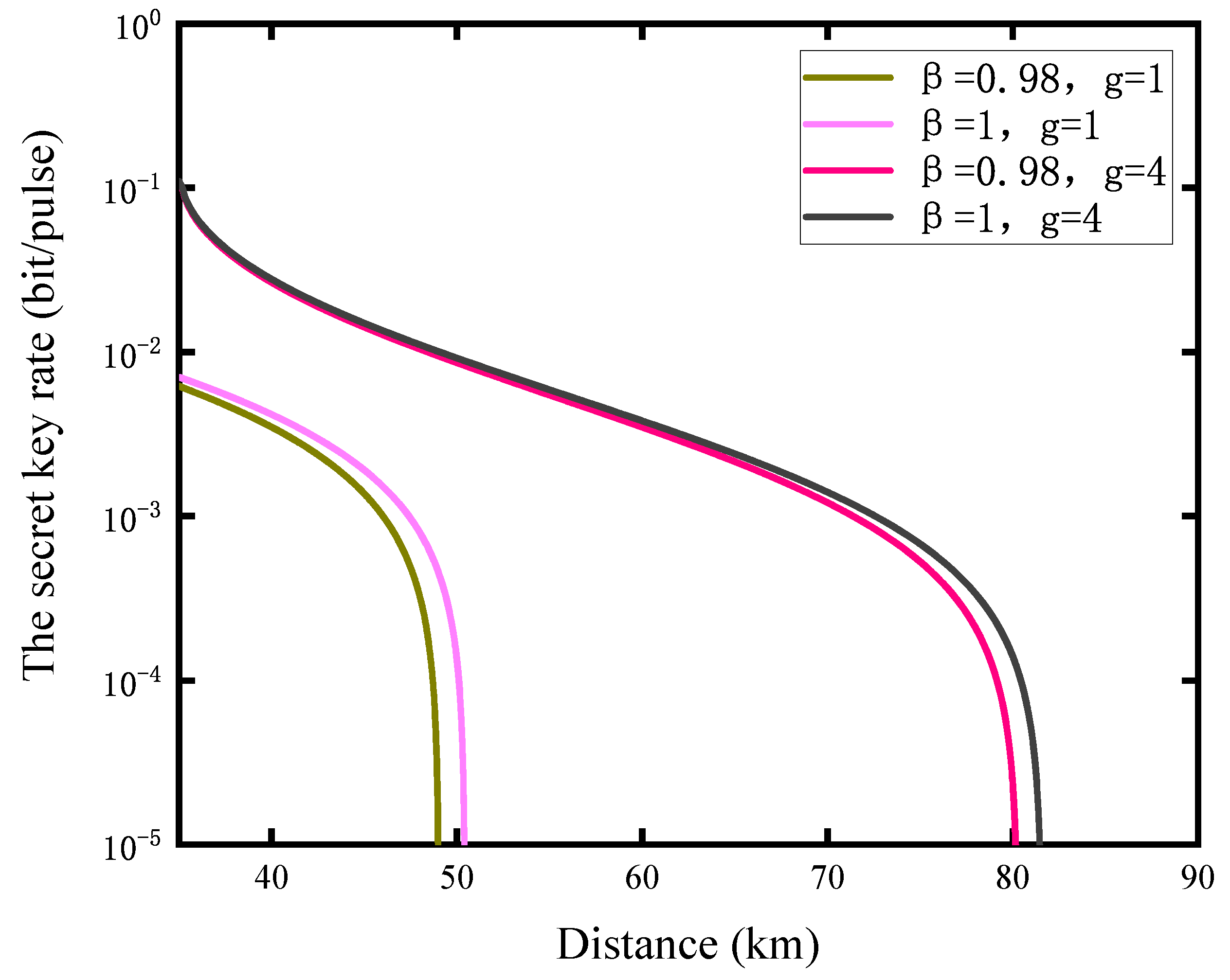
3. The Secret Key Rate of the CV-MDI-QKD System with NLA
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ghirardi, G.C.; Grassi, R.; Michelini, M. Thinking Physics for Teaching; Springer: Milan, Italy, 1995; pp. 329–334. [Google Scholar]
- Soujaeff, A.; Nishioka, T.; Hasegawa, T.; Takeuchi, S.; Matsui, M. Quantum key distribution at 1550 nm using a pulse heralded single photon source. Opt. Exp. 2007, 15, 726–734. [Google Scholar] [CrossRef] [PubMed]
- Scarani, V.; Bechmann-Pasquinucci, H.; Cerf, N.J. The security of practical quantum key distribution. Rev. Mod. Phys. 2009, 81, 1301. [Google Scholar] [CrossRef] [Green Version]
- Loeb, A.L. The Heisenberg Uncertainty Principle. Am. J. Phys. 1963, 12, 945. [Google Scholar] [CrossRef]
- Shokeir, H. Increasing the Bit Density of a Quantum-Confinement Physically Unclonable Function. Master’s Thesis, Master of Science, Lancaster University, Lancaster, UK, 2017. [Google Scholar]
- Goorden, S.A.; Horstmann, M.; Mosk, A.P.; Škorić, B.; Pinkse, P.W.H. Quantum-secure authentication of a physical unclonable key. Optica 2014, 1, 421–424. [Google Scholar] [CrossRef] [Green Version]
- Ekert, A.K. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 1991, 67, 661–663. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bennett, C.H.; Bessette, F.; Brassard, G.; Salvail, L.; Smolin, J.A. Experimental Quantum Cryptography. J. Cryptol. 1992, 5, 3–28. [Google Scholar] [CrossRef]
- Wang, J.Y.; Yang, B.; Liao, S.K.; Zhang, L.; Shen, Q.; Hu, X.F.; Wu, J.C.; Yang, S.J.; Jiang, H.; Tang, Y.L. Direct and full-scale experimental verifications towards ground-satellite quantum key distribution. Nat. Photonics 2013, 7, 387–393. [Google Scholar] [CrossRef]
- Chong, S.K.; Hwang, T. Quantum key agreement protocol based on BB84. Opt. Commun. 2010, 283, 1192–1195. [Google Scholar] [CrossRef]
- Walk, N.; Ralph, T.C.; Symul, T.; Ping, K.L. Security of Continuous Variable Quantum Cryptography. Phys. Rev. A 2013, 87, 20303. [Google Scholar] [CrossRef] [Green Version]
- Hillery, M. Quantum cryptography with squeezed states. Phys. Rev. A 1999, 61, 022309. [Google Scholar] [CrossRef] [Green Version]
- Grosshans, F.; Assche, G.V.; Wenger, J.; Brouri, R.; Cerf, N.J.; Grangier, P. Quantum key distribution using gaussian-modulated coherent states. Nature 2003, 421, 238–241. [Google Scholar] [CrossRef] [Green Version]
- Grosshans, F.; Grangier, P. Continuous Variable Quantum Cryptography Using Coherent States. Phys. Rev. Lett. 2002, 88, 057902. [Google Scholar] [CrossRef] [Green Version]
- Weedbrook, C.; Pirandola, S.; García-Patrón, R.; Cerf, N.; Ralph, T.; Shapiro, J.; Lloyd, S. Gaussian quantum information. Rev. Mod. Phys. 2011, 84, 621–669. [Google Scholar] [CrossRef]
- Berta, M.; Christandl, M.; Colbeck, R. The uncertainty principle in the presence of quantum memory. Nat. Phys. 2010, 6, 659–662. [Google Scholar] [CrossRef]
- Jain, N.; Stiller, B.; Khan, I.; Makarov, V.; Marquardt, C.; Leuchs, G. Unconditional security of the Bennett 1992 quantum key-distribution scheme with strong reference pulse. Phys. Rev. A 2009, 80, 84–85. [Google Scholar]
- Renner, R.; Cirac, J.I. de Finetti Representation Theorem for Infinite-Dimensional Quantum Systems and Applications to Quantum Cryptography. Phys. Rev. Lett. 2009, 102, 110504. [Google Scholar] [CrossRef] [Green Version]
- Leverrier, A. Security of continuous-variable quantum key distribution against general attacks. Phys. Rev. Lett. 2013, 110, 030502. [Google Scholar] [CrossRef] [Green Version]
- Leverrier, A. Composable security proof for continuous-variable quantum key distribution with coherent states. Phys. Rev. Lett. 2015, 114, 7. [Google Scholar] [CrossRef] [Green Version]
- Leverrier, A. Security of Continuous-Variable Quantum Key Distribution via a Gaussian de Finetti Reduction. Phys. Rev. Lett. 2017, 118, 200501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, W.Q.; Peng, J.Y.; Peng, H.; Duan, H.; Zeng, G.H. Monitoring of continuous-variable quantum key distribution system in real environment. Opt. Exp. 2017, 25, 19429. [Google Scholar] [CrossRef]
- Bugge, A.N.; Sauge, S.; Ghazali, A.M.M.; Skaar, J.; Lydersen, L.; Makarov, V. Laser Damage Helps the Eavesdropper in Quantum Cryptography. Phys. Rev. Lett. 2014, 112, 070503. [Google Scholar] [CrossRef] [Green Version]
- Huang, A.Q.; Navarrete, Á.; Sun, S.H.; Chaiwongkhot, P.; Curty, M.; Makarov, V. Laser-Seeding Attack in Quantum Key Distribution. Phys. Rev. Appl. 2019, 12, 064043. [Google Scholar] [CrossRef] [Green Version]
- Huang, A.Q.; Li, R.; Egorov, V.; Tchouragoulov, S.; Kumar, K.; Makarov, V. Laser-Damage Attack Against Optical Attenuators in Quantum Key Distribution. Phys. Rev. Appl. 2020, 13, 034017. [Google Scholar] [CrossRef] [Green Version]
- Jouguet, P.; Kunz-Jacques, S.; Diamanti, E. Preventing calibration attacks on the local oscillator in continuous-variable quantum key distribution. Phys. Rev. A 2013, 87, 062313. [Google Scholar] [CrossRef] [Green Version]
- Ma, X.C.; Sun, S.H.; Jiang, M.S.; Liang, L.M. Local oscillator fluctuation opens a loophole for Eve in practical continuous-variable quantum-key-distribution systems. Phys. Rev. A 2013, 88, 022339. [Google Scholar] [CrossRef] [Green Version]
- Ma, X.C.; Sun, S.H.; Jiang, M.S.; Liang, L.M. Wavelength attack on practical continuous-variable quantum-key-distribution system with a heterodyne protocol. Phys. Rev. A 2013, 87, 052309. [Google Scholar] [CrossRef] [Green Version]
- Huang, J.Z.; Weedbrook, C.; Yin, Z.Q.; Wang, S.; Li, H.W.; Chen, W.; Guo, G.C.; Han, Z.F. Quantum hacking of a continuous-variable quantum-key-distribution system using a wavelength attack. Phys. Rev. A 2013, 87, 062329. [Google Scholar] [CrossRef] [Green Version]
- Kunz-Jacques, S.; Jouguet, P. Robust shot-noise measurement for continuous-variable quantum key distribution. Phys. Rev. A 2015, 91, 022307. [Google Scholar] [CrossRef] [Green Version]
- Liu, W.Q.; Peng, J.Y.; Qi, J.; Cao, Z.W.; He, C. Imperfect basis choice in continuous-variable quantum key distribution. Laser Phys. Lett. 2020, 17, 055203. [Google Scholar] [CrossRef]
- Cives-Esclop, A.; Luis, A.; Sánchez-Soto, L.L. Unbalanced homodyne detection with a weak local oscillator. Opt. Commun. 2000, 175, 153–161. [Google Scholar] [CrossRef]
- Kühn, B.; Vogel, W. Unbalanced Homodyne Correlation Measurements. Phys. Rev. Lett. 2016, 116, 163603. [Google Scholar] [CrossRef] [Green Version]
- Huang, Y.; Zhang, Y.; Xu, B.; Huang, L.; Yu, S. A modified practical homodyne detector model for continuous-variable quantum key distribution: Detailed security analysis and improvement by the phase-sensitive amplifier. J. Phys. B-At. Mol. Opt. Phys. 2020, 54, 015503. [Google Scholar] [CrossRef]
- Almeida, M.; Pereira, D.; Facão, M.; Pinto, A.N.; Silva, N.A. Impact of imperfect homodyne detection on measurements of vacuum states shot noise. Opt. Quantum Electron. 2020, 52, 503. [Google Scholar] [CrossRef]
- Wallentowitz, S.; Vogel, W. Unbalanced homodyning for quantum state measurements. Phys. Rev. A 1996, 53, 4528. [Google Scholar] [CrossRef]
- Silva, N.A.; Pereira, D.; Muga, N.J.; Pinto, A.N. Practical imperfections affecting the performance of CV-QKD based on coherent detection. In Proceedings of the 2020 22nd International Conference on Transparent Optical Networks (ICTON), Bari, Italy, 19–23 July 2020; pp. 1–4. [Google Scholar]
- Li, Z.; Zhang, Y.; Xu, F.; Peng, X.; Guo, H. Continuous-variable measurement-device-independent quantum key distribution. Phys. Rev. A 2014, 89, 052301. [Google Scholar] [CrossRef] [Green Version]
- Papanastasiou, P.; Ottaviani, C.; Pirandola, S. Finite-size analysis of measurement-device-independent quantum cryptography with continuous variables. Phys. Rev. A 2017, 96, 4. [Google Scholar] [CrossRef] [Green Version]
- Tang, Z.; Liao, Z.; Xu, F.; Qi, B.; Qian, L.; Lo, H.K. Experimental demonstration of polarization encoding measurement-device-independent quantum key distribution. Phys. Rev. Lett. 2014, 112, 190503. [Google Scholar] [CrossRef] [Green Version]
- Ralph, T.C.; Lund, A.P. Nondeterministic noiseless linear amplification of quantum systems. Am. Inst. Phys. 2009, 1110, 155–160. [Google Scholar]
- Blandino, R.; Leverrier, A.; Barbieri, M.; Etesse, J.; Tualle-Brouri, R. Improving the maximum transmission distance of continuous-variable quantum key distribution using a noiseless amplifier. Phys. Rev. A 2014, 86, 1. [Google Scholar]
- Levenson, J.A.; Abram, I.; Rivera, T.; Grangier, P. Reduction of quantum noise in optical parametric amplification. JOSA B 1993, 10, 2233–2238. [Google Scholar] [CrossRef]
- Ralph, T.C. Quantum error correction of continuous-variable states against gaussian noise. Phys. Rev. A 2011, 84, 022339. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Huang, P.; Li, D.; Zhou, Y.; Zeng, G. Security analysis of practical continuous-variable quantum key distribution using a heralded noiseless amplifier. Int. J. Theor. Phys. 2019, 58, 2392–2406. [Google Scholar] [CrossRef]
- Leverrier, A.; Grosshans, F.; Grangier, P. Finite-size analysis of a continuous-variable quantum key distribution. Phys. Rev. A 2010, 81, 062343. [Google Scholar] [CrossRef] [Green Version]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, F.; Liu, W.; Kong, L.; He, C. Improving the Performance of Continuous-Variable Measurement-Device-Independent Quantum Key Distribution via a Noiseless Linear Amplifier. Entropy 2021, 23, 1691. https://doi.org/10.3390/e23121691
Jing F, Liu W, Kong L, He C. Improving the Performance of Continuous-Variable Measurement-Device-Independent Quantum Key Distribution via a Noiseless Linear Amplifier. Entropy. 2021; 23(12):1691. https://doi.org/10.3390/e23121691
Chicago/Turabian StyleJing, Fan, Weiqi Liu, Lingzhi Kong, and Chen He. 2021. "Improving the Performance of Continuous-Variable Measurement-Device-Independent Quantum Key Distribution via a Noiseless Linear Amplifier" Entropy 23, no. 12: 1691. https://doi.org/10.3390/e23121691
APA StyleJing, F., Liu, W., Kong, L., & He, C. (2021). Improving the Performance of Continuous-Variable Measurement-Device-Independent Quantum Key Distribution via a Noiseless Linear Amplifier. Entropy, 23(12), 1691. https://doi.org/10.3390/e23121691





