Performance Optimizations with Single-, Bi-, Tri-, and Quadru-Objective for Irreversible Diesel Cycle
Abstract
:1. Introduction
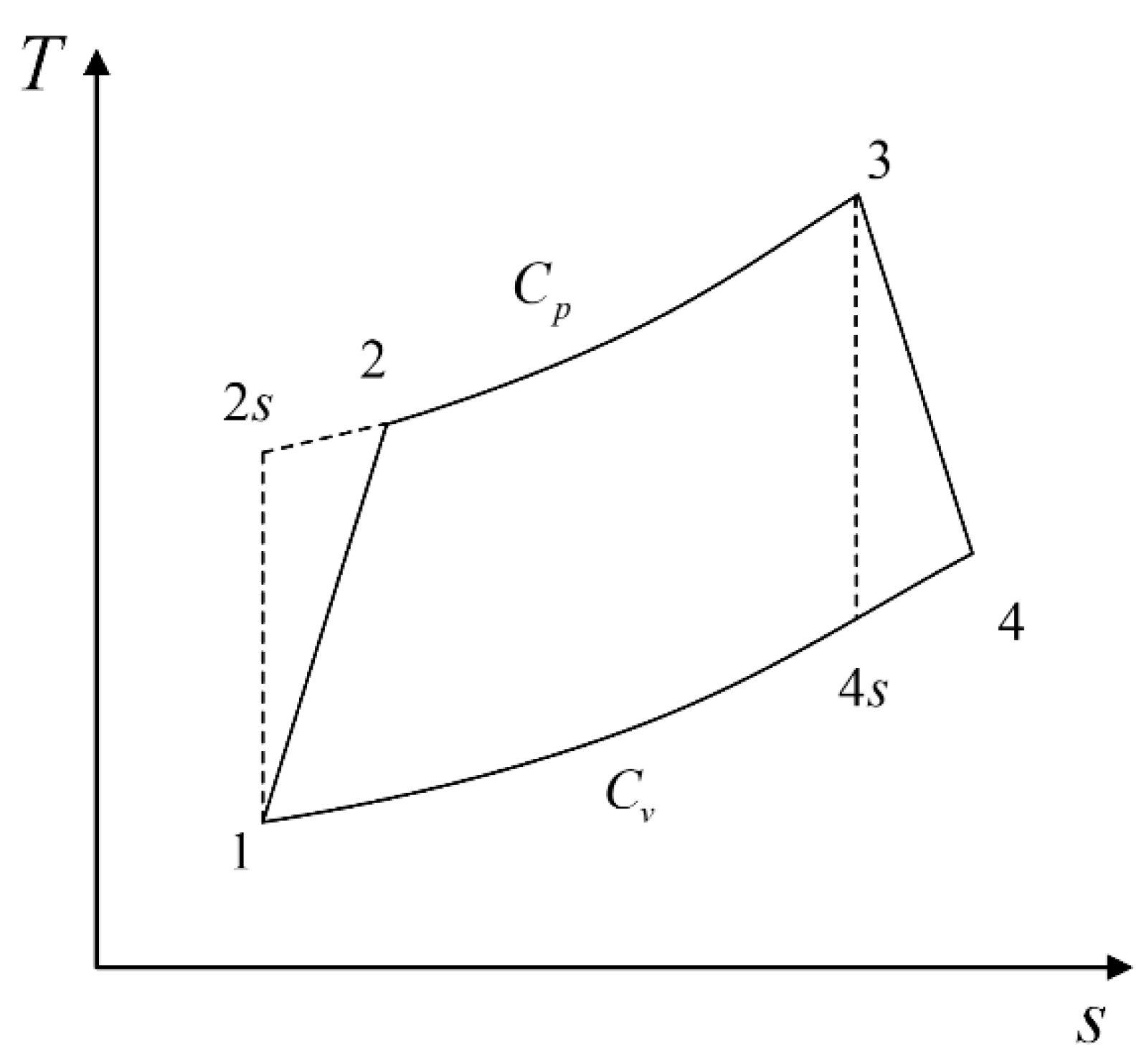
2. Cycle Model
3. Maximum Power Density Optimization
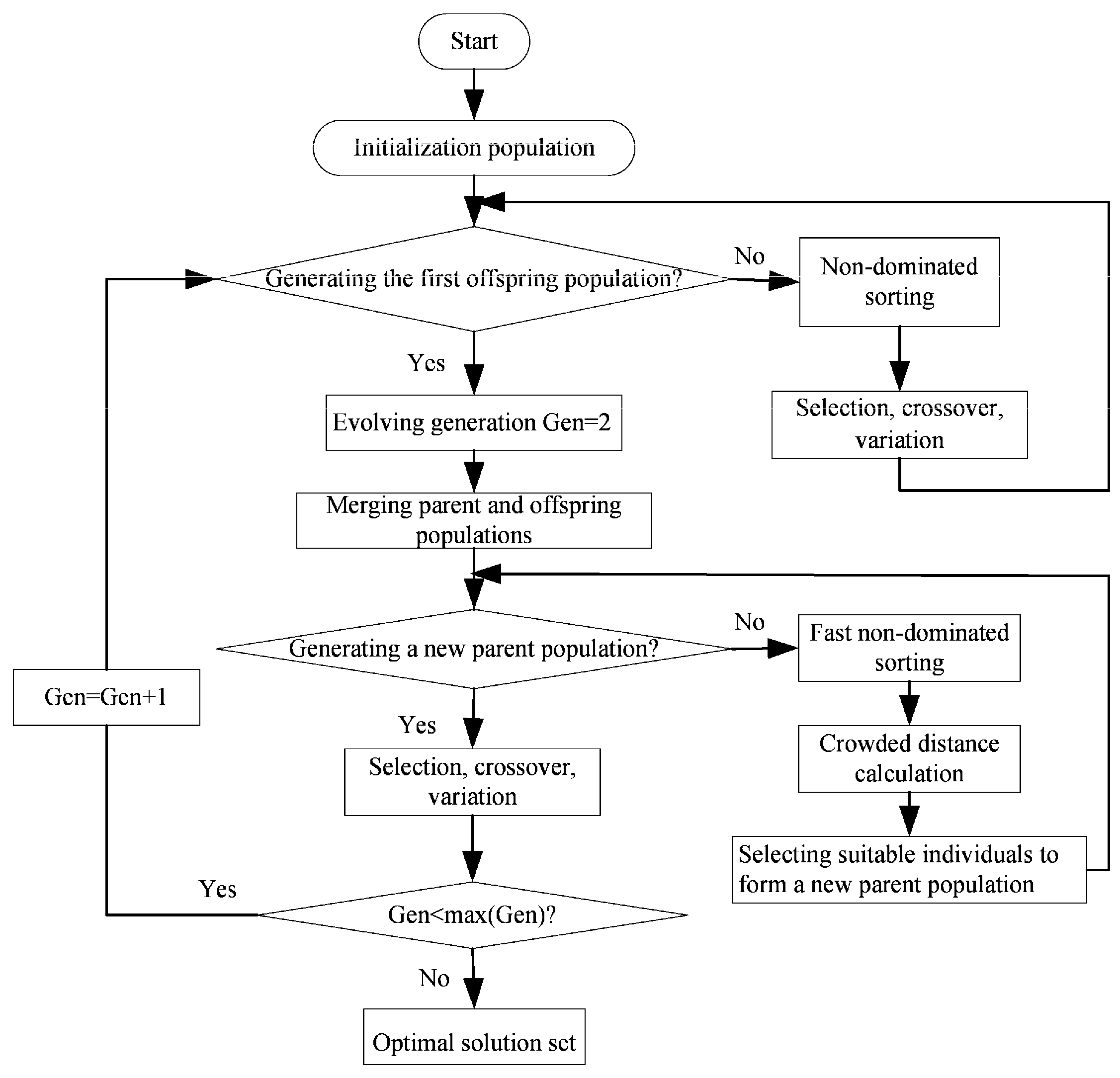
4. Multi-Objective Optimization with Power Output, Thermal Efficiency, Ecological Function, and Power Density
5. Conclusions
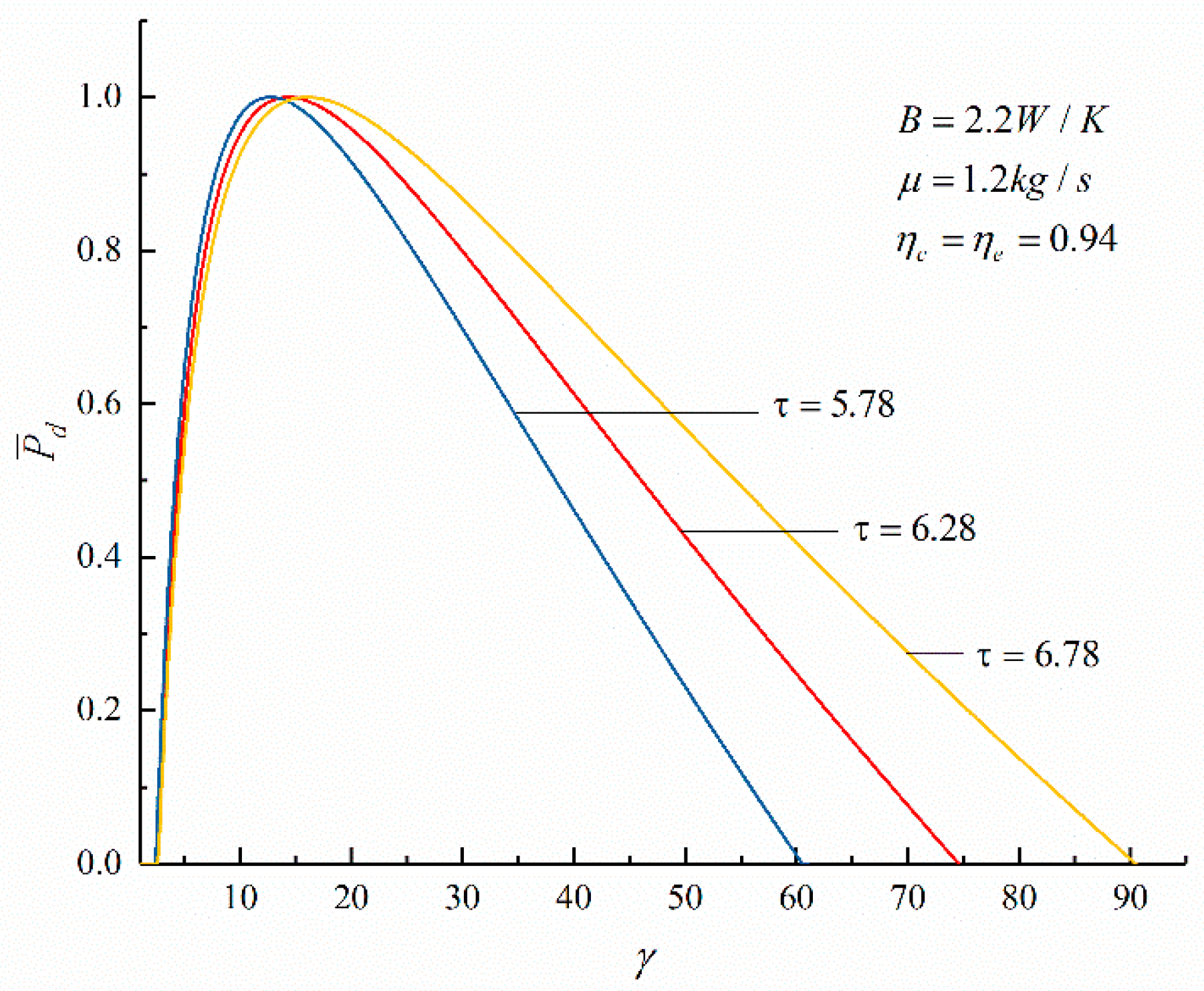
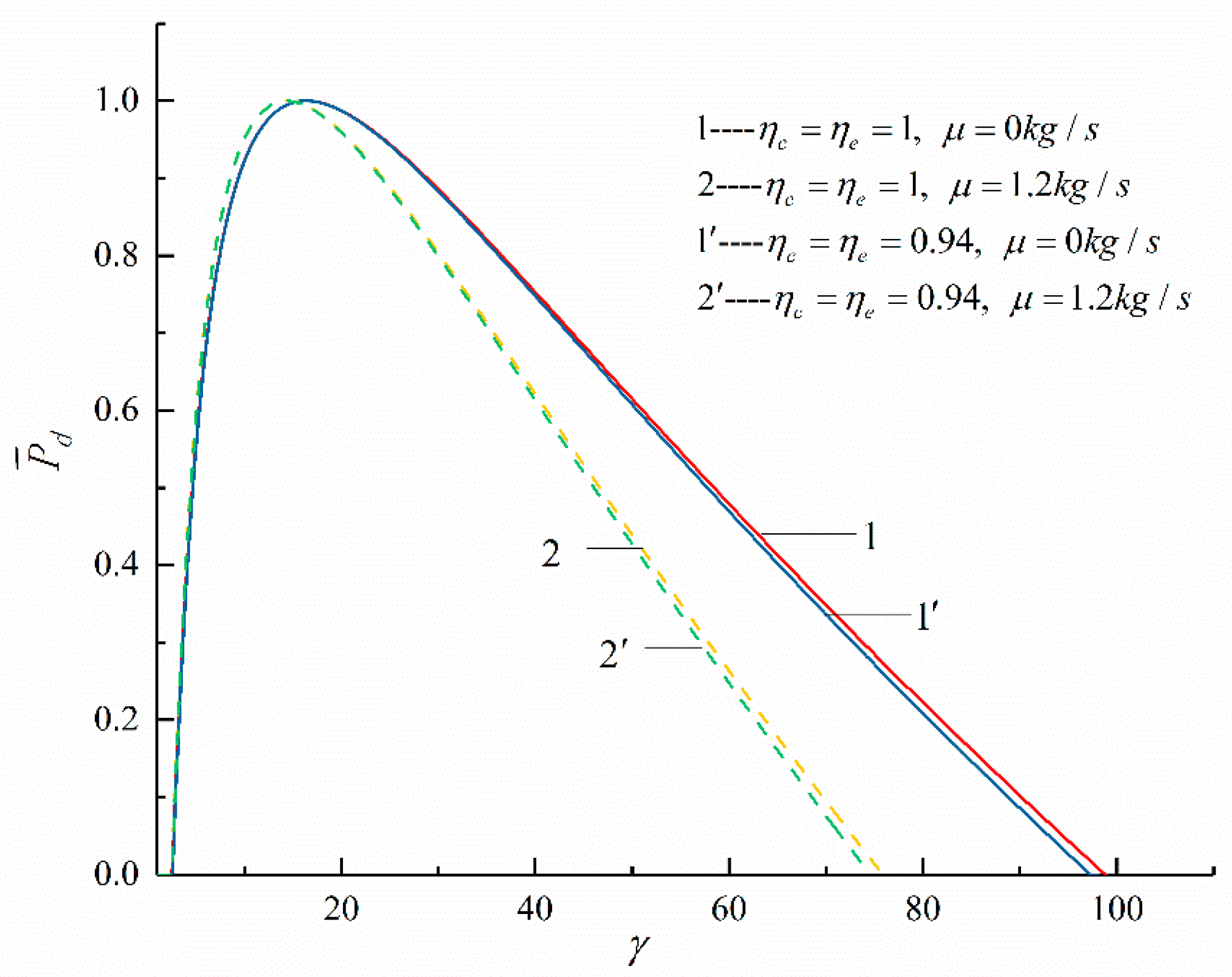
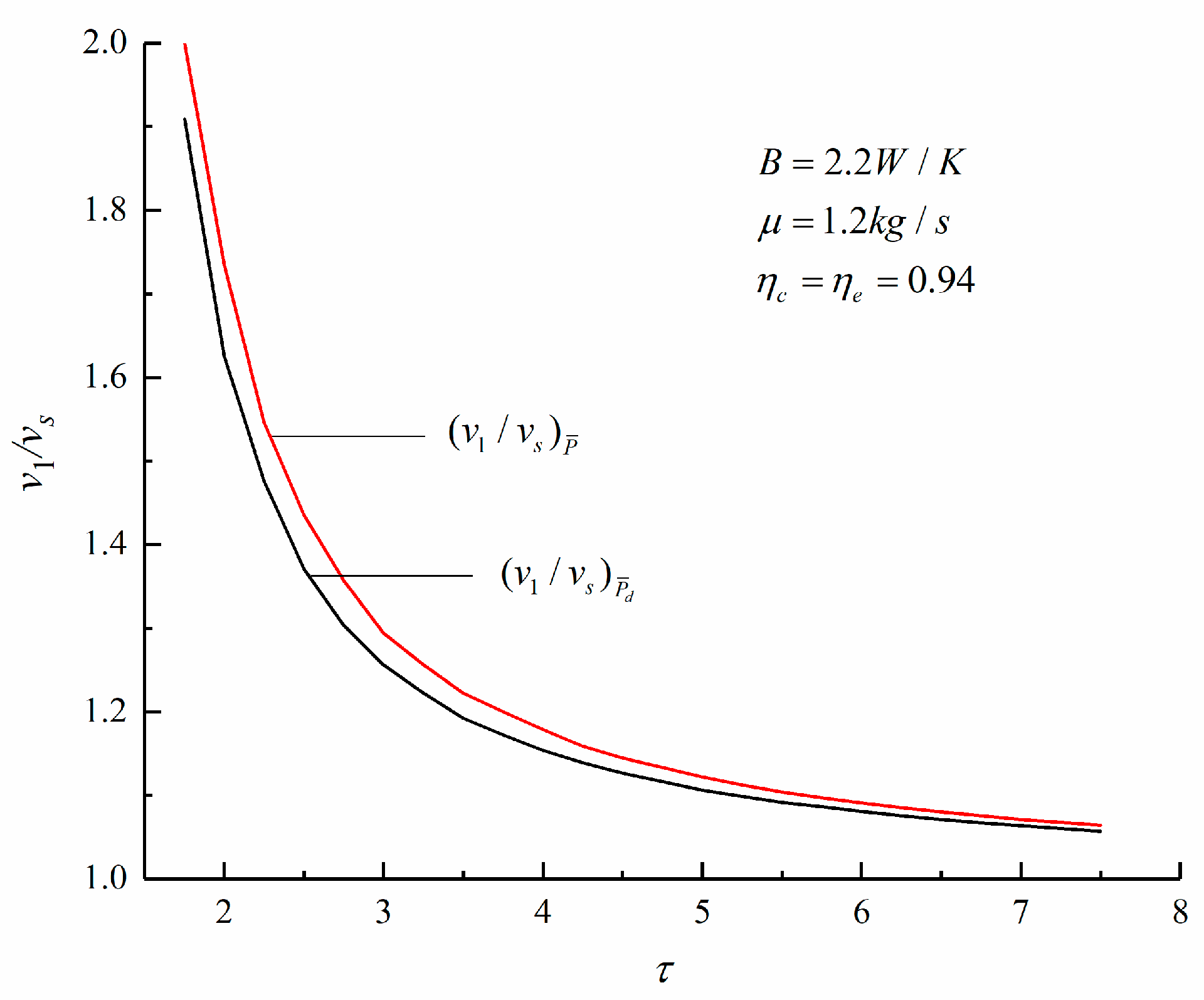
- The relationship curves of the cycles and were a parabolic-like one and a loop-shaped one, respectively. With the increases in the cycle temperature ratio, the and corresponding to the maximum increased. With the increases in HFL, FL, and IIL, the and corresponding to the maximum decreased.
- Under the maximum criterion, a smaller size and higher efficiency engine will be designed.
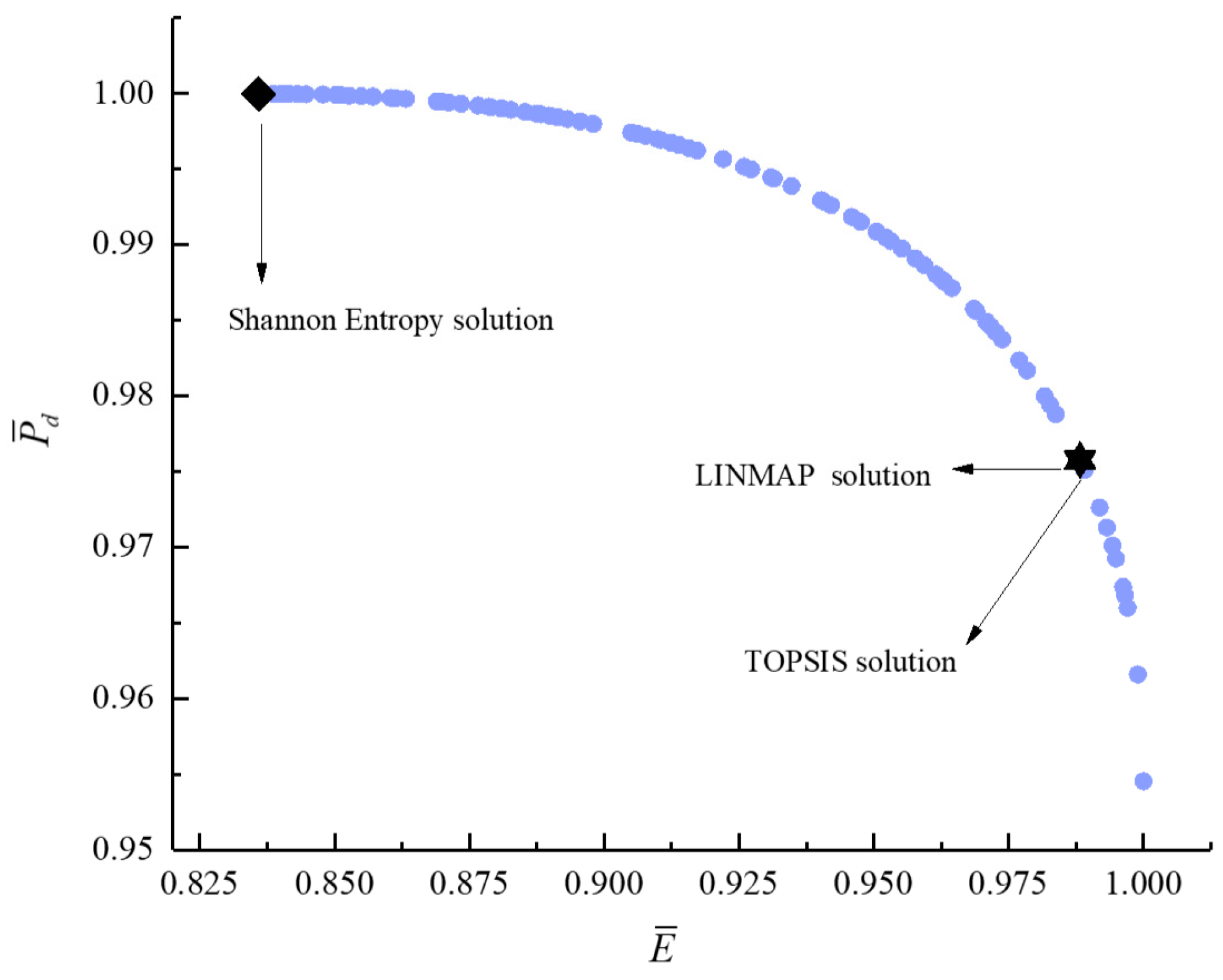
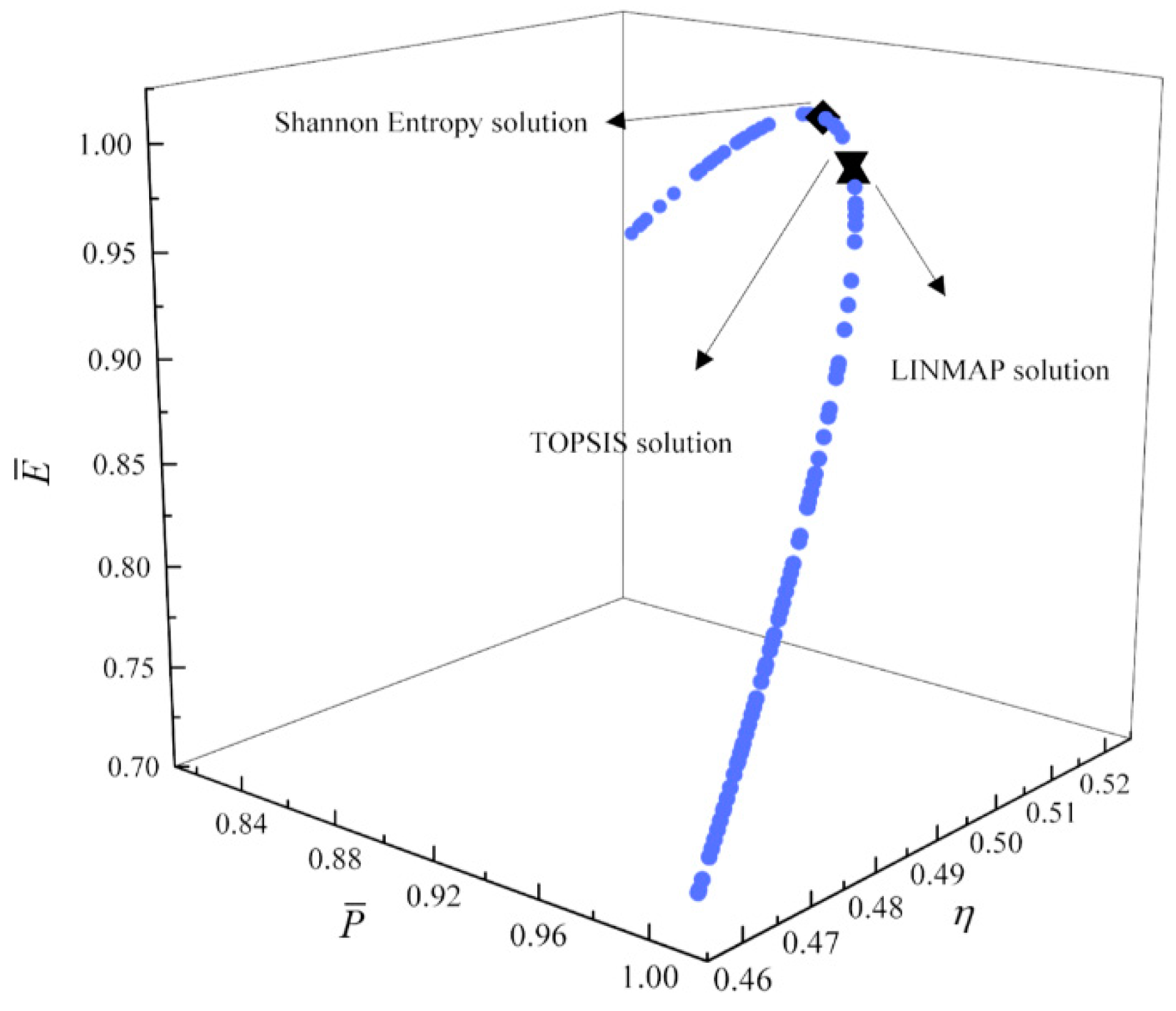
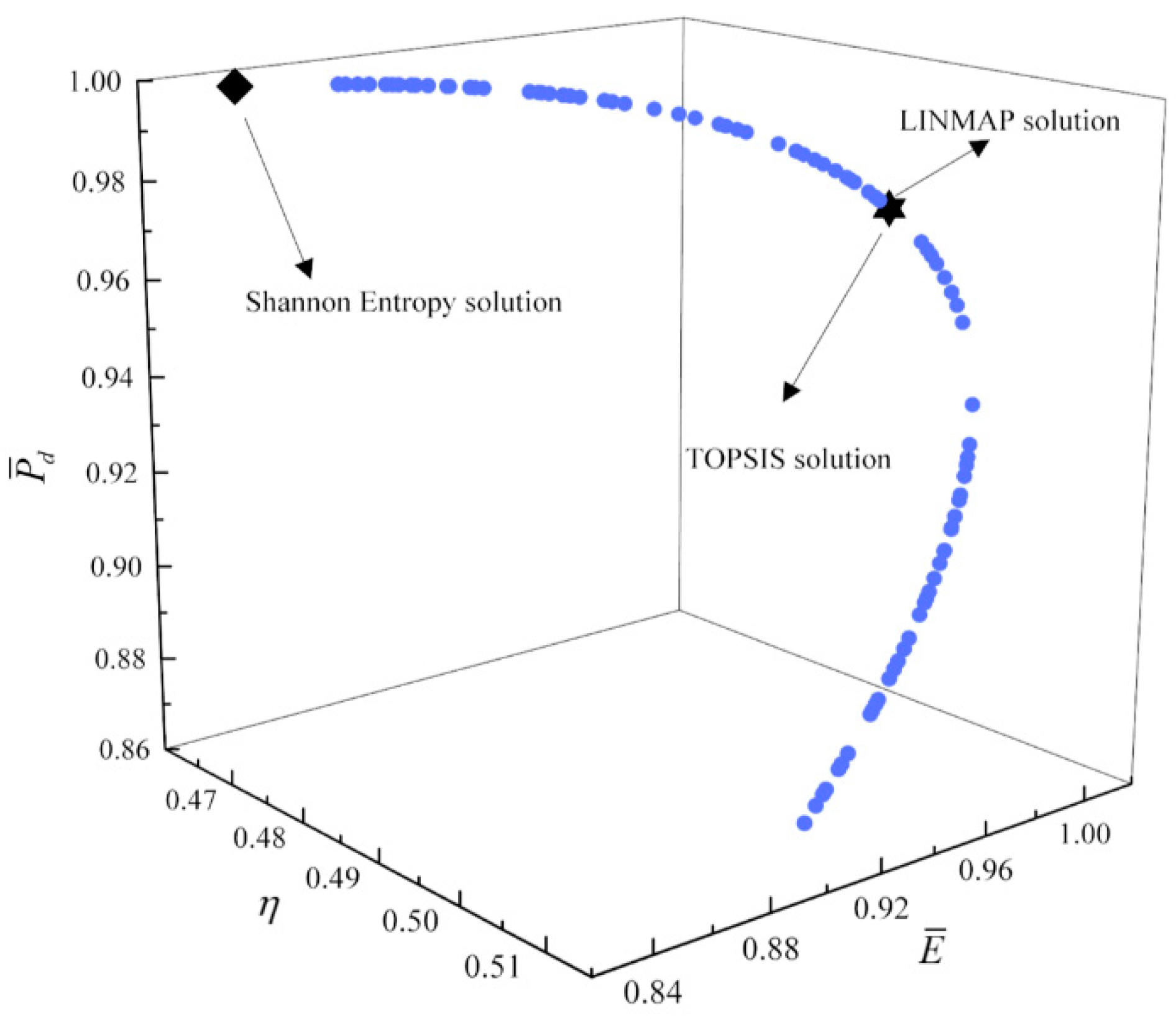
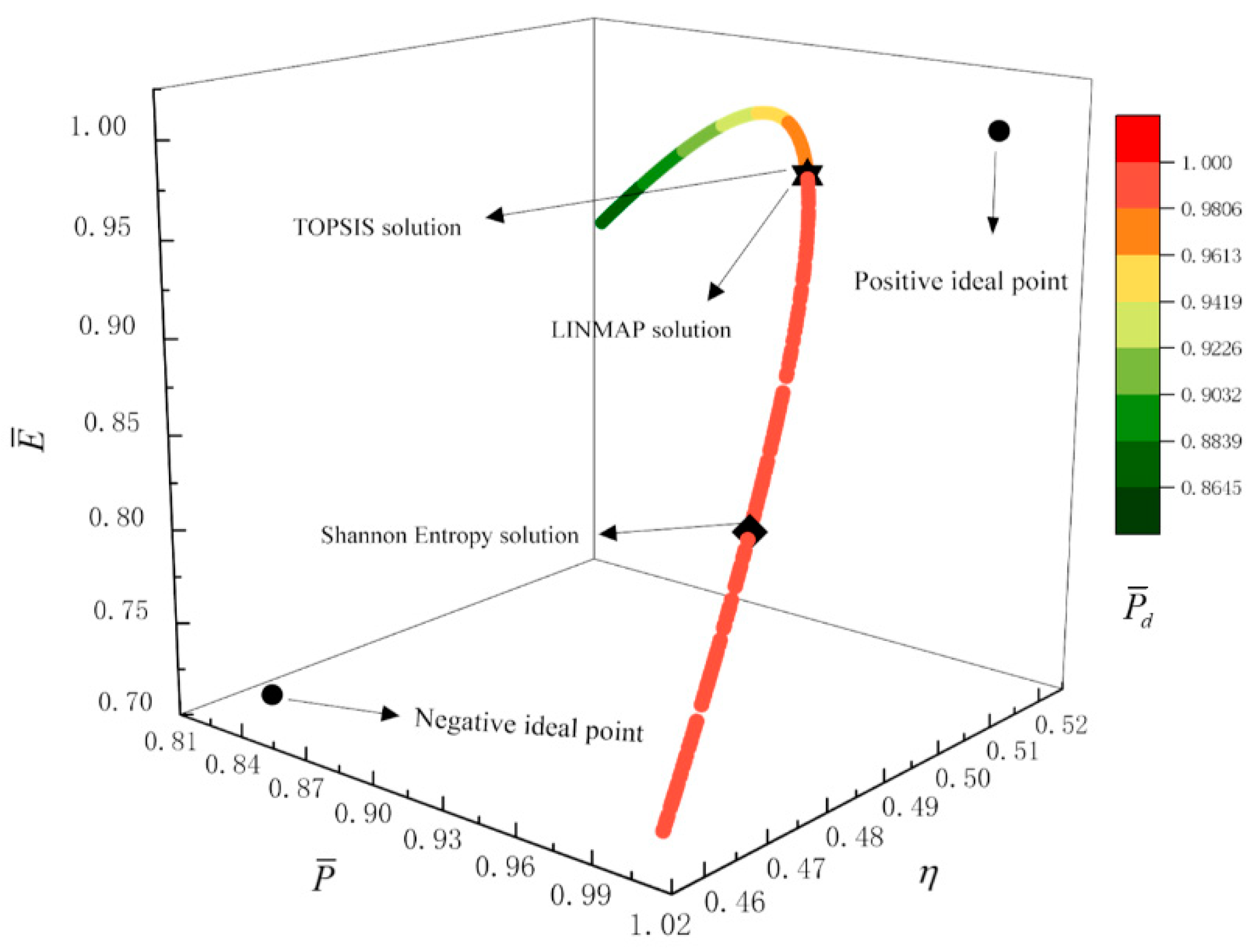
- The deviation index of MOO was smaller. When taking , , and as the optimization objectives to perform tri-objective optimization, the deviation index obtained by the LINMAP solution was smaller, and the design scheme was closer to the ideal scheme.
- The next step will be to use exergy efficiency optimization to further reinforce the results of MOO.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Heat transfer loss coefficient () | |
| Specific heat at constant pressure () | |
| Specific heat at constant volume () | |
| Dimensionless ecological function | |
| Dimensionless power output | |
| Dimensionless power density | |
| Heat transfer rate () | |
| T | Temperature () |
| Greek symbols | |
| Compression ratio (-) | |
| Thermal efficiency (-) | |
| Friction coefficient () | |
| Entropy generation rate () | |
| Temperature ratio (-) | |
| Subscripts | |
| Max power density condition | |
| Environment | |
| 1 − 4,, | Cycle state points |
| Abbreviations | |
| FL | Friction loss |
| HTL | Heat transfer loss |
| IIL | Internal irreversibility loss |
| MOO | Multi-objective optimization |
References
- Andresen, B.; Berry, R.S.; Ondrechen, M.J.; Salamon, P. Thermodynamics for processes in finite time. Acc. Chem. Res. 1984, 17, 266–271. [Google Scholar] [CrossRef]
- Chen, L.G.; Wu, C.; Sun, F.R. Finite time thermodynamic optimization or entropy generation minimization of energy systems. J. Non Equilib. Thermodyn. 1999, 24, 327–359. [Google Scholar] [CrossRef]
- Andresen, B. Current trends in finite-time thermodynamics. Angew. Chem. Int. Ed. 2011, 50, 2690–2704. [Google Scholar] [CrossRef]
- Feidt, M. Reconsideration of criteria and modeling in order to optimize the efficiency of irreversible thermomechanical heat engines. Entropy 2010, 12, 2470–2484. [Google Scholar] [CrossRef]
- Dong, Y.; El-Bakkali, A.; Descombes, G.; Feidt, M.; Périlhon, C. Association of finite-time thermodynamics and a bond-graph approach for modeling an endoreversible heat engine. Entropy 2012, 14, 642–653. [Google Scholar] [CrossRef] [Green Version]
- Ponmurugan, M. Attainability of maximum work and the reversible efficiency of minimally nonlinear irreversible heat engines. J. Non Equilib. Thermodyn. 2019, 44, 143–153. [Google Scholar] [CrossRef] [Green Version]
- Dai, D.D.; Liu, Z.C.; Long, R.; Yuan, F.; Liu, W. An irreversible Stirling cycle with temperature difference both in non-isothermal and isochoric processes. Energy 2019, 186, 115875. [Google Scholar] [CrossRef]
- Chen, L.G.; Feng, H.J.; Ge, Y.L. Power and efficiency optimization for open combined regenerative Brayton and inverse Brayton cycles with regeneration before the inverse cycle. Entropy 2020, 22, 677. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.F.; Li, Y.; Dong, H. Simulating finite-time isothermal processes with superconducting quantum circuits. Entropy 2021, 23, 353. [Google Scholar] [CrossRef] [PubMed]
- Dobre, C.; Lavinia, G.L.; Alexandru, D.A.; Chisiu, G.; Constantin, M. Stirling refrigerating machine modeling using Schmidt and finite physical dimensions thermodynamic models: A comparison with experiments. Entropy 2021, 23, 368. [Google Scholar] [CrossRef] [PubMed]
- Wang, R.B.; Ge, Y.L.; Chen, L.G.; Feng, H.J.; Wu, Z.X. Power and thermal efficiency optimization of an irreversible steady flow Lenoir cycle. Entropy 2021, 23, 425. [Google Scholar] [CrossRef]
- Valencia-Ortega, G.; Levario-Medina, S.; Barranco-Jiménez, M.A. Local and global stability analysis of a Curzon-Ahlborn model applied to power plants working at maximum k-efficient power. Phys. A Stat. Mech. Appl. 2021, 571, 125863. [Google Scholar] [CrossRef]
- Berry, R.S.; Salamon, P.; Andresen, B. How it all began. Entropy 2020, 22, 908. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.G.; Zen, F.M.; Sun, F.R.; Wu, C. Heat transfer effects on the net work output and power as function of efficiency for air standard Diesel cycle. Energy 1996, 21, 1201–1205. [Google Scholar] [CrossRef]
- Parlak, A. The effect of heat transfer on performance of the Diesel cycle and exergy of the exhaust gas stream in a LHR Diesel engine at the optimum injection timing. Energy Convers. Manag. 2005, 46, 167–179. [Google Scholar] [CrossRef]
- Parlak, A. Comparative performance analysis of irreversible Dual and Diesel cycles under maximum power conditions. Energy Convers. Manag. 2005, 46, 351–359. [Google Scholar] [CrossRef]
- Zhao, Y.R.; Lin, B.H.; Zhang, Y.; Chen, J.C. Performance analysis and parametric optimum design of an irreversible Diesel heat engine. Energy Convers. Manag. 2006, 47, 3383–3392. [Google Scholar] [CrossRef]
- Al-Hinti, I.; Akash, B.; Abu-Nada, E.; Al-Sarkhi, A. Performance analysis of air-standard Diesel cycle using an alternative irreversible heat transfer approach. Energy Convers. Manag. 2008, 49, 3301–3304. [Google Scholar] [CrossRef]
- Ebrahimi, R. Performance optimization of a Diesel cycle with specific heat ratio. J. Am. Sci. 2009, 5, 59–63. [Google Scholar]
- Zheng, S.Y.; Lin, G.X. Optimization of power and efficiency for an irreversible Diesel heat engine. Front. Energy Power Eng. China 2010, 4, 560–565. [Google Scholar] [CrossRef]
- Rashidi, M.M.; Hajipour, A. Comparison of performance of air-standard Atkinson, Diesel and Otto cycles with constant specific heats. Int. J. Adv. Des. Manuf. Technol. 2013, 6, 57–62. [Google Scholar]
- Zhu, F.L.; Chen, L.G.; Wang, W.H. Thermodynamic analysis and optimization of irreversible Maisotsenko-Diesel cycle. Int. J. Therm. Sci. 2019, 28, 659–668. [Google Scholar] [CrossRef]
- Wu, H.; Ge, Y.L.; Chen, L.G.; Feng, H.J. Power, efficiency, ecological function and ecological coefficient of performance optimizations of an irreversible Diesel cycle based on finite piston speed. Energy 2021, 216, 119235. [Google Scholar] [CrossRef]
- Ge, Y.L.; Chen, L.G.; Feng, H.J. Ecological optimization of an irreversible Diesel cycle. Eur. Phys. J. Plus 2021, 136, 1–13. [Google Scholar] [CrossRef]
- Rocha-Martinez, J.A.; Navarrete-Gonzalez, T.D.; Pava-Miller, C.G. Otto and Diesel engine models with cyclic variability. Rev. Mex. Fis. 2002, 48, 228–234. [Google Scholar]
- Al-Sarkhi, A.; Jaber, J.O.; Abu-Qudais, M.; Probert, S.D. Effects of friction and temperature-dependent specific-heat of the working fluid on the performance of a Diesel-engine. Appl. Energy 2006, 83, 153–165. [Google Scholar] [CrossRef]
- Zhao, Y.R.; Chen, J.C. Optimum performance analysis of an irreversible Diesel heat engine affected by variable heat capacities of working fluid. Energy Convers. Manag. 2007, 48, 2595–2603. [Google Scholar] [CrossRef]
- Ge, Y.L.; Chen, L.G.; Sun, F.R.; Wu, C. Performance of Diesel cycle with heat transfer, friction and variable specific heats of working fluid. J. Energy Inst. 2007, 80, 239–242. [Google Scholar] [CrossRef]
- Ge, Y.L.; Chen, L.G.; Sun, F.R.; Wu, C. Performance of an endoreversible Diesel cycle with variable specific heats of working fluid. Int. J. Ambient Energy 2008, 29, 127–136. [Google Scholar] [CrossRef]
- Ge, Y.L.; Chen, L.G.; Sun, F.R. Finite time thermodynamic modeling and analysis for an irreversible Diesel cycle. Proc. Inst. Mech. Eng. Part D 2008, 222, 887–894. [Google Scholar] [CrossRef]
- Sakhrieh, A.; Abu-Nada, E.; Akash, B.; Al-Hinti, I.; Al-Ghandoor, A. Performance of diesel engine using gas mixture with variable specific heats model. J. Energy Inst. 2010, 83, 217–224. [Google Scholar] [CrossRef]
- Hou, S.S.; Lin, J.C. Performance analysis of a Diesel cycle under the restriction of maximum cycle temperature with considerations of heat loss, friction, and variable specific heats. Acta Phys. Pol. A 2011, 120, 979–986. [Google Scholar] [CrossRef]
- Sahin, B.; Kodal, A.; Yavuz, H. Efficiency of a Joule-Brayton engine at maximum power density. J. Phys. D Appl. Phys. 1995, 28, 1309. [Google Scholar] [CrossRef]
- Sahin, B.; Kodal, A.; Yilmaz, T.; Yavuz, H. Maximum power density analysis of an irreversible Joule-Brayton engine. J. Phys. D Appl. Phys. 1996, 29, 1162. [Google Scholar] [CrossRef]
- Chen, L.G.; Lin, J.X.; Sun, F.R.; Wu, C. Efficiency of an Atkinson engine at maximum power density. Energy Convers. Manag. 1998, 39, 337–341. [Google Scholar] [CrossRef]
- Atmaca, M.; Gumus, M. Power and efficiency analysis of Diesel cycle under alternative criteria. Arab. J. Sci. Eng. 2014, 39, 2263–2270. [Google Scholar] [CrossRef]
- Raman, R.; Kumar, N. Performance analysis of Diesel cycle under efficient power density condition with variable specific heat of working fluid. J. Non-Equilib. Thermodyn. 2019, 44, 405. [Google Scholar] [CrossRef]
- Rai, R.K.; Sahoo, R.R. Effective power and effective power density analysis for water in diesel emulsion as fuel in Diesel engine performance. Energy 2019, 180, 893–902. [Google Scholar] [CrossRef]
- Gonca, G.; Palaci, Y. Performance investigation into a Diesel engine under effective effciency-power-power density conditions. Sci. Iran. B 2019, 26, 843–855. [Google Scholar]
- Ahmadi, M.H.; Ahmadi, M.A. Thermodynamic analysis and optimisation of an irreversible radiative-type heat engine by using non-dominated sorting genetic algorithm. Int. J. Ambient Energy 2016, 37, 403–408. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Jokar, M.A.; Ming, T.Z.; Feidt, M.; Pourfayaz, F.; Astaraei, F.R. Multi-objective performance optimization of irreversible molten carbonate fuel cell-Braysson heat engine and thermodynamic analysis with ecological objective approach. Energy 2018, 144, 707–722. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Sameti, M.; Sourkiaei, S.M.; Ming, T.Z.; Pourfayaz, F.; Chamkha, A.J.; Oztop, H.F.; Jokar, M.A. Multi-objective performance optimization of irreversible molten carbonate fuel cell-Stirling heat engine-reverse osmosis and thermodynamic assessment with ecological objective approach. Energy Sci. Eng. 2018, 6, 783–796. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Nazari, M.A.; Feid, M. Thermodynamic analysis and multi-objective optimisation of endoreversible Lenoir heat engine cycle based on the thermo-economic performance criterion. Int. J. Ambient Energy 2019, 40, 600–609. [Google Scholar] [CrossRef]
- Shi, S.S.; Ge, Y.L.; Chen, L.G.; Feng, H.J. Four-objective optimization of irreversible Atkinson cycle based on NSGA-II. Entropy 2020, 22, 1150. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Pourkiaei, S.M.; Ghazvini, M.; Pourfayaz, F. Thermodynamic assessment and optimization of performance of irreversible Atkinson cycle. Iran. J. Chem. Chem. Eng. 2020, 39, 267–280. [Google Scholar]
- Gonzalez-Ayala, J.; Roco, J.M.M.; Medina, A.; Calvo, H.A. Optimization, stability, and entropy in endoreversible heat engines. Entropy 2020, 22, 1323. [Google Scholar] [CrossRef]
- Ata, S.; Kahraman, A.; Şahin, R. Prediction and sensitivity analysis under different performance indices of R1234ze ORC with Taguchi’s multi-objective optimization. Case Stud. Therm. Eng. 2020, 22, 100785. [Google Scholar] [CrossRef]
- Herrera-Orozco, I.; Valencia-Ochoa, G.; Duarte-Forero, J. Exergo-environmental assessment and multi-objective optimization of waste heat recovery systems based on Organic Rankine cycle configurations. J. Clean. Prod. 2021, 288, 125679. [Google Scholar] [CrossRef]
- Li, Y.Y.; Li, W.Y.; Gao, X.Y.; Ling, X. Thermodynamic analysis and optimization of organic Rankine cycles based on radial-inflow turbine design. Appl. Therm. Eng. 2021, 184, 116277. [Google Scholar] [CrossRef]
- Garmejani, H.A.; Hossainpou, S.H. Single and multi-objective optimization of a TEG system for optimum power, cost and second law efficiency using genetic algorithm. Energy Convers. Manag. 2021, 228, 113658. [Google Scholar] [CrossRef]
- Tang, C.Q.; Chen, L.G.; Feng, H.J.; Ge, Y.L. Four-objective optimization for an irreversible closed modified simple Brayton cycle. Entropy 2021, 23, 282. [Google Scholar] [CrossRef] [PubMed]
- Nemogne, R.L.F.; Wouagfack, P.A.N.; Nouadje, B.A.M.; Tchinda, R. Multi-objective optimization and analysis of performance of a four-temperature-level multi-irreversible absorption heat pump. Energy Convers. Manag. 2021, 234, 113967. [Google Scholar] [CrossRef]
- Chen, L.G.; Tang, C.Q.; Feng, H.J.; Ge, Y.L. Power, efficiency, power density and ecological function optimizations for an irreversible modified closed variable-temperature reservoir regenerative Brayton cycle with one isothermal heating process. Energies 2020, 13, 5133. [Google Scholar] [CrossRef]
- Zhang, L.; Chen, L.G.; Xia, S.J.; Ge, Y.L.; Wang, C.; Feng, H.J. Multi-objective optimization for helium-heated reverse water gas shift reactor by using NSGA-II. Int. J. Heat Mass Transf. 2020, 148, 119025. [Google Scholar] [CrossRef]
- Sun, M.; Xia, S.J.; Chen, L.G.; Wang, C.; Tang, C.Q. Minimum entropy generation rate and maximum yield optimization of sulfuric acid decomposition process using NSGA-II. Entropy 2020, 22, 1065. [Google Scholar] [CrossRef]
- Wu, Z.X.; Feng, H.J.; Chen, L.G.; Ge, Y.L. Performance optimization of a condenser in ocean thermal energy conversion (OTEC) system based on constructal theory and multi-objective genetic algorithm. Entropy 2020, 22, 641. [Google Scholar] [CrossRef]
- Mozurkewich, M.; Berry, R.S. Finite-time thermodynamics: Engine performance improved by optimized piston motion. Proc. Natl. Acad. Sci. USA 1981, 78, 1986–1988. [Google Scholar] [CrossRef] [Green Version]
- Mozurkewich, M.; Berry, R.S. Optimal paths for thermodynamic systems. The ideal Otto cycle. J. Appl. Phys. 1982, 53, 34–42. [Google Scholar] [CrossRef]
- Hoffmann, K.H.; Watowich, S.J.; Berry, R.S. Optimal paths for thermodynamic systems. The ideal Diesel cycle. J. Appl. Phys. 1985, 58, 2125–2134. [Google Scholar] [CrossRef]












| Curve Number | Considered Loss | ||
|---|---|---|---|
| 1 | No loss | 61.51% | 0% |
| 2 | FL | 60.36% | 1.87% |
| 3 | HTL | 56.45% | 8.23% |
| 4 | FL and HTL | 55.41% | 9.92% |
| 1′ | IIL | 52.97% | 13.88% |
| 2′ | IIL and FL | 51.84% | 15.72% |
| 3′ | IIL and HTL | 48.67% | 20.87% |
| 4′ | IIL, HTL and FL | 47.64% | 22.55% |
| Optimization Methods | Solutions | Optimization Variable | Optimization Objectives | Deviation Index | |||
|---|---|---|---|---|---|---|---|
| Quadru-objective optimization (, , , and ) | LINMAP | 18.0466 | 0.9615 | 0.5008 | 0.9809 | 0.9804 | 0.1342 |
| TOPSIS | 18.0822 | 0.9611 | 0.5010 | 0.9815 | 0.9801 | 0.1346 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4769 | 0.8359 | 1.0000 | 0.4068 | |
| Tri-objective optimization (, , and ) | LINMAP | 18.2403 | 0.9591 | 0.5017 | 0.9842 | 0.9785 | 0.1366 |
| TOPSIS | 18.5159 | 0.9556 | 0.5029 | 0.9882 | 0.9758 | 0.1422 | |
| Shannon entropy | 20.3584 | 0.9299 | 0.5095 | 1.0000 | 0.9545 | 0.2068 | |
| Tri-objective optimization (, , and ) | LINMAP | 17.1965 | 0.9715 | 0.4966 | 0.9624 | 0.9878 | 0.1443 |
| TOPSIS | 16.8933 | 0.9749 | 0.4949 | 0.9540 | 0.9900 | 0.1574 | |
| Shannon entropy | 14.3433 | 0.9958 | 0.4768 | 0.8359 | 1.0000 | 0.4068 | |
| Tri-objective optimization (, , and ) | LINMAP | 17.8459 | 0.9640 | 0.4999 | 0.9772 | 0.9823 | 0.1333 |
| TOPSIS | 17.9598 | 0.9626 | 0.5004 | 0.9793 | 0.9812 | 0.1336 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4768 | 0.8359 | 1.0000 | 0.4068 | |
| Tri-objective optimization (, , and ) | LINMAP | 18.7911 | 0.9520 | 0.5040 | 0.9916 | 0.9729 | 0.1495 |
| TOPSIS | 18.7911 | 0.9520 | 0.5040 | 0.9916 | 0.9729 | 0.1495 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4769 | 0.8359 | 1.0000 | 0.4068 | |
| Bi-objective optimization ( and ) | LINMAP | 17.4129 | 0.9691 | 0.4977 | 0.9678 | 0.9860 | 0.1380 |
| TOPSIS | 17.3189 | 0.9722 | 0.4962 | 0.9655 | 0.9868 | 0.1384 | |
| Shannon entropy | 26.2726 | 0.8327 | 0.5176 | 0.9166 | 0.8647 | 0.5193 | |
| Bi-objective optimization ( and ) | LINMAP | 18.0043 | 0.9620 | 0.5006 | 0.9802 | 0.9808 | 0.1339 |
| TOPSIS | 18.2236 | 0.9593 | 0.5016 | 0.9839 | 0.9787 | 0.1364 | |
| Shannon entropy | 20.3584 | 0.9299 | 0.5095 | 1.0000 | 0.9545 | 0.2068 | |
| Bi-objective optimization ( and ) | LINMAP | 13.5850 | 0.9989 | 0.4699 | 0.7800 | 0.9989 | 0.5004 |
| TOPSIS | 13.5850 | 0.9989 | 0.4699 | 0.7800 | 0.9989 | 0.5004 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4768 | 0.8359 | 1.0000 | 0.4068 | |
| Bi-objective optimization ( and ) | LINMAP | 21.6879 | 0.9097 | 0.5129 | 0.9948 | 0.9367 | 0.2645 |
| TOPSIS | 21.6879 | 0.9097 | 0.5129 | 0.9948 | 0.9367 | 0.2645 | |
| Shannon entropy | 20.3584 | 0.9299 | 0.5095 | 1.0000 | 0.9545 | 0.2068 | |
| Bi-objective optimization ( and ) | LINMAP | 18.4344 | 0.9566 | 0.5026 | 0.9871 | 0.9766 | 0.1403 |
| TOPSIS | 18.1938 | 0.9597 | 0.5015 | 0.9834 | 0.9790 | 0.1359 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4768 | 0.8359 | 1.000 | 0.4068 | |
| Bi-objective optimization ( and ) | LINMAP | 18.5178 | 0.9555 | 0.5029 | 0.9882 | 0.9758 | 0.1422 |
| TOPSIS | 18.5178 | 0.9555 | 0.5029 | 0.9882 | 0.9758 | 0.1422 | |
| Shannon entropy | 14.3437 | 0.9958 | 0.4769 | 0.8359 | 0.9999 | 0.4068 | |
| Maximum of | - | 12.8106 | 1.0000 | 0.4617 | 0.7090 | 0.9952 | 0.5828 |
| Maximum of | - | 26.2980 | 0.8323 | 0.5176 | 0.9160 | 0.8643 | 0.5210 |
| Maximum of | - | 20.4061 | 0.9293 | 0.5096 | 1.0000 | 0.9540 | 0.2086 |
| Maximum of | - | 14.3205 | 0.9960 | 0.4765 | 0.8330 | 1.0000 | 0.4122 |
| Positive ideal point | - | 1.0000 | 0.5176 | 1.0000 | 1.0000 | - | |
| Negative ideal point | - | 0.8328 | 0.4618 | 0.7105 | 0.8647 | - | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, S.; Chen, L.; Ge, Y.; Feng, H. Performance Optimizations with Single-, Bi-, Tri-, and Quadru-Objective for Irreversible Diesel Cycle. Entropy 2021, 23, 826. https://doi.org/10.3390/e23070826
Shi S, Chen L, Ge Y, Feng H. Performance Optimizations with Single-, Bi-, Tri-, and Quadru-Objective for Irreversible Diesel Cycle. Entropy. 2021; 23(7):826. https://doi.org/10.3390/e23070826
Chicago/Turabian StyleShi, Shuangshuang, Lingen Chen, Yanlin Ge, and Huijun Feng. 2021. "Performance Optimizations with Single-, Bi-, Tri-, and Quadru-Objective for Irreversible Diesel Cycle" Entropy 23, no. 7: 826. https://doi.org/10.3390/e23070826
APA StyleShi, S., Chen, L., Ge, Y., & Feng, H. (2021). Performance Optimizations with Single-, Bi-, Tri-, and Quadru-Objective for Irreversible Diesel Cycle. Entropy, 23(7), 826. https://doi.org/10.3390/e23070826







