Relating Entropies of Quantum Channels
Abstract
:1. Introduction
2. Preliminaries
3. Quantum Unital Qubit Channels
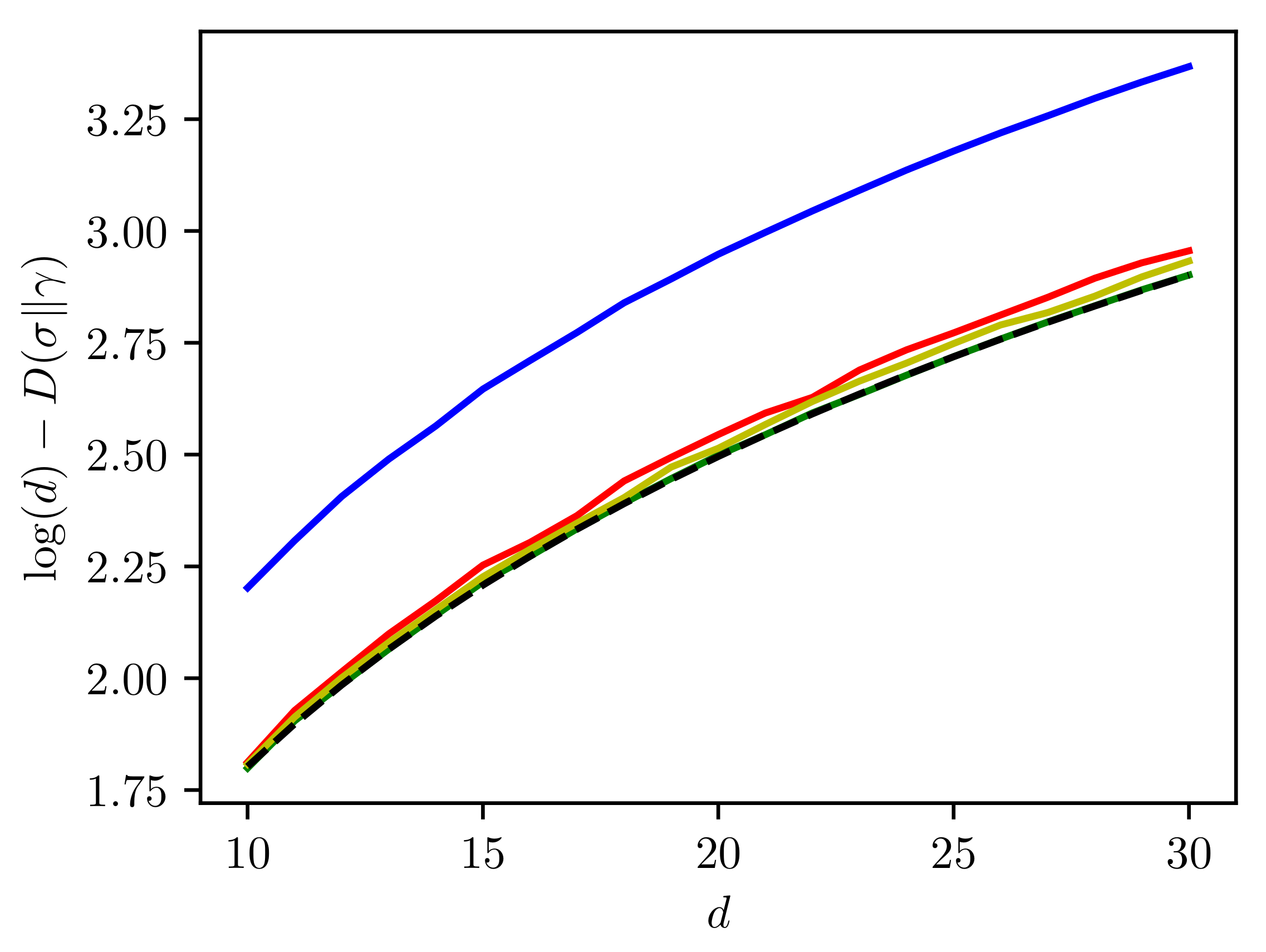
4. Asymptotic Case
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Devetak, I.; Winter, A. Distillation of secret key and entanglement from quantum states. Proc. R. Soc. Lond. A 2005, 461, 207–235. [Google Scholar] [CrossRef] [Green Version]
- Berta, M.; Christandl, M.; Colbeck, R.; Renes, J.M.; Renner, R. The uncertainty principle in the presence of quantum memory. Nat. Phys. 2010, 6, 659. [Google Scholar] [CrossRef]
- Chitambar, E.; Gour, G. Quantum resource theories. Rev. Mod. Phys. 2019, 91, 025001. [Google Scholar] [CrossRef] [Green Version]
- Gühne, O. Characterizing entanglement via uncertainty relations. Phys. Rev. Lett. 2004, 92, 117903. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Oppenheim, J.; Wehner, S. The uncertainty principle determines the nonlocality of quantum mechanics. Science 2010, 330, 1072–1074. [Google Scholar] [CrossRef] [Green Version]
- Rastegin, A.E. Separability conditions based on local fine-grained uncertainty relations. Quantum Inf. Process. 2016, 15, 2621–2638. [Google Scholar] [CrossRef] [Green Version]
- Enríquez, M.; Puchała, Z.; Życzkowski, K. Minimal rényi–ingarden–urbanik entropy of multipartite quantum states. Entropy 2015, 17, 5063–5084. [Google Scholar] [CrossRef] [Green Version]
- Roga, W.; Życzkowski, K.; Fannes, M. Entropic characterization of quantum operations. Int. J. Quantum Inf. 2011, 9, 1031–1045. [Google Scholar] [CrossRef] [Green Version]
- Roga, W.; Puchała, Z.; Rudnicki, Ł.; Życzkowski, K. Entropic trade-off relations for quantum operations. Phys. Rev. A 2013, 87, 032308. [Google Scholar] [CrossRef] [Green Version]
- Shaari, J.S.; Mancini, S. Entropic bounds for unitary testers and mutually unbiased unitary bases. Ann. Phys. 2020, 412, 168043. [Google Scholar] [CrossRef]
- Rudnicki, Ł.; Puchała, Z.; Życzkowski, K. Strong majorization entropic uncertainty relations. Phys. Rev. A 2014, 89, 052115. [Google Scholar] [CrossRef] [Green Version]
- Coles, P.J.; Piani, M. Improved entropic uncertainty relations and information exclusion relations. Phys. Rev. A 2014, 89, 022112. [Google Scholar] [CrossRef] [Green Version]
- Rastegin, A.E.; Życzkowski, K. Majorization entropic uncertainty relations for quantum operations. J. Phys. A Math. Theor. 2016, 49, 355301. [Google Scholar] [CrossRef] [Green Version]
- Kurzyk, D.; Pawela, Ł.; Puchała, Z. Conditional entropic uncertainty relations for tsallis entropies. Quantum Inf. Process. 2018, 17, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Puchała, Z.; Rudnicki, Ł.; Krawiec, A.; Życzkowski, K. Majorization uncertainty relations for mixed quantum states. J. Phys. A Math. Theor. 2018, 51, 175306. [Google Scholar] [CrossRef] [Green Version]
- Vedral, V. The role of relative entropy in quantum information theory. Rev. Mod. Phys. 2002, 74, 197. [Google Scholar] [CrossRef] [Green Version]
- Yuan, X. Hypothesis testing and entropies of quantum channels. Phys. Rev. A 2019, 99, 032317. [Google Scholar] [CrossRef] [Green Version]
- Gour, G.; Wilde, M.M. Entropy of a quantum channel. Phys. Rev. Res. 2021, 3, 023096. [Google Scholar] [CrossRef]
- Liu, Z.-W.; Winter, A. Resource theories of quantum channels and the universal role of resource erasure. arXiv 2019, arXiv:1904.04201. [Google Scholar]
- Katariya, V.; Wilde, M.M. Geometric distinguishability measures limit quantum channel estimation and discrimination. Quantum Inf. Process. 2021, 20, 1–170. [Google Scholar] [CrossRef]
- Fang, K.; Fawzi, O.; Renner, R.; Sutter, D. Chain rule for the quantum relative entropy. Phys. Rev. Lett. 2020, 124, 100501. [Google Scholar] [CrossRef] [Green Version]
- Leditzky, F.; Kaur, E.; Datta, N.; Wilde, M.M. Approaches for approximate additivity of the holevo information of quantum channels. Phys. Rev. A 2018, 97, 012332. [Google Scholar] [CrossRef] [Green Version]
- Fang, K.; Fawzi, H. Geometric rényi divergence and its applications in quantum channel capacities. Commun. Math. Phys. 2021, 384, 1615–1677. [Google Scholar] [CrossRef]
- Umegaki, H. Conditional Expectation in an Operator Algebra, IV (Entropy and Information). In Kodai Mathematical Seminar Reports; Department of Mathematics, Tokyo Institute of Technology: Tokyo, Japan, 1962; Volume 14, pp. 59–85. [Google Scholar]
- Haber, H.E. Notes on the Matrix Exponential and Logarithm. Available online: http://scipp.ucsc.edu/~haber/webpage/MatrixExpLog.pdf (accessed on 14 June 2021).
- Nechita, I.; Puchała, Z.; Pawela, Ł.; Życzkowski, K. Almost all quantum channels are equidistant. J. Math. Phys. 2018, 59, 052201. [Google Scholar] [CrossRef] [Green Version]
- Voiculescu, D. Multiplication of certain non-commuting random variables. J. Oper. Theory 1987, 18, 223–235. [Google Scholar]
- Życzkowski, K.; Penson, K.A.; Nechita, I.; Collins, B. Generating random density matrices. J. Math. Phys. 2011, 52, 062201. [Google Scholar] [CrossRef]
- Puchała, Z.; Pawela, Ł.; Życzkowski, K. Distinguishability of generic quantum states. Phys. Rev. A 2016, 93, 062112. [Google Scholar] [CrossRef] [Green Version]
- Kukulski, R.; Nechita, I.; Pawela, Ł.; Puchała, Z.; Życzkowski, K. Generating random quantum channels. J. Math. Phys. 2021, 62, 062201. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurzyk, D.; Pawela, Ł.; Puchała, Z. Relating Entropies of Quantum Channels. Entropy 2021, 23, 1028. https://doi.org/10.3390/e23081028
Kurzyk D, Pawela Ł, Puchała Z. Relating Entropies of Quantum Channels. Entropy. 2021; 23(8):1028. https://doi.org/10.3390/e23081028
Chicago/Turabian StyleKurzyk, Dariusz, Łukasz Pawela, and Zbigniew Puchała. 2021. "Relating Entropies of Quantum Channels" Entropy 23, no. 8: 1028. https://doi.org/10.3390/e23081028