Phase Transition in Modified Newtonian Dynamics (MONDian) Self-Gravitating Systems
Abstract
:1. Introduction
2. Statistical Mechanics of a Self-Gravitating Binary under Newtonian Potential: Microcanonical Ensemble
3. Statistical Mechanics of a Self-Gravitating Binary in MOND: Microcanonical Ensemble
4. Statistical Mechanics of a Self-Gravitating Binary in MOND: Canonical Ensemble
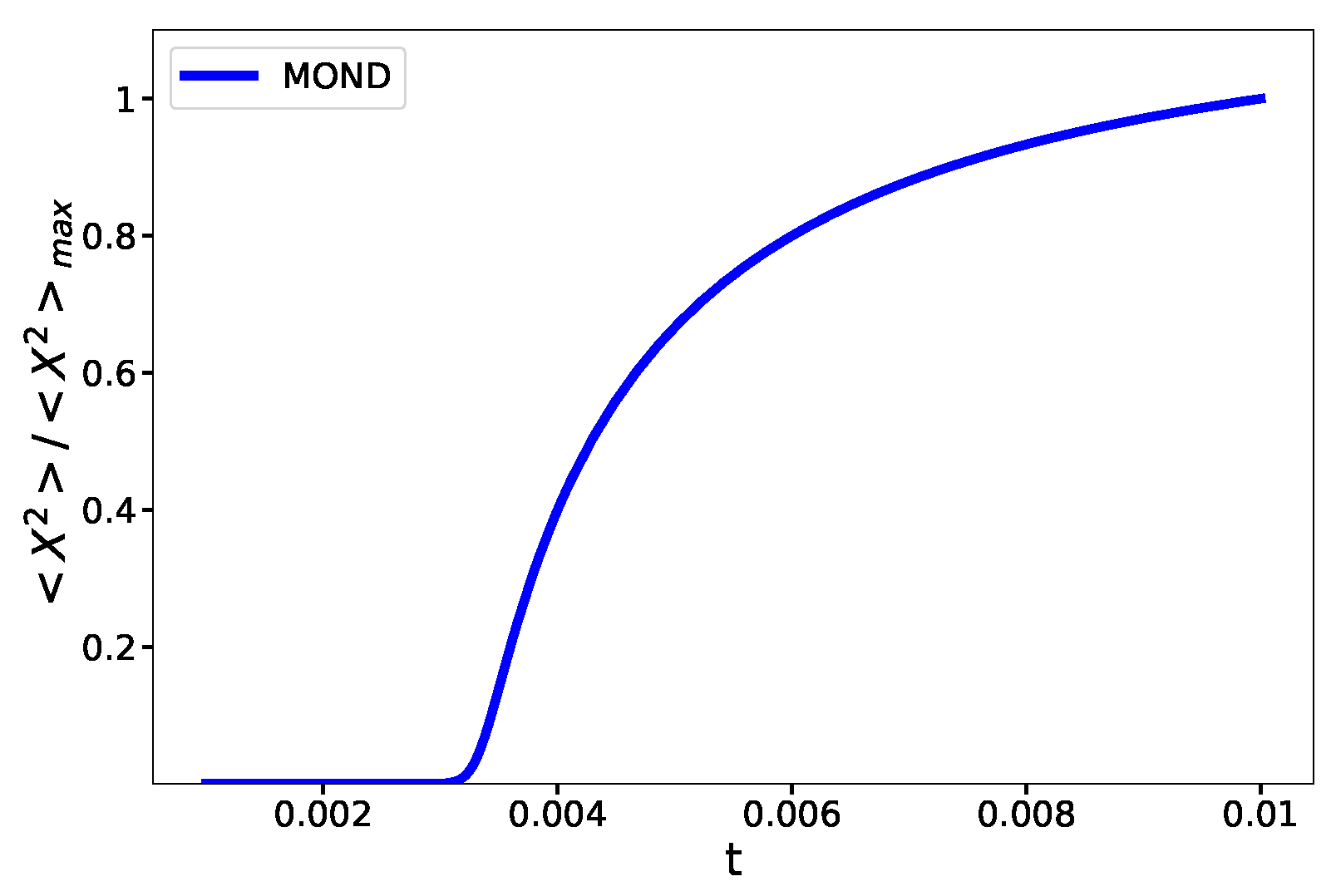
5. Statistical Mechanics of a Self-Gravitating Binary in MOND: Comoving Coordinates
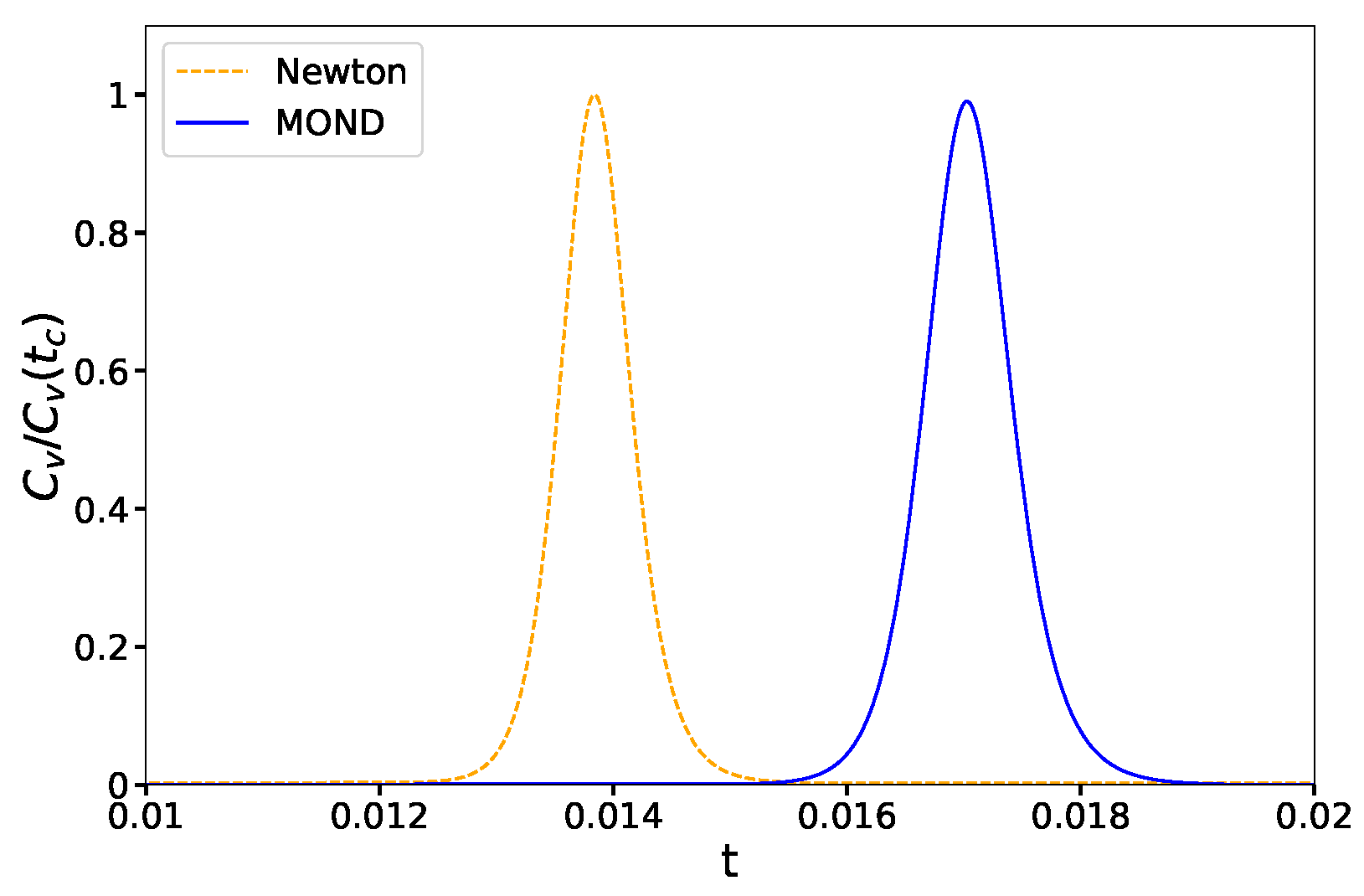
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Blumenthal, G.R.; Faber, S.M.; Primack, J.R.; Rees, M.J. Formation of galaxies and large-scale structure with cold dark matter. Nat. Cell Biol. 1984, 311, 517–525. [Google Scholar] [CrossRef]
- Planck Collaboration; Ade, P.; Aghanim, N.; Alves, M.I.R.; Armitage-Caplan, C.; Arnaud, M.; Ashdown, M.; Atrio-Barandela, F.; Aumont, J.; Aussel, H.; et al. Planck2013 results. I. Overview of products and scientific results. Astron. Astrophys. 2014, 571, A1. [Google Scholar] [CrossRef] [Green Version]
- Pawlowski, M.S.; Kroupa, P. The Milky Way’s disc of classical satellite galaxies in light of Gaia DR2. Mon. Not. R. Astron. Soc. 2020, 491, 3042–3059. [Google Scholar] [CrossRef] [Green Version]
- Secrest, N.J.; von Hausegger, S.; Rameez, M.; Mohayaee, R.; Sarkar, S.; Colin, J. A Test of the Cosmological Principle with Quasars. Astrophys. J. 2021, 908, L51. [Google Scholar] [CrossRef]
- Asencio, E.; Banik, I.; Kroupa, P. A massive blow for ΛCDM—The high redshift, mass, and collision velocity of the interacting galaxy cluster El Gordo contradicts concordance cosmology. Mon. Not. R. Astron. Soc. 2020, 500, 5249–5267. [Google Scholar] [CrossRef]
- Haslbauer, M.; Banik, I.; Kroupa, P. The KBC void and Hubble tension contradict ΛCDM on a Gpc scale Milgromian dynamics as a possible solution. Mon. Not. R. Astron. Soc. 2020, 499, 2845–2883. [Google Scholar] [CrossRef]
- Chavanis, P.H. Phase transition in self-gravitating system. Int. J. Mod. Phys. B 2006, B20, 3113–3198. [Google Scholar] [CrossRef]
- Abdalla, E.; Rahimi Tabar, M.R. Phase Transition in A Self-Gravitating Planar Gas. Phys. Lett. B 1998, 440, 339–344. [Google Scholar] [CrossRef] [Green Version]
- Emden, R. Gaskugeln, Teubner, Leipzig und Berlin. 1907. Chandrasekhar, S. An Introduction to the Study of Stellar Structure; Chicago University Press: Chicago, IL, USA, 1939. [Google Scholar]
- Bonnar, W.B. Boyle’s Law and Gravitational Instability. Mon. Not. R. Astron. Soc. 1956, 116, 351–359. [Google Scholar] [CrossRef] [Green Version]
- Ebert, R.Z. Über die Verdichtung von H I-Gebieten. Mit 5 Textabbildungen. Zeitschrift fur Astrophysik 1955, 37, 217. [Google Scholar]
- Antonov, V.A. Most probable phase distribution in spherical star systems and conditions for its existence. Vestnik Leningrad. Univ. 1962, 7, 135. [Google Scholar] [CrossRef] [Green Version]
- Lynden-Bell, D.; Wood, R. The gravo-thermal catastrophe in isothermal spheres and the onset of red-giant structure for stellar systems. Mon. Not. R. Astron. Soc. 1968, 138, 495–525. [Google Scholar] [CrossRef] [Green Version]
- Horwitz, G.; Katz, J. Steepest-descent technique and stellar equilibrium statistical mechanics. I-Newtonian clusters in a box. Astrophys. J. 1977, 211, 226–243. [Google Scholar] [CrossRef]
- Horwitz, G.; Katz, J. Steepest descent technique and stellar equilibrium statistical mechanics. III Stability of various ensembles. Astrophys. J. 1978, 222, 941–958. [Google Scholar] [CrossRef]
- Padmanabhan, T. Statistical mechanics of gravitating systems. Phys. Rep. 1990, 188, 285–362. [Google Scholar] [CrossRef]
- Saslaw, W.C. Gravitational Physics of Stellar and Galactic Systems; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- de Vega, H.J.; Sanchez, N.; Combes, F. Self-gravity as an explanation of the fractal structure of the interstellar medium. Nature 1996, 383, 56–58. [Google Scholar] [CrossRef] [Green Version]
- de Vega, H.J.; Sanchez, N.; Combes, F. Fractal dimensions and scaling laws in the interstellar medium: A new field theory approach. Phys. Rev. D 1996, 54, 6008. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- de Vega, H.J.; Sanchez, N.; Combes, F. Current Topics in Astrofundamental Physics: Primordial Cosmology, NATO ASI at Erice; Sanchez, N., Zichichi, A., Eds.; Springer Science & Business Media: Berlin, Germany, 1998; Volume 511. [Google Scholar]
- Peebles, P.J.E. The Large-Scale Structure of the Universe; Princeton University Press: Princeton, NJ, USA, 1980; p. 435. [Google Scholar]
- Padmanabhan, T. Structure Formation in the Universe; Cambridge University Press: Cambridge, UK, 1993; p. 499. [Google Scholar]
- Moffat, J.W. Scalar tensor vector gravity theory. J. Cosmol. Astropart. Phys. 2006, 0603, 004. [Google Scholar] [CrossRef]
- Moffat, J.W.; Rahvar, S. The MOG weak field approximation and observational test of galaxy rotation curves. Mon. Not. R. Astron. Soc. 2013, 436, 1439–1451. [Google Scholar] [CrossRef] [Green Version]
- Moffat, J.W.; Rahvar, S. The MOG weak field approximation—II. Observational test of Chandra X-ray clusters. Mon. Not. R. Astron. Soc. 2014, 441, 3724–3732. [Google Scholar] [CrossRef] [Green Version]
- Haghi, H.; Amiri, V.; Zonoozi, A.H.; Banik, I.; Kroupa, P.; Haslbauer, M. The Star Formation History and Dynamics of the Ultra-diffuse Galaxy Dragonfly 44 in MOND and MOG. Astrophys. J. 2019, 884, L25. [Google Scholar] [CrossRef]
- Mashhoon, B. Nonlocal Gravity; Oxford University Press: Oxford, UK, 2017; Volume 167. [Google Scholar]
- Rahvar, S.; Mashhoon, B. Observational tests of nonlocal gravity: Galaxy rotation curves and clusters of galaxies. Phys. Rev. D 2014, 89, 104011. [Google Scholar] [CrossRef] [Green Version]
- Milgrom, M. A modification of the Newtonian dynamics as a possible alternative to the hidden mass hypothesis. Astrophys. J. 1983, 270, 365–370. [Google Scholar] [CrossRef]
- McGaugh, S.; de Blok, W. Testing the Hypothesis of Modified Dynamics with Low Surface Brightness Galaxies and Other Evidence. Astrophys. J. 1998, 499, 66–81. [Google Scholar] [CrossRef] [Green Version]
- Levin, Y.; Pakter, R.; Rizzato, F.B.; Teles, T.N.; Benetti, F.P. Nonequilibrium statistical mechanics of systems with long-range interactions. Phys. Rep. 2014, 535, 1–60. [Google Scholar] [CrossRef] [Green Version]
- Padmanabhan, T. Statistical mechanics of gravitating systems: An Overview. arXiv 2008, arXiv:0812.2610. [Google Scholar]
- Dauxois, T.; Ruffo, S.; Arimondo, E.; Wilkens, M. Dynamics and Thermodynamics of Systems with Long-Range Interactions; Springer: Berlin/Heidelberg, Germany, 2002; p. 602. [Google Scholar]
- Begeman, K.G.; Broeils, A.H.; Sanders, R.H. Extended rotation curves of spiral galaxies: Dark haloes and modified dynamics. Mon. Not. R. Astron. Soc. 1991, 249, 523–537. [Google Scholar] [CrossRef] [Green Version]
- Tully, R.B.; Fisher, J.R. A new method of determining distances to galaxies. Astron. Astrophys. 1977, 54, 661–673. [Google Scholar]
- Felten, J.E. Milgrom’s revision of Newton’s laws—Dynamical and cosmological consequences. Astrophys. J. 1984, 286, 3–6. [Google Scholar] [CrossRef]
- Binney, J.; Tremaine, S. Galactic Dynamics; Princeton University Press: Princeton, NJ, USA, 1987; p. 747. [Google Scholar]
- Golovnev, A.; Masalaeva, N. Modified gravitational collapse, or the wonders of the MOND. Gen. Relativ. Gravit. 2014, 46, 1754. [Google Scholar] [CrossRef] [Green Version]
- Zonoozi, A.H.; Lieberz, P.; Banik, I.; Haghi, H.; Kroupa, P. The Kennicutt–Schmidt law and the main sequence of galaxies in Newtonian and milgromian dynamics. Mon. Not. R. Astron. Soc. 2021, 506, 5468–5478. [Google Scholar] [CrossRef]
- Bekenstein, J.; Milgrom, M. Does the missing mass problem signal the breakdown of Newtonian gravity? Astrophys. J. 1984, 286, 7–14. [Google Scholar] [CrossRef]
- Famaey, B.; Binney, J. Modified Newtonian dynamics in the Milky Way. Mon. Not. R. Astron. Soc. 2005, 363, 603–608. [Google Scholar] [CrossRef] [Green Version]
- Banik, I.; O’Ryan, D.; Zhao, H. Origin of the Local Group satellite planes. Mon. Not. R. Astron. Soc. 2018, 477, 4768–4791. [Google Scholar] [CrossRef] [Green Version]
- Banik, I.; Zhao, H. Testing gravity with wide binary stars like αCentauri. Mon. Not. R. Astron. Soc. 2018, 480, 2660–2688. [Google Scholar] [CrossRef]
- Sanju’an, M.A.F. Concepts in Thermal Physics. Contemp. Phys. 2011, 52, 98. [Google Scholar]
- De Vega, H.J.; Sanchez, N.; Combes, F. The Fractal Structure of the Universe: A New Field Theory Approach. Astrophys. J. 1998, 500, 8–13. [Google Scholar] [CrossRef] [Green Version]
- Herrera, L. New definition of complexity for self-gravitating fluid distributions: The spherically symmetric, static case. Phys. Rev. D 2018, 97, 044010. [Google Scholar] [CrossRef] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhoolideh Haghighi, M.H.; Rahvar, S.; Rahimi Tabar, M.R. Phase Transition in Modified Newtonian Dynamics (MONDian) Self-Gravitating Systems. Entropy 2021, 23, 1158. https://doi.org/10.3390/e23091158
Zhoolideh Haghighi MH, Rahvar S, Rahimi Tabar MR. Phase Transition in Modified Newtonian Dynamics (MONDian) Self-Gravitating Systems. Entropy. 2021; 23(9):1158. https://doi.org/10.3390/e23091158
Chicago/Turabian StyleZhoolideh Haghighi, Mohammad Hossein, Sohrab Rahvar, and Mohammad Reza Rahimi Tabar. 2021. "Phase Transition in Modified Newtonian Dynamics (MONDian) Self-Gravitating Systems" Entropy 23, no. 9: 1158. https://doi.org/10.3390/e23091158
APA StyleZhoolideh Haghighi, M. H., Rahvar, S., & Rahimi Tabar, M. R. (2021). Phase Transition in Modified Newtonian Dynamics (MONDian) Self-Gravitating Systems. Entropy, 23(9), 1158. https://doi.org/10.3390/e23091158







