Quantum Energy Current Induced Coherence in a Spin Chain under Non-Markovian Environments
Abstract
:1. Introduction
2. Formalism
2.1. Non-Markovian Quantum State Diffusion
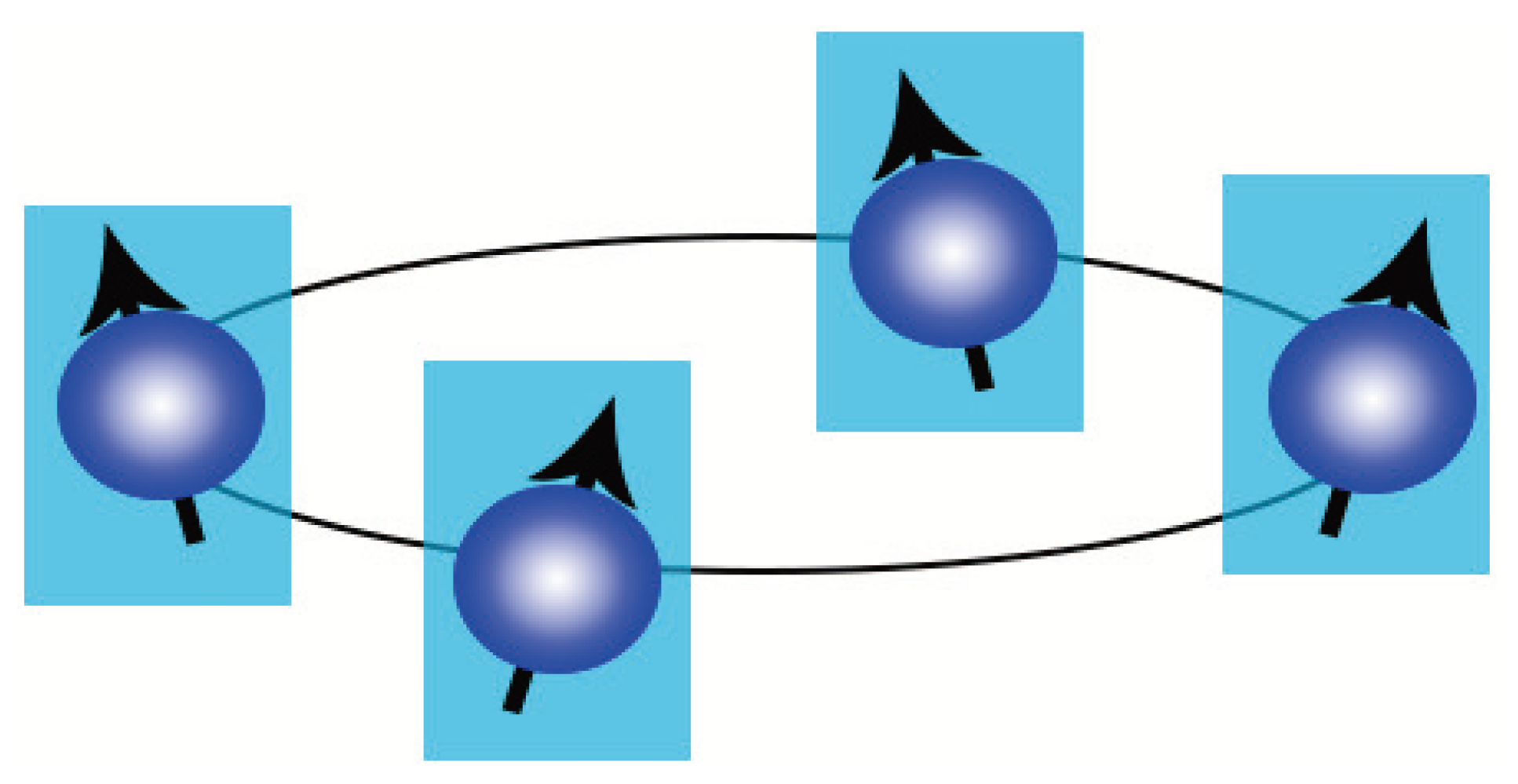
2.2. Spin Chain
2.3. Energy Current
2.4. Quantum Coherence
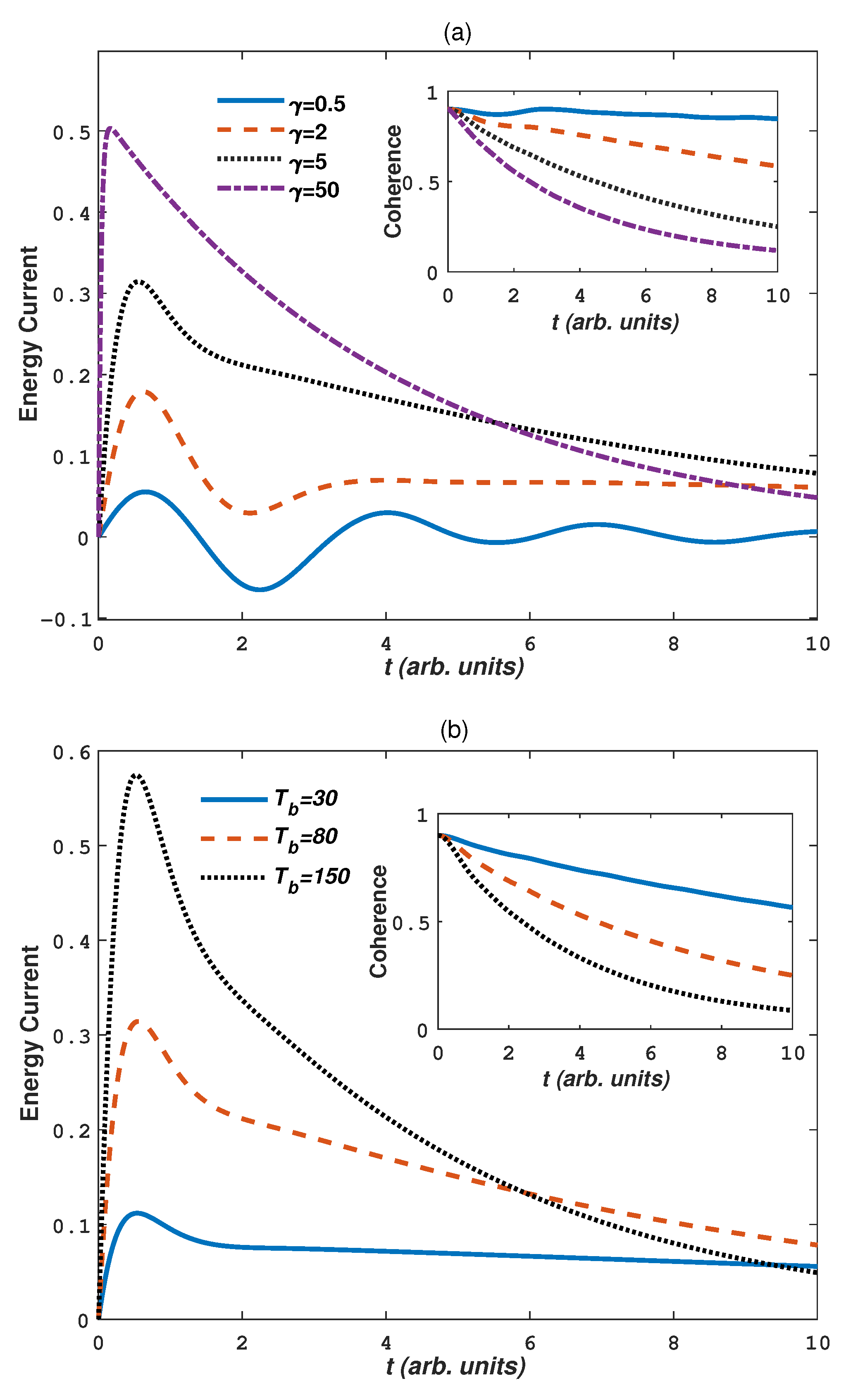
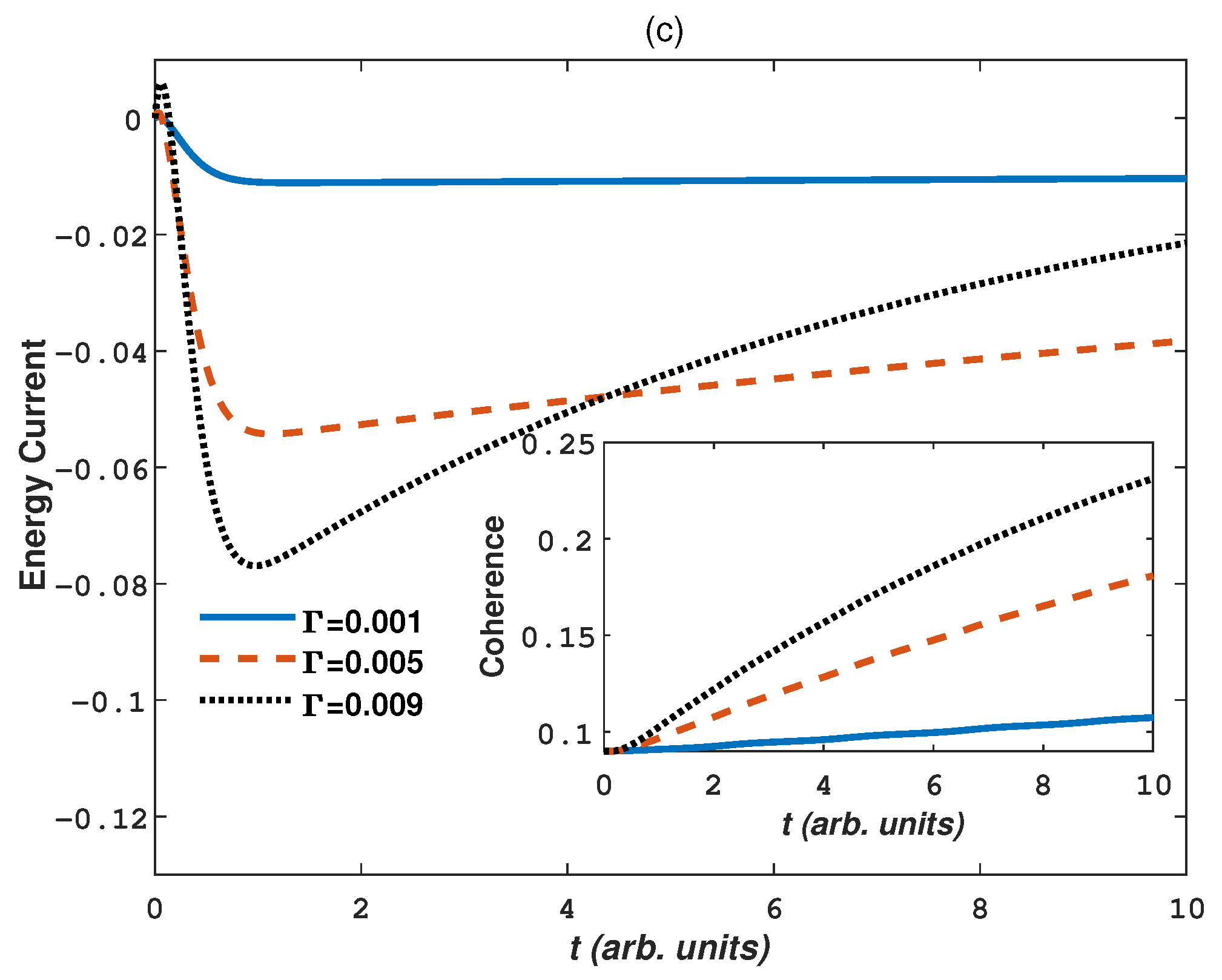
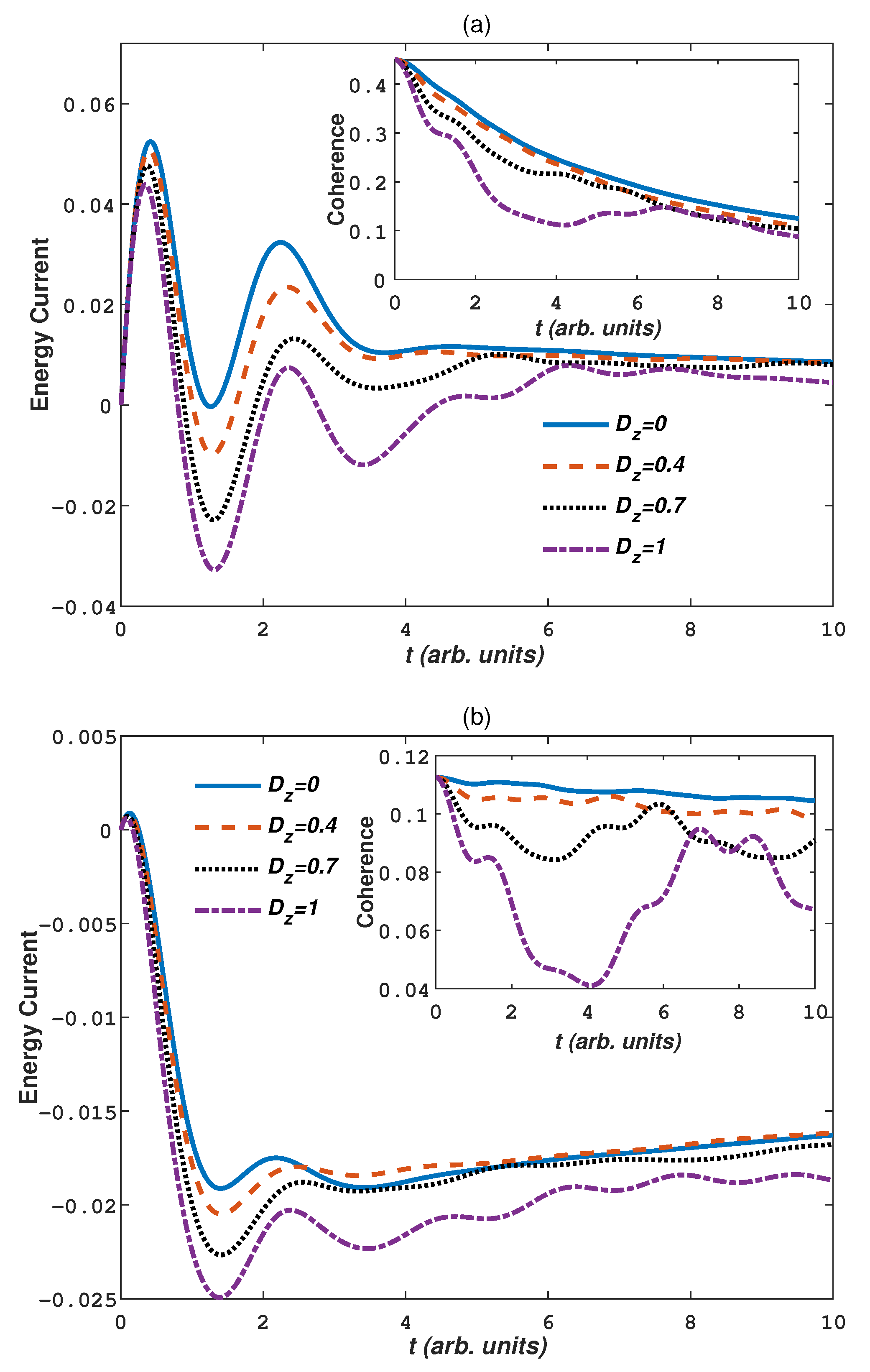
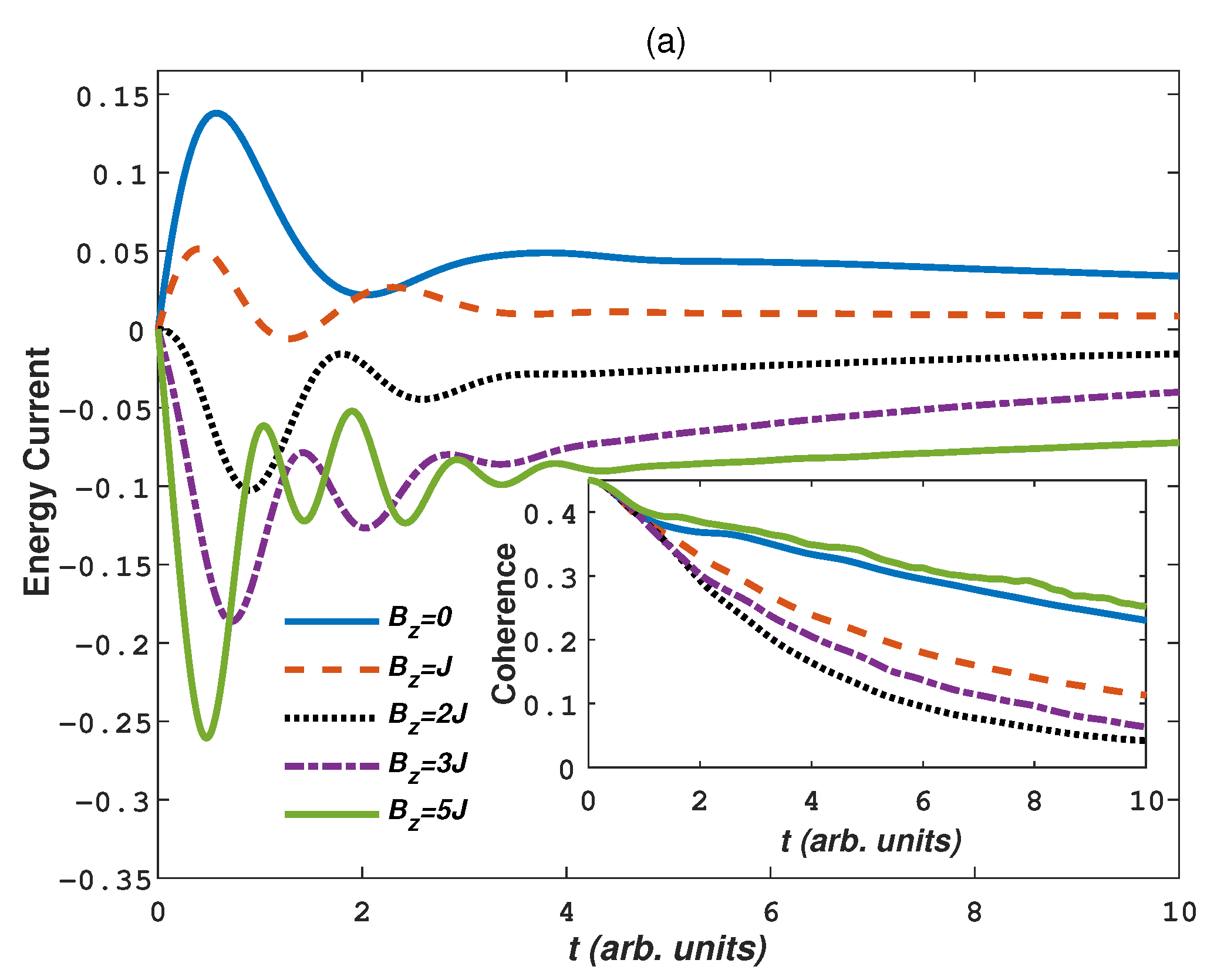
3. Numerical Results and Discussions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| NMQSD | Non-Markovian Quantum State Diffusion |
| DM | Dzyaloshinskii–Moriya |
References
- Woggon, U.; Gindele, F.; Langbein, W.; Hvam, J.M. Quantum kinetic exciton–LO-phonon interaction in CdSe. Phys. Rev. B. 2000, 61, 1935–1940. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.P.; Laine, E.M.; Piilo, J.; Vacchini, B. Colloquium: Non-Markovian dynamics in open quantum systems. Rev. Mod. Phys. 2016, 88, 021002. [Google Scholar] [CrossRef] [Green Version]
- Piilo, J.; Härkönen, K.; Maniscalco, S.; Suominen, K.A. Open system dynamics with non-Markovian quantum jumps. Phys. Rev. A 2009, 79, 062112. [Google Scholar] [CrossRef] [Green Version]
- Tarasov, V.E. General non-Markovian quantum dynamics. Entropy 2021, 23, 1006. [Google Scholar] [CrossRef] [PubMed]
- Czerwinski, A. Dynamics of open quantum systems—Markovian semigroups and beyond. Symmetry 2022, 14, 1752. [Google Scholar] [CrossRef]
- Breuer, H.P.; Laine, E.M.; Piilo, J. Measure for the degree of non-Markovian behavior of quantum processes in open systems. Phys. Rev. Lett. 2009, 103, 210401. [Google Scholar] [CrossRef] [Green Version]
- Helm, J.; Strunz, W.T. Decoherence and entanglement dynamics in fluctuating fields. Phys. Rev. A 2010, 81, 042314. [Google Scholar] [CrossRef] [Green Version]
- Guarnieri, G.; Uchiyama, C.; Vacchini, B. Energy backflow and non-Markovian dynamics. Phys. Rev. A 2016, 93, 012118. [Google Scholar] [CrossRef] [Green Version]
- Guarnieri, G.; Nokkala, J.; Schmidt, R.; Maniscalco, S.; Vacchini, B. Energy backflow in strongly coupled non-Markovian continuous-variable systems. Phys. Rev. A 2016, 94, 062101. [Google Scholar] [CrossRef] [Green Version]
- Wu, J.; Ren, F.H.; He, R.H.; Nie, S.S.; Wang, Z.M. Adiabatic speedup and quantum heat current in an open system. Eur. Phys. Lett. 2022, 139, 48001. [Google Scholar] [CrossRef]
- Schmidt, R.; Maniscalco, S.; Ala-Nissila, T. Heat flux and information backflow in cold environments. Phys. Rev. A 2016, 94, 010101. [Google Scholar] [CrossRef] [Green Version]
- Wittemer, M.; Clos, G.; Breuer, H.P.; Warring, U.; Schaetz, T. Measurement of quantum memory effects and its fundamental limitations. Phys. Rev. A 2018, 97, 020102. [Google Scholar] [CrossRef] [Green Version]
- Passos, M.; Obando, P.C.; Balthazar, W.; Paula, F.; Huguenin, J.; Sarandy, M. Non-Markovianity through quantum coherence in an all-optical setup. Opt. Lett. 2019, 44, 2478–2481. [Google Scholar] [CrossRef]
- Khurana, D.; Agarwalla, B.K.; Mahesh, T.S. Experimental emulation of quantum non-Markovian dynamics and coherence protection in the presence of information backflow. Phys. Rev. A 2019, 99, 022107. [Google Scholar] [CrossRef] [Green Version]
- Yan, Y.A.; Yang, F.; Liu, Y.; Shao, J. Hierarchical approach based on stochastic decoupling to dissipative systems. Chem. Phys. Lett. 2004, 395, 216–221. [Google Scholar] [CrossRef]
- Bundgaard-Nielsen, M.; Mørk, J.; Denning, E.V. Non-Markovian perturbation theories for phonon effects in strong-coupling cavity quantum electrodynamics. Phys. Rev. B 2021, 103, 235309. [Google Scholar] [CrossRef]
- Ricottone, A.; Rudner, M.S.; Coish, W.A. Topological transition of a non-Markovian dissipative quantum walk. Phys. Rev. A 2020, 102, 012215. [Google Scholar] [CrossRef]
- Rivas, A.; Huelga, S.F.; Plenio, M.B. Entanglement and non-Markovianity of quantum evolutions. Phys. Rev. Lett. 2010, 105, 050403. [Google Scholar] [CrossRef] [Green Version]
- Chruściński, D.; Kossakowski, A.; Rivas, A. Measures of non-Markovianity: Divisibility versus backflow of information. Phys. Rev. A 2011, 83, 052128. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.Y.; Zhang, N.N.; He, W.T.; Kong, X.Y.; Tao, M.J.; Deng, F.G.; Ai, Q.; Long, G.L. Global correlation and local information flows in controllable non-Markovian open quantum dynamics. Npj Quantum Inform. 2022, 8, 22. [Google Scholar] [CrossRef]
- Strathearn, A.; Lovett, B.W.; Kirton, P. Efficient real-time path integrals for non-Markovian spin-boson models. New J. Phys. 2017, 19, 093009. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.M.; Ren, F.H.; Luo, D.W.; Yan, Z.Y.; Wu, L.A. Quantum state transmission through a spin chain in finite-temperature heat baths. J. Phys. A Math. Theor. 2021, 54, 155303. [Google Scholar] [CrossRef]
- Wang, Z.M.; Luo, D.W.; Byrd, M.S.; Wu, L.A.; Yu, T.; Shao, B. Adiabatic speedup in a non-Markovian quantum open system. Phys. Rev. A 2018, 98, 062118. [Google Scholar] [CrossRef]
- Laine, E.M.; Breuer, H.P.; Piilo, J. Nonlocal memory effects allow perfect teleportation with mixed states. Sci. Rep. 2014, 4, 1–5. [Google Scholar] [CrossRef] [Green Version]
- Fahmy, A.F.; Marx, R.; Bermel, W.; Glaser, S.J. Thermal equilibrium as an initial state for quantum computation by NMR. Phys. Rev. A 2008, 78, 022317. [Google Scholar] [CrossRef] [Green Version]
- Genway, S.; Ho, A.F.; Lee, D.K.K. Dynamics of thermalization in small Hubbard-model systems. Phys. Rev. Lett. 2010, 105, 260402. [Google Scholar] [CrossRef] [Green Version]
- Ponomarev, A.V.; Denisov, S.; Hänggi, P. Thermal equilibration between Two quantum systems. Phys. Rev. Lett. 2011, 106, 010405. [Google Scholar] [CrossRef] [Green Version]
- Wu, S.X.; Yu, C.S. Quantum speed limit for a mixed initial state. Phys. Rev. A 2018, 98, 042132. [Google Scholar] [CrossRef] [Green Version]
- Jing, J.; Yu, T.; Lam, C.H.; You, J.Q.; Wu, L.A. Control relaxation via dephasing: A quantum-state-diffusion study. Phys. Rev. A 2018, 97, 012104. [Google Scholar] [CrossRef] [Green Version]
- Jing, J.; Yu, T. Non-Markovian relaxation of a three-level system: Quantum trajectory approach. Phys. Rev. Lett. 2010, 105, 240403. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.M.; Ren, F.H.; Luo, D.W.; Yan, Z.Y.; Wu, L.A. Almost-exact state transfer by leakage-elimination-operator control in a non-Markovian environment. Phys. Rev. A 2020, 102, 042406. [Google Scholar] [CrossRef]
- Link, V.; Strunz, W.T.; Luoma, K. Non-Markovian quantum dynamics in a squeezed reservoir. Entropy 2022, 24, 352. [Google Scholar] [CrossRef] [PubMed]
- Diósi, L.; Gisin, N.; Strunz, W.T. Non-Markovian quantum state diffusion. Phys. Rev. A 1998, 58, 1699–1712. [Google Scholar] [CrossRef] [Green Version]
- Flannigan, S.; Damanet, F.; Daley, A.J. Many-body quantum state diffusion for non-Markovian dynamics in strongly interacting systems. Phys. Rev. Lett. 2022, 128, 063601. [Google Scholar] [CrossRef]
- Chen, Y.; You, J.Q.; Yu, T. Exact non-Markovian master equations for multiple qubit systems: Quantum-trajectory approach. Phys. Rev. A 2014, 90, 052104. [Google Scholar] [CrossRef] [Green Version]
- Xu, J.; Zhao, X.; Jing, J.; Wu, L.A.; Yu, T. Perturbation methods for the non-Markovian quantum state diffusion equation. J. Phys. A Math. Theor. 2014, 47, 435301. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Thoss, M. From coherent motion to localization: II. Dynamics of the spin-boson model with sub-Ohmic spectral density at zero temperature. Chem. Phys. 2010, 370, 78–86. [Google Scholar] [CrossRef]
- Ritschel, G.; Eisfeld, A. Analytic representations of bath correlation functions for ohmic and superohmic spectral densities using simple poles. J. Chem. Phys. 2014, 141, 3365. [Google Scholar] [CrossRef] [Green Version]
- Meier, C.; Tannor, D.J. Non-Markovian evolution of the density operator in the presence of strong laser fields. J. Chem. Phys. 1999, 111, 3365. [Google Scholar] [CrossRef]
- Nie, S.S.; Ren, F.H.; He, R.H.; Wu, J.; Wang, Z.M. Control cost and quantum speed limit time in controlled almost-exact state transmission in open systems. Phys. Rev. A 2021, 104, 052424. [Google Scholar] [CrossRef]
- Ren, F.H.; Wang, Z.M.; Wu, L.A. Accelerated adiabatic quantum search algorithm via pulse control in a non-Markovian environment. Phys. Rev. A 2020, 102, 062603. [Google Scholar] [CrossRef]
- Marchukov, O.V.; Volosniev, A.G.; Valiente, M.; Petrosyan, D.; Zinner, N. Quantum spin transistor with a Heisenberg spin chain. Nat. Commun. 2016, 7, 13070. [Google Scholar] [CrossRef] [Green Version]
- Van-Diepen, C.J.; Hsiao, T.K.; Mukhopadhyay, U.; Reichl, C.; Wegscheider, W.; Vandersypen, L.M.K. Quantum simulation of antiferromagnetic Heisenberg chain with gate-defined quantum dots. Phys. Rev. X 2021, 11, 041025. [Google Scholar] [CrossRef]
- Dyszel, P.; Haraldsen, J.T. Thermodynamics of general Heisenberg spin tetramers composed of coupled quantum dimers. Magnetochemistry 2021, 7, 29. [Google Scholar] [CrossRef]
- Mohammed, W.W.; Al-Askar, F.M.; Cesarano, C.; Botmart, T.; El-Morshedy, M. Wiener process effects on the solutions of the fractional (2 + 1)-dimensional Heisenberg ferromagnetic spin chain equation. Mathematics 2022, 10, 2043. [Google Scholar] [CrossRef]
- Lebrun, R.; Ross, A.; Bender, S.; Qaiumzadeh, A.; Baldrati, L.; Cramer, J.; Brataas, A.; Duine, R.; Kläui, M. Tunable long-distance spin transport in a crystalline antiferromagnetic iron oxide. Nature 2018, 561, 222–225. [Google Scholar] [CrossRef]
- Wadley, P.; Howells, B.; Železn‘y, J.; Andrews, C.; Hills, V.; Campion, R.P.; Novák, V.; Olejník, K.; Maccherozzi, F.; Dhesi, S.; et al. Electrical switching of an antiferromagnet. Science 2016, 351, 6273. [Google Scholar] [CrossRef] [Green Version]
- Olejník, K.; Seifert, T.; Kašpar, Z.; Novák, V.; Wadley, P.; Campion, R.P.; Baumgartner, M.; Gambardella, P.; Nemec, P.; Wunderlich, J.; et al. Terahertz electrical writing speed in an antiferromagnetic memory. Sci. Adv. 2018, 4, 3. [Google Scholar] [CrossRef] [Green Version]
- Wei, D.; Chang, Y.; Glaser, S.J.; Yang, X. Cooperative pulses for pseudo-pure state preparation. Appl. Phys. Lett. 2014, 104, 242409. [Google Scholar] [CrossRef]
- Peng, X.; Zhu, X.; Fang, X.; Feng, M.; Gao, K.; Yang, X.; Liu, M. Preparation of pseudo-pure states by line-selective pulses in nuclear magnetic resonance. Chem. Phys. Lett. 2001, 340, 5–6. [Google Scholar] [CrossRef] [Green Version]
- Cory, D.G.; Fahmy, A.F.; Havel, T.F. Ensemble quantum computing by NMR spectroscopy. Proc. Natl. Acad. Sci. USA 1997, 94, 1634–1639. [Google Scholar] [CrossRef] [Green Version]
- Warren, W.S. The usefulness of NMR quantum computing. Science 1997, 277, 1688–1690. [Google Scholar] [CrossRef]
- Wang, Z.M.; Luo, D.W.; Li, B.; Wu, L.A. Quantum energy transfer between a nonlinearly coupled bosonic bath and a fermionic chain: An exactly solvable model. Phys. Rev. A 2020, 101, 042130. [Google Scholar] [CrossRef]
- Wang, Z.M.; Ren, F.H.; Sarandy, M.S.; Byrd, M.S. Nonequilibrium quantum thermodynamics in non-Markovian adiabatic speedup. Physica A 2022, 603, 127861. [Google Scholar] [CrossRef]
- Whitney, R.S. Non-Markovian quantum thermodynamics: Laws and fluctuation theorems. Phys. Rev. B 2018, 98, 085415. [Google Scholar] [CrossRef] [Green Version]
- Thomas, G.; Siddharth, N.; Banerjee, S.; Ghosh, S. Thermodynamics of non-Markovian reservoirs and heat engines. Phys. Rev. E 2018, 97, 062108. [Google Scholar] [CrossRef] [Green Version]
- Kato, A.; Tanimura, Y. Quantum heat current under non-perturbative and non-Markovian conditions: Applications to heat machines. J. Chem. Phys. 2016, 145, 224105. [Google Scholar] [CrossRef] [Green Version]
- Feng, G.; Xu, G.; Long, G. Experimental realization of non-adiabatic holonomic quantum computation. Phys. Rev. Lett. 2013, 110, 190501. [Google Scholar] [CrossRef] [Green Version]
- Altowyan, A.S.; Berrada, K.; Abdel-Khalek, S.; Eleuch, H. Quantum coherence and total phase in semiconductor microcavities for multi-photon excitation. Nanomaterials 2022, 12, 2671. [Google Scholar] [CrossRef]
- Hu, M.L.; Hu, X.; Wang, J.; Peng, Y.; Zhang, Y.R.; Fan, H. Quantum coherence and geometric quantum discord. Phys. Rep. 2018, 762–764, 1–100. [Google Scholar] [CrossRef] [Green Version]
- Baumgratz, T.; Cramer, M.; Plenio, M.B. Quantifying Coherence. Phys. Rev. Lett. 2014, 113, 140401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yu, T. Non-Markovian quantum trajectories versus master equations: Finite-temperature heat bath. Phys. Rev. A 2004, 69, 062107. [Google Scholar] [CrossRef] [Green Version]
- Sun, Y.J.; Zhang, W.M. Modeling neuronal systems as an open quantum system. Symmetry 2021, 13, 1603. [Google Scholar] [CrossRef]
- Ng, H. Decoherence of interacting Majorana modes. Sci. Rep. 2015, 5, 12530. [Google Scholar] [CrossRef] [PubMed]
- Moriya, T. New mechanism of anisotropic superexchange interaction. Phys. Rev. Lett. 1960, 4, 228–230. [Google Scholar] [CrossRef]
- Dzyaloshinsky, I. A thermodynamic theory of “weak” ferromagnetism of antiferromagnetics. J. Phys. Chem. Solids 1958, 4, 241–255. [Google Scholar] [CrossRef]
- Heide, M.; Bihlmayer, G.; Blügel, S. Dzyaloshinskii-Moriya interaction accounting for the orientation of magnetic domains in ultrathin films: Fe/W(110). Phys. Rev. B 2008, 78, 140403. [Google Scholar] [CrossRef] [Green Version]
- Emori, S.; Bauer, U.; Ahn, S.M.; Martinez, E.; Beach, G.S. Current-driven dynamics of chiral ferromagnetic domain walls. Nat. Mater. 2013, 12, 611–616. [Google Scholar] [CrossRef] [Green Version]
- Yu, X.; Kanazawa, N.; Zhang, W.; Nagai, T.; Hara, T.; Kimoto, K.; Matsui, Y.; Onose, Y.; Tokura, Y. Skyrmion flow near room temperature in an ultralow current density. Nat. Commun. 2012, 3, 988. [Google Scholar] [CrossRef] [Green Version]
- Qaiumzadeh, A.; Ado, I.A.; Duine, R.A.; Titov, M.; Brataas, A. Theory of the interfacial Dzyaloshinskii-Moriya interaction in Rashba antiferromagnets. Phys. Rev. Lett. 2018, 120, 197202. [Google Scholar] [CrossRef] [Green Version]
- Liu, B.Q.; Shao, B.; Li, J.G.; Zou, J.; Wu, L.A. Quantum and classical correlations in the one-dimensional XY model with Dzyaloshinskii-Moriya interaction. Phys. Rev. A 2011, 83, 052112. [Google Scholar] [CrossRef] [Green Version]
- Kavokin, K.V. Anisotropic exchange interaction of localized conduction-band electrons in semiconductors. Phys. Rev. B 2001, 64, 075305. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.M.; Shao, B.; Zou, J. Anisotropy and magnetic field effects on the entanglement transfer in two parallel Heisenberg spin chains. Int. J. Mod. Phys. B 2001, 27, 4853–4861. [Google Scholar] [CrossRef]
- Abliz, A.; Gao, H.J.; Xie, X.C.; Wu, Y.S.; Liu, W.M. Entanglement control in an anisotropic two-qubit Heisenberg XYZ model with external magnetic fields. Phys. Rev. A 2006, 74, 052105. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.M.; Shao, B.; Chang, P.; Zou, J. Quantum state transfer in a Heisenberg XY chain with energy current. Physica A 2008, 387, 2197–2204. [Google Scholar] [CrossRef]
- Felcher, G.; Kleb, R.; Jaccarino, V. Observation of the spin-flop transition of MnF2 by neutron diffraction. J. Appl. Phys. 1979, 50, 1837. [Google Scholar] [CrossRef]
- Welp, U.; Berger, A.; Miller, D.J.; Vlasko-Vlasov, V.K.; Gray, K.E.; Mitchell, J.F. Direct imaging of the first-order spin-flop transition in the layered manganite La1.4Sr1.6Mn2O7. Phys. Rev. Lett. 1999, 83, 4180–4183. [Google Scholar] [CrossRef] [Green Version]
- Vega, I.D.; Alonso, D. Dynamics of non-Markovian open quantum systems. Rev. Mod. Phys. 2017, 89, 015001. [Google Scholar] [CrossRef] [Green Version]
- Pozzobom, M.B.; Maziero, J. Environment-induced quantum coherence spreading of a qubit. Ann. Phys. 2017, 377, 243–255. [Google Scholar] [CrossRef] [Green Version]
- Latune, C.L.; Sinayskiy, I.; Petruccione, F. Energetic and entropic effects of bath-induced coherences. Phys. Rev. A 2019, 99, 052105. [Google Scholar] [CrossRef] [Green Version]
- Eastham, P.R.; Kirton, P.; Cammack, H.M.; Lovett, B.W.; Keeling, J. Bath-induced coherence and the secular approximation. Phys. Rev. A 2016, 94, 012110. [Google Scholar] [CrossRef] [Green Version]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ablimit, A.; He, R.-H.; Xie, Y.-Y.; Wu, L.-A.; Wang, Z.-M. Quantum Energy Current Induced Coherence in a Spin Chain under Non-Markovian Environments. Entropy 2022, 24, 1406. https://doi.org/10.3390/e24101406
Ablimit A, He R-H, Xie Y-Y, Wu L-A, Wang Z-M. Quantum Energy Current Induced Coherence in a Spin Chain under Non-Markovian Environments. Entropy. 2022; 24(10):1406. https://doi.org/10.3390/e24101406
Chicago/Turabian StyleAblimit, Arapat, Run-Hong He, Yang-Yang Xie, Lian-Ao Wu, and Zhao-Ming Wang. 2022. "Quantum Energy Current Induced Coherence in a Spin Chain under Non-Markovian Environments" Entropy 24, no. 10: 1406. https://doi.org/10.3390/e24101406
APA StyleAblimit, A., He, R.-H., Xie, Y.-Y., Wu, L.-A., & Wang, Z.-M. (2022). Quantum Energy Current Induced Coherence in a Spin Chain under Non-Markovian Environments. Entropy, 24(10), 1406. https://doi.org/10.3390/e24101406




