Quantum Nonlocality in Any Forked Tree-Shaped Network
Abstract
:1. Introduction
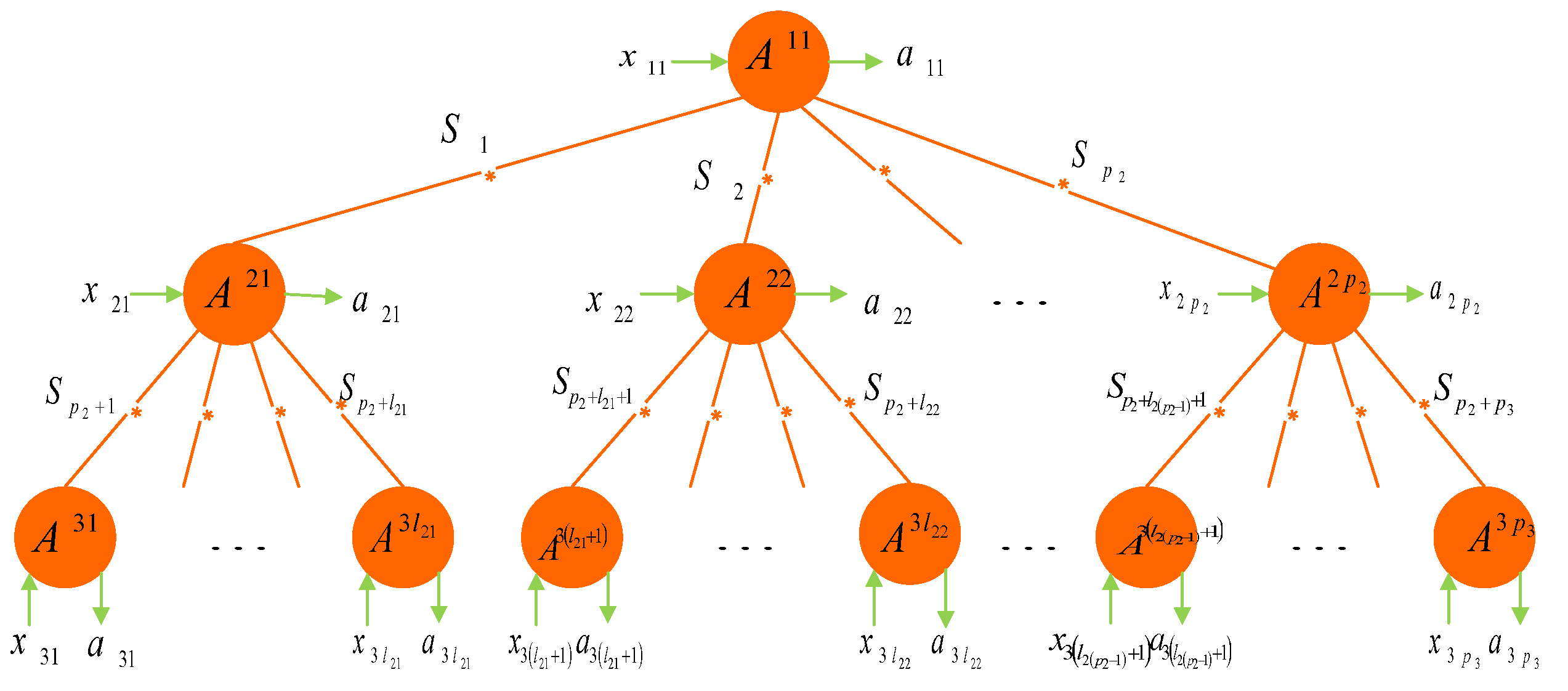
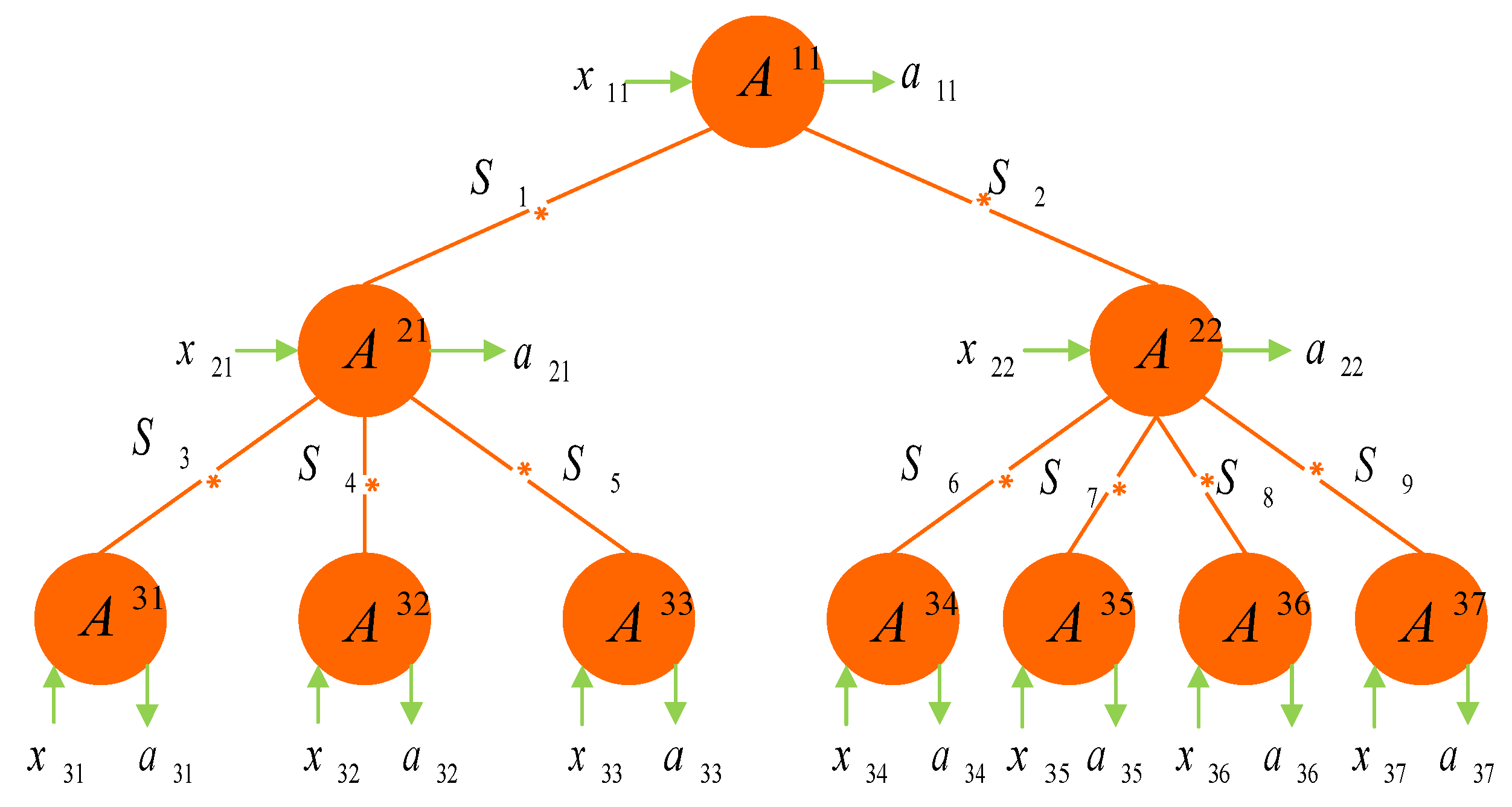
2. Nonlocality in Any Forked Tree-Shaped Network Scenario
2.1. -Local Network Scenario
2.1.1. -Locality Inequality
2.1.2. Quantum Violations of -Local Inequalities
2.1.3. Resistance to White Noise
2.2. -Local Network Scenario
2.3. Comparing Any Forked Tree-Shaped Network with Other Networks
3. Discussions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Einstein, A.; Podolsky, B.; Rosen, N. Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 1935, 47, 777. [Google Scholar] [CrossRef] [Green Version]
- Bell, J.S. On the Einstein-Podolsky-Rosen paradox. Physics 1964, 1, 195. [Google Scholar] [CrossRef] [Green Version]
- Brunner, N.; Cavalcanti, D.; Pironio, S.; Scarani, V.; Wehner, S. Bell nonlocality. Rev. Mod. Phys. 2014, 86, 419. [Google Scholar] [CrossRef] [Green Version]
- Brendel, J.; Mohler, E.; Martienssen, W. Experimental test of Bell’s inequality for energy and time. Europhys. Lett. 1992, 20, 575. [Google Scholar] [CrossRef]
- Gröblacher, S.; Paterek, T.; Kaltenbaek, R.; Brukner, Č.; Żukowski, M.; Aspelmeyer, M.; Zeilinger, A. An experimental test of non-local realism. Nature 2007, 446, 871. [Google Scholar] [CrossRef] [Green Version]
- Giustina, M.; Versteegh, M.A.M.; Wengerowsky, S.; Handsteiner, J.; Hochrainer, A.; Phelan, K.; Steinlechner, F.; Kofler, J.; Larsson, J.A.; Abellán, C.; et al. Significant-Loophole-Free test of Bell’s theorem with entangled photons. Phys. Rev. Lett. 2015, 115, 250401. [Google Scholar] [CrossRef]
- Ekert, A.K. Quantum cryptography based on Bell’s theorem. Phys. Rev. Lett. 1991, 67, 661. [Google Scholar] [CrossRef] [Green Version]
- Acín, A.; Brunner, N.; Gisin, N.; Massar, S.; Pironio, S.; Scarani, V. Device-independent security of quantum cryptography against collective attacks. Phys. Rev. Lett. 2007, 98, 230501. [Google Scholar] [CrossRef] [Green Version]
- Acín, A.; Gisin, N.; Masanes, L. From Bell’s theorem to secure quantum key distribution. Phys. Rev. Lett. 2006, 97, 120405. [Google Scholar] [CrossRef] [Green Version]
- Pironio, S.; Acín, A.; Massar, S.; Boyer de la Giroday, A.; Matsukevich, D.N.; Maunz, P.; Olmschenk, S.; Hayes, D.; Luo, L.; Manning, T.A.; et al. Random numbers certified by Bell’s theorem. Nature 2010, 464, 1021. [Google Scholar] [CrossRef] [Green Version]
- Buhrman, H.; Cleve, R.; Massar, S.; de Wolf, R. Nonlocality and communication complexity. Rev. Mod. Phys. 2010, 82, 665. [Google Scholar] [CrossRef] [Green Version]
- Clauser, J.F.; Horne, M.A.; Shimony, A.; Holt, R.A. Proposed experiment to test local hidden-variable theories. Phys. Rev. Lett. 1969, 23, 880. [Google Scholar] [CrossRef] [Green Version]
- Collins, D.; Gisin, N.; Linden, N.; Massar, S.; Popescu, S. Bell inequalities for arbitrarily high-dimensional systems. Phys. Rev. Lett. 2002, 88, 040404. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cruzeiro, E.Z.; Gisin, N. Complete list of tight Bell inequalities for two parties with four binary settings. Phys. Rev. A 2019, 99, 022104. [Google Scholar] [CrossRef] [Green Version]
- Tavakoli, A.; Pozas-Kerstjens, A.; Luo, M.-X.; Renou, M.-O. Bell nonlocality in networks. Rep. Prog. Phys. 2021, in press. [Google Scholar] [CrossRef]
- Żukowski, M.; Zeilinger, A.; Horne, M.A.; Ekert, A.K. “Event-Ready-Detectors” Bell experiment via entanglement swapping. Phys. Rev. Lett. 1993, 71, 4287. [Google Scholar] [CrossRef]
- Branciard, C.; Gisin, N.; Pironio, S. Characterizing the nonlocal correlations created via entanglement swapping. Phys. Rev. Lett. 2010, 104, 170401. [Google Scholar] [CrossRef]
- Branciard, C.; Rosset, D.; Gisin, N.; Pironio, S. Bilocal versus nonbilocal correlations in entanglement-swapping experiments. Phys. Rev. A 2012, 85, 032119. [Google Scholar] [CrossRef] [Green Version]
- Gisin, N.; Mei, Q.; Tavakoli, A.; Renou, M.O.; Brunner, N. All entangled pure quantum states violate the bilocality inequality. Phys. Rev. A 2017, 96, 020304. [Google Scholar] [CrossRef] [Green Version]
- Mukherjee, K.; Paul, B.; Sarkar, D. Correlations in n-local scenario. Quantum Inf. Process. 2015, 14, 2025. [Google Scholar] [CrossRef] [Green Version]
- Tavakoli, A.; Skrzypczyk, P.; Cavalcanti, D.; Acín, A. Nonlocal correlations in the star-network configuration. Phys. Rev. A 2014, 90, 062109. [Google Scholar] [CrossRef] [Green Version]
- Tavakoli, A.; Renou, M.O.; Gisin, N.; Brunner, N. Correlations in star networks: From Bell inequalities to network inequalities. New J. Phys. 2017, 19, 073003. [Google Scholar] [CrossRef] [Green Version]
- Andreoli, F.; Carvacho, G.; Santodonato, L.; Chaves, R.; Sciarrino, F. Maximal qubit violation of n-locality inequalities in a star-shaped quantum network. New J. Phys. 2017, 19, 113020. [Google Scholar] [CrossRef]
- Renou, M.-O.; B<i>a</i>¨umer, E.; Boreiri, S.; Brunner, N.; Gisin, N.; Beigi, S. Genuine quantum nonlocality in the triangle network. Phys. Rev. Lett. 2019, 123, 140401. [Google Scholar] [CrossRef] [Green Version]
- Luo, M.-X. Computationally efficient nonlinear Bell inequalities for quantum networks. Phys. Rev. Lett. 2018, 120, 140402. [Google Scholar] [CrossRef] [Green Version]
- Frey, M. A Bell inequality for a class of multilocal ring networks. Quantum Inf. Process 2017, 16, 266. [Google Scholar] [CrossRef]
- Fritz, T. Beyond Bell’s theorem: Correlation scenarios. New J. Phys. 2012, 14, 103001. [Google Scholar] [CrossRef]
- Tavakoli, A. Quantum correlations in connected multipartite Bell experiments. J. Phys. A Math. Theor. 2016, 49, 145304. [Google Scholar] [CrossRef] [Green Version]
- Chaves, R. Polynomial Bell inequalities. Phys. Rev. Lett. 2016, 116, 010402. [Google Scholar] [CrossRef]
- Luo, M.-X. Nonlocality of all quantum networks. Phys. Rev. A 2018, 98, 042317. [Google Scholar] [CrossRef] [Green Version]
- Mukherjee, K.; Paul, B.; Sarkar, D. Nontrilocality: Exploiting nonlocality from three-particle systems. Phys. Rev. A 2017, 96, 022103. [Google Scholar] [CrossRef] [Green Version]
- Mukherjee, K.; Paul, B.; Roy, A. Characterizing quantum correlations in a fixed-input n-local network scenario. Phys. Rev. A 2020, 101, 032328. [Google Scholar] [CrossRef] [Green Version]
- Bancal, J.-D.; Gisin, N. Nonlocal boxes for networks. Phys. Rev. A 2021, 104, 052212. [Google Scholar] [CrossRef]
- Šupić, I.; Bancal, J.-D.; Cai, Y.; Brunner, N. Genuine network quantum nonlocality and self-testing. Phys. Rev. A 2022, 105, 022206. [Google Scholar]
- Pozas-Kerstjens, A.; Gisin, N.; Tavakoli, A. Full network nonlocality. Phys. Rev. Lett. 2022, 128, 010403. [Google Scholar] [CrossRef] [PubMed]
- Shi, Y.-Y.; Duan, L.-M.; Vidal, G. Classical simulation of quantum many-body systems with a tree tensor network. Phys. Rev. A 2006, 74, 022320. [Google Scholar] [CrossRef] [Green Version]
- Tagliacozzo, L.; Evenbly, G.; Vidal, G. Simulation of two-dimensional quantum systems using a tree tensor network that exploits the entropic area law. Phys. Rev. B 2009, 80, 235127. [Google Scholar] [CrossRef] [Green Version]
- Murg, V.; Verstraete, F.; Legeza, Ö.; Noack, R.M. Simulating strongly correlated quantum systems with tree tensor networks. Phys. Rev. B 2010, 82, 205105. [Google Scholar] [CrossRef] [Green Version]
- Dumitrescu, E. Tree tensor network approach to simulating Shor’s algorithm. Phys. Rev. A 2017, 96, 062322. [Google Scholar] [CrossRef] [Green Version]
- Lopez-Piqueres, J.; Ware, B.; Vasseur, R. Mean-field entanglement transitions in random tree tensor networks. Phys. Rev. B 2020, 102, 064202. [Google Scholar] [CrossRef]
- Wall, M.L.; D’Aguanno, G. Tree-tensor-network classifiers for machine learning: From quantum inspired to quantum assisted. Phys. Rev. A 2021, 104, 042408. [Google Scholar] [CrossRef]
- Yang, L.-H.; Qi, X.-F.; Hou, J.-C. Nonlocal correlations in the tree-tensor-network configuration. Phys. Rev. A 2021, 104, 042405. [Google Scholar] [CrossRef]
- Horodecki, R.; Horodecki, P.; Horodecki, M. Violating Bell inequality by mixed spin-12 states: Necessary and sufficient condition. Phys. Lett. A 1995, 200, 340. [Google Scholar] [CrossRef]

| Networks | Multi-Local Inequalities | Relations |
|---|---|---|
| any forked tree-shaped | ||
| bilocal | n = 2, = 2 | |
| chain-shaped | ||
| star-shaped | n = 2 | |
| two-forked tree-shaped | = 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, L.; Qi, X.; Hou, J. Quantum Nonlocality in Any Forked Tree-Shaped Network. Entropy 2022, 24, 691. https://doi.org/10.3390/e24050691
Yang L, Qi X, Hou J. Quantum Nonlocality in Any Forked Tree-Shaped Network. Entropy. 2022; 24(5):691. https://doi.org/10.3390/e24050691
Chicago/Turabian StyleYang, Lihua, Xiaofei Qi, and Jinchuan Hou. 2022. "Quantum Nonlocality in Any Forked Tree-Shaped Network" Entropy 24, no. 5: 691. https://doi.org/10.3390/e24050691
APA StyleYang, L., Qi, X., & Hou, J. (2022). Quantum Nonlocality in Any Forked Tree-Shaped Network. Entropy, 24(5), 691. https://doi.org/10.3390/e24050691






